Non-Standard Analysis for Regularization of Geometric-Zeno Behaviour in Hybrid Systems
Abstract
1. Introduction
2. Non-Standard Hybrid Automata
- , , is a set of discrete states,
- is the non-standard continuous state space,
- is a set of non-standard initial states,
- : is a set of non-standard vector fields,
- : is a set of non-standard continuous invariants,
- , , is a set of discrete transitions,
- : is a set of non-standard guard conditions,
- : is a set of non-standard reset maps.
- 1.
- and when ,
- 2.
- for all such that : , , , ,
- 3.
- for all such that : , , , and ,
3. Geometric-Zeno: Case Study
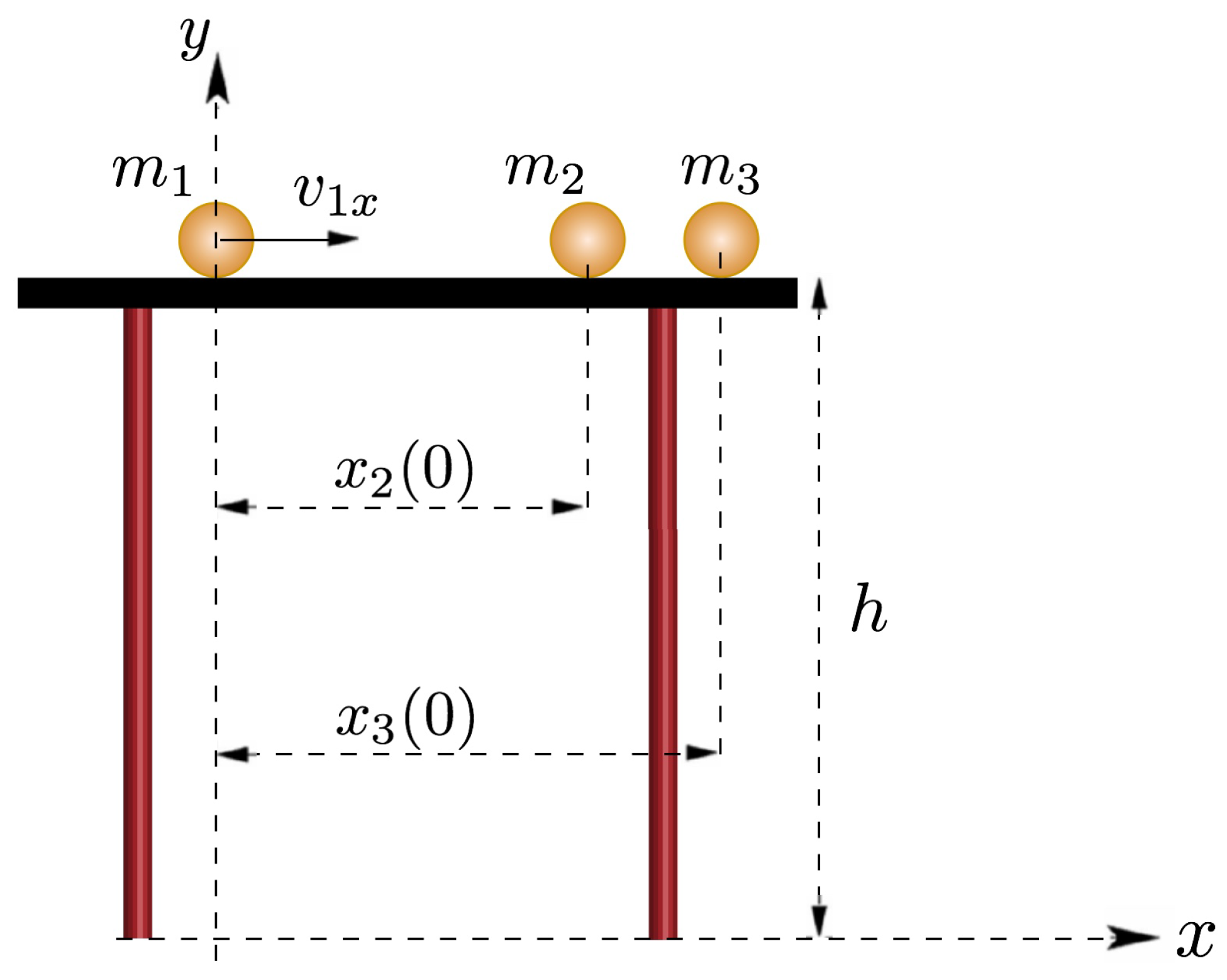
Example (Collision of Three Masses)
4. Non-Standard Regularization of Geometric-Zeno
- 1.
- The directed graph of contains a finite cyclic path.
- 2.
- For every non-standard hybrid state there exists a non-standard hybrid state such that with ρ being a geometric contractive simulation relation.
Example (Collision of Three Masses) Revisited
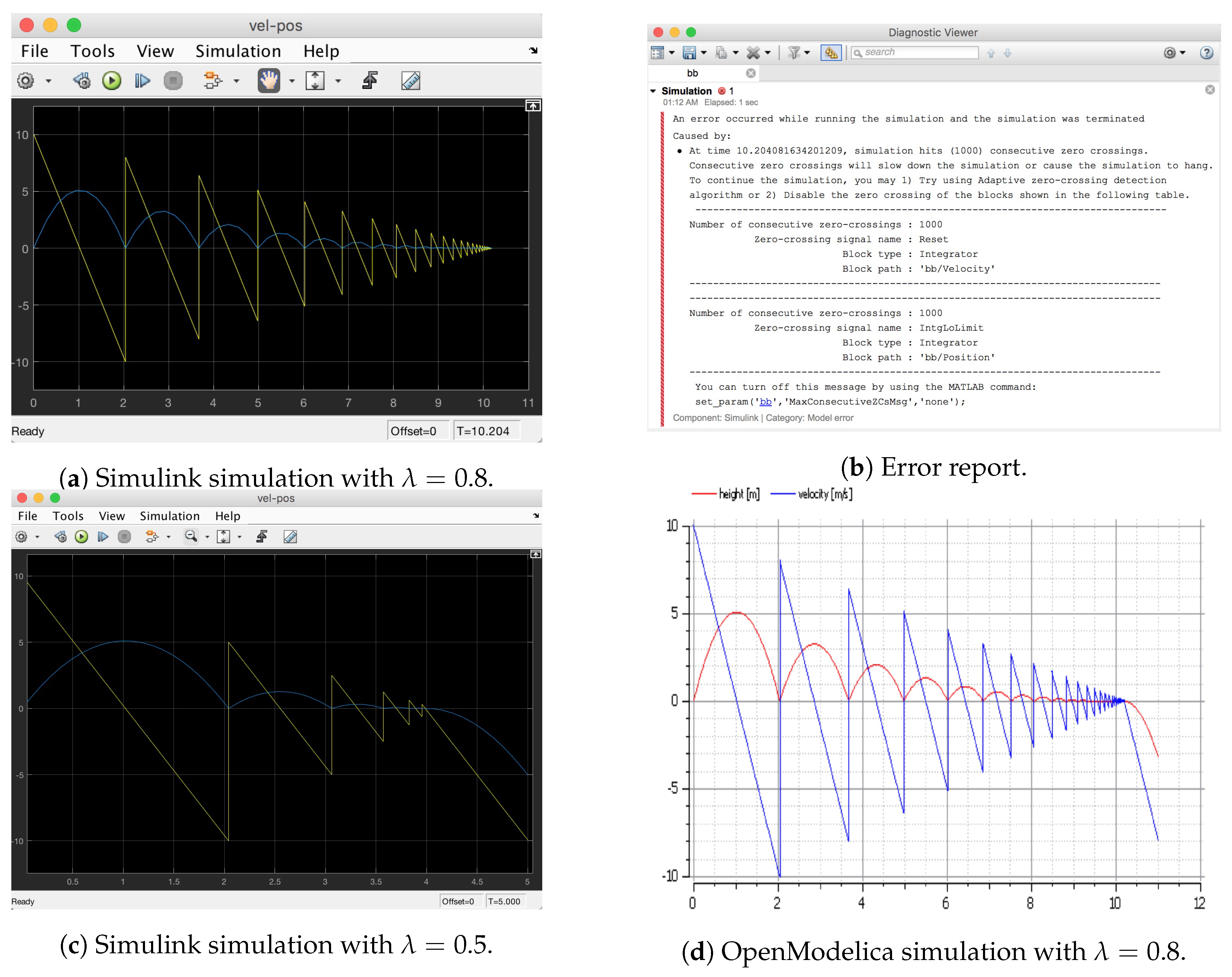
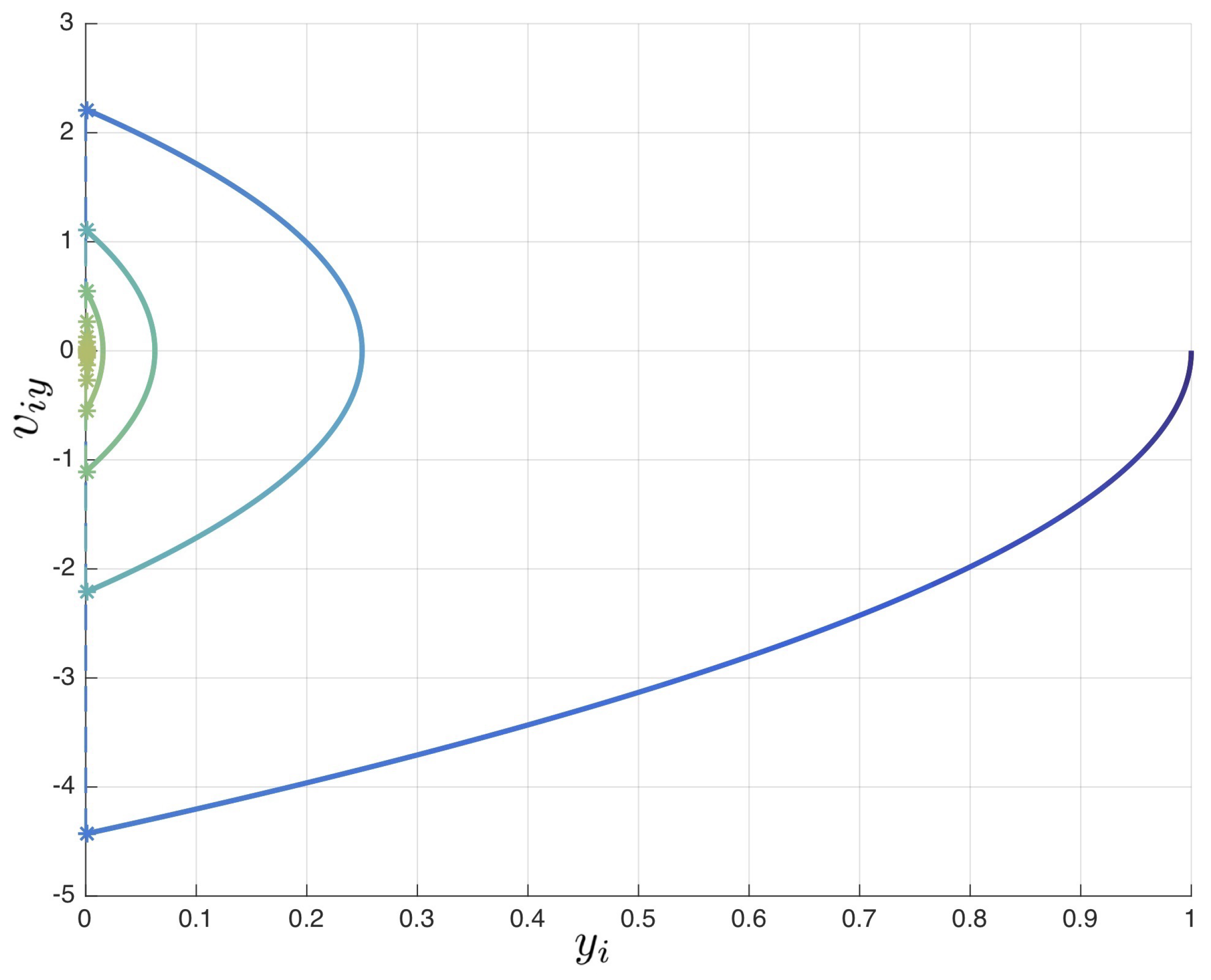
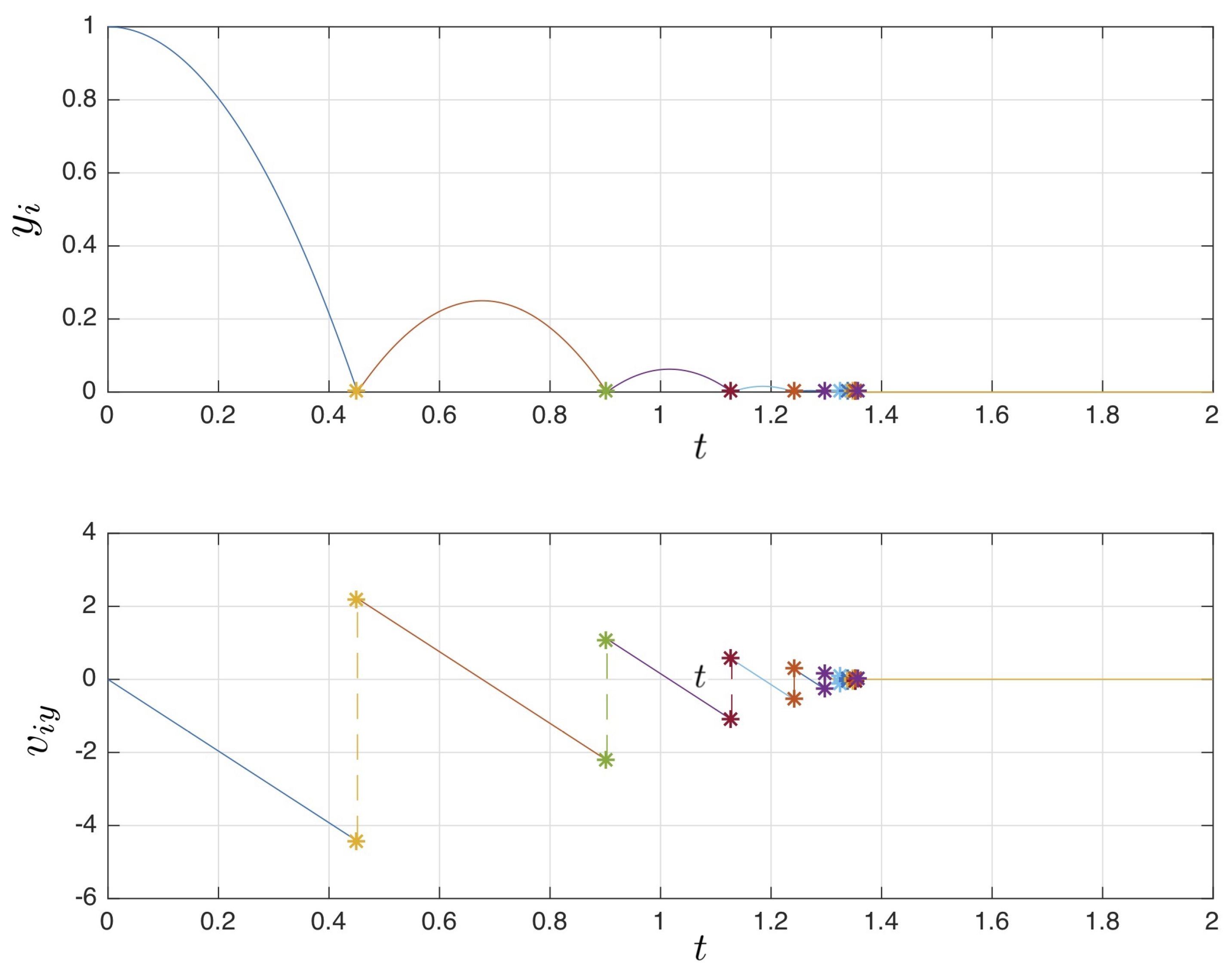
5. Simulation Results
6. Conclusions and Perspectives
Author Contributions
Funding
Conflicts of Interest
References
- Campbell, S.; Chancelier, J.P.; Nikoukhah, R. Modeling and Simulation in Scilab/Scicos with ScicosLab 4.4, 2nd ed.; Springer: New York, NY, USA, 2010; ISBN 978-1-4419-5526-5. [Google Scholar] [CrossRef]
- Lunze, J.; Lamnabhi-Lagarrigue, F. Handbook of Hybrid Systems Control: Theory, Tools, Applications; Cambridge University Press: Cambridge, UK, 2011. [Google Scholar] [CrossRef]
- Lee, E.A.; Seshia, S.A. Introduction to Embedded Systems, A Cyber-Physical Systems Approach, 2nd ed.; MIT Press: Cambridge, MA, USA, 2017; ISBN 978-0-262-53381-2. [Google Scholar]
- Betsuno, K.; Matsumoto, S.; Ueda, K. Symbolic Analysis of Hybrid Systems Involving Numerous Discrete Changes Using Loop Detection. In Cyber Physical Systems. Design, Modeling, and Evaluation; Lecture Notes in Computer Science; Berger, C., Mousavi, M., Wisniewski, R., Eds.; Springer: Cham, Switzerland, 2017; Volume 10107. [Google Scholar] [CrossRef]
- Antsaklis, P.J. Special issue on hybrid systems: Theory and applications a brief introduction to the theory and applications of hybrid systems. Proc. IEEE 2000, 88, 879–887. [Google Scholar] [CrossRef]
- Aljarbouh, A.; Caillaudr, B. Chattering-free simulation of hybrid dynamical systems with the functional mock-up interface 2.0. In Proceedings of the First Japanese Modelica Conferences, Linkoping Electronic Conference Proceedings, Tokyo, Japan, 23–24 May 2016; Volume 124, pp. 95–105. [Google Scholar] [CrossRef]
- Aljarbouh, A.; Zeng, Y.; Duracz, A.; Caillaud, B.; Taha, W. Chattering-free simulation for hybrid dynamical systems semantics and prototype implementation. In Proceedings of the 19th IEEE International Conference on Computational Science and Engineering (CSE) and IEEE International Conference on Embedded and Ubiquitous Computing (EUC) and 15th International Symposium on Distributed Computing and Applications for Business Engineering (DCABES), Paris, France, 24–26 August 2016; pp. 412–422. [Google Scholar] [CrossRef]
- Aljarbouh, A.; Caillaud, B. On the Regularization of Chattering Executions in Real Time Simulation of Hybrid Systems. In Baltic Young Scientists Conference, The 11th Baltic Young Scientists Conference; Cap, C., Ed.; Universität Rostock: Tallinn, Estonia, 2015; p. 49. Available online: https://hal.archives-ouvertes.fr/hal-01246853 (accessed on 14 January 2020).
- Lygeros, J.; Tomlin, C.; Sastry, S. Hybrid Systems: Modeling, Analysis and Control, Lecture Notes on Hybrid Systems. Available online: http://www-inst.cs.berkeley.edu/~ee291e/sp09/handouts/book.pdf (accessed on 16 January 2020).
- Raskin, J.-F. Reachability Problems for Hybrid Automata; Springer: Berlin/Heidelberg, Germany, 2011; pp. 28–30. [Google Scholar]
- Henzinger, T.A. The theory of hybrid automata. In Proceedings of the 11th Annual IEEE Symposium on Logic in Computer Science, New Brunswick, NJ, USA, 27–30 July 1996; pp. 278–292. [Google Scholar] [CrossRef]
- Brihaye, T.; Doyen, L.; Geeraerts, G.; Ouaknine, J.; Raskin, J.F.; Worrell, J. On Reachability for Hybrid Automata over Bounded Time. In Automata, Languages and Programming. ICALP 2011, Zurich, Switzerland, July 4–8, 2011; Lecture Notes in Computer Science; Aceto, L., Henzinger, M., Sgall, J., Eds.; Springer: Berlin/Heidelberg, Germany, 2011; Volume 6756. [Google Scholar]
- Frehse, G.; Le Guernic, C.; Donze, A.; Cotton, S.; Ray, R.; Lebeltel, O.; Ripado, R.; Girard, A.; Dang, T.; Maler, O. Spaceex: Scalable verification of hybrid systems. In Computer Aided Verification; Springer: Berlin/Heidelberg, Germany, 2011; pp. 379–395. [Google Scholar]
- Aljarbouh, A.; Caillaudr, B. Simulation for hybrid systems: Chattering path avoidance. In Proceedings of the 56th Conference on Simulation and Modelling (SIMS 56), Linkoping Electronic Conference Proceedings, Linkoping, Sweden, 7–9 October 2015; Volume 119, pp. 175–185. [Google Scholar] [CrossRef]
- Goebel, R.; Teel, A.R. Lyapunov characterization of zeno behavior in hybrid systems. In Proceedings of the 47th IEEE Conference on Decision and Control (CDC 2008), Cancun, Mexico, 9–11 December 2008; pp. 2752–2757. [Google Scholar] [CrossRef]
- Lamperski, A.; Ames, A.D. Lyapunov theory for zeno stability. IEEE Trans. Autom. Control 2013, 58, 100–112. [Google Scholar] [CrossRef]
- Egerstedt, M.; Johansson, K.H.; Sastry, S.; Lygeros, J. On the regularization of Zeno hybrid automata. Syst. Control Lett. 1999, 38, 141–150. [Google Scholar]
- Johansson, K.H.; Lygeros, J.; Sastry, S.; Egerstedt, M. Simulation of zeno hybrid automata. In Proceedings of the 38th IEEE Conference on Decision and Control, Phoenix, AZ, USA, 7–10 December 1999; Volume 4, pp. 3538–3543. [Google Scholar] [CrossRef]
- Ames, A.D.; Sastry, S. Blowing up affine hybrid systems. In Proceedings of the 43rd IEEE Conference on Decision and Control (CDC), Atlantis, Bahamas, 14–17 December 2004; Volume 1, pp. 473–478. [Google Scholar] [CrossRef]
- Camlibel, M.K.; Schumacher, J.M. On the zeno behavior of linear complementarity systems. In Proceedings of the 40th IEEE Conference on Decision and Control, Orlando, FL, USA, 4–7 December 2001; Volume 124, pp. 346–351. [Google Scholar] [CrossRef]
- Shen, J.; Pang, J.S. Linear complementarity systems: Zeno states. SIAM J. Control Opt. 2005, 44, 1040–1066. [Google Scholar] [CrossRef]
- Thuan, L.Q. Non-zenoness of piecewise affine dynamical systems and affine complementarity systems with inputs. Control Theory Technol. 2014, 12, 35–47. [Google Scholar] [CrossRef]
- Ames, A.D.; Zheng, H.; Gregg, R.D.; Sastry, S. Is there life after zeno? Taking executions past the breaking (zeno) point. In Proceedings of the 2006 American Control Conference, Minneapolis, MN, USA, 14–16 June 2006; p. 6. [Google Scholar] [CrossRef]
- Lamperski, A.; Ames, A.D. Lyapunov-like conditions for the existence of zeno behavior in hybrid and lagrangian hybrid systems. In Proceedings of the 2007 46th IEEE Conference on Decision and Control, New Orleans, LA, USA, 12–14 December 2007; pp. 115–120. [Google Scholar] [CrossRef][Green Version]
- Ames, A.D.; Abate, A.; Sastry, S. Sufficient conditions for the existence of zeno behavior. In Proceedings of the 44th IEEE Conference on Decision and Control, Seville, Spain, 12–15 December 2005; pp. 696–701. [Google Scholar] [CrossRef]
- Lamperski, A.; Ames, A.D. On the existence of zeno behavior in hybrid systems with non-isolated zeno equilibria. In Proceedings of the 47th IEEE Conference on Decision and Control (CDC 2008), Cancun, Mexico, 9–11 December 2008; pp. 2776–2781. [Google Scholar] [CrossRef][Green Version]
- Aljarbouh, A. Non-standard zeno-free simulation semantics for hybrid dynamical systems. In Verification and Evaluation of Computer and Communication Systems; Ganty, P., Kaâniche, M., Eds.; Springer International Publishing: Cham, Switzerland, 2019; pp. 16–31. [Google Scholar]
- Benveniste, A.; Bourke, T.; Caillaud, B.; Pouzet, M. Non-standard semantics of hybrid systems modelers. J. Comput. Syst. Sci. 2012, 78, 877–910. [Google Scholar] [CrossRef]
- Saghe, A. A new approach to nonstandard analysis. Sahand Commun. Math. Anal. 2018, 12. [Google Scholar] [CrossRef]
- Chen, L. Do simple infinitesimal parts solve Zeno’s paradox of measure? Synthese 2019. [Google Scholar] [CrossRef]
- Available online: https://www.mathworks.com/products/simulink.html (accessed on 11 December 2019).
- Available online: https://openmodelica.org/ (accessed on 11 December 2019).
- Available online: https://ptolemy.berkeley.edu/hyvisual/ (accessed on 13 December 2019).
- Available online: http://www.scicos.org/ (accessed on 4 January 2020).
- Available online: http://www.acumen-language.org/ (accessed on 11 January 2020).
- Available online: http://zelus.di.ens.fr/ (accessed on 6 January 2020).
- Sevama: A Simulink Toolbox and Simulator for Zeno-Free Simulation of Hybrid Dynamical Systems, with Zeno Detection and Avoidance in Run-Time. Available online: https://bil.inria.fr/fr/software/view/2679/tab (accessed on 23 January 2020).
- Domna: A Lite Matlab Simulator for Zeno-Free Simulation of Hybrid Dynamical Systems, with Zeno Detection and Avoidance in Run-Time. Available online: https://bil.inria.fr/fr/software/view/2691/tab (accessed on 23 January 2020).



| Transition | Guard Condition | Reset |
|---|---|---|
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aljarbouh, A.; Fayaz, M.; Qureshi, M.S. Non-Standard Analysis for Regularization of Geometric-Zeno Behaviour in Hybrid Systems. Systems 2020, 8, 15. https://doi.org/10.3390/systems8020015
Aljarbouh A, Fayaz M, Qureshi MS. Non-Standard Analysis for Regularization of Geometric-Zeno Behaviour in Hybrid Systems. Systems. 2020; 8(2):15. https://doi.org/10.3390/systems8020015
Chicago/Turabian StyleAljarbouh, Ayman, Muhammad Fayaz, and Muhammad Shuaib Qureshi. 2020. "Non-Standard Analysis for Regularization of Geometric-Zeno Behaviour in Hybrid Systems" Systems 8, no. 2: 15. https://doi.org/10.3390/systems8020015
APA StyleAljarbouh, A., Fayaz, M., & Qureshi, M. S. (2020). Non-Standard Analysis for Regularization of Geometric-Zeno Behaviour in Hybrid Systems. Systems, 8(2), 15. https://doi.org/10.3390/systems8020015





