1. Introduction
From the point of view of the chemical composition of lignocellulosic biomasses, the most abundant biopolymers in plants are those that make up the cell wall, such as cellulose, hemicellulose and lignin. The cellulose content in the softwood biomass is approximately 43–47%, the hemicelluloses content 25–27%, while the lignin content is close to 27–28%. The higher content of cellulose (47–49%) and hemicelluloses (28–39%) with the reduced content of lignin 8–11.5% is related to herbaceous biomass (birdseed), while the reduced content of cellulose (36–43%) and lignin (17–24%) with the highest hemicellulose content compared to softwood biomass is typical of willow biomass and agricultural residues [
1].
Xylan comprises up to 10 and 30% of all hemicelluloses found in soft and hard woods, respectively [
1]. Lignin is a highly substituted mononuclear aromatic polymer and is often found to be attached to adjacent cellulose fibers to form a lignocellulosic complex. The lignin content of various biomasses, including both softwood and hardwood, generally range from 10 to 40% of the total dry mass [
2]. The physicochemical properties of lignin largely depend on the plant species and isolation processes [
3].
Table 1 lists the approximate composition percentages of some biomass species.
Lignin is characterized by its high heating power, which can be used to produce thermal and/or electrical energy. The calorific value of the same type of wood varies according to the volume of moisture content [
6]. As a comparison,
Table 2 lists the lower heating value (LHV) of fuels commonly used in boilers.
If the moisture content in the biomass is high, direct combustion is somewhat unfavorable because the moisture content is too high for stable combustion [
2].
Therefore, in many cases it is considered important that the moisture content of the wood is reduced to be used as fuel, since it leads to a loss of heat in the flue gases in the form of water vapor because it absorbs energy in combustion [
11].
Table 3 lists some typical moisture percentages for some types of wood.
The combustion process that occurs in the boilers needs an amount of air in excess with respect to the stoichiometric balance, because in practice, to reach a complete oxidation, a greater quantity than the theoretical is needed, in
Table 4, where the percentages of air excess are listed for some fuels.
The chemical reaction of combustion from boilers, furnaces, etc. generates a large amount of combustion gases [
14] and water in the form of steam, which are then discharged into the environment; however, it could be condensed, it would be reused for some useful and economic purposes.
In fact, water is a fundamental, integral resource for human well-being, the environment, ecological and social activities, transportation and industrial development including energy production [
15] being the fluid mostly used for heating processes, due to its availability and high heat capacity. A large amount of water is used in the industrial sector around the world, where much of it is wasted in the process or is released to the environment as a waste stream [
16]. In recent decades, climate change and human socio-economic development have enormously changed global hydrological cycles, threatening human water security, the health of aquatic environments and the biodiversity of rivers [
17,
18] with water scarcity being a widespread problem in many parts of the world.
For this reason, the amount of energy and water that is lost represents a quantity of great importance that could be recovered to reduce the amounts of primary fuel or be used in other processes. The exhaust energy from the boiler can generally be used to heat air and water from the heating network [
19]. Instead, the condensed water from the exhaust gases could have different uses depending on its chemical composition.
Generally, for fossil fuels, up to 8% of the volume fraction of the combustion products is water vapor. If the temperature of the flue gases is reduced below the dew point, the water vapor in the flue gases will condense and both the sensible heat and the latent heat thus released can be recovered [
20].
In a coal-fired boiler, the thermal energy of the exhaust gases represents approximately 50–80% of the thermal loss and 4–8% of the heat content of the fuel is ultimately converted into thermal energy carried by the exhaust gases combustion [
19]. If the remaining heat can be recovered, it would significantly improve the thermal efficiency of the boiler. This has led to the development of numerous waste heat recovery strategies [
21,
22,
23,
24].
Recovering energy from exhaust gases can also help reduce CO
2 emissions by approximately 100 million tons/yeargenerating huge economic and social benefits [
19].
For the purposes of this discussion, in order to carry out a comparative analysis to produce the same amount of energy, the fuels normally used in the energy sector have been considered in the form of combustion reactions, heating value, excess air, humidity for biomass determining the real amount of fuel and air necessary for the combustion reaction; and as products, the amount of water and carbon dioxide. It is essential that a serious and concrete effort is made to conserve this water and use it in other processes.
3. Results and Discussion
3.1. Calculation of the Raw Material Required for Combustion
The mass of raw material was calculated based on the heating value of each fuel, setting an energy value to reach 1000 MJ.
Table 5 shows the results for biomass which requires a greater quantity of material, influenced by the presence of humidity.
Moreover, moisture reduces the energy content per unit weight, the combustion efficiency and implies higher transportation costs of biomass [
26]; consequently, humidity during combustion produces more steam. In fact, in a wooden biomass such as chips or briquettes, where the composition is more complex and the humidity is higher, there will be more water.
3.2. Stoichiometric Balance of Combustion Reaction
It was established that, through the computational tool of the reaction balancer developed for this work, we could simulate a combustion system mainly (but not only) focusing on the prediction of the amount of water produced. By changing the formula, it automatically balances the reaction, and as output, it calculates the quantity of water and carbon dioxide produced and the theoretical oxygen required for combustion.
3.3. Determination of the Amount of Water Produced Depending on the Composition of the Biomass
Table 6 shows the parameters obtained after entering the bibliographic values of LHV and the mean values of the essential components of biomass: cellulose (C
6H
10O
5), lignin (as average of its three monomers: C
9H
10O
2-C
10H
12O
3-C
11H
14O
4) and hemicellulose, since these are the highly variable percentages that are considered average values for biomass of woody origin, as reported in the table, to produce the same quantity of 1000 MJ.
With the mass of each component for these biomasses, the combustion reactions of their main components are therefore valid: oxidation reactions for cellulose, for hemicellulose, and for lignin, were calculated accordingly.
3.4. Calculation of the Heating Value of Synthetic Wood
By knowing the composition of the biomass, it is possible to determine the heating value of the biomass as a weighted average of its components; the lower heating value of this biomass depends on the type of plant of origin, the greater part is strongly influenced by the water content (biomass species might have a different moisture content).
Table 7 shows The LHV values, obtained as a weighted average of its components, deviate from the values given by the literature with an average error of 4%, since they are influenced by the humidity chosen within the humidity ranges reported in the literature for wood, briquettes and chips. As mentioned, moisture reduces the energy content per unit weight [
26], which consequently leads to the formation of more water vapor than the hydrocarbons normally used for this purpose with the same dissipated energy.
Considering the percentages of mass of the main components of the wood, it is possible to obtain a quantity of water equal to mH2O = 0.70 kg from 1 kg of dry wood.
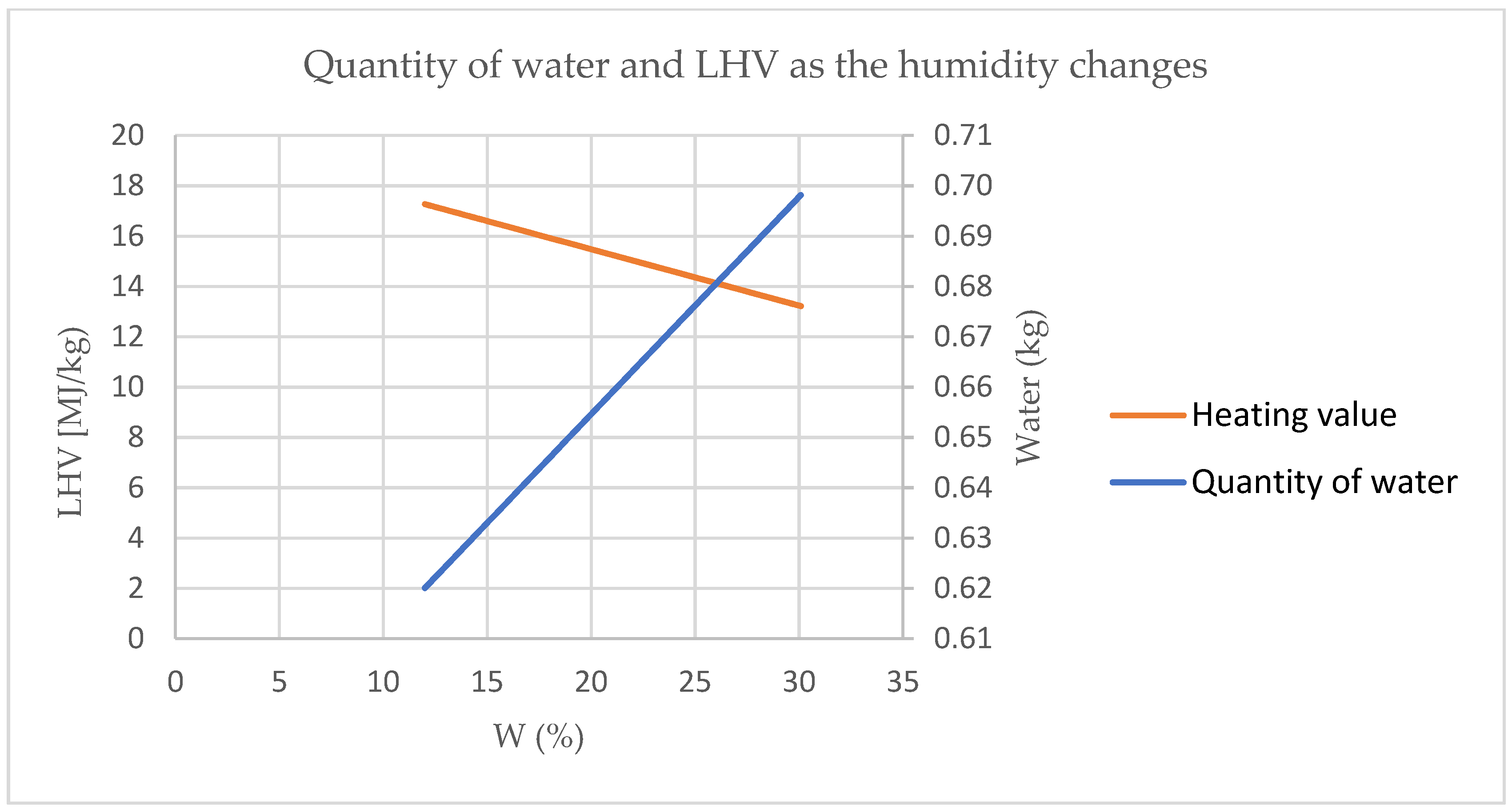
Figure 1 shows that the orange curve represents the variation of the heating value calculated according to the water content with the same mass of 1 kg and an indication of the values corresponding to the biomass normally used in the combustion heat generators and the blue one represents the quantity of water that could be recovered when the water content varies depending on the type of biomass.
The graph shows how humidity negatively affects the heating value since a certain amount of energy must be spent on the vaporization of the absorbed water [
27,
28], while the amount of water that could be recovered increases.
The water produced represents a quantity of matter and energy that can be recovered.
Table 8 evidences the recoverable thermal power of only water as a product of the combustion reaction. Only the recoverable latent power is considered, this being the most significant that can be recovered, though fumes have not been considered, and consequently the temperature at which they are found and the dew point are not taken into account; therefore, considering the enthalpy of the vaporization of water vapor at 373 K, hfg = 2256.92 kJ/kg [
25].
It is imperative that a serious and concrete effort should be launched for conserving this energy through waste heat recovery techniques. Such a waste heat recovery would ultimately reduce the overall energy requirement and the impact on global warming. Waste heat is generated in a process by the way of fuel combustion or chemical reaction, and then dumped into the environment even though it could still be reused for some useful and economic purpose. A large quantity of hot flue gases is generated from boilers, furnaces and IC engines. If some of this waste heat could be recovered, a considerable amount of primary fuel could be saved. However, much of the heat could be recovered and losses be minimized by adopting certain measures. Depending on the temperature level of the exhaust stream and the proposed application, different heat exchange devices, heat pipes and combustion equipment can be employed to facilitate the use of the recovered heat.
4. Conclusions
By simple assumptions, it is possible to calculate to the lower heating value for different biomasses of woody origin considering a weighted average of their major components and moisture.
In the case of woody biomass, the increase in the amount of condensable water proves to be interesting: as can be seen, it is greater than the amount of steam obtained in the case of methane (that is the hydrocarbon having the greatest H/C ratio). This is fundamentally due to the lower heating value which, with the same dissipated energy, requires a greater quantity of material but the presence of humidity in the starting biomass, which increases the quantity of water in the fumes. This obviously leads to the formation of more water vapor than the hydrocarbons normally used for this purpose with the same dissipated energy. From this study, it is therefore evident that the introduction of a technology capable of recovering the water produced by biomass boilers would be desirable and is still not exploited.
Moreover, the water recovered from biomass combustion could be used to clean fumes or, after purification, be employed in watering.
This study verified with sufficient approximation that the recovery of water is amenable in biomass boilers, constituting this work as a good starting point for a future technology.
Obviously, the air-to-fuel ratio should be taken into account since this could negatively affect the recovery of water when this value is high such as in biomass combustion. In order to have a better model of the real energy and water recovery from a biomass boiler, we are conducting experiments at the laboratory scale. The considerations presented here were of great importance for the design of the experiment.