Scale-Resolving Simulation of Shock-Induced Aerobreakup of Water Droplet
Abstract
1. Introduction
2. Physical Model
3. Turbulence Modeling
3.1. Unsteady RANS Approach
3.2. Detached-Eddy Simulation
3.3. Stress-Blended Eddy Simulation
4. Computational Model
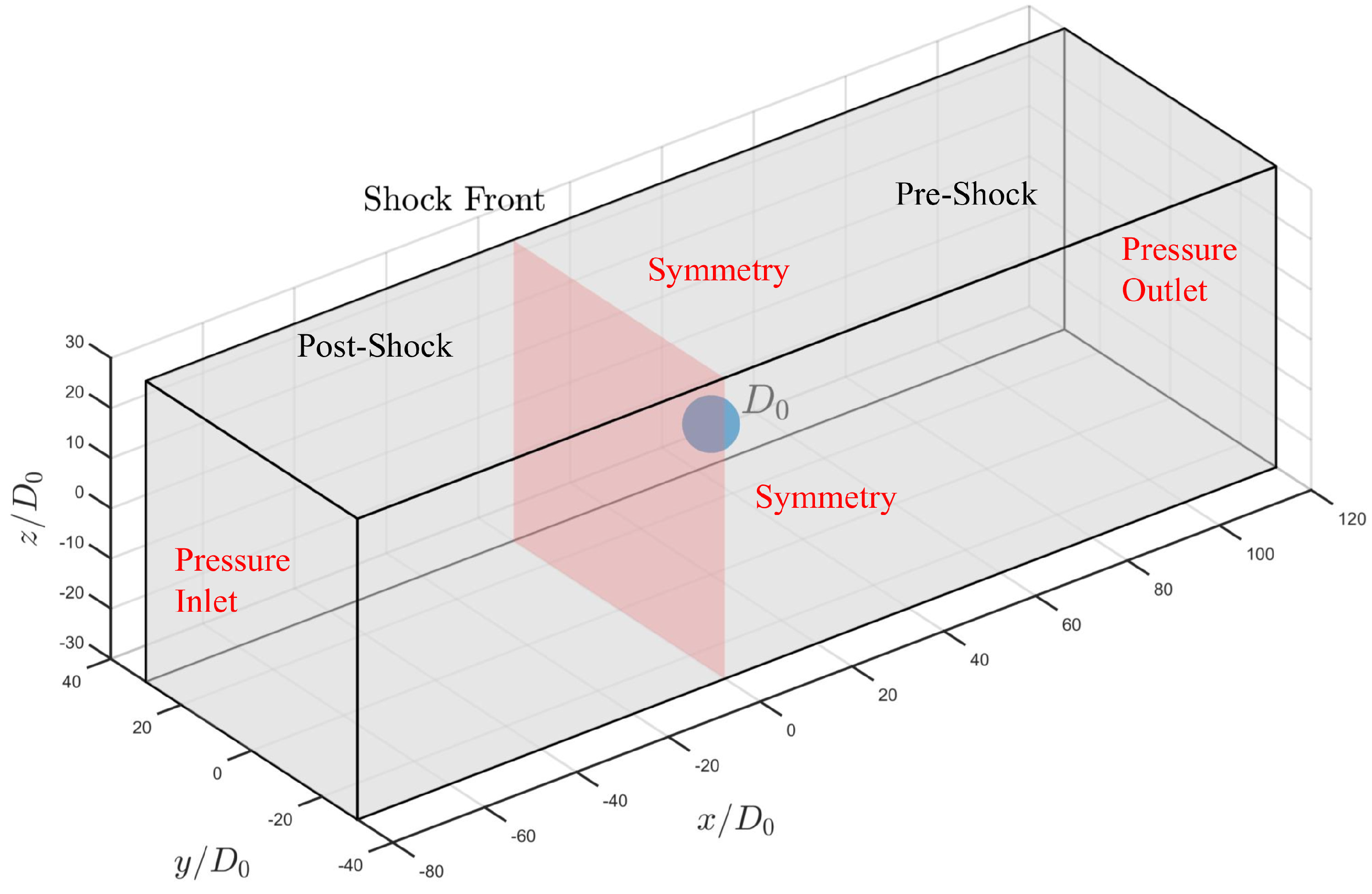
4.1. Flow Geometry
4.2. Two-Phase Flow Model
4.3. Numerical Settings
5. Results and Discussion
5.1. Droplet Movement and Deformation
5.2. Droplet Breakup Dynamics
5.3. Turbulence Resolution
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| AMR | adaptive mesh refinement |
| CFL | Courant–Friedrichs–Lewy (number) |
| CM | center-of-mass |
| CSF | continuum surface force (model) |
| DES | detached-eddy simulation |
| DNS | direct numerical simulation |
| FV | finite volume (method) |
| HPC | high-performance computing |
| KH | Kelvin–Helmholtz (instability) |
| LES | large-eddy simulation |
| RANS | Reynolds-averaged Navier–Stokes (equations) |
| RTP | Rayleigh–Taylor piercing |
| SBES | stress-blended eddy simulation |
| SGS | subgrid-scale (model) |
| SIE | shear-induced entrainment |
| SRS | scale-resolving simulation |
| SST | shear-stress transport (model) |
| VOF | volume-of-fluid (method) |
| WALE | wall-adapting local eddy-viscosity (model) |
References
- Theofanous, T.G.; Li, G. On the physics of aerobreakup. Phys. Fluids 2008, 20, 052103. [Google Scholar] [CrossRef]
- Guildenbecher, D.R.; López-Rivera, C.; Sojka, P.E. Secondary atomization. Exp. Fluids 2009, 46, 371–402. [Google Scholar] [CrossRef]
- Ranger, A.A.; Nicholls, J.A. Aerodynamic shattering of liquid drops. In Proceedings of the 6th AIAA Aerospace Sciences Meeting, New York, NY, USA, 22–24 January 1968. [Google Scholar]
- Moylan, B.; Landrum, B.; Russell, G. Investigation of the physical phenomena associated with rain impacts on supersonic and hypersonic flight vehicles. Procedia Eng. 2013, 58, 223–231. [Google Scholar] [CrossRef]
- Wang, Z.; Hopfes, T.; Giglmaier, M.; Adams, N.A. Effect of Mach number on droplet aerobreakup in shear stripping regime. Exp. Fluids 2020, 61, 193. [Google Scholar] [CrossRef] [PubMed]
- Poplavski, S.; Minakov, A.; Shebeleva, A.; Boiko, V. On the interaction of water droplet with a shock wave: Experiment and numerical simulation. Int. J. Multiph. Flow 2020, 127, 103273. [Google Scholar] [CrossRef]
- Engel, O.G. Fragmentation of water drops in the zone behind an air shock. J. Res. Nat. Bur. Stand. 1958, 60, 245–280. [Google Scholar] [CrossRef]
- Hinze, J.O. Fundamentals of the hydrodynamic mechanism of splitting in dispersion processes. AIChE J. 1955, 1, 289–295. [Google Scholar] [CrossRef]
- Theofanous, T.G. Aerobreakup of Newtonian and viscoelastic liquids. Annu. Rev. Fluid Mech. 2011, 43, 661–690. [Google Scholar] [CrossRef]
- Zhu, W.; Zhao, N.; Jia, X.; Chen, X.; Zheng, H. Effect of airflow pressure on the droplet breakup in the shear breakup regime. Phys. Fluids 2021, 33, 053309. [Google Scholar] [CrossRef]
- Meng, J.C.; Colonius, T. Numerical simulation of the aerobreakup of a water droplet. J. Fluid Mech. 2018, 835, 1108–1135. [Google Scholar] [CrossRef]
- Rossano, V.; Cittadini, A.; De Stefano, G. Computational evaluation of shock wave interaction with a liquid droplet. Appl. Sci. 2022, 12, 1349. [Google Scholar] [CrossRef]
- Wei, Y.; Dong, R.; Zhang, Y.; Liang, S. Study on the interface instability of a shock wave-sub-millimeter liquid droplet interface and a numerical investigation of its breakup. Appl. Sci. 2023, 13, 13283. [Google Scholar] [CrossRef]
- Ge, X.; Vasilyev, O.V.; De Stefano, G.; Hussaini, M.Y. Wavelet-based adaptive unsteady Reynolds-averaged Navier-Stokes computations of wall-bounded internal and external compressible turbulent flows. In Proceedings of the 56th AIAA Aerospace Sciences Meeting, Kissimme, FL, USA, 8–12 January 2018. [Google Scholar]
- Menter, F.R.; Hüppe, A.; Matyushenko, A.; Kolmogorov, D. An Overview of Hybrid RANS-LES Models Developed for Industrial CFD. Appl. Sci. 2021, 11, 2459. [Google Scholar] [CrossRef]
- Strelets, M. Detached eddy simulation of massively separated flows. In Proceedings of the 39th AIAA Aerospace Sciences Meeting, Reno, NV, USA, 8–11 January 2001. [Google Scholar]
- Menter, F. Stress-Blended Eddy Simulation (SBES)—A New Paradigm in Hybrid RANS-LES Modeling. In Progress in Hybrid RANS-LES Modelling, Proceedings of the Papers Contributed to the 6th Symposium on Hybrid RANS-LES Methods, Strasbourg, France, 26–28 September 2016; Hoarau, Y., Peng, S.-H., Schwamborn, D., Revell, A., Eds.; Springer: Cham, Switzerland, 2018; pp. 27–37. [Google Scholar]
- Hirt, C.W.; Nichols, B.D. Volume of fluid (VOF) method for the dynamics of free boundaries. J. Comput. Phys. 1981, 39, 201–225. [Google Scholar] [CrossRef]
- Denaro, F.M.; De Stefano, G. A new development of the dynamic procedure in large-eddy simulation based on a finite volume integral approach. Application to stratified turbulence. Theoret. Comput. Fluid Dyn. 2011, 25, 315–355. [Google Scholar] [CrossRef]
- Igra, D.; Takayama, K. Investigation of aerodynamic breakup of a cylindrical water droplet. At. Sprays 2001, 11, 167–185. [Google Scholar]
- Shyue, K. A fluid-mixture type algorithm for barotropic two-fluid flow problems. J. Comput. Phys. 1998, 200, 718–748. [Google Scholar] [CrossRef]
- Sembian, S.; Liverts, M.; Tillmark, N.; Apazidis, N. Plane shock wave interaction with a cylindrical water column. Phys. Fluids 2016, 28, 056102. [Google Scholar] [CrossRef]
- Rossano, V.; De Stefano, G. Computational evaluation of shock wave interaction with a cylindrical water column. Appl. Sci. 2021, 11, 4934. [Google Scholar] [CrossRef]
- Sharma, S.; Singh, A.P.; Rao, S.S.; Kumar, A.; Basu, S. Shock induced aerobreakup of a droplet. J. Fluid Mech. 2021, 929, A27. [Google Scholar] [CrossRef]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Menter, F.R. Review of the shear-stress transport turbulence model experience from an industrial perspective. Int. J. Comput. Fluid Dyn. 2009, 23, 305–316. [Google Scholar] [CrossRef]
- Wilcox, D.C. Turbulence Modeling for CFD; DCW Industries, Inc.: La Cañada, CA, USA, 2006. [Google Scholar]
- Polansky, J.; Schmelter, S. Implementation of turbulence damping in the OpenFOAM multiphase flow solver interFoam. Arch. Thermodyn. 2022, 43, 21–43. [Google Scholar]
- Nicoud, F.; Ducros, F. Subgrid-scale stress modelling based on the square of the velocity gradient tensor. Flow Turbul. Combust. 1999, 62, 183–200. [Google Scholar] [CrossRef]
- Chode, K.K.; Viswanathan, H.; Chow, K.; Reese, H. Investigating the aerodynamic drag and noise characteristics of a standard squareback vehicle with inclined side-view mirror configurations using a hybrid computational aeroacoustics (CAA) approach. Phys. Fluids 2023, 35, 075148. [Google Scholar] [CrossRef]
- Salomone, T.; Piomelli, U.; De Stefano, G. Wall-modeled and hybrid large-eddy simulations of the flow over roughness strips. Fluids 2023, 8, 10. [Google Scholar] [CrossRef]
- Rossano, V.; De Stefano, G. Hybrid VOF-Lagrangian CFD modeling of droplet aerobreakup. Appl. Sci. 2022, 12, 8302. [Google Scholar] [CrossRef]
- Zhu, W.; Zheng, H.; Zhao, N. Numerical investigations on the deformation and breakup of an n–decane droplet induced by a shock wave. Phys. Fluids 2022, 34, 063306. [Google Scholar] [CrossRef]
- Brackbill, J.U.; Kothe, D.B.; Zemach, C. A continuum method for modeling surface tension. J. Comput. Phys. 1992, 100, 335–354. [Google Scholar] [CrossRef]
- Chang, C.H.; Deng, X.; Theofanous, T.G. Direct numerical simulation of interfacial instabilities: A consistent, conservative, all-speed, sharp-interface method. J. Comput. Phys. 2013, 242, 946–990. [Google Scholar] [CrossRef]
- Boiko, V.M.; Poplavski, S.V. Experimental study of two types of stripping breakup of a drop in the flow behind the shock wave. Combust. Explos. Shock Waves 2012, 48, 440–445. [Google Scholar] [CrossRef]
- Theofanous, T.G.; Mitkin, V.V.; Ng, C.L.; Chang, C.H.; Deng, X.; Sushchikh, S. The physics of aerobreakup. II. Viscous liquids. Phys. Fluids 2012, 24, 022104. [Google Scholar] [CrossRef]
- Hosseinzadeh-Nik, Z.; Aslani, M.; Owkes, M.; Regele, J.D. Numerical simulation of a shock wave impacting a droplet using the adaptive wavelet-collocation method. In Proceedings of the ILASS-Americas 28th Annual Conference on Liquid Atomization and Spray Systems, Dearborn, MI, USA, 15–18 May 2016. [Google Scholar]
- Ge, X.; De Stefano, G.; Hussaini, M.Y.; Vasilyev, O.V. Wavelet-based adaptive eddy-resolving methods for modeling and simulation of complex wall-bounded compressible turbulent flows. Fluids 2021, 6, 331. [Google Scholar] [CrossRef]
- De Stefano, G.; Brown-Dymkoski, E.; Vasilyev, O.V. Wavelet-based adaptive large-eddy simulation of supersonic channel flow. J. Fluid Mech. 2020, 901, A13. [Google Scholar] [CrossRef]
- Ge, X.; Vasilyev, O.V.; De Stefano, G.; Hussaini, M.Y. Wavelet-based adaptive unsteady Reynolds-averaged Navier-Stokes simulations of wall-bounded compressible turbulent flows. AIAA J. 2020, 58, 1529–1549. [Google Scholar] [CrossRef]
- Daniel, K.A.; Guildenbecher, D.R.; Delgado, P.M.; White, G.E.; Reardon, S.M.; Stauffacher, H.L.; Beresh, S.J. Drop interactions with the conical shock structure generated by a Mach 4.5 projectile. AIAA J. 2023, 61, 2347–2355. [Google Scholar] [CrossRef]










| Parameter | Symbol | Pre-Shock | Post-Shock |
|---|---|---|---|
| Pressure (atm) | |||
| Temperature (K) | 293 | 381 | |
| Density () | |||
| Viscosity (dynamic) () | |||
| Velocity (m/s) | 0 | 226 |
| Parameter | Symbol | Value |
|---|---|---|
| Shock Mach number | ||
| Droplet diameter (mm) | ||
| Mach number | ||
| Reynolds number | ||
| Ohnesorge number | ||
| Weber number | ||
| Density ratio | 460 | |
| Viscosity ratio | 46 | |
| Timescale () | 455 |
| Denomination | Size | Resolution ( ) |
|---|---|---|
| Grid I | ||
| Grid II | ||
| Grid III |
| Denomination | Error | Error | Error | Error |
|---|---|---|---|---|
| Grid I | ||||
| Grid II |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rossano, V.; De Stefano, G. Scale-Resolving Simulation of Shock-Induced Aerobreakup of Water Droplet. Computation 2024, 12, 71. https://doi.org/10.3390/computation12040071
Rossano V, De Stefano G. Scale-Resolving Simulation of Shock-Induced Aerobreakup of Water Droplet. Computation. 2024; 12(4):71. https://doi.org/10.3390/computation12040071
Chicago/Turabian StyleRossano, Viola, and Giuliano De Stefano. 2024. "Scale-Resolving Simulation of Shock-Induced Aerobreakup of Water Droplet" Computation 12, no. 4: 71. https://doi.org/10.3390/computation12040071
APA StyleRossano, V., & De Stefano, G. (2024). Scale-Resolving Simulation of Shock-Induced Aerobreakup of Water Droplet. Computation, 12(4), 71. https://doi.org/10.3390/computation12040071







