Entropy Generation and Thermal Radiation Impact on Magneto-Convective Flow of Heat-Generating Hybrid Nano-Liquid in a Non-Darcy Porous Medium with Non-Uniform Heat Flux
Abstract
1. Introduction
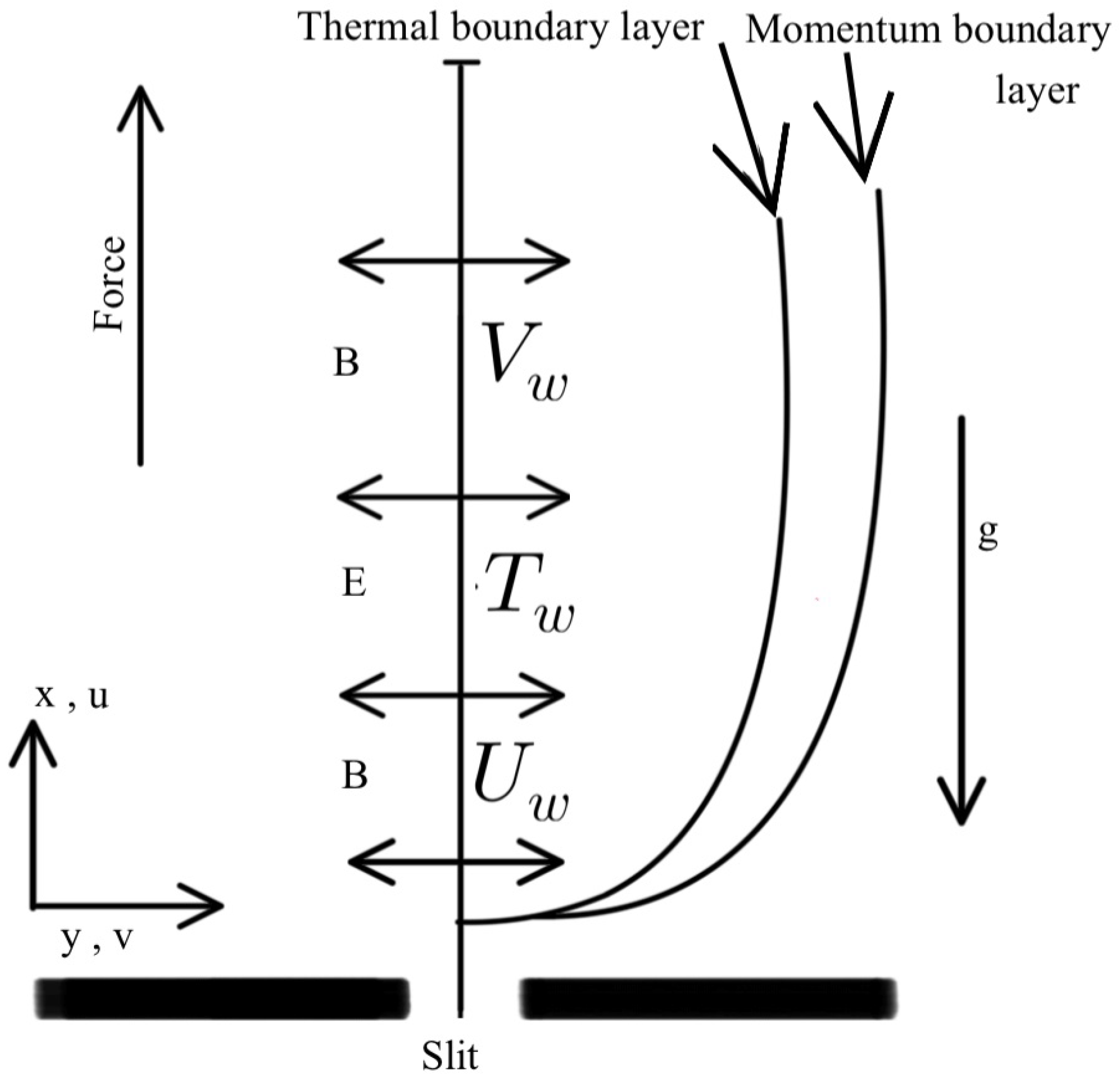
2. Equations and Physical Formulation
3. Method of Solution
4. Entropy Generation
5. Results and Discussion
6. Conclusions
- Increases in the injection, electric, and mixed convection parameters result in increases in the velocity profile. Conversely, as the suction parameter , unsteadiness parameter, magnetic parameter, and Forchheimer number increase, the velocity decreases.
- The temperature profile amplifies with increments in the Brinkman number , thermal radiation , space-dependent heat generation , temperature-dependent heat generation , and Forchheimer number . But it shows the reverse behaviour for the unsteadiness parameter , space-dependent heat absorption , and temperature-dependent heat absorption .
- The entropy generation is enhanced by increases in the Brinkmann number, magnetic parameter, Reynolds number, and temperature ratio parameter.
- Increases in the skin friction coefficient are caused by increases in the unsteadiness and magnetic parameters. Furthermore, as the electric field parameter increases, the skin friction coefficient decreases.
- The Nusselt number rises with increases in the unsteadiness and thermal radiation parameters. Additionally, as the Brinkman number increases, the Nusselt number decreases.
- This study is useful to thermal science applications in various areas of engineering and technology. Also, the study can be extended with different nano-particles and base fluids to explore the enhancement techniques.
- This study is beneficial to thermal science applications because it discusses the factors that lead to the working hybrid nano-liquid thermal enhancement.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Alizadeh, M.; Dogonchi, A.S.; Ganji, D.D. Micropolar nanofluid flow and heat transfer between penetrable walls in the presence of thermal radiation and magnetic field. Case Stud. Therm. Eng. 2018, 12, 319–332. [Google Scholar] [CrossRef]
- Mondal, H.; Almakki, M.; Sibanda, P. Dual solutions for three-dimensional magnetohydrodynamic nanofluid flow with entropy generation. J. Comput. Des. Eng. 2019, 6, 657–665. [Google Scholar] [CrossRef]
- Parveen, R.; Mahapatra, T. Numerical simulation of MHD double diffusive natural convection and entropy generation in a wavy enclosure filled with nanofluid with discrete heating. Heliyon 2019, 5, e02496. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.-X.; Alshbool, M.H.; Lv, Y.-P.; Khan, I.; Khan, M.R.; Issakhov, A. Heat and mass transfer in MHD Williamson nanofluid flow over an exponentially porous stretching surface. Case Stud. Therm. Eng. 2021, 26, 100975. [Google Scholar] [CrossRef]
- Yu, Y.; Khan, U.; Zaib, A.; Ishak, A.; Waini, I.; Raizah, Z.; Galal, A.M. Exploration of 3D stagnation-point flow induced by nanofluid through a horizontal plane surface saturated in a porous medium with generalized slip effects. Ain Shams Eng. J. 2023, 14, 101873. [Google Scholar] [CrossRef]
- Reddy, N.K.; Sankar, M. Buoyant heat transfer of nanofluids in a vertical porous annulus: A comparative study of different models. Int. J. Numer. Methods Heat Fluid Flow 2022, 33, 477–509. [Google Scholar] [CrossRef]
- Khan, U.; Zaib, A.; Pop, I.; Waini, I.; Ishak, A. MHD flow of a nanofluid due to a nonlinear stretching/shrinking sheet with a convective boundary condition: Tiwari–Das nanofluid model. Int. J. Numer. Methods Heat Fluid Flow 2022, 32, 3233–3258. [Google Scholar] [CrossRef]
- Hayat, T.; Nadeem, S. Heat transfer enhancement with Ag–CuO/water hybrid nanofluid. Results Phys. 2017, 7, 2317–2324. [Google Scholar] [CrossRef]
- Nadeem, S.; Abbas, N.; Khan, A. Characteristics of three dimensional stagnation point flow of Hybrid nanofluid past a circular cylinder. Results Phys. 2018, 8, 829–835. [Google Scholar] [CrossRef]
- Kaska, S.A.; Khalefa, R.A.; Hussein, A.M. Hybrid nanofluid to enhance heat transfer under turbulent flow in a flat tube. Case Stud. Therm. Eng. 2019, 13, 10039. [Google Scholar] [CrossRef]
- Khan, U.; Shafiq, A.; Zaib, A.; Baleanu, D. Hybrid nanofluid on mixed convective radiative flow from an irregular variably thick moving surface with convex and concave effects. Case Stud. Therm. Eng. 2020, 21, 100660. [Google Scholar] [CrossRef]
- Rajesh, V.; Sheremet, M.A.; Öztop, H.F. Impact of hybrid nanofluids on MHD flow and heat transfer near a vertical plate with ramped wall temperature. Case Stud. Therm. Eng. 2021, 28, 101557. [Google Scholar] [CrossRef]
- Mishra, A.; Upreti, H. A comparative study of Ag–MgO/water and Fe3O4–CoFe2O4/EG–water hybrid nanofluid flow over a curved surface with chemical reaction using Buongiorno model. Partial. Differ. Equations Appl. Math. 2022, 5, 100322. [Google Scholar] [CrossRef]
- Crane, L.J. Flow past a stretching plate. Zeitschrift für angewandte Mathematik und Physik ZAMP 1970, 21, 645–647. [Google Scholar] [CrossRef]
- Govardhan, K.; Nagaraju, G.; Kaladhar, K.; Balasiddulu, M. MHD and Radiation Effects on Mixed Convection Unsteady Flow of Micropolar Fluid Over A Stretching Sheet. Procedia Comput. Sci. 2015, 57, 65–76. [Google Scholar] [CrossRef][Green Version]
- Naramgari, S.; Sulochana, C. MHD flow over a permeable stretching/shrinking sheet of a nanofluid with suction/injection. Alex. Eng. J. 2016, 55, 819–827. [Google Scholar] [CrossRef]
- Ibrahim, W. Magnetohydrodynamic (MHD) boundary layer stagnation point flow and heat transfer of a nanofluid past a stretching sheet with melting. Propuls. Power Res. 2017, 6, 214–222. [Google Scholar] [CrossRef]
- Mohammadein, S.A.; Raslan, K.; Abdel-Wahed, M.S.; Abedel-Aal, E.M. KKL-model of MHD CuO-nanofluid flow over a stagnation point stretching sheet with nonlinear thermal radiation and suction/injection. Results Phys. 2018, 10, 194–199. [Google Scholar] [CrossRef]
- Kho, Y.B.; Hussanan, A.; Mohamed, M.K.A.; Salleh, M.Z. Heat and mass transfer analysis on flow of Williamson nanofluid with thermal and velocity slips: Buongiorno model. Propuls. Power Res. 2019, 8, 243–252. [Google Scholar] [CrossRef]
- Pal, D.; Mandal, G. Magnetohydrodynamic stagnation-point flow of Sisko nanofluid over a stretching sheet with suction. Propuls. Power Res. 2020, 9, 408–422. [Google Scholar] [CrossRef]
- Zainal, N.A.; Nazar, R.; Naganthran, K.; Pop, I. Unsteady MHD stagnation point flow induced by exponentially permeable stretching/shrinking sheet of hybrid nanofluid. Eng. Sci. Technol. Int. J. 2021, 24, 1201–1210. [Google Scholar] [CrossRef]
- Tawade, J.V.; Guled, C.; Noeiaghdam, S.; Fernandez-Gamiz, U.; Govindan, V.; Balamuralitharan, S. Effects of thermophoresis and Brownian motion for thermal and chemically reacting Casson nanofluid flow over a linearly stretching sheet. Results Eng. 2022, 15, 100448. [Google Scholar] [CrossRef]
- Daniel, Y.S.; Aziz, Z.A.; Ismail, Z.; Salah, F. Impact of thermal radiation on electrical MHD flow of nanofluid over nonlinear stretching sheet with variable thickness. Alex. Eng. J. 2018, 57, 2187–2197. [Google Scholar] [CrossRef]
- Daniel, Y.S.; Aziz, Z.A.; Ismail, Z.; Salah, F. Thermal radiation on unsteady electrical MHD flow of nanofluid over stretching sheet with chemical reaction. J. King Saud Univ.-Sci. 2019, 31, 804–812. [Google Scholar] [CrossRef]
- Gopal, D.; Saleem, S.; Jagadha, S.; Ahmad, F.; Almatroud, A.O.; Kishan, N. Numerical analysis of higher order chemical reaction on electrically MHD nanofluid under influence of viscous dissipation. Alex. Eng. J. 2020, 60, 1861–1871. [Google Scholar] [CrossRef]
- Niranjan, H.; Sivasankaran, S.; Bhuvaneswari, M. Analytical and numerical study on magnetoconvection stagnation-point flow in a porous medium with chemical reaction, radiation, and slip effects. Math. Probl. Eng. 2016, 2016, 4017076. [Google Scholar] [CrossRef]
- Sivasankaran, S.; Niranjan, H.; Bhuvaneswari, M. Chemical reaction, radiation and slip effects on MHD mixed convection stagnation-point flow in a porous medium with convective boundary condition. Int. J. Numer. Methods Heat Fluid Flow 2017, 27, 454–470. [Google Scholar] [CrossRef]
- Niranjan, H.; Sivasankaran, S.; Bhuvaneswari, M. Chemical reaction, soret and dufour effects on MHD mixed convection stagnation point flow with radiation and slip condition. Sci. Iran. 2017, 24, 698–706. [Google Scholar] [CrossRef]
- Sivasankaran, S.; Bhuvaneswari, M.; Alzahrani, A. Numerical simulation on convection of non-Newtonian fluid in a porous enclosure with non-uniform heating and thermal radiation. Alex. Eng. J. 2020, 59, 3315–3323. [Google Scholar] [CrossRef]
- Sivasankaran, S.; Bhuvaneswari, M.; Amer, A. Numerical study on buoyant convection and thermal radiation in a cavity with various thermal sources and Cattaneo-Christov heat flux. Case Stud. Therm. Eng. 2021, 27, 101207. [Google Scholar] [CrossRef]
- Mallawi, F.; Bhuvaneswari, M.; Sivasankaran, S.; Eswaramoorthi, S. Impact of double-stratification on convective flow of a non-Newtonian liquid in a Riga plate with Cattaneo-Christov double-flux and thermal radiation. Ain Shams Eng. J. 2020, 12, 969–981. [Google Scholar] [CrossRef]
- Khashi’ie, N.S.; Waini, I.; Arifin, N.M.; Pop, I. Dual solutions of unsteady two-dimensional electro-magneto-hydrodynamics (EMHD) axisymmetric stagnation-point flow of a hybrid nanofluid past a radially stretching/shrinking Riga surface with radiation effect. Int. J. Numer. Methods Heat Fluid Flow 2022, 33, 333–350. [Google Scholar] [CrossRef]
- Zainal, N.A.; Nazar, R.; Naganthran, K.; Pop, I. Unsteady stagnation point flow past a permeable stretching/shrinking Riga plate in Al2O3-Cu/H2O hybrid nanofluid with thermal radiation. Int. J. Numer. Methods Heat Fluid Flow 2021, 32, 2640–2658. [Google Scholar] [CrossRef]
- Bhuvaneswari, M.; Sivasankaran, S.; Kim, Y.J. Lie Group Analysis of Radiation Natural Convection Flow over An Inclined Surface in a Porous Medium with Internal Heat Generation. J. Porous Media 2012, 15, 1155–1164. [Google Scholar] [CrossRef]
- Sandeep, N.; Sulochana, C. Dual solutions for unsteady mixed convection flow of MHD micropolar fluid over a stretching/shrinking sheet with non-uniform heat source/sink. Eng. Sci. Technol. Int. J. 2015, 18, 738–745. [Google Scholar] [CrossRef]
- Karthikeyan, S.; Bhuvaneswari, M.; Sivanandam, S.; Rajan, S.; Anna University. Erode Arts and Science College Soret and Dufour Effects on MHD Mixed Convection Heat and Mass Transfer of a Stagnation Point Flow towards a Vertical Plate in a Porous Medium with Chemical Reaction, Radiation and Heat Generation. J. Appl. Fluid Mech. 2016, 9, 1447–1455. [Google Scholar]
- Ali, N.; Khan, S.U.; Sajid, M.; Abbas, Z. MHD flow and heat transfer of couple stress fluid over an oscillatory stretching sheet with heat source/sink in porous medium. Alex. Eng. J. 2016, 55, 915–924. [Google Scholar] [CrossRef]
- Irfan, M.; Khan, M.; Khan, W. Numerical analysis of unsteady 3D flow of Carreau nanofluid with variable thermal conductivity and heat source/sink. Results Phys. 2017, 7, 3315–3324. [Google Scholar] [CrossRef]
- Cheong, H.T.; Sivasankaran, S.; Bhuvaneswari, M. Natural convection in a wavy porous cavity with sinusoidal heating and internal heat generation. Int. J. Numer. Methods Heat Fluid Flow 2017, 27, 287–309. [Google Scholar] [CrossRef]
- Khan, M.; Irfan, M.; Khan, W. Impact of heat source/sink on radiative heat transfer to Maxwell nanofluid subject to revised mass flux condition. Results Phys. 2018, 9, 851–857. [Google Scholar] [CrossRef]
- Ragupathi, P.; Hakeem, A.A.; Al-Mdallal, Q.M.; Ganga, B.; Saranya, S. Non-uniform heat source/sink effects on the three-dimensional flow of Fe3O4/Al2O3 nanoparticles with different base fluids past a Riga plate. Case Stud. Therm. Eng. 2019, 15, 100521. [Google Scholar] [CrossRef]
- Agrawal, P.; Dadheech, P.K.; Jat, R.N.; Bohra, M.; Nisar, K.S.; Khan, I. Lie similarity analysis of MHD flow past a stretching surface embedded in porous medium along with imposed heat source/sink and variable viscosity. J. Mater. Res. Technol. 2020, 9, 10045–10053. [Google Scholar] [CrossRef]
- Chu, Y.-M.; Ramzan, M.; Shaheen, N.; Chung, J.D.; Kadry, S.; Howari, F.; Malik, M.; Ghazwani, H.A.S. Analysis of Newtonian heating and higher-order chemical reaction on a Maxwell nanofluid in a rotating frame with gyrotactic microorganisms and variable heat source/sink. J. King Saud Univ.-Sci. 2021, 33, 101645. [Google Scholar] [CrossRef]
- Bhuvaneswari, M.; Sivasankaran, S.; Malarselvi, A.; Ganga, B. Radiation and cross diffusion on unsteady chemically reactive convective flow through an extended surface in heat generating porous medium. Int. J. Energy Technol. Policy 2021, 17, 494–509. [Google Scholar] [CrossRef]
- Yesodha, P.; Bhuvaneswari, M.; Sivasankaran, S.; Saravanan, K. Nanofluid flow with activation energy and heat generation under slip boundary condition with convective heat and mass transfer. Mater. Today Proc. 2022, 59, 959–967. [Google Scholar] [CrossRef]
- Gautam, A.K.; Rajput, S.; Bhattacharyya, K.; Pandey, A.K.; Chamkha, A.J.; Begum, M. Comparative study of two non-Newtonian fluids with bioconvective induced MHD flow in presence of multiple slips, heat source/sink and nonlinear thermal radiation. Chem. Eng. J. Adv. 2022, 12, 100365. [Google Scholar] [CrossRef]
- Bejan, A. A Study of Entropy Generation in Fundamental Convective Heat Transfer. J. Heat Transf. 1979, 101, 718–725. [Google Scholar] [CrossRef]
- Bejan, A. The thermodynamic design of heat and mass transfer processes and devices. Int. J. Heat Fluid Flow 1987, 8, 258–276. [Google Scholar] [CrossRef]
- Goqo, S.; Oloniiju, S.; Mondal, H.; Sibanda, P.; Motsa, S. Entropy generation in MHD radiative viscous nanofluid flow over a porous wedge using the bivariate spectral quasi-linearization method. Case Stud. Therm. Eng. 2018, 12, 774–788. [Google Scholar] [CrossRef]
- Ijaz, M.; Ayub, M.; Khan, H. Entropy generation and activation energy mechanism in nonlinear radiative flow of Sisko nanofluid: Rotating disk. Heliyon 2019, 5, e01863. [Google Scholar] [CrossRef]
- Abbas, Z.; Naveed, M.; Hussain, M.; Salamat, N. Analysis of entropy generation for MHD flow of viscous fluid embedded in a vertical porous channel with thermal radiation. Alex. Eng. J. 2020, 59, 3395–3405. [Google Scholar] [CrossRef]
- Usman; Ghaffari, A.; Mustafa, I.; Muhammad, T.; Altaf, Y. Analysis of entropy generation in a power-law nanofluid flow over a stretchable rotatory porous disk. Case Stud. Therm. Eng. 2021, 28, 101370. [Google Scholar] [CrossRef]
- Ibrahim, W.; Gamachu, D.; Bedada, B. Entropy generation analysis of three dimensional mixed convection flow of couple stress nanofluid with non-Fourier’s heat and non-Fick’s mass flux model. Alex. Eng. J. 2022, 61, 8843–8857. [Google Scholar] [CrossRef]
- Alzahrani, A.K.; Sivasankaran, S.; Bhuvaneswari, M. Numerical Simulation on Convection and Thermal Radiation of Casson Fluid in an Enclosure with Entropy Generation. Entropy 2020, 22, 229. [Google Scholar] [CrossRef]
- Sivanandam, S.; Chamkha, A.J.; Mallawi, F.O.M.; Alghamdi, M.S.; Alqahtani, A.M. Effects of Entropy Generation, Thermal Radiation and Moving-Wall Direction on Mixed Convective Flow of Nanofluid in an Enclosure. Mathematics 2020, 8, 1471. [Google Scholar] [CrossRef]
- Agrawal, R.; Kaswan, P. Entropy generation minimization of Ag-Fe3O4/water-ethylene glycol squeezed hybrid nanofluid flow between parallel disks. Int. J. Numer. Methods Heat Fluid Flow 2022, 33, 65–95. [Google Scholar] [CrossRef]
- Bai, Y.; Fang, H.; Zhang, Y. Entropy generation analysis on unsteady flow of Maxwell nanofluid over the stretched wedge with Cattaneo-Christov double diffusion. Int. J. Numer. Methods Heat Fluid Flow 2021, 32, 2198–2220. [Google Scholar] [CrossRef]
- Mumraiz, S.; Ali, A.; Awais, M.; Shutaywi, M.; Shah, Z. Entropy generation in electrical magnetohydrodynamic flow of Al2O3–Cu/H2O hybrid nanofluid with non-uniform heat flux. J. Therm. Anal. Calorim. 2020, 143, 2135–2148. [Google Scholar] [CrossRef]
- Ibrahim, W.; Shankar, B. MHD boundary layer flow and heat transfer of a nanofluid past a permeable stretching sheet with velocity, thermal and solutal slip boundary conditions. Comput. Fluids 2013, 75, 1–10. [Google Scholar] [CrossRef]






| Constants | A | Space-dependent coefficient | |
| B | Temperature-dependent coefficient | Brinkman number | |
| Strength of magnetic field | Heat capacity | ||
| Skin friction coefficient | Strength of electric field | ||
| Electric field parameter | F | Local interia coefficient | |
| Forchheimer coefficient | g | Acceleration due to gravity | |
| Porous medium permeability | Mean absorption coefficient | ||
| M | Magnetic field parameter | n | Shape factor |
| Nusselt number | P | Fluid pressure | |
| Prandtl number | Radiative heat flux | ||
| Radiation number | Reynolds number | ||
| s | Suction/injection | T | Fluid temperature |
| Free stream temperature | Surface temperature | ||
| Velocity component | Stretching sheet velocity | ||
| Wall mass transfer | |||
| Greek symbols | |||
| Drag inverse number | Thermal expansion | ||
| Unsteadiness parameter | Thermal conductivity | ||
| Mixed convection parameter | Dynamic viscosity | ||
| Kinematic viscosity | Density | ||
| Stefan–Boltzmann constant | Electric conductivity | ||
| Nano-particles volume fraction | Nano-particles volume fraction | ||
| Stream function | Dimensionless temperature ratio | ||
| Subscripts | |||
| f | Base fluid | Nano-fluid | |
| Hybrid nano-fluid | First solid nano-particle | ||
| Second nano-particle |
| Physical Properties | Fluid () | () | ( ) |
|---|---|---|---|
| 997.1 | 8933 | 3970 | |
| 4180 | 385 | 765 | |
| 0.613 | 401 | 40 | |
| 21 | 1.67 | 0.85 | |
| 0.05 |
| Properties | Hybrid Nano-Fluid |
|---|---|
| Density | |
| Viscosity | |
| Heat capacity | |
| Thermal conductivity | |
| where | |
| Electrical conductivity | |
| where | |
| Thermal expansion coefficient |
| s | M | Ref. [59] | Present Study |
|---|---|---|---|
| 0 | 1 | 1.4142 | 1.41422 |
| 0.2 | 1.5177 | 1.51775 | |
| 0.7 | 1.8069 | 1.80688 | |
| 1 | 2.0000 | 2.00000 | |
| 0.5 | 0 | 1.2808 | 1.28083 |
| 0.5 | 1.5000 | 1.50000 | |
| 1 | 1.6861 | 1.68614 | |
| 1.5 | 1.8508 | 1.85078 | |
| 2 | 2.0000 | 2.00000 |
| M | |||||||
|---|---|---|---|---|---|---|---|
| 0 | 0 | 0 | 1.24055 | 0 | 0 | 0 | 3.15502 |
| 0.5 | 1.45188 | 0.2 | 3.07932 | ||||
| 1 | 1.63642 | 0.4 | 3.00371 | ||||
| 1.5 | 1.80205 | 0.6 | 2.92818 | ||||
| 0.2 | 0 | 1.27808 | 0.2 | 0 | 3.23935 | ||
| 0.5 | 1.47705 | 0.2 | 3.17179 | ||||
| 1 | 1.65304 | 0.4 | 3.1043 | ||||
| 1.5 | 1.81238 | 0.6 | 3.03688 | ||||
| 0.4 | 0 | 1.31173 | 0.4 | 0 | 3.31076 | ||
| 0.5 | 1.49989 | 0.2 | 3.24923 | ||||
| 1 | 1.66818 | 0.4 | 3.18776 | ||||
| 1.5 | 1.82171 | 0.6 | 3.12633 | ||||
| 0.6 | 0 | 1.34213 | 0.6 | 0 | 3.37259 | ||
| 0.5 | 1.52078 | 0.2 | 3.31573 | ||||
| 1 | 1.6821 | 0.4 | 3.25891 | ||||
| 1.5 | 1.83029 | 0.6 | 3.20213 | ||||
| 0.1 | 0 | 0 | 1.24055 | 0.2 | 0 | 0 | 3.44101 |
| 0.5 | 1.40459 | 0.2 | 3.362 | ||||
| 1 | 1.55522 | 0.4 | 3.28308 | ||||
| 1.5 | 1.69421 | 0.6 | 1.24055 | ||||
| 0.2 | 0 | 1.27808 | 0.2 | 0 | 3.58856 | ||
| 0.5 | 1.43461 | 0.2 | 3.51733 | ||||
| 1 | 1.57882 | 0.4 | 3.44617 | ||||
| 1.5 | 1.7125 | 0.6 | 3.37508 | ||||
| 0.4 | 0 | 1.31173 | 0.4 | 0 | 3.71083 | ||
| 0.5 | 1.46144 | 0.2 | 3.64531 | ||||
| 1 | 1.59988 | 0.4 | 3.57985 | ||||
| 1.5 | 1.72876 | 0.6 | 3.51444 | ||||
| 0.6 | 0 | 1.34213 | 0.6 | 0 | 3.8148 | ||
| 0.5 | 1.48566 | 0.2 | 3.75371 | ||||
| 1 | 1.61888 | 0.4 | 3.69265 | ||||
| 1.5 | 1.74337 | 0.6 | 3.63164 | ||||
| 0.2 | 0 | 0 | 1.24055 | 0.4 | 0 | 0 | 3.69209 |
| 0.5 | 1.3584 | 0.2 | 3.61041 | ||||
| 1 | 1.47601 | 0.4 | 3.52883 | ||||
| 1.5 | 1.58875 | 0.6 | 3.44733 | ||||
| 0.2 | 0 | 1.27808 | 0.2 | 0 | 3.89908 | ||
| 0.5 | 1.39288 | 0.2 | 3.82488 | ||||
| 1 | 1.506 | 0.4 | 3.75076 | ||||
| 1.5 | 1.61438 | 0.6 | 3.6767 | ||||
| 0.4 | 0 | 1.31173 | 0.4 | 0 | 4.06879 | ||
| 0.5 | 1.42347 | 0.2 | 4.00004 | ||||
| 1 | 1.53259 | 0.4 | 3.93133 | ||||
| 1.5 | 1.63711 | 0.6 | 3.86268 | ||||
| 0.6 | 0 | 1.34213 | 0.6 | 0 | 4.21217 | ||
| 0.5 | 1.45087 | 0.2 | 4.14762 | ||||
| 1 | 1.55637 | 0.4 | 4.0831 | ||||
| 1.5 | 1.65744 | 0.6 | 4.01863 | ||||
| 0.3 | 0 | 0 | 1.24055 | 0.6 | 0 | 0 | 3.91664 |
| 0.5 | 1.31316 | 0.2 | 3.83274 | ||||
| 1 | 1.39851 | 0.4 | 3.74893 | ||||
| 1.5 | 1.48536 | 0.6 | 3.66521 | ||||
| 0.2 | 0 | 1.27808 | 0.2 | 0 | 4.18034 | ||
| 0.5 | 1.3518 | 0.2 | 4.10367 | ||||
| 1 | 1.43441 | 0.4 | 4.02707 | ||||
| 1.5 | 1.51782 | 0.6 | 3.95053 | ||||
| 0.4 | 0 | 1.31173 | 0.4 | 0 | 4.39486 | ||
| 0.5 | 1.38595 | 0.2 | 4.32339 | ||||
| 1 | 1.4662 | 0.4 | 4.25197 | ||||
| 1.5 | 1.54665 | 0.6 | 4.1806 | ||||
| 0.6 | 0 | 1.34213 | 0.6 | 0 | 4.57545 | ||
| 0.5 | 1.41639 | 0.2 | 4.50798 | ||||
| 1 | 1.49453 | 0.4 | 4.76062 | ||||
| 1.5 | 1.57243 | 0.6 | 4.37318 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Albqmi, N.M.; Sivanandam, S. Entropy Generation and Thermal Radiation Impact on Magneto-Convective Flow of Heat-Generating Hybrid Nano-Liquid in a Non-Darcy Porous Medium with Non-Uniform Heat Flux. Computation 2024, 12, 43. https://doi.org/10.3390/computation12030043
Albqmi NM, Sivanandam S. Entropy Generation and Thermal Radiation Impact on Magneto-Convective Flow of Heat-Generating Hybrid Nano-Liquid in a Non-Darcy Porous Medium with Non-Uniform Heat Flux. Computation. 2024; 12(3):43. https://doi.org/10.3390/computation12030043
Chicago/Turabian StyleAlbqmi, Nora M., and Sivasankaran Sivanandam. 2024. "Entropy Generation and Thermal Radiation Impact on Magneto-Convective Flow of Heat-Generating Hybrid Nano-Liquid in a Non-Darcy Porous Medium with Non-Uniform Heat Flux" Computation 12, no. 3: 43. https://doi.org/10.3390/computation12030043
APA StyleAlbqmi, N. M., & Sivanandam, S. (2024). Entropy Generation and Thermal Radiation Impact on Magneto-Convective Flow of Heat-Generating Hybrid Nano-Liquid in a Non-Darcy Porous Medium with Non-Uniform Heat Flux. Computation, 12(3), 43. https://doi.org/10.3390/computation12030043






