On the Influence of Initial Stresses on the Velocity of Elastic Waves in Composites
Abstract
1. Homogenization in the Problem of the Elasticity Theory with Initial Stresses
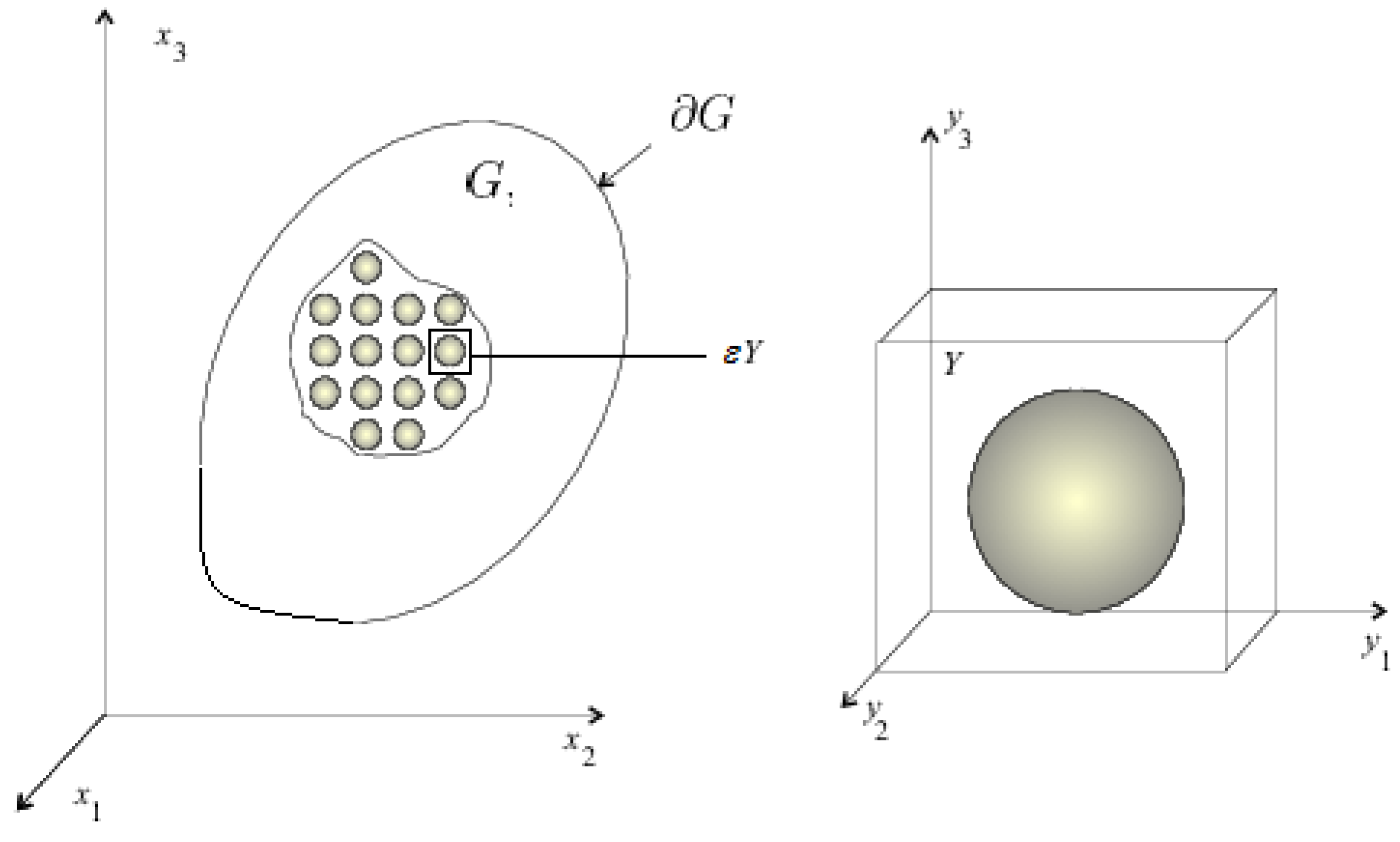
1.1. Asymptotic Expansions for the Elasticity Problem
- -
- These dependencies are the same or different for the homogeneous and the composite bodies;
- -
- If different, how large is the difference.
1.2. The Case of Small Initial Stresses
2. Laminated Materials with Initial Stresses
2.1. One Special Case
2.2. Small Initial Stresses
- -
- —the homogenized elastic constant of the composite without initial stresses;
- -
- —the term corresponding to the “intermediate” homogenization;
- -
- —the term .
2.3. The Homogenized Velocity of the Elastic Waves
3. The Non-Trivial Dependence of Speed of Elastic Waves on the Initial Stress in the “Inverted Honeycomb” Frame Structure
4. The Problem of “Intermediate” Homogenization
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Bakhvalov, N.S.; Panasenko, G.P. Homogenisation: Averaging Processes in Periodic Media; Kluwer: Dordrecht, The Netherlands, 1989. [Google Scholar]
- Sanchez-Palencia, E. Non-Homogeneous Media and Vibration Theory; Springer: Berlin/Heidelberg, Germany, 1980. [Google Scholar]
- Sanchez-Palencia, E. Equations aux deivees partielles. Solutions periodiques par repport aux variables d’espace et application. Comp. Rend. Acad. Sci. Paris Ser. A 1970, 271, 1129–1132. [Google Scholar]
- Alaire, G. Homogenization and two–scale convergence. SIAM J. Math. Anal. 1992, 23, 1482–1518. [Google Scholar] [CrossRef]
- Bensoussan, A.; Lions, J.-L.; Papanicolaou, G. Asymptotic Analysis for Periodic Structures; North–Holland Publ.: Amsterdam, The Netherlands, 1978. [Google Scholar]
- Jikov, V.V.; Kozlov, S.M.; Oleinik, O.A. Homogenization of Differential Operators and Integral Functionals; Springer: Berlin/Heidelberg, Germany, 1994. [Google Scholar]
- Kolpakov, A.G. Averaging of some systems of ordinary differential equations. Math. USSR-Sb. 1982, 119, 534–547. [Google Scholar] [CrossRef]
- Spagnolo, S. Sul limite delle soluzioni di problemi di Cauchy relativi all’equazione dell calore. Annali della Scuola Normale Superiore di Pisa 1967, 21, 657–699. [Google Scholar]
- Kolpakov, A.G. Stressed Composite Structures: Homogenized Models for Thin–Walled Nonhomogeneous Structures with Initial Stresses; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Xing, Y.F.; Gao, Y.H.; Chen, L.; Li, M. Solution methods for two key problems in multiscale asymptotic expansion method. Compos. Struct. 2017, 160, 854–866. [Google Scholar] [CrossRef]
- Fergoug, M.; Parret-Freaud, A.; Feld, N.; March, B.; Forest, S. A general boundary layer corrector for the asymptotic homogenization of elastic linear composite structures. Compos. Struct. 2022, 285, 115091. [Google Scholar] [CrossRef]
- Guz, A.N. Elastic Waves in Bodies with Initial Stresses. In 2 Volumes. Vol. 1. General Questions. Vol. 2. Regularities of Wave Propagation; Naukova Dumka: Kiev, Ukraine, 1986. (In Russian) [Google Scholar]
- Kolpakov, A.G. Effect of influation of initial stresses on the homogenized characteristics of composite. Mech. Mater. 2005, 37, 840–854. [Google Scholar] [CrossRef]
- Lekhnitskii, S.G. Theory of Elasticity of an Anisotropic Elastic Body; Holden-Day: San Francisco, CA, USA, 1963. [Google Scholar]
- Kalamkarov, A.L.; Kolpakov, A.G. Analysis, Design and Optimization of Composite Structures; John Wiley & Sons: Chichester, UK, 1997. [Google Scholar]
- Kolpakov, A.A.; Kolpakov, A.G. Capacity and Transport in Contrast Composite Structures: Asymptotic Analysis and Applications; CRC Press: Boca Raton, FL, USA, 2010. [Google Scholar]
- Gibson, L.J.; Ashby, M.F. Cellular Solids; Cambridge Univ. Press: Cambridge, UK, 1987. [Google Scholar]
- Bahrant, J.; Ashby, M.F.; Fleck, N.A. Cellular Metals and Metal Foaming Technology; Verlag MIT Publ.: Bremen, Germany, 2001. [Google Scholar]
- Kolpakov, A.G. Determination of the average characteristics of elastic frameworks. J. Appl. Math. Mech. 1985, 49, 739–745. [Google Scholar] [CrossRef]
- Andrianov, I.; Awrejcewicz, J.; Manevitch, L.I. Asymptotical Mechanics of Thin–Walled Structures; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Almgren, R.F. An isotropic three–dimensional structure with Poisson’s ratio = −1. J. Elast. 1985, 15, 427–430. [Google Scholar]
- Friis, E.A.; Lakes, R.S.; Park, J.B. Negative Poisson’s ratio polymeric and metalic foams. J. Mater. Sci. 1988, 23, 4406–4414. [Google Scholar] [CrossRef]
- Evans, K.E. Tensile network microstructures exhibiting negative Poisson’s ratios. Phys. D Appl. Phys. 1989, 22, 1870–1876. [Google Scholar] [CrossRef]
- Alderson, K.L.; Evans, K.E. The fabrication of microporous polyethylene having a negative Poisson’s ratio. Polymer 1992, 33, 4435–4438. [Google Scholar] [CrossRef]
- Boal, D.H.; Seifert, U.; Shillcock, J.C. Negative Poisson’s ratio in two–dimensional network under tension. Phys. Rev. E 1993, 48, 4274–4283. [Google Scholar] [CrossRef] [PubMed]
- Grima, J.; Alderson, A.; Evans, K.E. An alternative explanation for the negative Poisson’s ratios in auxetic foams. J. Phys. Soc. Jpn. 2005, 74, 1341–1342. [Google Scholar] [CrossRef]
- Lakes, R. Foam structures with negative Poisson’s ratio. Science 1987, 235, 1038. [Google Scholar] [CrossRef] [PubMed]
- Lakes, R. Deformation mechanisms of negative Poisson’s ratio materials: Structural aspects. J. Mater. Sci. 1991, 26, 2287–2292. [Google Scholar] [CrossRef]
- Lakes, R. Advances in negative Poisson’s ratio materials. Adv. Mater. 1993, 5, 293–296. [Google Scholar] [CrossRef]
- Larsen, U.D.; Sigmund, O.; Bouwstra, S. Design and fabrication of compliant micromechanisms and structures with negative Poisson’s ratio. J. Microelectromech. Syst. 1997, 6, 99–106. [Google Scholar] [CrossRef]
- Lee, J.; Choi, J.B. Application of homogenization FEM analysis to regular and re-entrant honeycomb structures. J. Mater. Sci. 1996, 31, 4105–4110. [Google Scholar] [CrossRef]
- Milton, G.W. Composite materials with Poisson’s ratio close to −1. J. Mech. Phys. Solids 1992, 40, 1105–1137. [Google Scholar] [CrossRef]
- Ninarello, A.; Ruiz-Franco, J.; Zaccarelli, E. Onset of criticality in hyper-auxetic polymer networks. Nat. Commun. 2022, 13, 527. [Google Scholar] [CrossRef] [PubMed]
- Choi, H.Y.; Shin, E.J.; Lee, S.H. Design and evaluation of 3D-printed auxetic structures coated by CWPU/graphene as strain sensor. Sci. Rep. 2022, 12, 7780. [Google Scholar] [CrossRef] [PubMed]
- Meeusen, L.C.; Idori, S.; Micoli, L.L.; Guidi, G.; Stankovic, T.; Graziosi, S. Auxetic structures used in kinesiology tapes can improve form-fitting and personalization. Sci. Rep. 2022, 12, 13509. [Google Scholar] [CrossRef] [PubMed]
- Cheng, X.; Zhang, Y.; Ren, X.; Han, D.; Jiang, W.; Gang, X.; Hui, Z.; Luo, C.; Xie, Y.M. Design and mechanical characteristics of auxetic metamaterial with tunable stiffness. Int. J. Mech. Sci. 2022, 223, 107286. [Google Scholar] [CrossRef]
- Acuna, D.; Gutierrez, F.; Silva, R.; Palza, H.; Nunez, A.S.; During, G. A three step recipe for designing auxetic materials on demand. Commun. Phys. 2022, 5, 113. [Google Scholar] [CrossRef]
- Dudek, K.K.; Martinez, J.A.; Ulliac, G.; Kadic, M. Micro-scale auxetic hierarchical mechanical metamaterials for shape morphing. Adv. Mater. 2022, 34, 2110115. [Google Scholar] [CrossRef]
- Reshetova, G.; Cheverda, V.; Khachkova, T. Numerical experiments with digital twins of core samples for estimating effective elastic parameters. In Russian Supercomputing Days; Voevodin, V., Sobolev, S., Eds.; Springer: Cham, Switzerland, 2019; pp. 290–301. [Google Scholar]
- Available online: https://www.slb.com/resource-library/article/2015/defining-coring (accessed on 20 May 2022).
- Monicard, R.P. Properties of Reservoir Rocks: Core Analysis; Technips: Paris, France, 1980. [Google Scholar]
- McPhee, C.; Reed, J.; Zubizarreta, I. (Eds.) Core Analysis. A Best Practice Guide; Elsevier: Amsterdam, The Netherlands, 2015. [Google Scholar]
- Wasidzu, K. Variational Methods in the Theory of Elasticity and Plasticity; Pergamon Press: Oxford, UK, 1982. [Google Scholar]
- Marcellini, P. Su una convergenza di funzioni convesse. Boll. Dell’Unione Mat. Ital. 1973, 8, 137–158. [Google Scholar]
- Marcellini, P. Un teorema di passagio de limite per la somma di convesse. Boll. Dell’Unione Mat. Ital. 1975, 4, 107–124. [Google Scholar]
- Zhou, S.; Jia, Y.; Wang, C. Global Sensitivity Analysis for the Polymeric Microcapsules in Self-Healing Cementitious Composites. Polymers 2020, 12, 2990. [Google Scholar] [CrossRef]
- Bazighifan, O.; Moaaz, O.; El-Nabulsi, R.A.; Muhib, A. Some new oscillation results for fourth-order neutral differential equations with delay argument. Symmetry 2020, 12, 1248. [Google Scholar] [CrossRef]
- Zadobrischi, E.; Cosovanu, L.-M.; Dimian, M. Traffic flow density model and dynamic traffic congestion model simulation based on practice case with vehicle network and system traffic intelligent communication. Symmetry 2020, 12, 1172. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kolpakov, A.G.; Andrianov, I.V.; Rakin, S.I. On the Influence of Initial Stresses on the Velocity of Elastic Waves in Composites. Computation 2023, 11, 15. https://doi.org/10.3390/computation11020015
Kolpakov AG, Andrianov IV, Rakin SI. On the Influence of Initial Stresses on the Velocity of Elastic Waves in Composites. Computation. 2023; 11(2):15. https://doi.org/10.3390/computation11020015
Chicago/Turabian StyleKolpakov, Alexander G., Igor V. Andrianov, and Sergey I. Rakin. 2023. "On the Influence of Initial Stresses on the Velocity of Elastic Waves in Composites" Computation 11, no. 2: 15. https://doi.org/10.3390/computation11020015
APA StyleKolpakov, A. G., Andrianov, I. V., & Rakin, S. I. (2023). On the Influence of Initial Stresses on the Velocity of Elastic Waves in Composites. Computation, 11(2), 15. https://doi.org/10.3390/computation11020015





