Benefits of Playing at School: Filler Board Games Improve Visuospatial Memory and Mathematical Skills
Abstract
1. Introduction
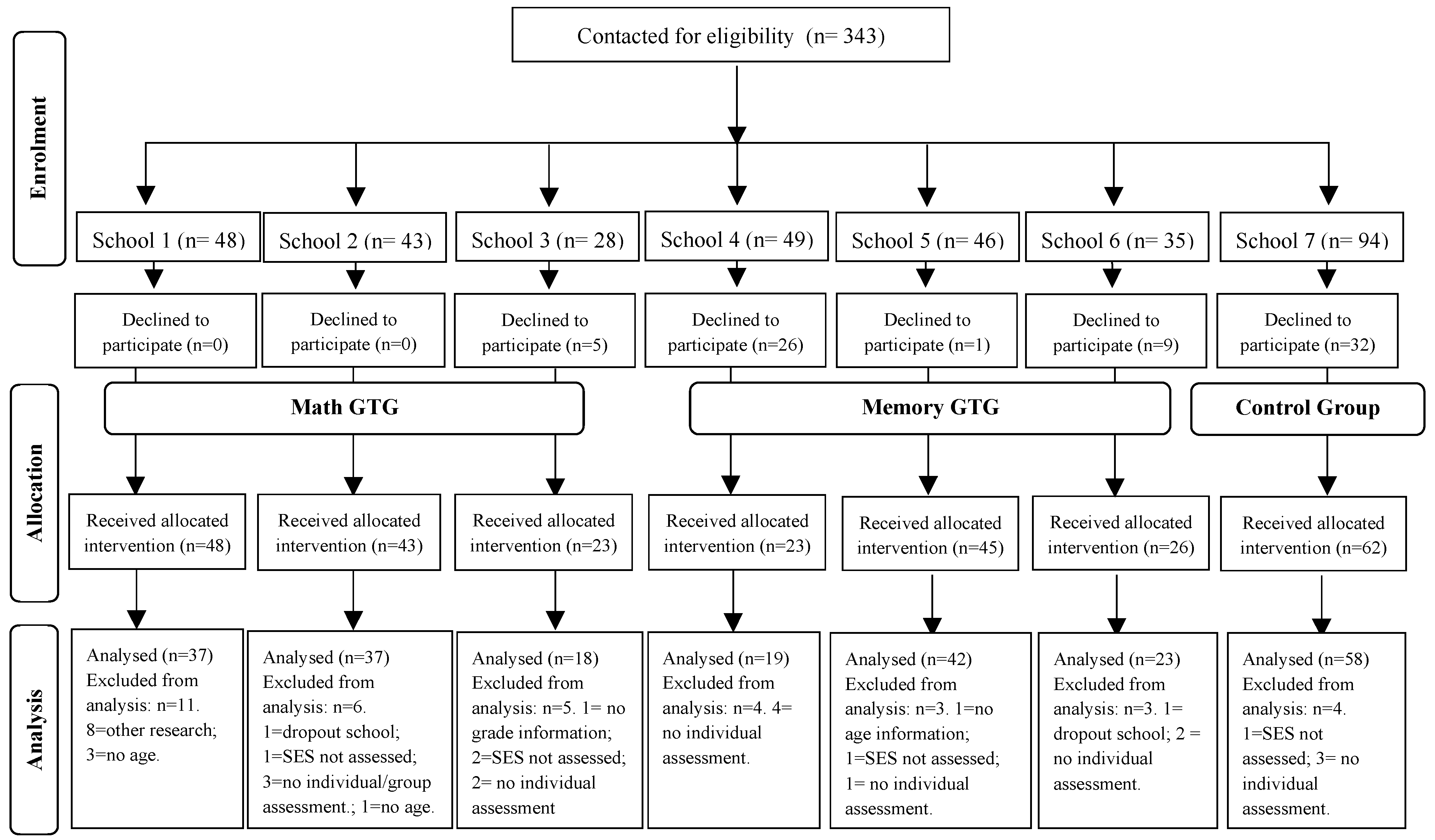
2. Materials and Methods
2.1. Participants
2.2. Measures
2.2.1. Covariate Measures
2.2.2. Memory Outcome Measures
2.2.3. Mathematical Outcome Measures
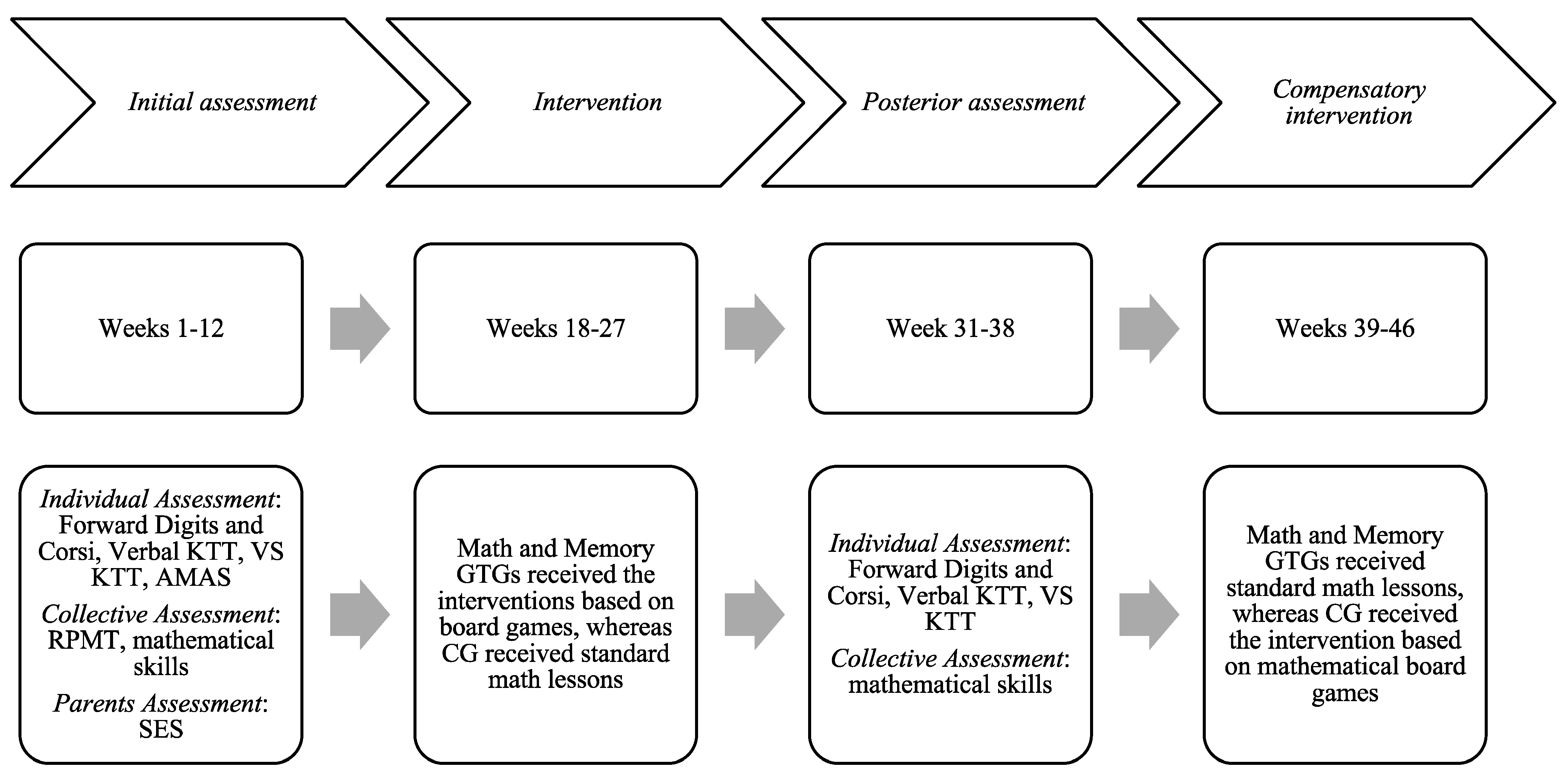
2.3. Procedure
2.4. Intervention
2.5. Statistical Analysis
3. Results
3.1. Compliance with the Intervention Program
3.2. Descriptive and Baseline Comparisons
3.2.1. Grade 3
3.2.2. Grade 4
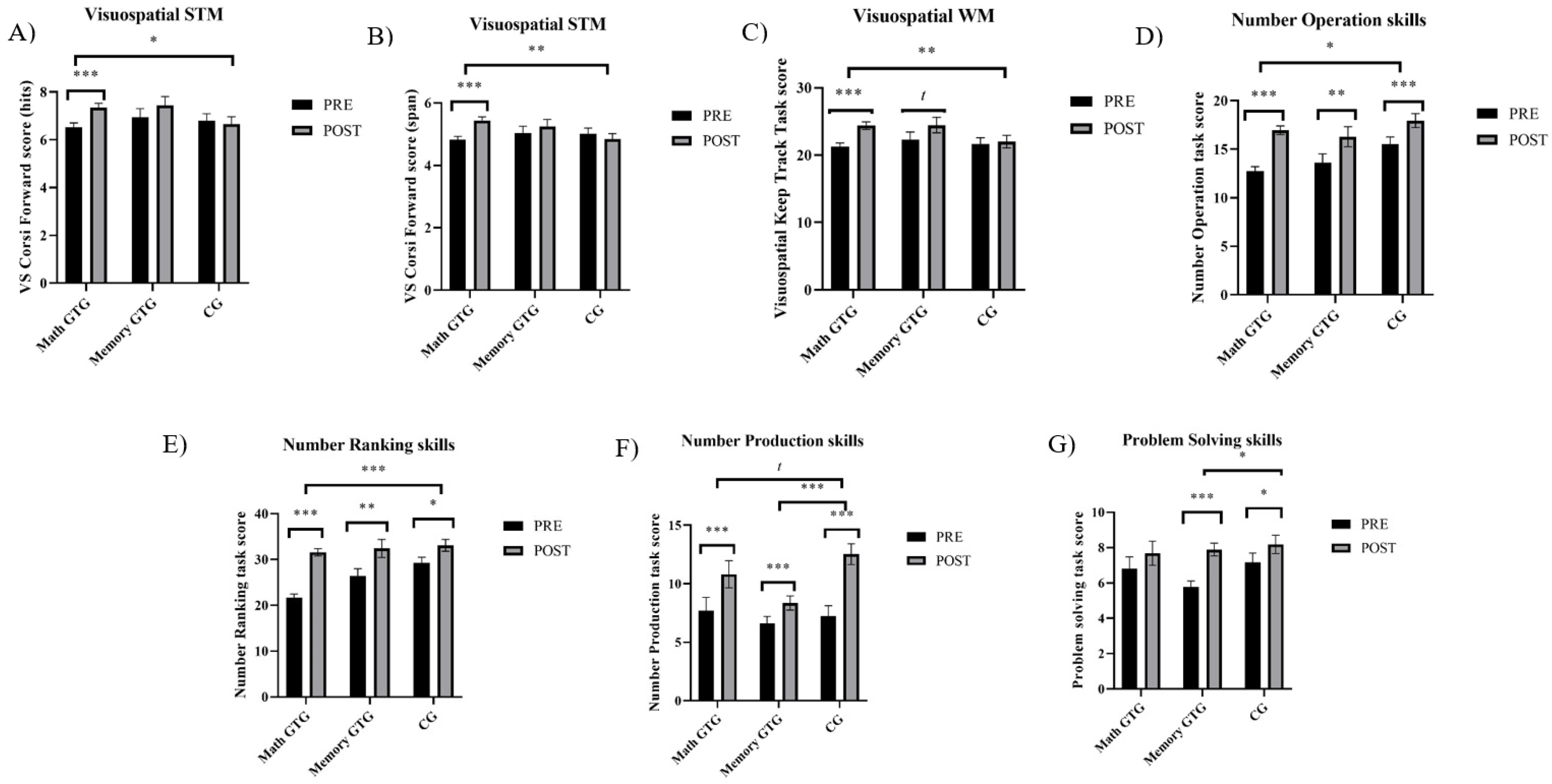
3.3. Intervention Effects in Grade 3
3.3.1. Memory Outcomes
3.3.2. Math Skills
3.4. Intervention Effects in Grade 4
3.4.1. Memory Outcomes
3.4.2. Math Skills
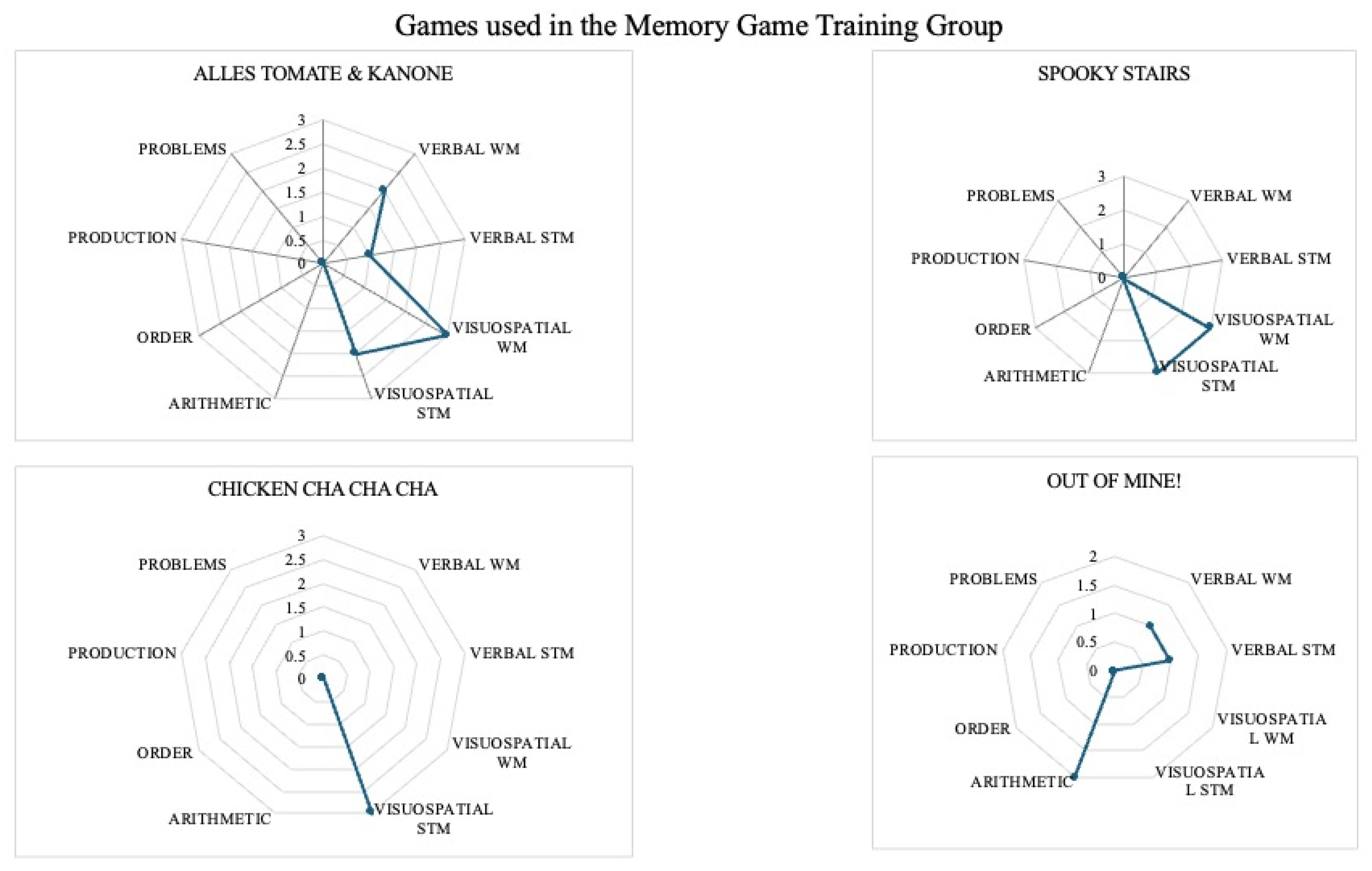
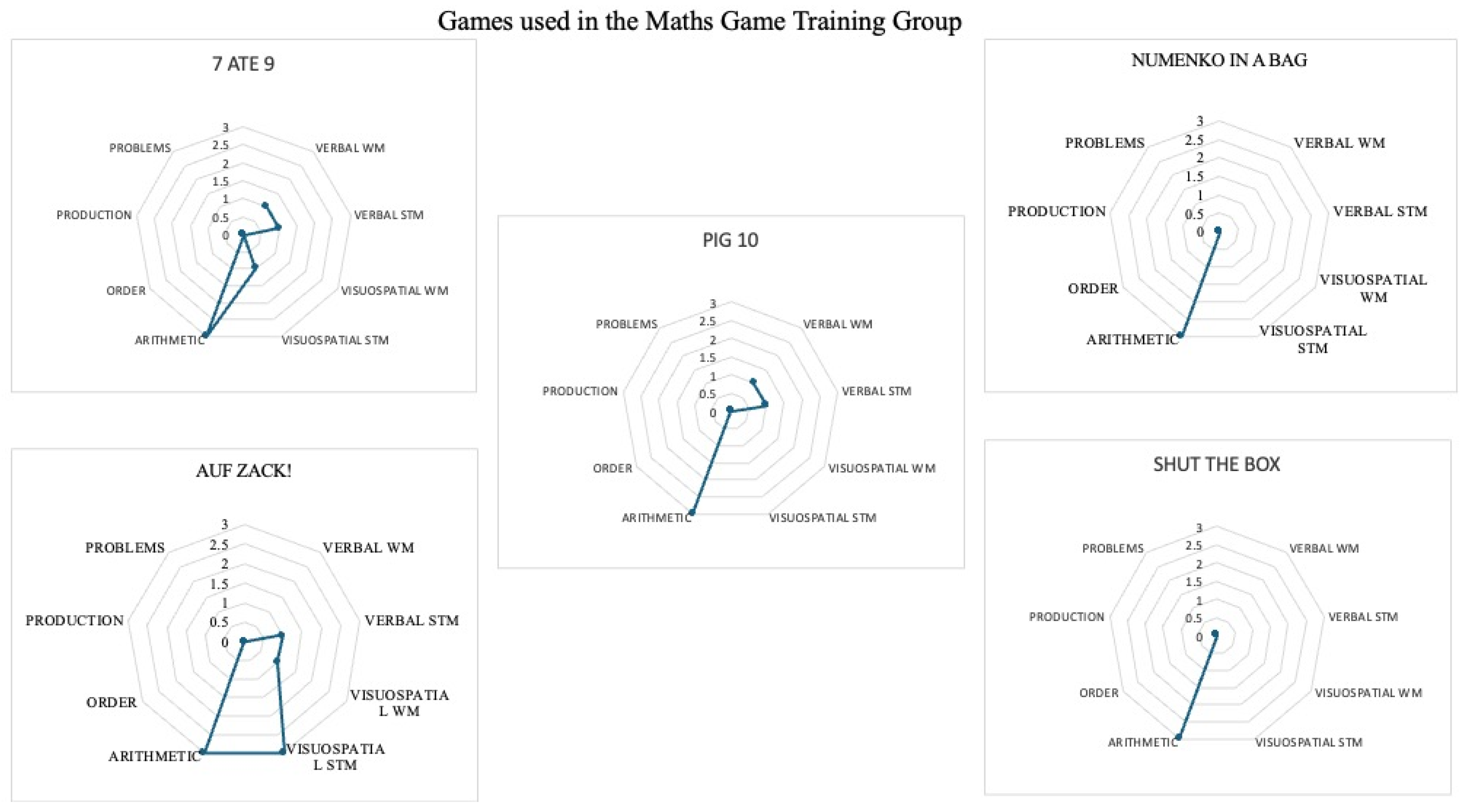
3.5. Educative and Memory Profile of the Games
4. Discussion
Generalizability and Limitations of the Study
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Titz, C.; Karbach, J. Working memory and executive functions: Effects of training on academic achievement. Psychol. Res. 2014, 78, 852–868. [Google Scholar] [CrossRef] [PubMed]
- Baddeley, A. Working memory. Science 2010, 20, 136–140. [Google Scholar]
- Alloway, T.P.; Gathercole, S.E.; Pickering, S.J. Verbal and Visuospatial Short-Term and Working Memory in Children: Are They Separable? Child Dev. 2006, 77, 1698–1716. [Google Scholar] [CrossRef] [PubMed]
- Baddeley, A. Working Memory: Theories, Models, and Controversies. Annu. Rev. Psychol. 2012, 63, 1–29. [Google Scholar] [CrossRef] [PubMed]
- Baddeley, A. Working memory: Looking back and looking forward. Nat. Rev. Neurosci. 2003, 4, 829–839. [Google Scholar] [CrossRef] [PubMed]
- Miyake, A.; Friedman, N.P.; Emerson, M.J.; Witzki, A.H.; Howerter, A.; Wager, T.D. The Unity and Diversity of Executive Functions and Their Contributions to Complex ‘Frontal Lobe’ Tasks: A Latent Variable Analysis. Cogn. Psychol. 2000, 41, 49–100. [Google Scholar] [CrossRef] [PubMed]
- Henry, L. The Working Memory Model. In The Development of Working Memory in Children; Henry, L., Ed.; City University London: London, UK; Sage Pub: Washington, DC, USA, 2011; pp. 1–36. [Google Scholar]
- Clair-Thompson, H.L.S.; Gathercole, S.E. Executive functions and achievements in school: Shifting, updating, inhibition, and working memory. Q. J. Exp. Psychol. 2006, 59, 745–759. [Google Scholar] [CrossRef]
- Den Bos, I.F.-V.; Van Der Ven, S.H.G.; Kroesbergen, E.H.; Van Luit, J.E.H. Working memory and mathematics in primary school children: A meta-analysis. Educ. Res. Rev. 2013, 10, 29–44. [Google Scholar] [CrossRef]
- Bull, R.; Espy, K.A.; Wiebe, S.A. Wiebe Short-term memory, working memory, and executive functioning in preschoolers: Longitudinal predictors of mathematical achievement at age 7 years. Dev. Neuropsychol. 2008, 33, 205–228. [Google Scholar] [CrossRef] [PubMed]
- Bahar-Fuchs, A.; Clare, L.; Woods, B. Cognitive training and cognitive rehabilitation for persons with mild to moderate dementia of the Alzheimer’s or vascular type: A review. Alzheimers. Res. Ther. 2013, 5, 35. [Google Scholar] [CrossRef]
- Tajik-Parvinchi, D.; Wright, L.; Schachar, R. Cognitive Rehabilitation for Attention Deficit/Hyperactivity Disorder (ADHD): Promises and Problems. J. Can. Acad. Child Adolesc. Psychiatry 2014, 23, 207–217. [Google Scholar] [PubMed]
- Sala, G.; Gobet, F. Does Far Transfer Exist? Negative Evidence from Chess, Music, and Working Memory Training. Curr. Dir. Psychol. Sci. 2017, 26, 515–520. [Google Scholar] [CrossRef] [PubMed]
- Astle, D.E.; Barnes, J.J.; Baker, K.; Colclough, G.L.; Woolrich, M.W. Cognitive Training Enhances Intrinsic Brain Connectivity in Childhood. J. Neurosci. 2015, 35, 6277–6283. [Google Scholar] [CrossRef] [PubMed]
- Rapport, M.D.; Orban, S.A.; Kofler, M.J.; Friedman, L.M. Do programs designed to train working memory, other executive functions, and attention benefit children with ADHD? A meta-analytic review of cognitive, academic, and behavioral outcomes. Clin. Psychol. Rev. 2013, 33, 1237–1252. [Google Scholar] [CrossRef] [PubMed]
- Tamm, L.; Epstein, J.N.; Peugh, J.L.; Nakonezny, P.A.; Hughes, C.W. Preliminary data suggesting the efficacy of attention training for school-aged children with ADHD. Dev. Cogn. Neurosci. 2013, 4, 16–28. [Google Scholar] [CrossRef] [PubMed]
- Sala, G.; Gobet, F. Working memory training in typically developing children: A meta-analysis of the available evidence. Dev. Psychol. 2017, 53, 671–685. [Google Scholar] [CrossRef] [PubMed]
- Schwaighofer, M.; Fischer, F.; Bühner, M.; Schwaighofer, M.; Fischer, F.; Markus, B. Does Working Memory Training Transfer? A Meta- Analysis Including Training Conditions as Moderators Does Working Memory Training Transfer? A Meta-Analysis Including Training Conditions as Moderators. Educ. Psychol. 2015, 50, 138–166. [Google Scholar] [CrossRef]
- Melby-Lervåg, M.; Hulme, C. Is working memory training effective? A meta-analytic review. Dev. Psychol. 2013, 49, 270–291. [Google Scholar] [CrossRef] [PubMed]
- Sanchez-Perez, N.; Castillo, A.; Lopez-Lopez, J.A.; Pina, V.; Puga, J.L.; Campoy, G.; Gonzalez-Salinas, C.; Fuentes, L.J. Computer-Based Training in Math and Working Memory Improves Cognitive Skills and Academic Achievement in Primary School Children: Behavioral Results. Front. Psychol. 2018, 8, 2327. [Google Scholar] [CrossRef]
- Ramani, G.B.; Jaeggi, S.M.; Daubert, E.N.; Buschkuehl, M. Domain-Specific and Domain-General Training to Improve Kindergarten Children’s Mathematics. J. Numer. Cogn. 2017, 3, 468–495. [Google Scholar] [CrossRef]
- Kuhn, J.-T.; Holling, H. Number sense or working memory? The effect of two computer-based trainings on mathematical skills in elementary school. Adv. Cogn. Psychol. 2014, 10, 59–67. [Google Scholar] [CrossRef] [PubMed]
- Clements, D.H.; Sarama, J.; Germeroth, C. Learning executive function and early mathematics: Directions of causal relations. Early Child. Res. Q. 2016, 36, 79–90. [Google Scholar] [CrossRef]
- Ninaus, M.; Pereira, G.; Stefitz, R.; Prada, R.; Paiva, A.; Neuper, C.; Wood, G. Game elements improve performance in a working memory training task. Int. J. Serious Games 2015, 2, 3–16. [Google Scholar] [CrossRef]
- Prins, P.J.M.; Dovis, S.; Ponsioen, A.; Brink, E.T.; van der Oord, S. Does Computerized Working Memory Training with Game Elements Enhance Motivation and Training Efficacy in Children with ADHD? Cyberpsychol. Behav. Soc. Netw. 2011, 14, 115–122. [Google Scholar] [CrossRef] [PubMed]
- Iizuka, A.; Suzuki, H.; Ogawa, S.; Kobayashi-Cuya, K.E.; Kobayashi, M.; Inagaki, H.; Sugiyama, M.; Awata, S.; Takebayashi, T.; Fujiwara, Y. Does social interaction influence the effect of cognitive intervention program? A randomized controlled trial using Go game. Int. J. Geriatr. Psychiatry 2019, 34, 324–332. [Google Scholar] [CrossRef] [PubMed]
- Gauthier, A.; Kato, P.M.; Bul, K.C.M.; Dunwell, I.; Walker-Clarke, A.; Lameras, P. Board Games for Health: A Systematic Literature Review and Meta-Analysis. Games Health J. 2019, 8, 85–100. [Google Scholar] [CrossRef] [PubMed]
- Gobet, F.; Retschitzki, J.; de Voogt, A. Introduction. In Moves in Mind: The Psychology of Board Games; Gobet, F., Retschitzki, J., de Voogt, A., Eds.; Psychology Press: London, UK, 2004; pp. 1–10. [Google Scholar]
- Sousa, M.; Bernardo, E. Back in the Game. In Proceedings of the 11th International Conference Videogame Sciences and Arts, Aveiro, Portugal, 27–29 November 2019; Zagalo, N., Veloso, A.I., Costa, L., Mealha, Ó., Eds.; Springer Nature: Cham, Switzerland, 2019; pp. 72–85. [Google Scholar] [CrossRef]
- Bartolucci, M.; Mattioli, F.; Batini, F. Do board games make people smarter? Two initial exploratory studies. Int. J. Game-Based Learn. 2019, 9, 1–14. [Google Scholar] [CrossRef]
- Jirout, J.J.; Newcombe, N.S. Building Blocks for Developing Spatial Skills: Evidence from a Large, Representative U.S. Sample. Psychol. Sci. 2015, 26, 302–310. [Google Scholar] [CrossRef] [PubMed]
- Newman, S.D.; Hansen, M.T.; Gutierrez, A. An fMRI Study of the Impact of Block Building and Board Games on Spatial Ability. Front. Psychol. 2016, 7, 1–9. [Google Scholar] [CrossRef]
- Nath, S.; Szücs, D. Construction play and cognitive skills associated with the development of mathematical abilities in 7-year-old children. Learn. Instr. 2014, 32, 73–80. [Google Scholar] [CrossRef]
- Benzing, V.; Schmidt, M.; Jäger, K.; Egger, F.; Conzelmann, A.; Roebers, C.M. A classroom intervention to improve executive functions in late primary school children: Too ‘old’ for improvements? Br. J. Educ. Psychol 2019, 89, 225–238. [Google Scholar] [CrossRef]
- Estrada-Plana, V.; Esquerda, M.; Mangues, R.; March-Llanes, J.; Moya-Higueras, J. A Pilot Study of the Efficacy of a Cognitive Training Based on Board Games in Children with Attention-Deficit/Hyperactivity Disorder: A Randomized Controlled Trial. Games Health J. 2019, 8, 265–274. [Google Scholar] [CrossRef]
- Vita-Barrull, N.; Estrada-Plana, V.; March-Llanes, J.; Sotoca-Orgaz, P.; Guzmán, N.; Ayesa, R.; Moya-Higueras, J. Do you play in class? Board games to promote cognitive and educational development in primary school: A cluster randomized controlled trial. Learn. Instr. 2024, 93, 101946. [Google Scholar] [CrossRef]
- Kaufman, D.; Kaufman, P. Strategy Training and Remedial Techniques. J. Learn. Disabil. 1975, 12, 416–419. [Google Scholar] [CrossRef]
- Kermani, F.K.; Mohammadi, M.R.; Yadegari, F.; Haresabadi, F.; Sadeghi, S.M. Working memory training in the form of structured games in children with attention deficit hyperactivity disorder. Iran. J. Psychiatry 2016, 11, 224–233. [Google Scholar]
- Kim, S.H.; Han, D.H.; Lee, Y.S.; Kim, B.N.; Cheong, J.H.; Han, S.H. Baduk (the game of go) improved cognitive function and brain activity in children with attention deficit hyperactivity disorder. Psychiatry Investig. 2014, 11, 143–151. [Google Scholar] [CrossRef]
- Ramani, G.B.; Siegler, R.S.; Hitti, A. Taking it to the classroom: Number board games as a small group learning activity. J. Educ. Psychol. 2012, 104, 661–672. [Google Scholar] [CrossRef]
- Ramani, G.B.; Siegler, R.S. Reducing the gap in numerical knowledge between low- and middle-income preschoolers. J. Appl. Dev. Psychol. 2011, 32, 146–159. [Google Scholar] [CrossRef]
- Siegler, R.S.; Ramani, G.B. Playing linear numerical board games promotes low-income children’s numerical development. Dev. Sci. 2008, 11, 655–661. [Google Scholar] [CrossRef]
- Siegler, R.S.; Ramani, G.B. Playing linear number board games—But not circular ones—Improves low-income preschoolers’ numerical understanding. J. Educ. Psychol. 2009, 101, 545–560. [Google Scholar] [CrossRef]
- Scalise, N.R.; Daubert, E.N.; Ramani, G.B. Benefits of Playing Numerical Card Games on Head Start Children’s Mathematical Skills. J. Exp. Educ. 2020, 88, 200–220. [Google Scholar] [CrossRef] [PubMed]
- Passolunghi, M.C.; Costa, H.M. Working memory and early numeracy training in preschool children. Child Neuropsychol. 2016, 22, 81–98. [Google Scholar] [CrossRef]
- Reeves, B.C.; Gaus, W. Guidelines for Reporting Non-Randomised Studies. Forsch Komplementärmed Kl. Naturheilkd 2004, 11, 46–52. [Google Scholar] [CrossRef]
- Hackman, D.A.; Gallop, R.; Evans, G.W.; Farah, M.J. Socioeconomic status and executive function: Developmental trajectories and mediation. Dev. Sci. 2015, 18, 686–702. [Google Scholar] [CrossRef] [PubMed]
- Dehn, M.J. How working memory enables fluid reasoning. Appl. Neuropsychol. Child 2017, 6, 245–247. [Google Scholar] [CrossRef]
- Green, C.T.; Bunge, S.A.; Chiongbian, V.B.; Barrow, M.; Ferrer, E. Fluid reasoning predicts future mathematical performance among children and adolescents. J. Exp. Child Psychol. 2017, 157, 125–143. [Google Scholar] [CrossRef]
- Luttenberger, S.; Wimmer, S.; Paechter, M. Spotlight on math anxiety. Psychol. Res. Behav. Manag. 2018, 11, 311–322. [Google Scholar] [CrossRef]
- Hollingshead, A.B. Four Factor Index of Social Status; Yale University: New Haven, CT, USA, 1975. [Google Scholar]
- Raven, J.C.; Court, J.H.; Raven, J. Manual Matrices Progresivas de Raven, 3rd ed.; TEA Ediciones: Madrid, Spain, 2001. [Google Scholar]
- Tejedor, B.; Santos, M.A.; García-Orza, J.; Carratalà, P.; Navas, M. Variables involved in math anxiety: A study in a sixth grade sample. Anu. Psicol. 2009, 40, 345–355. [Google Scholar]
- Wechsler, D. Escala de Inteligencia de Wechsler para Niños-IV (WISC-IV); TEA Ediciones: Madrid, Spain, 2003. [Google Scholar]
- Andersson, U.; Lyxell, B. Working memory deficit in children with mathematical difficulties: A general or specific deficit? J. Exp. Child Psychol. 2007, 96, 197–228. [Google Scholar] [CrossRef]
- Tamnes, C.K.; Østby, Y.; Walhovd, K.B.; Westlye, L.T.; Due-Tønnessen, P.; Fjell, A.M. Neuroanatomical correlates of executive functions in children and adolescents: A magnetic resonance imaging (MRI) study of cortical thickness. Neuropsychologia 2010, 48, 2496–2508. [Google Scholar] [CrossRef]
- Alloway, T.P.; Passolunghi, M.C. The relationship between working memory, IQ, and mathematical skills in children. Learn. Individ. Differ. 2011, 21, 133–137. [Google Scholar] [CrossRef]
- Knizia, R. Alles Kanone! Zoch Zum Spielen: Fürth, Germany, 2007. [Google Scholar]
- Knizia, R. Alles Tomate! Zoch Zum Spielen: Fürth, Germany, 2007. [Google Scholar]
- Schannen, M. Spooky Stairs; Iberia Devir: Barcelona, Spain, 2004. [Google Scholar]
- Nedergaard-Andersen, M. Out of Mine! Huch! & Friends: Günzburg, Germany, 2014. [Google Scholar]
- Zoch, K. Chicken Cha Cha Cha; Devir Iberia: Barcelona, Spain, 1998. [Google Scholar]
- Hiron, M. 7 Ate 9; 999 Games: Almere, The Netherlands, 2009. [Google Scholar]
- Lennett, T. Numenko in a Bag; TSL Enterprises Ltd.: Jasper, TX, USA, 2009. [Google Scholar]
- Pnueli, A. Pig 10; Zoch Zum Spielen: Fürth, Germany, 2010. [Google Scholar]
- Shut the Box; Gollnest & Kiesel: Güster, Germany, 1999.
- Becker, K.; Schliemann, J.-P. Auf Zack! Drei Hasen in der Abendsonne: Uehlfeld, Germany, 2009. [Google Scholar]
- The Jamovi Project. Jamovi (Version 1.6.15) [Computer Program]. 2021. Available online: https://www.jamovi.org (accessed on 1 April 2021).
- Ellis, P.D. The Essential Guide to Effect Sizes: Statistical Power, Meta-Analysis and the Interpretation of Research Results; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Brysbaert, M.; Stevens, M. Power Analysis and Effect Size in Mixed Effects Models: A Tutorial. J. Cogn. 2018, 1, 9. [Google Scholar] [CrossRef]
- Vita-Barrull, N.; March-Llanes, J.; Guzmán, N.; Estrada-Plana, V.; Mayoral, M.; Moya-Higueras, J.; Garrido, A.; Domínguez, G.; Cabezas, I.; González, J.; et al. The Cognitive Processes Behind Commercialized Board Games for Intervening in Mental Health and Education: A Committee of Experts. Games Health J. 2022, 11, 414–424. [Google Scholar] [CrossRef]
- Moya-Higueras, J.; Solé-Puiggené, M.; Vita-Barrull, N.; Estrada-Plana, V.; Guzmán, N.; Arias, S.; Garcia, X.; Ayesa-Arriola, R.; March-Llanes, J. Just Play Cognitive Modern Board and Card Games, It’s Going to Be Good for Your Executive Functions: A Randomized Controlled Trial with Children at Risk of Social Exclusion. Children 2023, 10, 1492. [Google Scholar] [CrossRef]
- Ready, D.D. Socioeconomic disadvantage, school attendance, and early cognitive development: The differential effects of school exposure. Sociol. Educ. 2010, 83, 271–286. [Google Scholar] [CrossRef]
- Garon-Carrier, G.; Boivin, M.; Lemelin, J.-P.; Kovas, Y.; Parent, S.; Séguin, J.R.; Vitaro, F.; Tremblay, R.E.; Dionne, G. Early developmental trajectories of number knowledge and math achievement from 4 to 10 years: Low-persistent profile and early-life predictors. J. Sch. Psychol. 2018, 68, 84–98. [Google Scholar] [CrossRef] [PubMed]
- Hattie, J.A.C. The nature of the evidence: A synthesis of meta-analysis. In Visible Learning: A Synthesis of over 800 Meta-Analysis Relating to Achievement; Hattie, J., Ed.; Routledge: New York, NY, USA, 2009. [Google Scholar]
- Klingberg, T.; Forssberg, H.; Westerberg, H. Increased Brain Activity in Frontal and Parietal Cortex Underlies the Development of Visuospatial Working Memory Capacity during Childhood. J. Cogn. Neurosci. 2002, 14, 1–10. [Google Scholar] [CrossRef] [PubMed]
- Ashkenazi, S.; Black, J.M.; Abrams, D.A.; Hoeft, F.; Menon, V. Neurobiological underpinnings of math and reading learning disabilities. J. Learn. Disabil. 2013, 46, 549–569. [Google Scholar] [CrossRef] [PubMed]
- Johann, V.E.; Karbach, J. Education Application of Cognitive Training. In Cognitive Training: An Overview of Features and Applications, 2nd ed.; Springer: New York, NY, USA, 2020; pp. 334–347. [Google Scholar]
- Lyons, I.M.; Beilock, S.L. Numerical ordering ability mediates the relation between number-sense and arithmetic competence. Cognition 2011, 121, 256–261. [Google Scholar] [CrossRef]
- Peng, P.; Namkung, J.; Barnes, M.; Congying, S. A Meta-Analysis of Mathematics and Working Memory: Moderating Effects of Working Memory Domain, Type of Mathematics Skill, and Sample Characteristics. J. Educ. Psychol. 2016, 108, 455–473. [Google Scholar] [CrossRef]
- Zheng, X.; Swanson, H.L.; Marcoulides, G.A. Working memory components as predictors of children’s mathematical word problem solving. J. Exp. Child Psychol. 2011, 110, 481–498. [Google Scholar] [CrossRef]
- Vita-Barrull, N.; Estrada-Plana, V.; March-Llanes, J.; Guzmán, N.; Fernández-Muñoz, C.; Ayesa, R.; Moya-Higueras, J. Board game-based intervention to improve executive functions and academic skills in rural schools: A randomized controlled trial. Trends Neurosci. Educ. 2023, 33, 100216. [Google Scholar] [CrossRef]
- Raghubar, K.P.; Barnes, M.A.; Hecht, S.A. Working memory and mathematics: A review of developmental, individual difference, and cognitive approaches. Learn. Individ. Differ. 2010, 20, 110–122. [Google Scholar] [CrossRef]
- McKenzie, B.; Bull, R.; Gray, C. The effects of phonological and visual-spatial interference on children’s arithmetical performance. Educ. Child Psychol. 2003, 20, 93–108. [Google Scholar] [CrossRef]
- de Souza-Salvador, L.; Moura, R.; Wood, G.; Haase, V.G. Cognitive heterogeneity of math difficulties: A bottom-up classification approach. J. Numer. Cogn. 2019, 5, 55–85. [Google Scholar] [CrossRef]
- Holmes, J.; Gathercole, S.E.; Place, M.; Dunning, D.L.; Hilton, K.A.; Elliott, J.G. Working memory deficits can be overcome: Impacts of training and medication on working memory in children with ADHD. Appl. Cogn. Psychol. 2010, 24, 827–836. [Google Scholar] [CrossRef]
- Kirk, H.; Gray, K.; Ellis, K.; Taffe, J.; Cornish, K. Impact of Attention Training on Academic Achievement, Executive Functioning, and Behavior: A Randomized Controlled Trial. Am. J. Intellect. Dev. Disabil. 2017, 122, 97–117. [Google Scholar] [CrossRef]
- Diamond, A.; Lee, K. Interventions Shown to Aid Executive Function Development in Children 4 to 12 Years Old. Science 2011, 333, 959–964. [Google Scholar] [CrossRef]
- Cheema, J.R.; Sheridan, K. Time spent on homework, mathematics anxiety and mathematics achievement: Evidence from a US sample. Issues Educ. Res. 2015, 25, 246–259. [Google Scholar]
| Grade 3 | Grade 4 | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Math GTG (n = 75) c | Memory GTG (n = 18) | CG (n = 28) | (p Value) | ε2/V | Math GTG (n = 17) c | Memory GTG (n = 66) | CG (n = 30) | (p Value) | ε2/V | |
| Demographical characteristics | Demographical characteristics | |||||||||
| Age (in years) M ± SD | 8.31 ± 0.38 | 8.47 ± 0.36 | 8.46 ± 0.28 | 7.13 * (0.028) | 0.06 | 9.42 ± 0.26 | 9.39 ± 0.40 | 9.39 ± 0.32 | 0.79 (0.673) | 0.01 |
| Sex a | 4.66 (0.097) | 0.20 | 1.64 (0.441) | 0.12 | ||||||
| Boys, n (%) | 46 (62%) | 14 (78%) | 13 (46%) | 6 (35%) | 34 (52%) | 13 (43%) | ||||
| Girls, n (%) | 28 (38%) | 4 (22%) | 15 (54%) | 11 (65%) | 32 (48%) | 17 (57%) | ||||
| SES Index, M ± SD | 32.80 ± 11.14 | 33.89 ± 9.74 | 35.46 ± 12.16 | 1.26 (0.532) | 0.01 | 25.74 ± 11.11 | 30.62 ± 12.01 | 36.47 ± 15.01 | 8.92 * (0.012) | 0.08 |
| Ethnicity b | 0.64 (0.728) | 0.08 | 7.86 (0.447) | 0.20 | ||||||
| Spanish | 57 (92%) | 15 (94%) | 27 (96%) | 10 (100%) | 54 (87%) | 29 (97%) | ||||
| European | 5 (8%) | 1 (6%) | 1 (4%) | 0 (0%) | 5 (8%) | 0 (0.0%) | ||||
| Others | 0 (0%) | 0 (0.0%) | 0 (0.0%) | 0 (0%) | 3 (5%) | 1 (3%) | ||||
| Psychological characteristics | Psychological characteristics | |||||||||
| Fluid reasoning, M ± SD | 29.32 ± 9.38 | 28.72 ± 9.83 | 33.43 ± 8.09 | 4.64 (0.098) | 0.04 | 31.88 ± 9.82 | 32.56 ± 8.92 | 31.70 ± 9.86 | 0.01 (0.993) | 0.00 |
| AMAS, M ± SD | 22.81 ± 6.43 | 18.67 ± 4.30 | 18.89 ± 6.87 | 11.11 ** (0.04) | 0.09 | 23.59 ± 7.26 | 21.45 ± 7.09 | 18.77 ± 5.90 | 5.19 (0.075) | 0.05 |
| Baseline outcome levels | Baseline outcome levels | |||||||||
| Verbal STM (hits), M ± SD | 7.30 ± 1.64 | 6.83 ± 1.65 | 6.96 ± 1.84 | 1.88 (0.390) | 0.02 | 7.00 ± 1.90 | 7.41 ± 1.75 | 8.20 ± 2.04 | 5.06 (0.080) | 0.05 |
| Verbal STM (span), M ± SD | 5.03 ± 0.99 | 4.78 ± 0.94 | 4.96 ± 0.96 | 1.02 (0.600) | 0.01 | 4.81 ± 1.11 | 5.18 ± 1.04 | 5.50 ± 1.11 | 4.49 (0.106) | 0.04 |
| VS STM (hits), M ± SD | 6.49 ± 1.56 | 6.89 ± 1.53 | 6.93 ± 1.76 | 0.80 (0.669) | 0.01 | 7.06 ± 1.73 | 6.67 ± 1.49 | 7.60 ± 2.04 | 7.47 * (0.024) | 0.07 |
| VS STM (span), M ± SD | 4.78 ± 0.91 | 5.00 ± 0.77 | 5.11 ± 1.10 | 1.42 (0.492) | 0.01 | 5.00 ± 0.89 | 4.97 ± 0.98 | 5.30 ± 1.06 | 3.05 (0.218) | 0.03 |
| Verbal WM, M ± SD | 21.70 ± 4.54 | 21.89 ± 3.32 | 21.75 ± 4.28 | 0.15 (0.929) | 0.00 | 21.00 ± 4.38 | 22.26 ± 4.18 | 24.47 ± 4.08 | 9.47 * (0.009) | 0.09 |
| VS WM M ± SD | 20.88 ± 5.42 | 22.28 ± 6.14 | 22.46 ± 5.30 | 2.63 (0.268) | 0.02 | 20.31 ± 6.76 | 21.59 ± 4.88 | 24.97 ± 4.47 | 11.25 ** (0.004) | 0.10 |
| Number Operations, M ± SD | 12.52 ± 4.00 | 13.67 ± 3.11 | 16.21 ± 3.44 | 17.77 *** (<0.001) | 0.15 | 16.18 ± 4.64 | 14.80 ± 4.47 | 18.30 ± 4.32 | 11.44 ** (0.003) | 0.10 |
| Number Ranking, M ± SD | 21.44 ± 8.49 | 26.11 ± 9.59 | 30.04 ± 4.26 | 30.17 *** (<0.001) | 0.25 | 29.65 ±9.73 | 29.23 ± 9.24 | 33.47 ± 7.61 | 4.75 (0.093) | 0.04 |
| Number Production, M ± SD | 4.96 ± 3.95 | 5.22 ± 5.38 | 6.29 ± 4.66 | 1.53 (0.465) | 0.01 | 7.06 ± 4.10 | 6.61 ± 5.14 | 7.67 ± 5.14 | 1.24 (0.538) | 0.01 |
| Problem Solving, M ± SD | 4.35 ± 2.24 | 4.11 ± 2.30 | 7.00 ± 2.82 | 19.52 *** (<0.001) | 0.16 | 6.47 ± 2.83 | 5.77 ± 3.04 | 7.37 ± 3.85 | 3.69 (0.158) | 0.03 |
| Grade 3 a | Grade 4 b | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Math GTG (n = 75) | Memory GTG (n = 18) | CG (n = 28) | Time (p) | Group (p) | Timex Group (p) | Math GTG (n = 17) | Memory GTG (n = 66) | CG (n = 30) | Time (p) | Group (p) | Timex Group (p) | ||
| Verbal STM (hits) | Pre | 7.31 ± 0.18 | 6.92 ± 0.37 | 6.88 ± 0.30 | 1.12 (0.292) | 0.74 (0.480) | 0.59 (0.553) | 7.20 ± 0.50 | 7.43 ± 0.24 | 8.04 ± 0.37 | 0.12 (0.730) | 0.46 (0.630) | 0.96 (0.386) |
| Post | 7.30 ± 0.18 | 7.37 ± 0.37 | 6.99 ± 0.30 | 7.58 ± 0.50 | 7.68 ± 0.24 | 7.67 ± 0.37 | |||||||
| Verbal STM (span) | Pre | 5.03 ± 0.11 | 4.84 ± 0.22 | 4.91 ± 0.17 | 1.34 (0.250) | 0.71 (0.493) | 0.62 (0.539) | 4.92 ± 0.25 | 5.20 ± 0.12 | 5.41 ± 0.19 | 0.89 (0.349) | 0.79 (0.458) | 0.59 (0.554) |
| Post | 5.14 ± 0.11 | 5.17 ± 0.22 | 4.87 ± 0.17 | 5.17 ± 0.25 | 5.35 ± 0.12 | 5.35 ± 0.19 | |||||||
| VS STM (hits) | Pre | 6.53 ± 0.18 | 6.94 ± 0.37 | 6.80 ± 0.30 | 4.66 * (0.033) | 0.66 (0.517) | 3.32 * (0.040) | 7.06 ± 0.38 | 6.66 ± 0.19 | 7.61 ± 0.28 | 3.96 * (0.049) | 4.90 ** (0.009) | 0.53 (0.590) |
| Post | 7.35 ± 0.18 | 7.44 ± 0.37 | 6.66 ± 0.30 | 7.19 ± 0.38 | 7.28 ± 0.19 | 8.04 ± 0.28 | |||||||
| VS STM (span) | Pre | 4.82 ± 0.11 | 5.03 ± 0.22 | 5.02 ± 0.18 | 3.25 (0.074) | 0.74 (0.481) | 5.19 ** (0.007) | 4.99 ± 0.24 | 4.97 ± 0.12 | 5.30 ± 0.17 | 3.07 (0.082) | 3.55 * (0.032) | 0.57 (0.566) |
| Post | 5.44 ± 0.11 | 5.25 ± 0.22 | 4.84 ± 0.18 | 4.99 ± 0.24 | 5.33 ± 0.12 | 5.70 ± 0.17 | |||||||
| Verbal WM | Pre | 22.09 ± 0.90 | 21.78 ± 0.44 | 21.42 ± 0.73 | 13.30 *** (<0.001) | 0.21 (0.808) | 0.24 (0.790) | 21.32 ± 1.01 | 22.30 ± 0.50 | 24.21 ± 0.74 | 9.30 * (0.003) | 1.97 (0.144) | 1.27 (0.284) |
| Post | 23.93 ± 0.90 | 23.23 ± 0.44 | 23.56 ± 0.73 | 23.82 ± 1.01 | 24.03 ± 0.50 | 24.64 ± 0.74 | |||||||
| VS WM | Pre | 21.23 ± 0.57 | 22.30 ± 1.15 | 21.66 ± 0.93 | 13.72 *** (<0.001) | 0.80 (0.452) | 3.55 * (0.032) | 20.81 ± 1.09 | 21.67 ± 0.53 | 24.53 ± 0.80 | 33.65 *** (<0.001) | 5.10 ** (0.008) | 0.54 (0.583) |
| Post | 24.38 ± 0.57 | 24.46 ± 1.15 | 22.01 ± 0.93 | 24.25 ± 1.09 | 24.85 ± 0.53 | 26.76 ± 0.80 | |||||||
| Number Operations | Pre | 12.76 ± 0.44 | 13.63 ± 0.89 | 15.56 ± 0.72 | 61.68 *** (<0.001) | 3.06 (0.050) | 3.73 * (0.027) | 16.51 ± 1.00 | 14.81 ± 0.50 | 18.06 ± 0.76 | 42.20 *** (<0.001) | 9.33 *** (<0.001) | 0.50 (0.606) |
| Post | 16.96 ± 0.44 | 16.29 ± 1.04 | 17.96 ± 0.72 | 18.63 ± 1.00 | 17.36 ± 0.53 | 21.23 ± 0.77 | |||||||
| Number Ranking | Pre | 21.69 ± 0.77 | 26.39 ± 1.58 | 29.22 ± 1.28 | 48.78 *** (<0.001) | 7.13 ** (0.001) | 6.22 ** (0.003) | 30.86 ± 2.00 | 29.35 ± 1.00 | 32.45 ± 1.52 | 37.26 *** (<0.001) | 2.44 (0.0.92) | 0.24 (0.789) |
| Post | 31.57 ± 0.78 | 32.41 ± 1.97 | 33.11 ± 1.28 | 35.62 ± 2.00 | 34.90 ± 1.08 | 38.90 ± 1.54 | |||||||
| Number Production | Pre | 5.28 ± 0.53 | 5.25 ± 1.09 | 5.39 ± 0.88 | 22.97 *** (<0.001) | 0.06 (0.943) | 0.09 (0.914) | 7.69 ± 1.16 | 6.63 ± 0.58 | 7.24 ± 0.88 | 60.92 *** (<0.001) | 3.48 * (0.034) | 8.00 *** (<0.001) |
| Post | 7.78 ± 0.54 | 7.30 ± 1.28 | 8.04 ± 0.88 | 10.81 ± 1.16 | 8.36 ± 0.61 | 12.53 ± 0.89 | |||||||
| Problem Solving | Pre | 4.53 ± 0.26 | 4.19 ± 0.54 | 6.48 ± 0.43 | 8.08 ** (0.005) | 7.36 *** (<0.001) | 1.71 (0.185) | 6.80 ± 0.68 | 5.77 ± 0.34 | 7.17 ± 0.52 | 27.40 *** (<0.001) | 1.13 (0.327) | 3.25 * (0.043) |
| Post | 5.88 ± 0.27 | 5.10 ± 0.66 | 6.80 ± 0.43 | 7.68 ± 0.68 | 7.89 ± 0.36 | 8.18 ± 0.52 | |||||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Estrada-Plana, V.; Martínez-Escribano, A.; Ros-Morente, A.; Mayoral, M.; Castro-Quintas, A.; Vita-Barrull, N.; Terés-Lleida, N.; March-Llanes, J.; Badia-Bafalluy, A.; Moya-Higueras, J. Benefits of Playing at School: Filler Board Games Improve Visuospatial Memory and Mathematical Skills. Brain Sci. 2024, 14, 642. https://doi.org/10.3390/brainsci14070642
Estrada-Plana V, Martínez-Escribano A, Ros-Morente A, Mayoral M, Castro-Quintas A, Vita-Barrull N, Terés-Lleida N, March-Llanes J, Badia-Bafalluy A, Moya-Higueras J. Benefits of Playing at School: Filler Board Games Improve Visuospatial Memory and Mathematical Skills. Brain Sciences. 2024; 14(7):642. https://doi.org/10.3390/brainsci14070642
Chicago/Turabian StyleEstrada-Plana, Verónica, Andrea Martínez-Escribano, Agnès Ros-Morente, Maria Mayoral, Agueda Castro-Quintas, Nuria Vita-Barrull, Núria Terés-Lleida, Jaume March-Llanes, Ares Badia-Bafalluy, and Jorge Moya-Higueras. 2024. "Benefits of Playing at School: Filler Board Games Improve Visuospatial Memory and Mathematical Skills" Brain Sciences 14, no. 7: 642. https://doi.org/10.3390/brainsci14070642
APA StyleEstrada-Plana, V., Martínez-Escribano, A., Ros-Morente, A., Mayoral, M., Castro-Quintas, A., Vita-Barrull, N., Terés-Lleida, N., March-Llanes, J., Badia-Bafalluy, A., & Moya-Higueras, J. (2024). Benefits of Playing at School: Filler Board Games Improve Visuospatial Memory and Mathematical Skills. Brain Sciences, 14(7), 642. https://doi.org/10.3390/brainsci14070642







