A Multicloud Model for Coastal Convection
Abstract
:1. Introduction
2. The Numerical Model
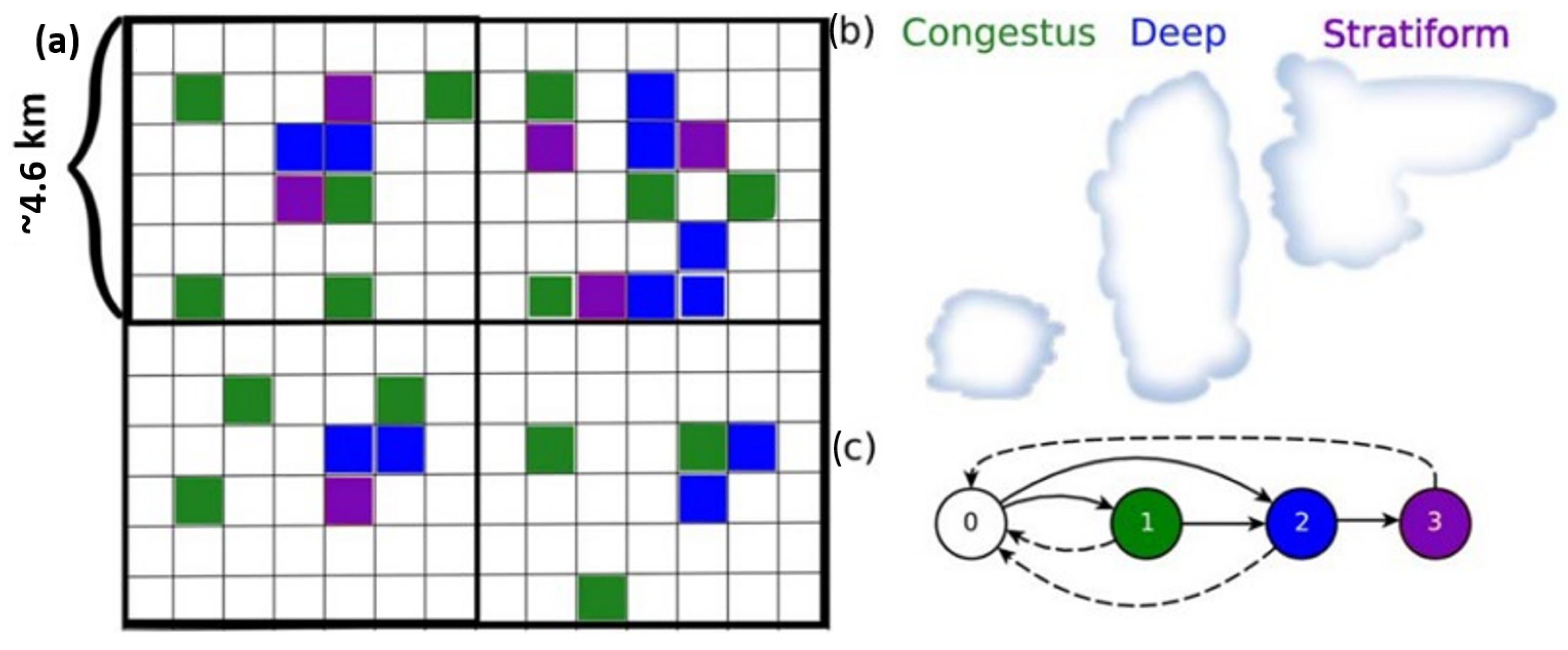
2.1. The Stochastic Multicloud Model (SMCM) Parameterization
- Convective available potential energy (CAPE): ;
- CAPE integrated over the lower troposphere: ;
- mid-troposphere dryness: , where is the equivalent potential temperature in the mid-troposphere and is a parameter whose value is given in Table 1.
- A clear site turns into a congestus site with high probability if low-level CAPE is positive and the middle troposphere is dry;
- A congestus or clear sky turns into a deep convective site with high probability if CAPE is positive and the middle troposphere is moist;
- A deep convective site turns into a stratiform site with high probability;
- All three cloud types decay naturally to clear sky at some fixed rate;
- All other transitions are assumed to have negligible probability.
- lattice sites for each macroscopic/model gridbox;
- macroscopic grid box dimension = 4.59 km.
2.2. The Simplified 2D Coastal Model
2.3. Model Setup
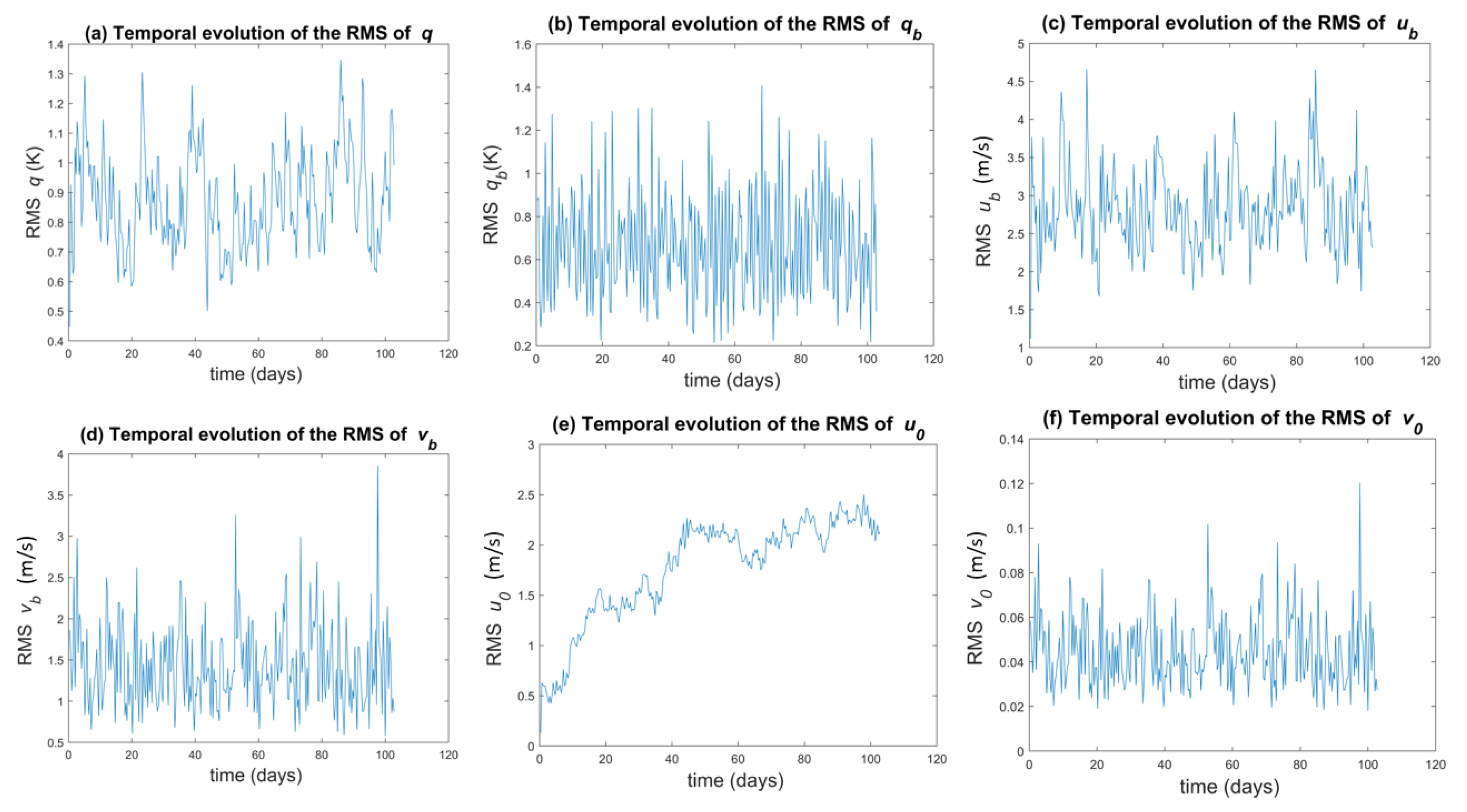
3. Data and Methodology
3.1. The Raw Data
3.2. Spectral Analysis
4. The Physical Features of the Main Modes of Variability in Coastal Convection
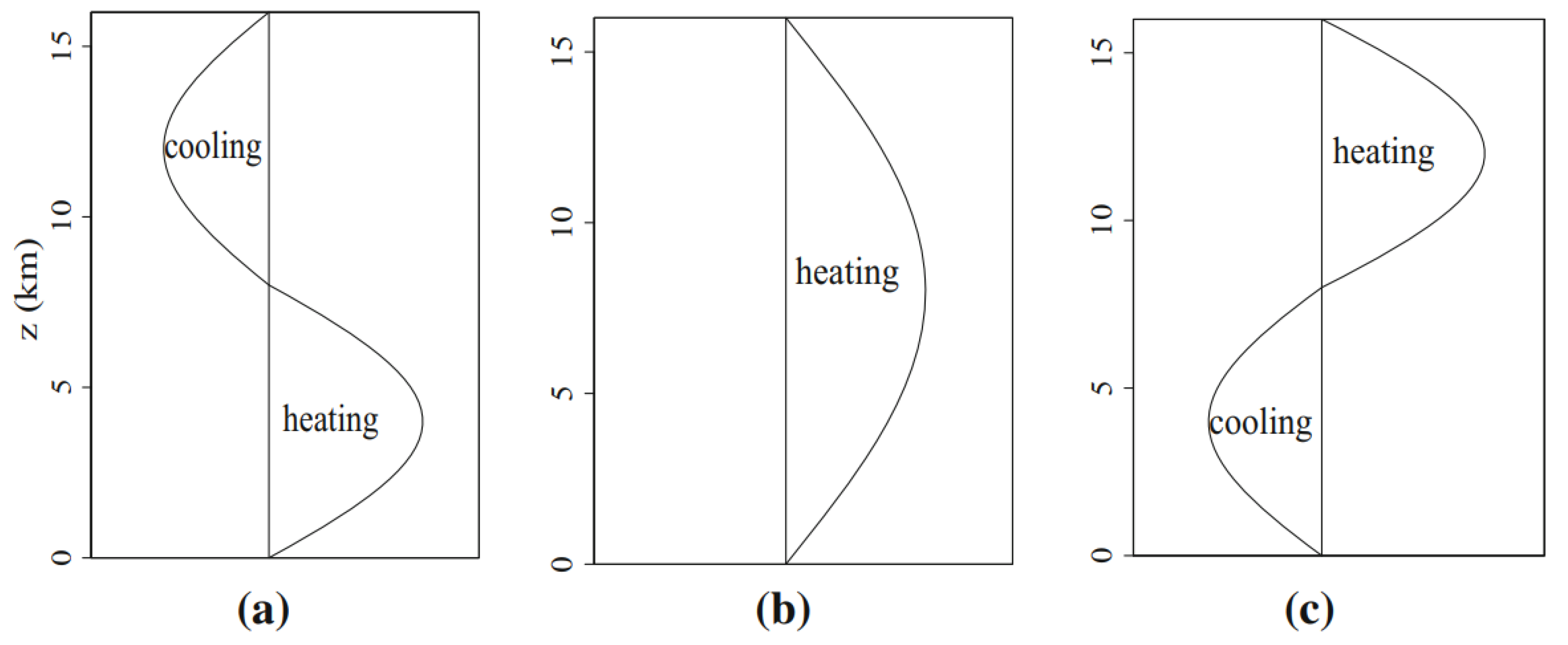
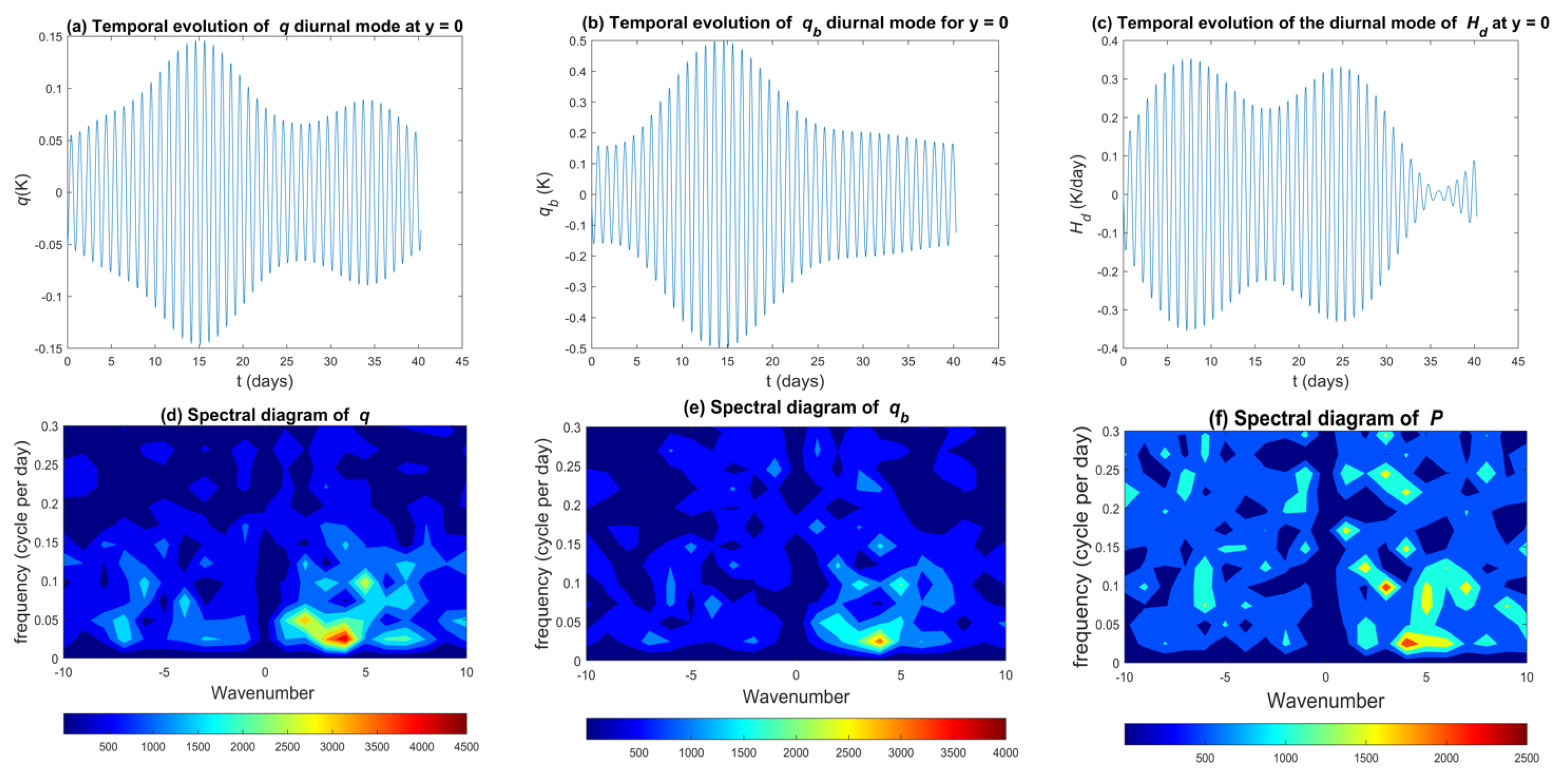
4.1. The Diurnal Mode
Slow Modulation and Transition from Hyperbolic to Elliptic Behavior
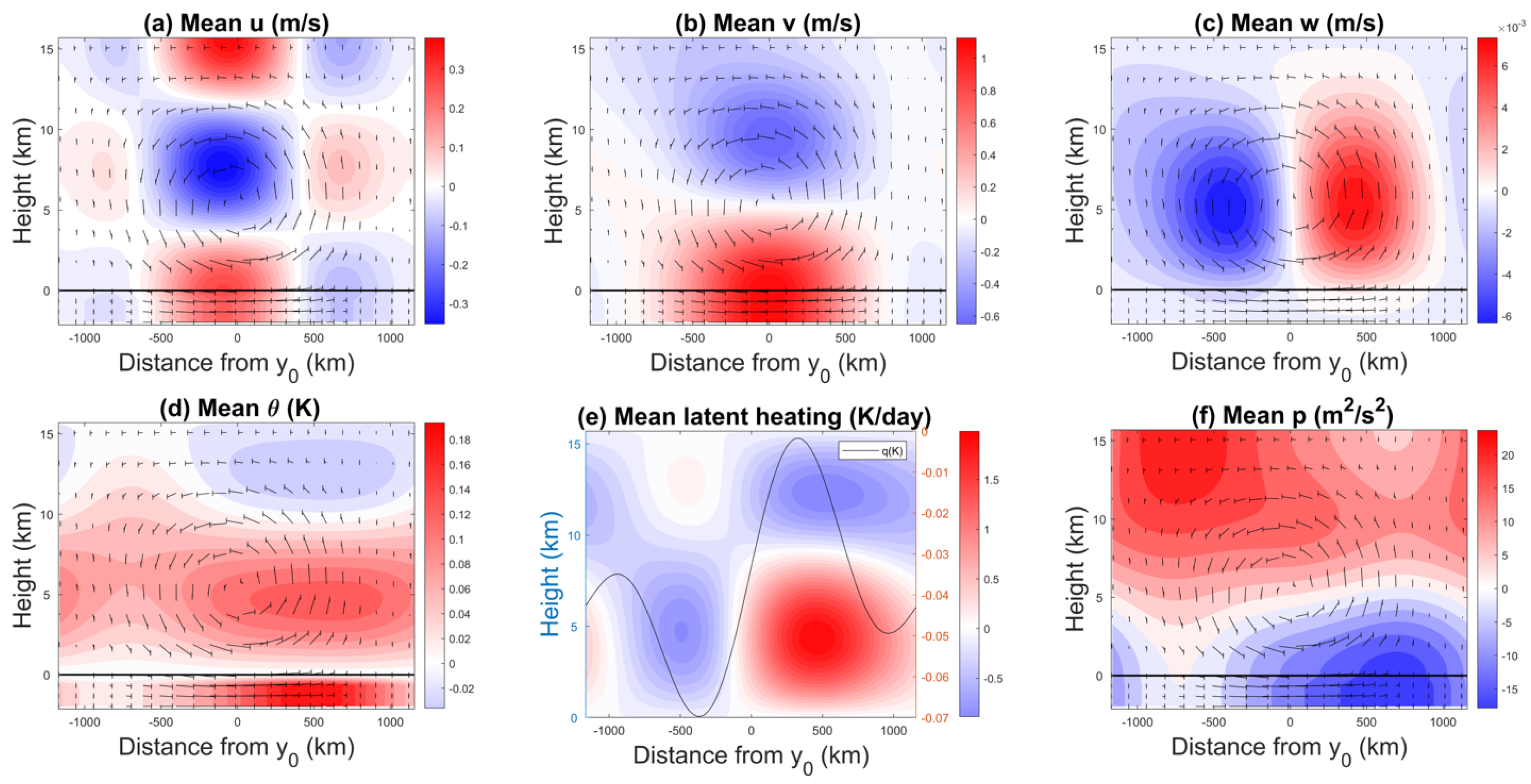
4.2. Dynamical and Thermodynamical Structure of the Diurnal Mode
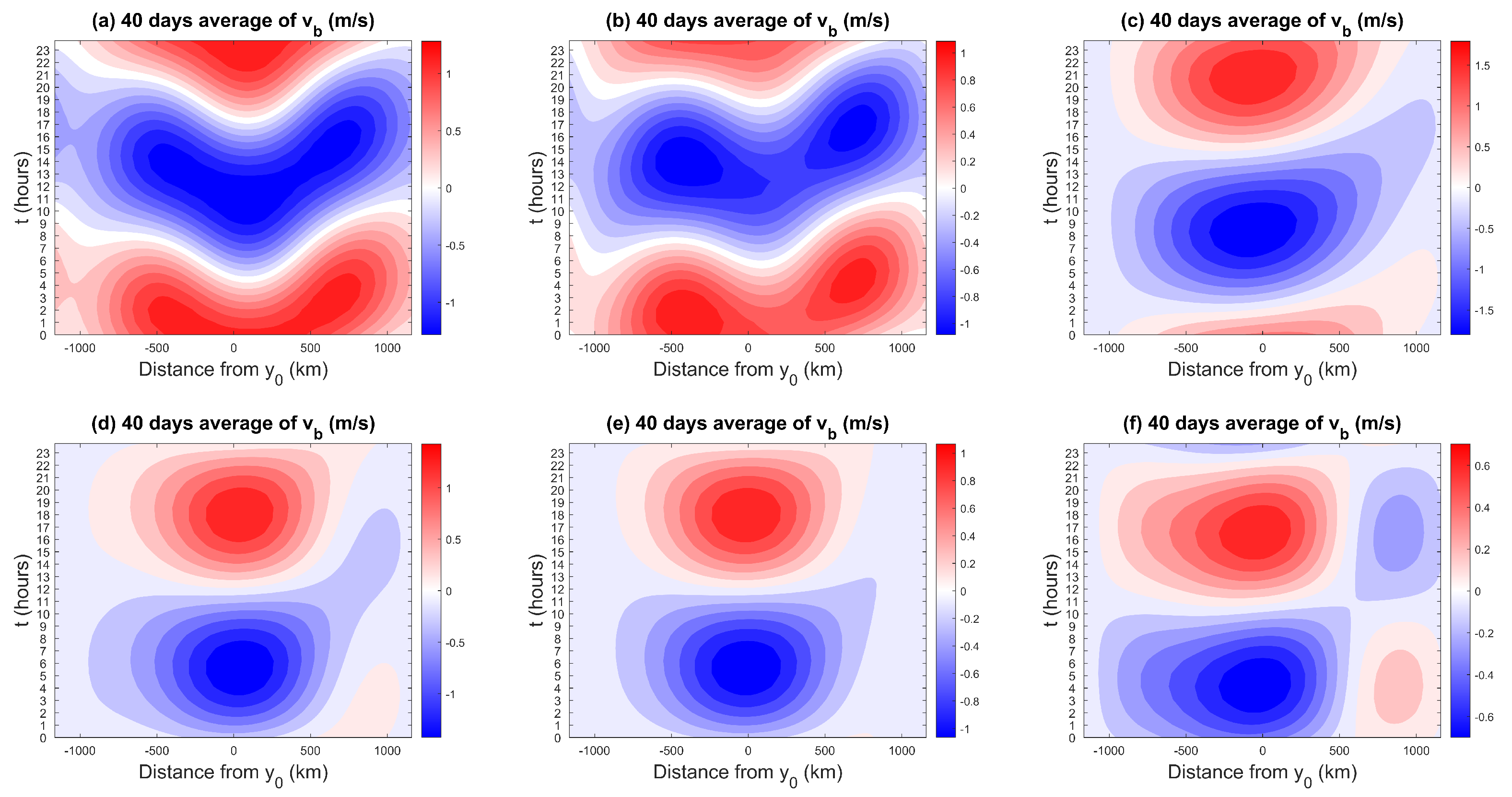
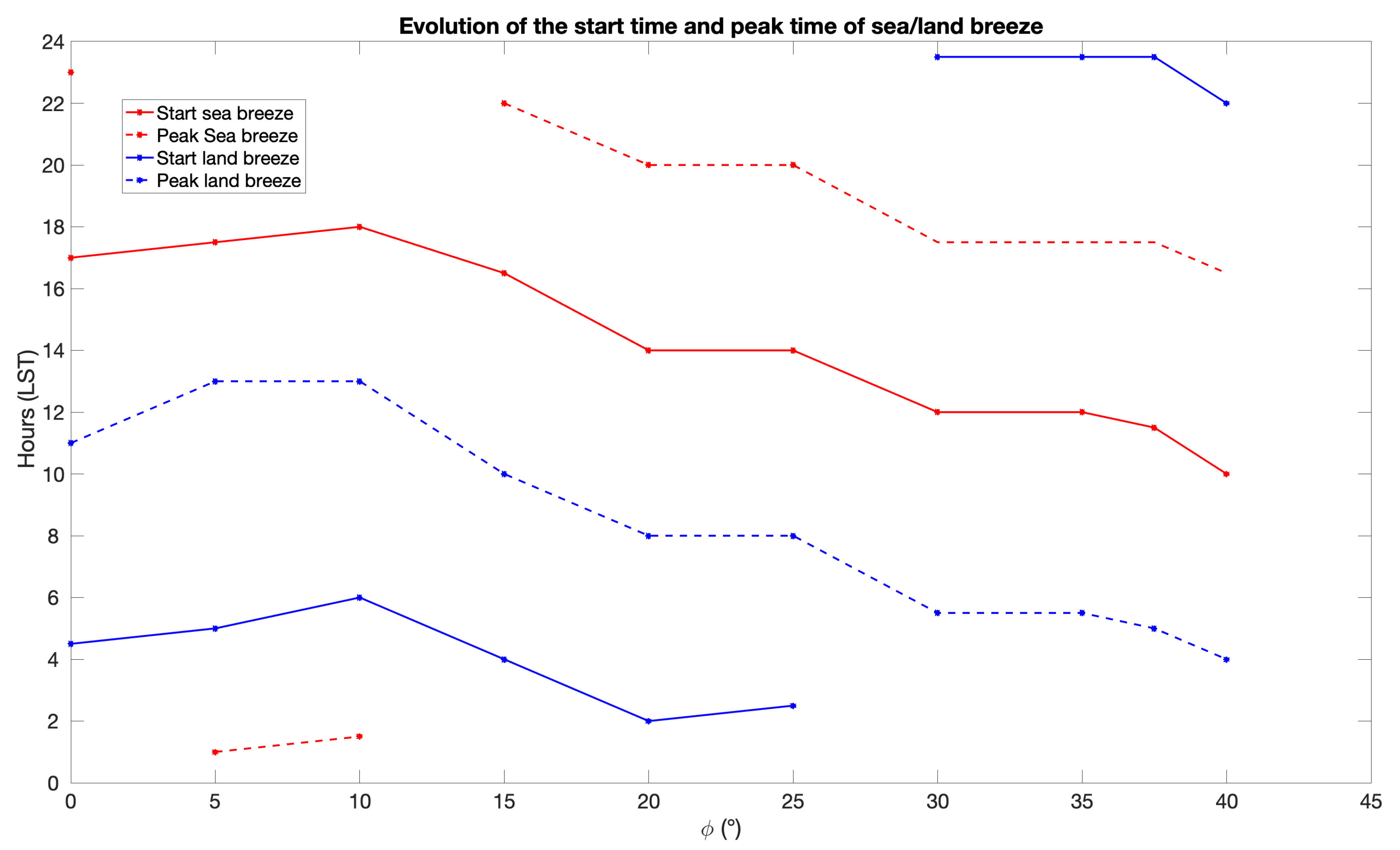
4.2.1. Sea and Land Breeze Initiation and Peak Times, and Physical Mechanisms of the Diurnal Mode
4.2.2. Sea and Land Breeze Penetration
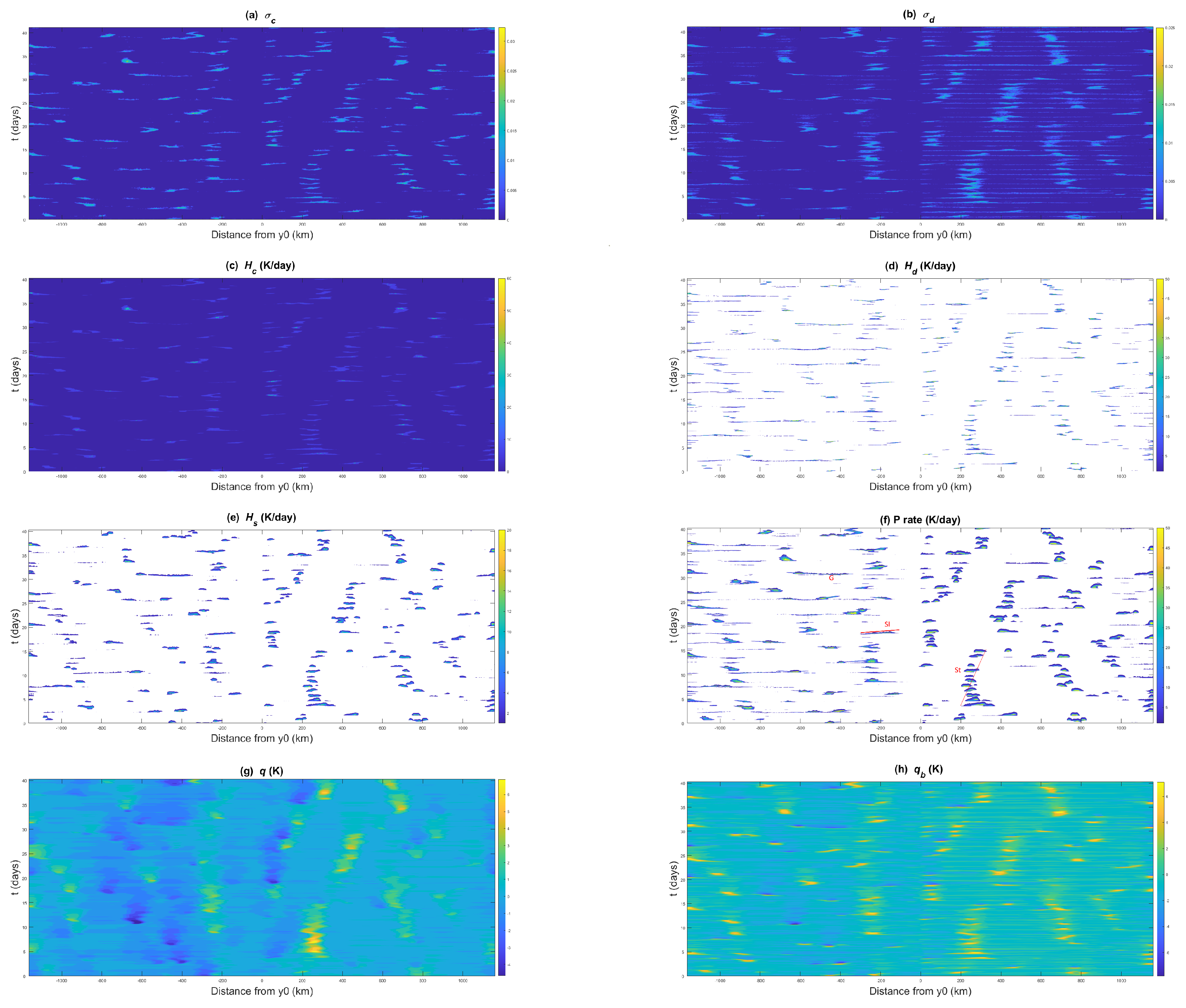
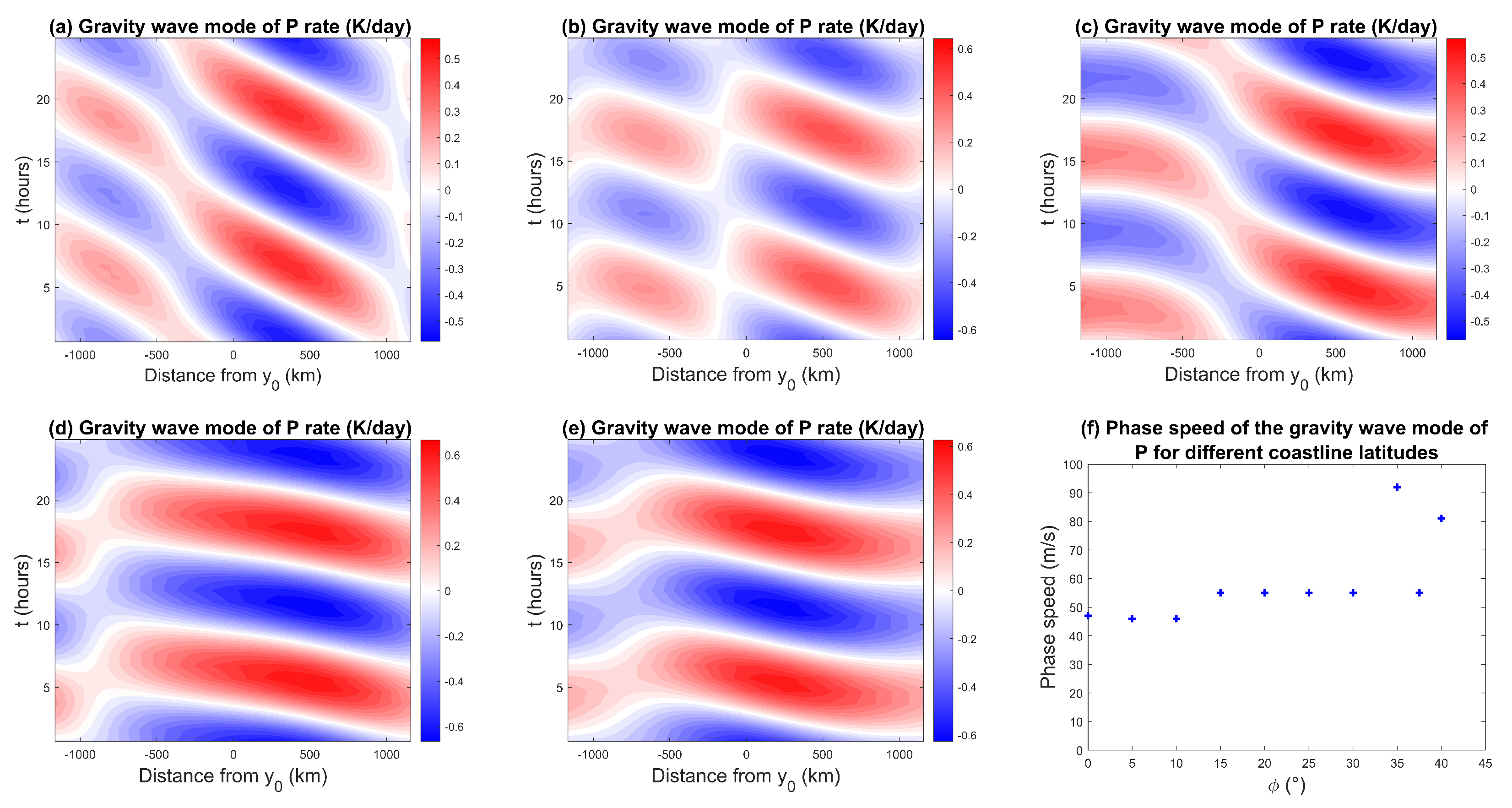
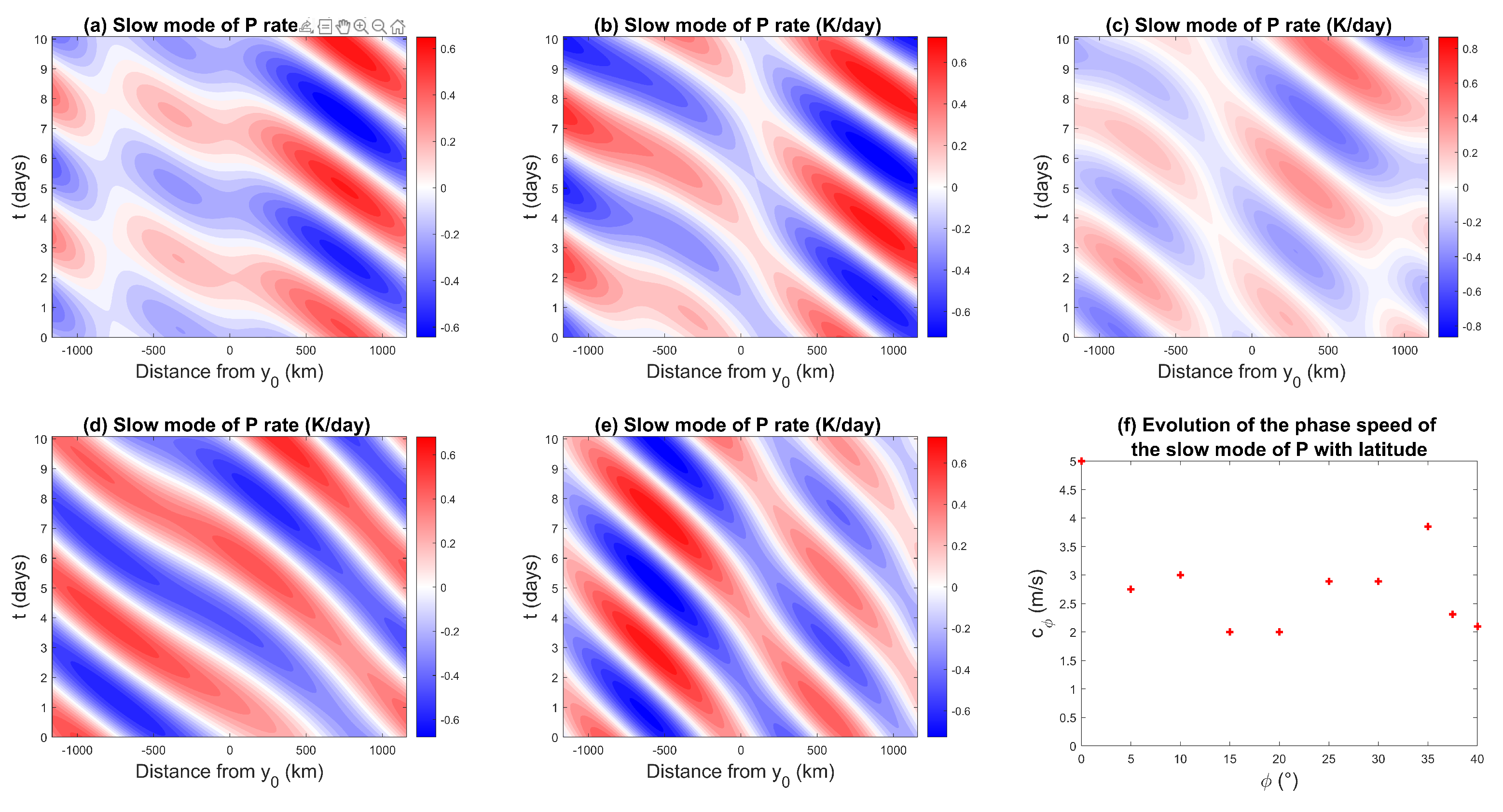
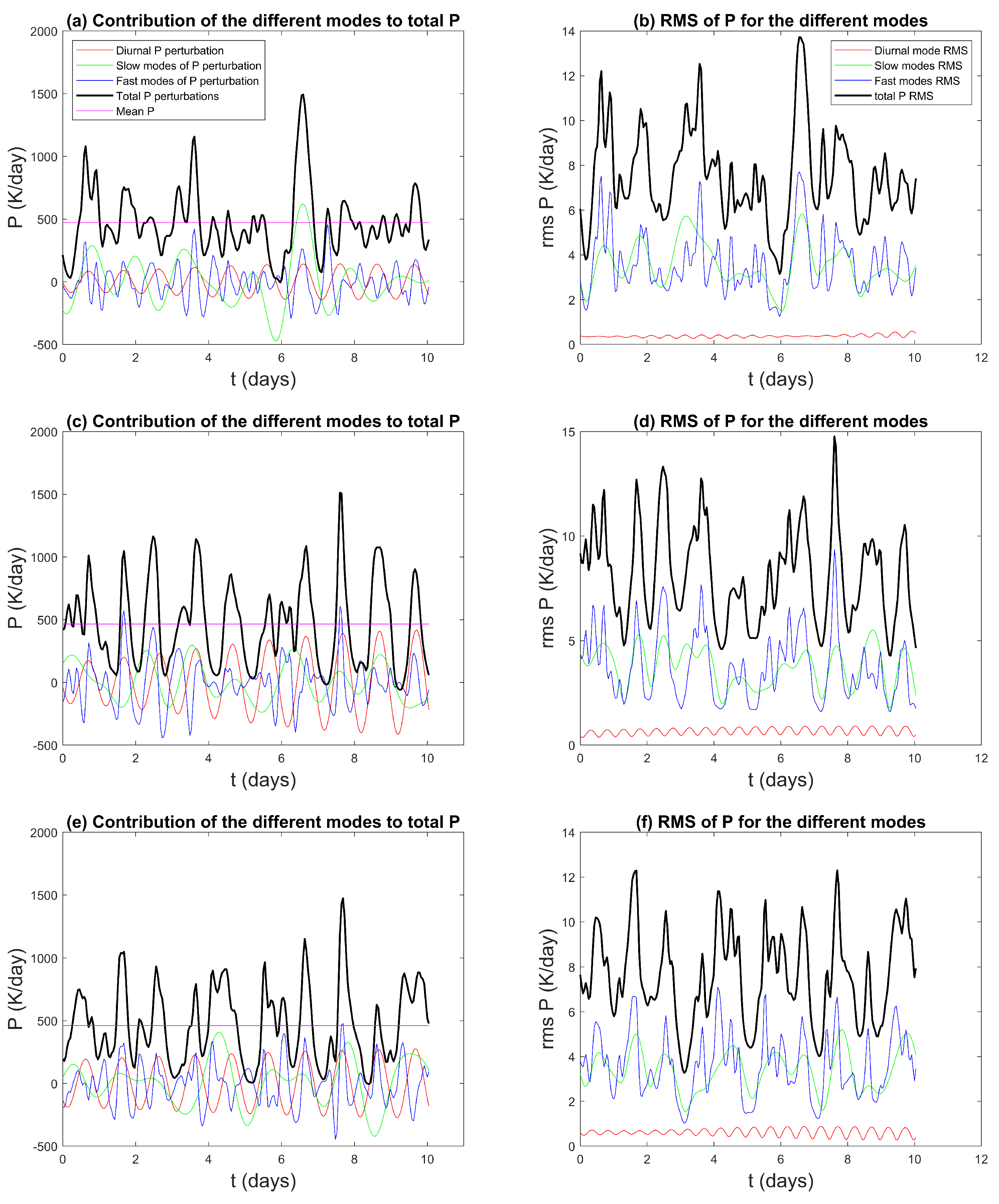
4.3. Fast and Slow Modes
Relative Precipitation Contributions
5. Conclusion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Tao, C.; Xie, S.; Tang, S.; Lee, J.; Ma, H.Y.; Zhang, C.; Lin, W. Diurnal cycle of precipitation over global monsoon systems in CMIP6 simulations. Clim. Dyn. 2022, 60, 3947–3968. [Google Scholar] [CrossRef]
- Xie, S.; Wang, Y.C.; Lin, W.; Ma, H.Y.; Tang, Q.; Tang, S.; Zheng, X.; Golaz, J.C.; Zhang, G.J.; Zhang, M. Improved Diurnal Cycle of Precipitation in E3SM With a Revised Convective Triggering Function. J. Adv. Model. Earth Syst. 2019, 11, 2290–2310. [Google Scholar] [CrossRef]
- Tang, S.; Xie, S.; Guo, Z.; Hong, S.Y.; Khouider, B.; Klocke, D.; Köhler, M.; Koo, M.S.; Krishna, P.M.; Larson, V.E.; et al. Long-term single-column model intercomparison of diurnal cycle of precipitation over midlatitude and tropical land. Q. J. R. Meteorol. Soc. 2021, 148, 641–669. [Google Scholar] [CrossRef]
- Bergemann, M.; Jakob, C.; Lane, T.P. Global Detection and Analysis of Coastline-Associated Rainfall Using an Objective Pattern Recognition Technique. J. Clim. 2015, 28, 7225–7236. [Google Scholar] [CrossRef]
- Gray, W.M.; Jacobson, R.W., Jr. Diurnal variation of deep cumulus convection. Mon. Weather. Rev. 1977, 105, 1171–1188. [Google Scholar] [CrossRef]
- Albright, M.D.; Recker, E.E.; Reed, R.J.; Dang, R. The diurnal variation of deep convection and inferred precipitation in the central tropical Pacific during January–February 1979. Mon. Weather. Rev. 1985, 113, 1663–1680. [Google Scholar] [CrossRef]
- Sui, C.; Lau, K.; Takayabu, Y.; Short, D. Diurnal variations in tropical oceanic cumulus convection during TOGA COARE. J. Atmos. Sci. 1997, 54, 639–655. [Google Scholar] [CrossRef]
- Garreaud, R.; Wallace, J.M. The diurnal march of convective cloudiness over the Americas. Mon. Weather. Rev. 1997, 125, 3157–3171. [Google Scholar] [CrossRef]
- Yang, G.Y.; Slingo, J. The diurnal cycle in the tropics. Mon. Weather. Rev. 2001, 129, 784–801. [Google Scholar] [CrossRef]
- Mapes, B.E.; Warner, T.T.; Xu, M. Diurnal Patterns of Rainfall in Northwestern South America. Part III: Diurnal Gravity Waves and Nocturnal Convection Offshore. Mon. Weather. Rev. 2003, 131, 830–844. [Google Scholar] [CrossRef]
- Yepes, J.; Mejía, J.F.; Mapes, B.; Poveda, G. Gravity Waves and Other Mechanisms Modulating the Diurnal Precipitation over One of the Rainiest Spots on Earth: Observations and Simulations in 2016. Mon. Weather. Rev. 2020, 148, 3933–3950. [Google Scholar] [CrossRef]
- Shin, K.S.; North, G.R.; Ahn, Y.S.; Arkin, P.A. Time scales and variability of area-averaged tropical oceanic rainfall. Mon. Weather. Rev. 1990, 118, 1507–1516. [Google Scholar] [CrossRef]
- Buchan, A. A Handy Book of Meteorology; Kessinger Pub Co: Whitefish, MT, USA, 1860. [Google Scholar]
- Halley, E. An historical account of the trade winds, and monsoons, observable in the seas between and near the Tropicks, with an attempt to assign the physical cause of the said winds. Philos. Trans. R. Soc. Lond. 1753, 16, 153–168. [Google Scholar] [CrossRef]
- Dampier, W. The Islands Galapagos (1697). In The Essential Naturalist: Timeless Readings in Natural History; University of Chicago: Chicago, IL, USA, 2011; p. 113. [Google Scholar]
- Dorman, C.E. Winds between San Diego and San Clemente Island. J. Geophys. Res. Ocean. 1982, 87, 9636–9646. [Google Scholar] [CrossRef]
- Simpson, J.E. Sea Breeze and Local Winds; Cambridge University Press: Cambridge, UK, 1994. [Google Scholar]
- Clarke, R. Some observations and comments on the seabreeze. Aust. Meteorol. Mag. 1955, 11, 47–68. [Google Scholar]
- Kurita, H.; Sasaki, K.; Muroga, H.; Ueda, H.; Wakamatsu, S. Long-range transport of air pollution under light gradient wind conditions. J. Appl. Meteorol. Climatol. 1985, 24, 425–434. [Google Scholar] [CrossRef]
- Haurwitz, B. Comments on the sea-breeze circulation. J. Atmos. Sci. 1947, 4, 1–8. [Google Scholar] [CrossRef]
- Schmidt, F. An elementary theory of the land-and sea-breeze circulation. J. Atmos. Sci. 1947, 4, 9–20. [Google Scholar] [CrossRef]
- Defant, F. Local winds. In Compendium of meteorology; Springer: Berlin/Heidelberg, Germany, 1951; pp. 655–672. [Google Scholar]
- Walsh, J.E. Sea breeze theory and applications. J. Atmos. Sci. 1974, 31, 2012–2026. [Google Scholar] [CrossRef]
- Sun, W.Y.; Orlanski, I. Large mesoscale convection and sea breeze circulation. Part I: Linear stability analysis. J. Atmos. Sci. 1981, 38, 1675–1693. [Google Scholar] [CrossRef]
- Rotunno, R. On the linear theory of the land and sea breeze. J. Atmos. Sci. 1983, 40, 1999–2009. [Google Scholar] [CrossRef]
- Ohsawa, T.; Ueda, H.; Hayashi, T.; Watanabe, A.; Matsumoto, J. Diurnal variations of convective activity and rainfall in tropical Asia. J. Meteorol. Soc. Jpn. Ser. II 2001, 79, 333–352. [Google Scholar] [CrossRef]
- Biasutti, M. Forced Sahel rainfall trends in the CMIP5 archive. J. Geophys. Res. Atmos. 2013, 118, 1613–1623. [Google Scholar] [CrossRef]
- Yokoi, S.; Mori, S.; Syamsudin, F.; Haryoko, U.; Geng, B. Environmental conditions for nighttime offshore migration of precipitation area as revealed by in situ observation off Sumatra Island. Mon. Weather. Rev. 2019, 147, 3391–3407. [Google Scholar] [CrossRef]
- Bai, H.; Deranadyan, G.; Schumacher, C.; Funk, A.; Epifanio, C.; Ali, A.; Endarwin.; Radjab, F.; Adriyanto, R.; Nurhayati, N.; et al. Formation of nocturnal offshore rainfall near the west coast of Sumatra: Land breeze or gravity wave? Mon. Weather. Rev. 2021, 149, 715–731. [Google Scholar] [CrossRef]
- Gregory, D.; Rowntree, P. A mass flux convection scheme with representation of cloud ensemble characteristics and stability-dependent closure. Mon. Weather. Rev. 1990, 118, 1483–1506. [Google Scholar] [CrossRef]
- Tiedtke, M. Representation of clouds in large-scale models. Mon. Weather. Rev. 1993, 121, 3040–3061. [Google Scholar] [CrossRef]
- Warner, T.T.; Mapes, B.E.; Xu, M. Diurnal patterns of rainfall in northwestern South America. Part II: Model simulations. Mon. Weather. Rev. 2003, 131, 813–829. [Google Scholar] [CrossRef]
- Vincent, C.L.; Lane, T.P. Evolution of the diurnal precipitation cycle with the passage of a Madden–Julian oscillation event through the Maritime Continent. Mon. Weather. Rev. 2016, 144, 1983–2005. [Google Scholar] [CrossRef]
- Bergemann, M.; Khouider, B.; Jakob, C. Coastal tropical convection in a stochastic modeling framework. J. Adv. Model. Earth Syst. 2017, 9, 2561–2582. [Google Scholar] [CrossRef]
- Khouider, B.; Biello, J.; Majda, A.J. A stochastic multicloud model for tropical convection. Commun. Math. Sci. 2010, 8, 187–216. [Google Scholar]
- Khouider, B.; Majda, A.J. A simple multicloud parameterization for convectively coupled tropical waves. Part I: Linear analysis. J. Atmos. Sci. 2006, 63, 1308–1323. [Google Scholar] [CrossRef]
- Peters, K.; Crueger, T.; Jakob, C.; Möbis, B. Improved MJO-simulation in ECHAM 6.3 by coupling a S tochastic M ulticloud M odel to the convection scheme. J. Adv. Model. Earth Syst. 2017, 9, 193–219. [Google Scholar] [CrossRef]
- Goswami, B.; Khouider, B.; Phani, R.; Mukhopadhyay, P.; Majda, A. Implementation and calibration of a stochastic multicloud convective parameterization in the ncep c limate f orecast s ystem (cfsv2). J. Adv. Model. Earth Syst. 2017, 9, 1721–1739. [Google Scholar] [CrossRef]
- Waite, M.L.; Khouider, B. Boundary layer dynamics in a simple model for convectively coupled gravity waves. J. Atmos. Sci. 2009, 66, 2780–2795. [Google Scholar] [CrossRef]
- Frenkel, Y.; Khouider, B.; Majda, A.J. Simple multicloud models for the diurnal cycle of tropical precipitation. Part I: Formulation and the case of the tropical oceans. J. Atmos. Sci. 2011, 68, 2169–2190. [Google Scholar] [CrossRef]
- Frenkel, Y.; Khouider, B.; Majda, A.J. Simple Multicloud Models for the Diurnal Cycle of Tropical Precipitation. Part II: The Continental Regime. J. Atmos. Sci. 2011, 68, 2192–2207. [Google Scholar] [CrossRef]
- De La Chevrotière, M.; Khouider, B. A zonally symmetric model for the monsoon-Hadley circulation with stochastic convective forcing. Theor. Comput. Fluid Dyn. 2017, 31, 89–110. [Google Scholar] [CrossRef]
- Yang, Q.; Khouider, B.; Majda, A.J.; De La Chevrotière, M. Northward propagation, initiation, and termination of boreal summer intraseasonal oscillations in a zonally symmetric model. J. Atmos. Sci. 2019, 76, 639–668. [Google Scholar] [CrossRef]
- Johnson, R.H.; Rickenbach, T.M.; Rutledge, S.A.; Ciesielski, P.E.; Schubert, W.H. Trimodal characteristics of tropical convection. J. Clim. 1999, 12, 2397–2418. [Google Scholar] [CrossRef]
- Houze, R.A., Jr. Mesoscale convective systems. Rev. Geophys. 2004, 42, 4. [Google Scholar] [CrossRef]
- Roy, K.; Mukhopadhyay, P.; Krishna, R.P.M.; Khouider, B.; Goswami, B.B.; Pandithurai, G. Evaluation of mean state in NCEP Climate Forecast System (version 2) simulation using a Stochastic Multicloud Model calibrated with Indian RADAR data. J. Adv. Earth Sys. Model. 2022, 8, e2020EA001455. [Google Scholar]
- Khouider, B.; Goswami, B.B.; Krishna, P.M.; Majda, A.J. A shallow-deep unified stochastic mass flux cumulus parameterization in the single column Community Climate Model. J. Adv. Modeling Earth Syst. 2022. submitted. [Google Scholar] [CrossRef]
- Da Rocha, H.R.; Goulden, M.L.; Miller, S.D.; Menton, M.C.; Pinto, L.D.; de Freitas, H.C.; e Silva Figueira, A.M. Seasonality of water and heat fluxes over a tropical forest in eastern Amazonia. Ecol. Appl. 2004, 14, 22–32. [Google Scholar] [CrossRef]
- Khouider, B. A coarse grained stochastic multi-type particle interacting model for tropical convection: Nearest neighbour interactions. Comm. Math. Sci. 2014, 12, 1379–1407. [Google Scholar] [CrossRef]
- Khouider, B. Models for Tropical Climate Dynamics: Waves, Clouds, and Precipitation; Springer: Berlin/Heidelberg, Germany, 2019; Volume 3. [Google Scholar]
- Strang, G. On the construction and comparison of difference schemes. Siam J. Numer. Anal. 1968, 5, 506–517. [Google Scholar] [CrossRef]
- De La Chevrotière, M. Stochastic and Numerical Models for Tropical Convection and Hadley–Monsoon Dynamics. Ph.D. Thesis, University of Victoria, Victoria, BC, Canada, 2015. [Google Scholar]
- Khouider, B.; Majda, A.J. Multicloud convective parametrizations with crude vertical structure. Theor. Comput. Fluid Dyn. 2006, 20, 351–375. [Google Scholar] [CrossRef]
- Mahrer, Y.; Pielke, R.A. The effects of topography on sea and land breezes in a two-dimensional numerical model. Mon. Weather. Rev. 1977, 105, 1151–1162. [Google Scholar] [CrossRef]
- Rafiq, S.; Pattiaratchi, C.; Janeković, I. Dynamics of the Land–Sea Breeze System and the Surface Current Response in South-West Australia. J. Mar. Sci. Eng. 2020, 9, 931. [Google Scholar] [CrossRef]
- Miller, S.; Keim, B.; Talbot, R.; Mao, H. Sea breeze: Structure, forecasting, and impacts. Rev. Geophys. 2003, 41, 3. [Google Scholar] [CrossRef]
- Eager, R.E.; Raman, S.; Wootten, A.; Westphal, D.L.; Reid, J.S.; Al Mandoos, A. A climatological study of the sea and land breezes in the Arabian Gulf region. J. Geophys. Res. Atmos. 2008, 113, D15. [Google Scholar] [CrossRef]
- Hounam, C. The sea breeze at Perth. Weather. Dev. Res. Bull. 1945, 3, 20–55. [Google Scholar]
- Lim, H.J.; Lee, Y.H. Characteristics of Sea Breezes at Coastal Area in Boseong. Atmosphere 2019, 29, 41–51. [Google Scholar] [CrossRef]
- Mori, S.; Jun-Ichi, H.; Tauhid, Y.I.; Yamanaka, M.D.; Okamoto, N.; Murata, F.; Sakurai, N.; Hashiguchi, H.; Sribimawati, T. Diurnal land–sea rainfall peak migration over Sumatera Island, Indonesian Maritime Continent, observed by TRMM satellite and intensive rawinsonde soundings. Mon. Weather. Rev. 2004, 132, 2021–2039. [Google Scholar] [CrossRef]
- Gille, S.T.; Llewellyn Smith, S.G.; Statom, N.M. Global observations of the land breeze. Geophys. Res. Lett. 2005, 32, L05605. [Google Scholar] [CrossRef]
- Katsumata, M.; Mori, S.; Hamada, J.I.; Hattori, M.; Syamsudin, F.; Yamanaka, M.D. Diurnal cycle over a coastal area of the Maritime Continent as derived by special networked soundings over Jakarta during HARIMAU2010. Prog. Earth Planet. Sci. 2018, 5, 64. [Google Scholar] [CrossRef]
- Houze, R.A.; Geotis, S.G.; Marks, F.D.; West, A.K. Winter Monsoon Convection in the Vicinity of North Borneo. Part I: Structure and Time Variation of the Clouds and Precipitation. Mon. Weather. Rev. 1981, 109, 1595–1614. [Google Scholar] [CrossRef]
- Eckart, C. Hydrodynamics of Oceans and Atmospheres; Elsevier: Amsterdam, The Netherlands, 2015. [Google Scholar]
- Neumann, J. The Coriolis Force in Relation to the Sea and Land Breezes–A Historical Note. Bull. Am. Meteorol. Soc. 1984, 65, 24–26. [Google Scholar] [CrossRef]
- Kikuchi, K.; Wang, B. Diurnal precipitation regimes in the global tropics. J. Clim. 2008, 21, 2680–2696. [Google Scholar] [CrossRef]
- Majda, A.; Shefter, M. Waves and instabilities for model tropical convective parametrizations. J. Atmos. Sci 2001, 58, 896–914. [Google Scholar] [CrossRef]





| Parameter | Value (in Dimensional Units) | Description |
|---|---|---|
| 5 km | Average height of the middle troposphere | |
| R | 320 J Kg K | CAPE constant |
| 1 K/day | Longwave first baroclinic radiative cooling rate | |
| Determined at RCE | Longwave second baroclinic radiative cooling rate | |
| Determined at RCE | Heating potential at RCE | |
| Determined at RCE | Downdraft velocity reference scale | |
| 2 h | Reference convective timescale | |
| , | 0.25, 0.5 | Contribution of CAPE to congestus, stratiform heating |
| 0.1 (ocean), 0.4 (land) | Bowen ratio [48] | |
| 1 (ocean), 7 (land) | Relative contribution of to deep convective heating anomalies | |
| 0.6 (ocean), 0.9 (land) | Relative contribution of to deep convective heating anomalies | |
| Relative contribution of q to deep convective heating anomalies | ||
| 1.7 | Contribution of to CAPE anomalies | |
| 0.1 | Relative contribution of to deep convective anomalies | |
| 2.0 | Relative contribution of to low-level CAPE anomalies | |
| 200 J K | Reference value of CAPE | |
| 10 K | Reference value of CAPE | |
| 0.1 | Relative contribution of to | |
| 0.25 | Contribution of convective downdrafts to | |
| , | 1 h, 3 h | Congestus, stratiform adjustment timescales |
| 15 days | Rayleigh drag timescale | |
| 10 days | Newtonian cooling timescale | |
| 4 h | Momentum entrainment timescale | |
| 2 h | Convective timescale | |
| 500 m | ABL, depth | |
| Determined at RCE, of O(7 h) | Surface evaporation timescale | |
| U | 2 ms | Strength of turbulent velocity |
| 0.001 | Surface drag coefficient | |
| Determined at RCE, of O(5 K/day) | ABL radiative cooling rate | |
| 0.03125 | Ratio of boundary layer depth to height of the troposphere | |
| 0.2 | Ratio of to | |
| 0.75 |
| Cloud Transition | Probability Rate | Timescale (h) |
|---|---|---|
| Formation of congestus | ||
| Decay of congestus | ||
| Conversion of congestus to deep | ||
| Formation of deep | ||
| Conversion of deep to stratiform | ||
| Decay of deep | ||
| Decay of stratiform |
| Parameter or Scaling Factors | Derivation | Approximate Value | Description |
|---|---|---|---|
| 16 km | Tropopause height | ||
| 300 K | Reference potential temperature | ||
| g | 9.8 ms | Gravitational acceleration | |
| Function of height z | Background potential temperature, in K | ||
| Brunt–Vaissälä buoyancy frequency | |||
| c | 50 ms | Horizontal velocity scale | |
| m s | Variation in Coriolis parameter | ||
| L | 1500 km | Equatorial Rossby deformation radius/Horizontal length scale | |
| T | 8.23 h | Reference timescale | |
| 15 K | Potential temperature scale | ||
| 0.17 ms | Free troposphere vertical velocity scale |
| Mode | Variable | Equation |
|---|---|---|
| Barotropic | ||
| 1st baroclinic | ||
| 2nd baroclinic | ||
| Free tropospheric moisture | q | |
| ABL dynamics | ||
| Forcing Term | Closure Equation |
|---|---|
| Momentum turbulent drag for barotropic mode | |
| Momentum turbulent drag for baroclinic modes | , j=1,2 |
| Velocity jump at top of boundary layer | |
| First baroclinic convective heating | |
| Deep convection | |
| Second baroclinic convective heating | |
| Convective available potential energy | |
| Congestus heating | |
| Stratiform heating calculated via an ODE [36] | |
| Low-level convective available potential energy | |
| Convective available potential energy at RCE | |
| Radiative cooling | |
| Total downdraft mass flux | |
| Convective updraft mass flux | |
| Large-scale and convective downdrafts mass flux | |
| Moist thermodynamic turbulent entrainment velocity at top of the ABL | |
| Momentum entrainment velocity | |
| Moisture source | |
| Boundary layer radiative cooling () |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dah, A.; Khouider, B.; Schumacher, C. A Multicloud Model for Coastal Convection. Geosciences 2023, 13, 264. https://doi.org/10.3390/geosciences13090264
Dah A, Khouider B, Schumacher C. A Multicloud Model for Coastal Convection. Geosciences. 2023; 13(9):264. https://doi.org/10.3390/geosciences13090264
Chicago/Turabian StyleDah, Abigail, Boualem Khouider, and Courtney Schumacher. 2023. "A Multicloud Model for Coastal Convection" Geosciences 13, no. 9: 264. https://doi.org/10.3390/geosciences13090264
APA StyleDah, A., Khouider, B., & Schumacher, C. (2023). A Multicloud Model for Coastal Convection. Geosciences, 13(9), 264. https://doi.org/10.3390/geosciences13090264








