Probabilistic Analyses of Root-Reinforced Slopes Using Monte Carlo Simulation
Abstract
:1. Introduction
2. Materials and Methods
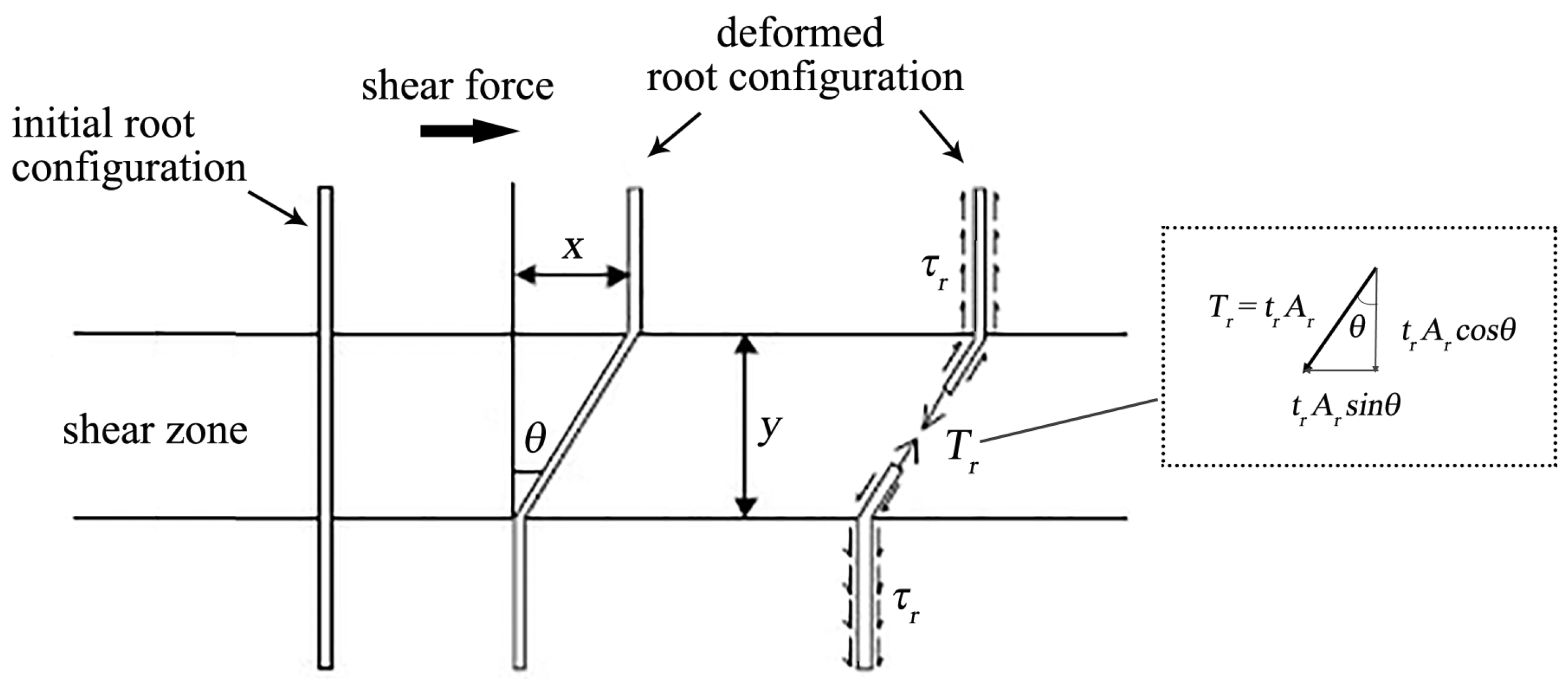
2.1. Root Reinforcement
2.2. Input Parameters Variability
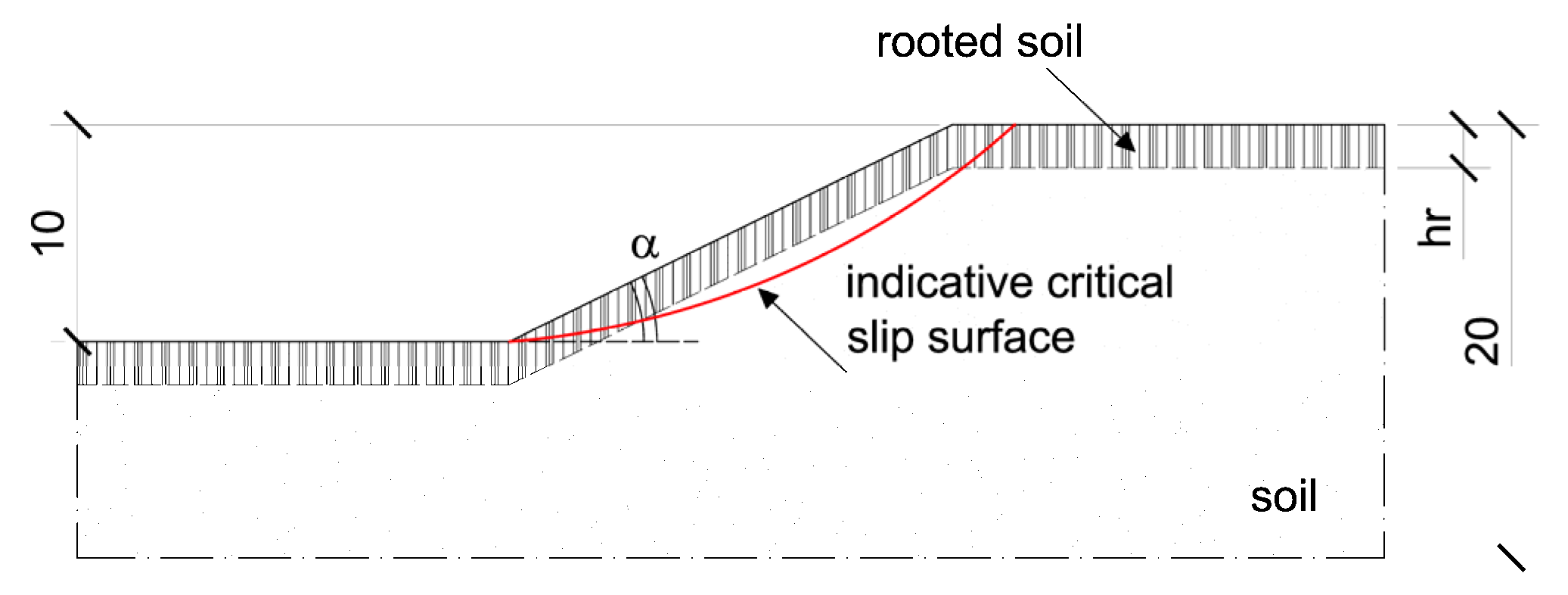
2.3. Analysis Methodology
- α, inclination angle of the slope to the horizontal;
- ϕ′, effective soil shear strength angle;
- hr, average root depth;
- Δτ, root contribution to the shear strength.
3. Results
3.1. Deterministic Benchmark
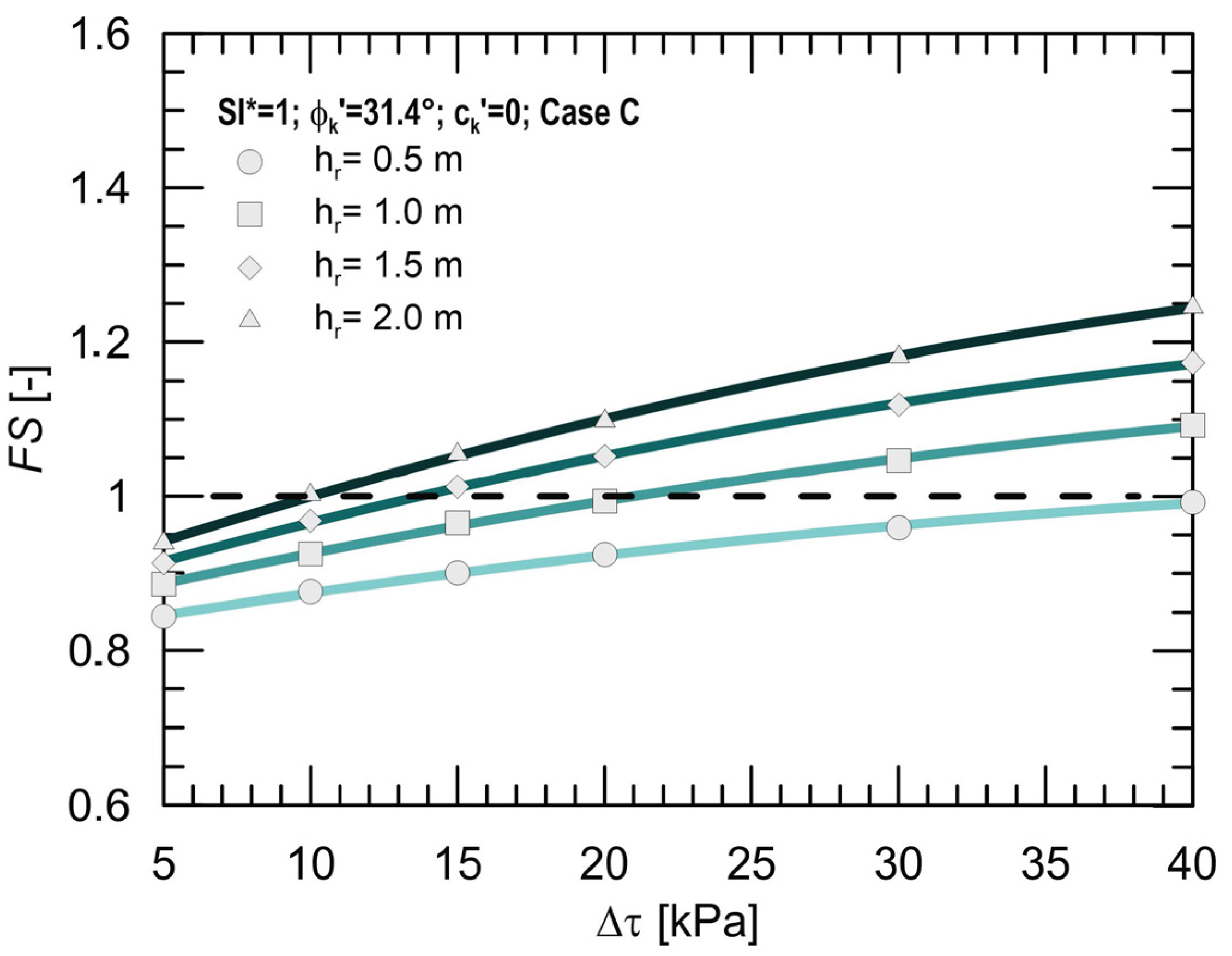
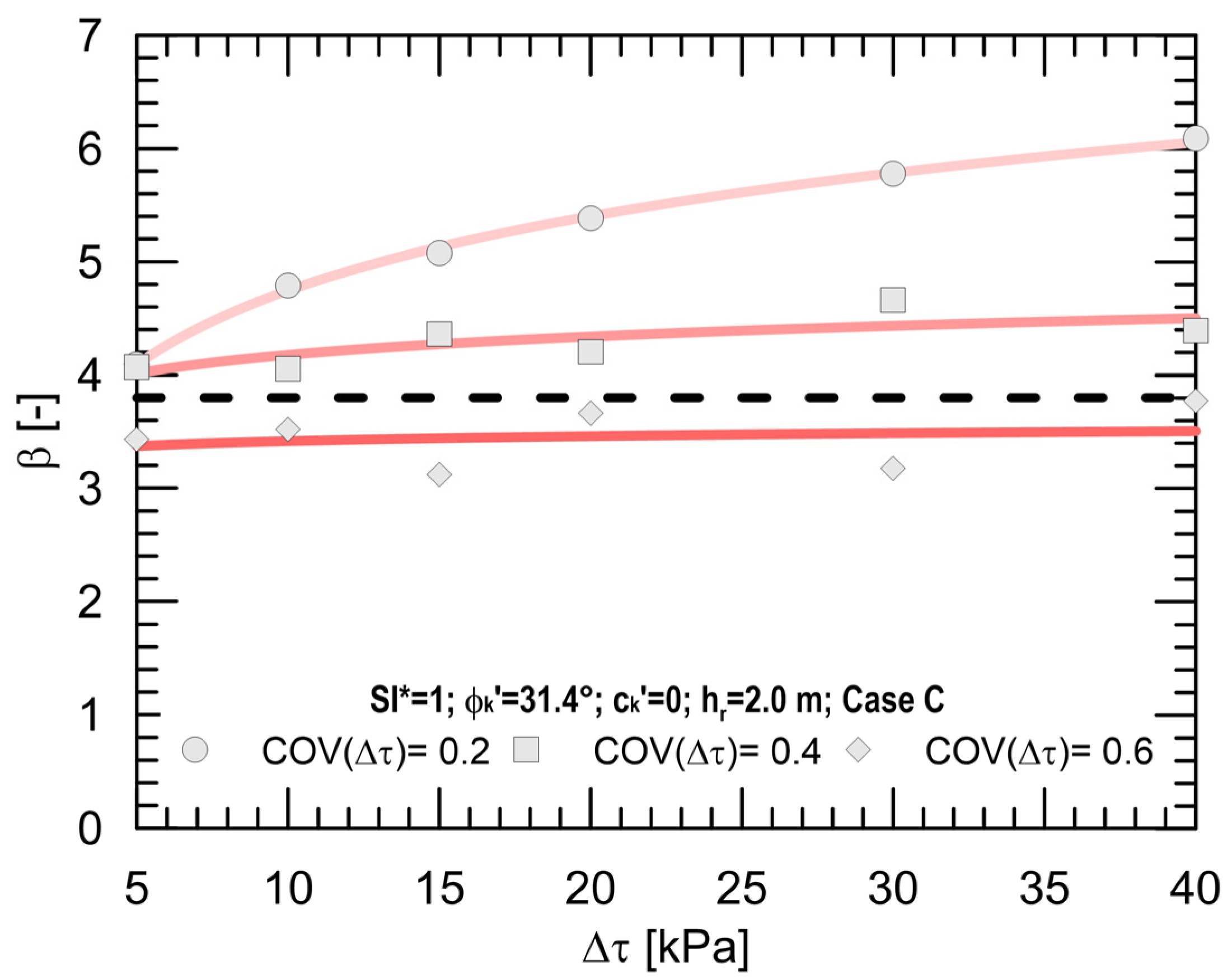
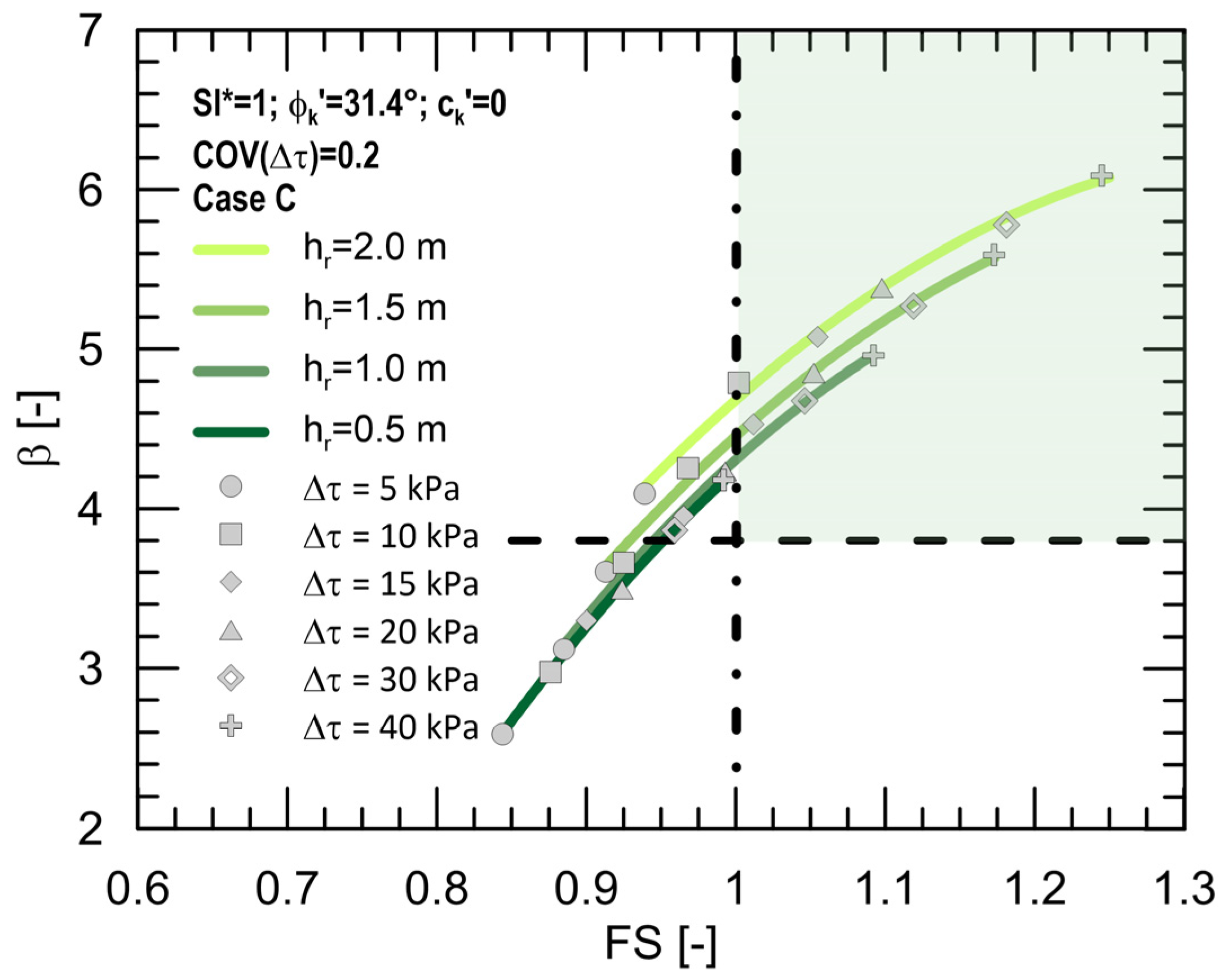
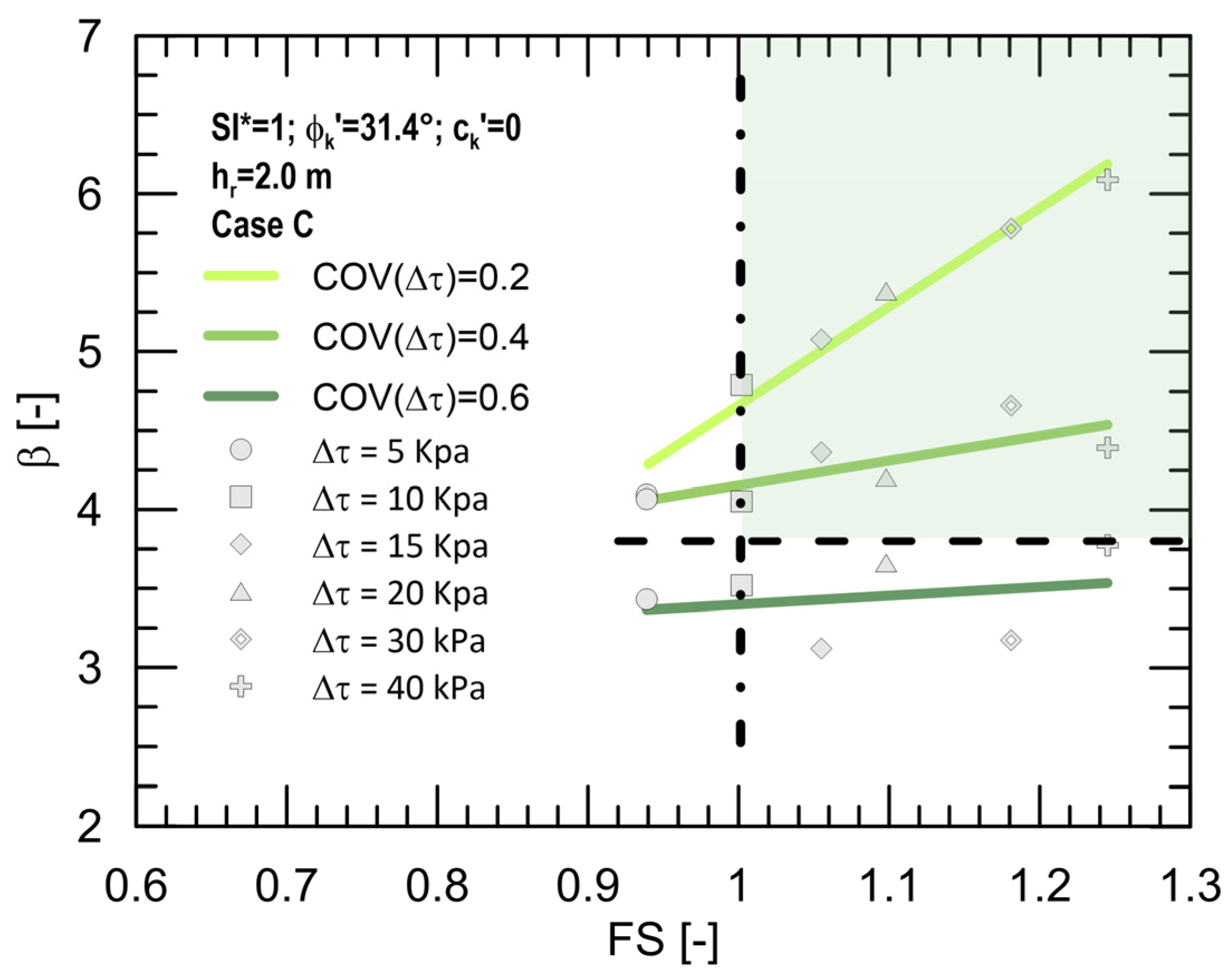
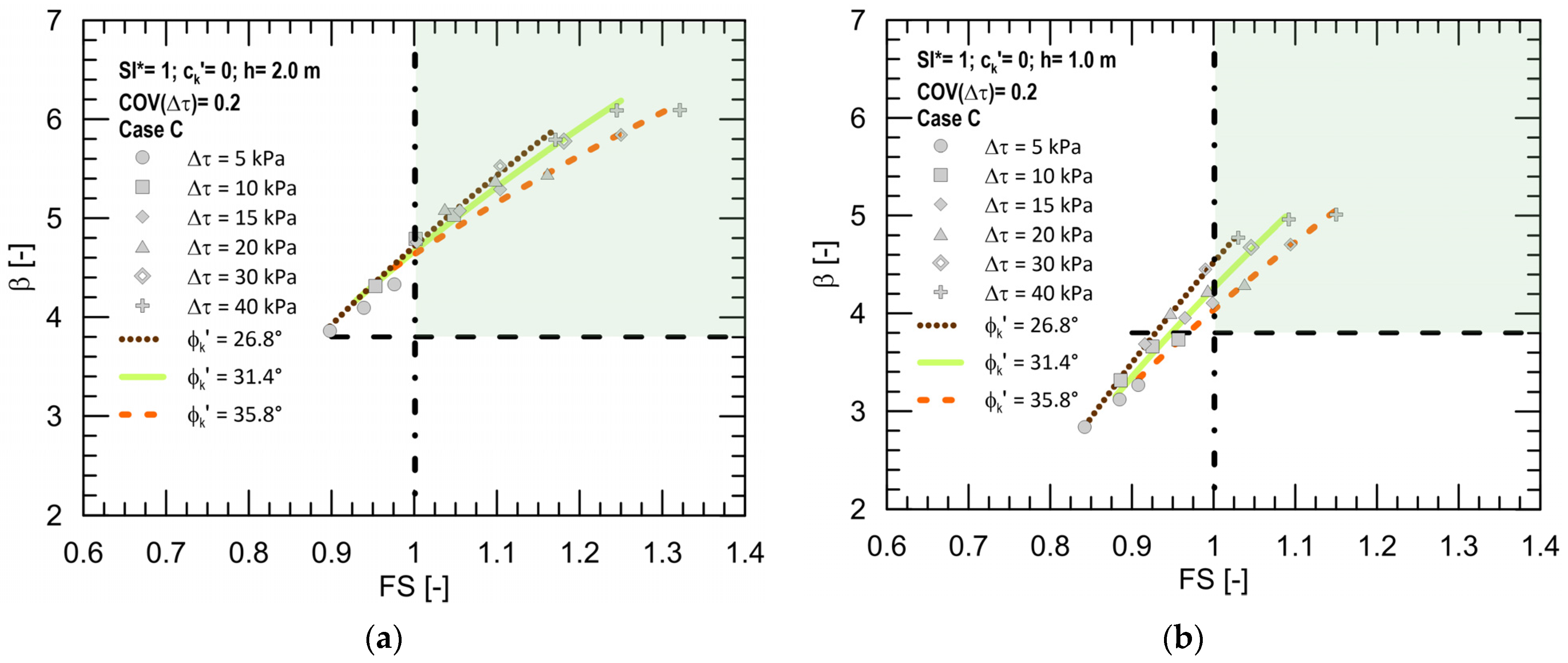
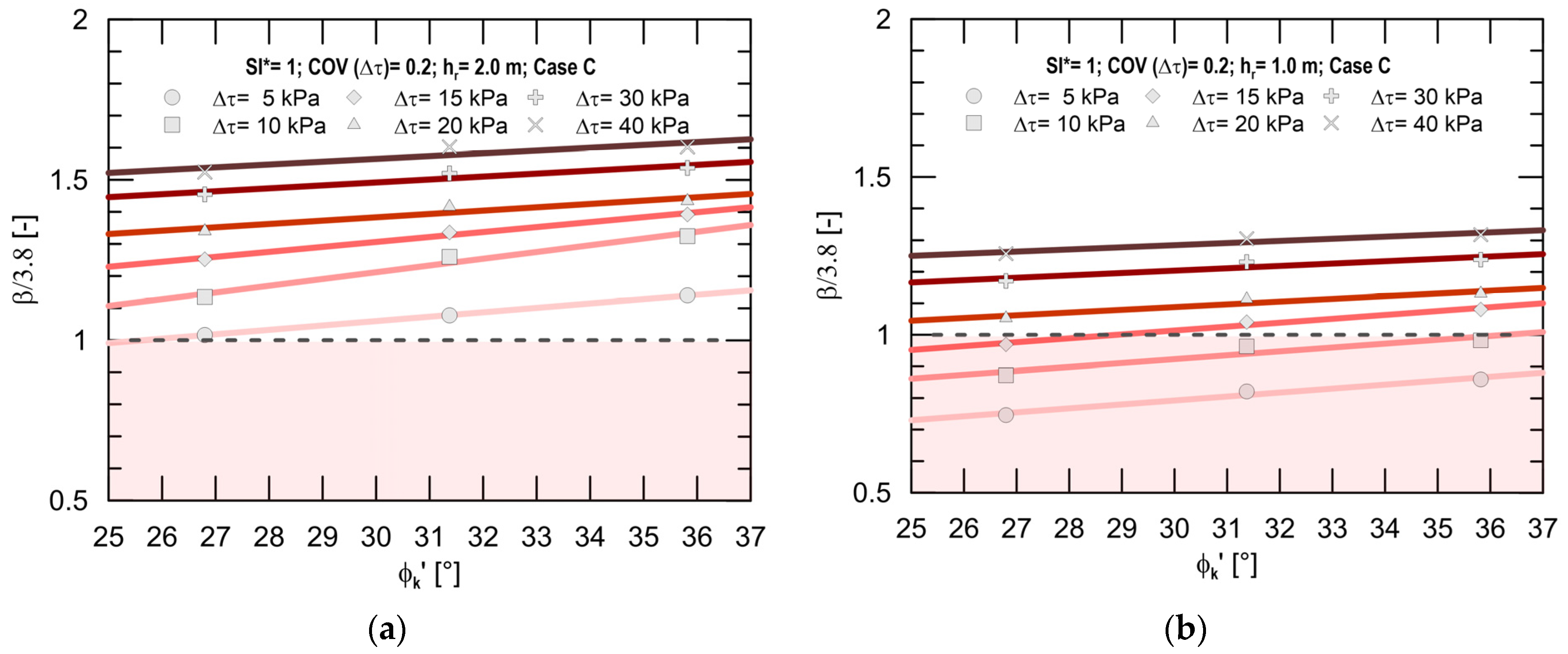
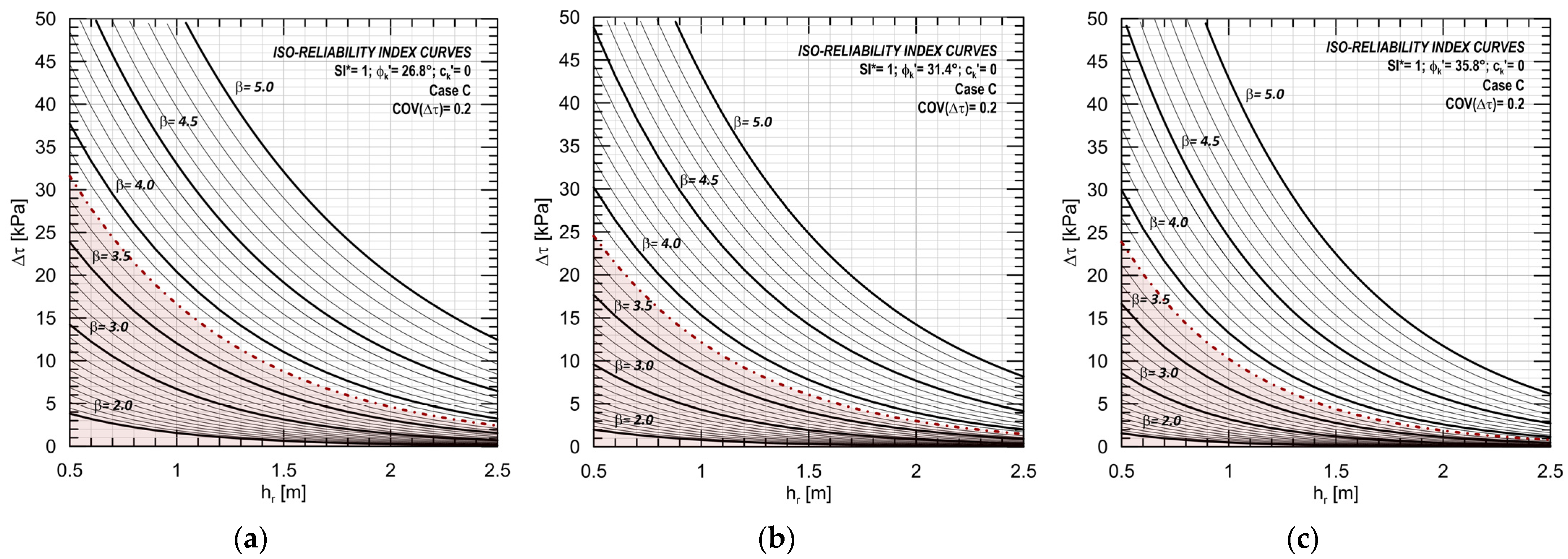
3.2. Probabilistic Analysis
4. Discussion and Conclusions
- The achievement of the limit equilibrium condition in a certain slope configuration derives from the combinations of strength and depth values of the roots and the seismic input. Root reinforcement generally improves the slope stability: FS increases with increasing both root depth and its contribution to the shear strength for all the geometries and seismic cases analysed.
- The reliability of root-reinforced slopes is highly affected by COV(Δτ) variation. When the coefficient of variation is low, the reliability index rises significantly with rising root contribution to the shear strength; however, this behaviour changes with increasing COV(Δτ): the higher the coefficient of variation, the lower the dependence of the reliability index on the root contribution to the shear strength.
- For all the analyses performed, whenever the safety criterion (FS = 1) is satisfied, the reliability criterion (β = 3.8) is guaranteed as well when the best condition in terms of data uncertainty is considered (COV(Δτ) = 0.2). On the contrary, high COV percentages lead to the achievement of reliability values that can be far from the target reliability index, implying that the ultimate limit state partial factors applied to actions and geotechnical parameters in the deterministic analyses cannot guarantee the reliability level required since they were not calibrated to bear in mind the large dispersion of data coming from the root contribution. In light of the above, it is evident that improving the methodology for evaluating root parameters that contribute to increasing soil shear strength is of utmost importance in order to obtain more reliable results.
- The gradient of the β-FS curves referring to different combinations of slope angle of inclination and soil shear strength angle (same initial stability index SI* = 1) decreases with increasing soil shear strength angle. Moreover, with equal Δτ, the greater the shear strength angle, the greater the combination of reliability index and factor of safety obtained.
- Considering both the deterministic and probabilistic aspects, the lower the seismic input, the better the slope stability (as expected). β-FS combinations that do not satisfy the safety and reliability criteria simultaneously are obtained only for the worst seismic case scenario and the lower root contributions to the shear strength investigated.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Hungr, O.; Leroueil, S.; Picarelli, L. The Varnes classification of landslide types, an update. Landslides 2014, 11, 167–194. [Google Scholar] [CrossRef]
- Ashwood, W.; Hungr, O. Estimating the total resisting force in a flexible barrier impacted by a granular avalanche using physical and numerical modeling. Can. Geotech. J. 2016, 53, 1700–1717. [Google Scholar] [CrossRef]
- Bugnion, L.; McArdell, B.W.; Bartelt, P.; Wendeler, C. Measurements of hillslope debris flow impact pressure on obstacles. Landslides 2011, 9, 179–187. [Google Scholar] [CrossRef] [Green Version]
- Canelli, L.; Ferrero, A.M.; Migliazza, M.; Segalini, A. Debris flow risk mitigation by the means of rigid and flexible barriers—Experimental tests and impact analysis. Nat. Hazards Earth Syst. Sci. 2012, 12, 1693–1699. [Google Scholar] [CrossRef] [Green Version]
- Moraci, N.; Pisano, M.; Mandaglio, M.C.; Gioffrè, D.; Pastor, M.; Leonardi, G.; Cola, S. Analyses and design procedure of a new physical model for debris flows: Results of numerical simulations by means of laboratory tests. Ital. J. Eng. Geol. Environ. 2015, 2, 29–40. [Google Scholar] [CrossRef]
- Vagnon, F.; Segalini, A. Debris flow impact estimation on a rigid barrier. Nat. Hazards Earth Syst. Sci. 2016, 16, 1691–1697. [Google Scholar] [CrossRef] [Green Version]
- Liu, W.; Yang, Z.; He, S. Modeling the landslide-generated debris flow from formation to propagation and run-out by considering the effect of vegetation. Landslides 2021, 18, 43–58. [Google Scholar] [CrossRef]
- Cardile, G.; Pisano, M.; Moraci, N.; Cazzuffi, D. Root reinforcement as a measure for shallow landslides risk mitigation. ArcHistoR 2019, 6, 616–627. [Google Scholar] [CrossRef]
- Masi, E.B.; Segoni, S.; Tofani, V. Root Reinforcement in Slope Stability Models: A Review. Geosciences 2021, 11, 212. [Google Scholar] [CrossRef]
- Tsige, D.; Senadheera, S.; Talema, A. Stability Analysis of Plant-Root-Reinforced Shallow Slopes along Mountainous Road Corridors Based on Numerical Modeling. Geosciences 2020, 10, 19. [Google Scholar] [CrossRef] [Green Version]
- Bischetti, G.B.; Di Fidio, M.; Florineth, F. On the origin of soil bioengineering. Landsc. Res. 2014, 39, 583–595. [Google Scholar] [CrossRef]
- Giupponi, L.; Borgonovo, G.; Giorgi, A.; Bischetti, G.B. How to renew soil bioengineering for slope stabilization: Some proposals. Landsc. Ecol. Eng. 2019, 15, 37–50. [Google Scholar] [CrossRef]
- Cazzuffi, D.; Cardile, G.; Gioffrè, D. Geosynthetic engineering and vegetation growth in soil reinforcement applications. Transp. Infrastruct. Geotechnol. 2014, 1, 262–300. [Google Scholar] [CrossRef]
- Kamchoom, V.; Leung, A.K.; Ng, C.W.W. Effects of root geometry and transpiration on pull-out resistance. Géotech. Lett. 2014, 4, 330–336. [Google Scholar] [CrossRef]
- Mickovski, S.B.; Hallett, P.D.; Bransby, M.F.; Davies, M.C.R.; Sonnenberg, R.; Bengough, A.G. Mechanical reinforcement of soil by willow roots: Impacts of root properties and root failure mechanism. Soil Sci. Soc. Am. J. 2009, 73, 1276–1285. [Google Scholar] [CrossRef]
- Mehtab, A.; Jiang, Y.-J.; Su, L.-J.; Shamsher, S.; Li, J.-J.; Mahfuzur, R. Scaling the Roots Mechanical Reinforcement in Plantation of Cunninghamia R. Br in Southwest China. Forests 2021, 12, 33. [Google Scholar] [CrossRef]
- Sonnenberg, R.; Bransby, M.F.; Hallett, P.D.; Bengough, A.G.; Mickovski, S.B.; Davies, M.C.R. Centrifuge modelling of soil slopes reinforced with vegetation. Can. Geotech. J. 2010, 47, 1415–1430. [Google Scholar] [CrossRef]
- Veylon, G.; Ghestem, M.; Stokes, A.; Bernard, A. Quantification of mechanical and hydric components of soil reinforcement by plant roots. Can. Geotech. J. 2015, 52, 1839–1849. [Google Scholar] [CrossRef]
- Wu, T.H. Root reinforcement of soil: Review of analytical models, test results, and applications to design. Can. Geotech. J. 2013, 50, 259–274. [Google Scholar] [CrossRef]
- Boldrin, D.; Leung, A.K.; Bengough, A.G. Hydro-mechanical reinforcement of contrasting woody species: A full-scale investigation of a field slope. Géotechnique 2021, 71, 970–984. [Google Scholar] [CrossRef]
- Kamchoom, V.; Leung, A.K. Hydro-mechanical reinforcements of live poles to slope stability. Soils Found. 2018, 58, 1423–1434. [Google Scholar] [CrossRef]
- Ng, C.W.W.; Menzies, B. Advanced Unsaturated Soil Mechanics and Engineering; CRC Press: Boca Raton, FL, USA, 2007; 712p. [Google Scholar]
- Ng, C.W.W.; Kamchoom, V.; Leung, A.K. Centrifuge modelling of the effects of root geometry on transpiration-induced suction and stability of vegetated slopes. Landslides 2016, 13, 925–938. [Google Scholar] [CrossRef] [Green Version]
- Bathurst, R.J.; Ezzein, F.M. Insights into geogrid–soil interaction using a transparent granular soil. Géotech. Lett. 2017, 7, 179–183. [Google Scholar] [CrossRef]
- Bergado, D.T.; Shivashankar, R.; Alfaro, M.C.; Chai, J.-C.; Balasubramaniam, A.S. Interaction behaviour of steel grid reinforcements in a clayey sand. Géotechnique 1993, 43, 589–603. [Google Scholar] [CrossRef]
- Derksen, J.; Ziegler, M.; Fuentes, R. Geogrid-soil interaction: A new conceptual model and testing apparatus. Geotext. Geomembr. 2021, 49, 1393–1406. [Google Scholar] [CrossRef]
- Peng, X.; Zornberg, J.G. Evaluation of soil-geogrid interaction using transparent soil with laser illumination. Geosynth. Int. 2019, 26, 206–221. [Google Scholar] [CrossRef]
- Dixon, N. Soil-geosynthetic interaction: Interface behaviour. In Proceedings of the 9th International Conference on Geosynthetics, Guarujá, Brazil, 23–27 May 2010; Palmeira, E.M., Vidal, D.M., Sayão, A.S.J.F., Ehrlich, M., Eds.; International Geosynthetics Society: Austin, TX, USA, 2010; pp. 563–582. [Google Scholar]
- Jewell, R.A. Reinforcement bond capacity. Géotechnique 1990, 40, 513–518. [Google Scholar] [CrossRef]
- Moraci, N.; Cardile, G.; Pisano, M. Soil-geosynthetic interface behaviour in the anchorage zone [Comportamento all’interfaccia terreno-geosintetico nella zona di ancoraggio]. Riv. Ital. Geotech. 2017, 51, 5–25. [Google Scholar]
- Cardile, G.; Pisano, M.; Moraci, N. The influence of a cyclic loading history on soil-geogrid interaction under pullout condition. Geotext. Geomembr. 2019, 47, 552–565. [Google Scholar] [CrossRef]
- Cardile, G.; Pisano, M.; Moraci, N. A predictive model for pullout bearing resistance of geogrids embedded in a granular soil. In Geotechnical Research for Land Protection and Development, Proceedings of the 7th Edition of the Italian National Congress of Geotechnical Researchers, Lecco, Italy, 3–5 July 2019; Lecture Notes in Civil Engineering; Springer International Publishing: Cham, Switzerland, 2020; Volume 40, pp. 438–445. [Google Scholar] [CrossRef]
- Cardile, G.; Pisano, M.; Recalcati, P.; Moraci, N. A new apparatus for the study of pullout behaviour of soil-geosynthetic interfaces under sustained load over time. Geotext. Geomembr. 2021, 49, 1519–1528. [Google Scholar] [CrossRef]
- Cardile, G.; Pisano, M. Advances in soil reinforcement with geosynthetics: From laboratory tests to design practice. Riv. Ital. Geotech. 2020, 54, 52–82. [Google Scholar] [CrossRef]
- Palmeira, E.M. Soil-geosynthetic interaction: Modelling and analysis. Geotext. Geomembr. 2009, 27, 368–390. [Google Scholar] [CrossRef]
- Pisano, M.; Cardile, G.; Moraci, N. The influence of cyclic loading histories on soil-geogrid interface behaviour under pullout conditions. In Proceedings of the 11th International Conference on Geosynthetics, ICG 2018, Seoul, Republic of Korea, 16–21 September 2018; Volume 4, pp. 2543–2548. [Google Scholar]
- Pisano, M.; Cardile, G.; Moraci, N. Soil-geogrid interface behaviour under cyclic pullout conditions. In Proceedings of the 7th lnternational Conference on Earthquake Geotechnical Engineering, Roma, Italy, 17–20 June 2019; Silvestri, F., Moraci, N., Eds.; Earthquake Geotechnical Engineering for Protection and Development of Environment and Constructions. CRC Press: Boca Raton, FL, USA; London, UK; New York, NY, USA, 2019; pp. 4507–4514. [Google Scholar]
- Wang, Z.; Jacobs, F.; Ziegler, M. Experimental and DEM investigation of geogrid-soil interaction under pullout loads. Geotext. Geomembr. 2016, 44, 230–246. [Google Scholar] [CrossRef]
- Deng, D.-p.; Zhao, L.-h.; Li, L. Limit equilibrium slope stability analysis using the nonlinear strength failure criterion. Can. Geotech. J. 2015, 52, 563–576. [Google Scholar] [CrossRef]
- Yang, X.L.; Yin, J.H. Slope stability analysis with nonlinear failure criterion. J. Eng. Mech. ASCE 2004, 130, 267–273. [Google Scholar] [CrossRef]
- Ingegneri, S.; Biondi, G.; Cascone, E.; Di Filippo, G. Influence of cyclic strength degradation on a Newmark-type analysis. In Proceedings of the 7th lnternational Conference on Earthquake Geotechnical Engineering, Roma, Italy, 17–20 June 2019; Silvestri, F., Moraci, N., Eds.; Earthquake Geotechnical Engineering for Protection and Development of Environment and Constructions. CRC Press: Boca Raton, FL, USA; London, UK; New York, NY, USA, 2019; pp. 2996–3004. [Google Scholar]
- Wilson, R.C.; Keefer, D.K. Dynamic analysis of a slope failure from the 6 August 1979 Coyote Lake, California, earthquake. Bull. Seismol. Soc. Am. 1983, 73, 863–877. [Google Scholar] [CrossRef]
- Pradel, D.; Smith, P.M.; Stewart, J.P.; Raad, G. Case history of landslide movement during the Northridge earthquake. J. Geotech. Geoenviron. Eng. 2005, 131, 1360–1369. [Google Scholar] [CrossRef]
- Yan, L.; Matasovic, N.; Kavazanjian, E., Jr. Seismic response of a block on an inclined plane to vertical and horizontal excitation acting simultaneously. In Proceedings of the 11th ASCE Engineering Mechanics Conference, Fort Lauderdale, FL, USA, 19–22 May 1996; pp. 1110–1113. [Google Scholar]
- Di Filippo, G.; Biondi, G.; Cascone, E. Influence of earthquake-induced pore-water pressure on the seismic stability of cohesive slopes. In Proceedings of the 7th International Conference on Earthquake Geotechnical Engineering, Roma, Italy, 17–20 June 2019; Silvestri, F., Moraci, N., Eds.; Earthquake Geotechnical Engineering for Protection and Development of Environment and Constructions. CRC Press: Boca Raton, FL, USA; London, UK; New York, NY, USA, 2019; pp. 2136–2144. [Google Scholar]
- Shinoda, M. Seismic stability and displacement analyses of earth slopes using non-circular slip surface. Soils Found. 2015, 55, 227–241. [Google Scholar] [CrossRef] [Green Version]
- Lacasse, S.; Nadim, F.; Liu, Z.Q.; Eidsvig, U.K.; Le, T.M.H.; Lin, C.G. Risk assessment and dams—Recent developments and applications. In Proceedings of the XVII European Conference on Soil Mechanics and Geotechnical Engineering, Reykjavík, Iceland, 1–6 September 2019; Sigursteinsson, H., Erlingsson, S., Bessason, B., Eds.; Geotechnical Engineering, Foundation of the Future. The Icelandic Geotechnical Society: Reykjavík, Iceland, 2019. [Google Scholar]
- CEN EN 1997-1; Eurocode 7—Geotechnical Design. European Committee for Standardization: Brussels, Belgium, 2004.
- CEN EN 1990; Eurocode—Basis of Structural Design. European Committee for Standardization: Brussels, Belgium, 2002.
- Reubens, B.; Poesen, J.; Danjon, F.; Geudens, G.; Muys, B. The role of fine and coarse roots in shallow slope stability and soil erosion control with a focus on root system architecture: A review. Trees 2007, 21, 385–402. [Google Scholar] [CrossRef]
- Wang, X.; Ma, C.; Wang, Y.; Wang, Y.; Li, T.; Dai, Z.; Li, M. Effect of root architecture on rainfall threshold for slope stability: Variabilities in saturated hydraulic conductivity and strength of root-soil composite. Landslides 2020, 17, 1965–1977. [Google Scholar] [CrossRef]
- Fishman, G.S. Monte Carlo: Concepts, Algorithms, and Applications; Springer: New York, NY, USA, 1996; 698p. [Google Scholar]
- Hammersley, J.M.; Handscomb, D.C. Monte Carlo Methods; Methuen & Co.: London, UK; John Wiley & Sons: New York, NY, USA, 1964; 178p. [Google Scholar]
- Rubinstein, R.Y.; Kroese, D.P. Simulation and the Monte Carlo Method; Wiley-Interscience: New York, NY, USA, 2007; 345p. [Google Scholar]
- Waldron, L.J. The shear resistance of root-permeated homogeneous and stratified soil. Soil Sci. Soc. Am. J. 1977, 41, 843–849. [Google Scholar] [CrossRef]
- Wu, T.H. Investigation of Landslides on Prince of Wales Island, Alaska; Geotechnical Engineering Report 5; Department of Civil Engineering, Ohio State University: Columbia, OH, USA, 1976; 106p. [Google Scholar]
- Eab, K.H.; Likitlersuang, S.; Takahashi, A. Laboratory and modelling investigation of root-reinforced system for slope stabilisation. Soils Found. 2015, 55, 1270–1281. [Google Scholar] [CrossRef] [Green Version]
- Docker, B.B.; Hubble, T.C.T. Quantifying root-reinforcement of river bank soils by four Australian tree species. Geomorphology 2008, 100, 401–418. [Google Scholar] [CrossRef]
- Giadrossich, F.; Schwarz, M.; Cohen, D.; Cislaghi, A.; Vergani, C.; Hubble, T.; Phillips, C.; Stokes, A. Methods to measure the mechanical behaviour of tree roots: A review. Ecol. Eng. 2017, 109, 256–271. [Google Scholar] [CrossRef]
- Karimzadeh, A.A.; Leung, A.K.; Hosseinpour, S.; Wu, Z.; Amini, P.F. Monotonic and cyclic behaviour of root-reinforced sand. Can. Geotech. J. 2021, 58, 1915–1927. [Google Scholar] [CrossRef]
- Lan, H.; Wang, D.; He, S.; Fang, Y.; Chen, W.; Zhao, P.; Qi, Y. Experimental study on the effects of tree planting on slope stability. Landslides 2020, 17, 1021–1035. [Google Scholar] [CrossRef]
- Liang, T.; Knappett, J.A.; Duckett, N. Modelling the seismic performance of rooted slopes from individual root–soil interaction to global slope behaviour. Géotechnique 2015, 65, 995–1009. [Google Scholar] [CrossRef] [Green Version]
- Meijer, G.J.; Bengough, G.; Knappett, J.; Loades, K.; Nicoll, B. In situ root identification through blade penetrometer testing—Part 2: Field testing. Géotechnique 2018, 68, 320–331. [Google Scholar] [CrossRef]
- Pallewattha, M.; Indraratna, B.; Heitor, A.; Rujikiatkamjorn, C. Shear strength of a vegetated soil incorporating both root reinforcement and suction. Transp. Geotech. 2019, 18, 72–82. [Google Scholar] [CrossRef]
- Pollen, N.; Simon, A. Estimating the mechanical effects of riparian vegetation on stream bank stability using a fiber bundle model. Water Resour. Res. 2005, 41, W07025. [Google Scholar] [CrossRef]
- Cohen, D.; Schwarz, M.; Or, D. An analytical fiber bundle model for pullout mechanics of root bundles. J. Geophys. Res. Earth Surf. 2011, 116, F03010. [Google Scholar] [CrossRef] [Green Version]
- Liang, T.; Knappett, J.A.; Leung, A.; Carnaghan, A.; Bengough, A.G.; Zhao, R. A critical evaluation of predictive models for rooted soil strength with application to predicting the seismic deformation of rooted slopes. Landslides 2020, 17, 93–109. [Google Scholar] [CrossRef] [Green Version]
- Mao, Z. Root reinforcement models: Classification, criticism and perspectives. Plant Soil 2022, 472, 17–28. [Google Scholar] [CrossRef]
- Murgia, I.; Giadrossich, F.; Mao, Z.; Cohen, D.; Capra, G.F.; Schwarz, M. Modeling shallow landslides and root reinforcement: A review. Ecol. Eng. 2022, 181, 106671. [Google Scholar] [CrossRef]
- Schwarz, M.; Cohen, D.; Or, D. Root-soil mechanical interactions during pullout and failure of root bundles. J. Geophys. Res. Earth Surf. 2010, 115, F04035. [Google Scholar] [CrossRef] [Green Version]
- Van Beek, L.P.H.; Wint, J.; Cammeraat, L.H.; Edwards, J.P. Observation and simulation of root reinforcement on abandoned mediterranean slopes. Plant Soil 2005, 278, 55–74. [Google Scholar] [CrossRef]
- Mickovski, S.B.; Stokes, A.; van Beek, R.; Ghestem, M.; Fourcaud, T. Simulation of direct shear tests on rooted and non-rooted soil using finite element analysis. Ecol. Eng. 2011, 37, 1523–1532. [Google Scholar] [CrossRef]
- Schmidt, K.M.; Roering, J.J.; Stock, J.D.; Dietrich, W.E.; Montgomery, D.R.; Schaub, T. The variability of root cohesion as an influence on shallow landslide susceptibility in the Oregon Coast Range. Can. Geotech. J. 2001, 38, 995–1024. [Google Scholar] [CrossRef]
- Thomas, R.E.; Pollen-Bankhead, N. Modeling root reinforcement with a fiber-bundle model and Monte Carlo simulation. Ecol. Eng. 2010, 36, 47–61. [Google Scholar] [CrossRef]
- Gray, D.H.; Sotir, R.B. Biotechnical and Soil Bioengineering Slope Stabilization: A Practical Guide for Erosion Control; John Wiley & Sons: New York, NY, USA, 1996; 400p. [Google Scholar]
- Wu, T.H. Slope Stabilization. In Slope Stabilization and Erosion Control—A Bioengineering Approach; Morgan, R.P.C., Rickson, R.J., Eds.; Chapman and Hall, University Press: Cambridge, UK, 1995; pp. 221–264. [Google Scholar]
- Vergani, C.; Chiaradia, E.A.; Bischetti, G.B. Variability in the tensile resistance of roots in Alpine forest tree species. Ecol. Eng. 2012, 46, 43–56. [Google Scholar] [CrossRef]
- Bischetti, G.B.; Chiaradia, E.A.; Simonato, T.; Speziali, B.; Vitali, B.; Vullo, P.; Zocco, A. Root strength and root area ratio of forest species in Lombardy (Northern Italy). Plant Soil 2005, 278, 11–22. [Google Scholar] [CrossRef]
- Cislaghi, A. Exploring the variability in elastic properties of roots in alpine tree species. J. For. Sci. 2021, 67, 338–356. [Google Scholar] [CrossRef]
- Leung, F.T.Y.; Yan, W.M.; Hau, B.C.H.; Tham, L.G. Root systems of native shrubs and trees in Hong Kong and their effects on enhancing slope stability. CATENA 2015, 125, 102–110. [Google Scholar] [CrossRef]
- Likitlersuang, S.; Phan, T.N.; Boldrin, D.; Leung, A.K. Influence of growth media on the biomechanical properties of the fibrous roots of two contrasting vetiver grass species. Ecol. Eng. 2022, 178, 106574. [Google Scholar] [CrossRef]
- Zhang, C.-B.; Chen, L.-H.; Jiang, J. Why fine tree roots are stronger than thicker roots: The role of cellulose and lignin in relation to slope stability. Geomorphology 2014, 206, 196–202. [Google Scholar] [CrossRef]
- Phoon, K.K.; Kulhawy, F.H. Characterization of geotechnical variability. Can. Geotech. J. 1999, 36, 612–624. [Google Scholar] [CrossRef]
- Lacasse, S.; Nadim, F. Uncertainties in characterising soil properties. In Uncertainty in the Geologic Environment: From Theory to Practice; Shackelford, C.D., Nelson, P.P., Roth, M.J.S., Eds.; ASCE GSP 58; American Society of Civil Engineers: Reston, VA, USA, 1996; pp. 49–75. [Google Scholar]
- Ji, J.; Kokutse, N.; Genet, M.; Fourcaud, T.; Zhang, Z. Effect of spatial variation of tree root characteristics on slope stability. A case study on Black Locust (Robinia pseudoacacia) and Arborvitae (Platycladus orientalis) stands on the Loess Plateau, China. CATENA 2012, 92, 139–154. [Google Scholar] [CrossRef]
- Cislaghi, A.; Chiaradia, E.A.; Bischetti, G.B. Including root reinforcement variability in a probabilistic 3-D stability model. Earth Surf. Process. Landf. 2017, 42, 1789–1806. [Google Scholar] [CrossRef]
- Nguyen, T.S.; Likitlersuang, S.; Jotisankasa, A. Influence of the spatial variability of the root cohesion on a slope-scale stability model: A case study of residual soil slope in Thailand. Bull. Eng. Geol. Environ. 2019, 78, 3337–3351. [Google Scholar] [CrossRef]
- Zhu, H.; Zhang, L.M.; Xiao, T.; Li, X.Y. Enhancement of slope stability by vegetation considering uncertainties in root distribution. Comput. Geotech. 2017, 85, 84–89. [Google Scholar] [CrossRef]
- Lacasse, S. Reliability and probabilistic methods. In Proceedings of the 13th International Conference on Soil Mechanics and Foundation Engineering, New Delhi, India, 5–10 January 1994; pp. 225–227. [Google Scholar]
- Cho, S.E. Probabilistic assessment of slope stability that considers the spatial variability of soil properties. J. Geotech. Geoenviron. Eng. ASCE 2010, 136, 975–984. [Google Scholar] [CrossRef]
- Chowdhury, R.N.; Xu, D.W. Geotechnical system reliability of slopes. Reliab. Eng. Syst. Saf. 1995, 47, 141–151. [Google Scholar] [CrossRef]
- Christian, J.T.; Ladd, C.C.; Baecher, G.B. Reliability applied to slope stability analysis. J. Geotech. Eng. 1994, 120, 2180–2207. [Google Scholar] [CrossRef]
- Griffiths, D.V.; Huang, J.; Fenton, G.A. Influence of spatial variability on slope reliability using 2-D random fields. J. Geotech. Geoenviron. Eng. ASCE 2009, 135, 1367–1378. [Google Scholar] [CrossRef] [Green Version]
- Huang, H.W.; Wen, S.C.; Zhang, J.; Chen, F.Y.; Martin, J.R.; Wang, H. Reliability analysis of slope stability under seismic condition during a given exposure time. Landslides 2018, 15, 2303–2313. [Google Scholar] [CrossRef]
- McGuire, M.P.; Vandenberge, D.R. Interpretation of shear strength uncertainty and reliability analyses of slopes. Landslides 2017, 14, 2059–2072. [Google Scholar] [CrossRef]
- Bovolenta, R.; Mazzuoli, M.; Berardi, R. Soil bio-engineering techniques to protect slopes and prevent shallow landslides. Riv. Ital. Geotech. 2018, 3, 44–65. [Google Scholar]
- Canadell, J.; Jackson, R.B.; Ehleringer, J.B.; Mooney, H.A.; Sala, O.E.; Schulze, E.-D. Maximum rooting depth of vegetation types at the global scale. Oecologia 1996, 108, 583–595. [Google Scholar] [CrossRef] [PubMed]
- Foresta, V.; Capobianco, V.; Cascini, L. Influence of grass roots on shear strength of pyroclastic soils. Can. Geotech. J. 2020, 57, 1320–1334. [Google Scholar] [CrossRef] [Green Version]
- Bordoni, M.; Cislaghi, A.; Vercesi, A.; Bischetti, G.B.; Meisina, C. Effects of plant roots on soil shear strength and shallow landslide proneness in an area of northern Italian Apennines. Bull. Eng. Geol. Environ. 2020, 79, 3361–3381. [Google Scholar] [CrossRef]
- Tron, S.; Dani, A.; Laio, F.; Preti, F.; Ridolfi, L. Mean root depth estimation at landslide slopes. Ecol. Eng. 2014, 69, 118–125. [Google Scholar] [CrossRef]
- Bishop, A.W. The use of the slip circle in the stability analysis of slope. Géotechnique 1955, 5, 7–17. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhang, J.; Chen, G.; Zheng, L.; Li, Y. Effects of vertical seismic force on initiation of the Daguangbao landslide induced by the 2008 Wenchuan earthquake. Soil Dyn. Earthq. Eng. 2015, 73, 91–102. [Google Scholar] [CrossRef]
- Bischetti, G.B.; De Cesare, G.; Mickovski, S.B.; Rauch, H.P.; Schwarz, M.; Stangl, R. Design and temporal issues in Soil Bioengineering structures for the stabilisation of shallow soil movements. Ecol. Eng. 2021, 169, 106309. [Google Scholar] [CrossRef]
- Harr, M.E. Reliability-Based Design in Design in Civil Engineering; McGraw-Hill: New York, NY, USA, 1987; 400p. [Google Scholar]
- Kulhawy, F.H. On the evaluation of static soil properties. Stability and Performance of Slopes and Embankments. ASCE Geotech. Spec. Publ. 1992, 31, 95–115. [Google Scholar]
- Lee, I.K.; Ingles, O.G.; White, W. Geotechnical Engineering; Pitman Publishing: London, UK, 1983; 508p. [Google Scholar]
- Phoon, K.K.; Kulhawy, F.H.; Grigoriu, M.D. Reliability-Based Design of Foundations for Transmission Line Structures; Electric Power Research Institute: Palo Alto, CA, USA, 1995; 384p. [Google Scholar]
- Javankhoshdel, S.; Bathurst, R.J. Influence of cross correlation between soil parameters on probability of failure of simple cohesive and c–ϕ slopes. Can. Geotech. J. 2015, 53, 839–853. [Google Scholar] [CrossRef]
- Wolff, T. Analysis and Design of Embankment Dam Slopes: A Probabilistic Approach (Soil Mechanics, Indiana). Ph.D. Thesis, Purdue University, Lafayette, IN, USA, 1986. [Google Scholar]
- Wu, X.Z. Trivariate analysis of soil ranking-correlated characteristics and its application to probabilistic stability assessments in geotechnical engineering problems. Soils Found. 2013, 53, 540–556. [Google Scholar] [CrossRef] [Green Version]
- Huang, L.; Dong, B.-C.; Xue, W.; Peng, Y.-K.; Zhang, M.-X.; Yu, F.-H. Soil particle heterogeneity affects the growth of a rhizomatous wetland plant. PLoS ONE 2013, 8, e69836. [Google Scholar] [CrossRef] [Green Version]
- Li, Z.Q.; Kong, L.Y.; Yang, L.F.; Zhang, M.; Cao, T.; Xu, J.; Wang, Z.X.; Lei, Y. Effect of substrate grain size on the growth and morphology of the submersed macrophyte Vallisneria natans L. Limnologica 2012, 42, 81–85. [Google Scholar] [CrossRef]
- Roscoe, R.; Buurman, P.; Velthorst, E.; Vasconcellos, C.A. Soil organic matter dynamics in density and particle-size fractions as revealed by the 13C/12C isotopic ratio in a Cerrado’s oxisol. Geoderma 2001, 104, 185–202. [Google Scholar] [CrossRef]
- Tsompanakis, Y.; Lagaros, N.D.; Psarropoulos, P.N.; Georgopoulos, E.C. Probabilistic seismic slope stability assessment of geostructures. Struct. Infrastruct. Eng. 2010, 6, 179–191. [Google Scholar] [CrossRef]

| Consequences Class | Description | |
|---|---|---|
| Loss of Human Life | Economic, Social, or Environmental Consequences | |
| CC3 | High | Very great |
| CC2 | Medium | Considerable |
| CC1 | Low | Small or negligible |
| Reliability Class | Minimum Values for β | |
|---|---|---|
| 1 Year Reference Period | 50 Years Reference Period | |
| RC3 | 5.2 | 4.3 |
| RC2 | 4.7 | 3.8 |
| RC1 | 4.2 | 3.3 |
| SLOPE | Parameter | Value |
|---|---|---|
| H (m) | slope height | 10 |
| α (°) | angle of inclination to the horizontal | 22, 26, 30 |
| ϕk′ (°) | characteristic value of soil shear strength angle | 26.8, 31.4, 35.8 |
| ck′ (kPa) | characteristic value of soil cohesion | 0 |
| γk (°kN/m3) | characteristic value of soil unit weight | 18.5 |
| ROOT | Parameter | Value |
| hr (m) | average root depth | 0, 0.5, 1, 1.5, 2 |
| Δτk (kPa) | characteristic value of root contribution to shear strength | 0, 5, 10, 15, 20, 30, 40 |
| SLOPE | Mean Value | COV (%) | Distribution Type |
|---|---|---|---|
| SI* = 1 α = 22° | ϕm′ = 30.28° | 7 | normal |
| γm = 17.09 kN/m3 | 5 | normal | |
| SI* = 1 α = 26° | ϕm′ = 35.45° | 7 | normal |
| γm = 17.09 kN/m3 | 5 | normal | |
| SI* = 1 α = 30° | ϕm′ = 40.48° | 7 | normal |
| γm = 17.09 kN/m3 | 5 | normal | |
| ROOT | Mean value Δ τm [kPa] | COV (%) | Distribution type |
| 7.06 | 20 | lognormal | |
| Δτk = 5 kPa | 10.15 | 40 | lognormal |
| 14.52 | 60 | lognormal | |
| 14.13 | 20 | lognormal | |
| Δτk = 10 kPa | 20.30 | 40 | lognormal |
| 29.04 | 60 | lognormal | |
| 21.19 | 20 | lognormal | |
| Δτk = 15 kPa | 30.45 | 40 | lognormal |
| 43.56 | 60 | lognormal | |
| 28.25 | 20 | lognormal | |
| Δτk = 20 kPa | 40.60 | 40 | lognormal |
| 58.07 | 60 | lognormal | |
| 42.37 | 20 | lognormal | |
| Δτk = 30 kPa | 60.90 | 40 | lognormal |
| 87.10 | 60 | lognormal | |
| 56.50 | 20 | lognormal | |
| Δτk = 40 kPa | 81.18 | 40 | lognormal |
| 116.14 | 60 | lognormal |
| Case | Mean Value (-) | COV (%) | Distribution Type |
|---|---|---|---|
| A | kh = 0 | - | - |
| kv = 0 | - | - | |
| B | kh = 0.05 | 10 | lognormal |
| kv = +0.5 kh | 10 | lognormal | |
| C | kh = 0.10 | 10 | lognormal |
| kv = +0.5 kh | 10 | lognormal |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pisano, M.; Cardile, G. Probabilistic Analyses of Root-Reinforced Slopes Using Monte Carlo Simulation. Geosciences 2023, 13, 75. https://doi.org/10.3390/geosciences13030075
Pisano M, Cardile G. Probabilistic Analyses of Root-Reinforced Slopes Using Monte Carlo Simulation. Geosciences. 2023; 13(3):75. https://doi.org/10.3390/geosciences13030075
Chicago/Turabian StylePisano, Marilene, and Giuseppe Cardile. 2023. "Probabilistic Analyses of Root-Reinforced Slopes Using Monte Carlo Simulation" Geosciences 13, no. 3: 75. https://doi.org/10.3390/geosciences13030075
APA StylePisano, M., & Cardile, G. (2023). Probabilistic Analyses of Root-Reinforced Slopes Using Monte Carlo Simulation. Geosciences, 13(3), 75. https://doi.org/10.3390/geosciences13030075