Evaluation of a Combustion-Based Mesoscale Thermal Actuator in Open and Closed Operating Cycles †
Abstract
:1. Introduction
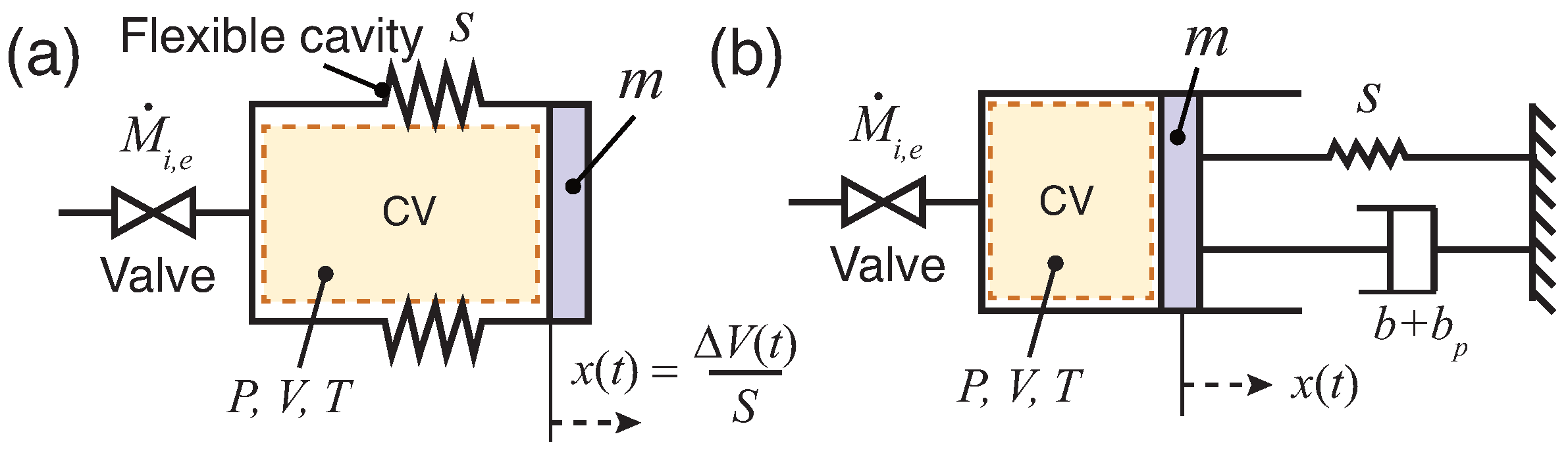
2. Thermal Actuator: Design and Model
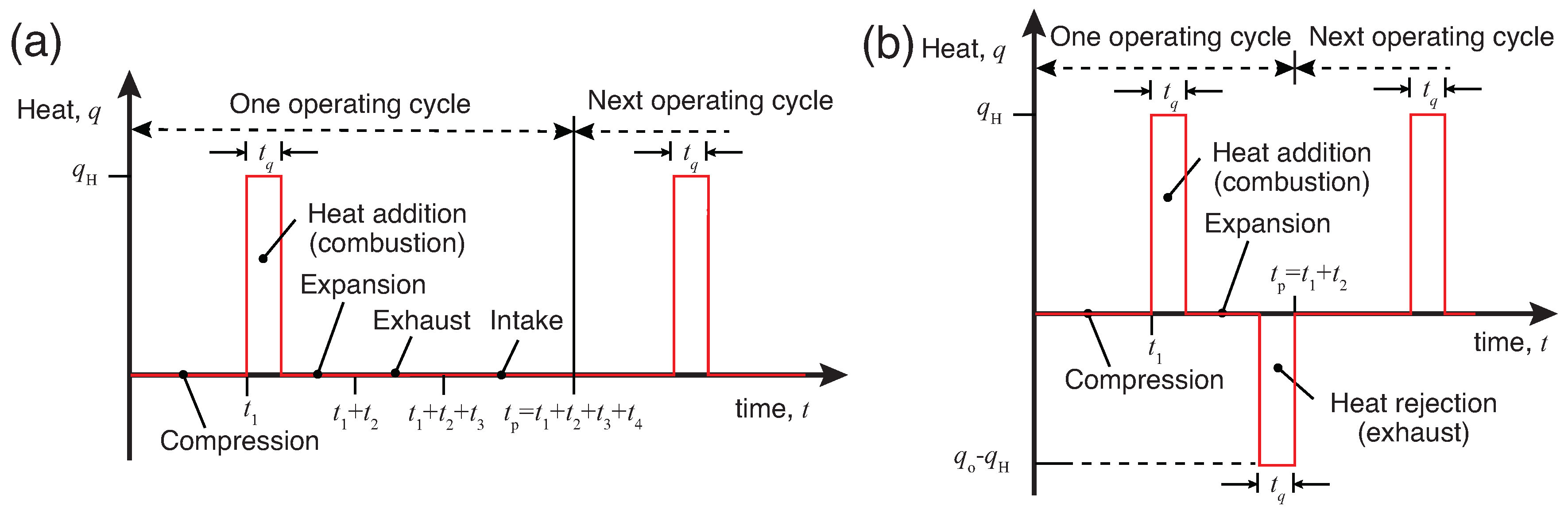
2.1. Solving the Open Cycle Model
2.2. Solving the Closed Cycle Model
3. Results and Discussion
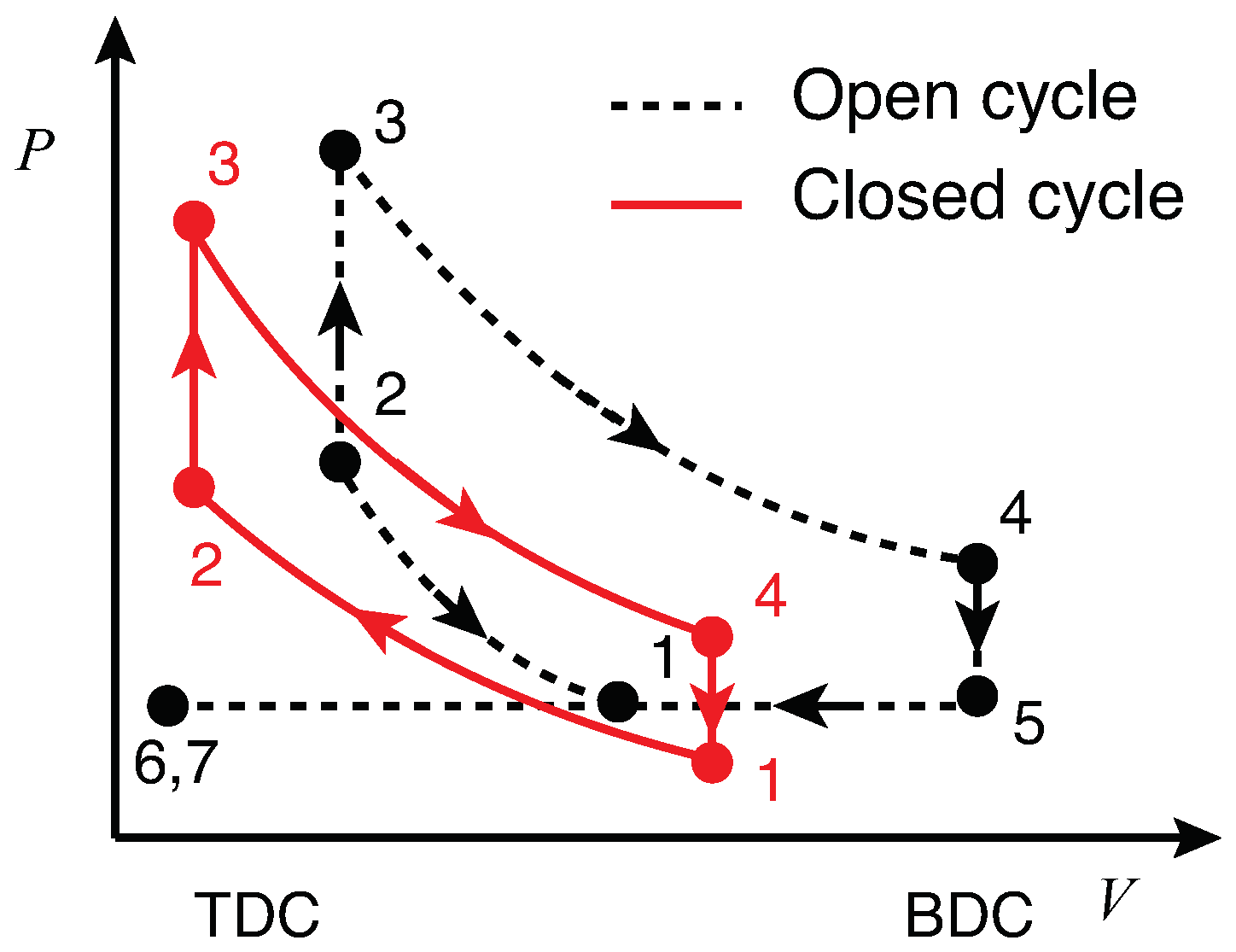
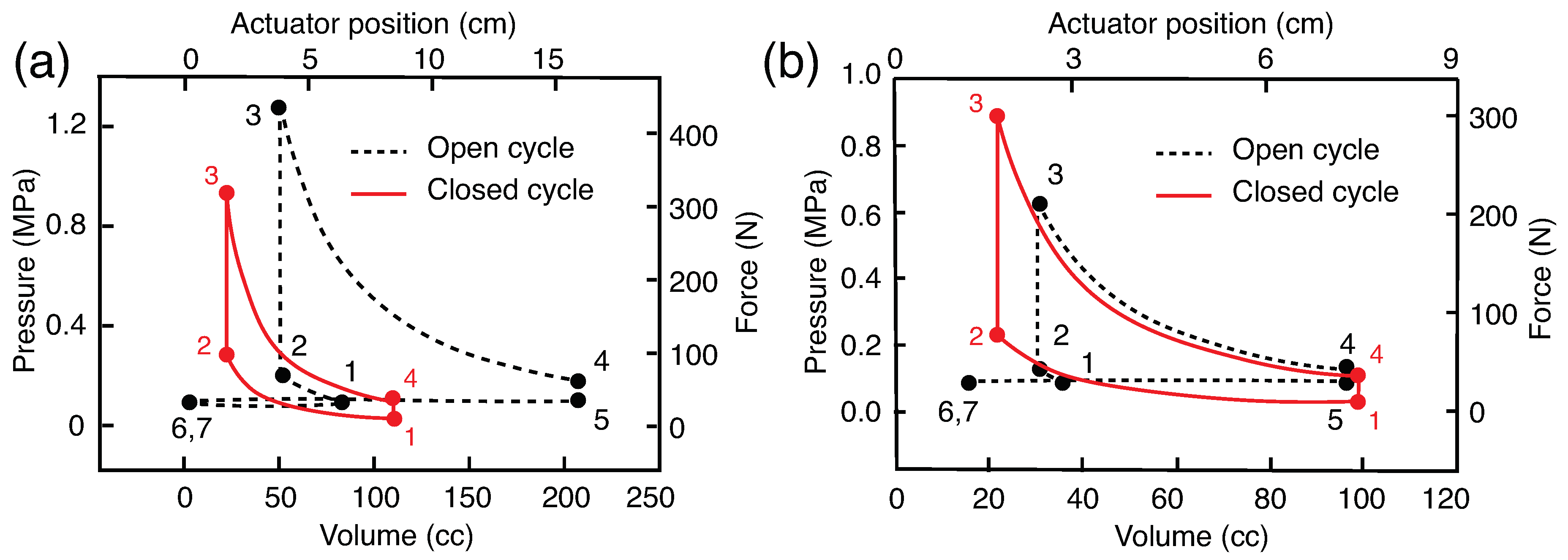
3.1. Pressure-Volume and Force-Displacement Diagrams
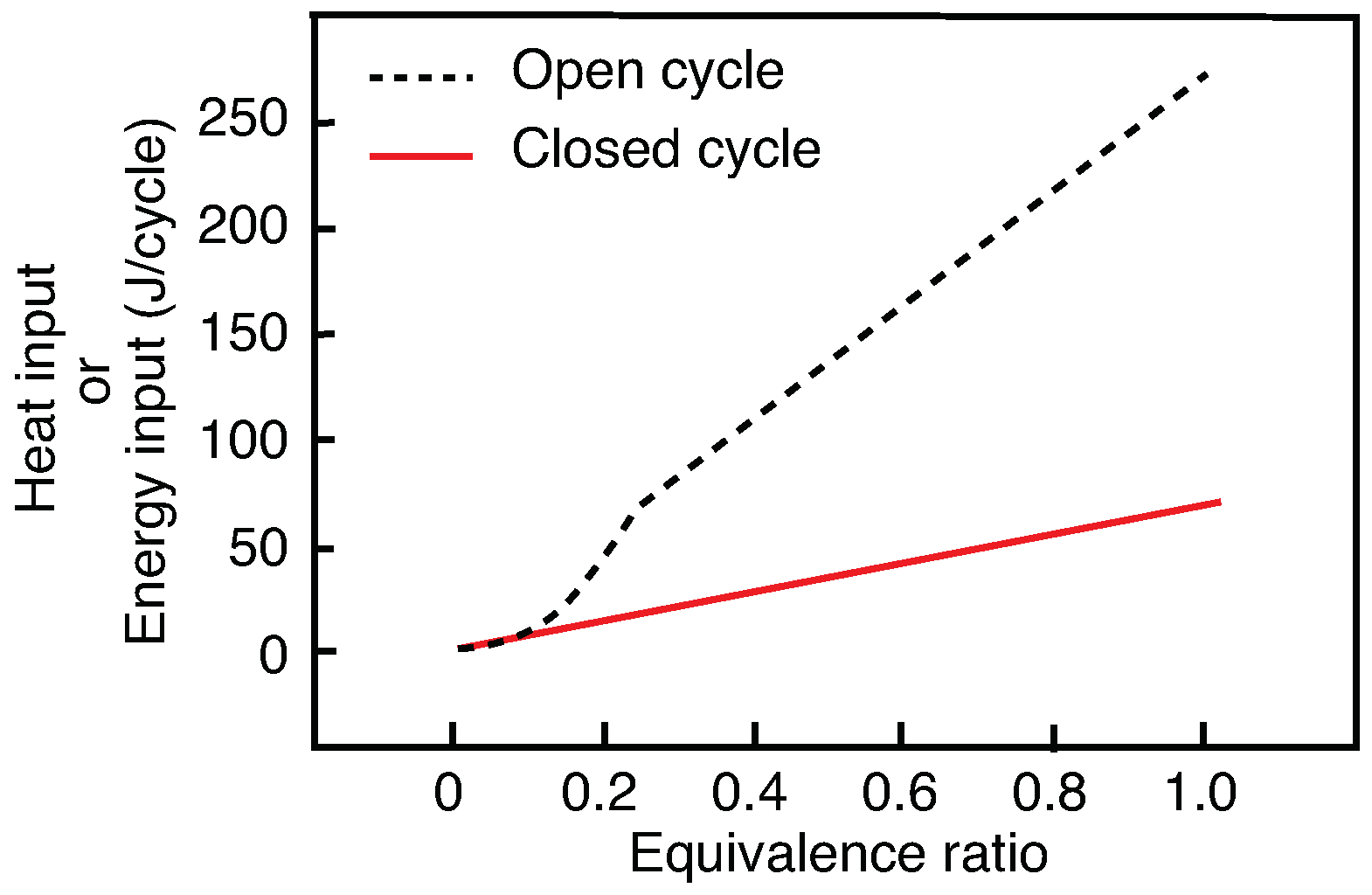
3.2. Equivalence Ratio and Heat Input Relationship
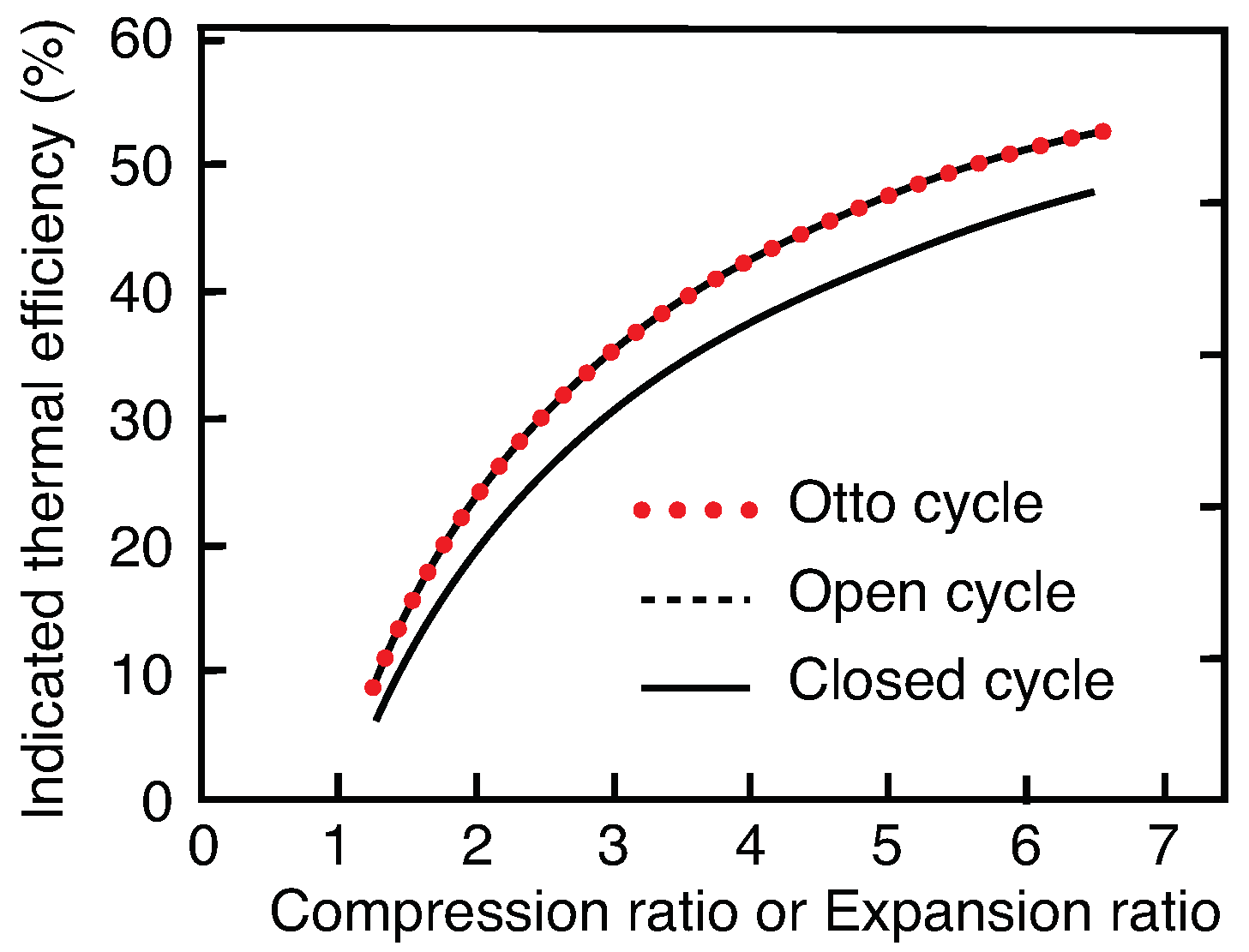
3.3. Efficiency and Volume Ratios
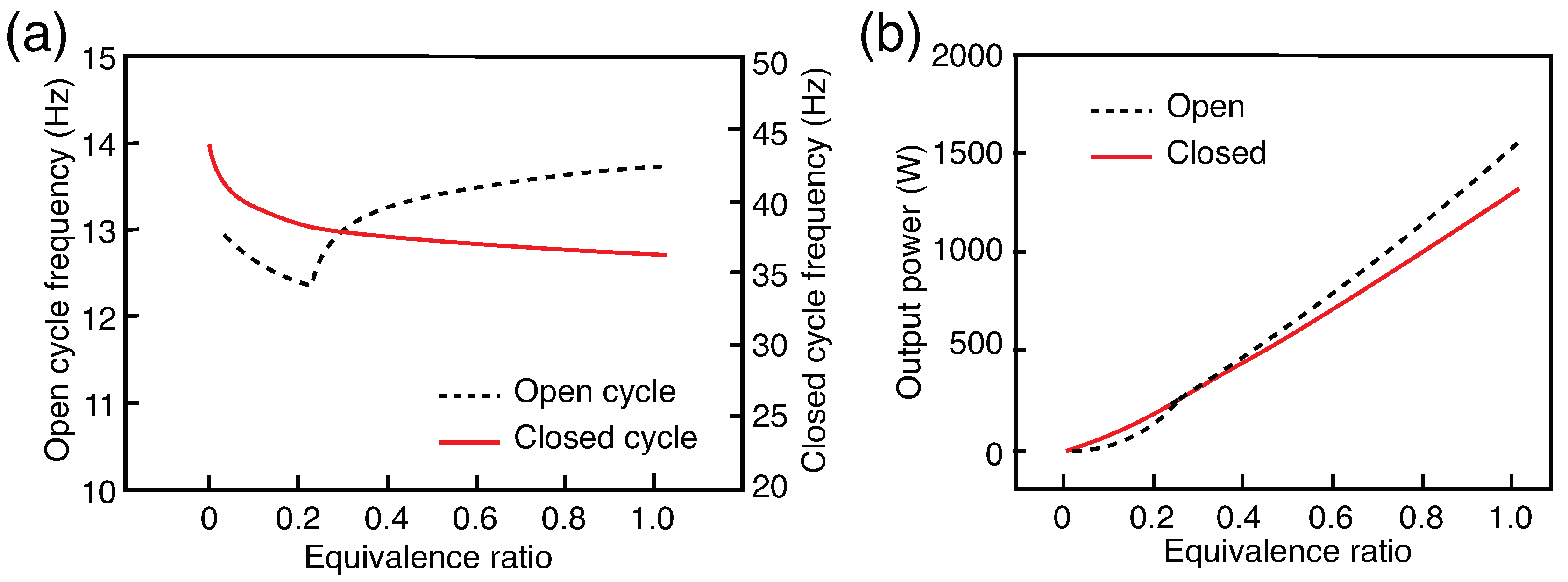
3.4. Operating Frequency and Power Output
4. Conclusions
Funding
Conflicts of Interest
Abbreviations
| ER | Expansion Ratio |
| CR | Compression Ratio |
| CV | Control Volume or Cavity |
| TDC | Top Dead Center |
| BDC | Bottom Dead Center |
References
- Bell, D.J.; Lu, T.J.; Fleck, N.A.; Spearing, S.M. MEMS actuators and sensors: Observations on their performance and selection for purpose. J. Micromech. Microeng. 2005, 15, S153–S164. [Google Scholar] [CrossRef]
- Henriksson, J.; Gullo, M.; Brugger, J. Integrated long-range thermal bimorph actuators for parallelizable Bio-AFM applications. In Proceedings of the 2012 IEEE SENSORS, Taipei, Taiwan, 28–31 October 2012; pp. 1–4. [Google Scholar] [CrossRef]
- Butler, J.T.; Bright, V.M.; Cowan, W.D. Average power control and positioning of polysilicon thermal actuators. Sens. Actuators A Phys. 1999, 72, 88–97. [Google Scholar] [CrossRef]
- Lu, T.; Hutchinson, J.; Evans, A. Optimal design of a flexural actuator. J. Mech. Phys. Solids 2001, 49, 2071–2093. [Google Scholar] [CrossRef]
- Bardaweel, H.; Preetham, B.S.; Richards, R.; Richards, C.; Anderson, M. MEMS-based resonant heat engine: Scaling analysis. Microsyst. Technol. 2011, 17, 1251–1261. [Google Scholar] [CrossRef]
- Nakajima, N.; Ogawa, K.; Fujimasa, I. Study on micro engines-miniaturizing Stirling engines for actuators and heatpumps. In Proceedings of the IEEE Micro Electro Mechanical Systems, ‘An Investigation of Micro Structures, Sensors, Actuators, Machines and Robots’, Salt Lake City, UT, USA, 20–22 February 1989; pp. 145–148. [Google Scholar] [CrossRef]
- Preetham, B.S.; Anderson, M.; Richards, C. Modeling of a resonant heat engine. J. Appl. Phys. 2012, 112, 124903. [Google Scholar] [CrossRef]
- Formosa, F.; Fréchette, L.G. Scaling laws for free piston Stirling engine design: Benefits and challenges of miniaturization. Energy 2013, 57, 796–808. [Google Scholar] [CrossRef]
- Preetham, B.S.; Anderson, M.; Richards, C. Estimation of parasitic losses in a proposed mesoscale resonant engine: Experiment and model. J. Appl. Phys. 2014, 115, 054904. [Google Scholar] [CrossRef]
- Preetham, B.S.; Anderson, M.; Richards, C. Mathematical modeling of a four-stroke resonant engine for micro and mesoscale applications. J. Appl. Phys. 2014, 116, 214904. [Google Scholar] [CrossRef]
- Burugupally, S.; Weiss, L. Power generation via small length scale thermo-mechanical systems: Current status and challenges, a review. Energies 2018, 11, 2253. [Google Scholar] [CrossRef]
- Burugupally, S.P. Development of a Small Scale Resonant Engine for Micro and Mesoscale Applications. Ph.D. Thesis, Washington State University, Washington, DC, USA, 2014. [Google Scholar]
- Burugupally, S.P.; Weiss, L. Design and performance of a miniature free piston expander. Energy 2019, 170, 611–618. [Google Scholar] [CrossRef]
- Burugupally, S.P.; Weiss, L.; Depcik, C. The effect of working fluid properties on the performance of a miniature free piston expander for waste heat harvesting. Appl. Therm. Eng. 2019, 151, 431–438. [Google Scholar] [CrossRef]
- Preetham, B.; Weiss, L. Investigations of a new free piston expander engine cycle. Energy 2016, 106, 535–545. [Google Scholar] [CrossRef]
- Ouyang, X.; Ding, S.; Fan, B.; Li, P.Y.; Yang, H. Development of a novel compact hydraulic power unit for the exoskeleton robot. Mechatronics 2016, 38, 68–75. [Google Scholar] [CrossRef]
- Raibert, M.; Blankespoor, K.; Nelson, G.; Playter, R. Bigdog, the rough-terrain quadruped robot. IFAC Proc. Vol. 2008, 41, 10822–10825. [Google Scholar] [CrossRef]
- Bradley, D.; Acosta-Marquez, C.; Hawley, M.; Brownsell, S.; Enderby, P.; Mawson, S. NeXOS – The design, development and evaluation of a rehabilitation system for the lower limbs. Mechatronics 2009, 19, 247–257. [Google Scholar] [CrossRef]
- Pulkrabek, W. Engineering Fundamentals of the Internal Combustion Engine; Prentice Hall: Englewood Cliffs, NJ, USA, 1997. [Google Scholar]
- Heywood, J. Internal Combustion Engine Fundamentals; McGraw-Hill Education: New York, NY, USA, 1988. [Google Scholar]

| Performance Parameter | Closed Cycle | Open Cycle |
|---|---|---|
| Indicated thermal efficiency (%) | 47.66 | 38.41 |
| Brake thermal efficiency (%) | 44.33 | 34.64 |
| Heat added per cycle (J/cycle) | 35.6 | 138.5 |
| Cycle frequency (Hz) | 37.03 | 13.37 |
| Compression ratio, CR | 5.05 | 1.76 |
| Expansion ratio, ER | 5.05 | 4.13 |
| Average piston speed (m/s) | 4.75 | 4.77 |
| Power output (W) | 584.7 | 641.6 |
| Mass of air in cylinder (kg/cycle) | 2.41 × 10 | 9.28 × 10 |
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Burugupally, S.P. Evaluation of a Combustion-Based Mesoscale Thermal Actuator in Open and Closed Operating Cycles. Actuators 2019, 8, 73. https://doi.org/10.3390/act8040073
Burugupally SP. Evaluation of a Combustion-Based Mesoscale Thermal Actuator in Open and Closed Operating Cycles. Actuators. 2019; 8(4):73. https://doi.org/10.3390/act8040073
Chicago/Turabian StyleBurugupally, Sindhu Preetham. 2019. "Evaluation of a Combustion-Based Mesoscale Thermal Actuator in Open and Closed Operating Cycles" Actuators 8, no. 4: 73. https://doi.org/10.3390/act8040073
APA StyleBurugupally, S. P. (2019). Evaluation of a Combustion-Based Mesoscale Thermal Actuator in Open and Closed Operating Cycles. Actuators, 8(4), 73. https://doi.org/10.3390/act8040073





