Angle Magnetization Rotation Method for Characterizing Co-Rich Amorphous Ferromagnetic Microwires
Abstract
1. Introduction
2. Numerical Simulation
2.1. Model Description
2.2. Equilibrium Magnetization Curves
2.3. Calculation of the Second Harmonic Amplitude
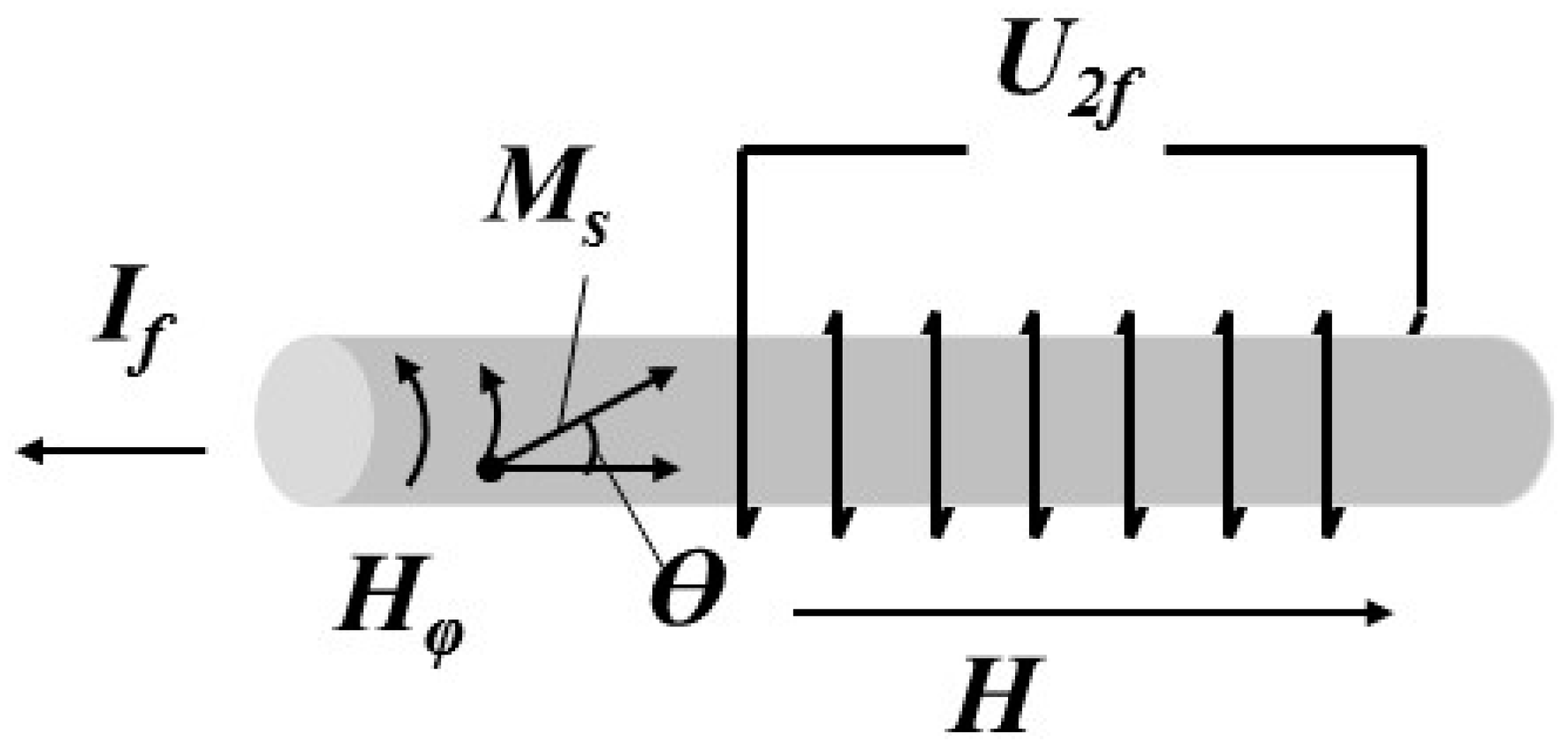
3. Materials and Methods
4. Results and Discussion
4.1. Magnetization Curves
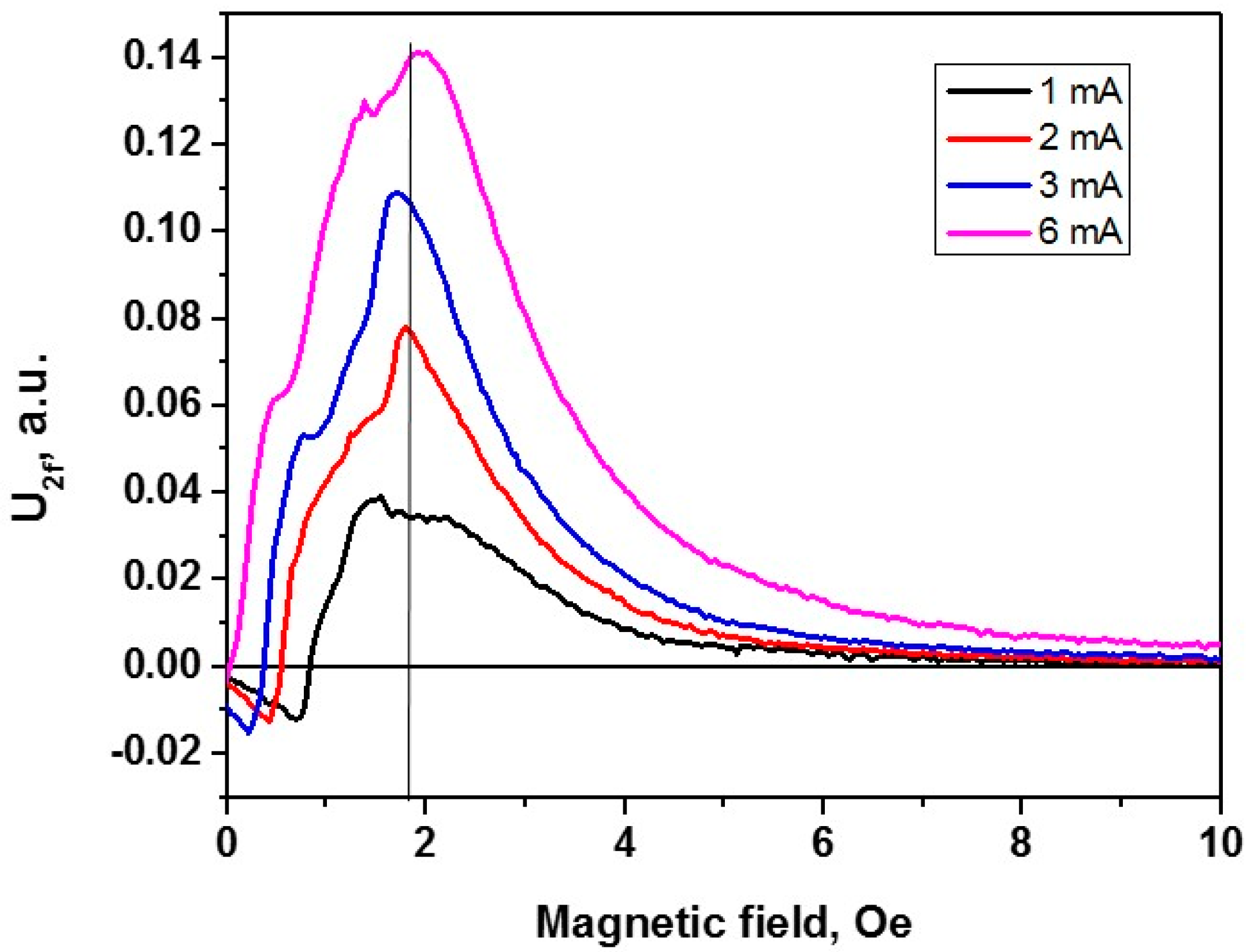
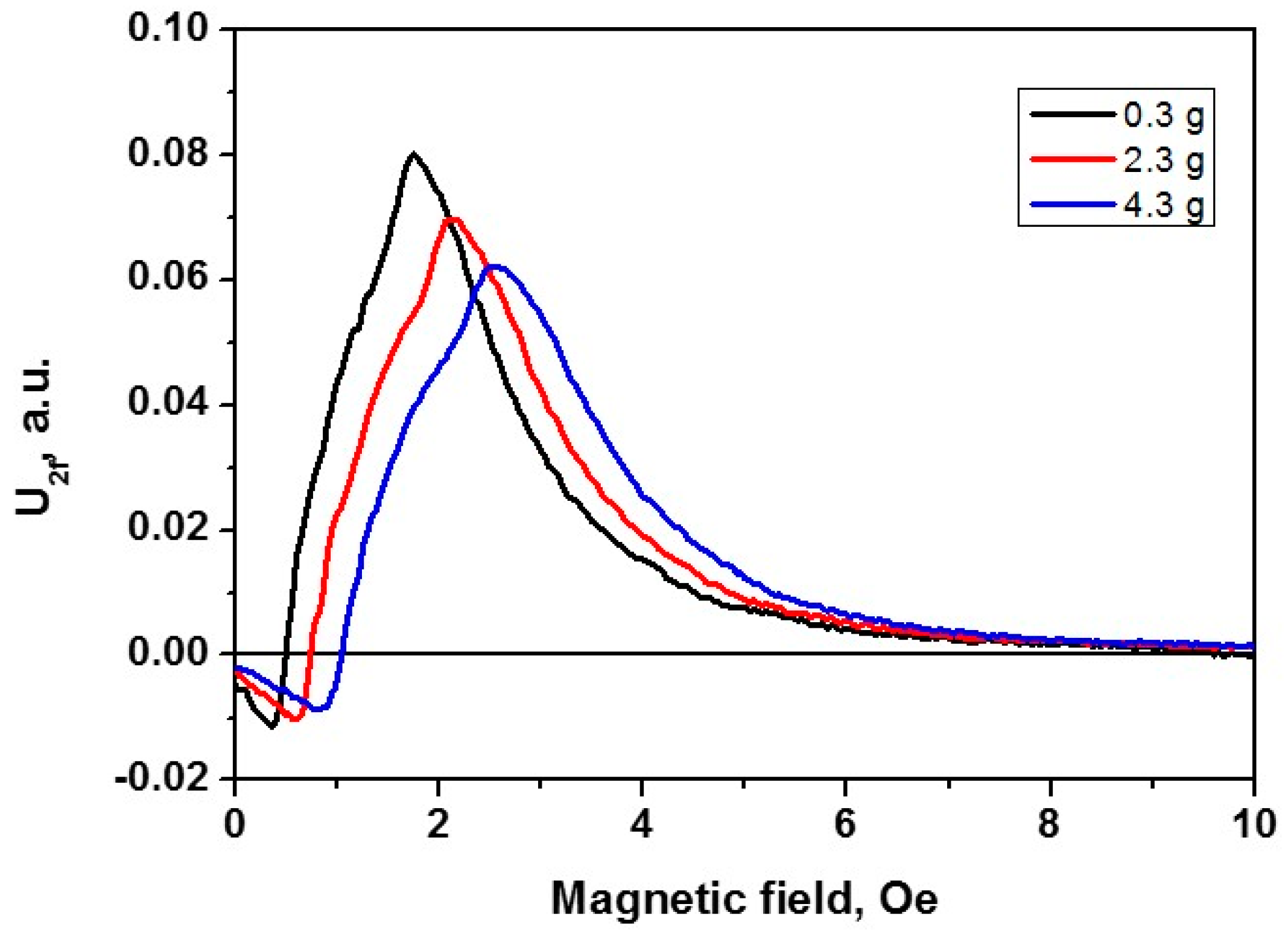
4.2. Measurements by the AMR Method
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Phan, M.H.; Peng, H.X. Giant magnetoimpedance materials: Fundamentals and applications. Prog. Mater. Sci. 2008, 53, 323–420. [Google Scholar] [CrossRef]
- Zhukov, A.; Zhukova, V. Magnetic Properties and Applications of Ferromagnetic Mircowires with Amorphous and Nanocrystalline Structure; Nova Science Publishers, Inc.: New York, NY, USA, 2009. [Google Scholar]
- Zhukov, A.; Ipatov, M.; del Val, J.J.; Zhukova, V.; Chernenko, V.A. Magnetic and structural properties of glass-coated Heusler-type microwires exhibiting martensitic transformation. Sci. Rep. 2018, 8, 621. [Google Scholar] [CrossRef]
- Garcia, C.; Zhukova, V.; Shevyrtalov, S.; Ipatov, M.; Corte-Leon, P.; Zhukov, A. Tuning of magnetic properties in Ni-Mn-Ga Heusler-type glass -coated microwires by annealing. J. Alloys Compd. 2020, 838, 155481. [Google Scholar] [CrossRef]
- Chiriac, H.; Marinescu, C.S.; Ovári, T.-A. Large gyromagnetic effect in magnetostrictive amorphous wires. Sens. Actuators A 2000, 81, 126–128. [Google Scholar] [CrossRef]
- Lunaa, C.; Raposo, V.; Garshelis, I.; Zhukov, A.P.; Iñiguez, J.I.; Vázquez, M. Inducing rotation and levitation in magnetostrictive wires and rods: Correlated amplitude and frequency of exciting ac axial magnetic field. Sens. Actuators 2003, 106, 274–277. [Google Scholar] [CrossRef]
- Sugino, T.; Takezawa, M.; Honda, T.; Yamasaki, J. Basic load characteristics of magnetostrictive amorphous wire micro-motor. IEEE Trans. Magn. 2001, 37, 2871–2873. [Google Scholar] [CrossRef]
- Panina, L.V.; Mohri, K. Magneto-impedance effect in amorphous wires. Appl. Phys. Lett. 1994, 65, 1189. [Google Scholar] [CrossRef]
- Beach, R.; Berkowitz, A. Giant magnetic field dependent impedance of amorphous FeCoSiB wire. Appl. Phys. Lett. 1994, 64, 3652–3654. [Google Scholar] [CrossRef]
- Knobel, M.; Vázquez, M.; Kraus, L. Giant magnetoimpedance. In Handbook of Magnetic Materials; Buschow, K.H.J., Ed.; Elsevier: Amsterdam, The Netherlands, 2003; Volume 15, pp. 497–563. [Google Scholar]
- Zhukov, A.; Ipatov, M.; Zhukova, V. Advances in Giant Magnetoimpedance of Materials. In Handbook of Magnetic Materials; Buschow, K.H.J., Ed.; Elsevier: Amsterdam, The Netherlands, 2015; Volume 24, pp. 139–236. [Google Scholar] [CrossRef]
- Ipatov, M.; Zhukova, V.; Zhukov, A.; Gonzalez, J. Magnetoimpedance sensitive to dc bias current in amorphous microwires. Appl. Phys. Lett. 2010, 97, 252507. [Google Scholar] [CrossRef]
- Kabanov Yu Zhukov, A.; Zhukova, V.; Gonzalez, J. Magnetic domain structure of wires studied by using the magneto-optical indicator film method. Appl. Phys. Lett. 2005, 87, 142507. [Google Scholar] [CrossRef]
- Chiriac, H.; Ovari, T.A. Amorphous glass-covered magnetic wires: Preparation, properties, applications. Prog. Mater Sci. 1996, 40, 333–407. [Google Scholar] [CrossRef]
- Antonov, A.S.; Borisov, V.T.; Borisov, O.V.; Prokoshin, A.F.; Usov, N.A. Residual quenching stresses in glass-coated amorphous ferromagnetic microwires. J. Phys. D Appl. Phys. 2000, 33, 1161. [Google Scholar] [CrossRef]
- Gudoshnikov, S.; Usov, N.; Nozdrin, A.; Ipatov, M.; Zhukov, A.; Zhukova, V. Highly sensitive magnetometer based on the off-diagonal GMI effect in Co-rich glass-coated microwire. Phys. Status Solidi A 2014, 211, 980–985. [Google Scholar] [CrossRef]
- Herrero-Gómez, C.; Aragón, A.M.; Hernando-Rydings, M.; Marín, P.; Hernando, A. Stress and field contactless sensor based on the scattering of electromagnetic waves by a single ferromagnetic microwire. Appl. Phys. Lett. 2014, 105, 092405. [Google Scholar] [CrossRef]
- Herrero-Gómez, C.; Marín, P.; Hernando, A. Bias free magnetomechanical coupling on magnetic microwires for sensing applications. Appl. Phys. Lett. 2013, 103, 142414. [Google Scholar] [CrossRef]
- Vazquez, M.; Fernengel, W.; Kronmüller, H. The effect of tensile stresses on the magnetic properties of Co58Fe5Ni10Si11B16 amorphous alloys. Phys. Status Solidi 1983, 80, 195–204. [Google Scholar] [CrossRef]
- Gonzalez, J.; Zhukov, A.P.; Blanco, J.M.; Cobeno, A.F.; Vazquez, M.; Kulakowski, K. Evaluation of the saturation magnetostriction in nearly zero magnetostrictive glass-coated amorphous microwires. J. Appl. Phys. 2000, 87, 5950. [Google Scholar] [CrossRef]
- Narita, K.; Yamasaki, J.; Fukunaga, H. Measurement of saturation magnetostriction of a thin amorphous ribbon by means of small-angle magnetization rotation. IEEE Trans. Magn. 1980, 16, 435–439. [Google Scholar] [CrossRef]
- Hernando, A.; Vazquez, M.; Madurga, V.; Kronmuller, H. Modification of the saturation magnetostriction constant after thermal treatments for the Co58Fe5Ni10B16Si11 amorphous ribbon. J. Magn. Magn. Mater. 1983, 37, 161–166. [Google Scholar] [CrossRef]
- Hernando, A.; Vazquez, M.; Madurga, V.; Ascasibar, E.; Liniers, M. Influence of the anisotropy on the “SAMR” method for measuring magnetostriction in amorphous ribbons. J. Magn. Magn. Mater. 1986, 61, 39–47. [Google Scholar] [CrossRef]
- Zhukova, V.; Blanco, J.M.; Zhukov, A.; Gonzalez, J. Studies of the magnetostriction of as-prepared and annealed glass-coated Co-rich amorphous microwires by SAMR method. J. Phys. D Appl. Phys. 2001, 34, L113. [Google Scholar] [CrossRef]
- Zhukova, V.; Blanco, J.M.; Zhukov, A.; Gonzalez, J.; Torcunov, A.; Larin, V. Magnetostriction of glass-coated Co-rich amorphous microwires and its dependence on current annealing. J. Magn. Magn. Mater. 2003, 254–255, 94–96. [Google Scholar] [CrossRef]
- Churyukanova, M.; Semenkova, V.; Kaloshkin, S.; Shuvaeva, E.; Gudoshnikov, S.; Zhukova, V.; Shchetinin, I.; Zhukov, A. Magnetostriction investigation of soft magnetic microwires. Phys. Status Solidi A 2015, 213, 363–367. [Google Scholar] [CrossRef]
- Zhukov, A.; Churyukanova, M.; Kaloshkin, S.; Sudarchikova, V.; Gudoshnikov, S.; Ipatov, M.; Talaat, A.; Blanco, J.M.; Zhukova, V. Magnetostriction of Co–Fe-based amorphous soft magnetic microwires. J. Electron. Mater. 2016, 45, 226–234. [Google Scholar] [CrossRef]
- Gudoshnikov, S.; Churyukanova, M.; Kaloshkin, S.; Zhukov, A.; Zhukova, V.; Usov, N.A. Investigation of the properties of Co-rich amorphous ferromagnetic microwires by means of small angle magnetization rotation method. J. Magn. Magn. Mater. 2015, 387, 53–57. [Google Scholar] [CrossRef]
- Popova, A.V.; Odintsov, V.I.; Menshov, S.A.; Kostitsyna, E.V.; Tarasov, V.P.; Zhukova, V.; Zhukov, A.; Gudoshnikov, S.A. Continuous control of a resistance in Corich amorphous ferromagnetic microwires during DC Joule heating. Intermetallics 2018, 99, 39–43. [Google Scholar] [CrossRef]
- Gudoshnikov, S.; Odintsov, V.; Liubimov, B.Y.; Menshov, S.; Popova, A.; Tarasov, V. Correlation of electrical and magnetic properties of Co-rich amorphous ferromagnetic microwires after DC Joule heating treatment. J. Alloys Compd. 2020, 845, 156220. [Google Scholar] [CrossRef]
- Kozlov, I.V.; Elmanov, G.N.; Prikhodko, K.E.; Kutuzov, L.V.; Tarasov, B.A.; Mikhalchik, V.V.; Svetogorov, R.D.; Mashera, V.S.; Gorelikov, E.S.; Gudoshnikov, S.A. The evolution of structure and magnetoimpedance characteristics of amorphous Co69Fe4Cr4Si12B11 microwires under heat treatment. J. Magn. Magn. Mater. 2020, 493, 165681. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gudoshnikov, S.; Grebenshchikov, Y.; Popova, A.; Tarasov, V.; Gorelikov, E.; Liubimov, B. Angle Magnetization Rotation Method for Characterizing Co-Rich Amorphous Ferromagnetic Microwires. Actuators 2021, 10, 93. https://doi.org/10.3390/act10050093
Gudoshnikov S, Grebenshchikov Y, Popova A, Tarasov V, Gorelikov E, Liubimov B. Angle Magnetization Rotation Method for Characterizing Co-Rich Amorphous Ferromagnetic Microwires. Actuators. 2021; 10(5):93. https://doi.org/10.3390/act10050093
Chicago/Turabian StyleGudoshnikov, Sergey, Yury Grebenshchikov, Anastasya Popova, Vadim Tarasov, Evgeny Gorelikov, and Boris Liubimov. 2021. "Angle Magnetization Rotation Method for Characterizing Co-Rich Amorphous Ferromagnetic Microwires" Actuators 10, no. 5: 93. https://doi.org/10.3390/act10050093
APA StyleGudoshnikov, S., Grebenshchikov, Y., Popova, A., Tarasov, V., Gorelikov, E., & Liubimov, B. (2021). Angle Magnetization Rotation Method for Characterizing Co-Rich Amorphous Ferromagnetic Microwires. Actuators, 10(5), 93. https://doi.org/10.3390/act10050093




