A Geometrical Method for Sound-Hole Size and Location Enhancement in Lute Family Musical Instruments: The Golden Method

Abstract

This paper presents a new analytical approach, the Golden Method, to enhance sound-hole size and location in musical instruments of the lute family in order to obtain better sound damping characteristics based on the concept of the golden ratio and the instrument geometry. The main objective of the paper is to increase the capability of lute family musical instruments in keeping a note for a certain time at a certain level to enhance the instruments’ orchestral characteristics. For this purpose, a geometry-based analytical method, the Golden Method is first described in detail in an itemized feature. A new musical instrument is then developed and tested to confirm the ability of the Golden Method in optimizing the acoustical characteristics of musical instruments from a damping point of view by designing the modified sound-hole. Finally, the new-developed instrument is tested, and the obtained results are compared with those of two well-known instruments to confirm the effectiveness of the proposed method. The experimental results show that the suggested method is able to increase the sound damping time by at least 2.4% without affecting the frequency response function and other acoustic characteristics of the instrument. This methodology could be used as the first step in future studies on design, optimization and evaluation of musical instruments of the lute family (e.g., lute, oud, barbat, mandolin, setar, and etc.).

1. Introduction

The stringed musical instrument family is the largest family in an orchestra. Therefore, the quality of these instruments plays a vital role in the performance and quality of the whole orchestra. The main orchestral requirements for these instruments are continuous playing range, fast melodic and run playing, ability to play several musical notes at the same time, and playing numerous different articulations. These requirements should be translated into acoustic characteristics to enable instrument makers to provide more practical instruments. One of these characteristics is the instrument capability in keeping a note for a certain time at a certain level (damping characteristics). In other words, the higher the damping time of a stringed musical instrument, the wider the playing range of the instrument.

In stringed musical instruments, the soundboard plays a vital role in the instrument performance. In fact, this element performs the most important effect in the frequency response of the resonator to the acoustical waves because it transfers sound waves between strings and the resonator. In 2015, Kamalian and Mobasseri (2015) (and especially setar1 in their research) showed that one third of the instrument sound is generated by the sound box and the rest (2/3) is from the sundboard plate. soundboards usually contain openings called sound-holes to help acoustic instruments project their sound more efficiently (Figure 1). Sound-holes have different shapes and sizes in different instruments depending on the area of the soundboard, range of frequencies derived by resources (strings), volume of the air inside the sound box, and stiffness of the plate. In 2015, researchers at MIT published an analysis charting the evolution and improvements in effectiveness of violin F-hole design over time (MIT News 2015; Nia et al. 2015). This paper will focus on sound-holes in lute family musical instruments.

Barbat can be considered as one of the first engineered prototypes of lute and one of the oldest instruments in the world, which probably originated in central Asia (Marcel-Dubois 1941). The oldest pictorial representations of this instrument are found in the first-century BC (Vyzgo 1980) of Ḵaḷčayān in North Bactria (present-day South Uzbekistan), while a more “clear cut” depiction of the barbat from Gandhara sculpture dates to the 2nd–4th centuries AD. According to Encyclopedia Iranica (1988), this type of instrument could have been introduced by the Kushans and was later adopted by the Persians. By the 7th century, the barbat was developed by the Arabs into its current form, called the oud. The modern Persian barbat is almost the same as the oud, although differences include a smaller body, longer neck and a slightly raised fingerboard.

Even in initial formations of these instruments, sound-holes as effective elements in acoustic behaviour of instruments were considered by designers and manufacturers. Historically, it is not possible to exactly discover the emergence of the sound-holes in ancient musical instruments, but a comprehensive historical survey on sound-hole design shows that in the middle ages they could be observed in some classical paintings (Figure 2) showing stringed instruments like the Rebec, Dulcimer and Psaltery in Europe and barbat in Persia (Rault 2014). There is other evidence that shows circular sound-holes in early violin ancestors in the 10th century (Engel 1883), semi-circular in the 12th century (Baines 1992), crescent in the 13th century (Sandys and Forster 1884), semi-circular strips in the 13th century (Van der Straeten 1968), and C-holes in the 13th and 14th centuries (Coates 1985). Between the 13th and 17th centuries, the sound-hole shape and size were approximated by simple circular and elliptical geometries in lute family musical instruments (Hutchins 1990; Cremer 1984), and by f-shape geometry in violin family instruments (Itakawa and Kumagai 1952; Shaw 1990). The instrument makers usually modified the errors in sound-holes using empirical fitting factors (Bissinger 1992). The last basic changes occurred in the 16th and 17th centuries. The lute rosset size and shape were modified by Warwick Hans in 1540, Wendelin Tieffenbrucker in 1590, Wendelio Venere in 1592, Padov in 1595, and Sebastian Schelle in 1744 (Barber and Harris 2016). Subsequently, the main shape of the sound-holes in violin and lute families remained almost unchanged.

In recent centuries, studies on air resonance fundamentals with application to musical instruments were initiated by Savart (1976), Von Helmholtz (1860; 1954), Rayleigh (1945), Lamb (1932), and Mcpherson (2002). However, with respect to Tavakoli Nia (2010, p. 3), “the design knowledge in such sound-holes accumulated by time is still uncovered”. In 2011, Tavakoli Nia started analysing sound-holes and the concept behind their geometrical design. He showed that the emergence of circular sound-holes in the lute family was due to passing the majority of air flow through the near-the-edge area of the opening (Tavakoli Nia 2010). He comprehensively investigated this area and finally concluded that his approach could be a good starting point for the modification of the instruments for musicians and instrument makers.

There are several parameters that have effects on acoustic characteristics of a musical instrument (e.g., material, age of the instrument and used wood, weather, manufacturing procedure and mechanism, geometry, etc.). All of the above-mentioned parameters can affect the instrument behaviour, quality, and capabilities. Some of them, like material, age, wood, and weather, are dynamic indices and subject to change and/or deteriorate over time and therefore require comprehensive research and facilities for examination purposes. However, other parameters like geometrical design and manufacturing procedure and mechanism are not time dependent and can be studied more easily.

Although the whole behaviour of a musical instrument is the result of the superposition of the effects of all parameters, this paper, as the first step, will focus on the effect of sound-hole design and location on the capability of the musical instrument in keeping a note which can enhance the orchestral characteristics of stringed musical instruments. Since the circular sound-hole has been proved as the optimized shape for the lute family based on the literature and especially an MIT researchers’ report (Tavakoli Nia 2010), the assumption here is that the circle is the optimized shape and only the size and location will be discussed. For this purpose, an analytical approach based on the geometry of instrument, the concept of the golden ratio, and their magical effects is proposed using the several years of experience and academic studies of the authors in the fields of optimization and musical instrument making. This methodology is called the Golden Method by the authors.

• The method is described in an itemized flowchart form in chapter two.
• Two well-known instruments will be checked with this approach as case studies in order to show the effectiveness of the Golden Method.
• A new musical instrument will be developed using the proposed method and its damping capability would be compared with that of two well-known instruments to confirm the effectiveness of the golden method in optimizing the sound-hole size and location in lute family musical instruments from a damping point of view.

2. Methodology

The golden ratio concept is used for the first time here for sound-hole size and location design in musical instruments of the lute family in order to enhance the damping capability. The golden ratio has been claimed to have held a special fascination for at least 2400 years, though without reliable evidence (Markowsky 1992). According to Mario Livio:

“Some of the greatest mathematical minds of all ages, from Pythagoras and Euclid in ancient Greece, through the medieval Italian mathematician Leonardo of Pisa and the Renaissance astronomer Johannes Kepler, to present-day scientific figures such as Oxford physicist Roger Penrose, have spent endless hours over this simple ratio and its properties. But the fascination with the Golden Ratio is not confined just to mathematicians. Biologists, artists, musicians, historians, architects, psychologists, and even mystics have pondered and debated the basis of its ubiquity and appeal. In fact, it is probably fair to say that the Golden Ratio has inspired thinkers of all disciplines like no other number in the history of mathematics.”

(Livio 2002)

This ratio has also inspired the researchers in the field of acoustics characteristics of instruments. Takegawa (2008) invented a drum with optimized air vent holes using the golden ratio in 2008, and King (2004; 2005) presented a comprehensive research study on locating the Stradivari’s violin hole locations using the golden ratio. The authors spent several hours analyzing, modelling and simulating different assumptions and the manufacturing process to find the optimized sound-hole for specific musical instruments to determine the maximum sound radiation capability. The results of all analytical and trial and error procedures are summarized here entitled: Golden Method. The assumptions used for this method are:

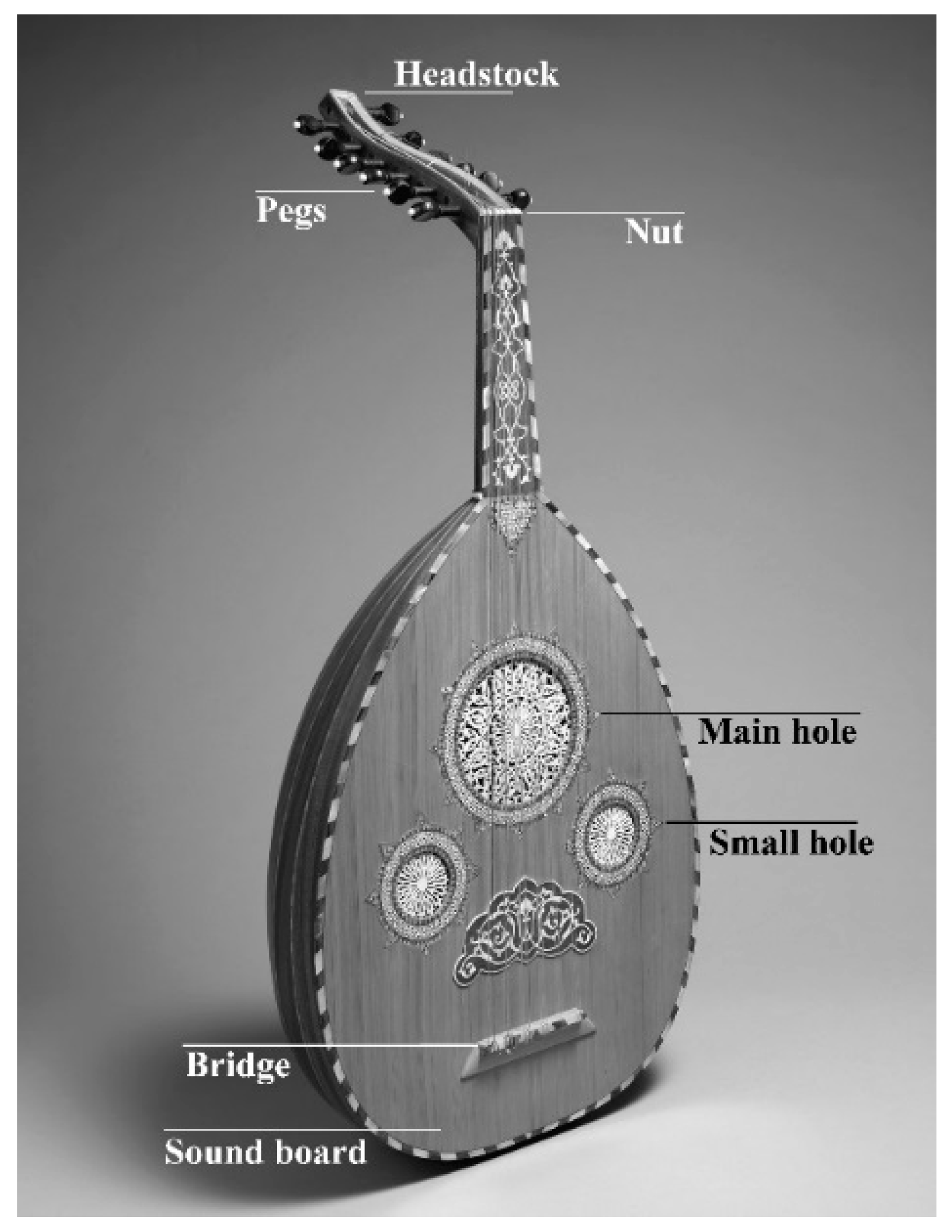
• The circular sound-hole has been assumed as the optimized shape for the lute family (based on the literature).
• The material and mechanical properties of the instrument are not discussed in this paper because of their dynamic-index nature. These parameters can be studied in a separate standalone research work.
• The main objective of the proposed method is to enhance the musical note keeping capability of the instrument for orchestral consideration.

Moreover, without loss of generality, the lute is basically used to describe the method because it is a world-wide well-reputed instrument. However, the procedure is exactly the same for all lute family musical instruments.

The Golden Method is presented for a typical professional lute:

In a musical instrument of the lute family, to optimize the sound-hole location and size (or to check that a sound-hole is optimized) from a damping capability point of view, perform the following steps:

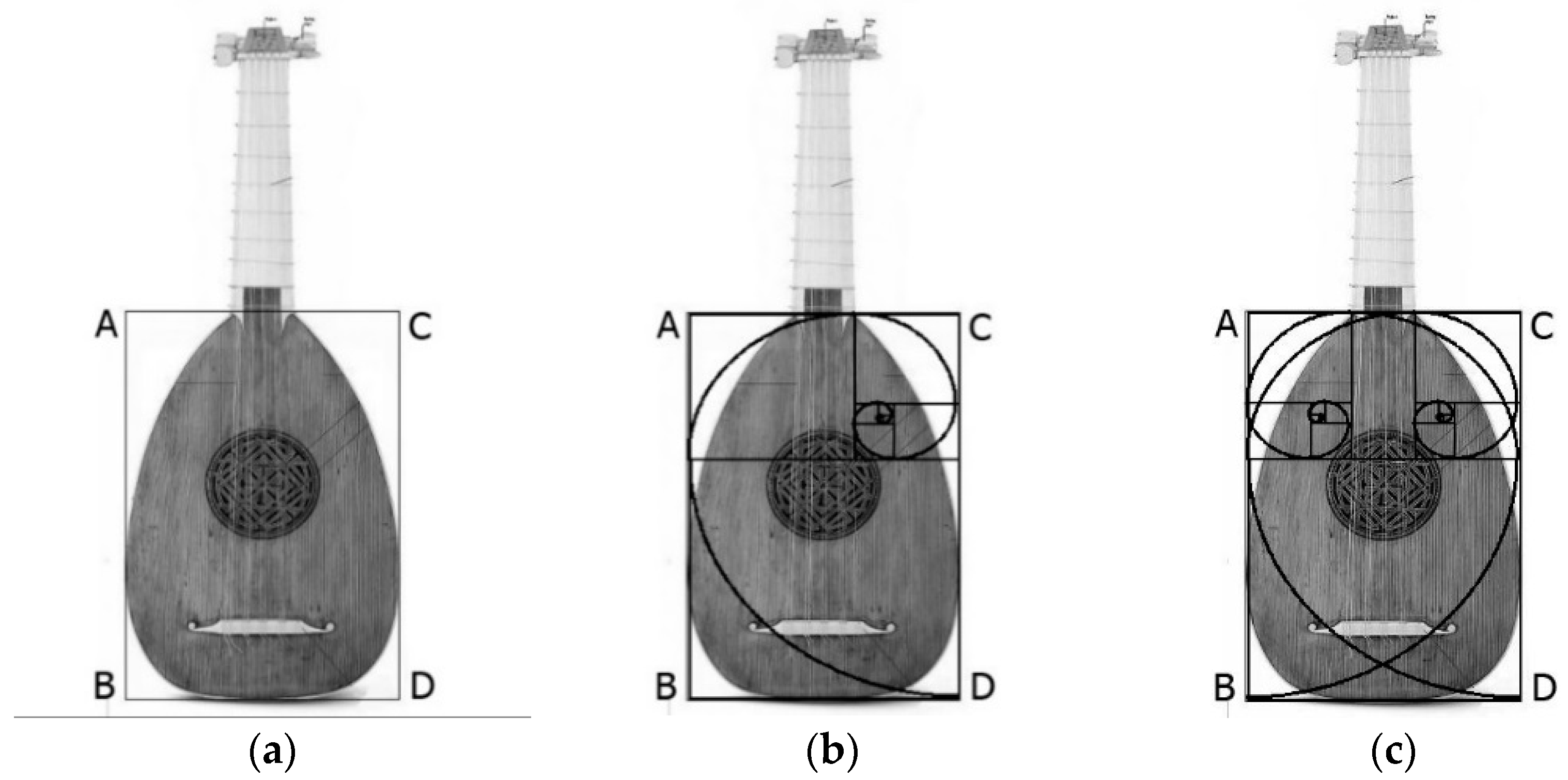
• Plot the rectangle ABCD covering the instrument soundboard and tangent to it as shown in Figure 3a.
• Plot the golden rectangle and its logarithmic spiral from one side of the ABCD as shown in Figure 3b. Full details on how to plot such a golden rectangle and spiral are very straightforward and can be found in (Chang 2002, Hemenway 2005).
• Repeat step 2 from another side of the ABCD as shown in Figure 3c and draw another golden rectangle and logarithmic spiral that mirror those of step 2.
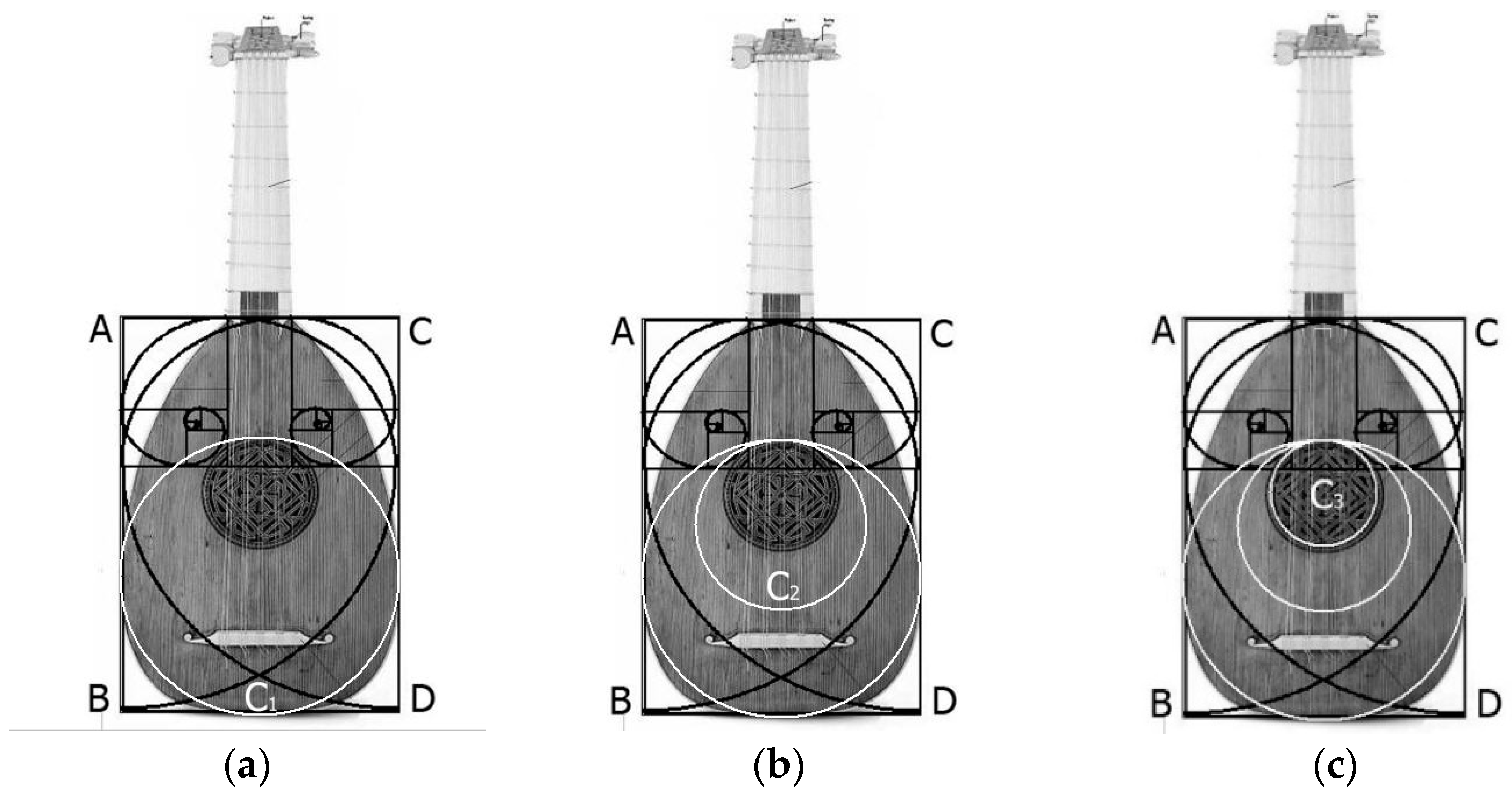
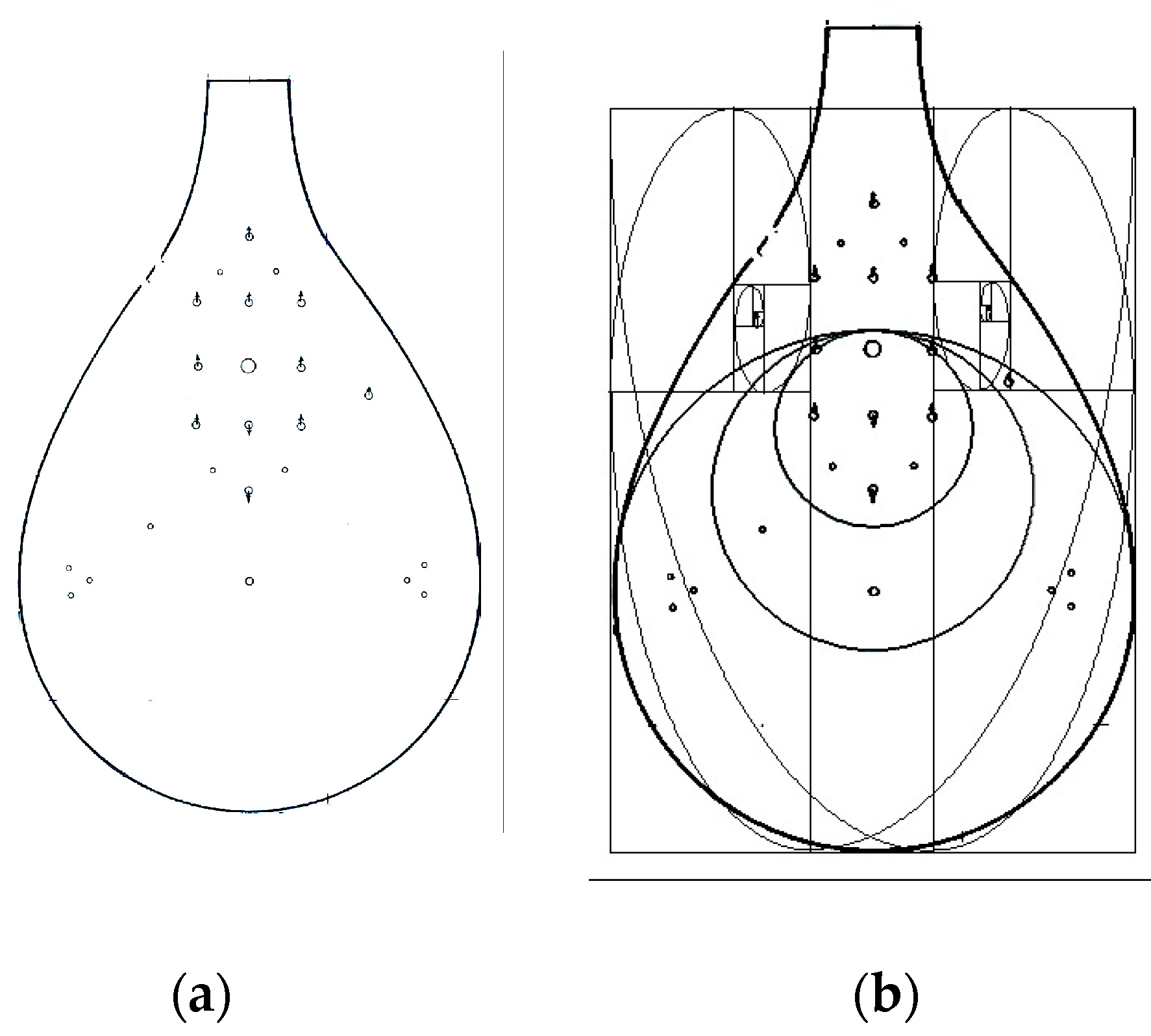
• Plot a circle C₁ tangent over the 3 lines AB, BD, and CD as shown in Figure 4a.
• Plot the first golden circle C₂ so that
  • The vertical diameters and upper vertex of the two circles are common.
  • The diameter ratio: $\frac{\mathit{d}({\mathbf{c}}_{\mathbf{1}})}{\mathit{d}({\mathbf{c}}_{\mathbf{2}})}(\mathbf{diameter}\mathbf{ratio})=\mathbf{1.618}=\mathbf{\Phi }$
• Plot the second golden circle C₃; this circle is drawn like C₂ (but C₃ should follow the above-mentioned conditions for C₂) (Figure 4c).
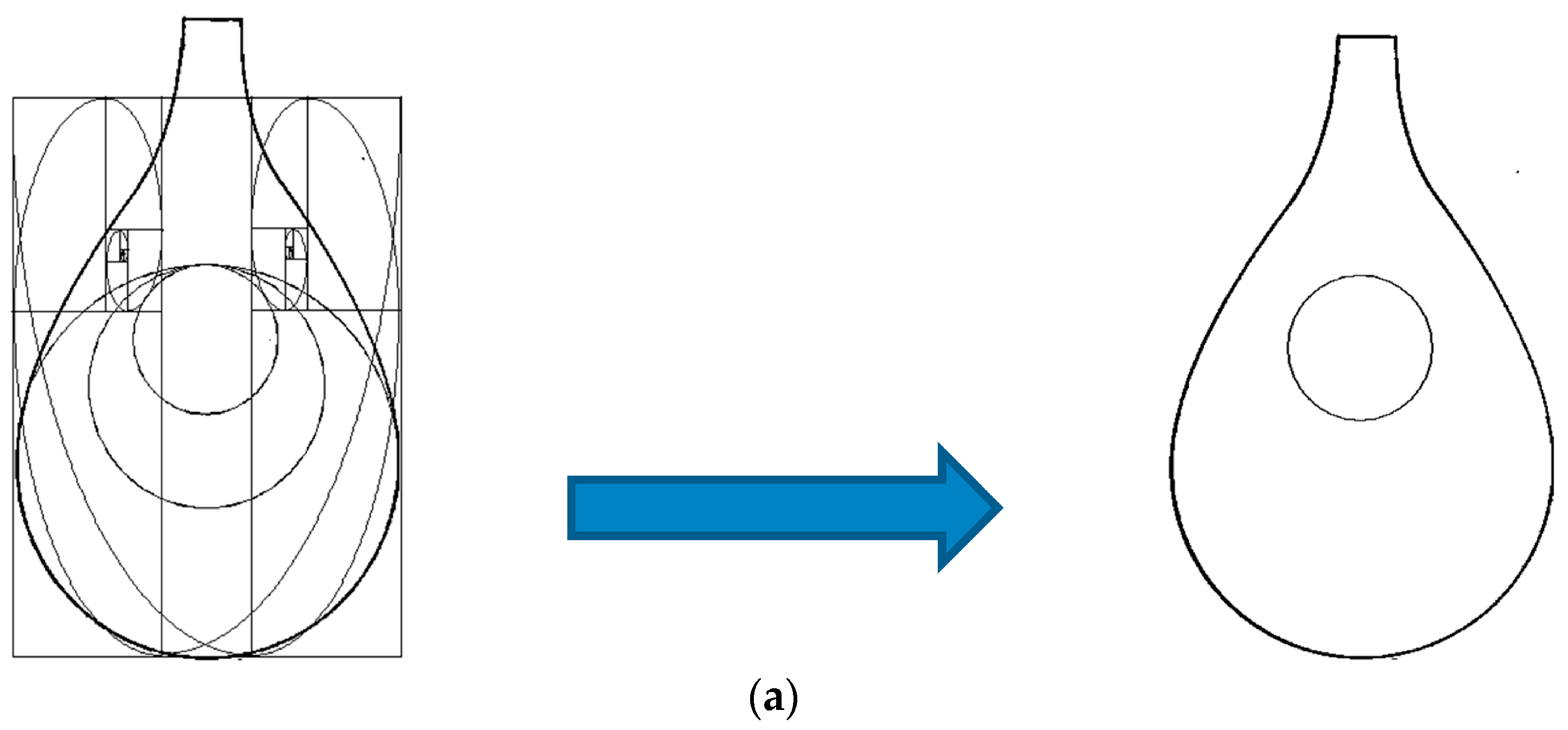
• Now, if C₃ touches the two golden spirals (Figure 5a), this can locate the precise location and size of the optimized sound-hole.

Note: It rarely happens that in an oval soundboard (with an almost correct geometry and proportion) the second golden circle does not touch the golden spirals and does not follow this golden method.

Note that C₃ usually touches the spirals in the 4th golden rectangle (Figure 5b); it can be seen in Figure 5 that C₃ precisely tangents on the sound hole of the manufactured lute. In this way, one can optimize the sound hole of any lute family instrument by relation between geometry and the golden ratio (Φ) or appraise the manufacturing process from the sound-hole design and location point of view.

3. Experimental Evaluation

In order to confirm the effectiveness of the proposed Golden Method, an experimental case study is presented in this section. The setar is selected as the case study. A setar (from seh, meaning “three” and tār, meaning “string”) is a Persian musical instrument. It is a member of the lute family, which is played with the index finger of the right hand. Two and a half centuries ago, a fourth string was added to the setar (Khaleghi 1963). It has 25–27 moveable frets which are usually made of animal intestines or silk. It originated in Persia before the spread of Islam. Figure 6 shows a typical setar (dimensions are in millimetres). The sound box structure of this instrument is similar to that of the lute but only with respect to the size of soundboard and the type of sound-holes.

Setar makers believe that the holes adjust the sound of the instrument. Usually after final shaving of the soundboard, they punch the holes. A review of several setar patterns shows that there is no fixed pattern for making the sound-holes; the setar makers design their pattern based on their manner and experience. One of the main differences between setar patterns is the templates of sound-holes that are bored in the soundboard and guttoral are of the setar. The manufacturers usually find the location of the main hole by using a tuning fork at note of A = 440 Hz. In this way, they vibrate the tuning fork, put it on the bridge area and move it to the neck. The point where the sound reaches its peak is marked, and the procedure is repeated several times to specify the main sound-hole location precisely. The manufacturers punch a 5-mm hole and the other holes should be along the perimeter of this hole (Figure 7) (Shirazi 1990).

Some researchers claim that the main air resonance frequency might be approximated by the linear superposition of the effect of each hole (Shaw 1990; Takegawa 2008). Some conventional types of setar sound-hole patterns are shown in Figure 8.

For comparison, two well-reputed setar patterns are selected in this study:

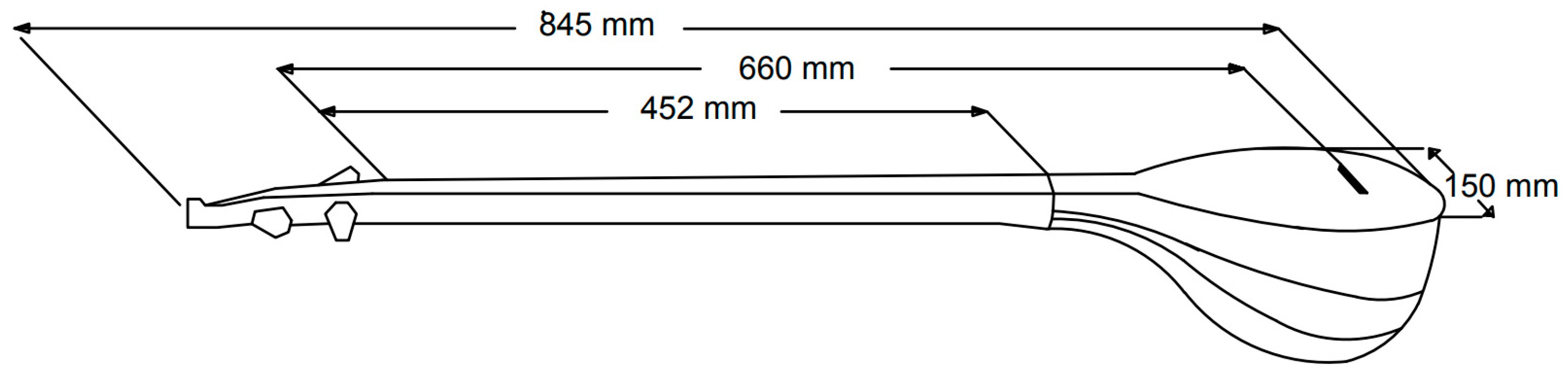
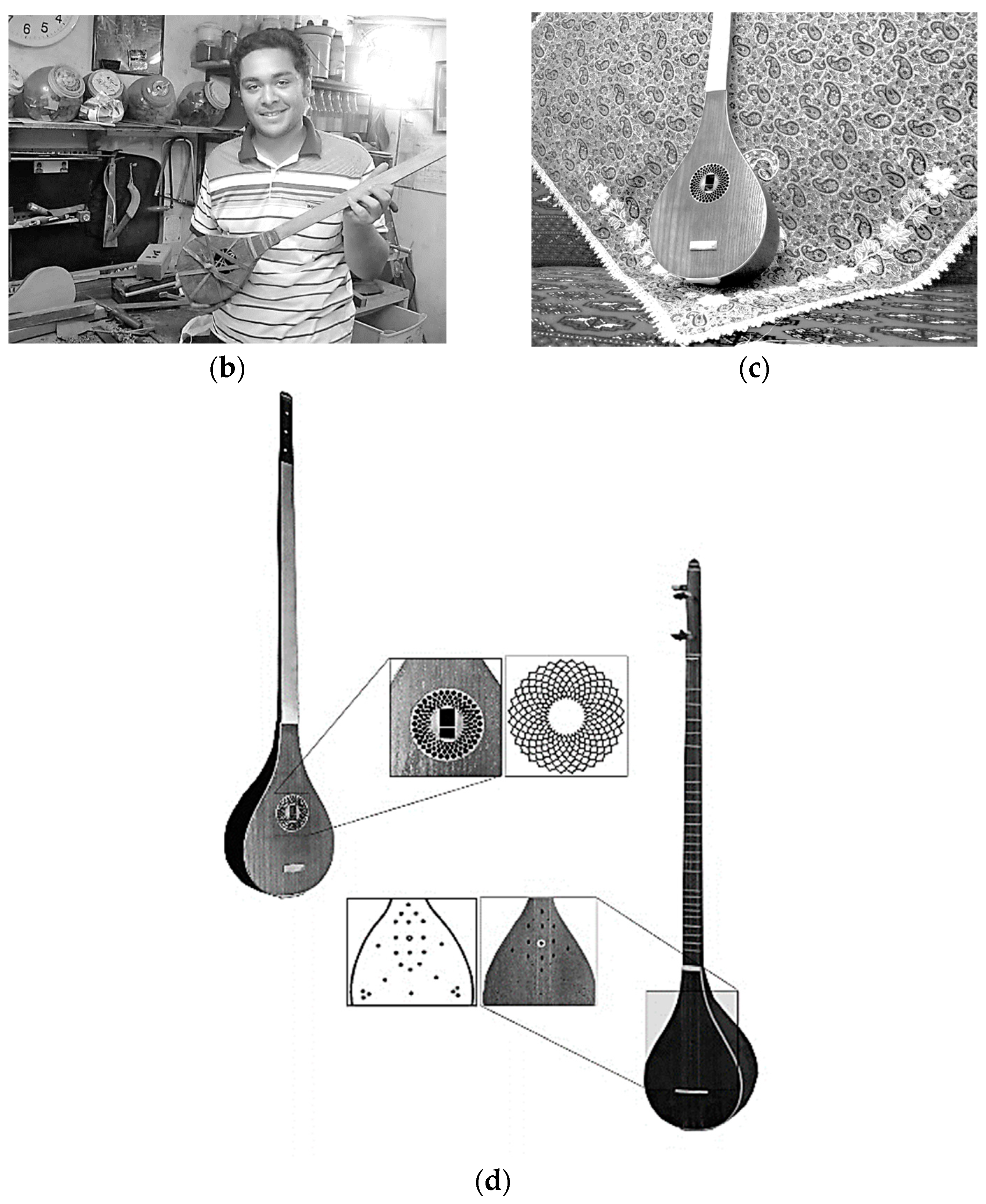
3.1. Case Study I: Delroba2

The first one is called the Delroba, shown in Figure 9a, designed by the legendary classical luthier Mehdi Kamalian (1918–1997) and is a well-preserved example of his work. Here, we analyze the air mode schema of this pattern for optimizing the sound-holes using the Golden Method.

Using the Golden Method described in Section 2, the following pattern shown in Figure 9b is achieved for the Delroba. As shown in this figure, the middle holes as well as the main hole are located inside the golden circle.

3.2. Case Study II: Eshghi3

The second case study is the Eshghi pattern by Mohammad Navaei (1903–1987). Masters of setar making believe that this pattern is the best scale pattern ever designed and implemented. This model has fewer holes than the Delroba. Note that all Eshghi holes are located in the golden circle as shown in Figure 10.

3.3. Case Study III: Mahava4

Finally, in order to prove the effectiveness of the Golden method in enhancing the sound radiation ability, a new musical instrument with an optimized sound-hole was developed to compare with the above-mentioned instruments. We called this the Mahava. It is worthwhile to mention that the Delroba, Eshghi, and Mahava are exactly the same in sound-hole size, location and pattern to ensure fair comparison. Figure 11 shows the construction procedure of the Mahava.

4. Experimental Apparatus and Procedure

After developing the Mahava, experimental tests were carried out on all discussed instruments to confirm the ability of the Golden Method to optimize the sound-hole placement and size. The experimental procedure was to elicit the same note in all instruments and to record the response until it decayed to zero to analyze the sound damping capability of the instruments.

4.1. Data Acquisition System

Figure 12 shows a photograph of the microphone array. Identical RØDE NT1000 Cardioid polar pattern microphones (RØDE Microphones, Silverwater, Australia) were positioned at the vertices and front side of the samples, pointing directly inward. The microphone position was adjusted so that there was exactly 18” (45.72 cm) between the microphone and sample; the axis was aimed toward the opposite vertex in the array. The array was suspended in the center (near the floor) of an 8 × 6 × 3 m studio. The microphones were routed through preamps with a flat frequency response to a Focusrite Scarlett 6i6 sound card (Focusrite Audio Engineering Ltd., High Wycombe, UK). All microphone signal paths were normalized to within 1 dB, using a test tone generator.

4.2. Test Procedure

The main issue in the test procedure was that there was no control over the force of the strike on the string. In fact, one could easily imagine that the harder the pluck, the louder the sound, and the longer the sound would take to decay; this problem would affect the analysis results. To avoid this issue, three musicians who were not aware of the target of the research were selected, and the experiments were performed by them.

Then, sets of “perfect” impulse responses were selected based on the excitation signal. This was done by eliminating any double hammer hits, eliminating any recordings where the researcher commented during the experiment that it was a bad hit, then finally choosing from the remaining possibilities the force hammer impulse with the narrowest and highest peak. Selected signals were transferred digitally to a computer for analysis.

The experiment was performed on the third string of samples that was tuned to 440 Hz. The third string was composed of phosphor bronze or brass. It has a minimum tensile strength of 900 N/mm². The diameter of the third string of a Persian setar is normally 0.02 mm thicker than that of steel strings. (The diameter of steel strings is normally 0.2 mm, and the thickness of bronze strings is 0.22 mm).

5. Results and Analysis

Figure 13 shows one time record graph for the Mahava. It is worth mentioning that experimental tests were conducted several times as discussed in the previous section, and the worst-case scenario results were selected to gain confidence about the validity of the analysis. Figure 14 compares the time response of the Delroba (Kamalian), Eshghi, and Mahava. The results obtained from the experiments are summarized in

Table 1. Time response results.

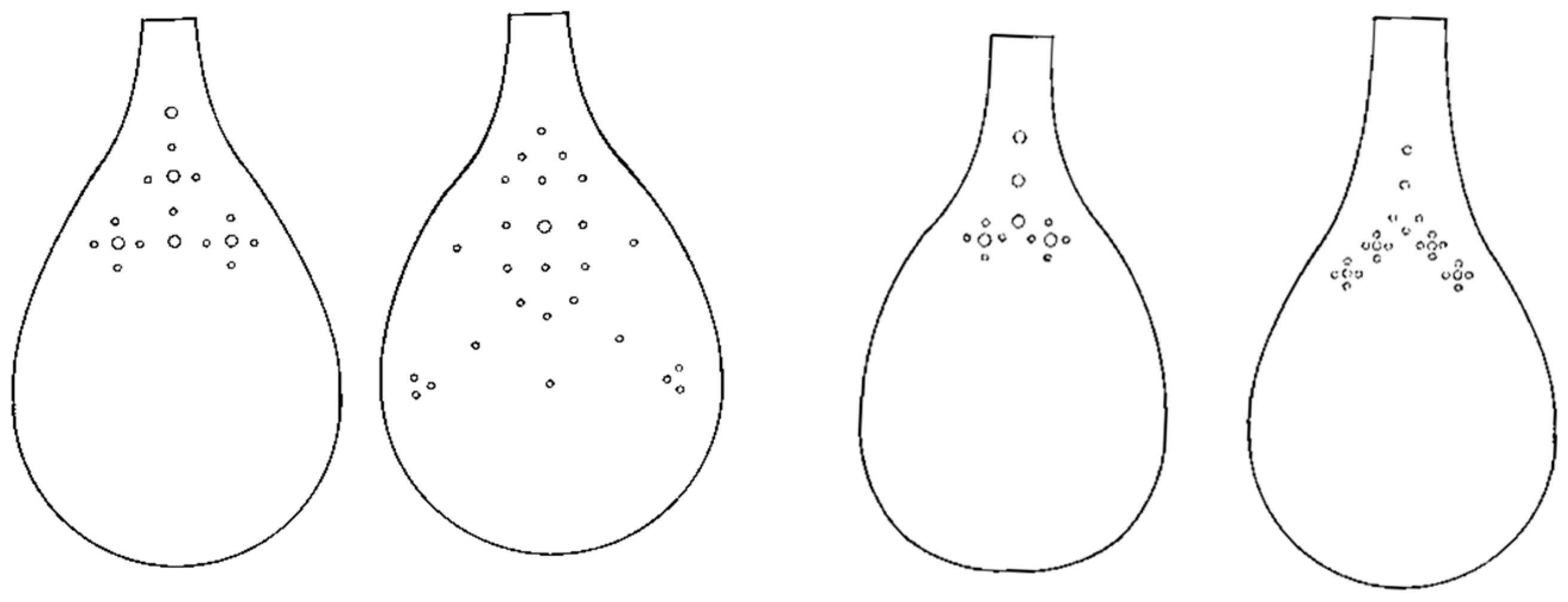
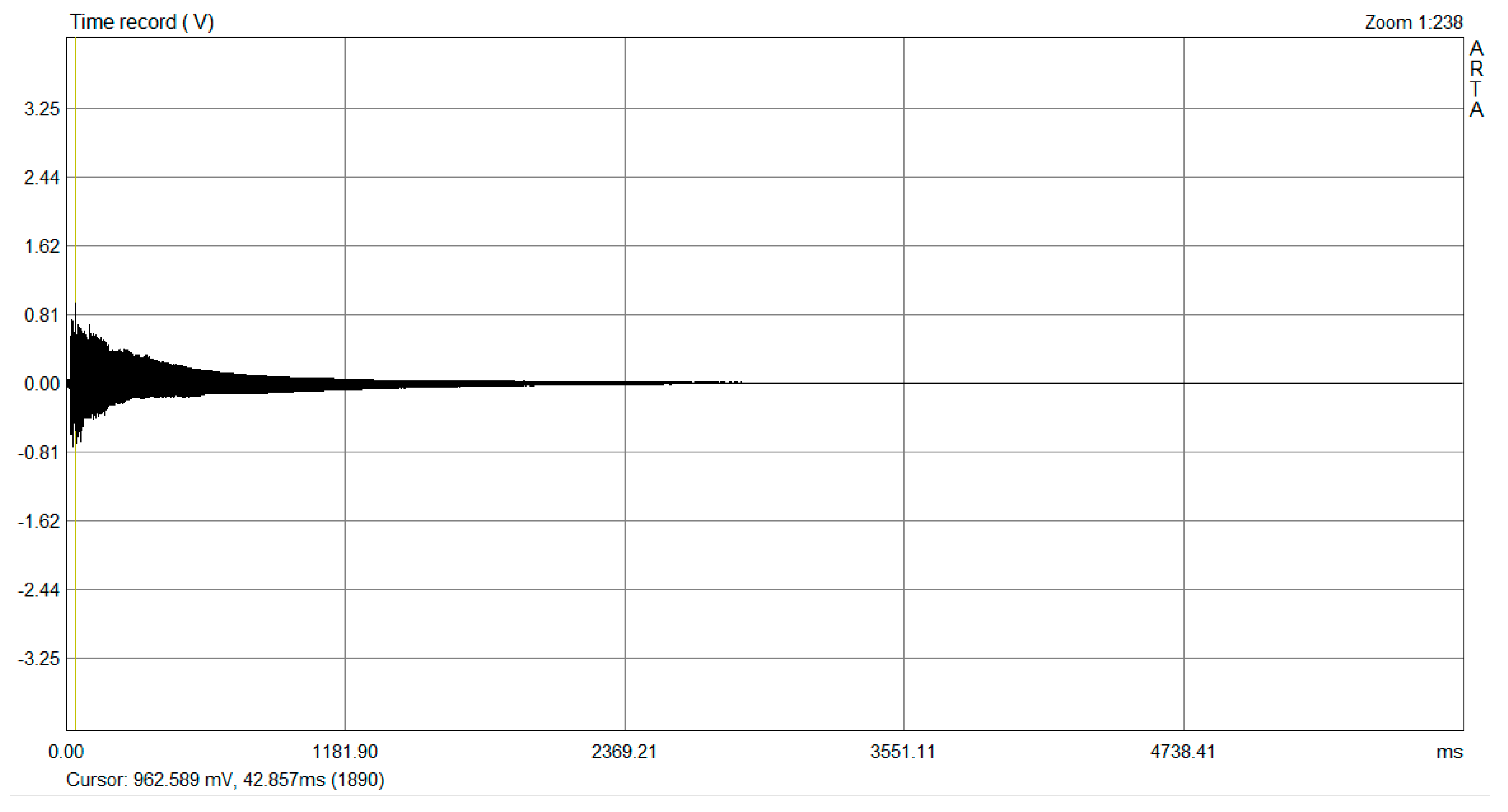
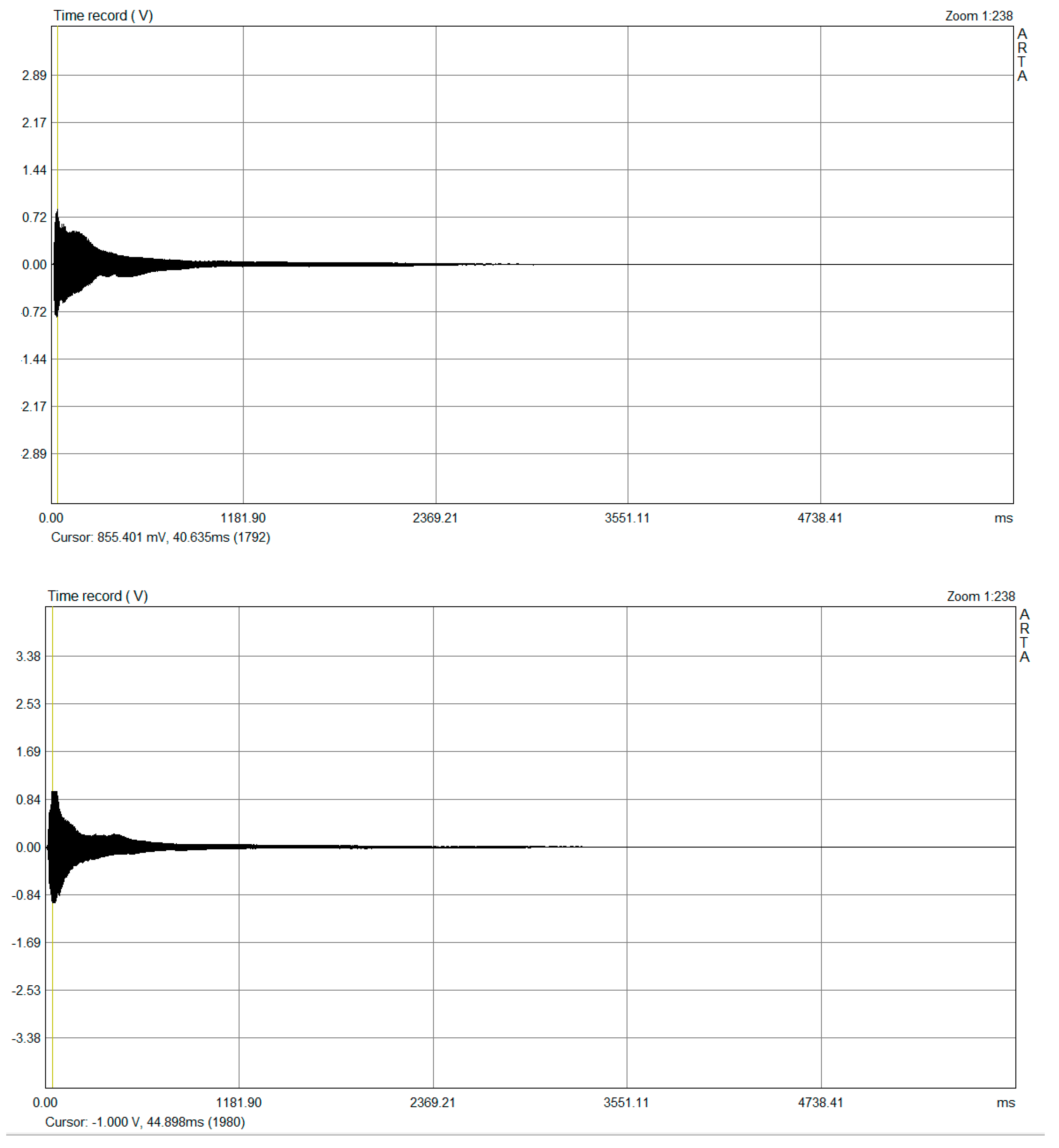
	Maximum Amplitude (mV)	Maximum Amplitude Time (ms)	Damping Time (ms) §
Delroba	962	42.9	3004
Eshghi	855	40.6	2955
Mahava	1000	44.8	3155

§ The time in which (and after which) the response amplitude would remain in the band of ±5% of the final amplitude.

. As shown in this table, the Golden Method improved the radiation time of the Mahava by 2.4% and 3.2% compared with the Delroba and Eshghi, respectively. Moreover, the maximum recorded amplitude of the Mahava was slightly higher than that of the two other instruments.

In other words, Table 1 confirms that the Golden Method is able to enhance the capability of the setar in note keeping, even in comparison with well-reputed setars. Consequently, the results confirm the effectiveness of the proposed method in sound-hole size and location design in lute family musical instruments to promote their orchestral capabilities.

Moreover, the frequency response function (FRF) of the three instruments is also shown in Figure 15. As shown in this figure, the FRF trends are the same for all instruments and consequently, the golden method does not affect the frequency characteristics of the instruments. In other words, it is not a trade-off approach.

As shown in the above figures and tables, the Mahava is superior to the other well-known instruments from the sound radiation point of view, which is an important orchestral characteristic of a musical instrument. The other characteristics of the Mahava are the same as those of the Delbora and Eshghi. Consequently, the Golden Method could be introduced as a new successful approach to design and locate optimized sound-holes in musical instruments of the lute family.

6. Conclusions

The paper focused on enhancing the damping characteristic of lute family musical instruments which is one of the most important orchestral characteristics of stringed instruments. The Golden Method, a geometry-based analytical approach using the golden ratio concept, is introduced as a novel procedure to optimize the size and location of the sound-holes in musical instruments of the lute family (e.g., lute, oud, barbat, mandolin, setar, and etc.). The proposed method claims that if the golden circle generated by the golden method is tangent to the golden spirals of the soundboard, it locates the optimized location and size of the sound-hole. To prove this claim, three case studies were considered including two well-reputed musical instruments and a new setar, Mahava, developed to show the effectiveness of the Golden Method in increasing the sound damping capability of musical instruments. The results from experiments confirm that the instrument constructed based on the Golden Method is superior to other well-known instruments from a sound radiation point of view without affecting other acoustic characteristics. Consequently, the Golden Method could be used in future studies to optimize the sound-hole design procedure in lute family musical instruments.