Influence of Stator/Rotor Torque Ratio on Torque Performance in External-Rotor Dual-Armature Flux-Switching PM Machines
Abstract
1. Introduction
2. Harmonic Analysis of Airgap Fields
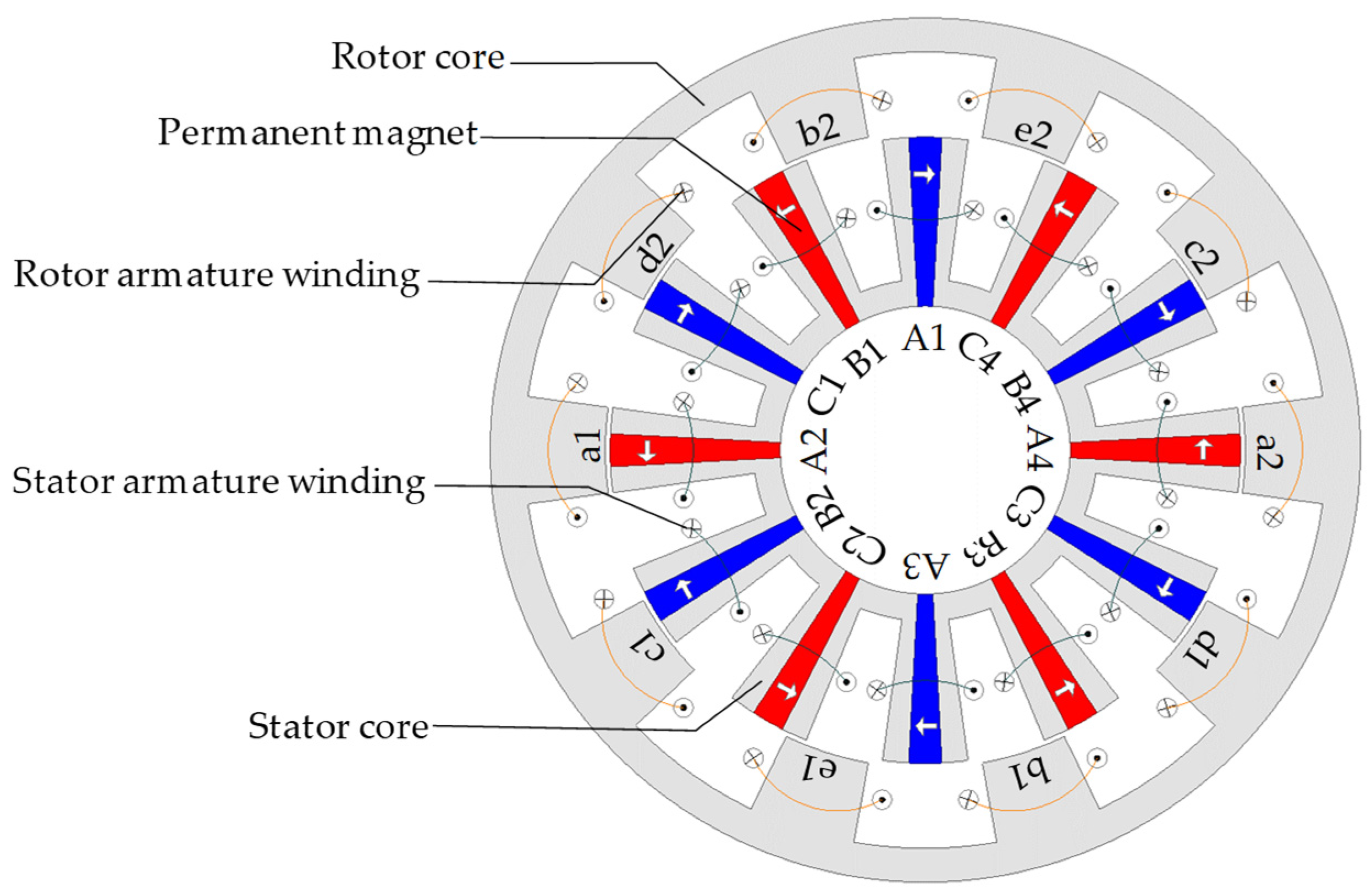
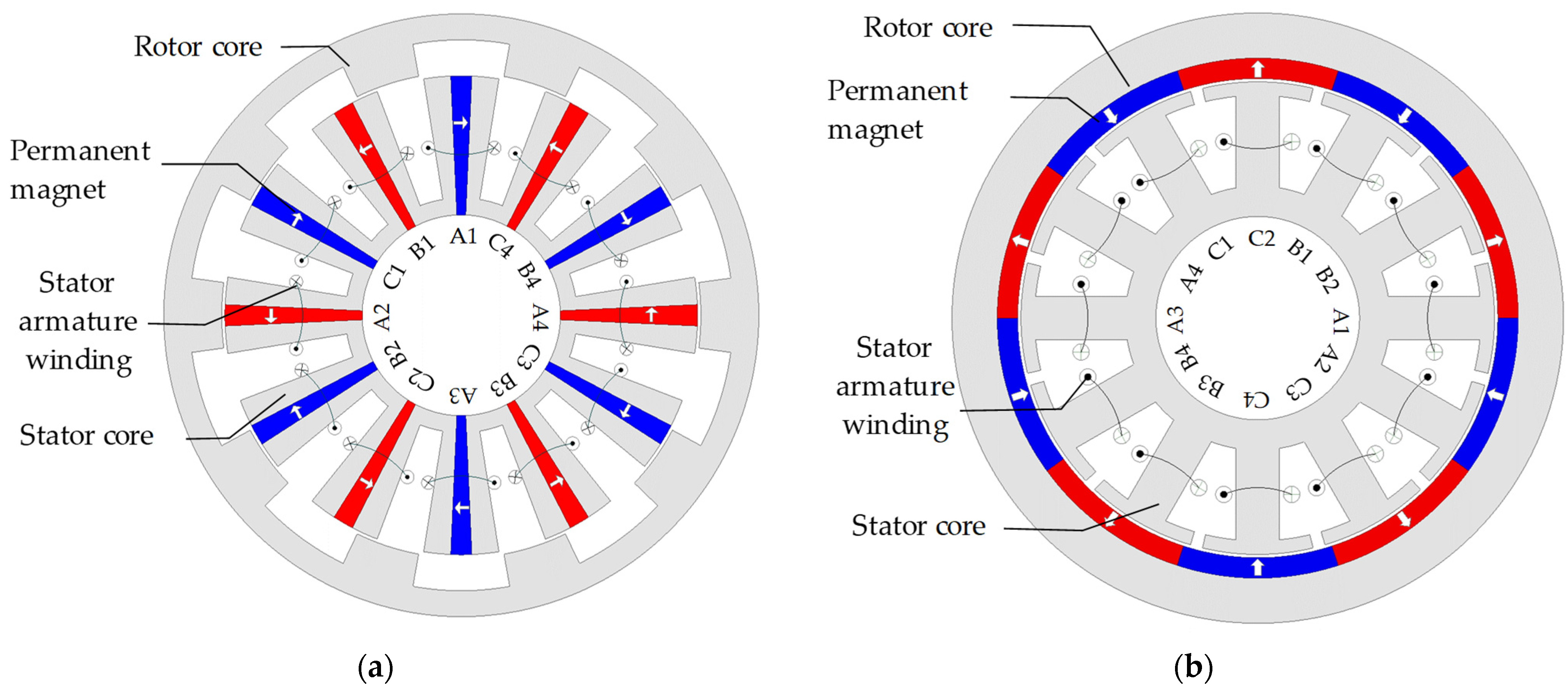
2.1. Topology of ER-DA-FSPM Machine
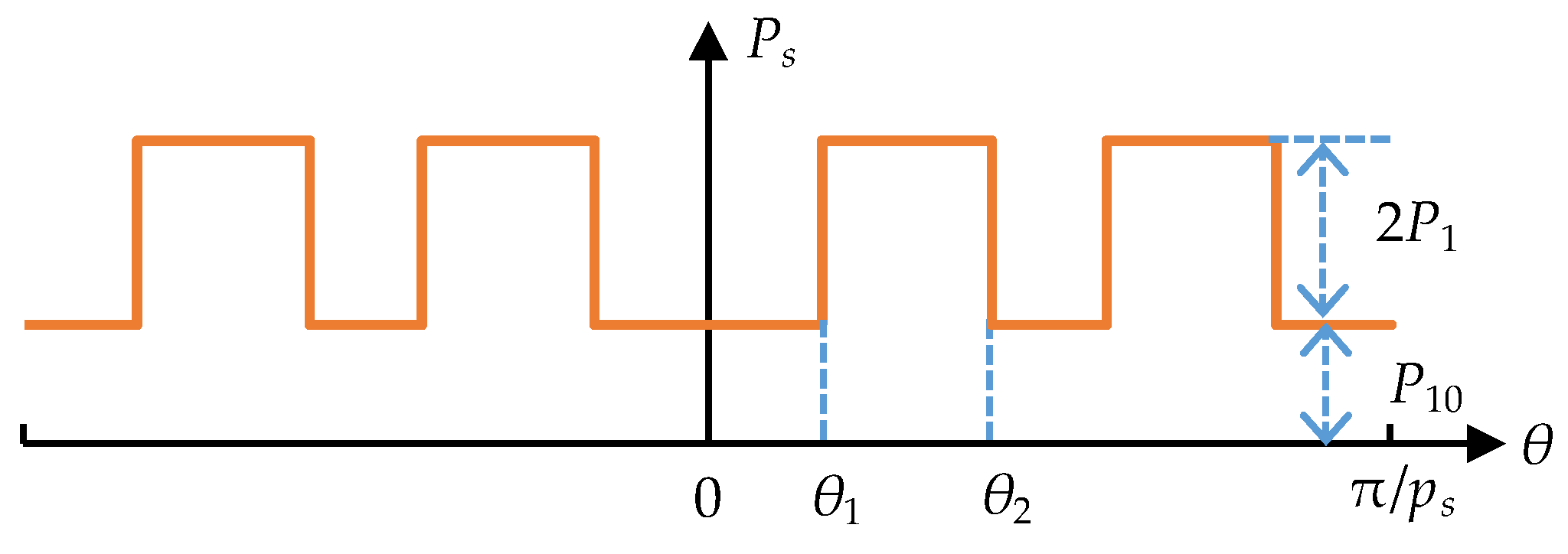
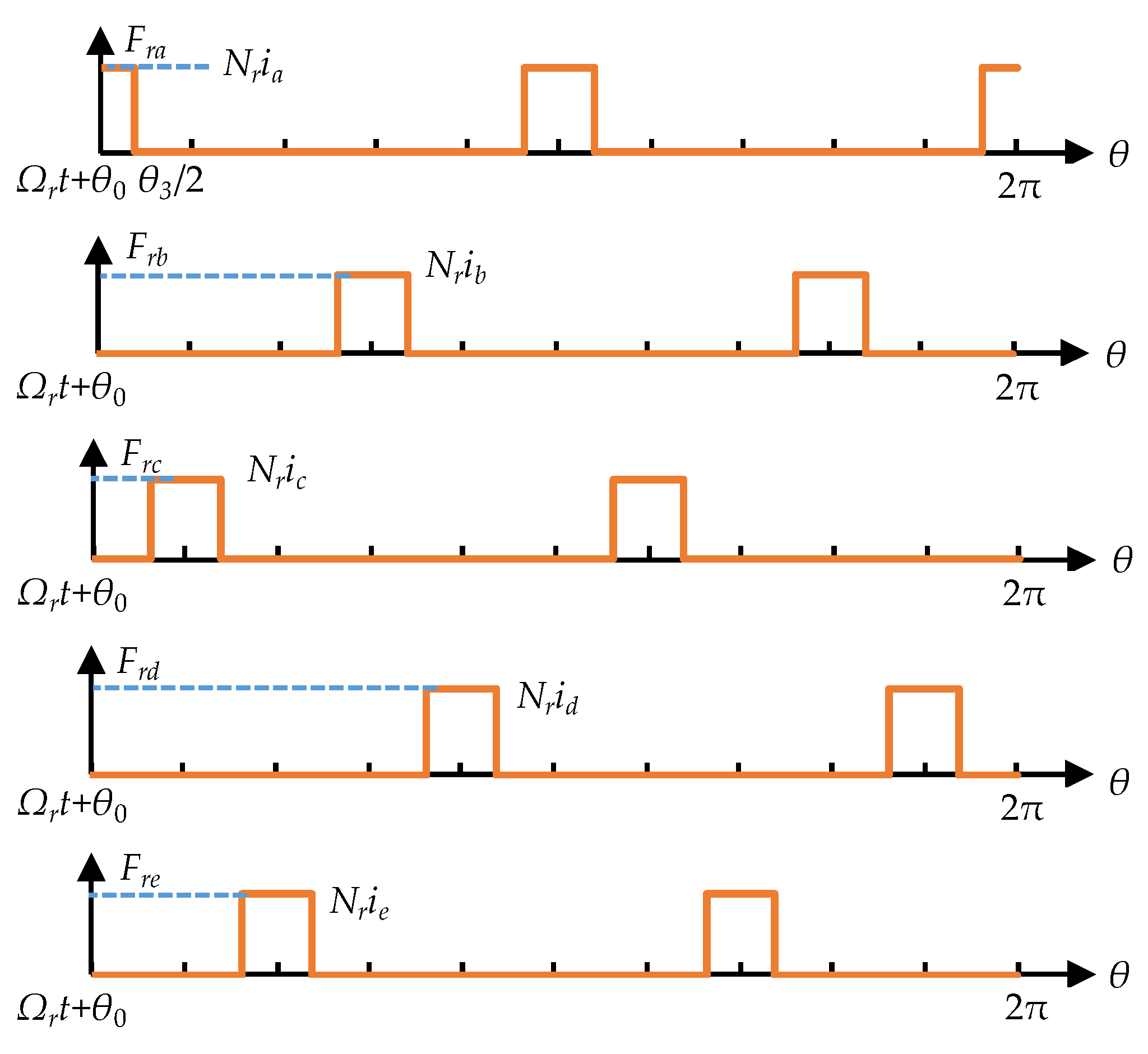
2.2. Harmonic Analysis of Airgap Flux Density
2.3. FE Validation
3. Influence of Stator/Rotor Torque Ratio
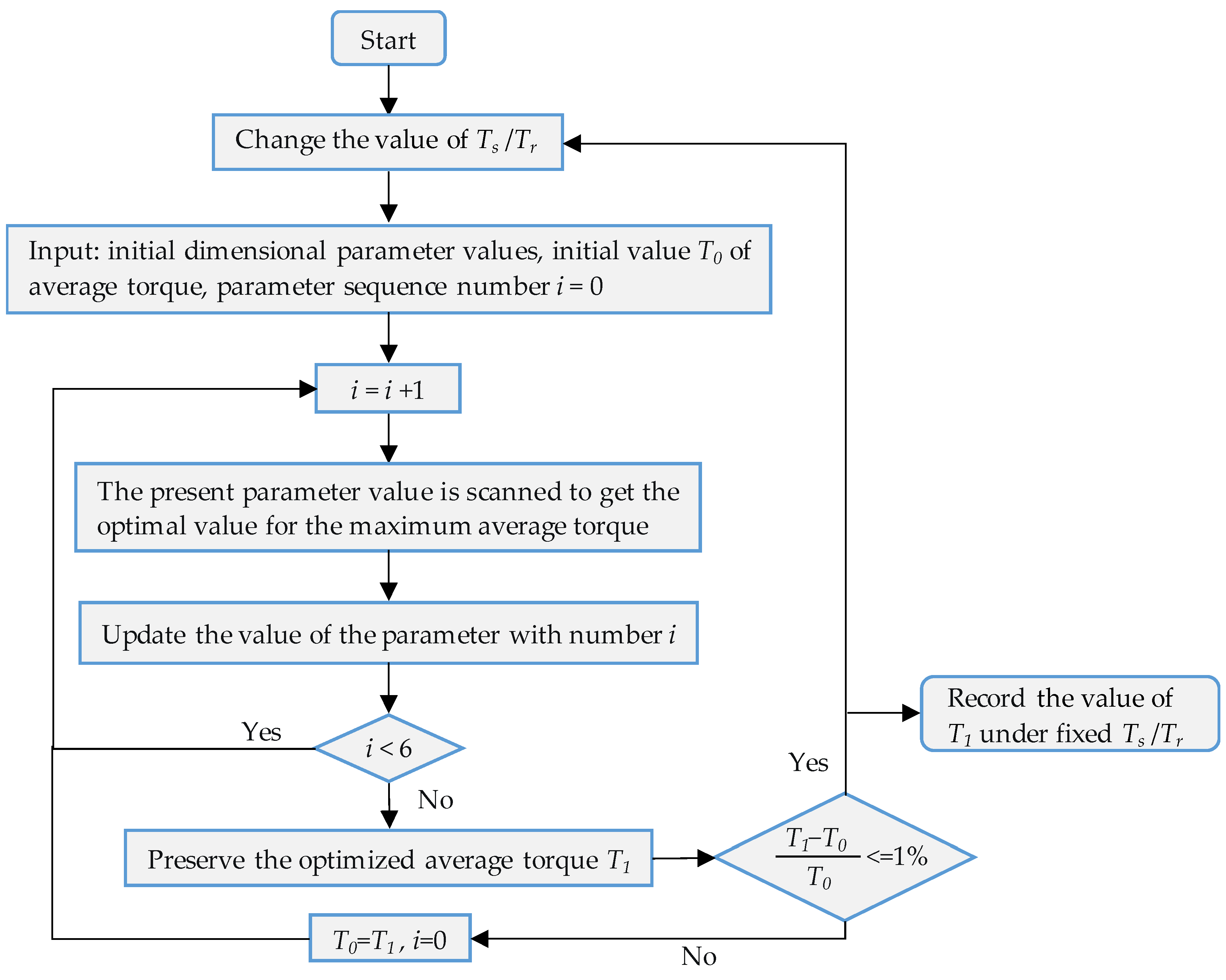
3.1. Parameter Optimization
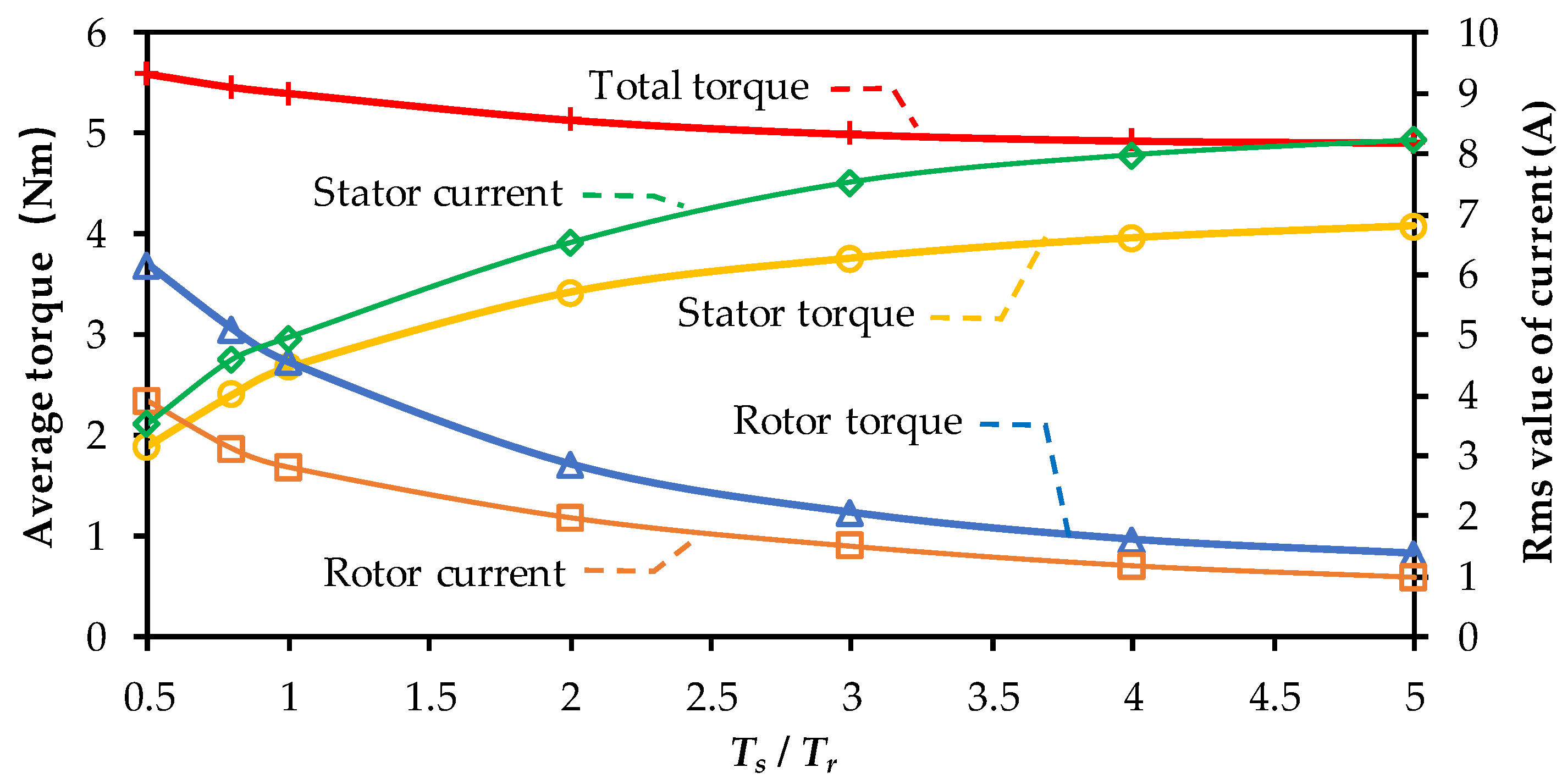
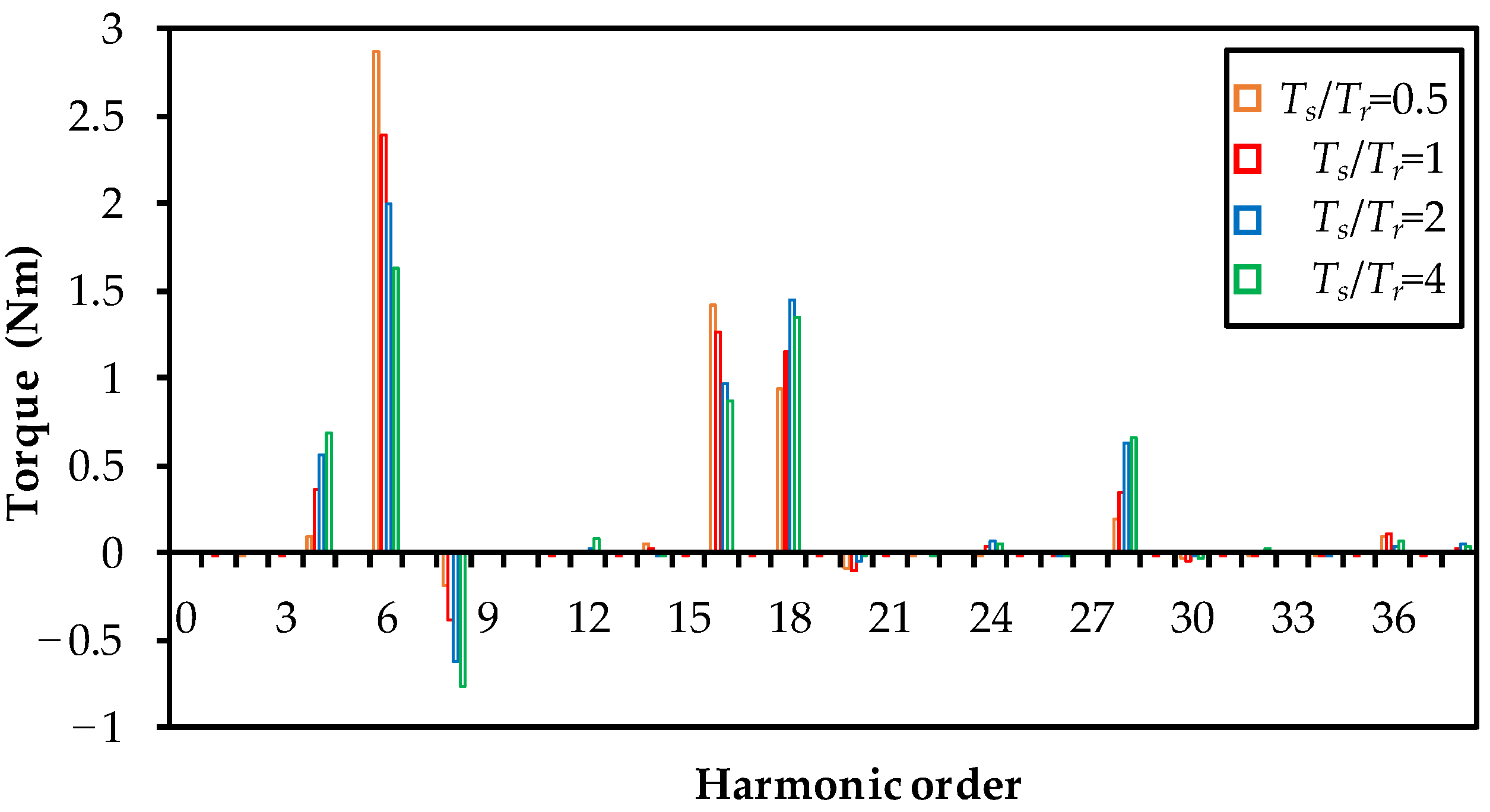
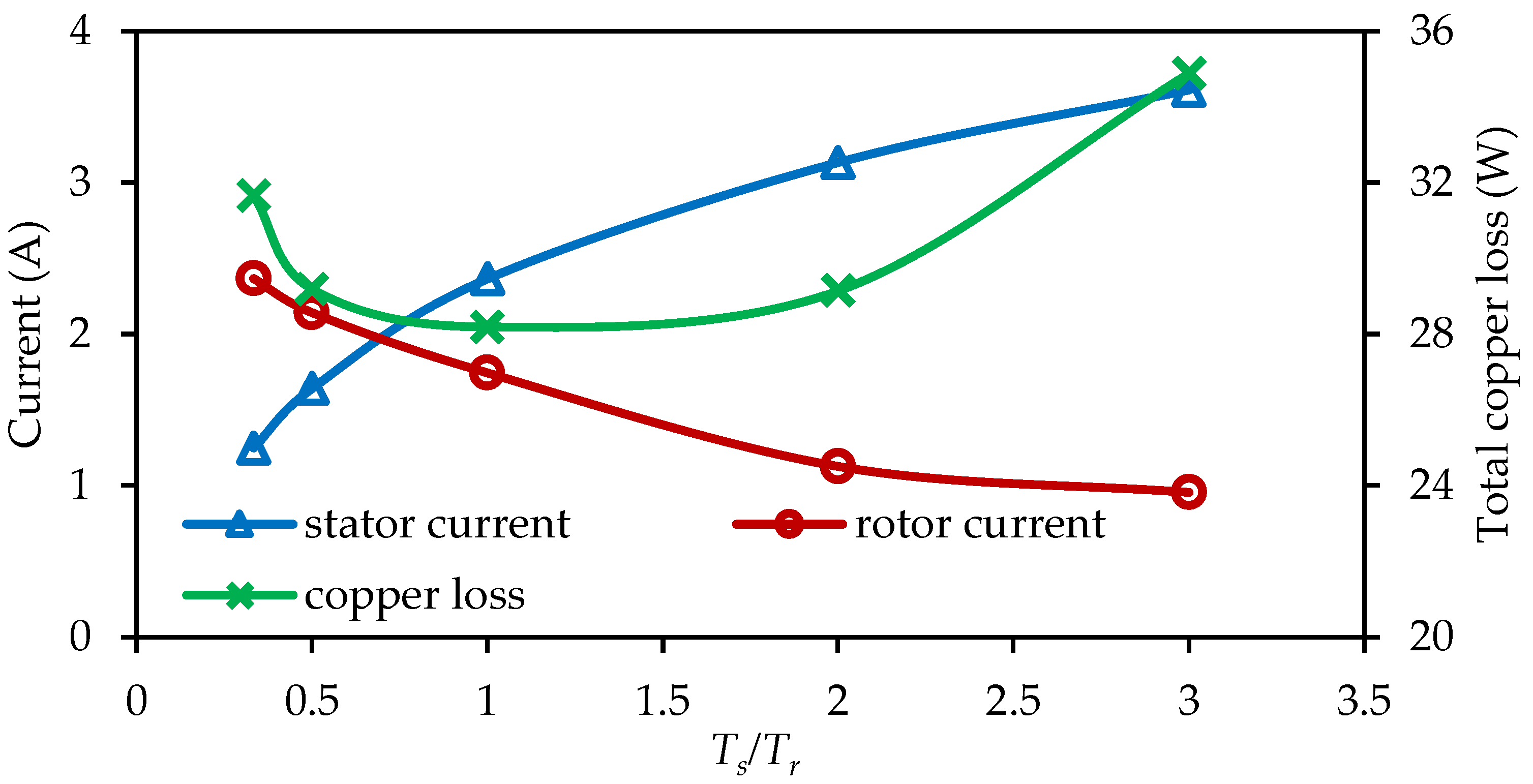
3.2. Influence of Stator/Rotor Torque Ratio on Maximum Average Torque
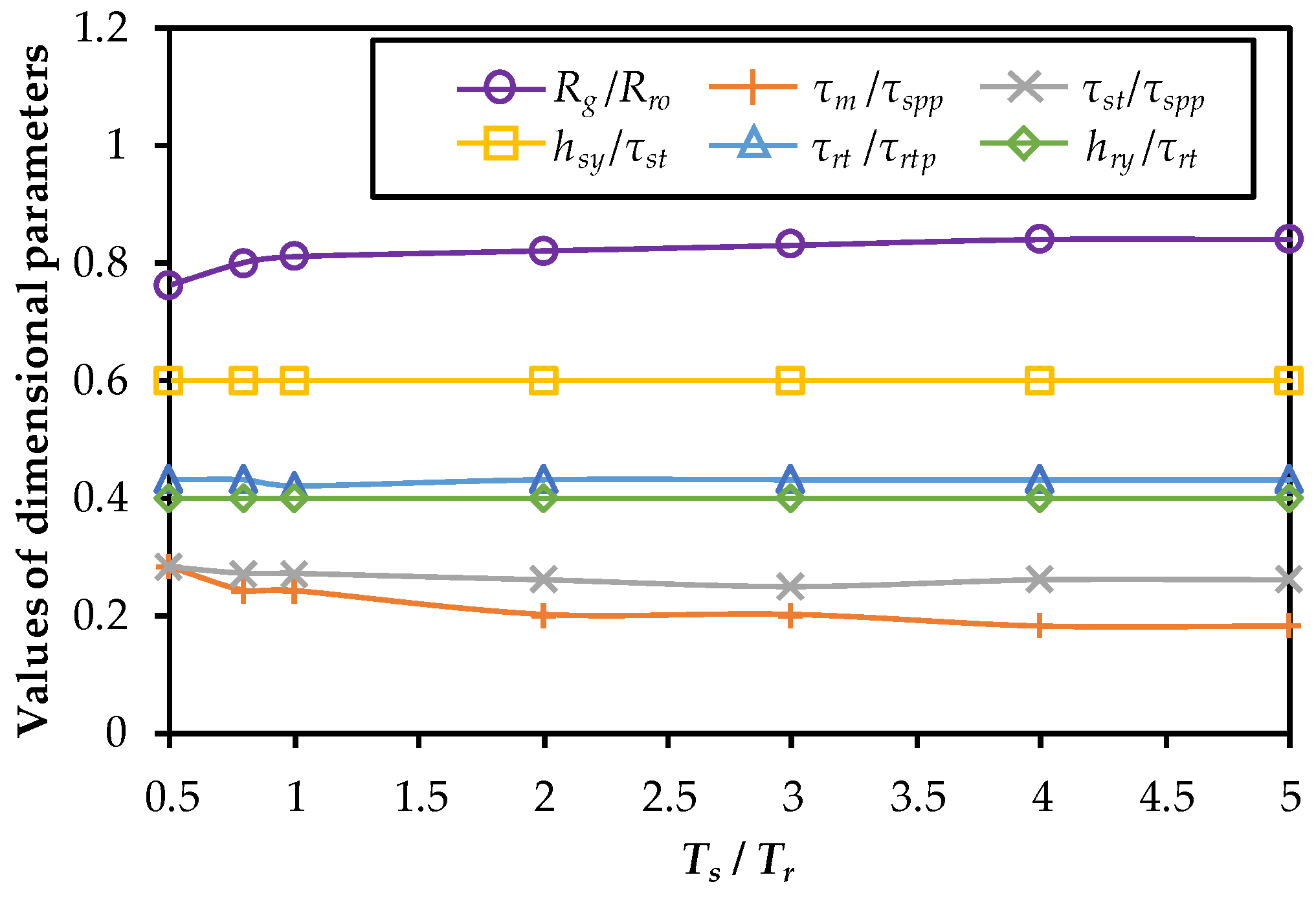
3.3. Influence of Stator/Rotor Torque Ratio on Parameter Design
4. Advantage of ER-DA-FSPM Machine
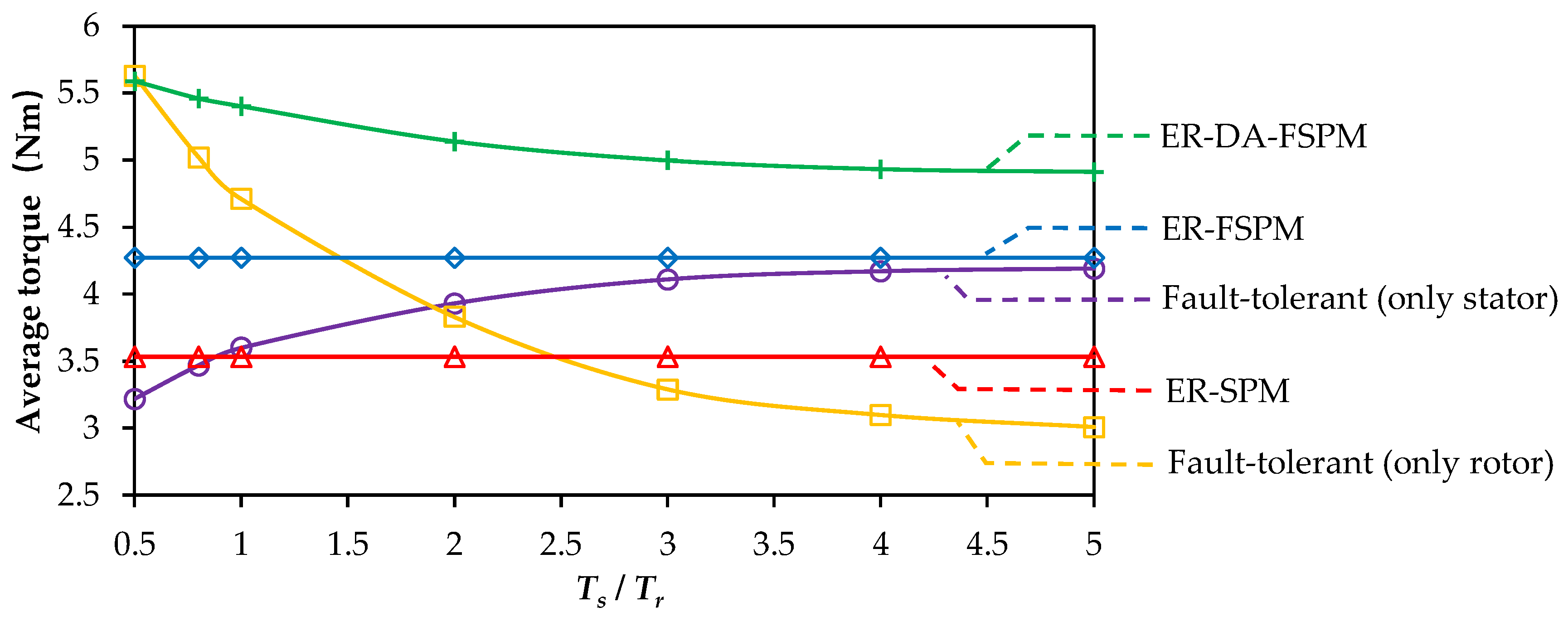
4.1. Comparison of Average Torque for Different Machines
4.2. Comparison of Fault-Tolerant Capability for Different Machines
5. Experimental Validation
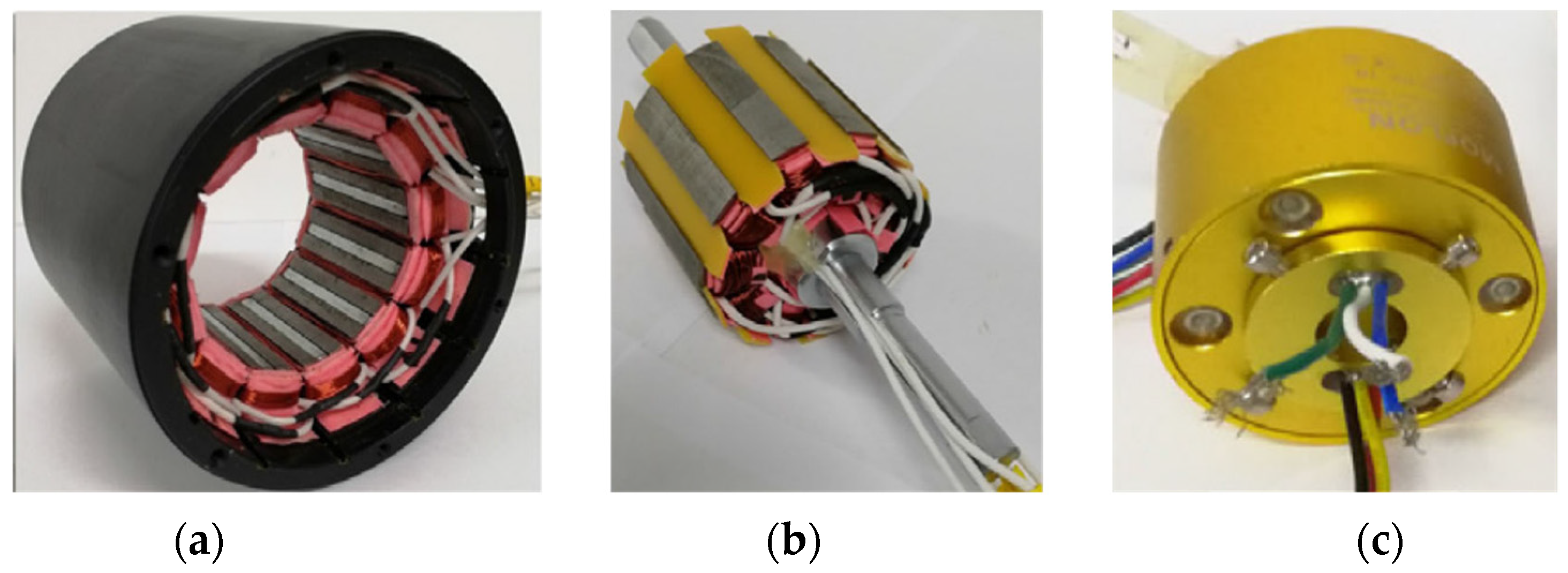
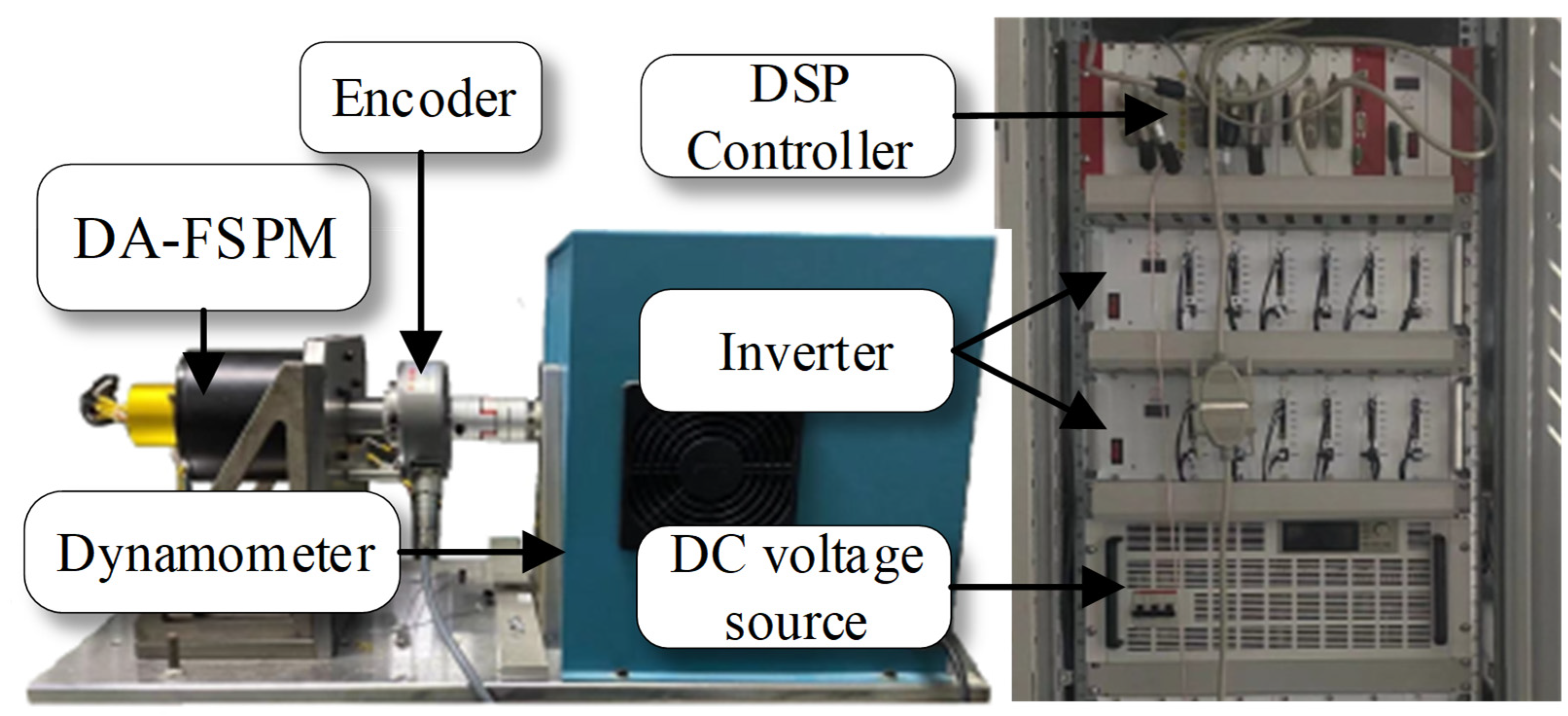
5.1. Prototype Machine and Experimental Setup
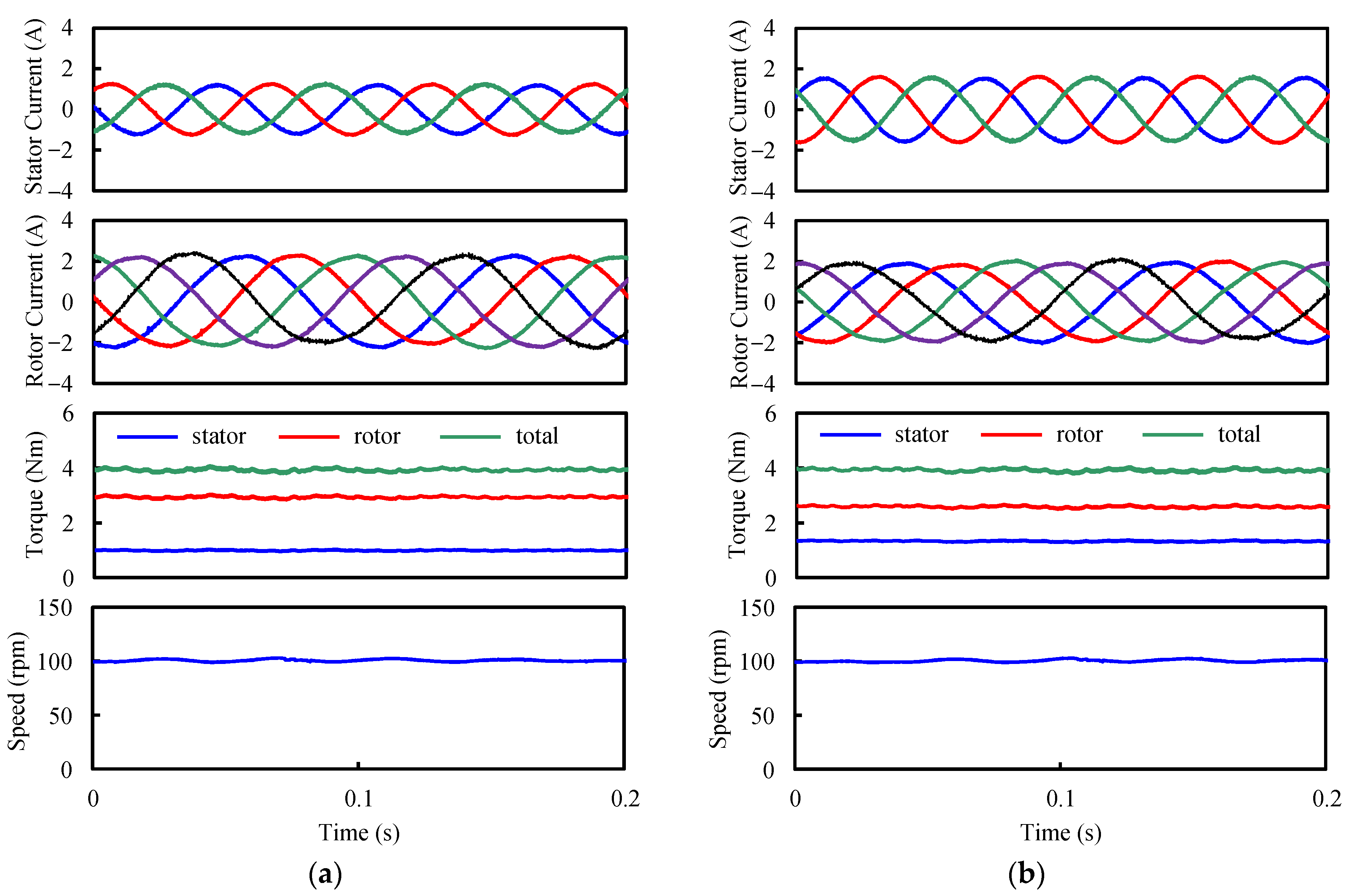
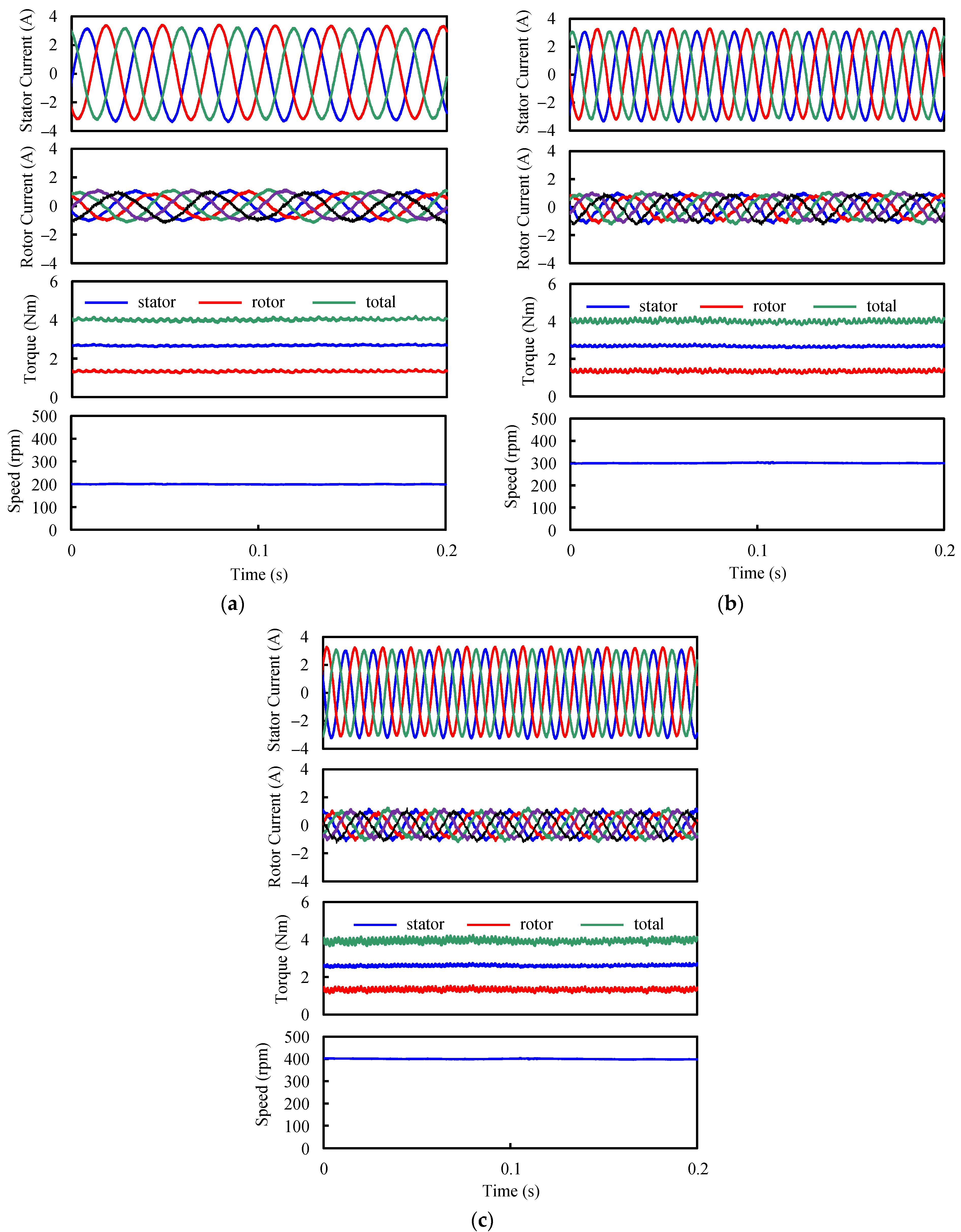
5.2. Torque Experiments and Discussion
5.3. Speed Experiments and Discussion
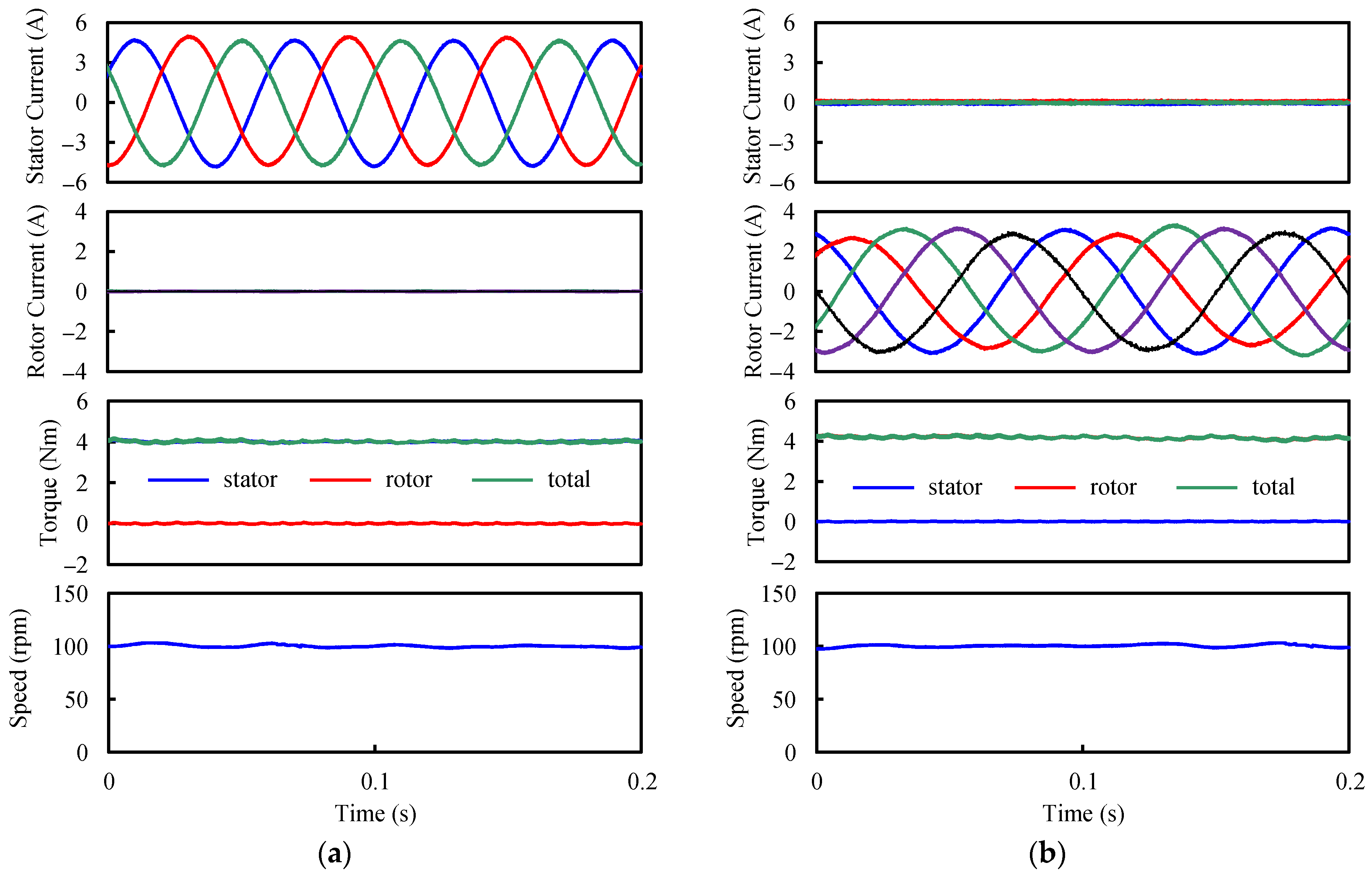
5.4. Fault-Tolerant Experiments and Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhao, X.; Doering, O.C.; Tyner, W.E. The Economic Competitiveness and Emissions of Battery Electric Vehicles in China. Appl. Energy 2015, 156, 666–675. [Google Scholar] [CrossRef]
- Rodrigues Teixeira, A.C.; Sodre, J.R. Impacts of Replacement of Engine Powered Vehicles by Electric Vehicles on Energy Consumption and CO2 Emissions. Transp. Res. Part D Transp. Environ. 2018, 59, 375–384. [Google Scholar] [CrossRef]
- Fu, S.; Fu, H. A Method to Predict Electric Vehicles’ Market Penetration as Well as Its Impact on Energy Saving and CO2 Mitigation. Sci. Prog. 2021, 104, 00368504211040286. [Google Scholar] [CrossRef] [PubMed]
- de Moraes, D.R.; Soares, L.O.; Guimaraes, V.d.A.; de Oliveira, K.F.; Hernandez-Callejo, L.; Ribeiro Vieira, G.M.; Mancebo Boloy, R.A. Energy-Ecological Efficiency of the Fuel Cell Electric Vehicle Powered by Different Biofuels. Clean Technol. Environ. Policy 2022, 24, 1389–1402. [Google Scholar] [CrossRef]
- Dadashnialehi, A.; Bab-Hadiashar, A.; Cao, Z.; Kapoor, A. Intelligent Sensorless ABS for In-Wheel Electric Vehicles. IEEE Trans. Ind. Electron. 2014, 61, 1957–1969. [Google Scholar] [CrossRef]
- Fan, Y.; Zhang, L.; Huang, J.; Han, X. Design, Analysis, and Sensorless Control of a Self-Decelerating Permanent-Magnet In-Wheel Motor. IEEE Trans. Ind. Electron. 2014, 61, 5788–5797. [Google Scholar] [CrossRef]
- Li, C.; Guo, X.; Fu, J.; Fu, W.; Liu, Y.; Chen, H.; Wang, R.; Li, Z. Design and Analysis of a Novel Double-Stator Double-Rotor Motor Drive System for In-Wheel Direct Drive of Electric Vehicles. Machines 2022, 10, 27. [Google Scholar] [CrossRef]
- Huang, J.; Liu, Y.; Liu, M.; Cao, M.; Yan, Q. Multi-Objective Optimization Control of Distributed Electric Drive Vehicles Based on Optimal Torque Distribution. IEEE Access 2019, 7, 16377–16394. [Google Scholar] [CrossRef]
- Wei, H.; Zhang, N.; Liang, J.; Ai, Q.; Zhao, W.; Huang, T.; Zhang, Y. Deep Reinforcement Learning Based Direct Torque Control Strategy for Distributed Drive Electric Vehicles Considering Active Safety and Energy Saving Performance. Energy 2022, 238, 121725. [Google Scholar] [CrossRef]
- Li, Y.; Huang, X.; Yu, D.; Zhang, K.; Zhang, J. Design and Analysis of a Outer-Rotor Permanent-Magnet Flux-Modulated Motor for Electric Vehicles. In Proceedings of the 2016 IEEE Conference on Electromagnetic Field Computation (CEFC), Miami, FL, USA, 13–16 November 2016; p. 1. [Google Scholar]
- Zhu, X.; Shu, Z.; Quan, L.; Xiang, Z.; Pan, X. Design and Multicondition Comparison of Two Outer-Rotor Flux-Switching Permanent-Magnet Motors for In-Wheel Traction Applications. IEEE Trans. Ind. Electron. 2017, 64, 6137–6148. [Google Scholar] [CrossRef]
- Bonthu, S.S.R.; Tarek, M.T.B.; Choi, S. Optimal Torque Ripple Reduction Technique for Outer Rotor Permanent Magnet Synchronous Reluctance Motors. IEEE Trans. Energy Convers. 2018, 33, 1184–1192. [Google Scholar] [CrossRef]
- Rauch, S.E.; Johnson, L.J. Design Principles of Flux-Switch Alternators [Includes Discussion]. Trans. Am. Inst. Electr. Eng. Part III Power Appar. Syst. 1955, 74, 1261–1268. [Google Scholar] [CrossRef]
- Chen, H.; EL-Refaie, A.M.; Demerdash, N.A.O. Flux-Switching Permanent Magnet Machines: A Review of Opportunities and Challenges-Part I: Fundamentals and Topologies. IEEE Trans. Energy Convers. 2020, 35, 684–698. [Google Scholar] [CrossRef]
- Chen, H.; EL-Refaie, A.M.; Demerdash, N.A.O. Flux-Switching Permanent Magnet Machines: A Review of Opportunities and Challenges-Part II: Design Aspects, Control, and Emerging Trends. IEEE Trans. Energy Convers. 2020, 35, 699–713. [Google Scholar] [CrossRef]
- Fei, W.; Luk, P.C.K.; Shen, J.X.; Wang, Y.; Jin, M. A Novel Permanent-Magnet Flux Switching Machine with an Outer-Rotor Configuration for In-Wheel Light Traction Applications. IEEE Trans. Ind. Appl. 2012, 48, 1496–1506. [Google Scholar] [CrossRef]
- Fei, W.; Luk, P.C.K.; Miao, D.-M.; Shen, J.-X. Investigation of Torque Characteristics in a Novel Permanent Magnet Flux Switching Machine with an Outer-Rotor Configuration. IEEE Trans. Magn. 2014, 50, 1–10. [Google Scholar] [CrossRef]
- Chen, J.T.; Zhu, Z.Q.; Iwasaki, S.; Deodhar, R.P. Influence of Slot Opening on Optimal Stator and Rotor Pole Combination and Electromagnetic Performance of Switched-Flux PM Brushless AC Machines. IEEE Trans. Ind. Appl. 2011, 47, 1681–1691. [Google Scholar] [CrossRef]
- Chen, J.T.; Zhu, Z.Q.; Iwasaki, S.; Deodhar, R.P. A Novel Hybrid-Excited Switched-Flux Brushless AC Machine for EV/HEV Applications. IEEE Trans. Veh. Technol. 2011, 60, 1365–1373. [Google Scholar] [CrossRef]
- Zhu, Z.Q.; Chen, J.I.; Pang, Y.; Howe, D.; Iwasaki, S.; Deodhar, R. Analysis of a Novel Multi-Tooth Flux-Switching PM Brushless AC Machine for High Torque Direct-Drive Applications. IEEE Trans. Magn. 2008, 44, 4313–4316. [Google Scholar] [CrossRef]
- Zhou, Y.J.; Zhu, Z.Q. Torque Density and Magnet Usage Efficiency Enhancement of Sandwiched Switched Flux Permanent Magnet Machines Using V-Shaped Magnets. IEEE Trans. Magn. 2013, 49, 3834–3837. [Google Scholar] [CrossRef]
- Hua, W.; Zhang, H.; Cheng, M.; Meng, J.; Hou, C. An Outer-Rotor Flux-Switching Permanent-Magnet-Machine with Wedge-Shaped Magnets for In-Wheel Light Traction. IEEE Trans. Ind. Electron. 2017, 64, 69–80. [Google Scholar] [CrossRef]
- Jin, M.-J.; Wang, C.-F.; Shen, J.-X.; Xia, B. A Modular Permanent-Magnet Flux-Switching Linear Machine with Fault-Tolerant Capability. IEEE Trans. Magn. 2009, 45, 3179–3186. [Google Scholar] [CrossRef]
- Taras, P.; Li, G.-J.; Zhu, Z.Q. Comparative Study of Fault-Tolerant Switched-Flux Permanent-Magnet Machines. IEEE Trans. Ind. Electron. 2017, 64, 1939–1948. [Google Scholar] [CrossRef]
- Shao, L.; Hua, W.; Zhu, Z.Q.; Tong, M.; Zhao, G.; Yin, F.; Wu, Z.; Cheng, M. Investigation of Torque Characteristics in a Novel Permanent Magnet Flux Switching Machine with an Outer-Rotor Con-Figuration. IEEE Trans. Ind. Appl. 2017, 53, 3305–3316. [Google Scholar] [CrossRef]
- Zong, Z.; Quan, L.; Xiang, Z. Comparison of Double-Stator Flux-Switching Permanent Magnet Machine and Double-Stator Permanent Magnet Synchronous Machine for Electric Vehicle Applications. In Proceedings of the 2014 17th International Conference on Electrical Machines and Systems (ICEMS), Hangzhou, China, 22–25 October 2014; pp. 234–239. [Google Scholar]
- Kim, D.; Hwang, H.; Bae, S.; Lee, C. Analysis and Design of a Double-Stator Flux-Switching Permanent Magnet Machine Using Ferrite Magnet in Hybrid Electric Vehicles. IEEE Trans. Magn. 2016, 52, 8106604. [Google Scholar] [CrossRef]
- Kana Padinharu, D.K.; Li, G.J.; Zhu, Z.Q.; Azar, Z.; Clark, R.; Thomas, A. Effect of Airgap Length on Electromagnetic Performance of Surface Mounted Permanent Magnet Vemier Machine. In Proceedings of the 2020 International Conference on Electrical Machines (ICEM), Gothenburg, Sweden, 23 August 2020; pp. 1882–1888. [Google Scholar]
- Wu, L.; Zhu, J.; Fang, Y. A Novel Doubly-Fed Flux-Switching Permanent Magnet Machine with Armature Windings Wound on Both Stator Poles and Rotor Teeth. IEEE Trans. Ind. Electron. 2020, 67, 10223–10232. [Google Scholar] [CrossRef]
- Zhu, J.; Wu, L.; Wen, H. Optimization and Comparison of Dual-Armature Flux-Switching Permanent Magnet Machines with Different Stator Core Shapes. IEEE Trans. Ind. Appl. 2022, 58, 314–324. [Google Scholar] [CrossRef]
- Zhang, G.; Hua, W.; Cheng, M.; Liao, J.; Wang, K.; Zhang, J. Investigation of an Improved Hybrid-Excitation Flux-Switching Brushless Machine for HEV/EV Applications. IEEE Trans. Ind. Appl. 2015, 51, 3791–3799. [Google Scholar] [CrossRef]
- Lin, T.C.; Zhu, Z.Q.; Liu, K.; Liu, J.M. Improved Sensorless Control of Switched-Flux Permanent-Magnet Synchronous Machines Based on Different Winding Configurations. IEEE Trans. Ind. Electron. 2016, 63, 123–132. [Google Scholar] [CrossRef]
- Shu, Z.; Zhu, X.; Quan, L.; Du, Y.; Liu, C. Electromagnetic Performance Evaluation of an Outer-Rotor Flux-Switching Permanent Magnet Motor Based on Electrical-Thermal Two-Way Coupling Method. Energies 2017, 10, 677. [Google Scholar] [CrossRef]
- Tovar-Barranco, A.; Lopez-de-Heredia, A.; Villar, I.; Briz, F. Modeling of End-Space Convection Heat-Transfer for Internal and External Rotor PMSMs with Fractional-Slot Concentrated Windings. IEEE Trans. Ind. Electron. 2021, 68, 1928–1937. [Google Scholar] [CrossRef]
- Feng, T.; Shu, L. Game-Based Multiobjective Optimization of Suspension System for In-Wheel Motor Drive Electric Vehicle. Math. Probl. Eng. 2021, 2021, 5589199. [Google Scholar] [CrossRef]
- Awah, C.C.; Zhu, Z.Q.; Wu, Z.Z.; Shi, J.T.; Wu, D. Comparison of Partitioned Stator Switched Flux Permanent Magnet Machines Having Single- and Double-Layer Windings. In Proceedings of the 2015 Tenth International Conference on Ecological Vehicles and Renewable Energies (EVER), Monte Carlo, Monaco, 31 March–2 April 2015; pp. 1–5. [Google Scholar]
- Li, G.; Ojeda, J.; Hoang, E.; Gabsi, M. Double and Single Layers Flux-Switching Permanent Magnet Motors: Fault Tolerant Model for Critical Applications. In Proceedings of the 2011 International Conference on Electrical Machines and Systems, Beijing, China, 20–23 August 2011; pp. 1–6. [Google Scholar]
- Chen, J.T.; Zhu, Z.Q. Comparison of All- and Alternate-Poles-Wound Flux-Switching PM Machines Having Different Stator and Rotor Pole Numbers. IEEE Trans. Ind. Appl. 2010, 46, 1406–1415. [Google Scholar] [CrossRef]
- Owen, R.L.; Zhu, Z.Q.; Thomas, A.S.; Jewell, G.W.; Howe, D. Alternate Poles Wound Flux-Switching Permanent-Magnet Brushless AC Machines. IEEE Trans. Ind. Appl. 2010, 46, 790–797. [Google Scholar] [CrossRef]
- Zheng, Y.; Wu, L.; Li, H.; Wen, H.; Qiu, L. Harmonic Analysis of Airgap Magnetic Fields in Doubly-Fed Flux Reversal Permanent Magnet Machines. IEEE Access 2020, 8, 134856–134867. [Google Scholar] [CrossRef]
- Zhang, L.; Wu, L.; Wen, H.; Niu, F.; Lu, Q. Improved Primary/Secondary Pole Number Combinations for Dual-Armature Linear Switched Flux Permanent Magnet Machines. IEEE Trans. Transp. Electrif. 2021, 7, 2589–2599. [Google Scholar] [CrossRef]
- Wu, Z.Z.; Zhu, Z.Q. Analysis of Air-Gap Field Modulation and Magnetic Gearing Effects in Switched Flux Permanent Magnet Machines. IEEE Trans. Magn. 2015, 51, 8105012. [Google Scholar] [CrossRef]
- Zhu, Z.Q.; Liu, Y. Analysis of Air-Gap Field Modulation and Magnetic Gearing Effect in Fractional-Slot Concentrated-Winding Permanent-Magnet Synchronous Machines. IEEE Trans. Ind. Electron. 2018, 65, 3688–3698. [Google Scholar] [CrossRef]
- Huang, L.; Feng, J.; Guo, S.; Shi, J.; Zhu, Z.Q. Analysis of Power Factor in Variable Flux Reluctance Machines with MMF-Permeance Model. IET Electr. Power Appl. 2019, 13, 614–624. [Google Scholar] [CrossRef]
- Zhu, X.; Hua, W.; Wu, Z.; Huang, W.; Zhang, H.; Cheng, M. Analytical Approach for Cogging Torque Reduction in Flux-Switching Permanent Magnet Machines Based on Magnetomotive Force-Permeance Model. IEEE Trans. Ind. Electron. 2018, 65, 1965–1979. [Google Scholar] [CrossRef]
- Chen, H.; Li, D.; Qu, R.; Zou, T. Torque Capacity Improvement of Flux-Switching PM Machines Based on Directional Stator Permeance Design. IEEE Trans. Ind. Electron. 2024, 71, 4551–4561. [Google Scholar] [CrossRef]
- Pang, Y.; Zhu, Z.Q.; Howe, D. Analytical Determination of Optimal Split Ratio for Permanent Magnet Brushless Motors. IEE Proc.-Electr. POWER Appl. 2006, 153, 7–13. [Google Scholar] [CrossRef]






| Parameter | Value |
|---|---|
| Rotor external radius Rro | 45 mm |
| Rotor inner radius Rri | 35.4 mm |
| Stator external radius Rso | 34.9 mm |
| Stator inner radius Rsi | 17 mm |
| Axis length | 45 mm |
| Magnet arc τm | 6.1° |
| Side stator tooth arc τst | 5.6° |
| Rotor tooth arc τrt | 14.7° |
| Stator yoke thickness hsy | 1.9 mm |
| Rotor yoke thickness hry | 3.8 mm |
| Air gap length | 0.5 mm |
| Stator pole/rotor tooth number | 12/10 |
| Stator/rotor phase number | 3/5 |
| Rated copper loss | 24 W |
| Number of stator winding turns per phase | 72 |
| Number of rotor winding turns per phase | 68 |
| Slot fill factor | 0.4 |
| Magnet remanence | 1.2 T |
| Magnet relative permeability | 1.05 |
| Lamination material | M19_24G |
| Rated speed | 400 rpm |
| No. | Pole-Pairs | Rotating Speeding, Ωr | |
|---|---|---|---|
| m = 5l − 3 | 1 | 2m = 10l − 6 = ltr − ps | (2m − ps)/2m |
| 2 | 2|nps − m| = |2nps − 10l + 6| = |(2n + 1)ps − ltr| | (2m − ps)/(2m − 2nps) | |
| 3 | 2(nps + m) = 2nps + 10l − 6 = [2(n − 1) + 1]ps + ltr | (2m − ps)/(2m + 2nps) | |
| m = 5l − 2 | 1 | 2m = 10l − 4=(l − 1)tr + ps | (2m + ps)/2m |
| 2 | 2(nps + m) = 2nps + 10l − 4=(2n + 1)ps + (l − 1)tr | (2m + ps)/(2m + 2nps) | |
| 3 | 2|nps − m| = |2nps − 10l + 4| = |[2(n − 1) + 1]ps − (l − 1)tr| | (2m + ps)/(2m − 2nps) |
| No. | Pole-Pairs | Rotating Speeding, Ωr |
|---|---|---|
| 1 | 2(i − 1)ps | 0(static) |
| 2 | ktr + 2(i − 1)ps | ktr/[ktr + 2(i − 1)ps] |
| 3 | |ktr − 2(i − 1)ps| | ktr/[ktr − 2(i − 1)ps] |
| No. | Pole-Pairs | Rotating Speeding, Ωr | |
|---|---|---|---|
| q = 3r − 2 | 1 | 4q = 12r − 8=tr + [2(r − 1) − 1]ps | tr/4q |
| 2 | |ktr −4q| = |ktr − 12r + 8| = |(k − 1)tr − [2(r − 1) − 1]ps| | (k − 1)tr/(ktr − 4q) | |
| 3 | ktr + 4q = ktr + 12r – 8 = (k + 1)tr + [2(r − 1) − 1]ps | (k + 1)tr/(ktr + 4q) | |
| q = 3r − 1 | 1 | 4q = 12r − 4= − [tr − [2(r + 1) − 1]ps] | −tr/4q |
| 2 | ktr + 4q = ktr + 12r – 4 = (k − 1)tr + [2(r + 1) − 1]ps | (k − 1)tr/(ktr + 4q) | |
| 3 | |ktr − 4q| = |ktr − 12r + 4| = |(k + 1)tr − [2(r + 1) − 1]ps| | (k + 1)tr/(ktr − 4q) |
| Order | Parameter | Value |
|---|---|---|
| 1 | Split ratio, Rg/Rro | 0.78 |
| 2 | Magnet-arc-to-stator-pole-pitch ratio, τm/τspp | 0.2 |
| 3 | Side stator tooth arc-to-pole pitch ratio, τst/τspp | 0.17 |
| 4 | Stator-yoke-thickness-to-tooth-arc ratio, hsy/τst | 0.6 |
| 5 | Rotor-tooth-arc-to-tooth-pitch ratio, τrt/τrtp | 0.4 |
| 6 | Rotor-yoke-thickness-to-tooth-arc ratio, hry/τrt | 0.4 |
| Parameter | ER-DA-FSPM | ER-FSPM | ER-SPM |
|---|---|---|---|
| Rotor external radius | 45 mm | ||
| Stator inner radius | 17 mm | 17 mm | 15 mm |
| Axis length | 45 mm | ||
| Air gap length | 0.5 mm | ||
| Pole/tooth number | 12/10 | 12/10 | 10/12 |
| Stator/rotor phase number | 3/5 | 3/0 | 3/0 |
| Rated copper loss | 24 W | ||
| Number of stator winding turns per phase | 72 | ||
| Number of rotor winding turns per phase | 68 | ||
| Slot fill factor | 0.4 | ||
| Magnet remanence | 1.2 T | ||
| Magnet relative permeability | 1.05 | ||
| Lamination material | M19_24G | ||
| Rated speed | 400 rpm | ||
| Parameter | Value |
|---|---|
| Stator outer radius | 50 mm |
| Stator inner radius | 35.25 mm |
| Active axial length | 50 mm |
| Stator tooth arc to pole pitch ratio | 0.28 |
| Magnet arc to stator pole pitch ratio | 0.24 |
| Stator yoke thickness to tooth arc ratio | 0.6 |
| Airgap length | 0.5 mm |
| Rotor inner radius | 10 mm |
| Rotor tooth arc to tooth pitch ratio | 0.37 |
| Rotor tooth tip arc to tooth pitch ratio | 0.055 |
| Rotor yoke thickness to tooth body arc ratio | 0.7 |
| Rotor tooth tip height | 0.8 mm |
| Number of stator winding turns per phase | 160 |
| Number of rotor winding turns per phase | 144 |
| Stator phase resistance | 1.55 Ω |
| Rotor phase resistance | 2 Ω |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zuo, Z.; Du, Y.; Yu, L. Influence of Stator/Rotor Torque Ratio on Torque Performance in External-Rotor Dual-Armature Flux-Switching PM Machines. Machines 2024, 12, 588. https://doi.org/10.3390/machines12090588
Zuo Z, Du Y, Yu L. Influence of Stator/Rotor Torque Ratio on Torque Performance in External-Rotor Dual-Armature Flux-Switching PM Machines. Machines. 2024; 12(9):588. https://doi.org/10.3390/machines12090588
Chicago/Turabian StyleZuo, Zijie, Yidong Du, and Lei Yu. 2024. "Influence of Stator/Rotor Torque Ratio on Torque Performance in External-Rotor Dual-Armature Flux-Switching PM Machines" Machines 12, no. 9: 588. https://doi.org/10.3390/machines12090588
APA StyleZuo, Z., Du, Y., & Yu, L. (2024). Influence of Stator/Rotor Torque Ratio on Torque Performance in External-Rotor Dual-Armature Flux-Switching PM Machines. Machines, 12(9), 588. https://doi.org/10.3390/machines12090588





