Compensation Method for Correcting the Topography Convolution of the 3D AFM Profile Image of a Diffraction Grating
Abstract
1. Introduction
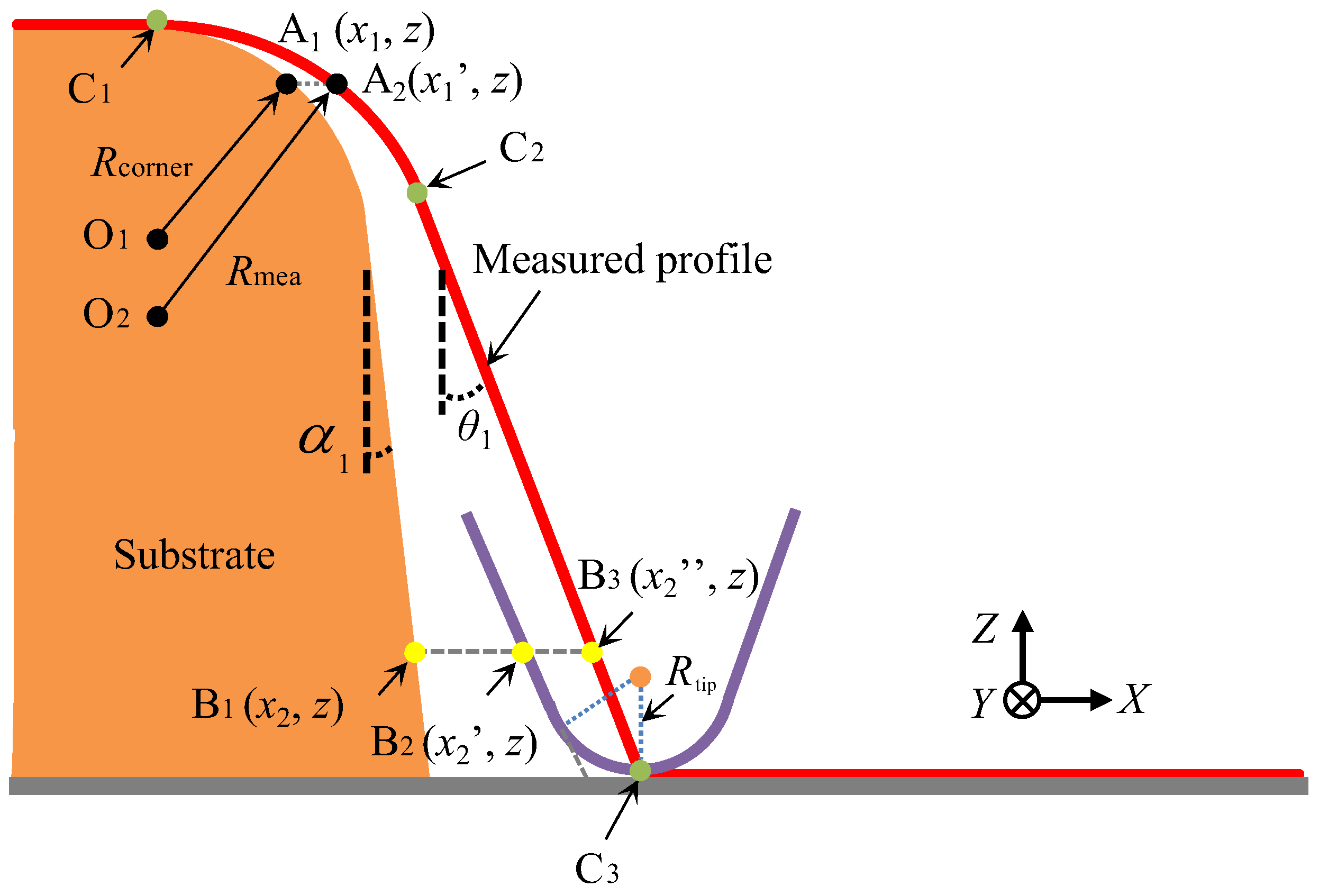
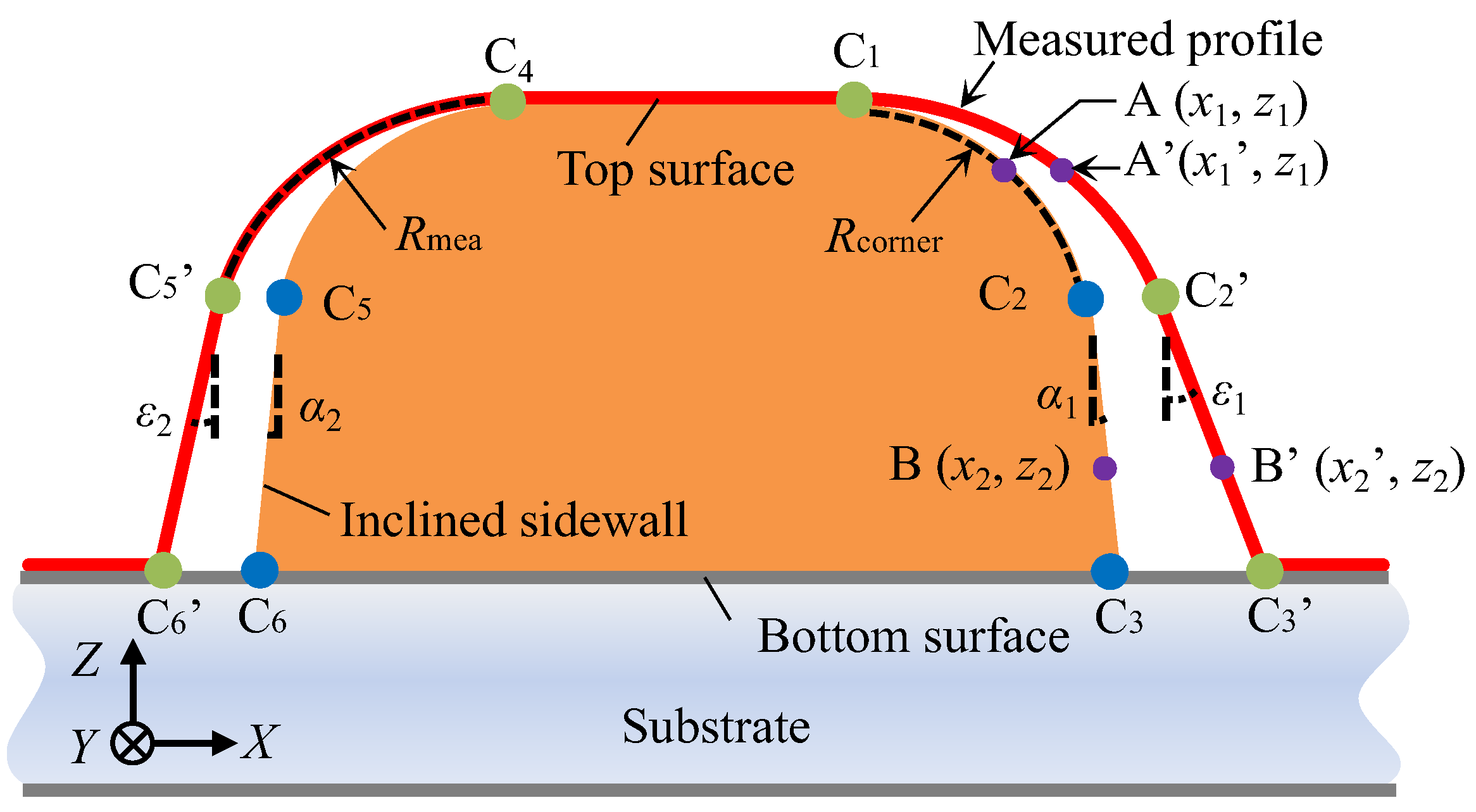
2. Measurement Principle
2.1. Principle for AFM Measurement of a Diffraction Grating with a Rectangular Profile
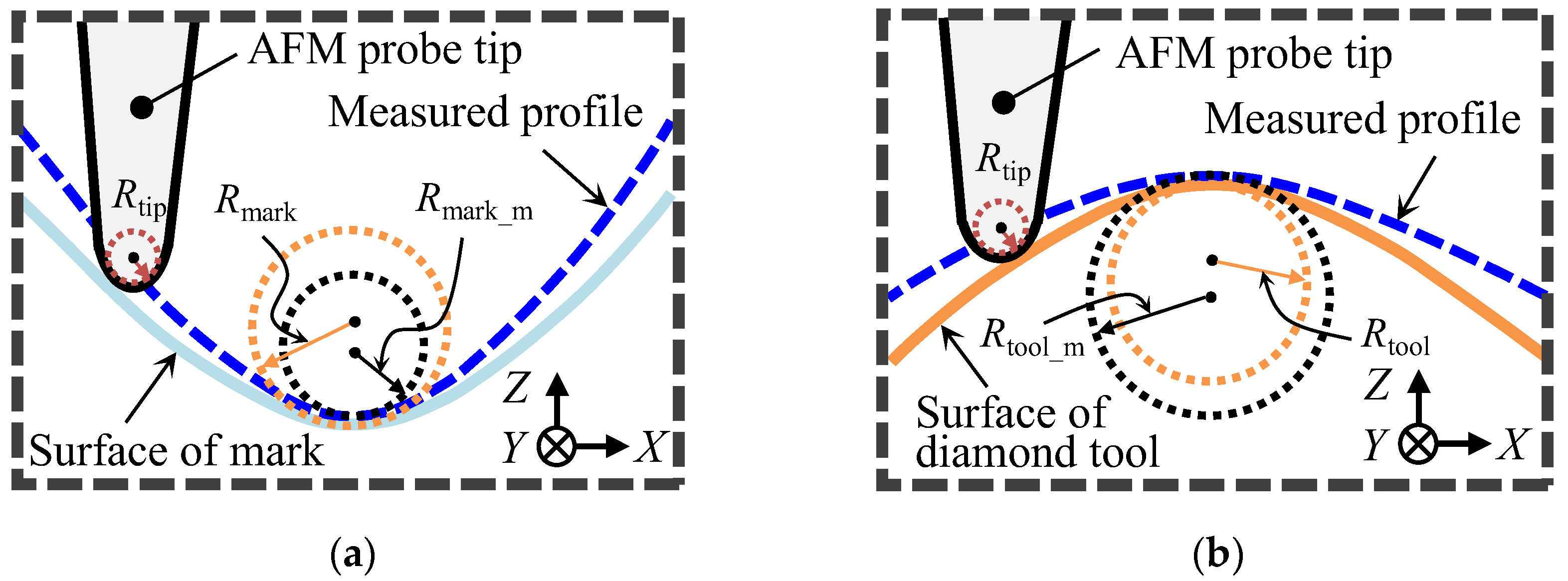
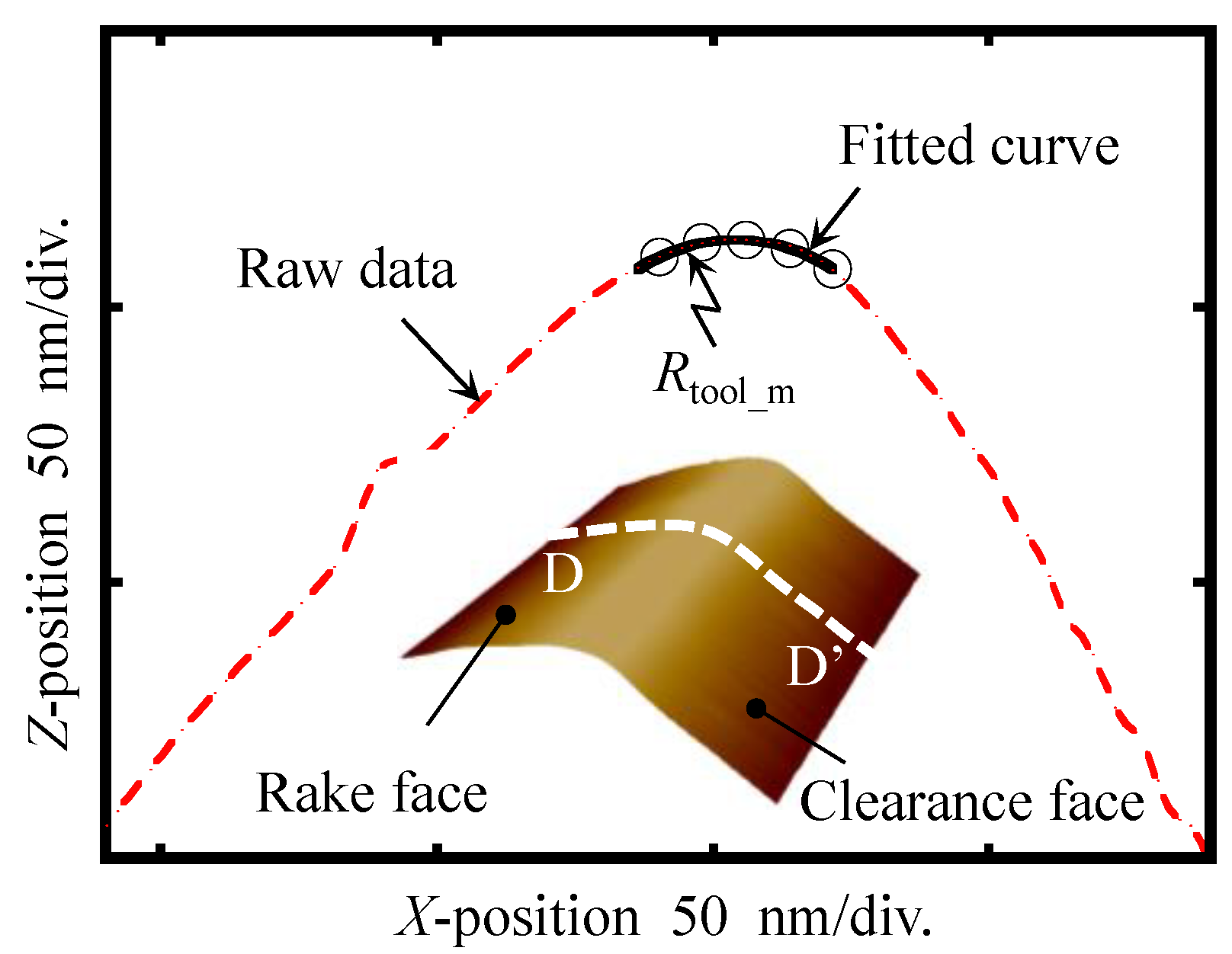
2.2. Method for Evaluating the Geometry of an AFM Probe Tip
3. Experimental Results and Discussion
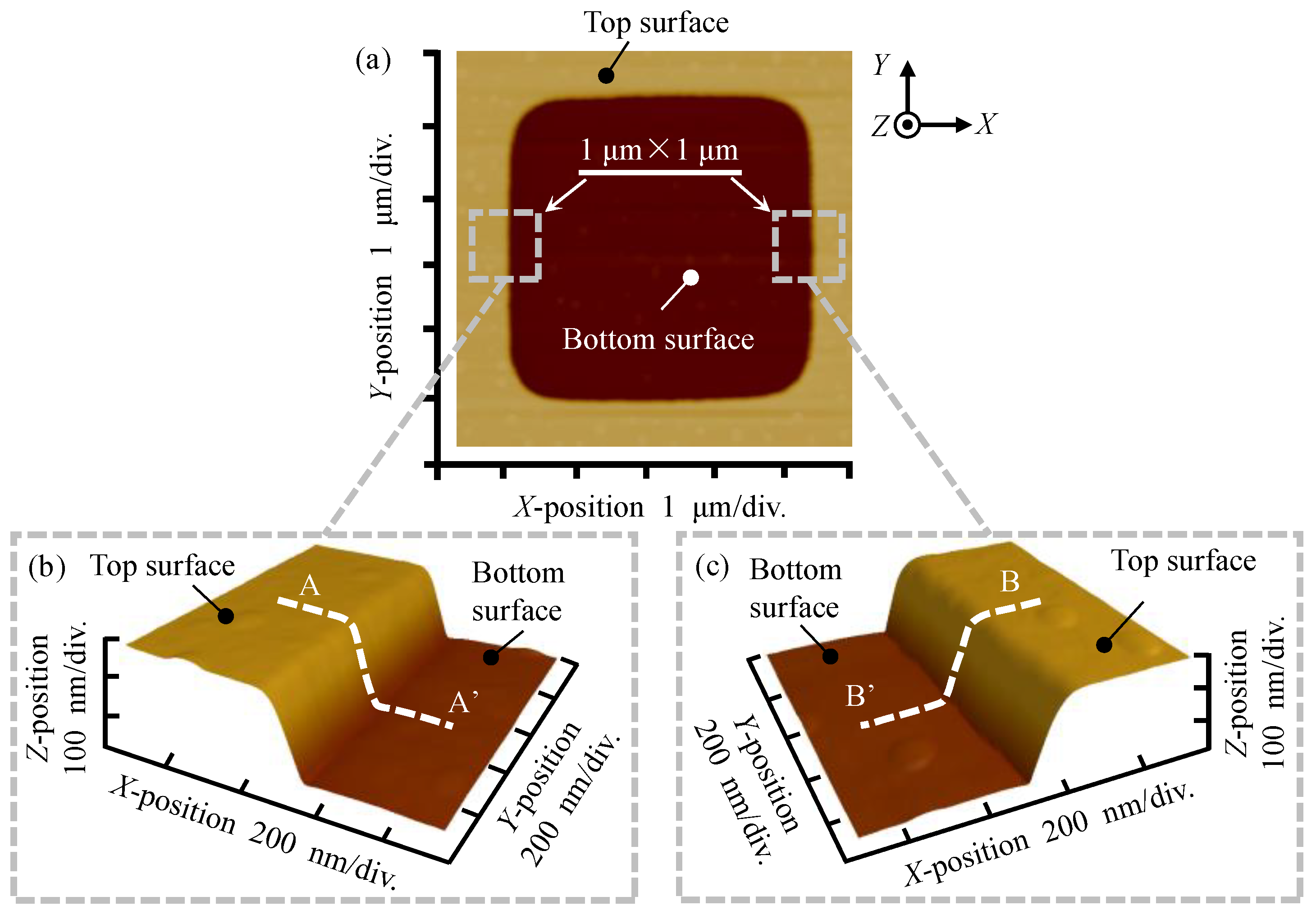
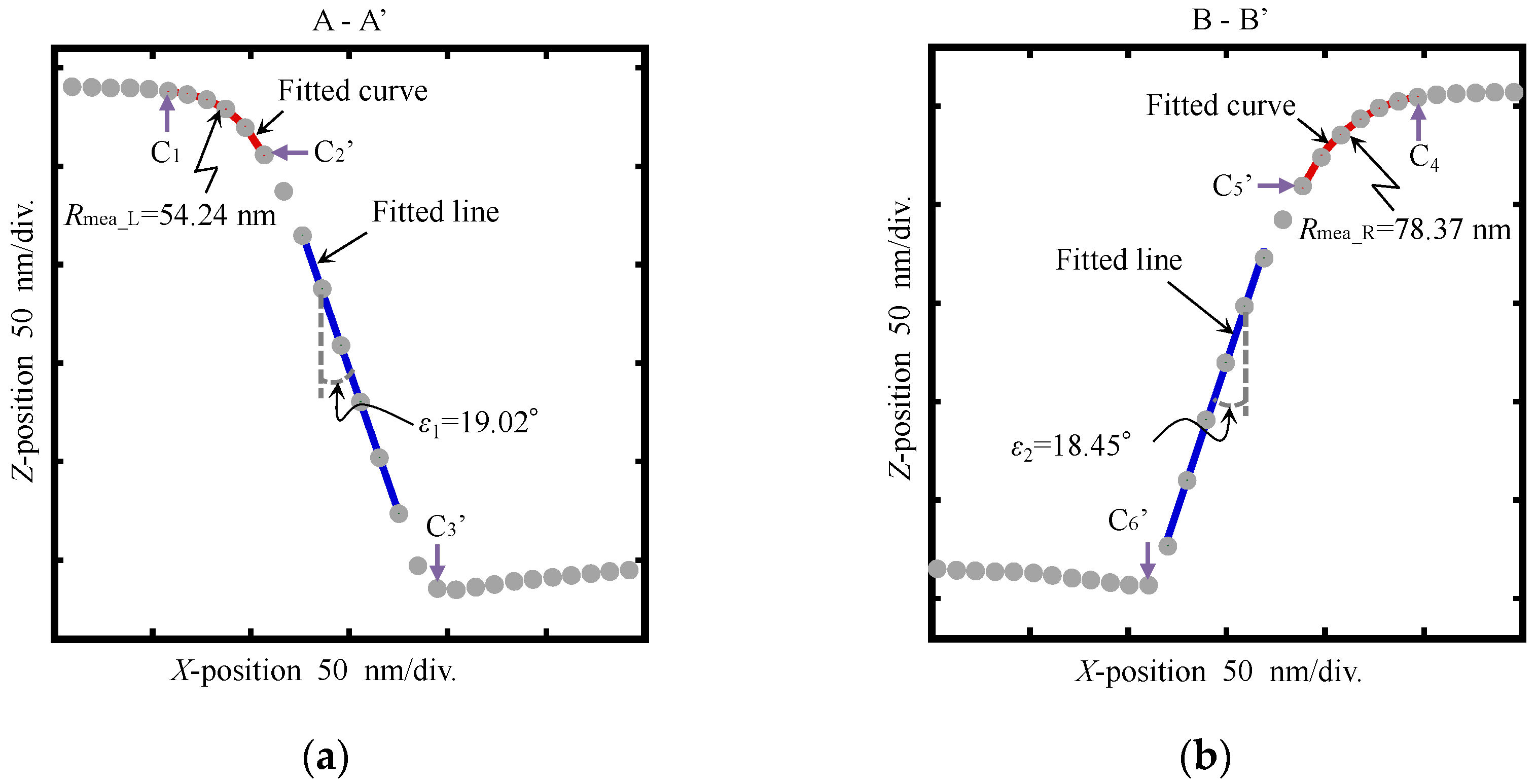
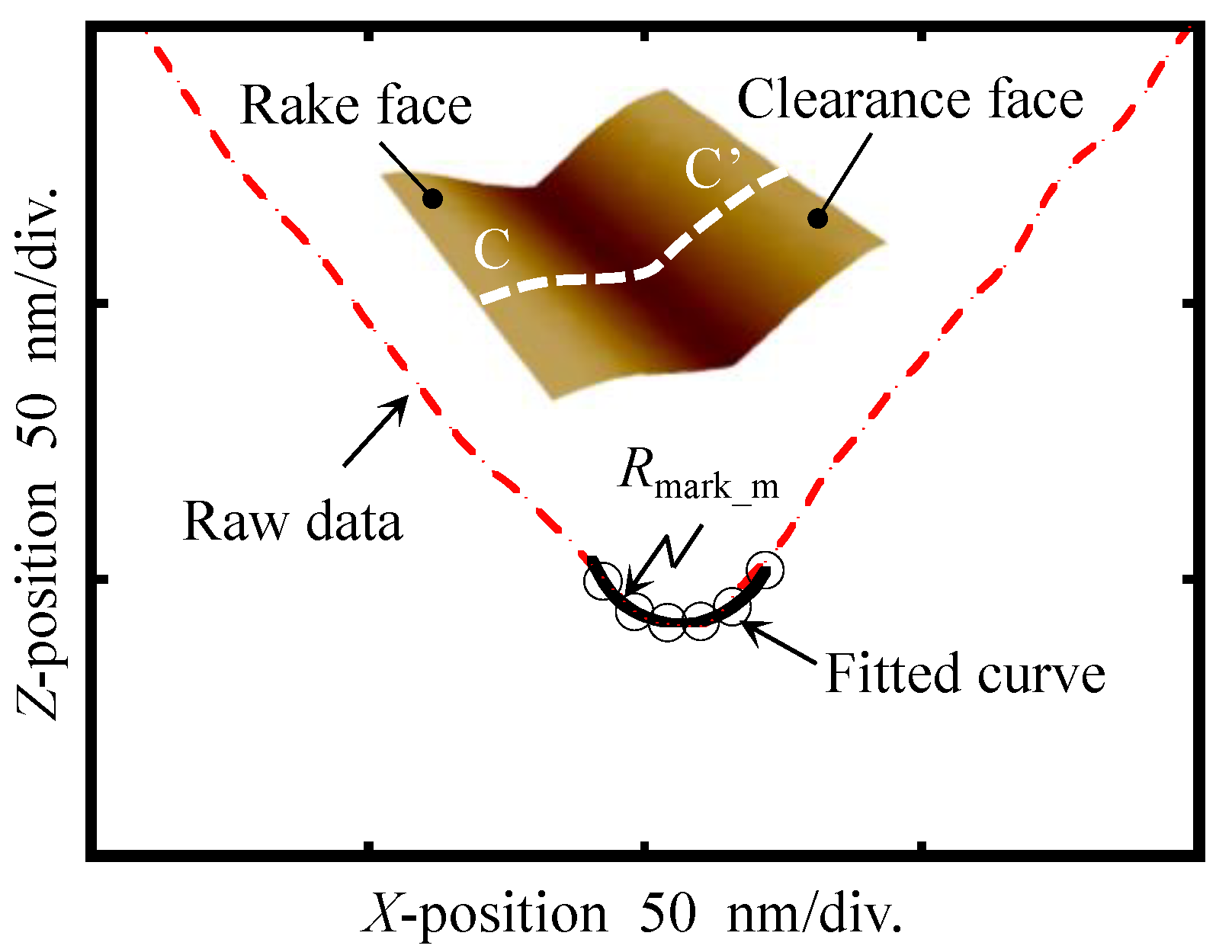
3.1. Evaluation of the Included Angle of the AFM Probe
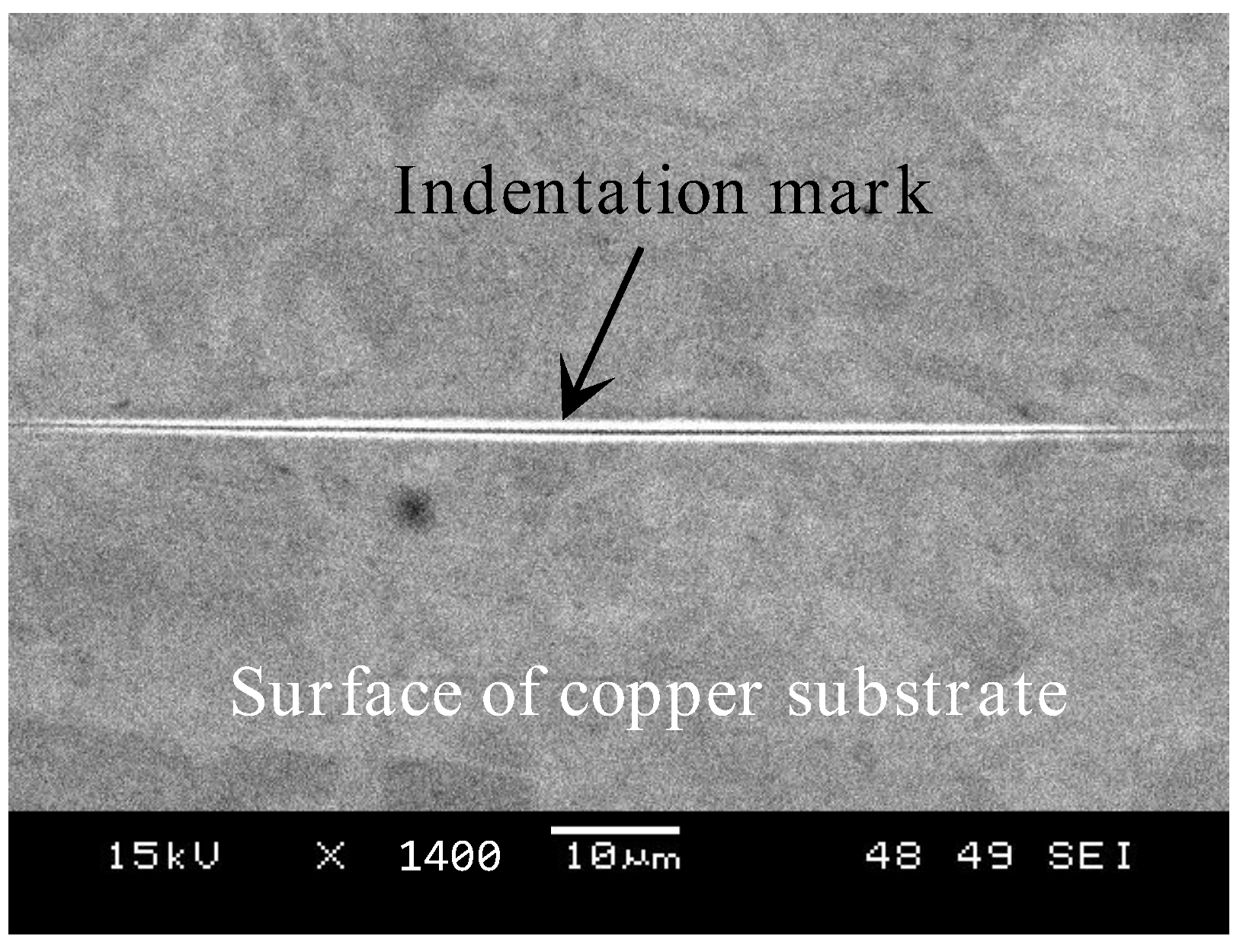
3.2. Evaluation of the Tip Radius of the AFM Probe
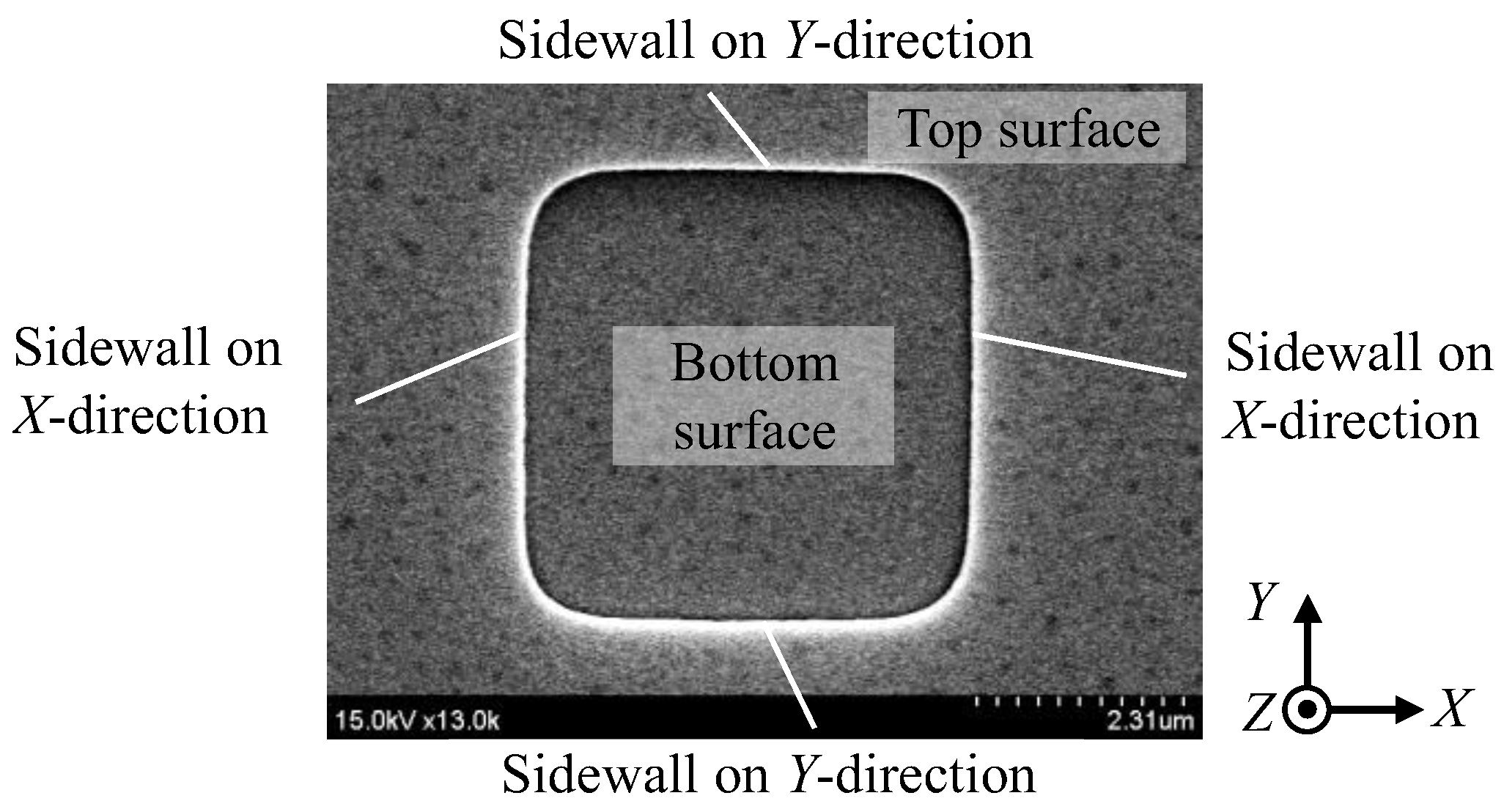
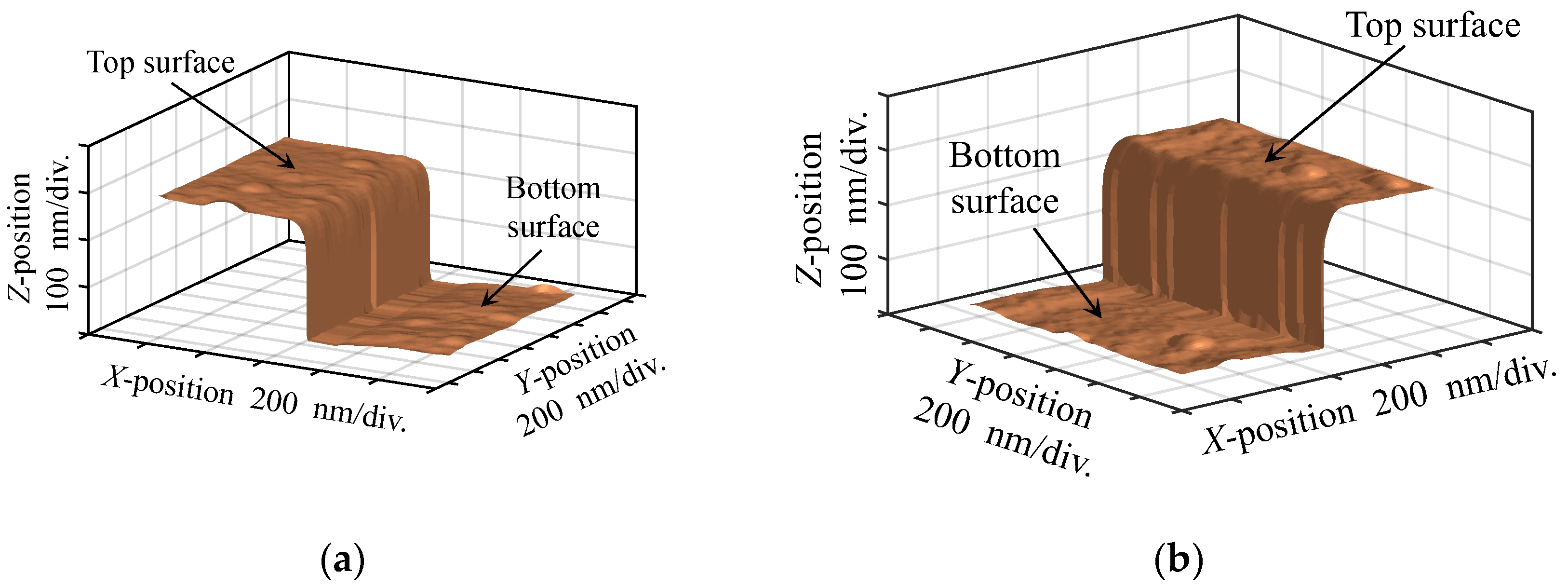
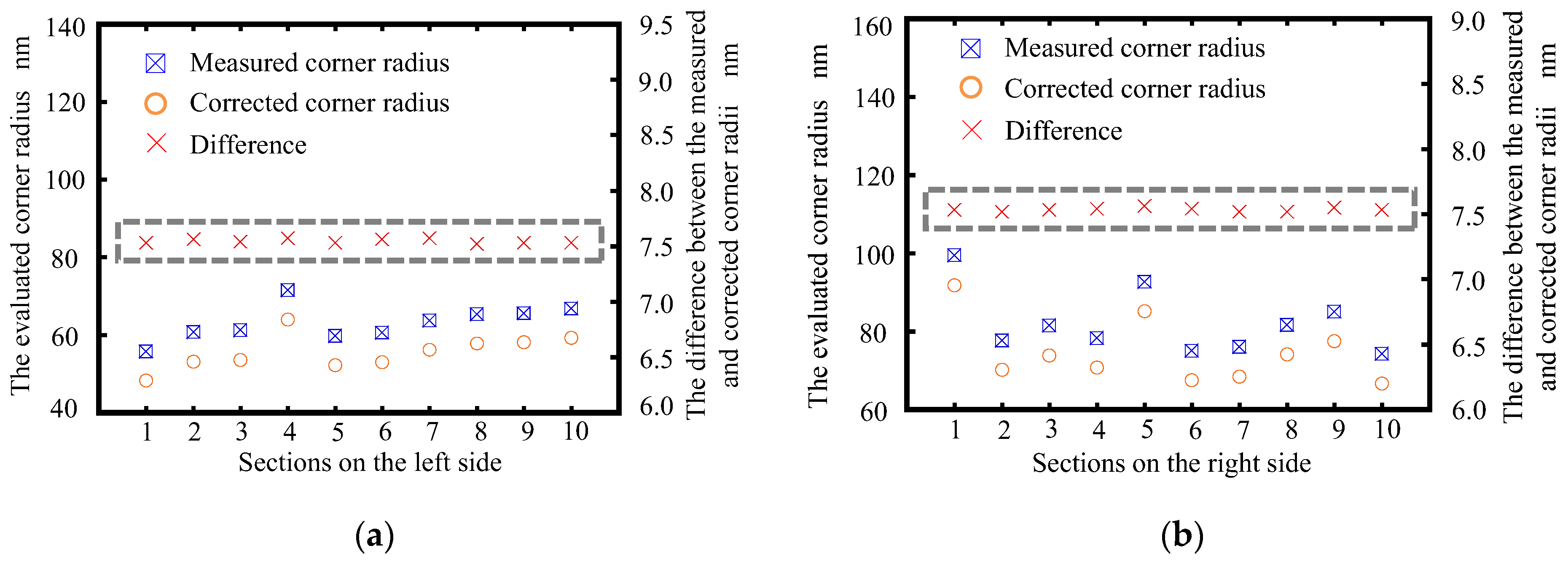
3.3. Reconstruction of 3D AFM Image of the Diffraction Grating
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Yan, J.-N.; Chang, C.-W.; Lee, Y.-C. A metal-embedded photo-mask for contact photolithography with application on patterned sapphire substrate. Microelectron. Eng. 2014, 122, 20–24. [Google Scholar] [CrossRef]
- Gao, W. Precision Nanometrology–Sensors and Measuring Systems for Nanomanufacturing; Springer: London, UK, 2010. [Google Scholar]
- Li, X.; Gao, W.; Muto, H.; Shimizu, Y.; Ito, S.; Dian, S. A six-degree-of-freedom surface encoder for precision positioning of a planar motion stage. Precis. Eng. 2013, 37, 771–781. [Google Scholar] [CrossRef]
- Gao, W.; Haitjema, H.; Fang, F.; Leach, R.; Cheung, C.; Savio, E.; Linares, J. On-machine and in-process surface metrology for precision manufacturing. CIRP Ann. 2019, 68, 843–866. [Google Scholar] [CrossRef]
- Quan, L.; Shimizu, Y.; Xiong, X.; Matsukuma, H.; Gao, W. A new method for evaluation of the pitch deviation of a linear scale grating by an optical angle sensor. Precis. Eng. 2021, 67, 1–13. [Google Scholar] [CrossRef]
- Shimizu, Y.; Uehara, K.; Matsukuma, H.; Gao, W. Evaluation of the grating period based on laser diffraction by using a mode-locked femtosecond laser beam. J. Adv. Mech. Des. Syst. Manuf. 2018, 12, JAMDSM0097. [Google Scholar] [CrossRef]
- Yu, Z.; Chen, L.; Wu, W.; Ge, H.; Chou, S.Y. Fabrication of nanoscale gratings with reduced line edge roughness using nanoimprint lithography. J. Vac. Sci. Technol. B Microelectron. Nanometer Struct. Process. Meas. Phenom. 2003, 21, 2089–2092. [Google Scholar] [CrossRef][Green Version]
- Shin, D.W.; Quan, L.; Shimizu, Y.; Matsukuma, H.; Cai, Y.; Manske, E.; Gao, W. In-Situ Evaluation of the Pitch of a Reflective-Type Scale Grating by Using a Mode-Locked Femtosecond Laser. Appl. Sci. 2021, 11, 8028. [Google Scholar] [CrossRef]
- Dai, G.; Koenders, L.; Pohlenz, F.; Dziomba, T.; Danzebrink, H.-U. Accurate and traceable calibration of one-dimensional gratings. Meas. Sci. Technol. 2005, 16, 1241–1249. [Google Scholar] [CrossRef]
- Dai, G.; Pohlenz, F.; Dziomba, T.; Xu, M.; Diener, A.; Koenders, L.; Danzebrink, H.-U. Accurate and traceable calibration of two-dimensional gratings. Meas. Sci. Technol. 2007, 18, 415–421. [Google Scholar] [CrossRef]
- Gao, W.; Asai, T.; Arai, Y. Precision and fast measurement of 3D cutting edge profiles of single point diamond micro-tools. CIRP Ann.-Manuf. Technol. 2009, 58, 451–454. [Google Scholar] [CrossRef]
- Itoh, H.; Fujimoto, T.; Ichimura, S. Tip characterizer for atomic force microscopy. Rev. Sci. Instrum. 2006, 77, 103704. [Google Scholar] [CrossRef]
- Villarrubia, J. Algorithms for scanned probe microscope image simulation, surface reconstruction, and tip estimation. J. Res. Natl. Inst. Stand. Technol. 1997, 102, 425–454. [Google Scholar] [CrossRef]
- Dongmo, L.; Villarrubia, J.; Jones, S.; Renegar, T.; Postek, M.; Song, J. Experimental test of blind tip reconstruction for scanning probe microscopy. Ultramicroscopy 2000, 85, 141–153. [Google Scholar] [CrossRef]
- Orji, N.G.; Dixson, R.G. Higher order tip effects in traceable CD-AFM-based linewidth measurements. Meas. Sci. Technol. 2007, 18, 448–455. [Google Scholar] [CrossRef]
- Nguyen, C.V.; Ye, Q.; Meyyappan, M. Carbon nanotube tips for scanning probe microscopy: Fabrication and high aspect ratio nanometrology. Meas. Sci. Technol. 2005, 16, 2138–2146. [Google Scholar] [CrossRef]
- Dai, H.; Hafner, J.H.; Rinzler, A.G.; Colbert, D.T.; Smalley, R.E. Nanotubes as nanoprobes in scanning probe microscopy. Nature 1996, 384, 147–150. [Google Scholar] [CrossRef]
- Martin, Y.; Wickramasinghe, H.K. Method for imaging sidewalls by atomic force microscopy. Appl. Phys. Lett. 1994, 64, 2498–2500. [Google Scholar] [CrossRef]
- Dahlen, G.; Osborn, M.; Liu, H.-C.; Jain, R.; Foreman, W.; Osborne, J.R. Critical dimension AFM tip characterization and image reconstruction applied to the 45-nm node. In Proceedings of the SPIE 31st International Symposium on Advanced Lithography, San Jose, CA, USA, 19–24 February 2006; Volume 6152, pp. 945–955. [Google Scholar]
- Hussain, D.; Ahmad, K.; Song, J.; Xie, H. Advances in the atomic force microscopy for critical dimension metrology. Meas. Sci. Technol. 2016, 28, 012001. [Google Scholar] [CrossRef]
- Bykov, V.; Novikov, Y.; Rakov, A.; Shikin, S. Defining the parameters of a cantilever tip AFM by reference structure. Ultramicroscopy 2003, 96, 175–180. [Google Scholar] [CrossRef]
- Vesenka, J.; Miller, R.; Henderson, E. Three-dimensional probe reconstruction for atomic force microscopy. Rev. Sci. Instrum. 1994, 65, 2249–2251. [Google Scholar] [CrossRef][Green Version]
- Yue, X.; Lei, D.; Cui, H.; Zhang, X.; Xu, M.; Kong, L. An integrated method for measurement of diamond tools based on AFM. Precis. Eng. 2017, 50, 132–141. [Google Scholar] [CrossRef]
- Liu, J.; Notbohm, J.K.; Carpick, R.W.; Turner, K.T. Method for Characterizing Nanoscale Wear of Atomic Force Microscope Tips. ACS Nano 2010, 4, 3763–3772. [Google Scholar] [CrossRef] [PubMed]
- Chen, L.; Cheung, C.L.; Ashby, P.D.; Lieber, C.M. Single-Walled Carbon Nanotube AFM Probes: Optimal Imaging Resolution of Nanoclusters and Biomolecules in Ambient and Fluid Environments. Nano Lett. 2004, 4, 1725–1731. [Google Scholar] [CrossRef]
- Chen, Z.; Luo, J.; Doudevski, I.; Erten, S.; Kim, S.H. Atomic Force Microscopy (AFM) Analysis of an Object Larger and Sharper than the AFM Tip. Microsc. Microanal. 2019, 25, 1106–1111. [Google Scholar] [CrossRef]
- Morgenroth, W.; Meyer, H.; Sulzbach, T.; Brendel, B.; Hübner, U.; Mirandé, W. Downwards to metrology in nanoscale: Determination of the AFM tip shape with well-known sharp-edged calibration structures. Appl. Phys. A Mater. Sci. Process. 2003, 76, 913–917. [Google Scholar] [CrossRef]
- Chen, Y.-L.; Cai, Y.; Xu, M.; Shimizu, Y.; Ito, S.; Gao, W. An edge reversal method for precision measurement of cutting edge radius of single point diamond tools. Precis. Eng. 2017, 50, 380–387. [Google Scholar] [CrossRef]
- Zhang, K.; Shimizu, Y.; Matsukuma, H.; Cai, Y.; Gao, W. An application of the edge reversal method for accurate reconstruction of the three-dimensional profile of a single-point diamond tool obtained by an atomic force microscope. Int. J. Adv. Manuf. Technol. 2021, 117, 2883–2893. [Google Scholar] [CrossRef]
- Cai, Y.; Chen, Y.-L.; Xu, M.; Shimizu, Y.; Ito, S.; Matsukuma, H.; Gao, W. An ultra-precision tool nanoindentation instrument for replication of single point diamond tool cutting edges. Meas. Sci. Technol. 2018, 29, 054004. [Google Scholar] [CrossRef]
- Cai, Y.; Chen, Y.-L.; Shimizu, Y.; Ito, S.; Gao, W. Molecular dynamics simulation of elastic–plastic deformation associated with tool–workpiece contact in force sensor–integrated fast tool servo. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2018, 232, 1893–1902. [Google Scholar] [CrossRef]
- Cai, Y.; Chen, Y.-L.; Shimizu, Y.; Ito, S.; Gao, W.; Zhang, L. Molecular dynamics simulation of subnanometric tool-workpiece contact on a force sensor-integrated fast tool servo for ultra-precision microcutting. Appl. Surf. Sci. 2016, 369, 354–365. [Google Scholar] [CrossRef]
- Zhang, K.; Cai, Y.; Shimizu, Y.; Matsukuma, H.; Gao, W. High-Precision Cutting Edge Radius Measurement of Single Point Diamond Tools Using an Atomic Force Microscope and a Reverse Cutting Edge Artifact. Appl. Sci. 2020, 10, 4799. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, K.; Bai, Y.; Zhang, Z. Compensation Method for Correcting the Topography Convolution of the 3D AFM Profile Image of a Diffraction Grating. Machines 2024, 12, 126. https://doi.org/10.3390/machines12020126
Zhang K, Bai Y, Zhang Z. Compensation Method for Correcting the Topography Convolution of the 3D AFM Profile Image of a Diffraction Grating. Machines. 2024; 12(2):126. https://doi.org/10.3390/machines12020126
Chicago/Turabian StyleZhang, Kai, Yang Bai, and Zhimin Zhang. 2024. "Compensation Method for Correcting the Topography Convolution of the 3D AFM Profile Image of a Diffraction Grating" Machines 12, no. 2: 126. https://doi.org/10.3390/machines12020126
APA StyleZhang, K., Bai, Y., & Zhang, Z. (2024). Compensation Method for Correcting the Topography Convolution of the 3D AFM Profile Image of a Diffraction Grating. Machines, 12(2), 126. https://doi.org/10.3390/machines12020126






