Study of the Dynamic Properties of the Miniature Electro-Hydrostatic Actuator
Abstract
1. Introduction
2. System Composition and Working Principle
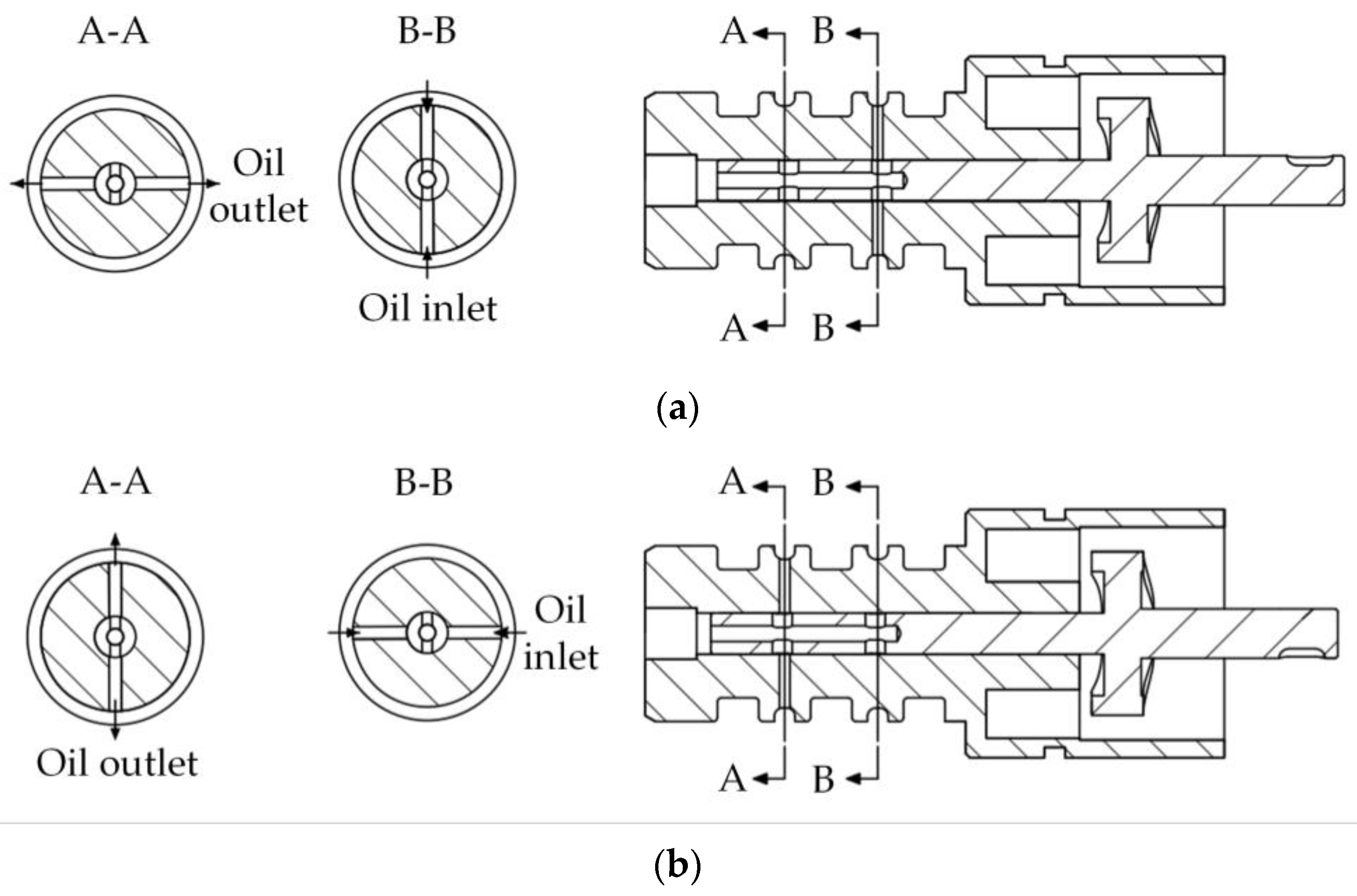
2.1. The Structure and Working Principle of Micro Two-Dimensional (2D) Pump
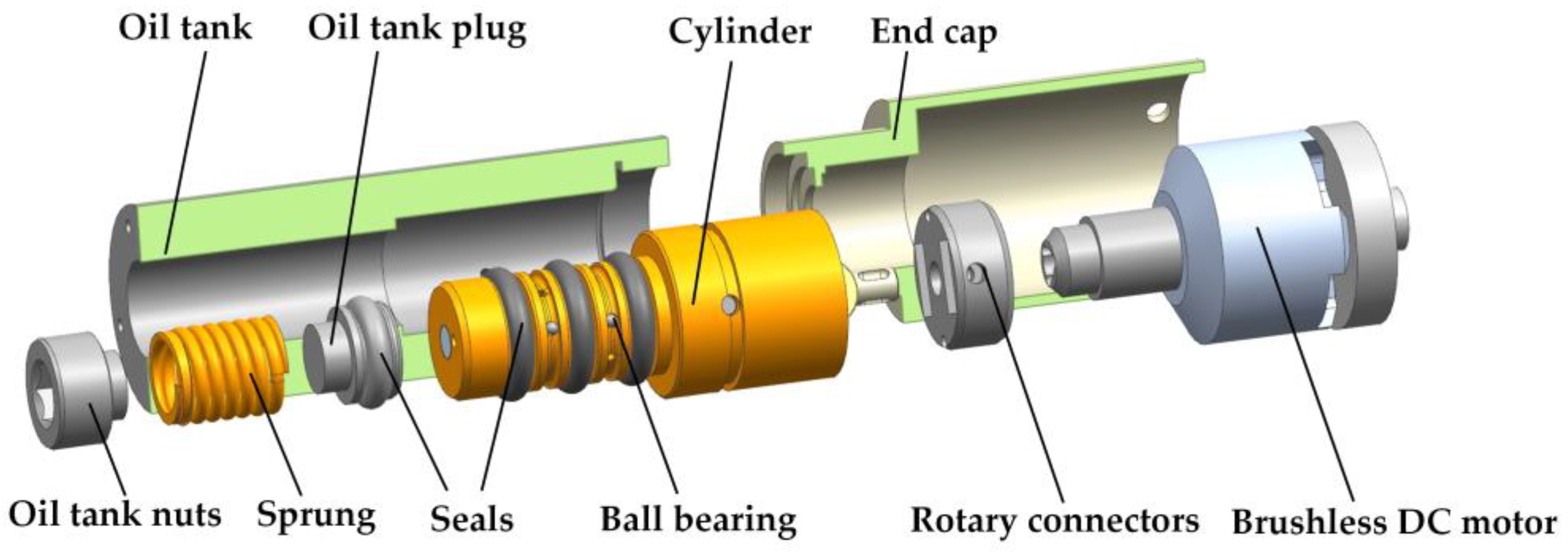
2.2. Components and Working Principle of the Miniature Electro-Hydrostatic Actuator (EHA)
3. Motor Modeling and Control Design
3.1. The Mathematical Model of Brushless DC Motor
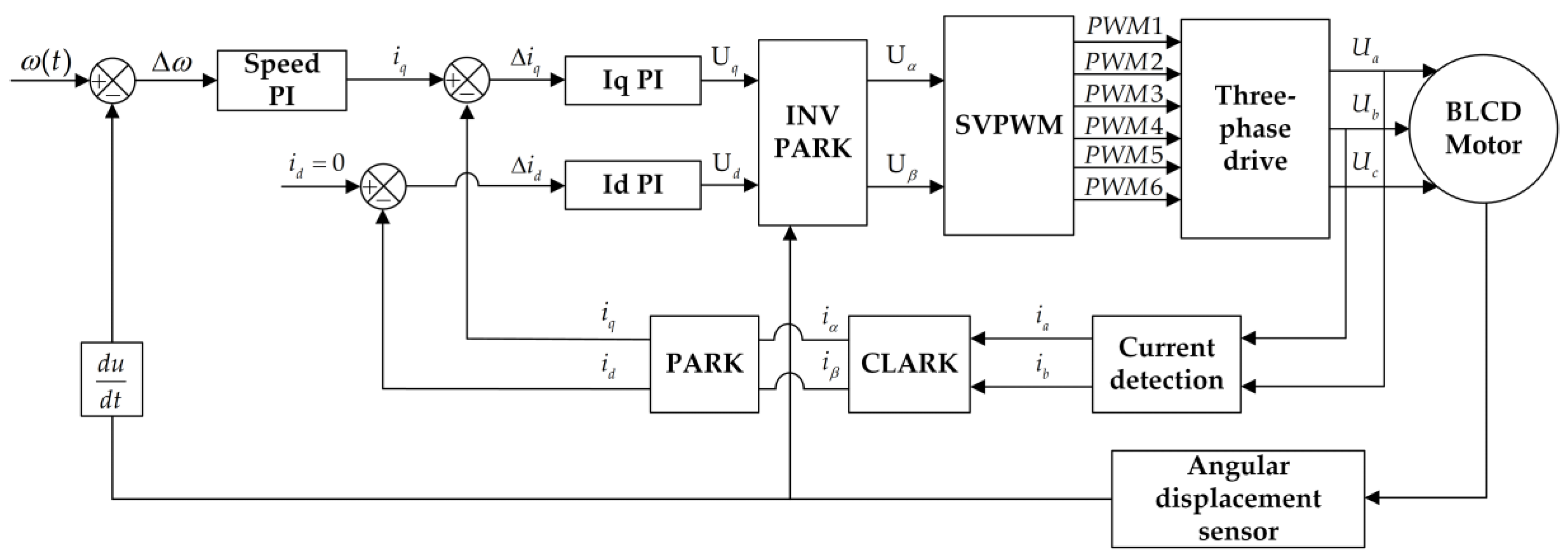
3.2. Control Strategy Design
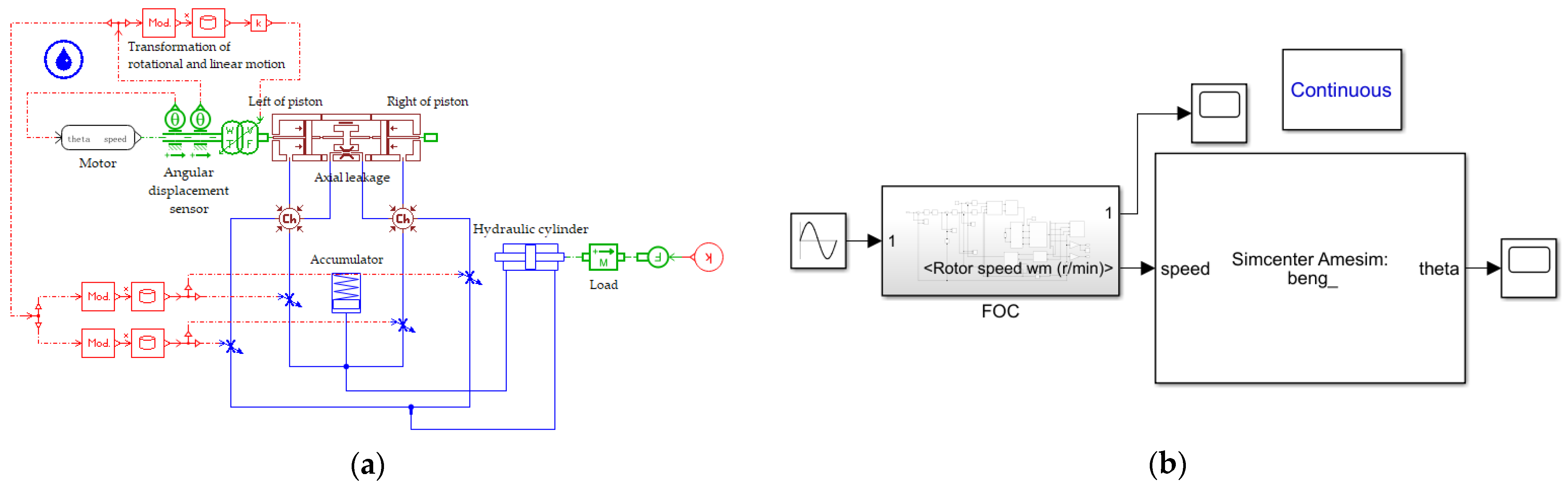
3.3. Simulation Model of the Controller
4. Modelling of The EHA Hydraulic System
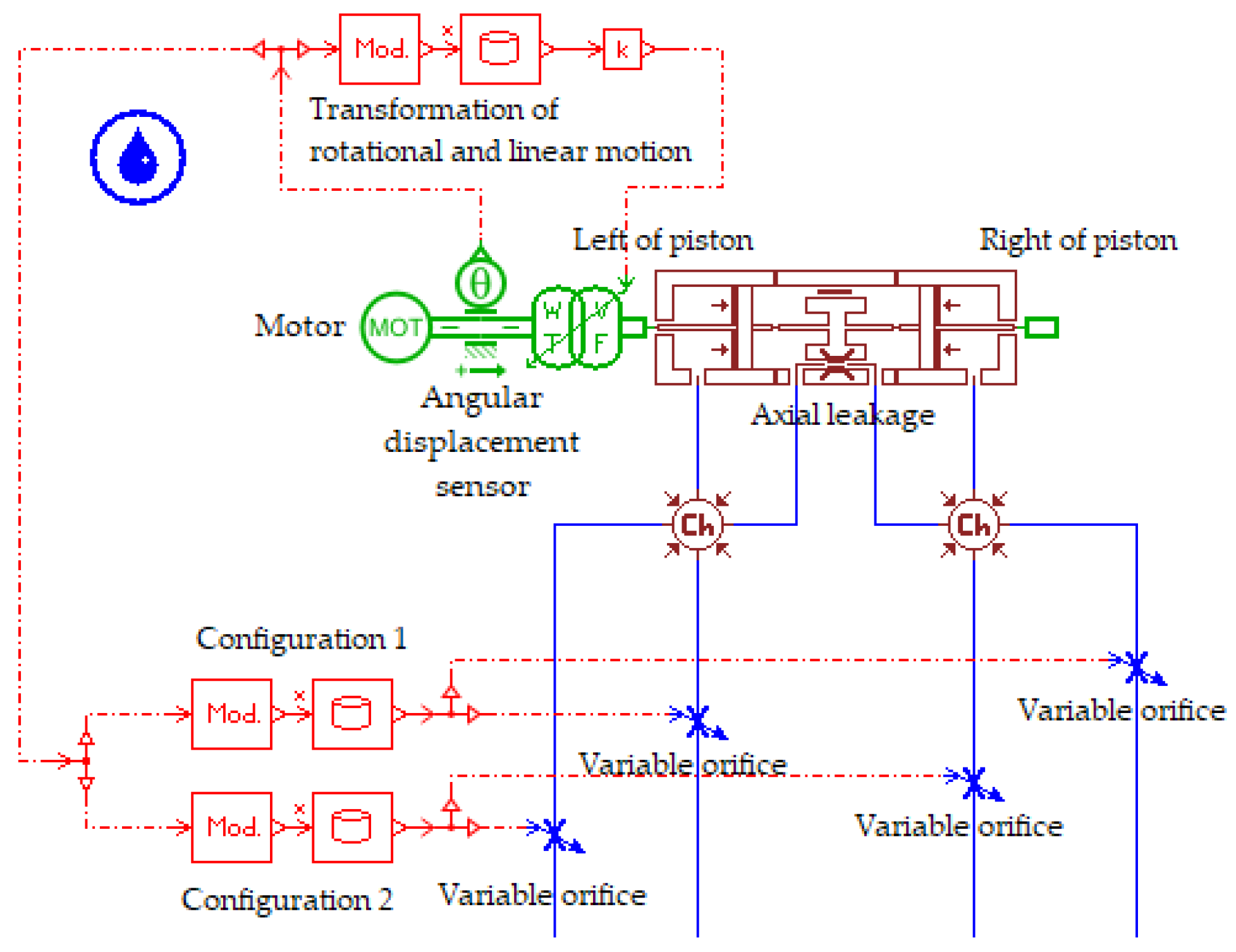
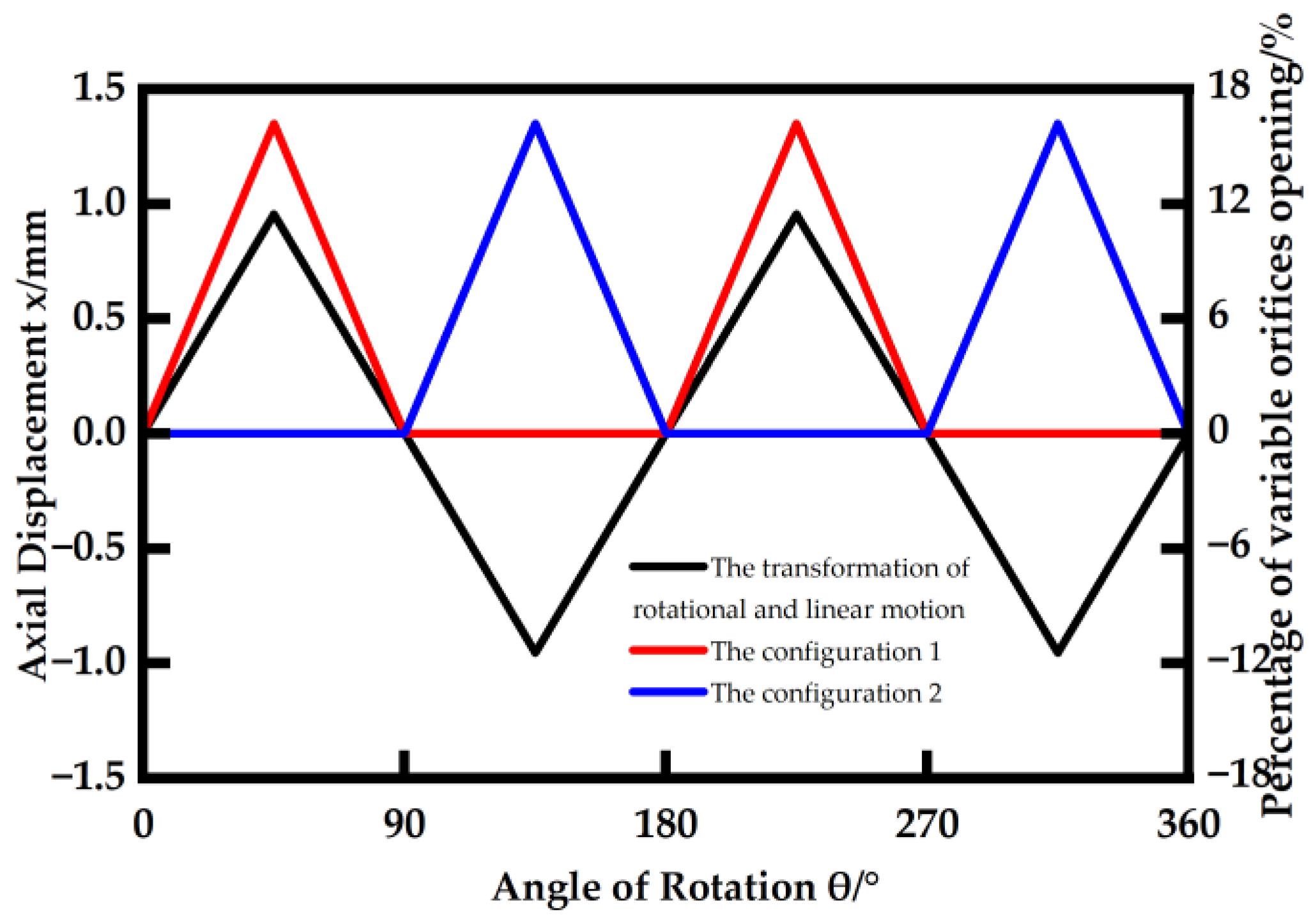
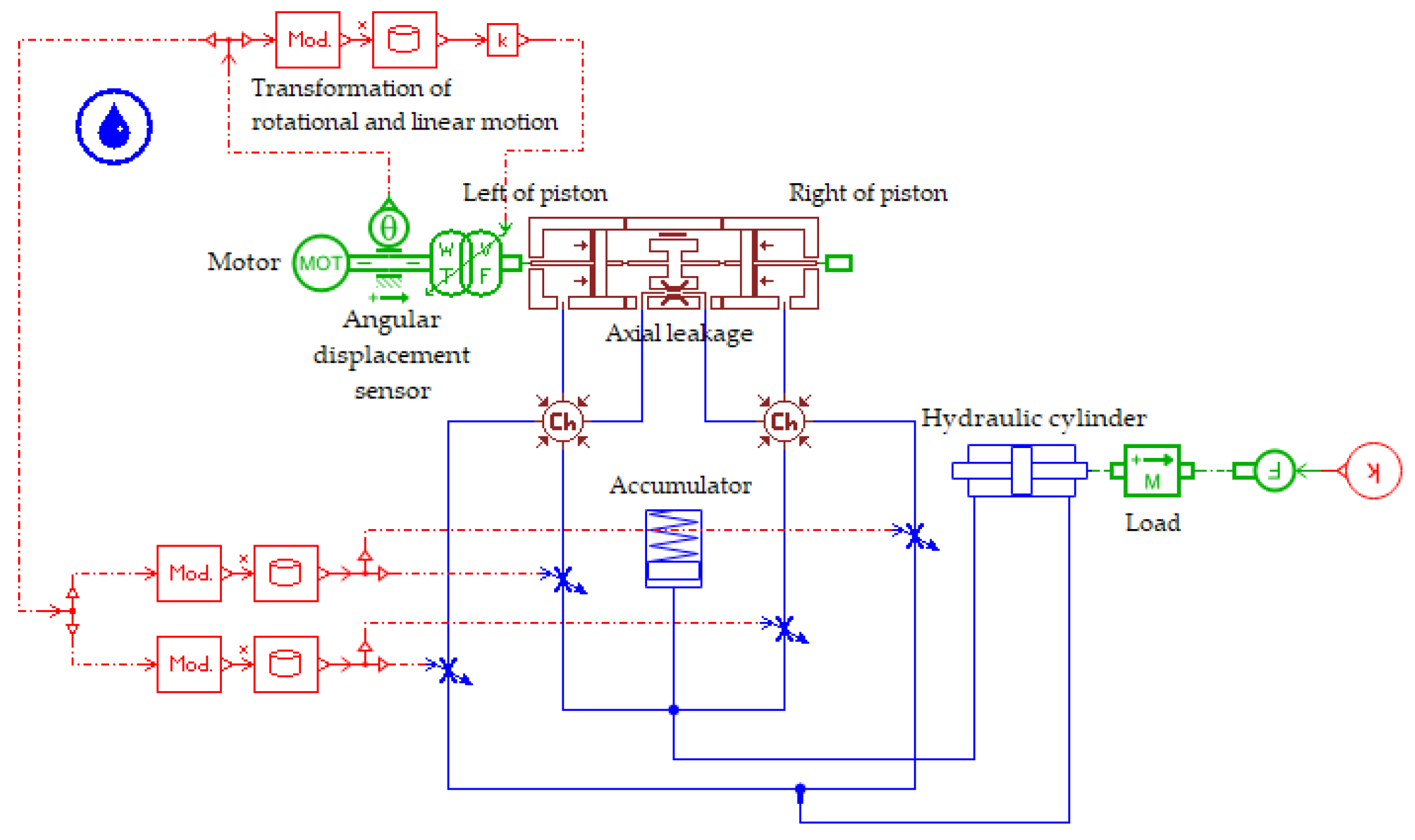
4.1. The Mathematical Model of the Pump and the Hydraulic Cylinder
4.2. Simulation Modeling of Micro Two-Dimensional (2D) Pump
4.3. Simulation Modeling of the Hydraulic System
5. Simulation and Experimentation
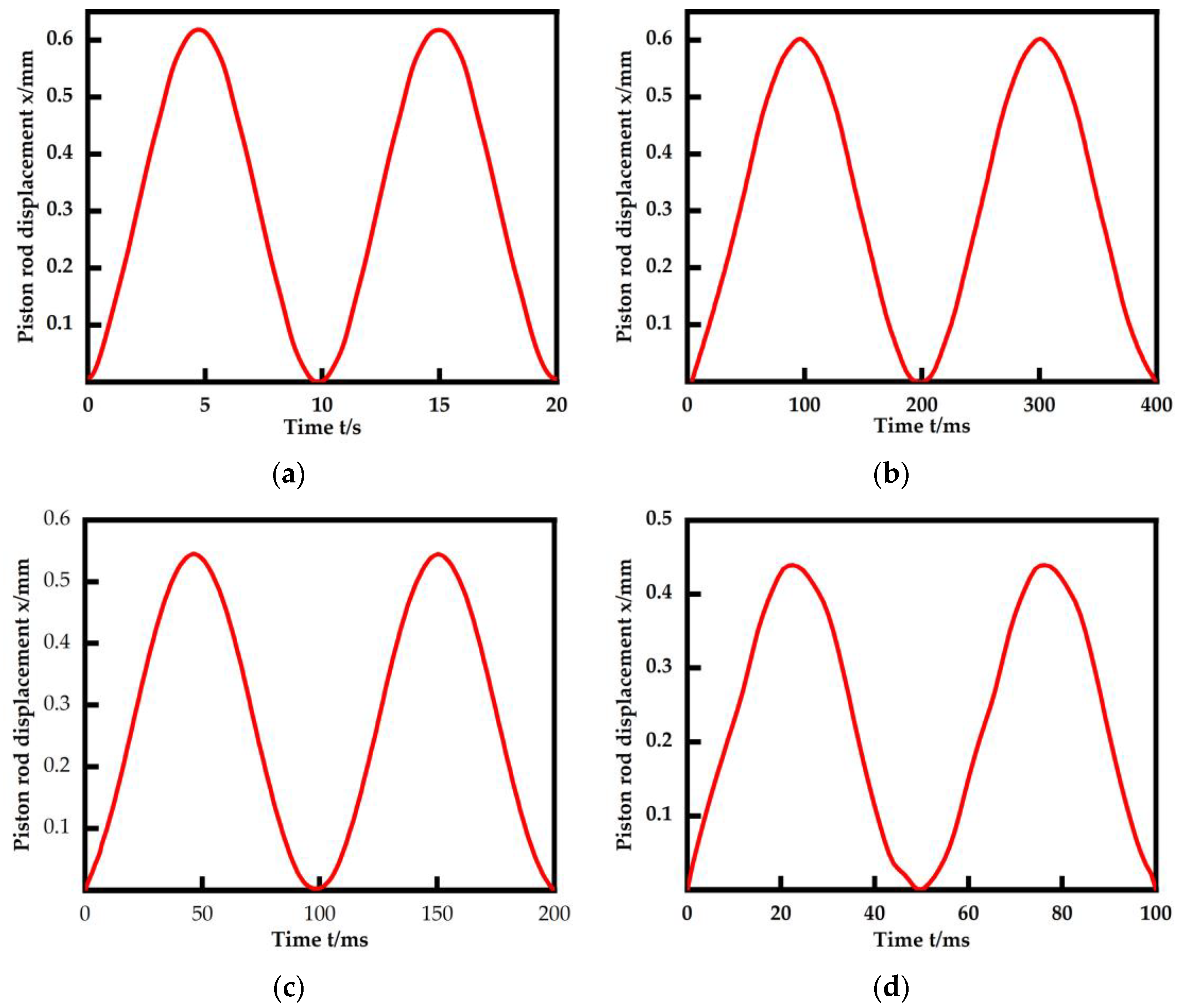
5.1. Simulation
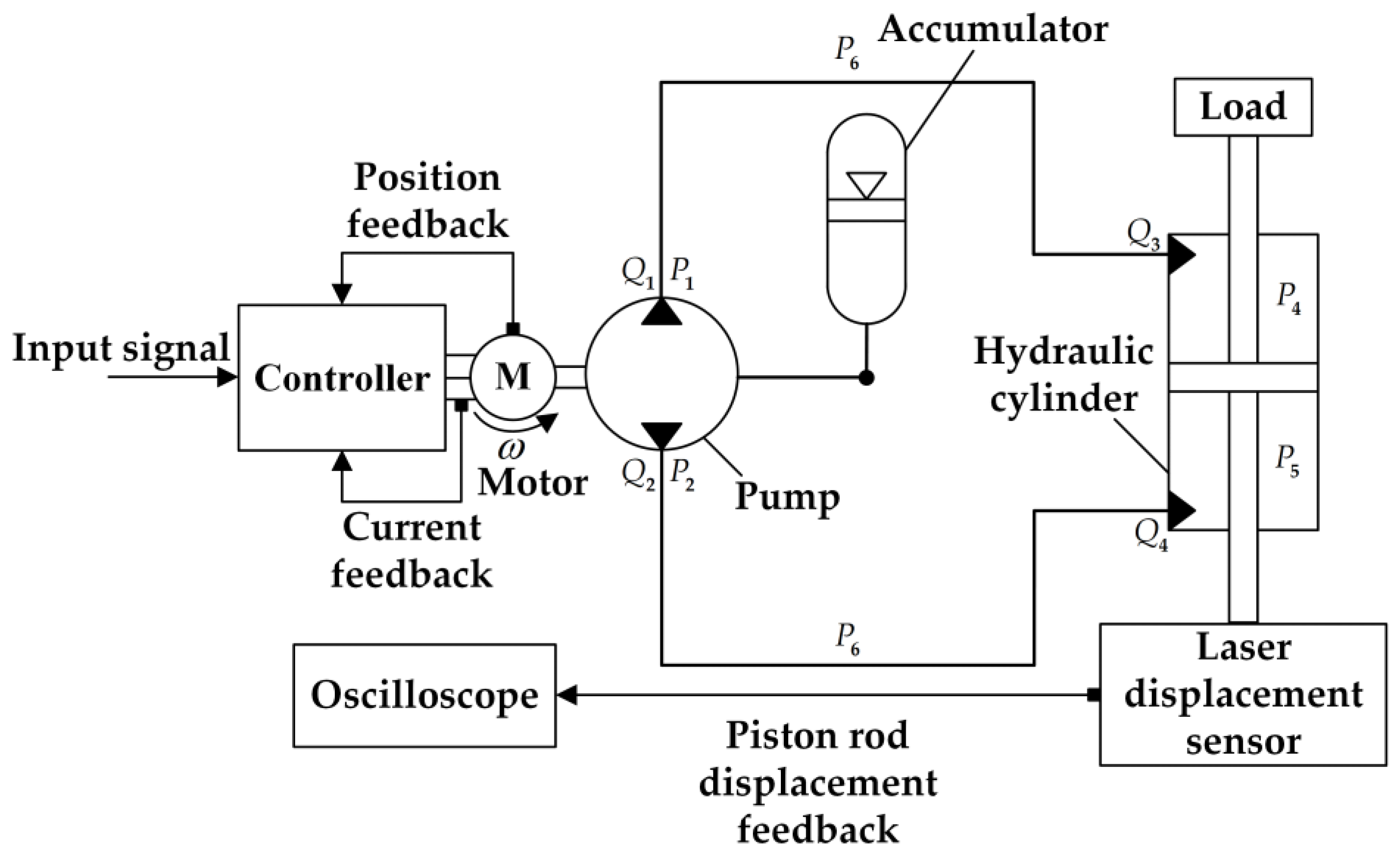
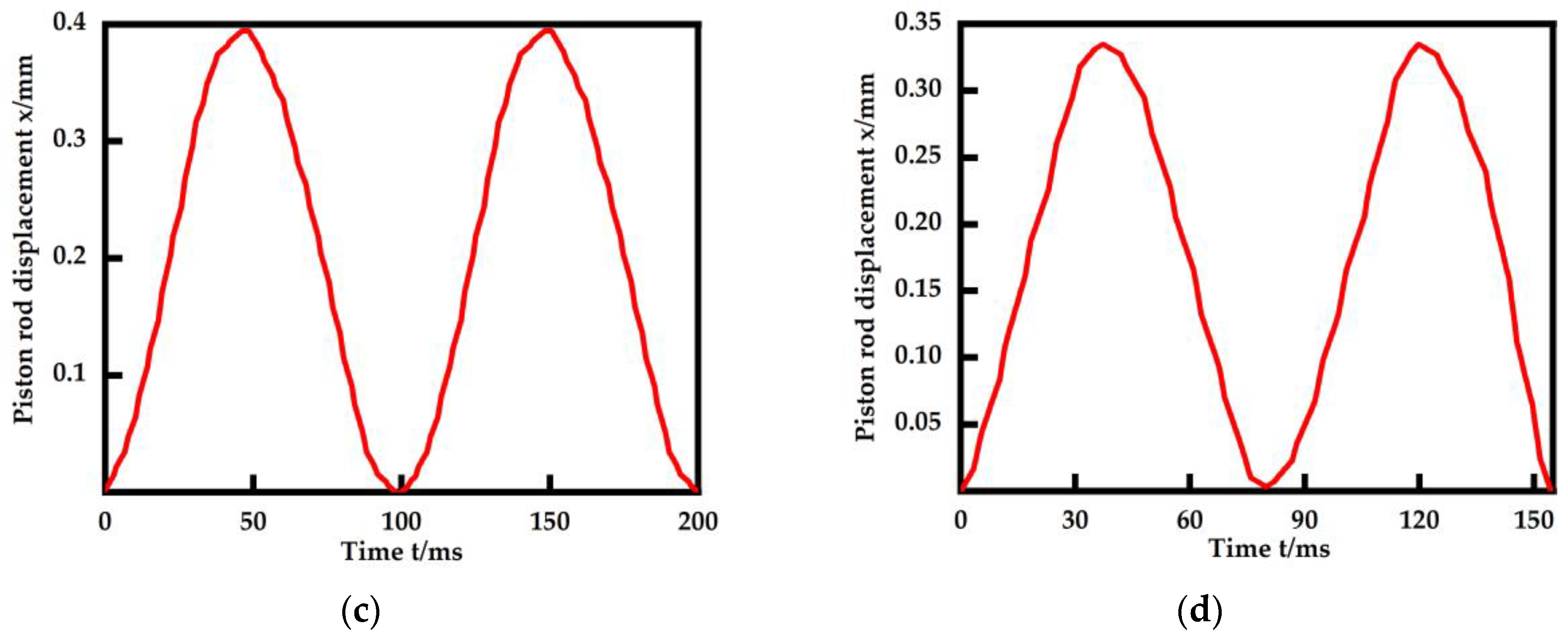
5.2. Experimental Research
6. Conclusions and Future Work
- An analysis of the structure and working principle of the micro 2D pump and the EHA system was performed. Compared with previous EHAs, the miniature EHA designed in this paper is smaller in size and simpler in structure. The total weight of this EHA does not exceed 300 g.
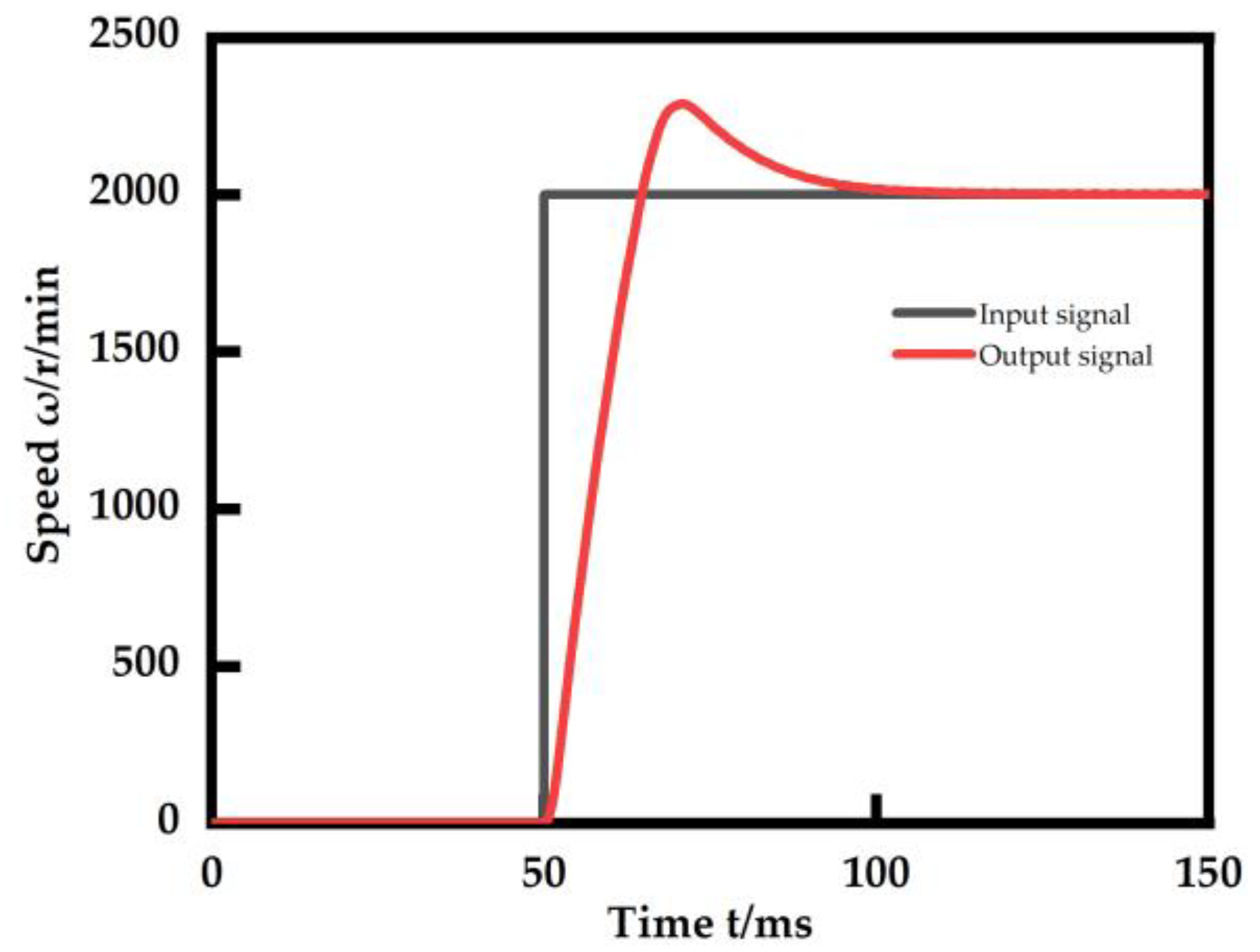
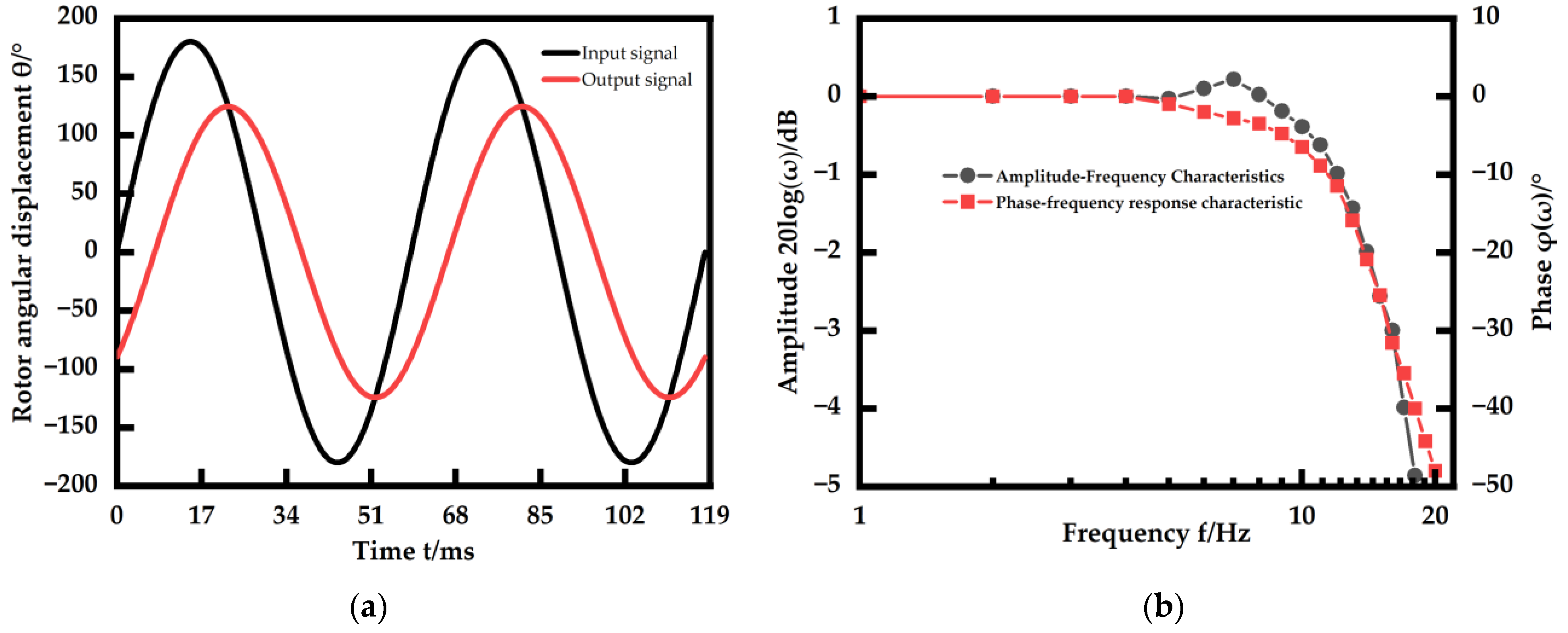
- A simulation model of the miniature EHA system was developed, and it was controlled using a suitable control algorithm. A joint simulation of Simulink and AMESim was used, and the simulation data show that the rise time of this EHA system is 0.158 s with a step signal of 6000 r/min and a bandwidth of 20 Hz.
- An experimental verification of the results of theoretical studies was carried out on a specially designed experimental stand for studying the dynamic properties of the EHA system. The rise time of the miniature EHA at a step signal of 6000 r/min is 0.242 s and the bandwidth is 13 Hz. The experimental results can correspond to the simulation results, indicating the reliability of this EHA system design scheme.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| Parameter | Value |
| Internal resistance of motor | |
| Quadrature axis inductance | |
| Direct axis inductance | |
| Motor speed | |
| Flux linkage coefficient | |
| Stator phase winding current | |
| Stator phase winding electromotive force | |
| Rotor moment of inertia | |
| Load torque | |
| Total pump displacement | |
| Pump moment of inertia | |
| Friction coefficient | |
| Loss torque | |
| Pump discharge pressure | |
| Pump inlet pressure | |
| Pump volume change | |
| Pump displacement | |
| Pump input flow | |
| Pump output flow | |
| Internal leakage coefficient | |
| External leakage coefficient | |
| Pump external leakage port pressure | |
| Volume of the fluid | |
| Effective area of the piston of the hydraulic cylinder | |
| Displacement of the piston rod of the hydraulic cylinder | |
| Modulus of elasticity of the volume of the fluid | |
| Pressure of the two chambers of the hydraulic cylinder | |
| Flow rate of the two chambers of the hydraulic cylinder | |
| Flow rate of the load of the hydraulic cylinder | |
| Pressure drop in the hydraulic line of the EHA | |
| Mass of the piston rod of the hydraulic cylinder | |
| Elastic stiffness of the load | |
| Piston damping coefficient | |
| External resistance |
References
- Li, B.; Liu, Y.; Tan, C.; Qin, Q.; Lu, Y. Review on electro-hydrostatic actuator: System configurations, design methods and control technologies. Int. J. Mechatron. Manuf. Syst. 2020, 13, 323–346. [Google Scholar] [CrossRef]
- Gaile, A.; Lue, Y. Electro-hydraulic Actuation (EHA) Systems for Primary Flight Control, Landing Gear and Other Type of Actuation. In Proceedings of the 2016 IEEE International Conference on Aircraft Utility Systems (AUS), Beijing, China, 10–12 October 2016; IEEE: New York, NY, USA, 2016; pp. 723–728. [Google Scholar]
- Todeschi, M. Airbus-EMAs for flight controls actuation system 2012 status and perspectives. In Proceedings of the 5th International Conference on Recent Advances in Aerospace Actuation Systems and Components, Toulouse, France, 13–14 June 2012; pp. 1–10. [Google Scholar]
- Fu, Y.; Han, X.; Yang, R. A review of design methods for electro-hydrostatic actuators. J. Beijing Univ. Aeronaut. Astronaut. 2017, 43, 1939–1952. [Google Scholar] [CrossRef]
- Zhu, T.; Xie, H.; Yang, H. Design and tracking control of an electro-hydrostatic actuator for a disc cutter replacement manipulator. Autom. Constr. 2022, 142, 104480. [Google Scholar] [CrossRef]
- Liu, Z.; Lei, X.; Li, L. Research on the effect of servo motor response speed on the performance of EHA position control. Hydraul. Pneum. Seal. 2022, 42, 41–46. [Google Scholar] [CrossRef]
- Nakanishi, T.; Komagata, M.; Yamamoto, K.; Nakamura, Y. Toward High Power-to-Weight Ratio Electro-hydrostatic Actuators for Robots. In Springer Proceedings in Advanced Robotics; Springer Science and Business Media B.V.: Berlin/Heidelberg, Germany, 2021; Volume 19, pp. 116–125. [Google Scholar] [CrossRef]
- Niu, Z.; Liu, Y.S.; Wang, L.; Yang, S.; Li, X. Portable electro-hydraulic actuator technology based on spherical micro pump. In Proceedings of the 2016 IEEE International Conference on Aircraft Utility Systems (AUS), Beijing, China, 10–12 October 2016; pp. 114–118. [Google Scholar] [CrossRef]
- Aubin, C.A.; Choudhury, S.; Jerch, R.; Archer, L.A.; Pikul, J.H.; Shepherd, R.F. Electrolytic vascular systems for energy-dense robots. Nature 2019, 571, 51–57. [Google Scholar] [CrossRef] [PubMed]
- Song, X.; Li, H.Y.; Li, Y.; Luo, X. The development of a high-speed miniature pump with dynamic bearing. J. Phys. Conf. Ser. 2022, 2217, 012049. [Google Scholar] [CrossRef]
- Huang, Y.; Ruan, J.; Chen, Y.; Ding, C.; Li, S. Research on the volumetric efficiency of 2D piston pumps with a balanced force. Energies 2020, 13, 4796. [Google Scholar] [CrossRef]
- Jin, D.; Ruan, J.; Li, S.; Meng, B.; Wang, L. Modelling and validation of a roller-cam rail mechanism used in a 2D piston pump. J. Zhejiang Univ. Sci. A 2019, 20, 201–217. [Google Scholar] [CrossRef]
- Zhong, Q.; Xu, E.G.; Jia, T.W.; Yang, H.Y.; Zhang, B.; Li, Y.B. Dynamic performance and control accuracy of a novel proportional valve with a switching technology-controlled pilot stage. J. Zhejiang Univ.-Sci. A 2022, 23, 272–285. [Google Scholar] [CrossRef]
- Lu, L.; Chen, Y.; Tong, C.; Ruan, J.; Li, S. Design strategy and performance evaluation of novel miniature two-dimensional (2D) piston pump with a dual stacking mechanism. Alex. Eng. J. 2023, 62, 541–554. [Google Scholar] [CrossRef]
- Chao, Q.; Xu, Z.; Tao, J.; Liu, C. Capped piston: A promising design to reduce compressibility effects, pressure ripple and cavitation for high-speed and high-pressure axial piston pumps. Alex. Eng. J. 2023, 62, 509–521. [Google Scholar] [CrossRef]
- Zielinski, M.; Myszkowski, A.; Pelic, M.; Staniek, R. Low-speed radial piston pump as an effective alternative power transmission for small hydropower plants. Renew. Energy 2022, 182, 1012–1027. [Google Scholar] [CrossRef]
- Wang, F.; Wu, J.; Lin, Z.; Zhang, H.; Xu, B. A power-sharing electro-hydraulic actuator system to downsize electric motors for electric mobile machines. Energy 2023, 284, 129343. [Google Scholar] [CrossRef]
- Komagata, M.; Yamamoto, K.; Nakamura, Y. Compact, backdrivable, and efficient design of linear electro-hydrostatic actuator module. Adv. Robot. 2022, 36, 1030–1047. [Google Scholar] [CrossRef]
- Shkolnikov, V.; Ramunas, J.; Santiago, J.G. A self-priming, roller-free, miniature, peristaltic pump operable with a single, reciprocating actuator. Sens. Actuators A Phys. 2010, 160, 141–146. [Google Scholar] [CrossRef] [PubMed]
- Hadla, H.; Santos, F. Performance Comparison of Field-oriented Control, Direct Torque Control, and Model-predictive Control for SynRMs. Chin. J. Electr. Eng. 2022, 8, 24–37. [Google Scholar] [CrossRef]
- Dörr, M.; Leitenberger, F.; Wolter, K.; Matthiesen, S.; Gwosch, T. Model-Based Control Design of an EHA Position Control Based on Multicriteria Optimization. Machines 2022, 10, 1190. [Google Scholar] [CrossRef]
- Liu, J.; Huang, Y.; Helian, B.; Nie, Y.; Chen, Z. Flow match and precision motion control of asymmetric electro-hydrostatic actuators with complex external force in four-quadrants. J. Frankl. Inst. 2023, 361, 1025–1039. [Google Scholar] [CrossRef]
- Banaszek, A.; Dašić, P.; Turmanidze, E. The influence of tilt angle of the inclined plate on the gradient of the pressure increase in the piston axial pump cylinder. In Proceedings of the Fluid Power International Conference, Maribor, Slovenia, 21 September 2023. [Google Scholar] [CrossRef]
- Petrović, R.; Banaszek, A.; Andjelković, M.; Qananah, H.R.; Alnagasa, K.A. Experimental Tests of the Piston Axial Pump with Constant Pressure and Variable Flow. Designs 2024, 8, 5. [Google Scholar] [CrossRef]
- Zhao, H.; Zhou, J.; Ma, S.; Du, S.; Liu, H.; Han, L. Design and Experiments of Electro-Hydrostatic Actuator for Wheel-Legged Robot with Fast Force Control Response. Machines 2023, 11, 685. [Google Scholar] [CrossRef]
- Kumar, M. A survey on electro hydrostatic actuator: Architecture and way ahead. Mater. Today Proc. 2021, 45 Pt 7, 6057–6063. [Google Scholar] [CrossRef]








| Component Name | Types | Weight/g | Basic Size/mm |
|---|---|---|---|
| Motor | D1806 | 20 | 26 × 18 |
| Pump | Two-dimensional piston pumps | 51.5 | 37 × 21 |
| Hydraulic cylinder | Double rod hydraulic cylinder | 49.6 | 39 × 10 |
| Parameters | Value | Unit |
|---|---|---|
| Resistance | 0.1139 | Ω |
| Quadrature axis inductance | 8.24 | μH |
| Direct axis inductance | 13.35 | μH |
| Flux linkage coefficient | 0.00407 | Wb |
| Rotor moment of inertia | 5.64 | g·cm2 |
| Pole pairs | 7 | none |
| Parameters | Value | Unit |
|---|---|---|
| Oil density | 850 | kg/m3 |
| Volumetric modulus of oil | 700 | MPa |
| Displacement of the pump | 0.0035 | mL/r |
| Pump RPM | Max 15,000 | r/min |
| Volumetric efficiency of the pump | 90% | none |
| Piston rod displacement | ±10 | mm |
| Weight of the load | 0.5 | kg |
| Basic pressure | 10 | MPa |
| Pump moment of inertia | 62.3 | g·mm2 |
| Effective area of the piston of the hydraulic cylinder | 12.567 | mm2 |
| Mass of the piston rod of the hydraulic cylinder | 21.2 | g |
| Piston damping coefficient | 0.25 | none |
| Parameters | Value |
|---|---|
| Current | 21 |
| Current | 284.75 |
| Current | 33 |
| Current | 284.75 |
| Speed | 4.5 |
| Speed | 7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
An, Y.; Mao, J.; Tong, C.; Zhou, X.; Ruan, J.; Li, S. Study of the Dynamic Properties of the Miniature Electro-Hydrostatic Actuator. Machines 2024, 12, 114. https://doi.org/10.3390/machines12020114
An Y, Mao J, Tong C, Zhou X, Ruan J, Li S. Study of the Dynamic Properties of the Miniature Electro-Hydrostatic Actuator. Machines. 2024; 12(2):114. https://doi.org/10.3390/machines12020114
Chicago/Turabian StyleAn, Yiqiang, Jiazhe Mao, Chengwei Tong, Xiaoyun Zhou, Jian Ruan, and Sheng Li. 2024. "Study of the Dynamic Properties of the Miniature Electro-Hydrostatic Actuator" Machines 12, no. 2: 114. https://doi.org/10.3390/machines12020114
APA StyleAn, Y., Mao, J., Tong, C., Zhou, X., Ruan, J., & Li, S. (2024). Study of the Dynamic Properties of the Miniature Electro-Hydrostatic Actuator. Machines, 12(2), 114. https://doi.org/10.3390/machines12020114





