Trajectory Tracking Control of Quadrotor Based on Fractional-Order S-Plane Model
Abstract
1. Introduction
2. Quaternions Model for Quadrotor UAV
3. Design of Controller
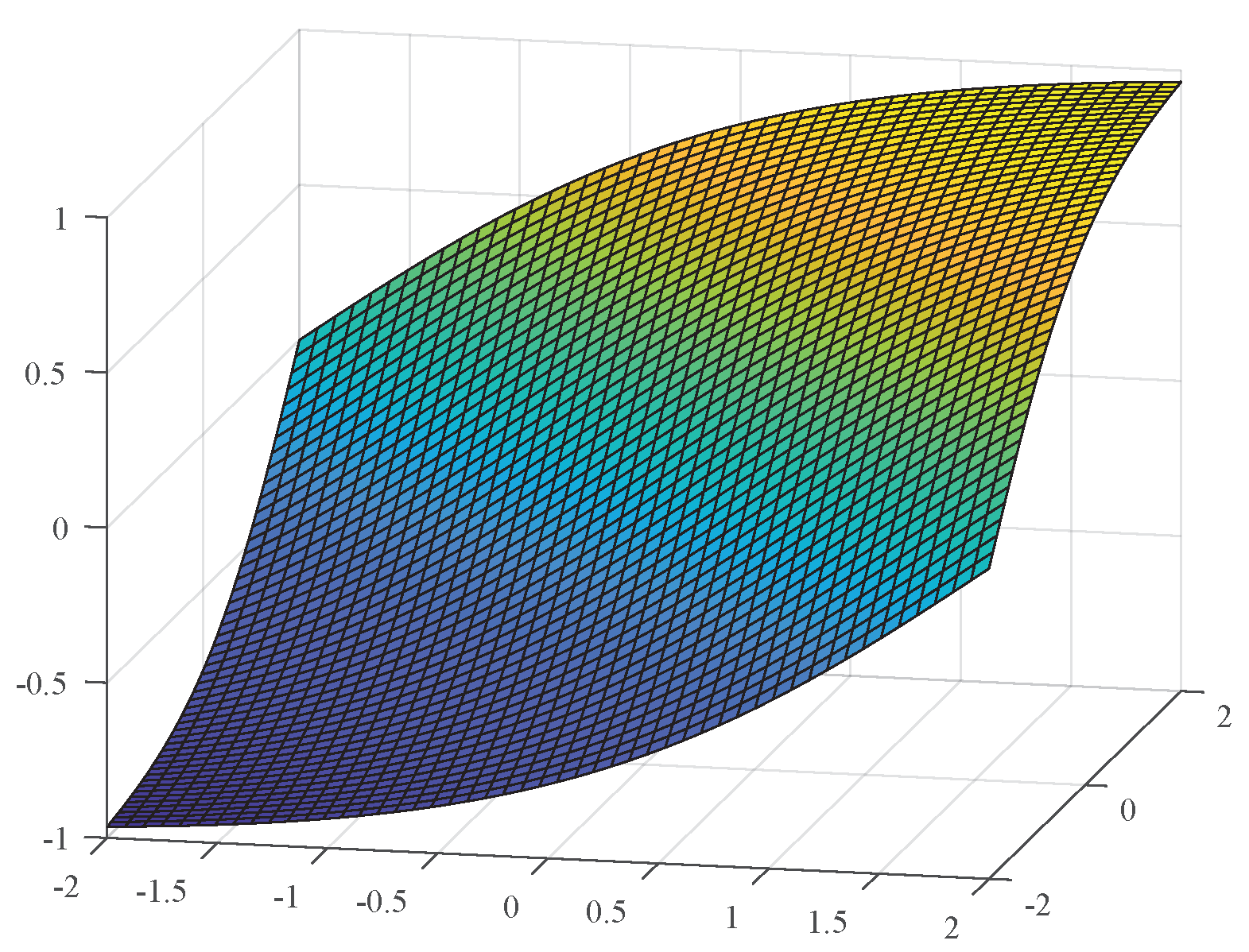
3.1. S-Plane Control
3.2. Fractional-Order Calculus
3.3. Fractional-Order Control
4. Simulation and Result Analysis
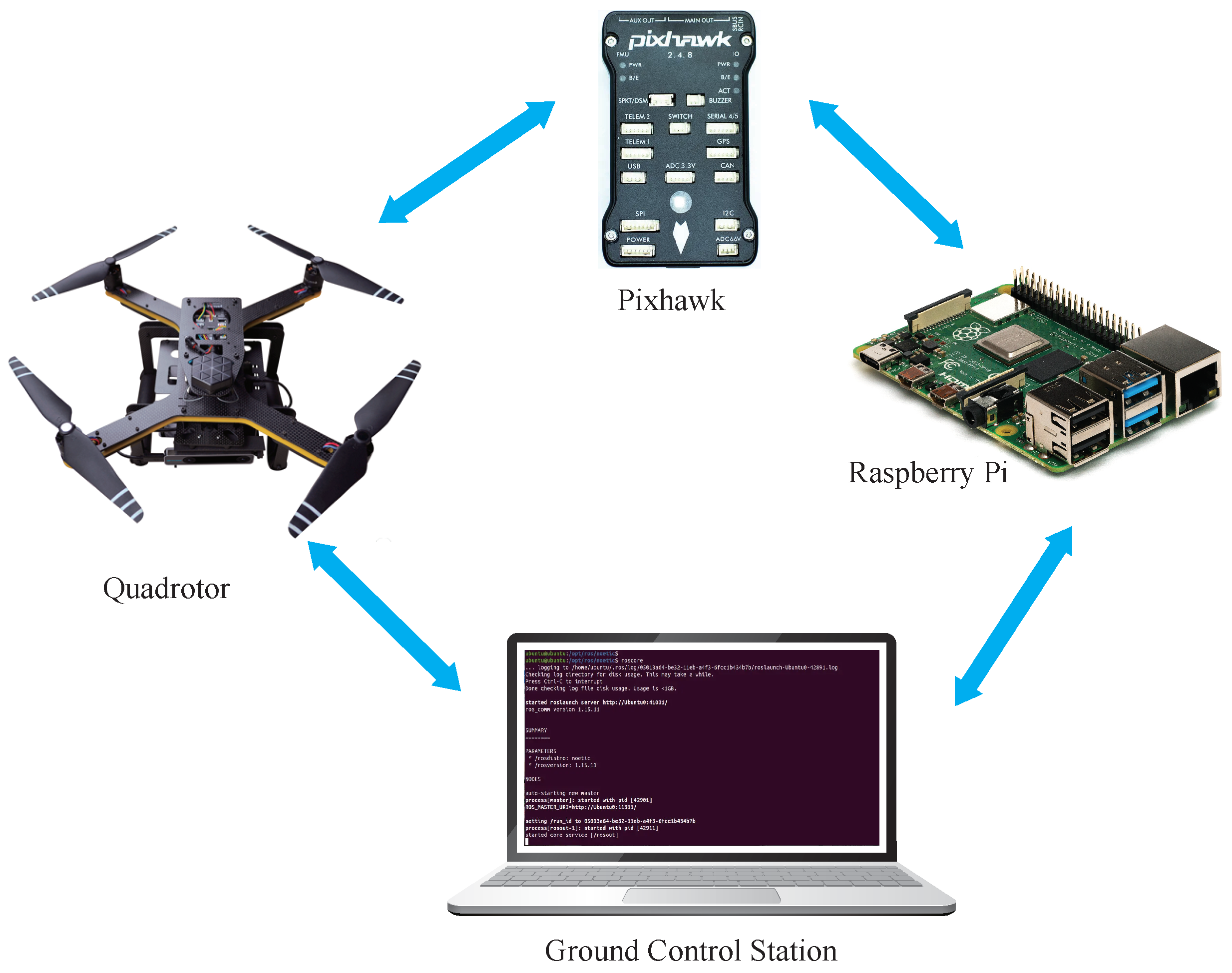
4.1. Semi-Physical Simulation
- Host computer with a virtual machine of Ubuntu 22.04 and ROS system installed.
- Z410 drone, an experimental model designed for the entry-level development of drones.
- Pixhawk2.4.8 flight controller, necessary hardware for the normal flight of the drone, controlling the attitude of the drone.
- Raspberry Pi 4B, running external control programs and other system integrations, sending external control commands or network signals to the flight controller.
- Electronic speed controller (ESC), receiving the output signal of the flight controller, processing it and driving the motor to rotate.
- T-motor 2216 motor, where the motor rotation drives the propeller blades, providing upward power to the drone.
- Battery, the power source of the drone.
- Current meter, a component that supplies a dependable power source to the flight controller while detecting real-time voltage levels. It also takes preset actions for autonomous landing or return when the battery voltage is too low.
- UBEC, providing stable power supply to the Raspberry Pi.
- Receiver: paired with the remote controller, this receives the control signal from the remote controller to control the flight of the drone.
4.2. Matlab Simulation
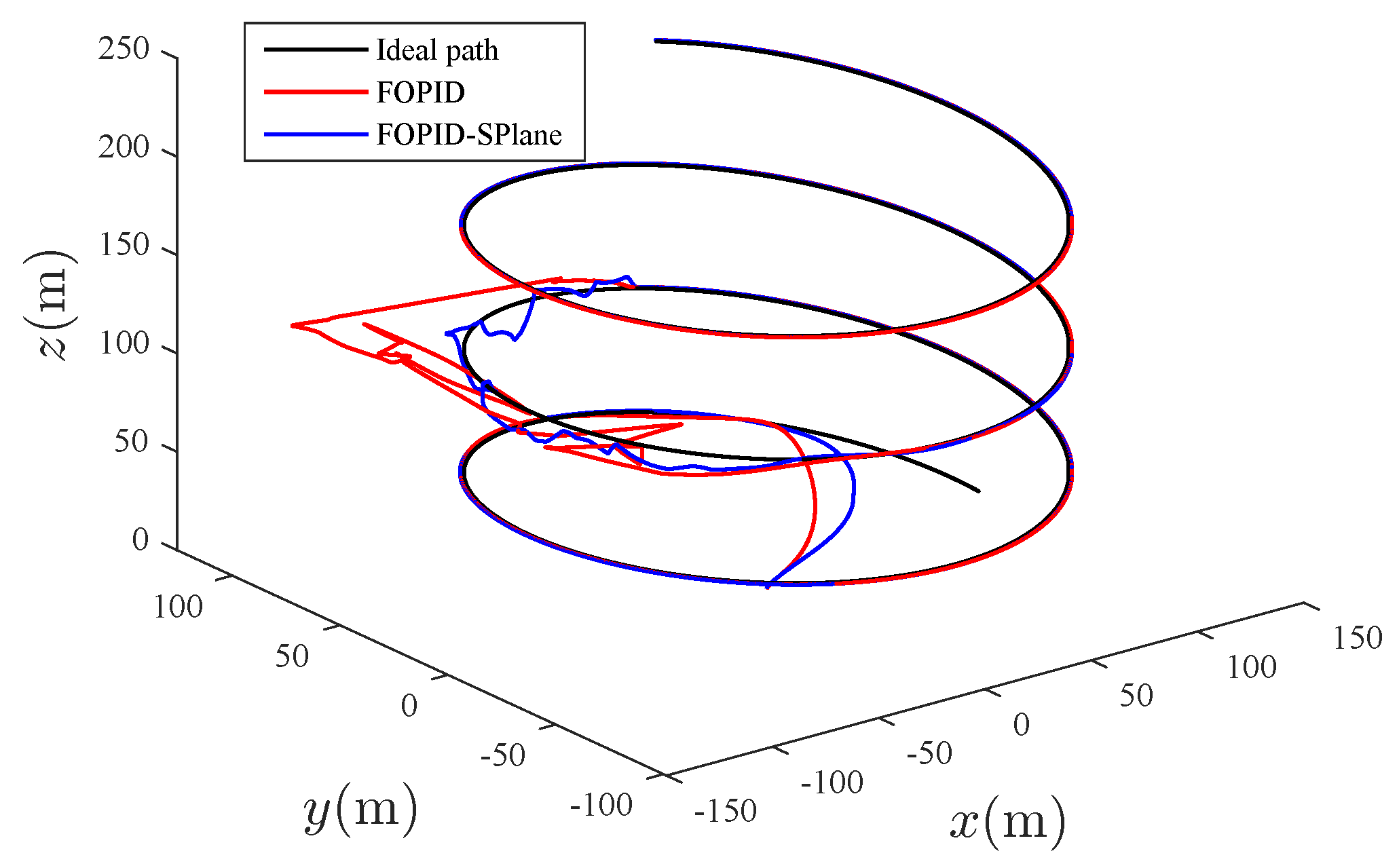
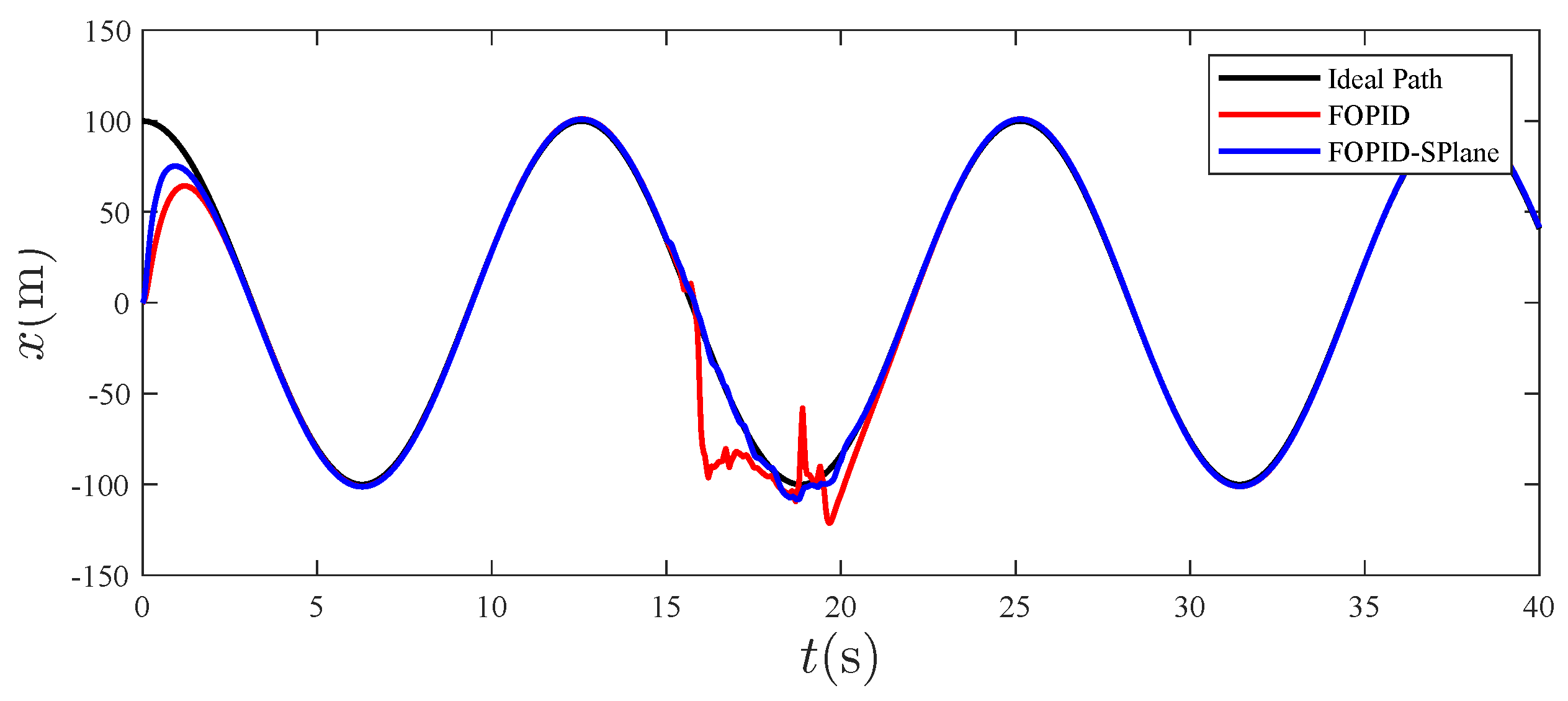
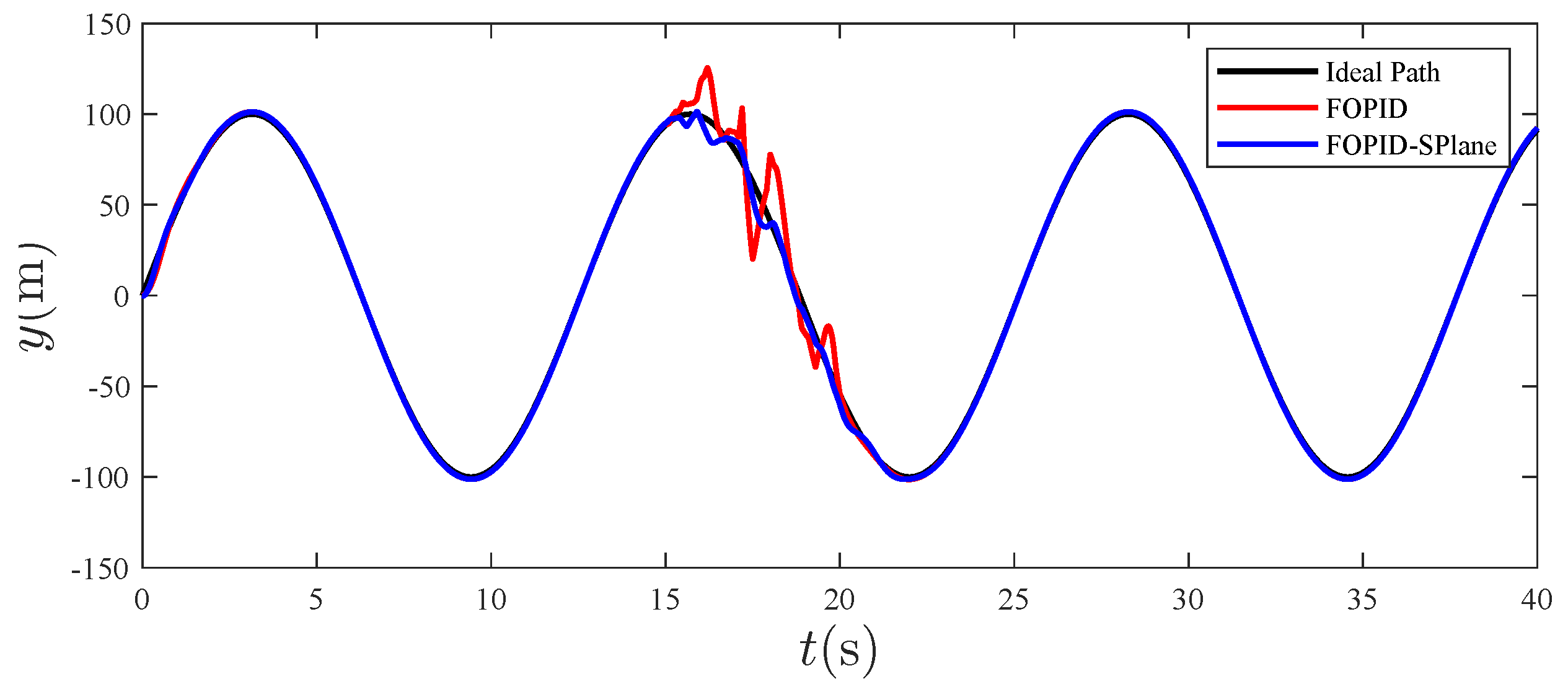
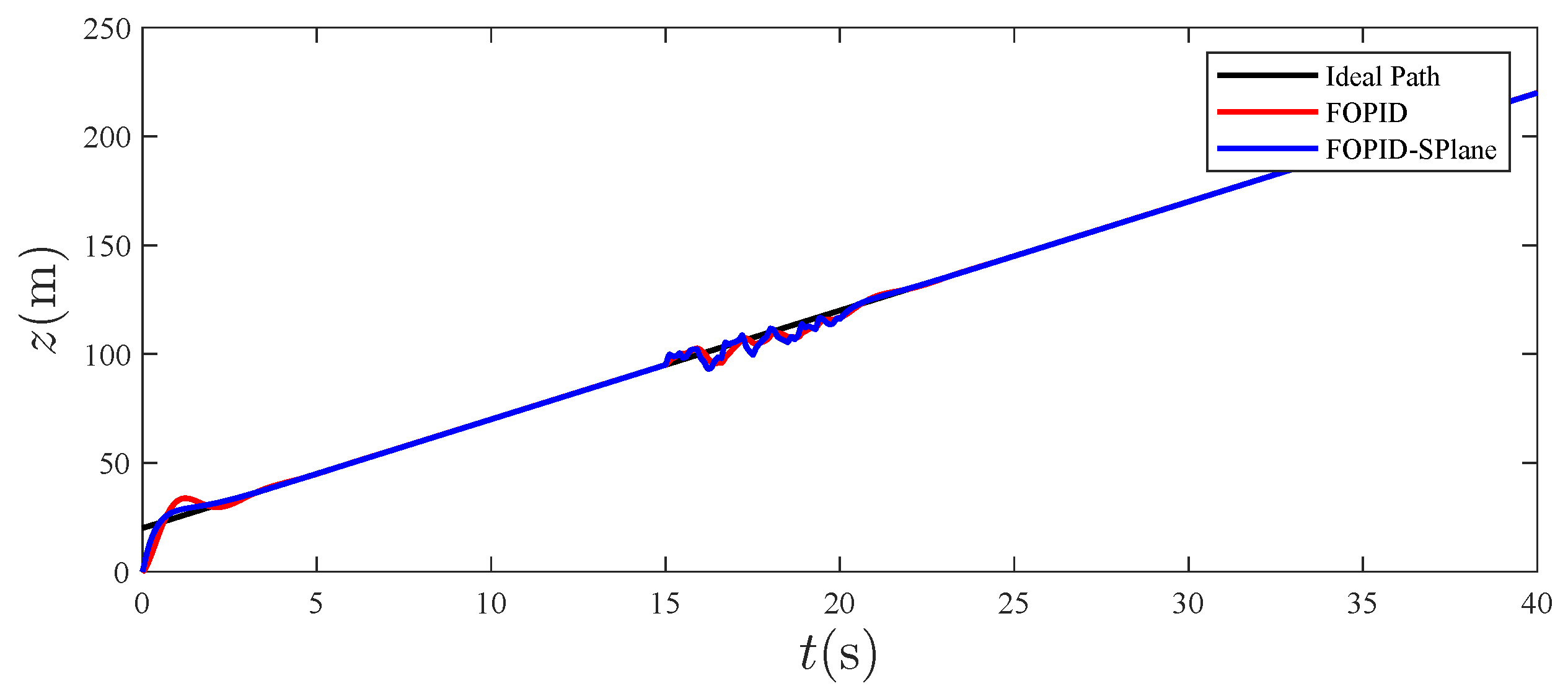
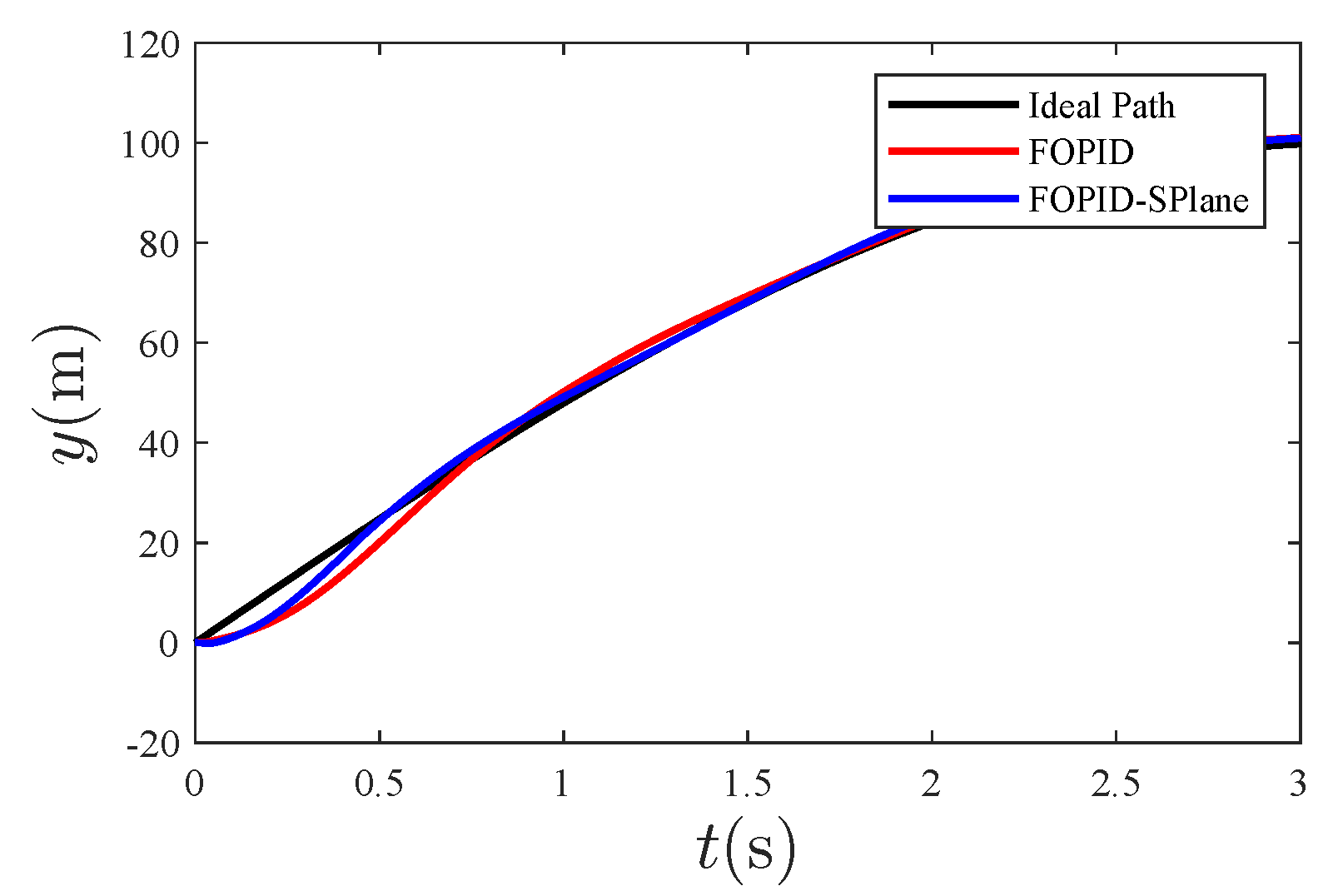
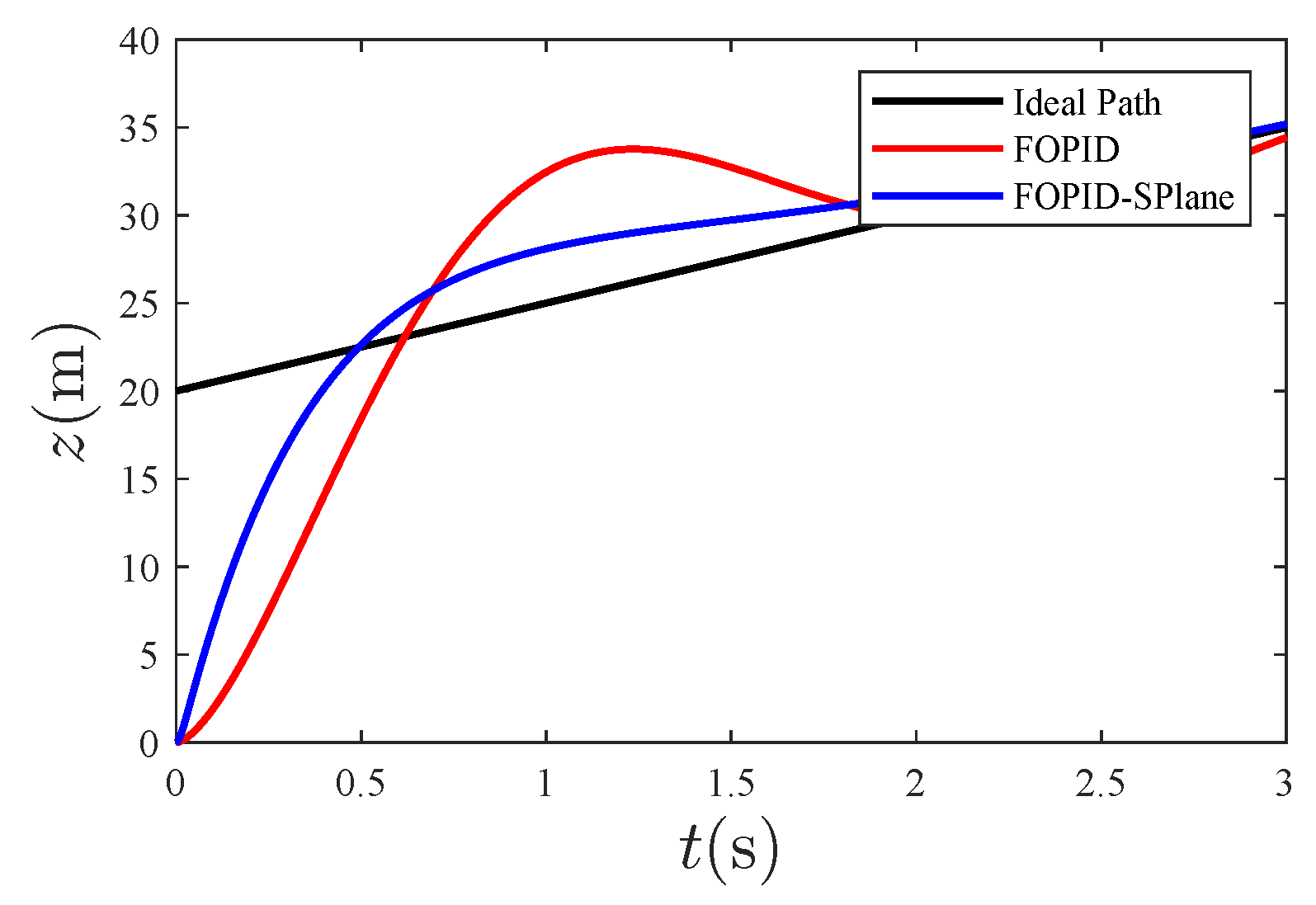
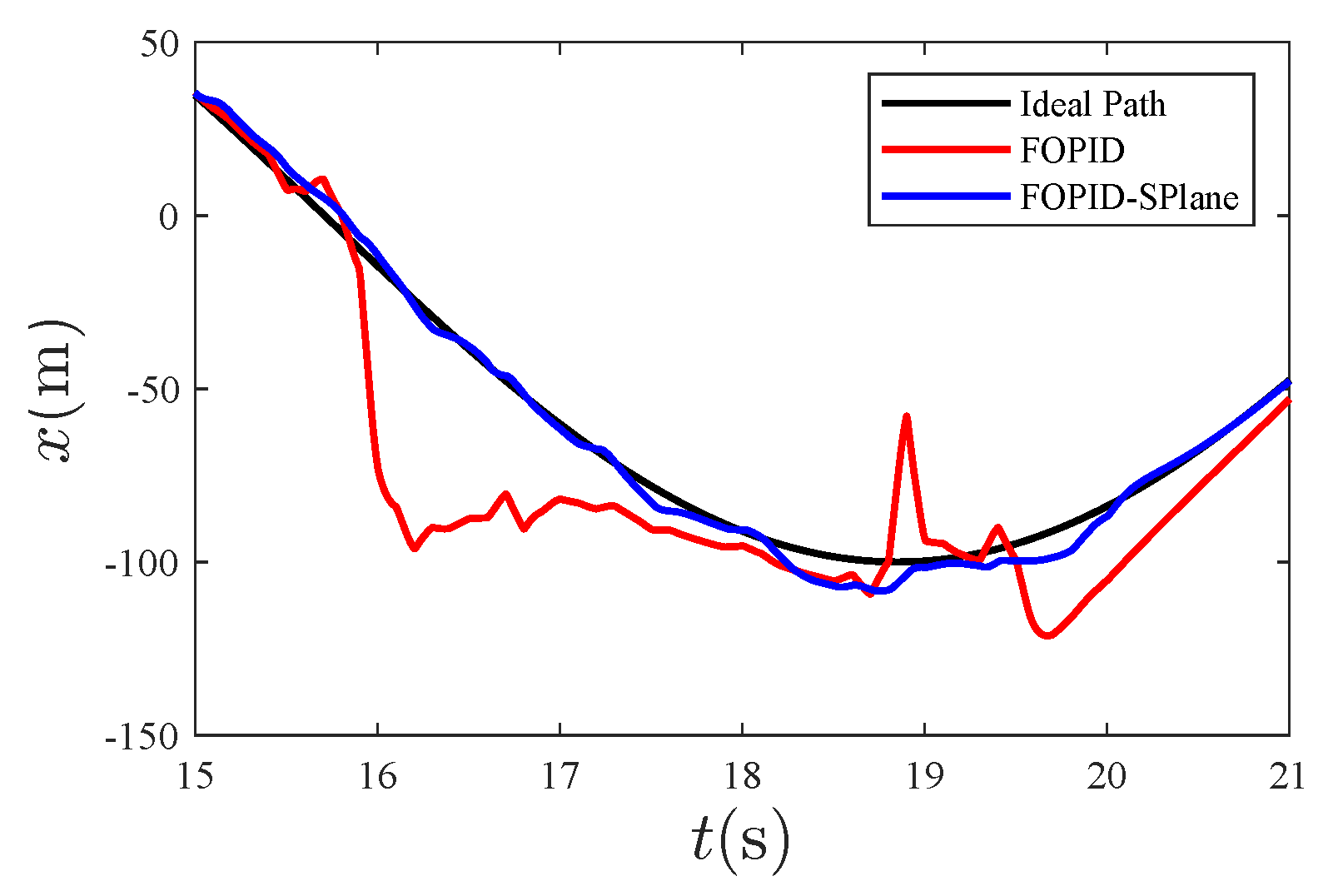
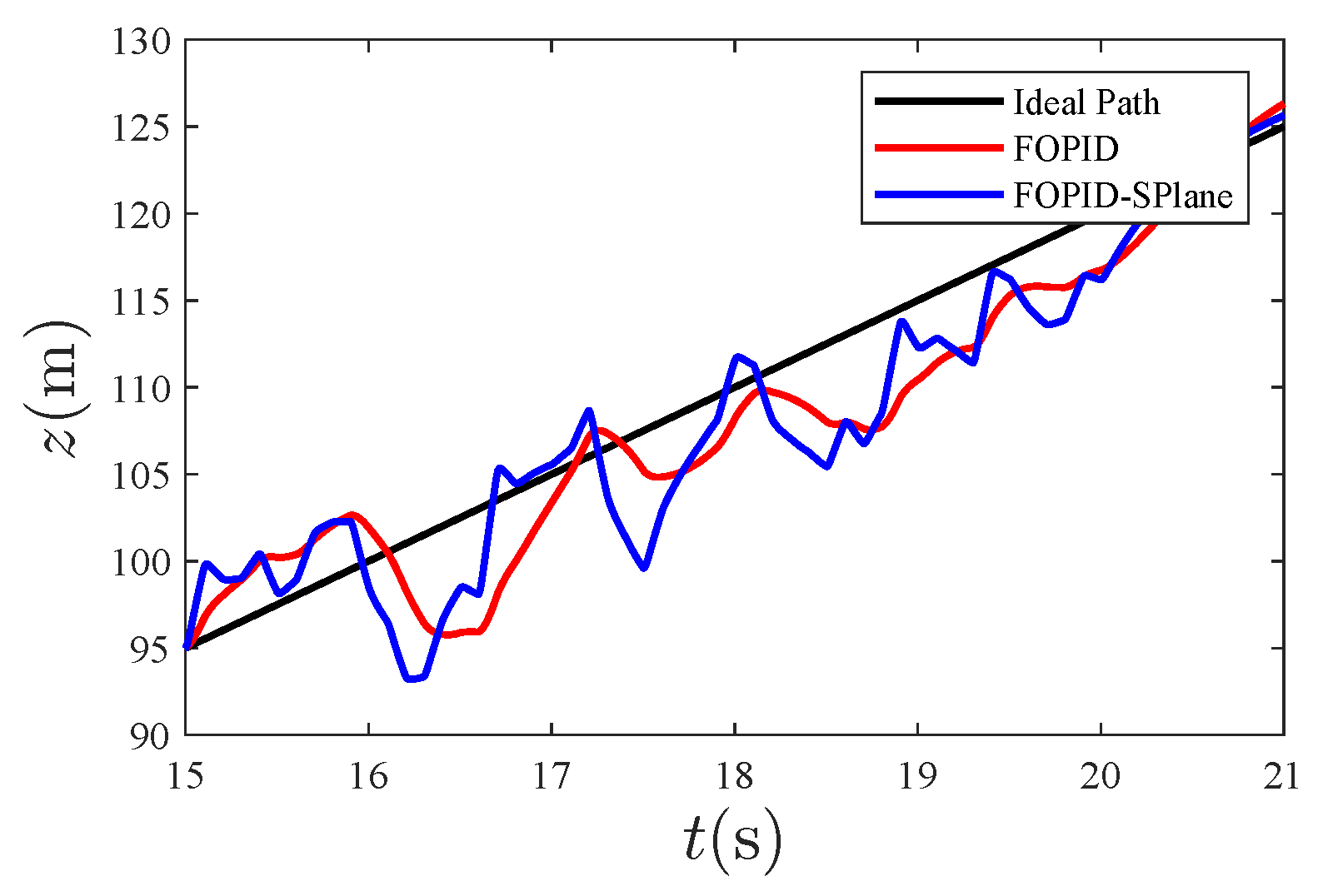
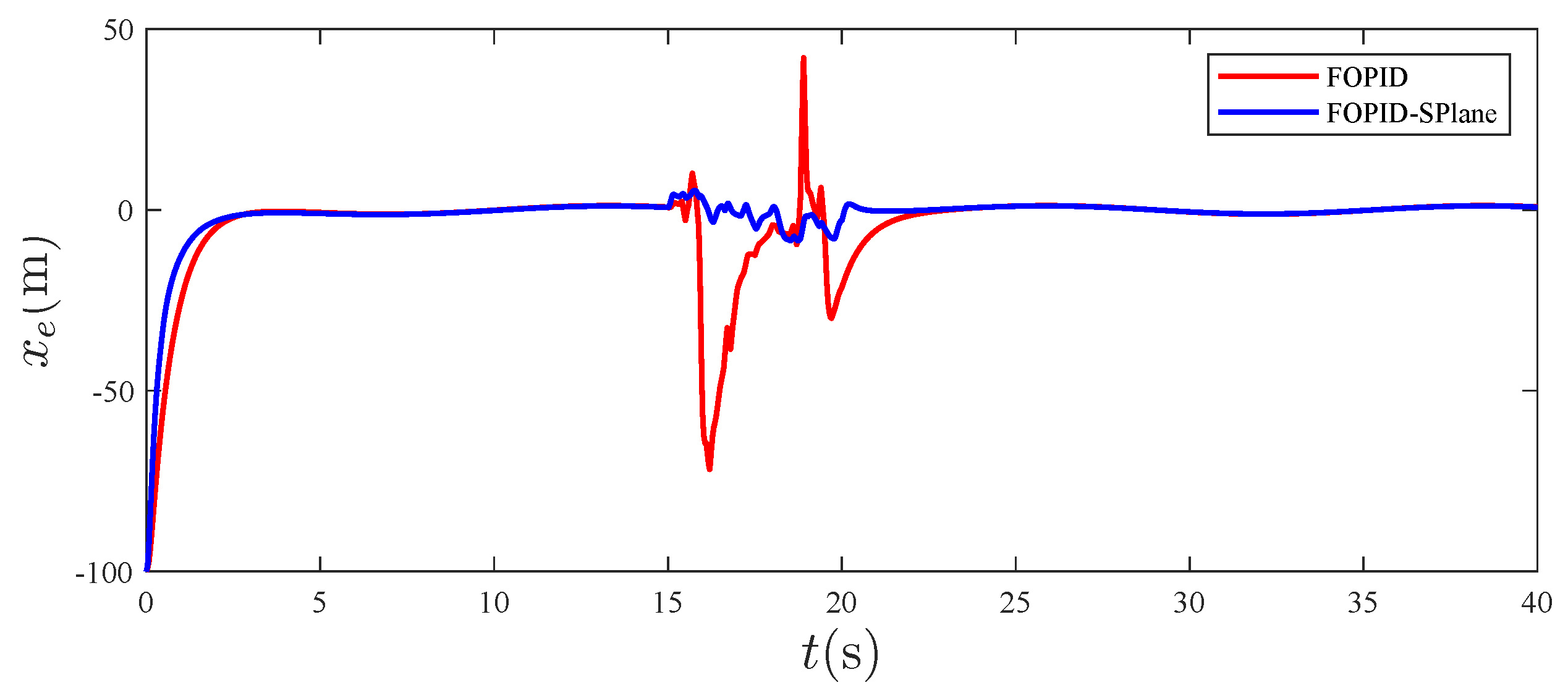
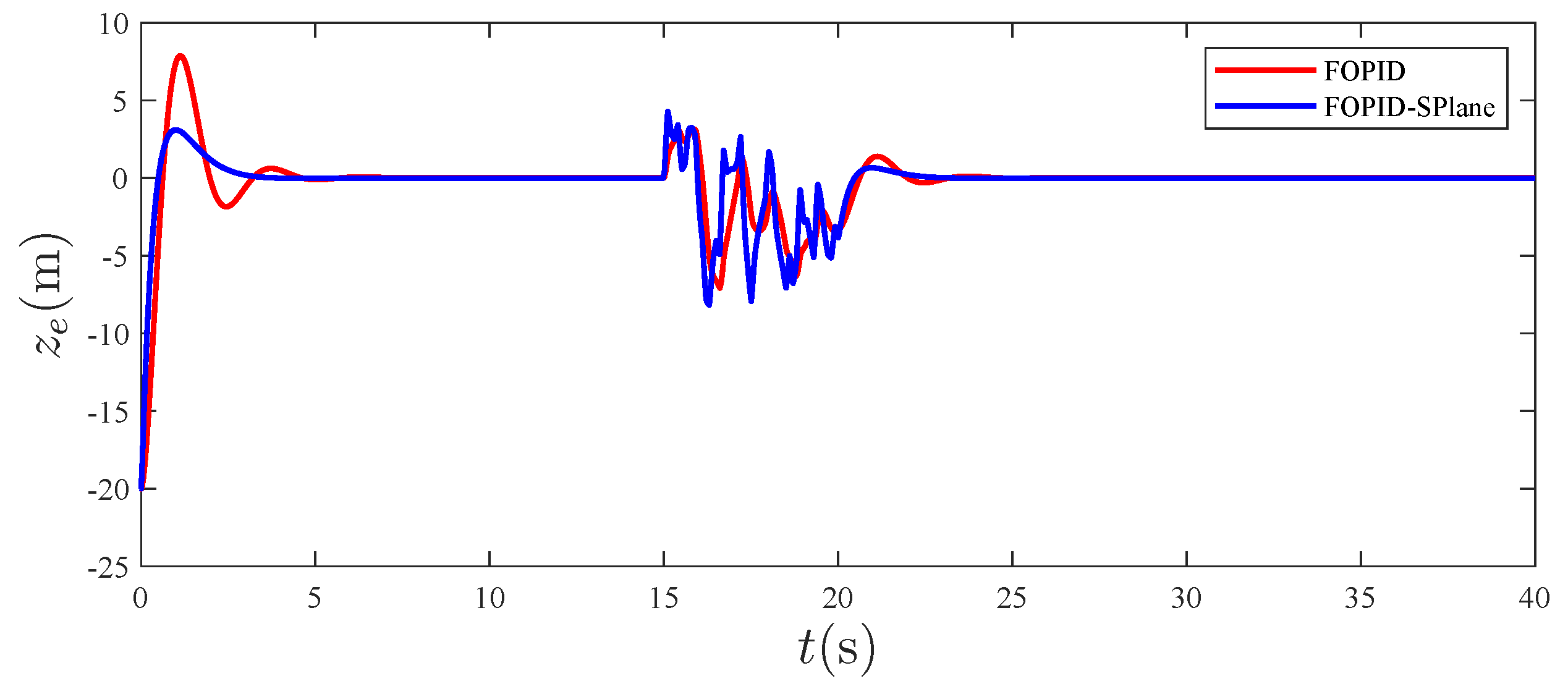
4.3. Results Analysis
5. Conclusions
5.1. Overall Summary
- The quaternion-based control model can effectively avoid singularity problems and facilitate the calculation of attitude angles.
- The designed fractional-order S-surface controller inherits the advantages of conventional PID controllers and can finetune the control system order to make the control process smoother.
- The simulation results show that, compared with fractional-order PID control, the fractional-order S-surface controller can give the control system a higher control accuracy and stronger robustness.
5.2. Further Research
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| FOPID | Fractional order PID |
| SPlane | Sigmoid Plane |
References
- Li, J.; Li, Y. Dynamic analysis and PID control for a quadrotor. In Proceedings of the 2011 IEEE International Conference on Mechatronics and Automation, Beijing, China, 7–10 August 2011; pp. 573–578, ISSN 2152-744X. [Google Scholar] [CrossRef]
- Timis, D.D.; Muresan, C.I.; Dulf, E.H. Design and Experimental Results of an Adaptive Fractional-Order Controller for a Quadrotor. Fractal Fract. 2022, 6, 204. [Google Scholar] [CrossRef]
- Shi, X.; Cheng, Y.; Yin, C.; Dadras, S.; Huang, X. Design of fractional-order backstepping sliding mode control for quadrotor UAV. Asian J. Control 2019, 21, 156–171. [Google Scholar] [CrossRef]
- Han, J. Active Disturbance Rejection Control Technique—The Technique for Estimating and Compensating the Uncertainties; National Defense Industry Press: Beijing, China, 2008. [Google Scholar]
- Wang, C.; Chen, Z.; Sun, Q.; Zhang, Q. Design of PID and ADRC based quadrotor helicopter control system. In Proceedings of the 2016 Chinese Control and Decision Conference (CCDC), Yinchuan, China, 28–30 May 2016; pp. 5860–5865, ISSN 1948-9447. [Google Scholar] [CrossRef]
- Xu, R.; Ozguner, U. Sliding Mode Control of a Quadrotor Helicopter. In Proceedings of the 45th IEEE Conference on Decision and Control, San Diego, CA, USA, 13–15 December 2006; pp. 4957–4962, ISSN 0191-2216. [Google Scholar] [CrossRef]
- Shi, L.; Shen, J.; Wang, Q.; Jiang, J. Fuzzy Active Disturbance Rejection Attitude Control of Quadrotor Aircraft. Electr. Autom. 2021, 50, 157–162. [Google Scholar] [CrossRef]
- Muro, C.; Castillo-Toledo, B.; Loukianov, A.; Luque-Vega, L.; González-Jiménez, L. Quaternion-based trajectory tracking robust control for a quadrotor. In Proceedings of the 10th System of Systems and Engineering Conference, San Antonio, TX, USA, 17–20 May 2015; pp. 386–391. [Google Scholar] [CrossRef]
- Cheng, P.; Zhang, P.; Zhao, X.; Chang, J.; Yuan, M.; Shen, T. Expert S-Plane Control Method for Unmanned Aerial Vehicle. Aerosp. Control 2018, 36, 65–71. [Google Scholar] [CrossRef]
- Mandić, P.; Lazarević, M.; Šekara, T. D-decomposition technique for stabilization of Furuta pendulum: Fractional approach. Bull. Pol. Acad. Sci. Tech. Sci. 2016, 64, 189–196. [Google Scholar] [CrossRef]
- Liu, T. Fractional Order PID Control For a Quadrotor UAV. Master’s Thesis, Wuhan University of Science and Technology, Wuhan, China, 2021. [Google Scholar]
- Harrison, J.V.; Gallagher, J.L.; Grace, E.J. An algorithm providing all-attitude capability for three-gimballed inertial systems. IEEE Trans. Aerosp. Electron. Syst. 1971, AES-7, 532–543. [Google Scholar] [CrossRef]
- Liu, K.; Wang, R. Antisaturation Adaptive Fixed-Time Sliding Mode Controller Design to Achieve Faster Convergence Rate and Its Application. IEEE Trans. Circuits Syst. II Express Briefs 2022, 69, 3555–3559. [Google Scholar] [CrossRef]
- Islam, M.; Okasha, M. A Comparative Study of PD, LQR and MPC on Quadrotor Using Quaternion Approach. In Proceedings of the 7th International Conference on Mechatronics Engineering, Putrajaya, Malaysia, 30–31 October 2019; pp. 1–6. [Google Scholar] [CrossRef]
- Luukkonen, T. Modelling and control of quadcopter. Indep. Res. Proj. Appl. Math. Espoo 2011, 22. [Google Scholar]
- Zhou, Z.; Zhang, Q.; Liu, Q.; Zeng, Q.; Tian, X. Adaptive quaternion particle filter using generalized likelihood ratio test for aircraft attitude estimation in the presence of anomalous measurement. Meas. Sci. Technol. 2021, 32, 045004. [Google Scholar] [CrossRef]
- Figueredo, L.F.C.; Adorno, B.V.; Ishihara, J.Y.; Borges, G.A. Robust kinematic control of manipulator robots using dual quaternion representation. In Proceedings of the 2013 IEEE International Conference on Robotics and Automation, Karlsruhe, Germany, 6–10 May 2013; pp. 1949–1955. [Google Scholar]
- Antonelli, G.; Chiaverini, S.; Sarkar, N.; West, M. Adaptive control of an autonomous underwater vehicle: Experimental results on ODIN. IEEE Trans. Control Syst. Technol. 2001, 9, 756–765. [Google Scholar] [CrossRef]
- Voight, J. Quaternion Algebras; Springer International Publishing: Berlin/Heidelberg, Germany, 2021. [Google Scholar]
- Quan, Q. Introduction to Multicopter Design and Control; Springer: Singapore, 2018. [Google Scholar]
- Cariño, J.; Abaunza, H.; Castillo, P. Quadrotor quaternion control. In Proceedings of the 2015 International Conference on Unmanned Aircraft Systems (ICUAS), Denver, CO, USA, 9–12 June 2015; pp. 825–831. [Google Scholar] [CrossRef]
- Hu, S. Design of Control Method for Nonlinear Underactuated Quadrotor Aircraft; National Defense Industry Press: Beijing, China, 2021. [Google Scholar]
- Liu, K.; Wang, R.; Wang, X.; Wang, X. Anti-saturation adaptive finite-time neural network based fault-tolerant tracking control for a quadrotor UAV with external disturbances. Aerosp. Sci. Technol. 2021, 115, 106790. [Google Scholar] [CrossRef]
- Liu, K.; Wang, R.; Zheng, S.; Dong, S.; Sun, G. Fixed-time disturbance observer-based robust fault-tolerant tracking control for uncertain quadrotor UAV subject to input delay. Nonlinear Dyn. 2022, 107, 2363–2390. [Google Scholar] [CrossRef]
- Wu, G.; Luo, W.; Guo, J.; Zhang, J. A Sigmoid-plane adaptive control algorithm for unmanned surface vessel considering marine environment interference. Trans. Inst. Meas. Control 2022, 44, 2076–2090. [Google Scholar] [CrossRef]
- Li, Y.; Pang, Y.; Wan, L. Adaptive S Plane Control for Autonomous Underwater Vehicle. J. Shanghai Jiaotong Univ. 2012, 46, 195–200+206. [Google Scholar] [CrossRef]
- Chen, P.; Zhang, G.; Guan, T.; Yuan, M.; Shen, J. The Motion Controller Based on Neural Network S-Plane Model for Fixed-Wing UAVs. IEEE Access 2021, 9, 93927–93936. [Google Scholar] [CrossRef]
- Monje, C.A.; Chen, Y.; Vinagre, B.M.; Xue, D.; Feliu-Batlle, V. Fractional-Order Systems and Controls: Fundamentals and Applications; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Podlubny, I. Fractional-order systems and PIλDμ-controllers. IEEE Trans. Autom. Control 1999, 44, 208–214. [Google Scholar] [CrossRef]




| Representation | Symbol | Value | Unit |
|---|---|---|---|
| Mass | m | kg | |
| Acceleration of gravity | g | ||
| Moment of inertia | |||
| Moment of inertia | |||
| Moment of inertia | |||
| Arm length | l | m | |
| Lift coefficent | b | ||
| Torque coefficent | d | ||
| Air resistance coefficent | |||
| Air resistance coefficent | |||
| Air resistance coefficent | |||
| Air resistance moment coefficent | |||
| Air resistance moment coefficent | |||
| Air resistance moment coefficent |
| Methods | ||||
|---|---|---|---|---|
| FOPID | 200.56 | 98.36 | 33.8 | 332.72 |
| FOPID-SPlane | 90.54 | 53.59 | 26.14 | 170.27 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, J.; Chen, P.; Chang, Z.; Zhang, G.; Guo, L.; Zhao, C. Trajectory Tracking Control of Quadrotor Based on Fractional-Order S-Plane Model. Machines 2023, 11, 672. https://doi.org/10.3390/machines11070672
Li J, Chen P, Chang Z, Zhang G, Guo L, Zhao C. Trajectory Tracking Control of Quadrotor Based on Fractional-Order S-Plane Model. Machines. 2023; 11(7):672. https://doi.org/10.3390/machines11070672
Chicago/Turabian StyleLi, Jiacheng, Pengyun Chen, Zhe Chang, Guobing Zhang, Luji Guo, and Chenbo Zhao. 2023. "Trajectory Tracking Control of Quadrotor Based on Fractional-Order S-Plane Model" Machines 11, no. 7: 672. https://doi.org/10.3390/machines11070672
APA StyleLi, J., Chen, P., Chang, Z., Zhang, G., Guo, L., & Zhao, C. (2023). Trajectory Tracking Control of Quadrotor Based on Fractional-Order S-Plane Model. Machines, 11(7), 672. https://doi.org/10.3390/machines11070672








