1. Introduction
Orthogonal polynomial sequences play a fundamental role in mathematics (approximation theory, Fourier series, numerical analysis, special functions, etc.), and also in many other computational and applied sciences, physics, chemistry, engineering, etc. In this paper, we give an account on polynomials that are orthogonal on the radial rays in the complex plane, as well as some new results and examples for such kinds of orthogonal polynomials. We introduced and studied such polynomials in several papers [
1,
2,
3,
4,
5], including an electrostatic interpretation of their zeros in the case of the generalized Gegenbauer polynomials, assuming a logarithmic potential [
6]. Polynomials that are orthogonal on radial rays were mentioned in the book [
7] (p. 111). An extended Dunkl oscillator model based on our generalized Hermite polynomials on radial rays [
1,
2] was discussed very recently by Bouzeffour [
8] (see also [
9]). Also, we mention here the references [
10,
11], which may have some connection to our results.
This paper is organized as follows. In
Section 2, we present some basic facts from the standard theory of orthogonality on the real line, necessary for the development of orthogonality on the radial rays in the complex plane, while in
Section 3, we very briefly mention some classes of orthogonal polynomials in the complex plane. Orthogonal polynomials on the radial rays are presented in
Section 4, including the existence and uniqueness of such polynomials, the general distribution of their zeros and some interesting examples. The numerical construction of this class of orthogonal polynomials is presented in
Section 5. In particular, the cases on finite and infinite radial rays are considered, as well as the cases with Jacobi weight functions on equidistant rays. An approach, called the
discretized Stieltjes–Gautschi procedure, has been developed as the main method for the numerical construction of orthogonal polynomials on arbitrary radial rays and with arbitrary weight functions. The fully symmetric cases of orthogonal polynomials on radial rays and their zero distributions are studied in
Section 6. In particular, the cases with Jacobi and Legendre weight functions on
and generalized Laguerre and Hermite weights on
, as well as their connection with the standard orthogonal polynomials on the real line, are discussed, including differential equations. Finally, in
Section 7, some applications in physics and electrostatics are considered.
2. Orthogonal Polynomials on the Real Line
Orthogonal polynomials on the real line
, related to the inner product
are the most important in applications. Here,
is a positive measure on
, with finite or unbounded support, for which all moments
,
exist and are finite, and
(cf. [
12,
13]). If we work with complex polynomials (polynomials with complex coefficients ), then the second component
in (
1) should be conjugated, i.e.,
.
An interesting class of the measures are those when is an absolutely continuous function. Then, is a weight function, which is non-negative and measurable in Lebesgue’s sense for which all moments exist and .
Because of the property
, the orthogonal polynomials
on
satisfy a three-term recurrence relation of the form
with
and
. The recursion coefficients
and
in (
2) can be expressed over the inner product as
and they depend on the weight function
w (in general, on the measure
). The coefficient
, which is multiplied by
in (
2), can be arbitrary, but usually, it is convenient to take
. In this case, we have
.
Alternatively, these recursion coefficients can be expressed in terms of the Hankel determinants
where
The polynomials
and
, which are orthogonal with respect to the inner product (
1), have only real zeros, are mutually different and are located in the support of the measure
. Moreover, the zeros of two consecutive polynomials,
and
, interlace, i.e.,
where
denotes the zeros of
in increasing order (see [
13], pp. 99–101).
The zeros of the orthogonal polynomials
, in notation
,
, play an important role in the Gauss quadrature formula related to the measure
. Namely, for each
, there exists the
n-point Gauss formula
which is exact for all algebraic polynomials of degree at most
, i.e.,
for each
(
denotes the space of all algebraic polynomials, and
is its subspace of polynomials of degree at most
m). Thus, there is a deep connection between the Gauss quadrature Formula (
3) and the orthogonal polynomial sequence
. The quadrature nodes
,
, are zeros of the polynomial
, i.e., the eigenvalues of the symmetric tridiagonal Jacobi matrix
while the weight coefficients in the quadrature rule (
3) are given by
,
, where
is the first component of the (normalized) eigenvector
, corresponding to the eigenvalue
, with Euclidean norm equal to unity (
).
The Golub–Welsch procedure [
14] is one of standard methods for solving this eigenvalue problem. Thus, the knowledge of the coefficients
and
in the three-term recurrence relation (
2) is of exceptional importance. Unfortunately, only for certain narrow classes of orthogonal polynomials are these coefficients known in the explicit form, including
classical orthogonal polynomials, which can be classified as follows:
The Jacobi polynomials, with the weight on the finite interval ;
The generalized Laguerre polynomials, with the weight on ;
The Hermite polynomials, with the weight function on .
There are several characterizations of the classical orthogonal polynomials (cf. [
15,
16,
17]). Orthogonal polynomials for which the recursion coefficients are not known in explicit form are known as
strongly non-classical polynomials ([
13], p. 159), and they must be constructed numerically, but such a construction is usually an ill-conditioned process. Because of this, the use of strongly non-classical polynomials has long been limited.
Four decades ago, Walter Gautschi developed the so-called
constructive theory of orthogonal polynomials on for numerical generating orthogonal polynomials with respect to an arbitrary measure (see [
18,
19] and the book [
12]). Three approaches for generating recursion coefficients were developed: the
method of modified moments as a generalization of the classical
Chebyshev method of moments, the
discretized Stieltjes–Gautschi procedure, and the
Lanczos algorithm. This constructive theory opened the door for extensive computational work on orthogonal polynomials, many applications, as well as further development of the theory of orthogonality in different directions (
s and
-orthogonality [
20,
21,
22,
23], Sobolev type of orthogonality, multiple orthogonality [
24], etc.).
Recently, however, there has been substantial progress in computer architecture (arithmetic of variable precision), as well as progress in symbolic calculations. These advances enabled the direct generation of recurrence coefficients in the relation (
2), using only the original Chebyshev method of moments, but with an arithmetic of sufficiently high precision, which avoids the ill-conditioning of the numerical process. The corresponding symbolic/variable-precision software for orthogonal polynomials and quadrature formulae is now available: Gautschi’s
Matlab package
SOPQ and our
Mathematica package
OrthogonalPolynomials (see [
25,
26]), which are freely downloadable from the website (Mathematical Institute of the Serbian Academy of Sciences and Arts, Belgrade, Serbia):
http://www.mi.sanu.ac.rs/~gvm/.
4. Orthogonal Polynomials on Radial Rays
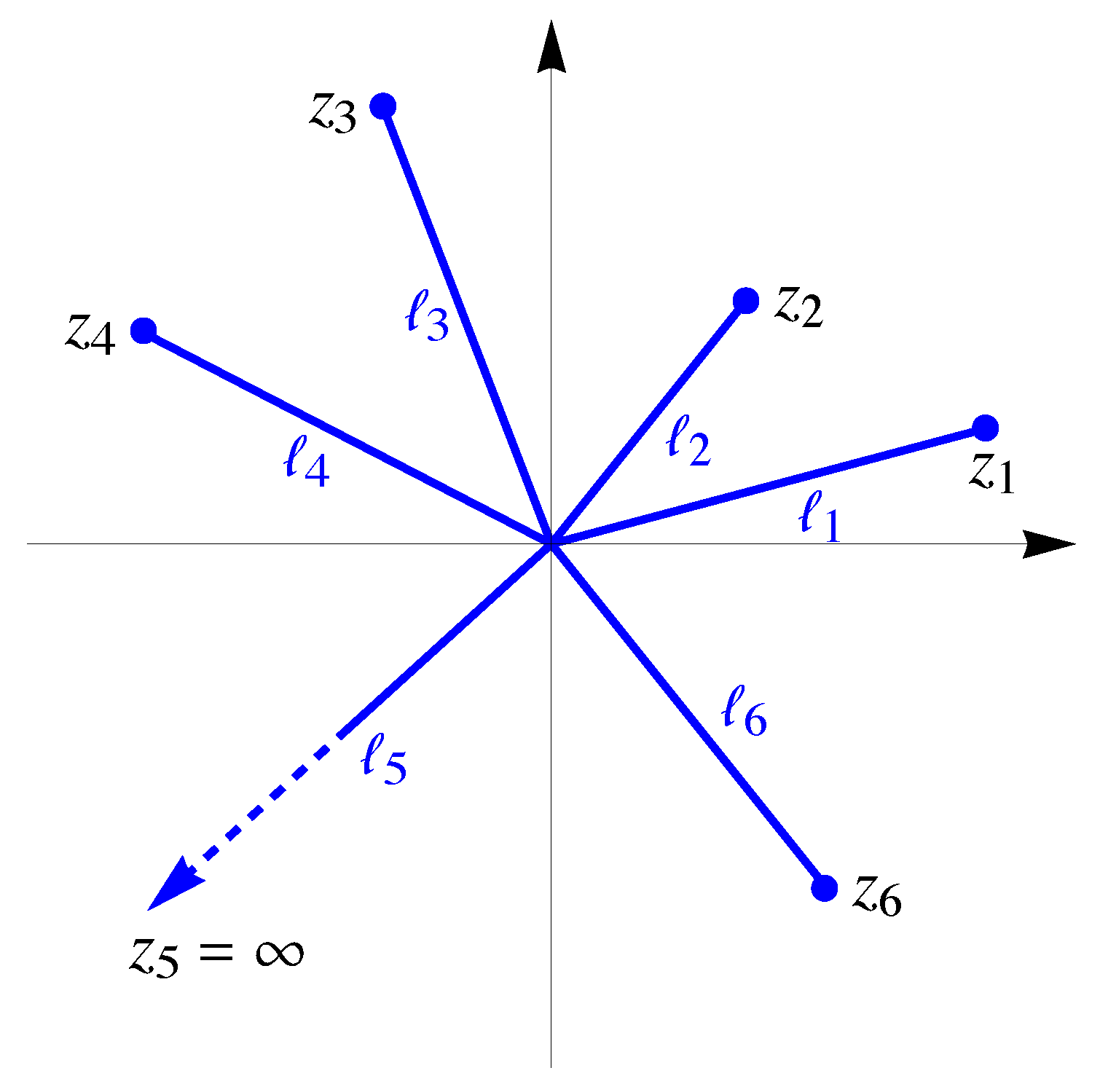
We consider
radial rays
, given by complex points
,
,
,
, with different arguments
,
. Some of
(or all) can be
∞. The case
, with
, is shown in
Figure 1.
We now define an inner product
over all radial rays
, which connects the origin
and the points
,
, in the following way:
where
are suitable complex (weight) functions on the radial rays
, respectively. Here, we suppose that the functions
are weight functions on
, i.e., they are non-negative on
and
. In the case
, it is required that all moments exist and are finite.
As we can see, this inner product (
5) can also be expressed in the form
Remark 1. Without loss of generality, we can assume that .
Remark 2. In a simple case when , and , (6) becomesi.e.,where , , and which means that it reduces to the case of polynomials orthogonal to by the weight functionx . By the characteristic function of a set
L, defined by
and taking
, the inner product (
5) reduces to a standard form
with the measure
4.1. Existence and Uniqueness of Polynomials Orthogonal on the Radial Rays
Consider again the inner product defined by (
6). As we can see,
and
except
. The corresponding moments are given by
Let
denote the single moments (of order
k), which correspond to the weight functions
on the rays
, i.e.,
and then,
Since
for each
, from (
10), we can conclude that
Now, we use the so-called Gram matrix of order
n, constructed by the moments (
9), i.e., (
10),
According to (
11), the matrix
is Hermitian (
) and non-singular, i.e.,
, because the system of functions
is linearly independent. Moreover, the Gram matrix is also positive-definite, which means that the moment determinant
. Formally, we introduce
.
The existence of a sequence of orthogonal polynomials is ensured by the following result:
Theorem 1. For each , the monic polynomials , with respect to the inner product (6), exist uniquely. Their determinant representation is given byas well as the norm of polynomials: Proof. Let us denote the monic polynomial of order
n, from the sequence
, by
and consider the orthogonality conditions
where
is Kronecker’s delta. These conditions give the following system of equations:
Since
, the system (
14) has a unique solution for the coefficients
,
. Notice that the leading coefficient
is given by
, according to (
13).
Similar to the proof for orthonormal polynomials (cf. [
13], Thm. 2.1.1), we prove the equality (
12) for monic polynomials, which are orthogonal with respect to the inner product (
6). □
Now, we prove an important extremal property of polynomials orthogonal on the radial rays. With
, we denote the subspace of all monic polynomials of degree
n and consider the following extremal problem:
Theorem 2. For each , we havewhere is the sequence of monic orthogonal polynomials on M radial rays with respect to the inner product (6). Proof. Let
. Then,
can be expressed in the form
where
,
, and
. Since
we obtain (
16), with equality if and only if
. □
According to (
15) and (
16), we conclude that the best
-approximation of the monomial
, with respect to the norm
, in the space of polynomials of lower degree
, i.e.,
is given by
. The problem of
-approximation of functions will not be treated in this paper.
4.2. General Distribution of Zeros of Orthogonal Polynomials on the Radial Rays
Let
be monic polynomials orthogonal with respect to the inner product
, where the measure is given by (
8), i.e.,
Let
be the smallest convex set containing
A, known as the
convex hull of a set
, and let the support of the measure
be denoted by
. Since
, using a result of Fejér, we can state the following theorems (cf. Saff [
46,
47]).
Theorem 3. All the zeros of the orthogonal polynomial lie in the convex hull of the rays .
Furthermore, an improvement can be also performed.
Theorem 4. If the support of the measure, , is not a line segment, then all the zeros of the polynomial are in the interior of .
4.3. Some Examples
Using the previous “determinant approach”, here, we give a few simple examples of polynomials orthogonal on the radial rays, including the distribution of their zeros.
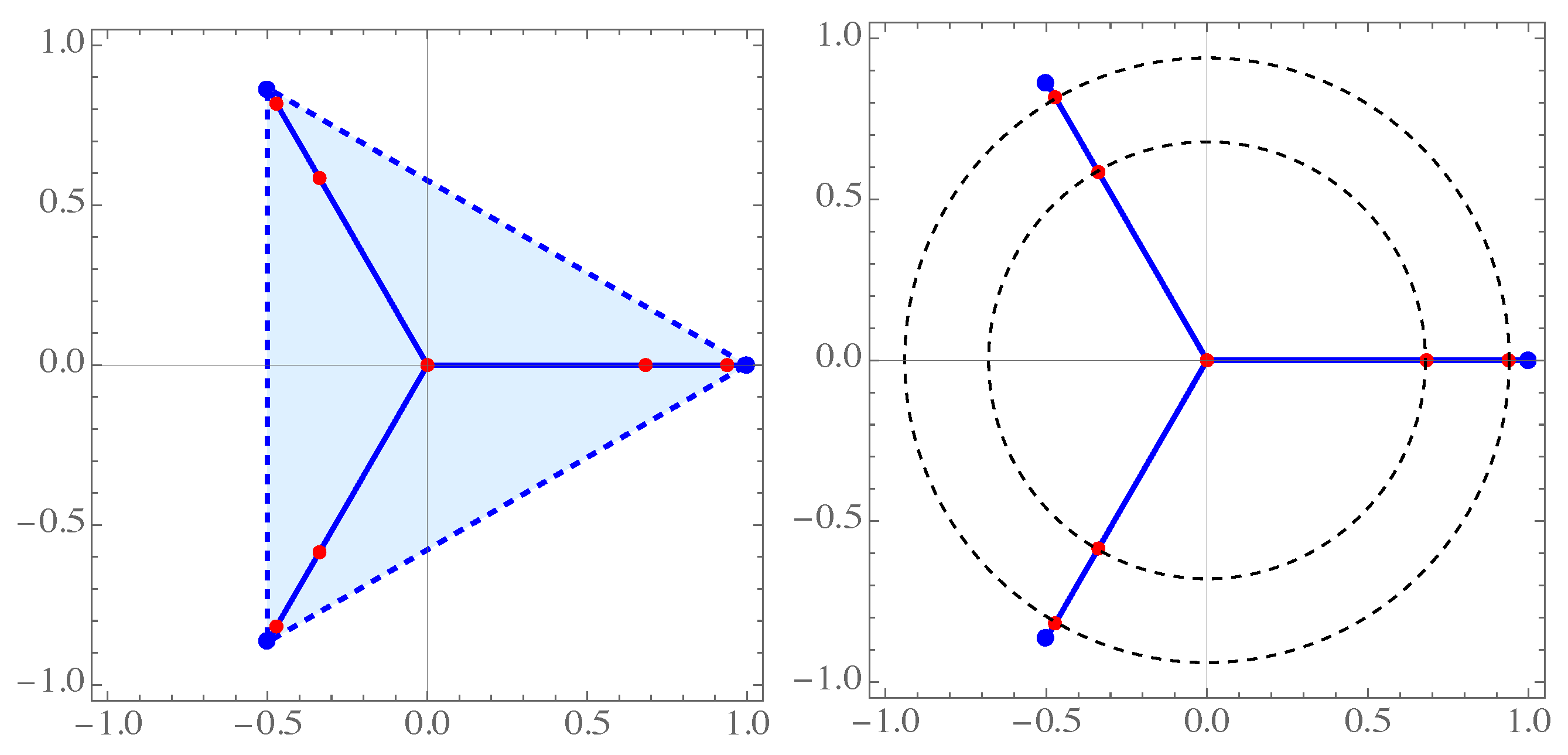
Example 1. We consider the case with thee unit rays (, , ), with , , , and the Legendre weight on the rays , (see Figure 2). The moments (
10)
areso that, for example, for , we obtain the symmetric matrixThe determinants areas well as the corresponding orthogonal polynomials, with respect to the inner product (
12):
Zeros of the polynomial are presented in Figure 3. According to Theorem 4, these zeros lie in the convex hull of the rays (see Figure 3, left). Notice that , so that we can calculate its zeros in the explicit form. Indeed, (double zero), and other six zeros are solutions of the equationswhere and . Thus,As we can see, all zeros also lie on the concentric circles with radii and . This property will be analyzed in the sequel. Example 2. (i)
An interesting inner product iswith the same (Legendre) weight function on all the rays. It is a case with four unit rays (), equidistantly distributed: , , . Since , the moments (
10)
arei.e.,so that, e.g., for , we haveAs we can see, this matrix is symmetric. The corresponding determinants areso that the orthogonal polynomials with respect to the inner product (
17)
areetc. In the sequel, in Remark 5, we present a simple way for calculating this sequence of orthogonal polynomials. These polynomials are discussed in detail in [
2].
Their zeros are simple and located symmetrically on the radial rays, with the possible exception a zero of order at the origin . (ii)
Similarly, introducing an inner product with the weight function , instead of Legendre’s as in (
17)
, we haveIn the same way, after much calculation, we obtain the corresponding orthogonal polynomials:etc.Example 3. We consider the case with four unit rays (, , ), with , , , and the Legendre weight on the rays , .
Since , the moments (
10)
areFor example, for , we havewhile for , we can calculateso thatetc., with norms, according to (
13),
The zeros of polynomials for are presented in Figure 4 and Figure 5. Example 4. Consider now a case with three rays , given by , , with , , and the weight functions on the rays and .
Since the inner product is defined bythe moments areso that the corresponding orthogonal polynomials arewhere . These three rays and zeros of polynomials , where , are presented in Figure 6. 5. Numerical Construction of Orthogonal Polynomials Related to the Inner Product (6)
In this section, we describe a much better and simpler numerical method for constructing orthogonal polynomials on arbitrary radial rays.
Let
be monic orthogonal polynomials related to the inner product (
6), i.e., (
7). Since
and the polynomial
is of degree at most
, then for each
, it can be expressed in the form
i.e.,
where
are some constants. If, for a fixed
, we introduce two
n-dimensional vectors
as well as the following lower (unreduced)
n-order Hessenberg matrix:
then the system of equalities (
20) can be expressed in the matrix form
Now, we state an important result on the determinant form of the monic orthogonal polynomials and their zeros.
Theorem 5. The monic orthogonal polynomials can be expressed in the following determinant form:where is the identity matrix. Moreover, , , are zeros of this polynomial if and only if they are eigenvalues of the Hessenberg matrix . Proof. Let
,
, be zeros of the polynomial
. Taking
z in (
21) to be any of
, then the matrix relation (
21) reduces to the eigenvalue problem
for the the Hessenberg matrix
, where
,
…,
are eigenvalues of the matrix
, and
,
…,
are the corresponding eigenvectors.
From (
21), i.e.,
, we conclude that
,
, are zeros of the polynomial
if and only if they are the eigenvalues of the matrix
. Evidently, (
22) is true. □
For computing zeros of as the eigenvalues of the square matrix m), we use the Mathematica command Eigenvalues[m].
Using the relation (
19) and orthogonality of the polynomials
from
for
, we obtain
Now, we have the problem of how to numerically compute these coefficients.
5.1. Case When All Are Finite
The inner product (
6), in this case, can be transformed to
M integrals over
, with respect to the weight functions
,
. Namely,
Since we have
M integrals with the weight functions
,
, it is enough to apply the
n-point quadrature rules of Gaussian type, with respect to the weight functions (i.e., with respect to the measures
,
),
where
and
,
, are the corresponding nodes and weight coefficients of these quadratures (see (
3)).
The construction of these quadrature parameters (with respect to the weight functions
) can be performed by using our
Mathematica package
OrthogonalPolynomials (see [
25,
26]). Since each of quadratures in (
25) has the maximal degree of precision
, i.e.,
for each
, we conclude that the inner product (
24) can be calculated exactly as
Since for each
and
, the maximal degree of polynomials in the inner product
in the numerator of (
23) is
it is absolutely sufficient to take
n nodes in the quadrature formulas (
25).
Thus, in this way, all the elements of the Hessenberg matrix can be accurately computed, except for rounding errors.
5.2. Cases When Some of (or All) Are Infinity
In these cases, we should take the corresponding
n-point quadrature rules of Gaussian type over
. For example, in the case considered in Example 4, for the first integral in the inner product (
18), we can use the one-sided Gauss–Hermite quadrature formula:
where
and
,
, are the corresponding nodes and weight coefficients. Since the moments for this weight function are
,
, the recurrence coefficients in the relation (
2) (i.e., in the Jacobi matrix (
4)), as well as the quadrature parameters in (
27), can be calculated by our
Mathematica package
OrthogonalPolynomials (see [
26], p. 176). Therefore, only the knowledge of the moments of the weight function is required.
5.3. Discretized Stieltjes–Gautschi Procedure
The main problem is how to numerically calculate the elements of the Hessenberg matrix
in an efficient way. Our proposal to solve this problem is to use a kind of Stieltjes procedure, which we call the
discretized Stieltjes–Gautschi procedure ([
13], pp. 162–166). Namely, we apply Formula (
23) for recurrence coefficients
, with the inner products in a discretized form, in tandem with the basic linear relations (
19), i.e., (
20).
Since
, we can compute
from (
23). Having obtained
, we then use the first relation in (
20) to compute
for all
to obtain its values needed to reapply (
23) with
. This yields
and
, which in turn can be used in (
20) to obtain the corresponding values of
needed to return to (
23) for computing
,
and
. Thus, in this way, alternating between (
23) and (
20), we can ‘bootstrap’ ourselves up to any desired order
n.
In a numerical implementation of the previous procedure, it is very convenient to use Wolfram (Mathematica) or Matlab.
As we have seen, a good way of discretizing the original measures on the radial rays can be obtained by applying suitable quadrature formulae to the corresponding integrals like (
26) or (
27).
In the general (asymmetric) cases, we have to use the discretized Stieltjes–Gautschi procedure as a basic method in numerical construction.
5.4. Jacobi Weight Functions on the Equidistant Rays
We now consider an important case with
M equidistant points on the unit circle in the complex plane,
,
, but with different Jacobi weight functions on the rays, when
where
, with
,
.
In this case, we can successfully apply the discretized Stieltjes–Gautschi procedure, with discretization using Gauss–Jacobi quadratures, to construct the corresponding orthogonal polynomials on the radial rays. Namely, for
,
so that the weights of the integrals in (
28) reduce to the Jacobi weights on
. For calculating these integrals (
28) on
, we simply apply the standard Gauss–Jacobi quadrature formula:
where
and
,
, are nodes and weights of the
n-point Gauss–Jacobi quadrature formula, with respect to the Jacobi weight
,
. These parameters are connected with the symmetric tridiagonal Jacobi matrix (
4), via the Golub–Welsch algorithm [
14], which is realized in our software
OrthogonalPolynomials (see [
25,
26]) as
<< orthogonalPolynomials‘
{nodes, weights} =
aGaussianNodesWeights[n,{aJacobi,alpha,beta},
WorkingPrecision -> WP,Precision ->PR];
thereby giving, e.g., WorkingPrecision->40 and requiring Precision->30, if we need parameters with the relative errors about .
Below, we give a few examples.
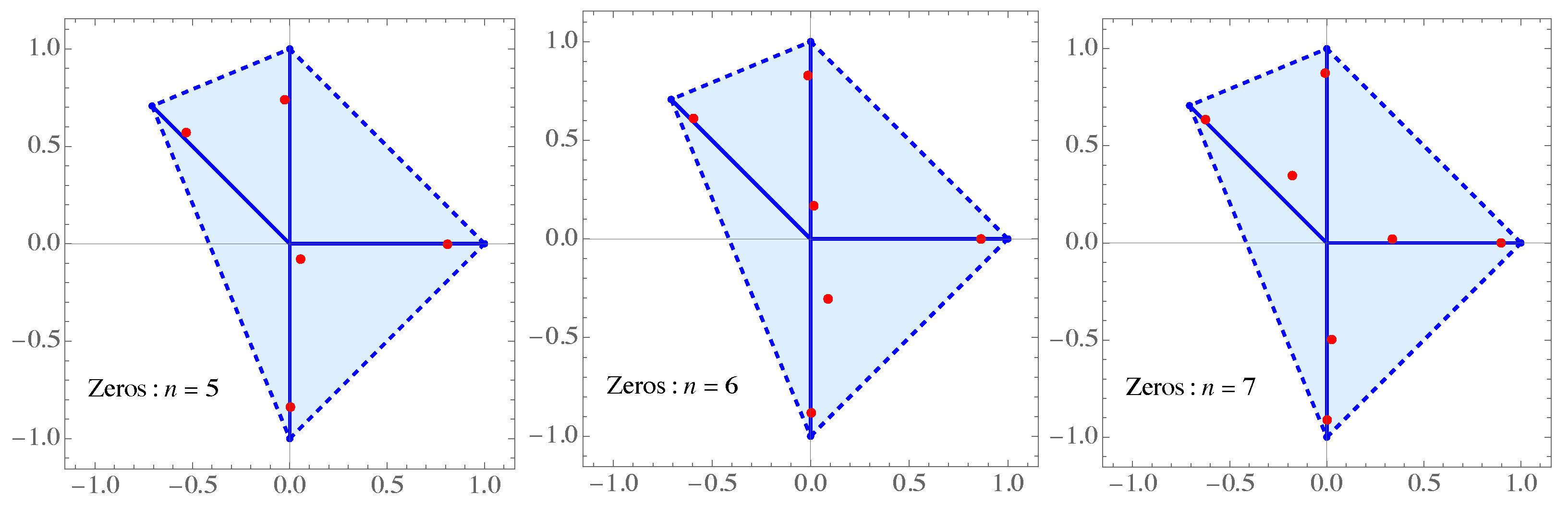
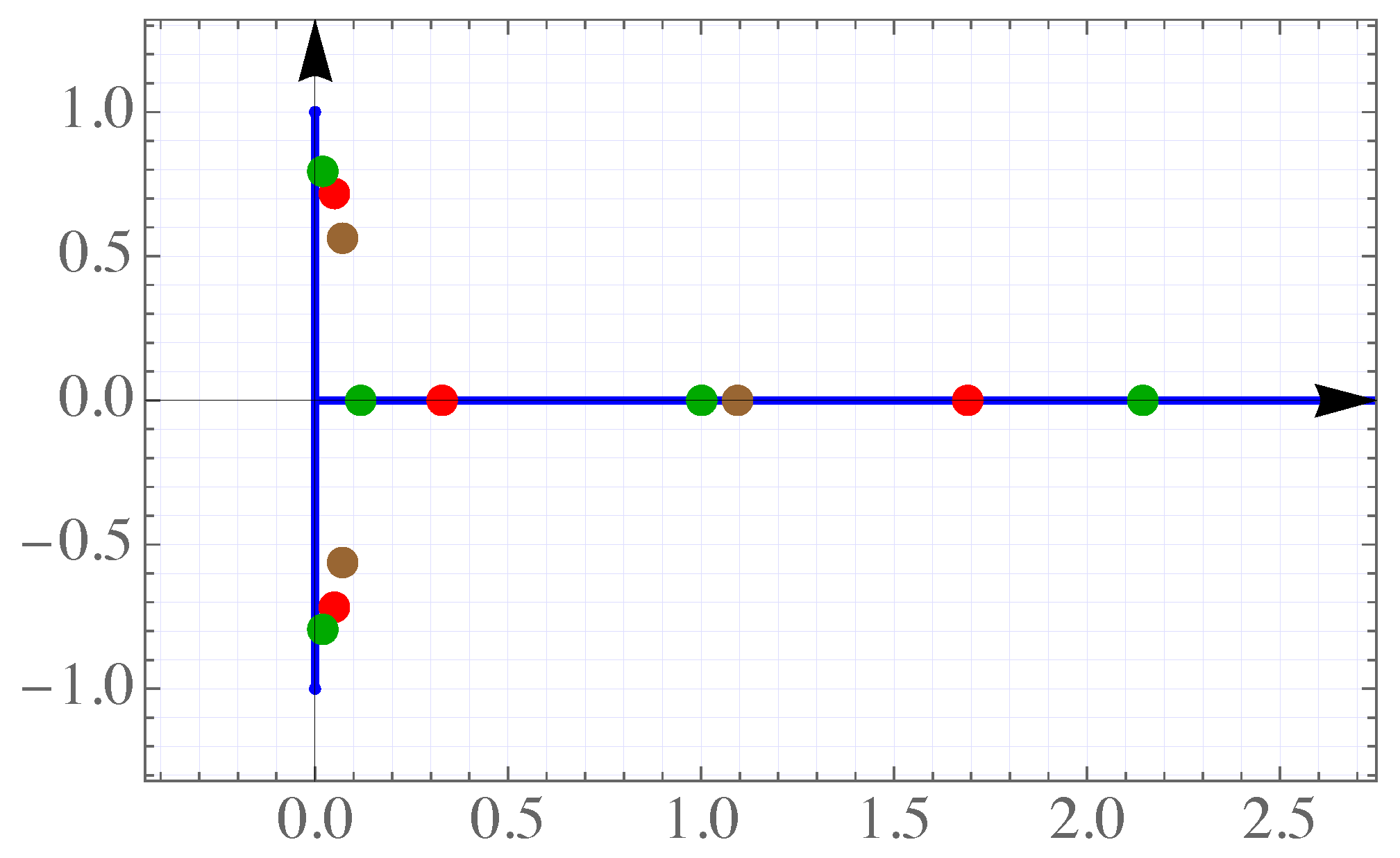
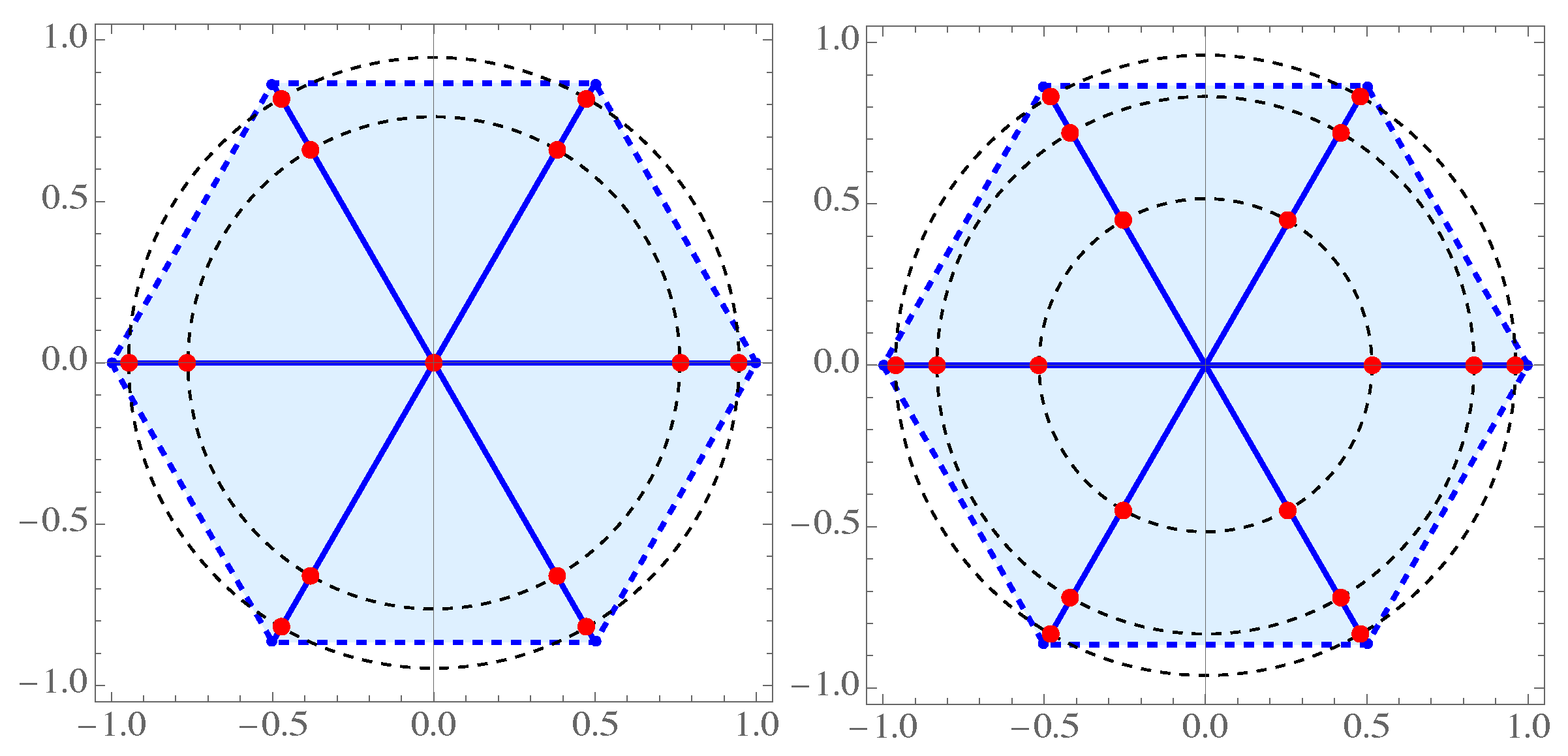
Example 5. Let M be the number of unit rays and n be the degree of the orthogonal polynomial related to the inner product (28) with the same weight function on all rays. In this example we consider two cases. (i) Chebyshev case of the first kind, when , with rays. Using the discretized Stieltjes–Gautschi procedure, we obtain the corresponding polynomials:etc. In Figure 7, we present zeros of and . In the first case, ten zeros are on the five rays while two concentric circles and one double zero is at the origin, whereas in the second case, all twenty zeros lie on the five rays and the four concentric circles (see Theorem 9 in the sequel). (ii) Chebyshev case of the second kind , with rays.
As in (i),
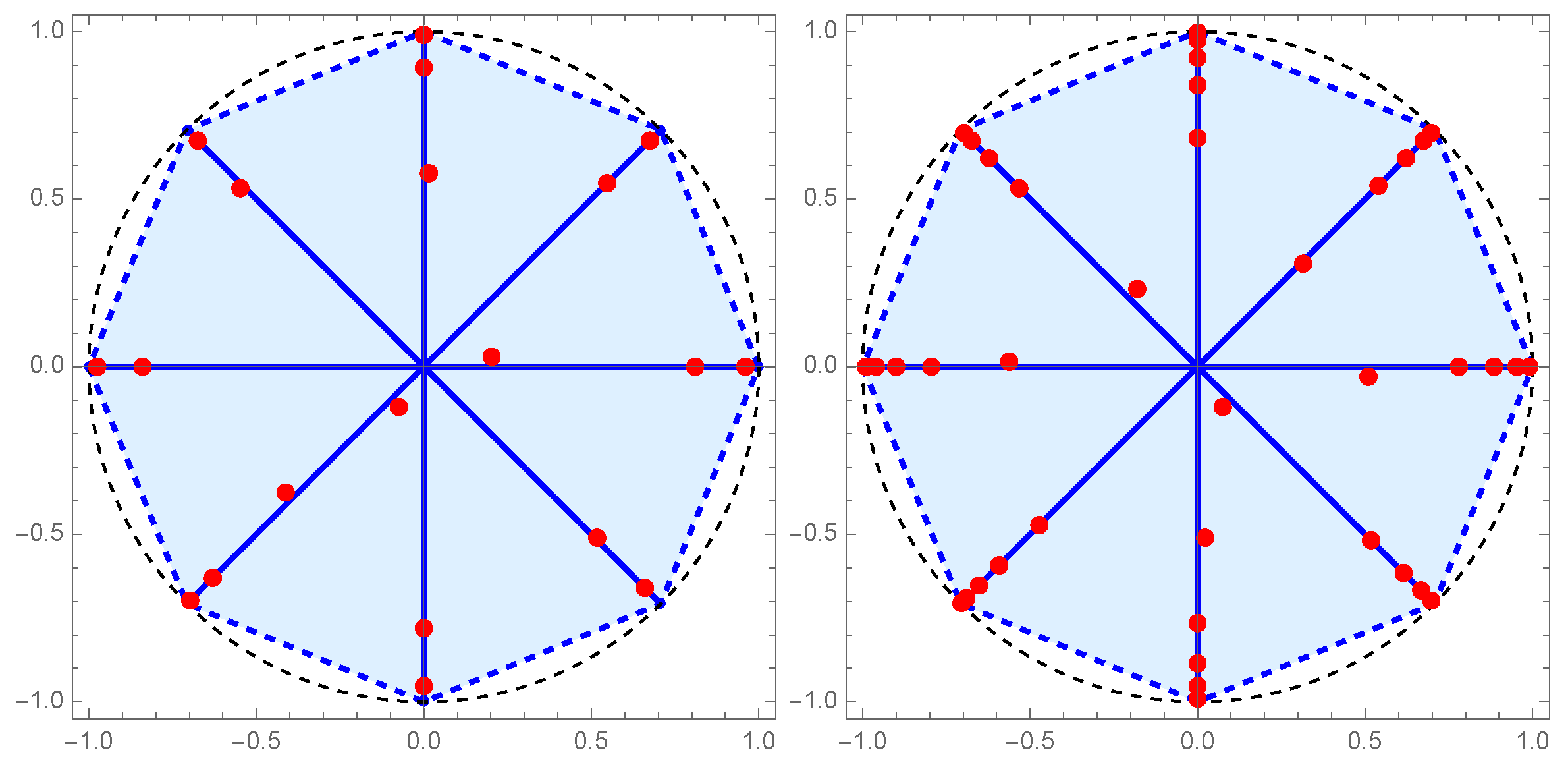
we obtain the following polynomials:etc. In Figure 8, we present zeros of and . Figure 8 (left) shows twelve zeros on six rays, and two concentric circles and one triple zero at the origin, while the Figure 8 (right) displays eighteen zeros on three concentric circles and six rays. Example 6. Here, we consider eight rays () and the inner product (28), with eight different Jacobi weights on :Using the discretized Stieltjes–Gautschi procedure, we calculate , so that we are now able to construct all polynomials for and determine their zeros by solving the eigenvalue problem for the Hessenberg matrix . To save space, we now only mention the matrix , whose elements are given with only a few decimal digits:The first five orthogonal polynomials are The zeros of the polynomials , , and are presented in Figure 9 and Figure 10. We notice that as the degree of the orthogonal polynomial increases, we have a buildup of zeros towards the ends of the radial rays. 6. Symmetric Cases of Orthogonal Polynomials on the Radial Rays
In some special cases, it is possible to find the moment determinants in an explicit form. Then, we can obtain the corresponding orthogonal polynomials, as well as some other properties of these orthogonal polynomials, including recurrence relations, zero distribution, or even an electrostatic interpretation of their zeros (see [
6]).
6.1. Symmetrical Case of Equal Rays, Equidistantly Spaced and with the Same Weight Function
Consider the symmetric case with
,
,
, with the same weight function on the rays
Some of such cases are presented in Examples 1 and 2, with Legendre weight on , except Example 2 (ii), where we used on .
The inner product, in this case, becomes
and the moments are given by (see also (
9))
Note that
and
. As in [
2], we obtain that
as well as the following result (see [
3]).
Theorem 6. The monic orthogonal polynomials , related to the inner product (
30)
, satisfy the recurrence relationwith , , and for . The recursion coefficients in (
32)
are given by In the case of an even number of rays
, the previous result can be simplified (see [
2]). Notice that, in that case, for the inner product, we have
.
Theorem 7. Let in the inner product (
30).
The monic polynomials satisfy the recurrence relationwith for . The coefficient in (
33)
is given by Because for , the coefficients are arbitrary for , and we can take, e.g., for .
Remark 3. There is a connection between the recurrence relations (
33)
and (
32).
Namely, using (
33)
, one can obtain the recurrence relationi.e., (
32)
, where and We return again to the general case. For
, where
and
, we see that (
32) reduces to
so that, for
and
, we have
etc. We can conclude that the monic polynomials
can be expressed in the following form, with the real coefficients:
where
and
. Thus, we have the following representation:
where
,
, are monic polynomials of the degree
k. Putting (
35) in (
34), we obtain
i.e., the following result (see [
3]).
Theorem 8. For each , the monic polynomials satisfy the three-term recurrence relationswith , , where and for , , and . Moreover, these polynomials are orthogonal on with respect to the weight functionwhere is the weight function in the inner product (
30).
The second part of this theorem can be proven by using the equality
obtained by (
30) and (
35) and the change in variables
. Defining
we conclude that
. As we mentioned in
Section 2, the coefficient
can be arbitrary, but it is convenient to take
so that we have
Notice that
and
, as well as
If we write the recurrence relation (
36) with the index
j instead of
k, the corresponding recurrence relation for orthonormal polynomials
is
Taking
in this relation, for a fixed
, we obtain the following matrix relation:
where
and
is the symmetric tridiagonal Jacobi matrix given by
It is clear that if and only if , i.e., the zeros of are the same as the eigenvalues of the Jacobi matrix . Also, , where is the identity matrix of order k.
6.2. Zero Distribution
The following theorem gives the zero distribution of the polynomials .
Theorem 9. All zeros of the orthogonal polynomials , , related to the inner product (
30)
, are simple and lie on the radial rays, with the possible exception of a multiple zero at the origin of the order ν if . Let
,
, denote the zeros of the polynomial
, defined in (
35), in an increasing order, i.e.,
These zeros can be easily calculated using the Golub–Welsch algorithm [
14], implemented in our
Mathematica package
OrthogonalPolynomials (see [
25,
26]).
Then, each zero
generates
M zeros
of the polynomial
. On each ray, we have
If , where and , then there exists one zero of of order at the origin .
6.3. Legendre Weight on
Let
be a Jacobi weight on the interval
, where
. Suppose that this weight is on all rays, i.e., the inner product (
30), in the form
Then, the moments (39) become
i.e.,
and
in other cases. In fact, this is a special case of the general one considered earlier in
Section 5.4. Here, we study Legendre’s case (
) in particular, for which we can obtain some analytical results.
Thus, we take
and the moments (39) become
i.e., for
and
,
In this case, the corresponding moment determinants can be evaluated as (see [
3,
5])
where
and
Lemma 1. The value of is Using Theorem 1 and the previous lemma, we can calculate the norm
of the orthogonal polynomial in this symmetric case, when
,
and
, because (see Equation (
13))
Theorem 10. We havewhere . Using these explicit expressions, we can state the following corollary of Theorem 6, for the Legendre weight:
Corollary 1. Let , and . The monic orthogonal polynomials , with respect to the inner product (
30)
, with , satisfy the recurrence relation (
32)
, whereexcept , when . Example 7. Recurrence coefficients and in (
32)
for polynomials from Example 1 (), regarding Corollary 1, are Consider now an interesting simple case when
(even number of rays
) and inner product is given by (
17), as in Example 2. Then, Theorem 7 reduces to the following result (see [
2]):
Corollary 2. The sequence of monic orthogonal polynomials , related to the inner product (
17)
, satisfies the recurrence relationwith , , where In this simple symmetric case
, with inner product given in Example 2 (i) by (
17), the extremal problem from Theorem 2 reduces to the following (see [
48]):
Corollary 3. For each , where , , and , we havewhereand for . Here, we used Theorem 10 for .
6.4. An Analog of the Jacobi Polynomials (M—Generalized Gegenbauer Polynomials)
We consider a symmetric case of
M unit rays, equidistantly spaced (
,
), with the same weight function on the rays:
The inner product is given by
Let
be the monic Jacobi polynomial on
, orthogonal with respect to the weight function
. It is connected to the standard monic Jacobi polynomial
on
as
Using the three-term recurrence relation for
(cf. [
13], p. 132), we obtain the corresponding recurrence relation for the polynomial
,
where
and
According to Theorems 6 and 8, we can prove the following result:
Theorem 11. The monic polynomials , orthogonal with respect to the inner product (41), with the weight function (40), satisfy the recurrence relation of the form (32), and can be expressed in the formwhere and Remark 4. The case when was considered in [
2].
Remark 5. As we can see, from (
40)
and (
41)
for , we obtain the monic orthogonal polynomials in Legendre case (cf.
Section 6.3)
:where and . For , it reduces to , where and .
Since the leading coefficient in the standard Jacobi polynomial is given by (cf. [
13], p. 132)
, for generating, e.g., the first 12
(monic) orthogonal polynomials , only one command in the Wolfram (Mathematica) 14.1
is enough: Flatten[Table[(k!/Pochhammer[(4k+2v+1)/4,k])z^v
JacobiP[k,0,(2v-3)/4,2z^4-1],{k,0,2},{v,0,3}]]//Simplify
Note that these polynomials for were calculated by the complicated “determinant approach” in Example 2 (i).
Remark 6. Taking , the weight function (
40)
becomes , where and . Since it can be written in the form , as well as the inner product (
41)
,we conclude that the orthogonal polynomials , where and , in this case are, in fact, the generalized Gegenbauer polynomials introduced in 1953 by Laščenov [
49] (cf.
Chihara ([
45], pp. 155–156)
and Mastroianni and Milovanović ([
13], p. 147)
. This is the reason we refer the polynomials (
42)
as the M-generalized Gegenbauer polynomials. 6.5. Some Analogs of the Generalized Laguerre (M-Generalized Hermite Polynomials)
We now consider a symmetric case of
M infinity rays, equidistantly spaced as in
Section 6.4, with the same weight function on the rays
and the inner product given by
with
denoting the monic generalized orthogonal Laguerre polynomials related to the weight function
on
. Such polynomials satisfy the three-term recurrence relation: ([
13], p. 141)
Using Theorems 6 and 8, we can prove the following result:
Theorem 12. The monic orthogonal polynomials , related to the inner product (
44)
, with the weight function (
43)
, satisfy the recurrence relation of the form (
32)
, and can be expressed in the formwhere Remark 7. The case when was considered in [
1,
2].
Then, according to Theorem 7, the polynomials , , satisfy (
33)
, where for , 6.6. Differential Equation
In the completely symmetric case, with
M rays, using (
35), we can prove the following result (cf. [
3]).
Theorem 13. If polynomials , , defined in (35), satisfy linear differential equations of the second order of the formthen the monic orthogonal polynomial satisfies the following second-order linear differential equation:where Starting from the Jacobi differential equation for
(cf. [
50], p. 781)
i.e., from the corresponding equation for polynomials
orthogonal on
,
and Theorem 13, we conclude that the monic polynomials
from Theorem 12 satisfy the second-order linear differential equation:
A similar result can be obtained for orthogonal polynomials from Theorem 12 (see [
1]).
8. Concluding Remarks
The main concept on orthogonal polynomials on the radial rays is presented, including existence and uniqueness, a general method for constructing (the so-called Stieltjes–Gautschi procedure), the main properties of such polynomials, as well as some applications.
Special attention is paid to completely symmetric cases, i.e., when the rays are distributed equidistant in the complex plane, with equal lengths and the same weights on the rays. A recurrence relation for these polynomials, a connection with standard polynomials orthogonal on the real line and a differential equation are derived. It is shown that their zeros are simple and distributed symmetrically on the radial rays, with the possible exception of a multiple zero at the origin. In a symmetric radial ray scenario, using the corresponding differential equations, a few applications are given.