Mathematical Model for the Control of Red Palm Weevil
Abstract
1. Introduction
2. Mathematical Formulation
Positivity and Boundedness
3. Model Analysis
3.1. Equilibrium Points
3.2. Basic Reproduction Number
3.3. Stability of the RPW System
- The eigenvalues of at the extinction equilibrium point are , , , ; thus, is always unstable. Therefore, the entire population cannot become extinct at the same time.
- The eigenvalues of at the weevil-free equilibrium point are , , , and ; thus, is locally stable if and .
- One of the eigenvalues of is positive; so, , the palm tree extinction equilibrium point, is unstable.
- The eigenvalues of the Jacobian matrix at the infected palm tree extinction equilibrium point are , , , and . Therefore, is unstable.
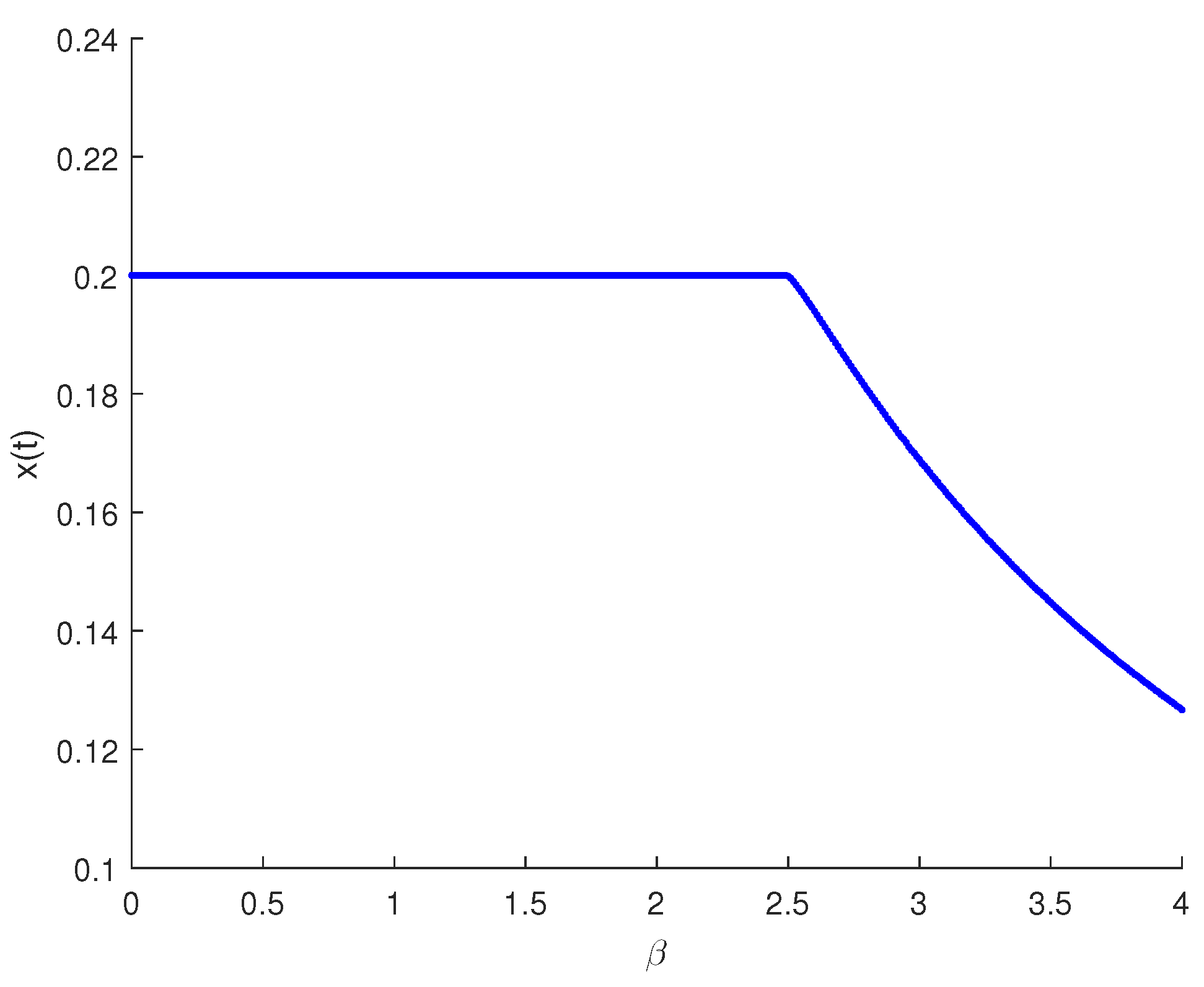
4. Model Analysis Optimization of the Biological Control
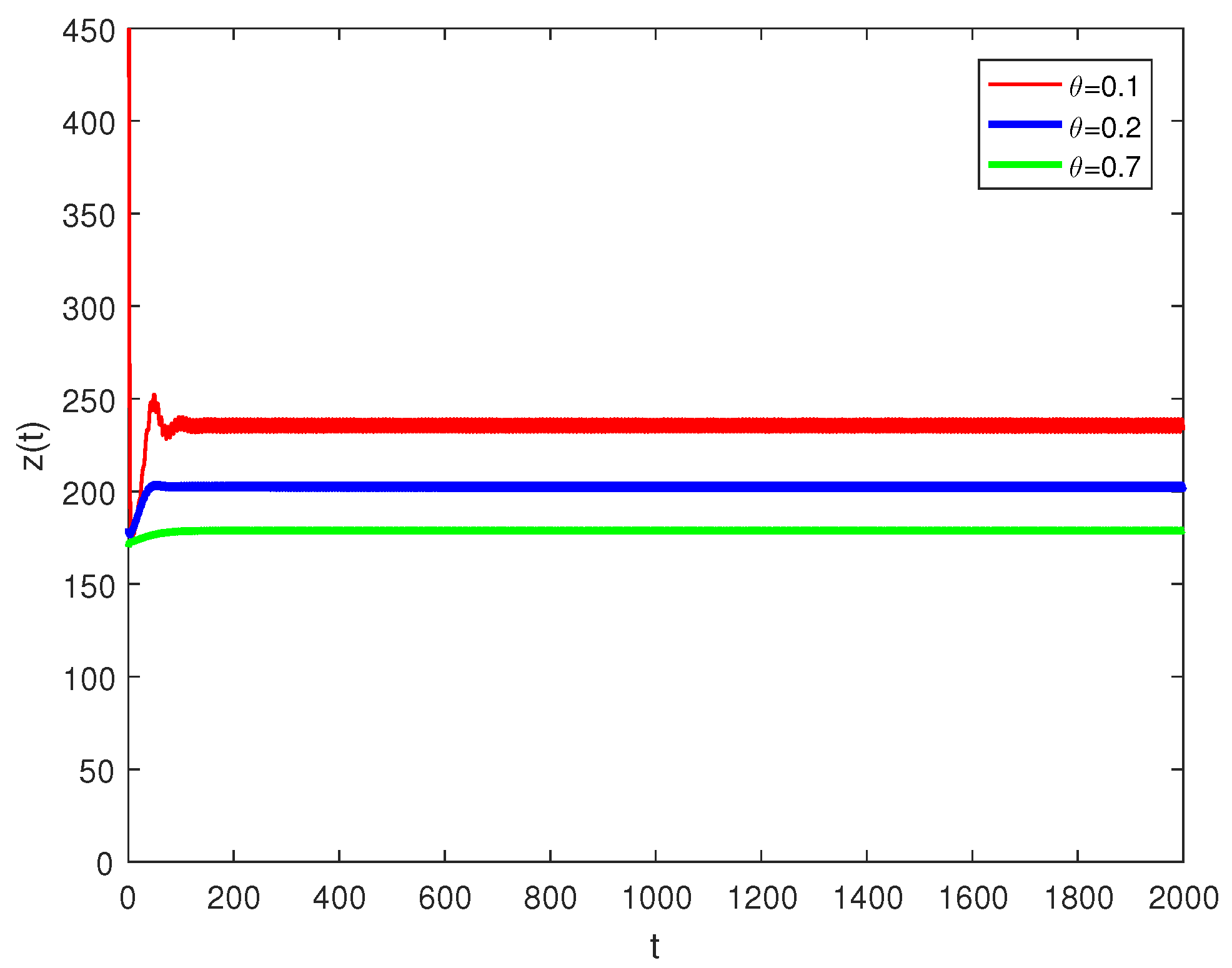
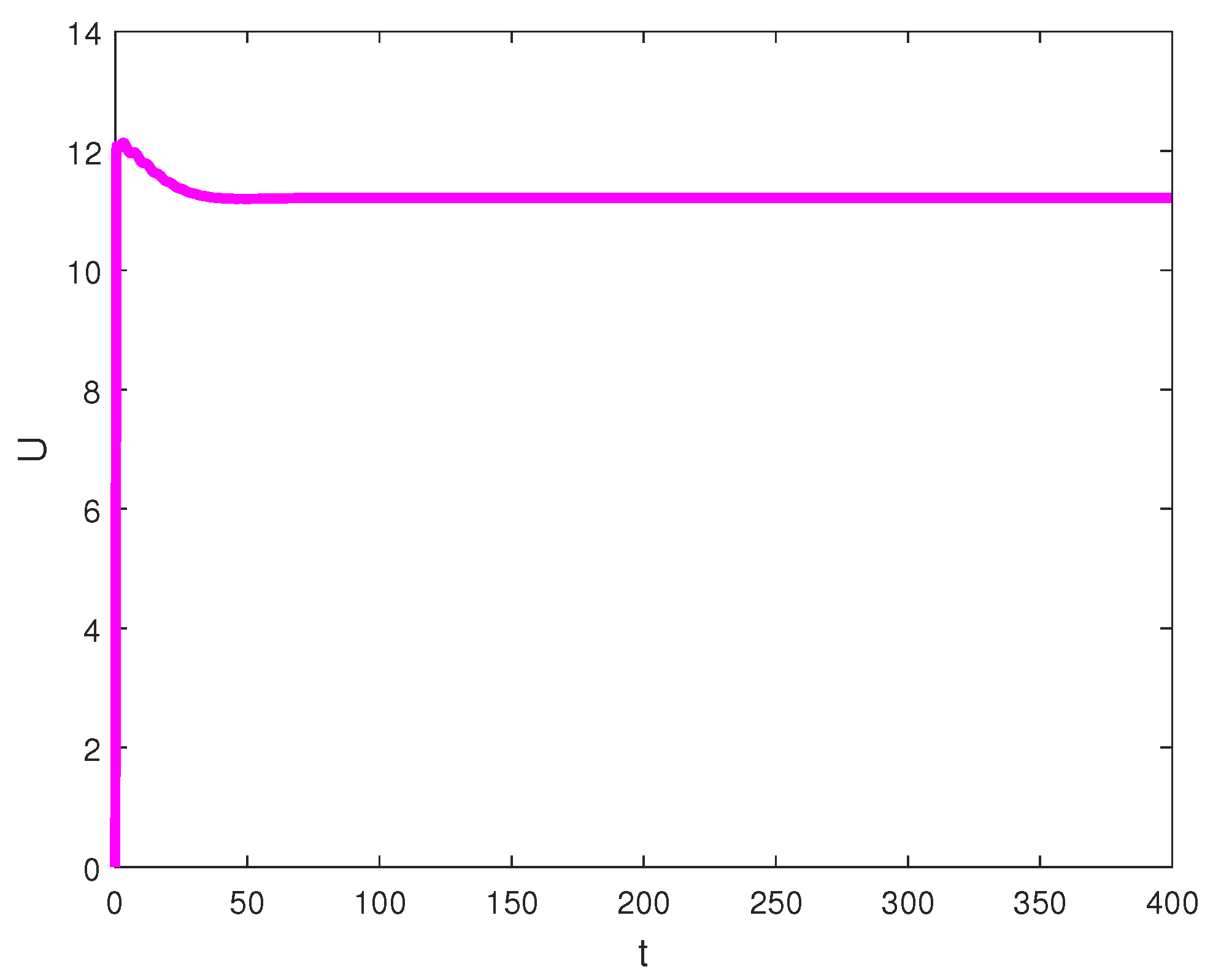
5. Numerical Simulations
6. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Correction Statement
References
- Aldawood, A.; Rasool, K. Rearing optimization of red palm weevil: Rhynchophorus ferrugineus (Coleoptera: Curculionidae) on date palm: Phoenix dactylifera. Fla. Entomol. 2011, 94, 756. [Google Scholar] [CrossRef]
- Abdel-Banat, B.; El-Shafie, H. Management of the Red Palm Weevil in Date Palm Plantations in Al-Ahsa Oasis of Saudi Arabia. Plant Health Cases 2023, 2023, phcs20230001. [Google Scholar] [CrossRef]
- Seman-Kamarulzaman, A.F.; Pariamiskal, F.A.; Azidi, A.N.; Hassan, M. A Review on Digestive System of Rhynchophorus ferrugineus as Potential Target to Develop Control Strategies. Insects 2023, 14, 506. [Google Scholar] [CrossRef] [PubMed]
- Hoddle, M.S.; Antony, B.; El-Shafie, H.A.; Chamorro, M.L.; Milosavljević, I.; Löhr, B.; Faleiro, J.R. Taxonomy, Biology, Symbionts, Omics, and Management of Rhynchophorus Palm Weevils (Coleoptera: Curculionidae: Dryophthorinae). Annu. Rev. Entomol. 2024, 69, 455–479. [Google Scholar] [CrossRef] [PubMed]
- Manee, M.M.; Alqahtani, F.H.; Al-Shomrani, B.M.; El-Shafie, H.A.; Dias, G.B. Omics in the Red Palm Weevil Rhynchophorus ferrugineus (Olivier) (Coleoptera: Curculionidae): A Bridge to the Pest. Insects 2023, 14, 255. [Google Scholar] [CrossRef]
- Al-Khayri, J. Date palm Phoenix dactylifera micropropagation. In Protocols for Micropropagation of Woody Trees and Fruits; Springer: Berlin/Heidelberg, Germany, 2007; pp. 509–526. [Google Scholar]
- Eldin, H.A.; Waleed, K.; Samir, M.; Tarek, M.; Sobeah, H.; Salam, M.A. A survey on detection of Red Palm Weevil inside palm trees: Challenges and applications. In Proceedings of the 9th International Conference on Software and Information Engineering, Cairo, Egypt, 11–13 November 2020; pp. 119–125. [Google Scholar]
- Mashal, M.M.; Obeidat, B.F. The efficacy assessment of emamectin benzoate using micro injection system to control red palm weevil. Heliyon 2019, 5, e01833. [Google Scholar] [CrossRef]
- Refaat, E.S.M.; El Shazly, M.; El Deeb, M.; Eliwa, A. Study the effect of mechanical injection technique on controlling red palm weevil. Zagazig J. Agric. Res. 2017, 44, 1889–1900. [Google Scholar]
- Abbas, M.S.T. IPM of the red palm weevil, Rhynchophorus ferrugineus. In Integrated Management of Arthropod Pests and Insect Borne Diseases; Springer: Berlin/Heidelberg, Germany, 2010; pp. 209–233. [Google Scholar]
- Chihaoui-Meridja, S.; Harbi, A.; Abbes, K.; Chaabane, H.; La Pergola, A.; Chermiti, B.; Suma, P. Systematicity, persistence and efficacy of selected insecticides used in endotherapy to control the red palm weevil Rhynchophorus ferrugineus (Olivier, 1790) on Phoenix canariensis. Phytoparasitica 2020, 48, 75–85. [Google Scholar] [CrossRef]
- El-Juhany, L.I. Degradation of date palm trees and date production in Arab countries: Causes and potential rehabilitation. Aust. J. Basic Appl. Sci. 2010, 4, 3998–4010. [Google Scholar]
- Hoddle, M.; Al-Abbad, A.H.; El-Shafie, H.; Faleiro, J.; Sallam, A.; Hoddle, C. Assessing the impact of areawide pheromone trapping, pesticide applications, and eradication of infested date palms for Rhynchophorus ferrugineus (Coleoptera: Curculionidae) management in Al Ghowaybah, Saudi Arabia. Crop Prot. 2013, 53, 152–160. [Google Scholar] [CrossRef]
- Milosavljević, I.; El-Shafie, H.A.; Faleiro, J.R.; Hoddle, C.D.; Lewis, M.; Hoddle, M.S. Palmageddon: The wasting of ornamental palms by invasive palm weevils, Rhynchophorus spp. J. Pest Sci. 2019, 92, 143–156. [Google Scholar] [CrossRef]
- Sarwar, M. Biological control of red palm weevil Rhynchophorus Ferrugineus (Coleoptera: Curculionidae) by the natural enemies. Int. J. Res. Biol. Pharm. 2016, 2, 22–35. [Google Scholar]
- El Sadawy, H.A.; Namaky, A.H.E.; Al Omari, F.; Bahareth, O.M. Susceptibility of Rhynchophorus Ferrugineus (Olivier) (Coleoptera: Curculionidae) to entomopathogenic nematodes with regard to its immune response. Biol. Control 2020, 148, 104308. [Google Scholar] [CrossRef]
- Rehman, G.; Mamoon-ur Rashid, M. Evaluation of entomopathogenic nematodes against red palm weevil, Rhynchophorus ferrugineus (Olivier) (Coleoptera: Curculionidae). Insects 2022, 13, 733. [Google Scholar] [CrossRef]
- El-Shahed, M.; Al-Dubiban, A.M. Mathematical Modelling of Lesser Date Moth Using Sex Pheromone Traps and Natural Enemies. Math. Probl. Eng. 2021, 2021, 8835321. [Google Scholar] [CrossRef]
- El-Shahed, M.; Al-Dububan, A.M. Deterministic and Stochastic Fractional Order Model for Lesser Date Moth. Comput. Syst. Sci. Eng. 2022, 40, 749–764. [Google Scholar] [CrossRef]
- El-Shahed, M.; Al-Nujiban, A.; Abdel-Baky, N.F. Stochastic Modelling of Red Palm Weevil Using Chemical Injection and Pheromone Traps. Axioms 2022, 11, 334. [Google Scholar] [CrossRef]
- Ben Dhahbi, A.; Chargui, Y.; Boulaaras, S.M.; Ben Khalifa, S. A one-sided competition mathematical model for the sterile insect technique. Complexity 2020, 2020, 1–12. [Google Scholar] [CrossRef]
- Ben Dhahbi, A.; Chargui, Y.; Boulaaras, S.M.; Ben Khalifa, S.; Koko, W.; Alresheedi, F. Mathematical modelling of the sterile insect technique using different release strategies. Math. Probl. Eng. 2020, 2020, 8896566. [Google Scholar] [CrossRef]
- Solano-Rojas, Y.; Gámez, M.; López, I.; Garay, J.; Varga, Z.; Cabello, T. Conservation Strategy for Palm Groves: Optimal Chemical Control Model for Red Palm Weevil, Rhynchophorus ferrugineus. Agronomy 2021, 11, 920. [Google Scholar] [CrossRef]
- Alnafisah, Y.; El-Shahed, M. Optimal control of red palm weevil model incorporating sterile insect technique, mechanical injection, and pheromone traps. Alex. Eng. J. 2024, 93, 382–391. [Google Scholar] [CrossRef]
- Gulati, P.; Chauhan, S.; Mubayi, A.; Singh, T.; Rana, P. Dynamical analysis, optimum control and pattern formation in the biological pest (EFSB) control model. Chaos Solitons Fractals 2021, 147, 110920. [Google Scholar] [CrossRef]
- Barik, M.; Chauhan, S.; Misra, O.P.; Bhatia, S.K. Optimal control using linear feedback control and neutralizing antibodies for an HIV model with dynamical analysis. J. Appl. Math. Comput. 2022, 68, 4361–4389. [Google Scholar] [CrossRef]
- Molter, A.; Bezerra, J.I.; Rafikova, E.; Nava, D.E.; Rafikov, M. Dynamics and biological control of the sugarcane borer with two parasitoids. Ecol. Model. 2023, 481, 110371. [Google Scholar] [CrossRef]
- Rodrigues, L.R.; Gabrielli, F. State Feedback as a Strategy for Control and Analysis of COVID-19. arXiv 2024, arXiv:2405.17735. [Google Scholar]
- Farman, M.; Saleem, M.U.; Tabassum, M.F.; Ahmad, A.; Ahmad, M.O. A linear control of composite model for glucose insulin glucagon pump. Ain Shams Eng. J. 2019, 10, 867. [Google Scholar] [CrossRef]
- Di Giamberardino, P.; Iacoviello, D. A linear quadratic regulator for nonlinear SIRC epidemic model. In Proceedings of the 2019 23rd International Conference on System Theory, Control and Computing (ICSTCC), Sinaia, Romania, 9–11 October 2019; pp. 733–738. [Google Scholar]
- Lewis, F.L.; Vrabie, D.; Syrmos, V.L. Optimal Control; John Wiley and Sons: Hoboken, NJ, USA, 2012. [Google Scholar]
- Anderson, B.D.; Moore, J.B. Optimal Control: Linear Quadratic Methods; Courier Corporation: Washington, DC, USA, 2007. [Google Scholar]
- Bernstein, D.S. Nonquadratic cost and nonlinear feedback control. Int. J. Robust Nonlinear Control. 1993, 3, 211–229. [Google Scholar] [CrossRef]
- Asri, S.; Rodrigues, L. Data-Driven LQR using Reinforcement Learning and Quadratic Neural Networks. arXiv 2023, arXiv:2311.10235. [Google Scholar]
- Ismail, A.I.; Hassaballa, A.A.; Almadini, A.M.; Daffalla, S. Analyzing the Spatial Correspondence between Different Date Fruit Cultivars and Farms’ Cultivated Areas, Case Study: Al-Ahsa Oasis, Kingdom of Saudi Arabia. Appl. Sci. 2022, 12, 5728. [Google Scholar] [CrossRef]
- Ali-Bob, M. Management of the red palm weevil Rhynchophorus ferrugineus (Olivier) using sustainable options in Saudi Arabia. Arab. J. Plant Prot. 2019, 37, 2019. [Google Scholar] [CrossRef]
- Abbas, M.K.; Hou, Y.; Wu, S.Y.; Batt, M.A. Evaluation of some control methods against the red palm weevil, Rhynchophorus ferrugineus Olivier (Coleoptera: Curculionidae) infesting date palm trees. Menoufia J. Plant Prot. 2024, 9, 223–229. [Google Scholar] [CrossRef]
- Fenton, A.; Norman, R.; Fairbairn, J.P.; Hudson, P.J. Modelling the efficacy of entomopathogenic nematodes in the regulation of invertebrate pests in glasshouse crops. J. Appl. Ecol. 2000, 37, 309–320. [Google Scholar] [CrossRef]
- Mohanny, K.M.; Mohamed, G.S.; Abdo, O.M. Laboratory study of life table and demographic parameters of Red Palm Weevil (Rhynchophorus ferrugineus, Coleoptera: Dryophthoridae) on sugarcane slices. SVU-Int. J. Agric. Sci. 2020, 2, 93–103. [Google Scholar] [CrossRef]
- Mehdi, M.Z.; Wakil, W.; Jan, H.; Raza, M.M.; Shah, Q.; Zia-ul-Haq, M. Evaluation of entomopathogenic nematode and fungi alone and their combination against red palm weevil, Rhynchophorus ferrugineus (Olivier). J. Entomol. Zool. Stud. 2018, 6, 2038–2042. [Google Scholar]
- Alwaneen, W.S.; Wakil, W.; Kavallieratos, N.G.; Qayyum, M.A.; Tahir, M.; Rasool, K.G.; Husain, M.; Aldawood, A.S.; Shapiro-Ilan, D. Efficacy and persistence of entomopathogenic fungi against Rhynchophorus ferrugineus on date palm: Host to host transmission. Agronomy 2024, 14, 642. [Google Scholar] [CrossRef]
- Fenton, A.; Norman, R.; Fairbairn, J.P.; Hudson, P.J. Evaluating the efficacy of entomopathogenic nematodes for the biological control of crop pests: A nonequilibrium approach. Am. Nat. 2001, 158, 408–425. [Google Scholar] [CrossRef]
- Mushanyu, J.; Chazuka, Z.; Mudzingwa, F.; Ogbogbo, C. Modelling the impact of detection on COVID-19 transmission dynamics in Ghana. RMS Res. Math. Stat. 2021, 8, 1953722. [Google Scholar] [CrossRef]
- Gatyeni, S.P.; Chirove, F.; Nyabadza, F. Modelling the potential impact of stigma on the transmission dynamics of COVID-19 in South Africa. Mathematics 2022, 10, 3253. [Google Scholar] [CrossRef]
- Birkhoff, G.; Rota, G. Ordinary Differential Equation; John Wiley and Sons: Hoboken, NJ, USA, 1982. [Google Scholar]
- Hassan, K.; Mustafa, A.; Hama, M. An Eco-Epidemiological Model Incorporating Harvesting Factors. Symmetry 2021, 13, 2179. [Google Scholar] [CrossRef]
- Brauer, F. Boundedness of solutions of predator-prey systems. Theor. Popul. Biol. 1979, 15, 268–273. [Google Scholar] [CrossRef]
- Diekmann, O.; Heesterbeek, J.A.P.; Roberts, M.G. The construction of next-generation matrices for compartmental epidemic models. J. R. Soc. Interface 2010, 7, 873–885. [Google Scholar] [CrossRef] [PubMed]
- Van den Driessche, P.; Watmough, J. Reproduction numbers and sub-threshold endemic equilibria for compartmental models of disease transmission. Math. Biosci. 2002, 180, 29–48. [Google Scholar] [CrossRef] [PubMed]
- La Salle, J.P. The Stability of Dynamical Systems; SIAM: Philadelphia, PA, USA, 1976. [Google Scholar]
- Castillo-Chavez, C.; Song, B. Dynamical models of tuberculosis and their applications. Math. Biosci. Eng. 2004, 1, 361–404. [Google Scholar] [CrossRef] [PubMed]
- Kumar, R.P.; Santra, P.K.; Mahapatra, G.S. Global stability and analysing the sensitivity of parameters of a multiple-susceptible population model of SARS-CoV-2 emphasising vaccination drive. Math. Comput. Simul. 2023, 203, 741–766. [Google Scholar] [CrossRef] [PubMed]
- Brunton, S.L.; Kutz, J.N. Data-Driven Science and Engineering: Machine Learning, Dynamical Systems, and Control; Cambridge University Press: Cambridge, UK, 2022. [Google Scholar]
- Rafikov, M.; de Holanda Limeira, E. Mathematical modelling of the biological pest control of the sugarcane borer. Int. J. Comput. Math. 2012, 89, 390–401. [Google Scholar] [CrossRef]
- Rafikov, M.; Silveira, J.C. On dynamical behavior of the sugarcane borer–Parasitoid agroecosystem. Ecol. Complex. 2014, 18, 67–73. [Google Scholar] [CrossRef]
- Shehab, B.; Ammr, M. THE INFLUENCE OF PREY AND PREDATOR DENSITY ON THE PREDACIOUS BEHAVIOR OF Chrysoperla mutata (MACLACHLAN) LARVAE FEEDING ON DUBAS NYMPHS Ommatissus lybicus DEBERG. Iraqi J. Sci. 2008, 49, 40–49. [Google Scholar]
- Al-Rawy, M.A.; Hamad, B.S.; Abdullatif, A.M. Factors affecting the effectiveness of Chrysoperla mutata (McL.) larvae feeding on dubas nymphs Ommatissus lybicus DeBerg. J. Educ. Sci. Stud. 2013, 1, 387–398. [Google Scholar]



| Parameter | Description | Value | Source |
|---|---|---|---|
| r | The growth rate of susceptible date palm trees | 0.038 | [35] |
| k | The carrying capacity of the date palm farm | 30000 | [36] |
| Red palm weevil infection rate | 0.025 | [36] | |
| Recovery rate of infected palm trees as a result of aluminum phosphide fumigation | 0.5 | [37] | |
| The rate of predation by larvae on the interior of the infected palm trunk | 0.08 | [38] | |
| Palm tree mortality rate as a result of red palm weevil infestation | 0.008 | [36] | |
| The rate of increase in the number of larvae as a result of eggs | 0.17 | [39] | |
| Predation intensity between larvae and entomopathogenic nematode | 0.7 | [40] | |
| The mortality rate of larvae | 0.15 | [41] | |
| Entomopathogenic nematodes mortality rate. | 0.1 | [42] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alqahtani, Z.; Almuneef, A.; El-Shahed, M. Mathematical Model for the Control of Red Palm Weevil. Axioms 2024, 13, 637. https://doi.org/10.3390/axioms13090637
Alqahtani Z, Almuneef A, El-Shahed M. Mathematical Model for the Control of Red Palm Weevil. Axioms. 2024; 13(9):637. https://doi.org/10.3390/axioms13090637
Chicago/Turabian StyleAlqahtani, Zuhur, Areej Almuneef, and Moustafa El-Shahed. 2024. "Mathematical Model for the Control of Red Palm Weevil" Axioms 13, no. 9: 637. https://doi.org/10.3390/axioms13090637
APA StyleAlqahtani, Z., Almuneef, A., & El-Shahed, M. (2024). Mathematical Model for the Control of Red Palm Weevil. Axioms, 13(9), 637. https://doi.org/10.3390/axioms13090637







