The Maximum Domain for an Analytical Approximate Solution to a Nonlinear Differential Equation in the Neighborhood of a Moving Singular Point
Abstract
:1. Introduction
2. Research Technology
- 1.
- within , where
- 2.
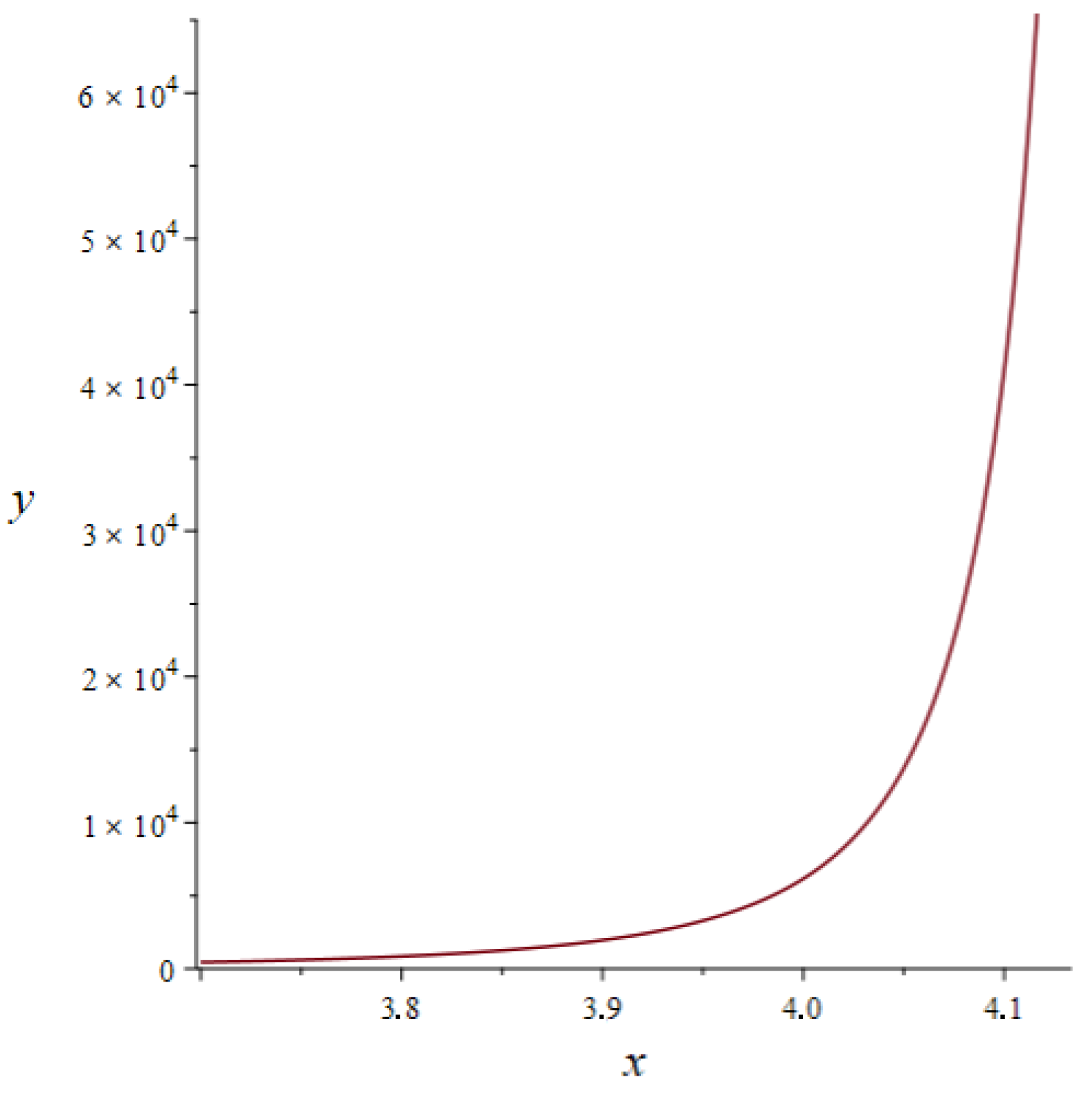
- 3.
- 4.
- There is an error estimation for
- 5.
3. Discussion of the Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yuqiang, F. Existence and uniqueness results for a third-order implicit differential equation. Comput. Math. Appl. 2008, 56, 2507–2514. [Google Scholar]
- Chugainova, A.P. Nonsteady solutions to the generalized Korteweg-de Fries-Burgers equation. Proc. Steklov Inst. Math. 2013, 281, 204–212. [Google Scholar] [CrossRef]
- Orlov, V.N.; Kovalchuk, O.A. An analytical solution with a given accuracy for a nonlinear mathematical model of a console-type construction. J. Phys. Conf. Ser. 2020, 1425, 012127. [Google Scholar] [CrossRef]
- Orlov, V.N.; Kovalchuk, O.A. Mathematical modeling of complex structures and nonlinear differential equations with movable points. IOP Conf. Ser. Mater. Sci. Eng. 2018, 456, 012122. [Google Scholar] [CrossRef]
- Chichurin, A.; Filipuk, G. The properties of certain linear and nonlinear differential equations of the fourth order arising in beam models. J. Phys. Conf. Ser. 2020, 1425, 012107. [Google Scholar] [CrossRef]
- Veresovich, P.P.; Yablonsky, A.I. Moving singular points of systems of third-order differential equations. Differ. Equ. 1977, 13, 1932–1939. [Google Scholar]
- Pisarenok, V.P.; Yablonsky, A.I. A differential equation having solutions with algebraic moving singular points. Differ. Equ. 1976, 12, 928–930. [Google Scholar]
- Sobolevsky, S.L. Movable singular points of polynomial ordinary differential equations. Differ. Equ. 2004, 40, 756–762. [Google Scholar] [CrossRef]
- Sobolevsky, S.L. Movable singular points of algebraic ordinary differential equations. Differ. Equ. 2005, 41, 1146–1154. [Google Scholar] [CrossRef]
- Filipuk, G.; Kecker, T. Singularities of certain non-linear second-order ordinary differential equations. Results Math 2022, 77, 41. [Google Scholar] [CrossRef]
- Filipuk, G.; Halburd, R.G. Movable algebraic singularities of second-order ordinary differential equations. J. Math. Phys. 2009, 50, 023509. [Google Scholar] [CrossRef]
- Conte, R.; Musette, M. The Painlevé Handbook; Springer Science+Business Media: Dordrecht, The Netherlands, 2008. [Google Scholar]
- Filipuk, G.; Chichurin, A. The properties of certain linear and nonlinear differential equations. Adv. Mech. Math. 2019, 41, 193–200. [Google Scholar] [CrossRef]
- Chichurin, A.; Shvychkina, H. Computer simulation of two chemostat models for one nutrient resource. Math. Biosci. 2016, 278, 30–36. [Google Scholar] [CrossRef]
- Evtushenko, S. A nonlinear system of differential equations in supercritical flow spread problem and its solution technique. Axioms 2023, 12, 11. [Google Scholar] [CrossRef]
- Dukhnovskii, S.A. Global existence theorems of a solution of the Cauchy problem for systems of the kinetic Carleman and Godunov–Sultangazin equations. Eurasian Math. J 2021, 12, 97–102. [Google Scholar] [CrossRef]
- Dukhnovsky, S.A. A self–similar solution and the tanh–function method for the kinetic Carleman system. Bul. Acad. Ştiinţe Repub. Mold. Mat 2022, 99–110. [Google Scholar] [CrossRef]
- Dukhnovsky, S.A. New exact solutions for the time fractional Broadwell system. Adv. Stud. Euro-Tbil. Math. J. 2022, 15, 53–66. [Google Scholar] [CrossRef]
- Dukhnovskii, S.A. New exact solutions for the two-dimensional Broadwell system. Bull. Saratov Univ. New Episode. Ser. Math. Mech. Inform. 2022, 22, 4–14. [Google Scholar] [CrossRef]
- Dukhnovsky, S.A. Painlevé test and self-similar solution of the kinetic model. In Proceedings of the XVII All-Russian Youth School-Conference “Lobachevsky Readings-2018”, Kazan, Russia, 23–28 November 2018; pp. 91–94. [Google Scholar] [CrossRef]
- Dukhnovskii, S.A. Solutions of the Carleman system through the Painlevé expansion. Vladikavkaz. Math. J. 2020, 22, 58–67. [Google Scholar] [CrossRef]
- Kudryashov, N.A.; Sinelshchikov, D.I. Analytical solutions of the Rayleigh equation for empty and gas-filled bubble. J. Phys. A Math. Theory 2014, 47, 405202. [Google Scholar] [CrossRef]
- Kudryashov, N.A.; Sinelshchikov, D.I. Analytical solutions for problems of bubble dynamics. Phys. Lett. A 2015, 379, 798–802. [Google Scholar] [CrossRef]
- Leont’eva, T.Y. About one generalization of exact criteria for the existence moving singular points of one class of nonlinear ordinary differential equations in the complex area. Belgorod State Univ. Sci. Bull. Math. Phys. 2017, 13, 262. [Google Scholar]
- Pchelova, A.Z. Boundaries of approximate solution in the neighbourhood of perturbed moving singular point of one differential equation in the complex domain. Vestn. VSU. Ser. Phys. Math. 2014, 4, 170–179. [Google Scholar]
- Yves, B.B. Existence theorem for a solution of one class of nonlinear differential equations of the fourth order with polynomial right-hand side of the second degree in the vicinity of a moving singular point. Bull. Bashkir Univ. 2018, 23, 980–986. [Google Scholar]
- Orlov, V.N.; Kolle, K.V. Analytical approximate solution of a third-order nonlinear differential equation with a polynomial right-hand side of the second degree in the neighborhood of a moving singular point. Vestn. Yakovlev Chuvash State Pedagog. Univ. Ser. Mech. Limit State 2015, 4, 96–108. [Google Scholar]
- Aleroev, T.S.; Gasanov, M.V. Perturbation of a moving singular point and analytical approximate solution for one class of third order nonlinear differential equations. Vestn. Yakovlev Chuvash State Pedagog. Univ. Ser. Mech. Limit State 2021, 1, 16–27. [Google Scholar] [CrossRef]
- Aleroev, T.S.; Gasanov, M.V. Necessary and sufficient condition of existence of a moving singular point for the nonlinear differential equation of third order. Vestn. Yakovlev Chuvash State Pedagog. Univ. Ser. Mech. Limit State 2021, 1, 49–55. [Google Scholar] [CrossRef]
- Orlov, V.; Gasanov, M. Technology for obtaining the approximate value of moving singular points for a class of nonlinear differential equations in a complex domain. Mathematics 2022, 10, 3984. [Google Scholar] [CrossRef]
- Bakhvalov, N.S. Numerical Methods; Nauka: Moscow, Russia, 1970; p. 632. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Orlov, V.; Gasanov, M. The Maximum Domain for an Analytical Approximate Solution to a Nonlinear Differential Equation in the Neighborhood of a Moving Singular Point. Axioms 2023, 12, 844. https://doi.org/10.3390/axioms12090844
Orlov V, Gasanov M. The Maximum Domain for an Analytical Approximate Solution to a Nonlinear Differential Equation in the Neighborhood of a Moving Singular Point. Axioms. 2023; 12(9):844. https://doi.org/10.3390/axioms12090844
Chicago/Turabian StyleOrlov, Victor, and Magomedyusuf Gasanov. 2023. "The Maximum Domain for an Analytical Approximate Solution to a Nonlinear Differential Equation in the Neighborhood of a Moving Singular Point" Axioms 12, no. 9: 844. https://doi.org/10.3390/axioms12090844
APA StyleOrlov, V., & Gasanov, M. (2023). The Maximum Domain for an Analytical Approximate Solution to a Nonlinear Differential Equation in the Neighborhood of a Moving Singular Point. Axioms, 12(9), 844. https://doi.org/10.3390/axioms12090844








