Hamiltonian Indices of Three Classes of Graphs Obtained from Petersen Graph

Abstract

In this paper, we mainly consider the Hamiltonian indices of three classes of graphs obtained from Petersen graph, that is, the minimum integer m of m-time iterated line graph $L^m\left(G\right)$ of these three classes of graphs such that $L^m\left(G\right)$ is Hamiltonian. We show that the Hamiltonian indices of those graphs obtained by replacing every vertex of Petersen graph with a n-cycle or a complete graph of order n, or adding n pendant edges to each vertex of Petersen graph are both 2. In addition, we also study the situations of adding an edge to these three classes of graphs and obtain that their Hamiltonian indices are both 2.

1. Preliminaries

The line graph $L\left(G\right)$ of a graph G is the graph with vertex set $E\left(G\right)$, in which two vertices are adjacent if, and only if, the corresponding edges have a common endvertex in G. For $m\ge 1$, the m-time iterated line graph $L^m\left(G\right)$ is defined recursively by $L^0\left(G\right)=G$, $L^1\left(G\right)=L\left(G\right)$ and $L^m\left(G\right)$ = $L\left(L^{m-1}\left(G\right)\right)$, and we assume that $E\left(L^{m-1}\left(G\right)\right)$ is not empty. The Hamiltonian index is the smallest integer m such that $L^m\left(G\right)$ is Hamiltonian, i.e., it has a Hamiltonian cycle, denoted by $h\left(G\right)$. The supereulerian index is the smallest integer n such that $L^n\left(G\right)$ is supereulerian, denoted by $s\left(G\right)$. A $factor$ of a graph G is a spanning subgraph of G. If each vertex in a factor has a positive even degree, then we call such a factor an $even$ $factor$. If the degree of each vertex in an even factor is 2, then it is called a 2-factor. A graph containing a connected even factor is called $supereulerian$. The path and cycle with n vertices are denoted $P_n$ and $C_n$, respectively; a n-cycle is a cycle with n vertices. A $complete$ $graph$ is a simple graph whose vertices are pairwise adjacent; the $complete$ $graph$ with n vertices is denoted $K_n$. The Petersen graph is a simple graph whose vertices are the 2-element subsets of a 5-element set and whose edges are the pairs of disjoint 2-element subsets. In this paper, we mainly study the Hamiltonian indices of three classes of graphs obtained from Petersen graph, and the notation and terminology refer to [1].

A dominating closed trail (abbreviated DCT) in a graph G is a closed trail (or, equivalently, an eulerian subgraph) T in G such that every edge of G has at least one vertex on T. In 1965, the following result by Harary and Nash-Williams [2] related the existence of a DCT in a graph G and the existence of a Hamiltonian cycle in its line graph $L\left(G\right)$. The main results are as follows:

Theorem 1

([2]). Let G be a connected graph with at least three edges. Then $h\left(G\right)\le 1$ if and only if G has a DCT.

In 1968, Chartrand [3] showed that if G is a connected graph that is not a path, then for some integers $m>0$, $L^m\left(G\right)$ is Hamiltonian. In 1973, Chartrand and Wall [4] proved a well-known result which stated that:

Theorem 2

([4]). Let G be a connected graph with minimum degree at least 3. Then $h\left(G\right)\le 2$.

In [5,6], Veldman and Lai considered that both the graph of the diameter was at most 2 and obtained that:

Theorem 3

([5,6]). If G is a graph of diameter at most 2 with $\left|V\right(G\left)\right|\ge 4$, then $L\left(G\right)$ is Hamiltonian.

In 1983, Clark and Wormald [7] generalized the definition of Hamiltonian indices and introduced the concept of a Hamiltonian-like index. In 1990, Catlin et al. [8] studied Hamiltonian cycles and closed trails (supereulerian graphs) in iterated line graphs, and they had given the relationship between Hamiltonian index and Supereulerian index. In 2009, Chen and Lai [9] gave some relations between the Hamilton-connected index and the minimum and maximum degrees of a graph. A graph is $Hamilton$-$connected$ if for any two vertices $u,v\in V\left(G\right)$, there exists a $(u,v)$-path containing all vertices of G. The Hamilton-connected index $hc\left(G\right)$ is the least nonnegative integer m such that $L^m\left(G\right)$ is Hamilton-connected. The following result is obtained in [9]:

Theorem 4

([9]). Let G be a connected graph with minimum degree at least 3. Then $hc\left(G\right)\le 3$.

Let $d_G\left(P\right)=d_G\left(v_1\right)+d_G\left(v_2\right)+\cdots +d_G\left(v_m\right)$, and $V\left(P\right)=\{v_1,v_2,\cdots ,v_m\}$ denote ${\overline{\sigma }}_m\left(G\right)$ = min $\{d_G\left(P\right):P$ is a path of G with $\left|V\right(P\left)\right|=m\}$. Recently, in 2021, Liu and Xiong [10] showed that a sharp lower bound of min $\{{\sum }_{i=1}^m{d}_G\left(v_i\right):v_1{v}_2\cdots v_m$ is a path of a connected or 2-connected graph G} such that $(m-1)$-iterated line graphs $L^{m-1}\left(G\right)$ are Hamiltonian. The main results follow:

Theorem 5

([10]). Let $m\ge 3$ be an integer and let G be a connected graph of order $n>m+2$ such that ${\overline{\sigma }}_m\left(G\right)>n+m-3$, then $L^{m-1}\left(G\right)$ is Hamiltonian, i.e., $h\left(G\right)\le m-1$.

Theorem 6

([10]). Let $m\ge 3$ be an integer and let G be a 2-connected graph of order $n>6m+3$ such that ${\overline{\sigma }}_m\left(G\right)>\frac{2n}{5}-\frac{2m}{5}-\frac{1}{5}$, then $L^{m-1}\left(G\right)$ is Hamiltonian, i.e., $h\left(G\right)\le m-1$.

Xiong et al. [11] had a result such that adding an edge to a graph cannot increase its Hamiltonian index. The main results follow:

Theorem 7

([11]). Let G be a connected graph with at least three edges that is not a path. Then for any two vertices $a,b\in V\left(G\right)$ with $d_G\left(a\right)+d_G\left(b\right)\ge 3$, either $h\left(G\right)=1$ and $h(G+ab)=2$ or $h\left(G\right)\ge h(G+ab)$. Moreover, if $dis{t}_G(a,b)=2$, then $h\left(G\right)\ge h(G+ab)$.

Additional results on Hamiltonian indices can be found in [12,13,14,15]. Motivated by these researches, we consider the Hamiltonian indices of three classes of graphs obtained from Petersen graph and the graphs obtained by adding an edge to these three classes of graphs.

2. Our Main Results

In this section, we first consider the Hamiltonian indices of three classes of graphs obtained from Petersen graph: the graph obtained by replacing every vertex of Petersen graph with a n-cycle $(n\ge 3)$, the graph obtained by replacing every vertex of Petersen graph with a complete graph of order $n(n\ge 3)$ and the graph obtained by adding $n(n\ge 3)$ pendant edges to each vertex of Petersen graph. Secondly, we consider the results of adding an edge to these three classes of graphs.

Because the Petersen graph, as well as adding an edge to the Petersen graph, satisfies the condition of Theorem 3, we have the following results:

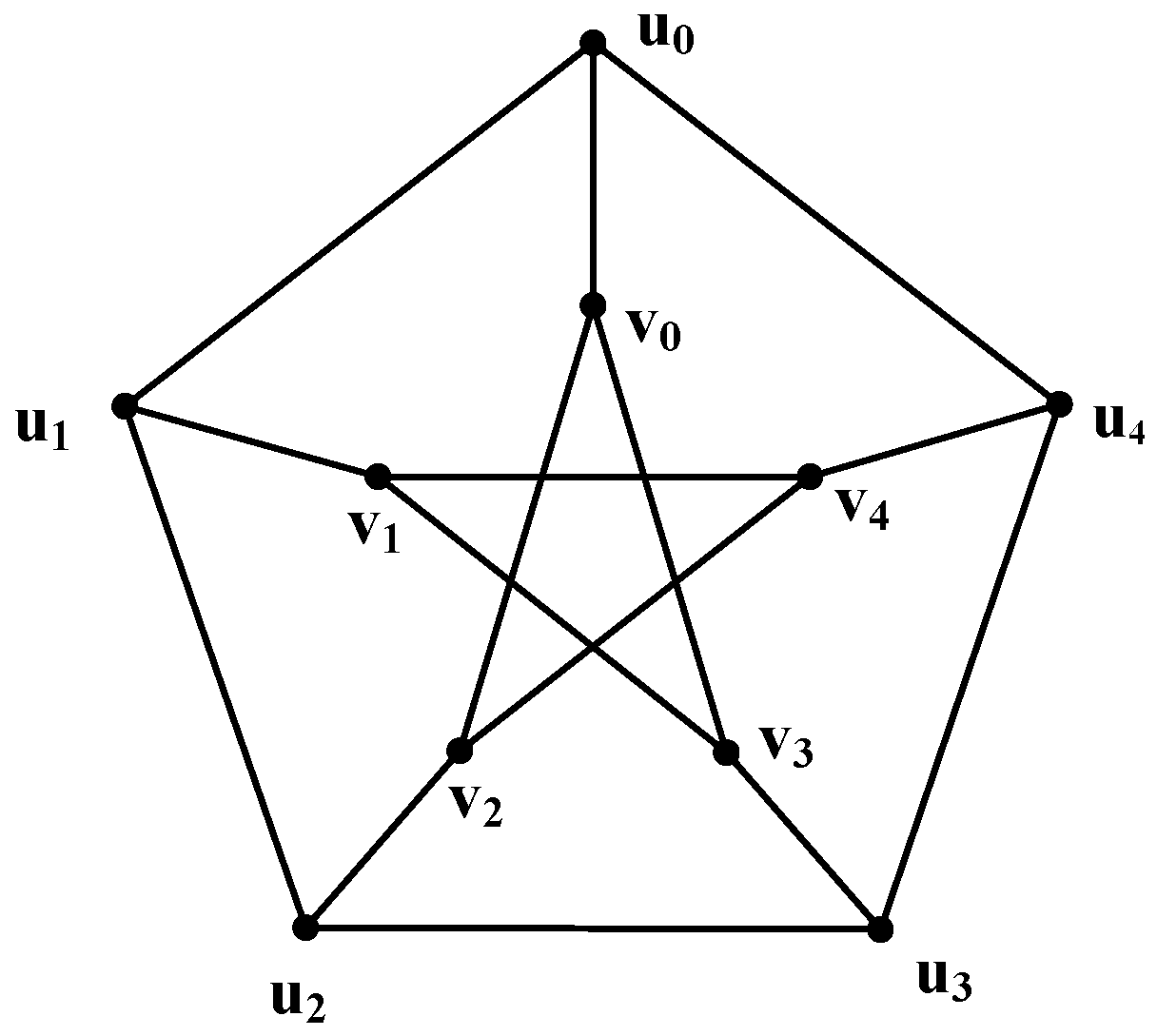
Corollary 1.

Let G be a Petersen graph (see Figure 1), then $h\left(G\right)=1$.

Corollary 2.

Let G be a Petersen graph, for any two vertices $a,b\in V\left(G\right)$, then $h(G+ab)=1$.

Before giving our main results, we introduce some additional necessary concepts. If $P=u_1{u}_2\dots u_k$ is a path in a graph G and $S,T\subset G$, then we say that P is an $(S,T)$-$path$ if $u_1\in V\left(T\right)$ and $u_k\in V\left(S\right)$. The $distance$ of two subgraphs $S,T\subset G (denoted$ by $d_G(S,T))$ is the minimum length of an $(S,T)$-$path$. $d_G\left(v\right)$ which denotes the degree of a vertex v in G. For any integer $i\ge 0$, let $V_i\left(G\right)=\{v\in V\left(G\right):d_G\left(v\right)=i\}$. A $branch$ in G is a nontrivial path whose internal vertices have a degree of 2 and end vertices have a degree other than 2. If a branch has a length of 1, then it has no internal vertex. Let $B\left(G\right)$ denote the set of branches of G, and $B_1\left(G\right)$ be the subset of $B\left(G\right)$ in which every branch has an end vertex in $V_1\left(G\right)$. For any subgraph H of G, let $B_H\left(G\right)$ be the set of those branches of G which have all edges in H.

If G is a graph and $m\ge 2$ is an integer, then $E{U}_m\left(G\right)$ denotes the set of all subgraphs H of G that satisfy the following conditions:

(I)

$d_H\left(x\right)\equiv 0$ (mod 2) for every $x\in V\left(H\right)$;

(II)

$V_0\left(H\right)\subseteq {\displaystyle \bigcup _{i\ge 3}^{△\left(G\right)}}{V}_i\left(G\right)\subseteq V\left(H\right)$;

(III)

$\left|E\right(b\left)\right|\le m+1$ for any branch $b\in B\left(G\right)\setminus B_H\left(G\right)$;

(IV)

$\left|E\right(b\left)\right|\le m$ for any branch $b\in B_1\left(G\right)$;

(V)

$d_G(H_1,H-H_1)\le m-1$ for every subgraph $H_1$ of H.

In 2002, Xiong and Liu gave the following result in [16]:

Theorem 8

([16]). Let G be a connected graph with at least three edges and $m\ge 2$ be an integer. Then $L^m\left(G\right)$ is Hamiltonian if and only if $E{U}_m\left(G\right)\ne \varnothing$.

Theorem 8 easily deduces the following corollary:

Corollary 3.

Let G be a connected graph with at least three edges. Then $L^2\left(G\right)$ is Hamiltonian if and only if $E{U}_2\left(G\right)\ne \varnothing$.

For a nonnegative integer k, let $F_m^k\left(G\right)$ denote the set of subgraphs H of G satisfying the following five conditions:

(I)

H is an even graph;

(II)

$V_0\left(H\right)\subseteq {\bigcup }_{i\ge 3}{V}_i\left(G\right)\subseteq V\left(H\right)$;

(III)

$\left|E\right(b\left)\right|\le m+1$ for any branch $b\in B\left(G\right)\setminus B_H\left(G\right)$;

(IV)

$\left|E\right(b\left)\right|\le m$ for any branch $b\in B_1\left(G\right)$;

(V)

H can be decomposed into at most k pairwise vertex-disjoint subgraphs $H_1,\cdots ,H_t$ ($t\le k$) such that for every j and for every induced subgraph F of $H_j$ with $\varnothing \ne V\left(F\right)⊊V\left(H_j\right)$, it holds $d_G(F,H_j-V\left(F\right))\le m$.

Lv and Xiong gave the following result in [17]:

Theorem 9

([17]). Let G be a connected graph with at least three edges and let m and k be positive integer. Then for $m\ge 1$, $L^m\left(G\right)$ has an even factor with at most k components if and only if $F_m^k\left(G\right)\ne \varnothing .$

Theorem 9 easily infers the following theorem:

Theorem 10

([17]). Let G be a connected graph with at least three edges. Then $L\left(G\right)$ has an even factor with at most k components if and only if $F_1^k\left(G\right)\ne \varnothing .$

Motivated by Xiong [11,14,15,16], we consider the Hamiltonian indices of three classes of graphs obtained from Petersen graph and the graphs obtained by adding an edge to these three classes of graphs. We obtain the following results:

Theorem 11.

Let G be the graph obtained by replacing every vertex of Petersen graph with a n-cycle, then $h\left(G\right)=2$.

Theorem 12.

Let G be the graph obtained by replacing every vertex of Petersen graph with a complete graph of order n, then $h\left(G\right)=2$.

Theorem 13.

Let G be the graph obtained by adding n pendant edges to each vertex of Petersen graph, then $h\left(G\right)=2$.

Theorem 14.

Let G be the graph obtained by replacing every vertex of Petersen graph with a n-cycle, for any two vertices $a,b\in V\left(G\right)$, then $h(G+ab)=2$.

Theorem 15.

Let G be the graph obtained by replacing every vertex of Petersen graph with a complete graph of order n, for any two vertices $a,b\in V\left(G\right)$, then $h(G+ab)=2$.

Theorem 16.

Let G be the graph obtained by adding n pendant edges to each vertex of Petersen graph, for any two vertices $a,b\in V\left(G\right)$, then $h(G+ab)=2$.

3. Proof of Main Results

Proof of Theorem 11.

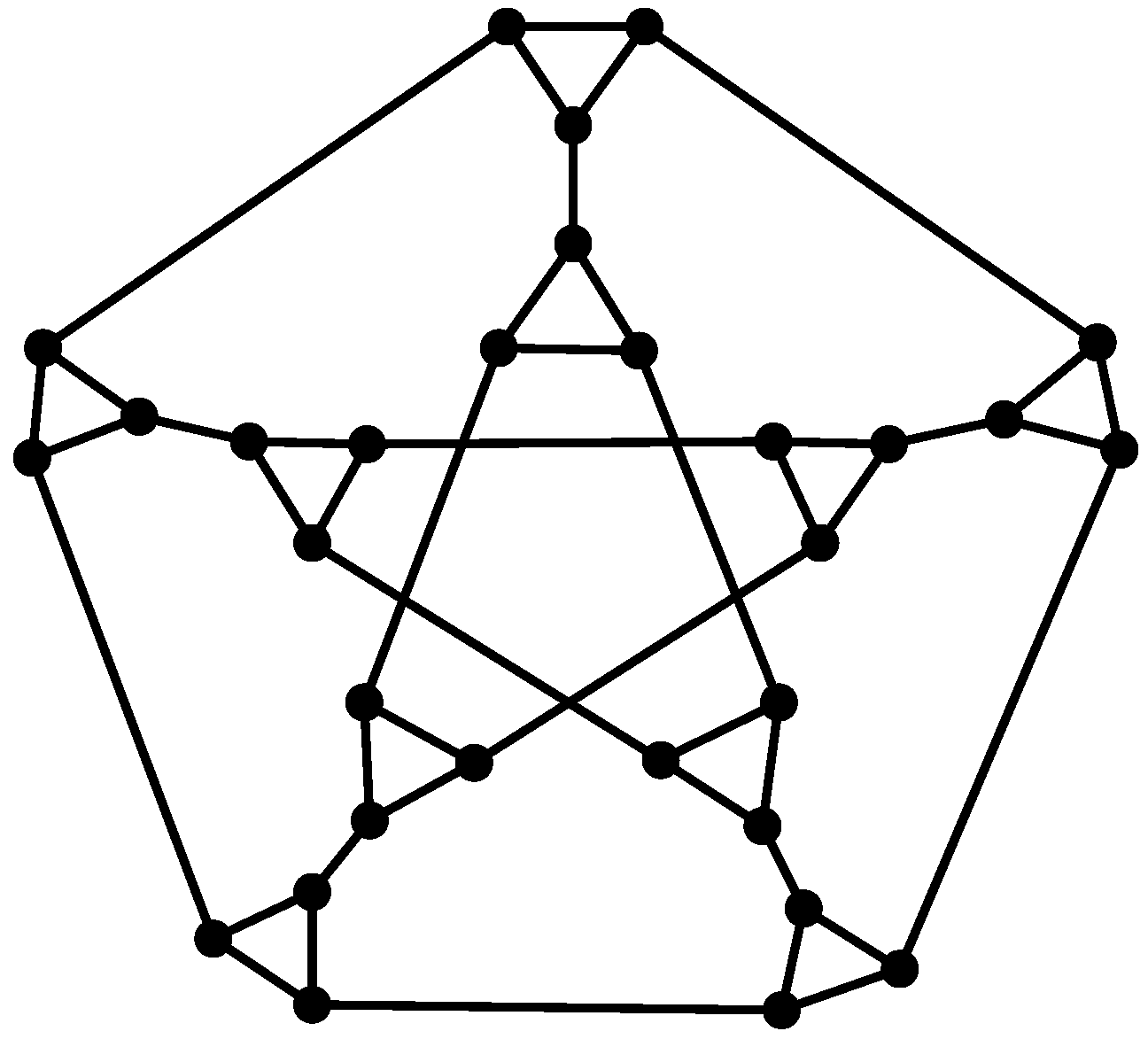
By Corollary 3, we only need to prove $E{U}_2\left(G\right)\ne \varnothing$. Let H be a subgraph of G such that $H\in E{U}_2\left(G\right)$. Next we prove that H satisfies (I)–(V) of $E{U}_2\left(G\right)$. Choose a subgraph H of G such that $H={\displaystyle \bigcup _{i=1}^{10}}{H}_i\left(G\right)$, every $H_i\left(G\right)$ is a n-cycle in G (Figure 2 is the situation when $n=3$). Then H satisfies that:

(I)

$d_H\left(x\right)\equiv 0$ (mod 2) for every $x\in V\left(H\right)$;

(II)

$V_0\left(H\right)\subseteq {\displaystyle \bigcup _{i\ge 3}^{△\left(G\right)}}{V}_i\left(G\right)\subseteq V\left(H\right)$.

Next, we prove that (III) holds. It is that obvious for every branch $b\in B\left(G\right)\setminus B_H\left(G\right)$, $\left|E\right(b\left)\right|=1\le 2+1=m+1$, where $m=2$. Then the condition (III) holds.

For the condition (IV), since there is no $B_1\left(G\right)$ in G, it is obvious that $\left|E\right(b\left)\right|=0\le 2=m$ for any branch $b\in B_1\left(G\right)$.

For the condition (V), we can take every $H_i\left(G\right)$ as $H_1$, then $d_G(H_1,H-H_1)=1\le 2-1=m-1$, where $m=2$. Then H satisfies condition (V).

Thus, $E{U}_2\left(G\right)\ne \varnothing$, then $L^2\left(G\right)$ is Hamiltonian, i.e., $h\left(G\right)\le 2$.

By Theorem 1, we only need to prove there is no DCT in G. See Figure 1, where $u_0{u}_1{u}_2{u}_3{v}_3{v}_1{v}_4{v}_2{v}_0{u}_0$ is one of the longest DCTs in Petersen graph. Therefore, when replacing $u_4$ by a n-cycle, not all edges on the n-cycle are dominated. Then there is no DCT in G, $h\left(G\right)\ne 1$. Through the structure of graph G, we can easily know there exists no Hamiltonian cycle in G, $h\left(G\right)\ne 0$.

Thus, $h\left(G\right)=2$. □

Proof of Theorem 12.

By Corollary 3, we only need to prove $E{U}_2\left(G\right)\ne \varnothing$. Let H be a subgraph of G such that $H\in E{U}_2\left(G\right)$. Next we prove that H satisfies (I)–(V) of $E{U}_2\left(G\right)$. Choose a subgraph H of G such that $H={\displaystyle \bigcup _{i=1}^{10}}{H}_i\left(G\right)$, every $H_i\left(G\right)$ is a n-cycle included in every complete graph of order n of G. Then H satisfies that:

(I)

$d_H\left(x\right)\equiv 0$ (mod 2) for every $x\in V\left(H\right)$;

(II)

$V_0\left(H\right)\subseteq {\displaystyle \bigcup _{i\ge 3}^{△\left(G\right)}}{V}_i\left(G\right)\subseteq V\left(H\right)$.

For the condition (III), it is obvious that for every branch $b\in B\left(G\right)\setminus B_H\left(G\right)$, $\left|E\right(b\left)\right|=1\le 2+1=m+1$, where $m=2$. Then the condition (III) holds.

For the condition (IV), since there is no $B_1\left(G\right)$ in G, it is obvious that $\left|E\right(b\left)\right|=0\le 2=m$ for any branch $b\in B_1\left(G\right)$.

For the condition (V), we can take every $H_i\left(G\right)$ as $H_1$, then $d_G(H_1,H-H_1)=1\le 2-1=m-1$, where $m=2$. Thus, (V) also holds.

Thus, $E{U}_2\left(G\right)\ne \varnothing$, then $L^2\left(G\right)$ is Hamiltonian, i.e., $h\left(G\right)\le 2$.

By Theorem 1, we only need to prove that there is no DCT in G. See Figure 1, where $u_0{u}_1{u}_2{u}_3{v}_3{v}_1{v}_4{v}_2{v}_0{u}_0$ is one of the longest DCTs in Petersen graph. Therefore, replacing $u_4$ by a complete graph of order n, all edges on the complete graph of order n are not dominated. Then there is no DCT in G, $h\left(G\right)\ne 1$. Through the structure of graph G, we can easily know there exists no Hamiltonian cycle in G, $h\left(G\right)\ne 0$.

Thus, $h\left(G\right)=2$. □

Proof of Theorem 13.

We also prove this result by Corollary 3. Let H be a subgraph of G such that $H\in E{U}_2\left(G\right)$. Next, we prove that H satisfies (I)–(V) of $E{U}_2\left(G\right)$. Choose a subgraph H of G such that $H={\displaystyle \bigcup _{i=1}^{10}}{H}_i\left(G\right)$, every $H_i\left(G\right)$ is one of the vertices of Petersen graph. Then H satisfies that:

(I)

$d_H\left(x\right)\equiv 0$ (mod 2) for every $x\in V\left(H\right)$;

(II)

$V_0\left(H\right)\subseteq {\displaystyle \bigcup _{i\ge 3}^{△\left(G\right)}}{V}_i\left(G\right)\subseteq V\left(H\right)$.

For the condition (III), it is clear that for every branch $b\in B\left(G\right)\setminus B_H\left(G\right)$, $\left|E\right(b\left)\right|=1\le 2+1=m+1$, where $m=2$. Then (III) holds.

For the condition (IV), it is obvious that $\left|E\right(b\left)\right|=1\le 2=m$ for any branch $b\in B_1\left(G\right)$, where $m=2$.

For the condition (V), we can take every $H_i\left(G\right)$ as $H_1$, then $d_G(H_1,H-H_1)=1\le 2-1=m-1$, where $m=2$. Then H satisfies condition (V).

Thus, $E{U}_2\left(G\right)\ne \varnothing$, then $L^2\left(G\right)$ is Hamiltonian, i.e., $h\left(G\right)\le 2$.

By Theorem 1, we only need to prove there is no DCT in G. See Figure 1, where $u_0{u}_1{u}_2{u}_3{v}_3{v}_1{v}_4{v}_2{v}_0{u}_0$ is one of the longest DCTs in Petersen graph. Therefore, adding n pendant edges to $u_4$, the n pendant edges on $u_4$ are not dominated. Then there is no DCT in G, $h\left(G\right)\ne 1$. Through the structure of graph G, we can easily know there exists no Hamiltonian cycle in G, $h\left(G\right)\ne 0$.

Thus, $h\left(G\right)=2$. □

Proof of Theorem 14.

Let $G^{\prime }=G+ab$.

By Theorem 10, we first need to prove $F_1^k\left(G^{\prime }\right)\ne \varnothing$. Let $H^{\prime }$ be a subgraph of $G^{\prime }$ such that $H^{\prime }\in F_1^k\left(G^{\prime }\right)$. Next we prove that $H^{\prime }$ satisfies (I)–(V) of $F_1^k\left(G^{\prime }\right)$. We distinguish the following two cases:

Case 1.

Adding an edge between any two vertices of any one n-cycle.

Choose a subgraph $H^{\prime }$ of $G^{\prime }$ such that $H^{\prime }={\displaystyle \bigcup _{i=1}^{10}}{H}_i^{\prime }\left(G^{\prime }\right)$, every $H_i^{\prime }\left(G^{\prime }\right)$ is a n-cycle in $G^{\prime }$.

In this case, it is obviously that $H^{\prime }$ satisfies (I)–(V) of $F_1^k\left(G^{\prime }\right)$.

Case 2.

Adding an edge between any two n-cycles.

Choose a subgraph $H^{\prime }$ of $G^{\prime }$ such that $H^{\prime }={\displaystyle \bigcup _{i=1}^{<10}}{H}_i^{\prime }\left(G^{\prime }\right)\bigcup H_i^{″}\left(G^{\prime }\right)$. $H_i^{\prime }\left(G^{\prime }\right)$ is any one n-cycle in $G^{\prime }$, $H_i^{″}\left(G^{\prime }\right)$ is the larger cycle obtained by two or more n-cycles and the edge $ab$.

In this case, it is obvious that $H^{\prime }$ satisfies that:

(I)

$H^{\prime }$ is an even graph;

(II)

$V_0\left(H^{\prime }\right)\subseteq {\bigcup }_{i\ge 3}{V}_i\left(G^{\prime }\right)\subseteq V\left(H^{\prime }\right)$.

Next, we prove that (III) holds. For every branch $b\in B\left(G^{\prime }\right)\setminus B_{H^{\prime }}\left(G^{\prime }\right)$, it is obvious that $\left|E\right(b\left)\right|=1\le 1+1=m+1$, where $m=1$. Then the condition (III) holds.

For the condition (IV), since there is no $B_1\left(G^{\prime }\right)$ in $G^{\prime }$, it is obviously that $\left|E\right(b\left)\right|=0\le 1=m$ for any branch $b\in B_1\left(G^{\prime }\right)$.

For the condition (V), we take $k=1$, thus $j=1$ in (V) of $F_n^k\left(G^{\prime }\right)$. For every induced subgraph F of $H_1^{\prime }$ with $\varnothing \ne V\left(F\right)⊊V\left(H_1^{\prime }\right)$, we can take every $H_i^{\prime }\left(G^{\prime }\right)$ or $H_i^{″}\left(G^{\prime }\right)$ as F, then $d_{G^{\prime }}(F,H_1^{\prime }-V\left(F\right))=1\le 1=m$, where $m=1$. Then $H^{\prime }$ satisfies condition (V).

Thus, $F_1^k\left(G^{\prime }\right)\ne \varnothing$, that is, $L\left(G^{\prime }\right)$ has an even factor with at most k components ($k=1$), then $s\left(G^{\prime }\right)\le 1$.

Since there exists no connected even factor in G, there exists no connected even factor in $G^{\prime }$, $s\left(G^{\prime }\right)\ne 0$. Thus, $s\left(G^{\prime }\right)=1$.

Then $h\left(G^{\prime }\right)=2$, i.e., $h(G+ab)=2$. □

Proof of Theorem 15.

Let $G^{\prime }=G+ab$.

By Theorem 10, we first need to prove $F_1^k\left(G^{\prime }\right)\ne \varnothing$. Let $H^{\prime }$ be a subgraph of $G^{\prime }$ such that $H^{\prime }\in F_1^k\left(G^{\prime }\right)$. Next, we prove that $H^{\prime }$ satisfies (I)–(V) of $F_1^k\left(G^{\prime }\right)$. We distinguish the following two cases:

Case 1.

Adding an edge between any two vertices of any one complete graph of order n of $G^{\prime }$.

Choose a subgraph $H^{\prime }$ of $G^{\prime }$ such that $H^{\prime }={\displaystyle \bigcup _{i=1}^{10}}{H}_i^{\prime }\left(G^{\prime }\right)$, every $H_i^{\prime }\left(G^{\prime }\right)$ is a n-cycle included in every complete graph of order n of $G^{\prime }$.

In this case, it is obviously that $H^{\prime }$ satisfies (I)–(V) of $F_1^k\left(G^{\prime }\right)$.

Case 2.

Adding an edge between any two complete graph of order n.

Choose a subgraph $H^{\prime }$ of $G^{\prime }$ such that $H^{\prime }={\displaystyle \bigcup _{i=1}^{<10}}{H}_i^{\prime }\left(G^{\prime }\right)\bigcup H_i^{″}\left(G^{\prime }\right)$. $H_i^{\prime }\left(G^{\prime }\right)$ is any one n-cycle included in every complete graph of order n of $G^{\prime }$, $H_i^{″}\left(G^{\prime }\right)$ is a larger cycle which consists of two or more n-cycles and the edge $ab$, where the n-cycles are included in any complete graph of order n of $G^{\prime }$.

In this case, clearly $H^{\prime }$ satisfies that:

(I)

$H^{\prime }$ is an even graph;

(II)

$V_0\left(H^{\prime }\right)\subseteq {\bigcup }_{i\ge 3}{V}_i\left(G^{\prime }\right)\subseteq V\left(H^{\prime }\right)$.

For the condition (III), it is obviously, for every branch $b\in B\left(G^{\prime }\right)\setminus B_{H^{\prime }}\left(G^{\prime }\right)$, $\left|E\right(b\left)\right|=1\le 1+1=m+1$, where $m=1$. Then (III) holds.

For the condition (IV), since there is no $B_1\left(G\right)$ in $G^{\prime }$, it is obviously that $\left|E\right(b\left)\right|=0\le 1=m$ for any branch $b\in B_1\left(G^{\prime }\right)$.

For the condition (V), we take $k=1$, thus $j=1$ in (V) of $F_n^k\left(G^{\prime }\right)$. For every induced subgraph F of $H_1^{\prime }$ with $\varnothing \ne V\left(F\right)⊊V\left(H_1^{\prime }\right)$, we can take every $H_i^{\prime }\left(G^{\prime }\right)$ or $H_i^{″}\left(G^{\prime }\right)$ as F, then $d_{G^{\prime }}(F,H_1^{\prime }-V\left(F\right))=1\le 1=m$, where $m=1$. Thus, (V) also holds.

Thus, $F_1^k\left(G^{\prime }\right)\ne \varnothing$, that is, $L\left(G^{\prime }\right)$ has an even factor with at most k components ($k=1$), then $s\left(G^{\prime }\right)\le 1$.

Since no connected even factor exists in G, there exists no connected even factor in $G^{\prime }$, $s\left(G^{\prime }\right)\ne 0$. Thus, $s\left(G^{\prime }\right)=1$.

Then $h\left(G^{\prime }\right)=2$, i.e., $h(G+ab)=2$. □

Proof of Theorem 16.

Let $G^{\prime }=G+ab$.

By Theorem 10, we first need to prove $F_1^k\left(G^{\prime }\right)\ne \varnothing$. Let $H^{\prime }$ be a subgraph of $G^{\prime }$ such that $H^{\prime }\in F_1^k\left(G^{\prime }\right)$. Next we prove that $H^{\prime }$ satisfies (I)–(V) of $F_1^k\left(G^{\prime }\right)$. We distinguish the following four cases. For convenience, we define those vertices of original Petersen graph in $G^{\prime }$ as center vertices of $G^{\prime }$.

Case 1.

a and b are both center vertices of $G^{\prime }$.

Choose a subgraph $H^{\prime }$ of $G^{\prime }$ such that $H^{\prime }={\displaystyle \bigcup _{i=1}^{10}}{H}_i^{\prime }\left(G^{\prime }\right)$, where every $H_i^{\prime }\left(G^{\prime }\right)$ is any one center vertex of $G^{\prime }$.

In this case, it is obvious that $H^{\prime }$ satisfies (I) (II) (IV) of $F_1^k\left(G^{\prime }\right)$.

For the condition (III), every branch in $B\left(G^{\prime }\right)\setminus B_{H^{\prime }}\left(G^{\prime }\right)$ is same with the branches in G except the branch $ab$. By the proof of Theorem 13, these branches satisfy the condition (III), and the length of the branch $ab$(denoted by $\left|E\right(ab\left)\right|$) with $\left|E\right(ab\left)\right|=1\le m+1=2$, where $m=1$.

For the condition (V), $k=1$, thus $j=1$. By the time, every $H_i^{\prime }\left(G^{\prime }\right)$ may be F of $H_1^{\prime }$, then $d_{G^{\prime }}(F,H_1^{\prime }-V\left(F\right))=1\le 1=m$, where $m=1$. Thus, (V) holds.

Case 2.

a and b are the end vertices of the different pendant edges with the same center vertex of $G^{\prime }$.

Choose a subgraph $H^{\prime }$ of $G^{\prime }$ such that $H^{\prime }={\displaystyle \bigcup _{i=1}^{<10}}{H}_i^{\prime }\left(G^{\prime }\right)\bigcup H_i^{″}\left(G^{\prime }\right)$. $H_i^{\prime }\left(G^{\prime }\right)$ is any one center vertex of $G^{\prime }$. $H_i^{″}\left(G^{\prime }\right)$ is a cycle obtained by two pendant edges with a common center vertex of $G^{\prime }$ and the edge $ab$.

Case 3.

a and b are the end vertices of the pendant edges with the difference center vertices of $G^{\prime }$.

Choose a subgraph $H^{\prime }$ of $G^{\prime }$ such that $H^{\prime }={\displaystyle \bigcup _{i=1}^{<10}}{H}_i^{\prime }\left(G^{\prime }\right)\bigcup H_i^{″}\left(G^{\prime }\right)$. $H_i^{\prime }\left(G^{\prime }\right)$ is any one center vertex of $G^{\prime }$. $H_i^{″}\left(G^{\prime }\right)$ is a cycle obtained by two pendant edges with different center vertices of $G^{\prime }$ and the edge $ab$.

Case 4.

a is one of center vertices of $G^{\prime }$, b is the end vertex of some one pendant edge of center vertices different from a in $G^{\prime }$.

Choose a subgraph $H^{\prime }$ of $G^{\prime }$ such that $H^{\prime }={\displaystyle \bigcup _{i=1}^{<10}}{H}_i^{\prime }\left(G^{\prime }\right)\bigcup H_i^{″}\left(G^{\prime }\right)$. $H_i^{\prime }\left(G^{\prime }\right)$ is any one center vertex of $G^{\prime }$. $H_i^{″}\left(G^{\prime }\right)$ is a cycle obtained by vertex a and another center vertex adjacent to b and the edge $ab$.

Next, we prove that $H^{\prime }$ satisfies (I)–(V) of $F_1^k\left(G^{\prime }\right)$ in cases 2–4.

In cases 2–4, $H^{\prime }$ satisfies that:

(I)

$H^{\prime }$ is an even graph;

(II)

$V_0\left(H^{\prime }\right)\subseteq {\bigcup }_{i\ge 3}{V}_i\left(G^{\prime }\right)\subseteq V\left(H^{\prime }\right)$.

For the condition (III), according to the choice of $H^{\prime }$ in any case, it is obviously, $\left|E\right(b\left)\right|=1\le 1+1=m+1$, where $m=1$ for every branch $b\in B\left(G^{\prime }\right)\setminus B_{H^{\prime }}\left(G^{\prime }\right)$. Then (III) holds.

For the condition (IV), it is obviously that $\left|E\right(b\left)\right|=1\le 1=m$ for any branch $b\in B_1\left(G^{{}^{\prime }}\right)$, where $m=1$.

For the condition (V), we take $k=1$, thus $j=1$ in (V) of $F_1^k\left(G^{\prime }\right)$. For every induced subgraph F of $H_1^{\prime }$ with $\varnothing \ne V\left(F\right)⊊V\left(H_1^{\prime }\right)$, we can take every $H_i^{\prime }\left(G^{\prime }\right)$ or $H_i^{″}\left(G^{\prime }\right)$ as F, then $d_{G^{\prime }}(F,H_1^{\prime }-V\left(F\right))=1\le 1=m$, where $m=1$. Thus, (V) also holds.

Thus, $F_1^k\left(G^{\prime }\right)\ne \varnothing$, that is, $L\left(G^{\prime }\right)$ has an even factor with at most k components ($k=1$), then $s\left(G^{\prime }\right)\le 1$.

Since there exists no connected even factor in G, there exists no connected even factor in $G^{\prime }$, $s\left(G^{\prime }\right)\ne 0$. Thus, $s\left(G^{\prime }\right)=1$.

Then $h\left(G^{\prime }\right)=2$, i.e., $h(G+ab)=2$. □

4. Concluding Remarks

Determining the Hamiltonian index $h\left(G\right)$ of a graph is NP-hard, so its research is meaningful. In this paper, we consider the Hamiltonian indices of three classes of graphs obtained from Petersen graph and the graphs obtained by adding an edge to these three classes of graphs. Unexpectedly, we found that the Hamiltonian indices of three types of graphs obtained from Petersen graph are the same, and we also found that the Hamiltonian indices of the graphs obtained by adding an edge to these three classes of graphs do not change. This makes us to think:

• Whether the Hamiltonian indices are the same for all classes of graphs obtained from Petersen graph?
• Whether the Hamiltonian indices are the same for the graphs obtained by adding an edge to all classes of graphs obtained from Petersen graph?
• What are the Hamilton-connected indices for above all types of graphs?

This will be the direction of our future research.