Dynamics in an n-Species Lotka–Volterra Cooperative System with Delays
Abstract
1. Introduction
2. Preliminaries
3. Main Results
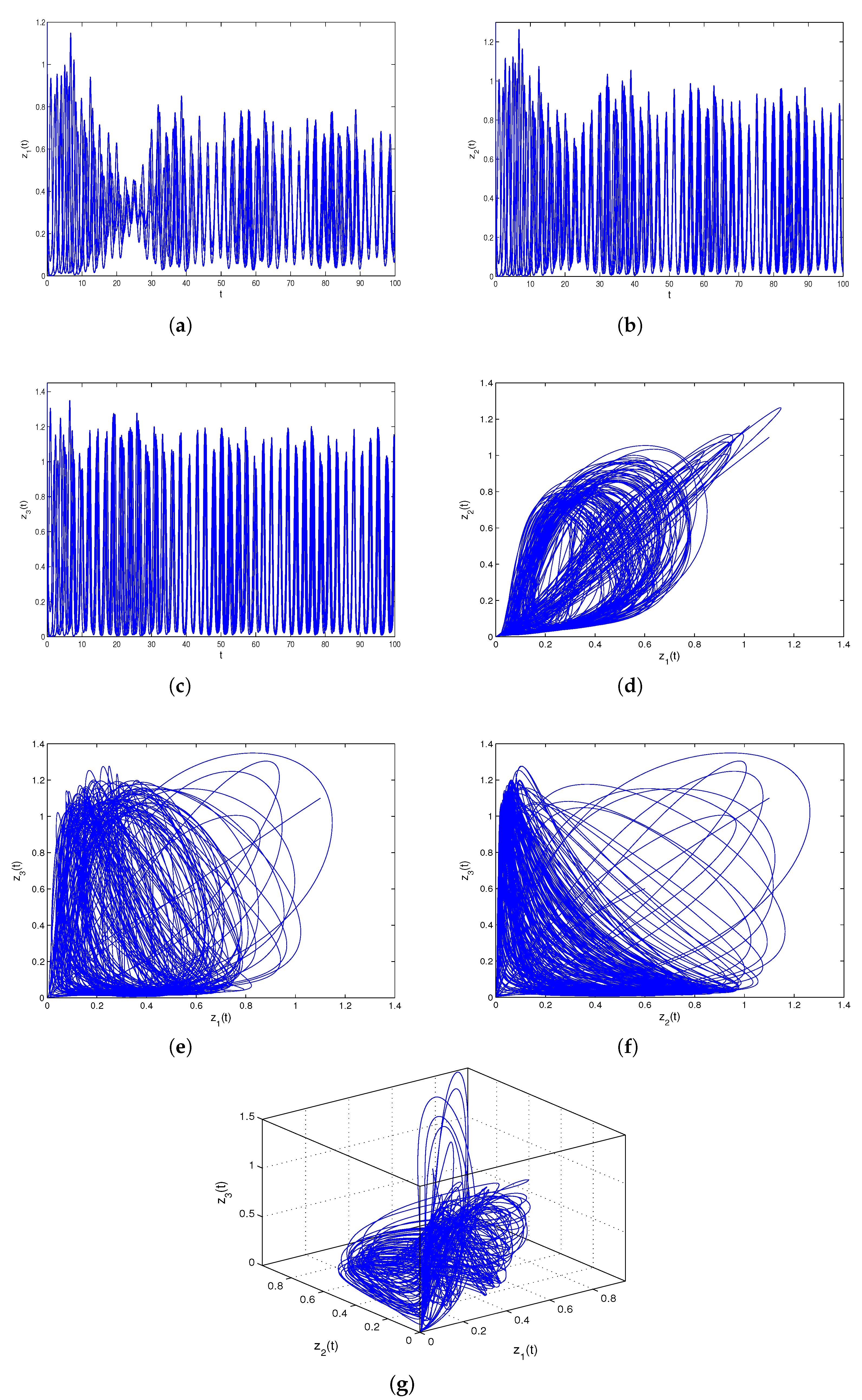
4. Examples
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Tang, S.; Xiao, Y.; Liang, J.; Wang, X. Matheynatical Biology; Science Press: Beijing, China, 2019. (In Chinese) [Google Scholar]
- Zhang, Z. Mutualism or cooperation among competitors promotes coexistence and competitive ability. Ecol. Model. 2003, 164, 271–282. [Google Scholar] [CrossRef]
- Chen, F.; Xie, X. A Study on the Dynamics of Cooperative Population Models; Science Press: Beijing, China, 2014. (In Chinese) [Google Scholar]
- Zouache, D.; Moussaoui, A.; Abdelaziz, F.B. A cooperative swarm intelligence algorithm for multi-objective discrete optimization with application to the knapsack problem. Eur. J. Oper. Res. 2008, 264, 74–88. [Google Scholar] [CrossRef]
- Heimerl, A.; Baur, T.; Lingenfelser, F.; Wagner, J.; Andre, E. Nova—A tool for explainable cooperative machine learning. In Proceedings of the 8th International Conference on Affective Computing and Intelligent Interaction (ACII), Cambridge, UK, 3–6 September 2019; pp. 109–115. [Google Scholar] [CrossRef]
- Vidhate, D.; Kulkarni, P. Cooperative machine learning with information fusion for dynamic decision making in diagnostic applications. In Proceedings of the 2012 International Conference on Advances in Mobile Network, Communication and Its Applications, Bangalore, India, 1–2 August 2012; pp. 70–74. [Google Scholar] [CrossRef]
- Abdurahman, X.; Teng, Z. On the persistence of a nonautonomous n-species Lotka–Volterra cooperative system. Appl. Math. Comput. 2004, 152, 885–895. [Google Scholar] [CrossRef]
- Enatsu, Y. Permanence for multi-species nonautonomous Lotka–Volterra cooperative systems. Aip Conf. Proc. 2009, 1124, 109–118. [Google Scholar]
- Lu, G.; Lu, Z.; Enatsu, Y. Permanence for Lotka–Volterra systems with multiple delays. Nonlinear Anal. Real World Appl. 2011, 12, 2552–2560. [Google Scholar] [CrossRef]
- Chen, L.; Xie, X. Permanence of an N-species cooperation system with continuous time delays and feedback controls. Nonlinear Anal. Real World Appl. 2011, 12, 34–38. [Google Scholar] [CrossRef]
- Abdurahman, X.; Teng, Z. Persistence and Extinction for General Non-autonomous n-Species Lotka–Volterra Cooperative Systems with Delays. Stud. Appl. Math. 2007, 118, 17–43. [Google Scholar] [CrossRef]
- Lu, S. On the existence of positive periodic solutions to a Lotka Volterra cooperative population model with multiple delays. Nonlinear Anal. 2008, 68, 1746–1753. [Google Scholar] [CrossRef]
- Liao, X.; Yang, X.; Huang, T. Persistence of delayed cooperative models: Impulsive control method. Appl. Math. Comput. 2019, 342, 130–146. [Google Scholar] [CrossRef]
- Mukherjee, D. Permanence and global attractivity for facultative mutualism system with delay. Math. Methods Appl. Sci. 2003, 26, 1–9. [Google Scholar] [CrossRef]
- Lin, S.; Lu, Z. Permanence for two-species Lotka–Volterra systems with delays. Math. Biosci. Eng. 2006, 3, 137–144. [Google Scholar] [CrossRef] [PubMed]
- Lu, G.; Lu, Z. Permanence for two-species Lotka–Volterra cooperative systems with delays. Math. Biosci. Eng. 2008, 5, 477–484. [Google Scholar] [PubMed]
- Nakata, Y. Permanence for the Lotka–Volterra cooperative system with several delays. Int. J. Biomath. 2009, 2, 267–285. [Google Scholar] [CrossRef]
- Lu, G.; Lu, Z.; Lian, X. Delay effect on the permanence for Lotka–Volterra cooperative systems. Nonlinear Anal. Real World Appl. 2010, 11, 2810–2816. [Google Scholar] [CrossRef]
- Chen, L.; Lu, Z.; Wang, W. The effect of delays on the permanence for Lotka–Volterra systems. Appl. Math. Lett. 1995, 8, 71–73. [Google Scholar] [CrossRef]
- Zhao, L.; Qin, B.; Chen, F. Permanence and global stability of a May cooperative system with strong and weak cooperative partners. Adv. Differ. Equ. 2018, 2018, 172. [Google Scholar] [CrossRef]
- Jiang, Z.; Muhammadhaji, A.; Hu, C.; Teng, Z. Dynamics of N-Species cooperation models with feedback controls and continuous delays. Qual. Theory Dyn. Syst. 2023, 22, 41. [Google Scholar] [CrossRef]
- Muhammadhaji, A. Dynamics of a predator-prey-competition system with pured elays. Differ. Equ. Dyn. Syst. 2022, 30, 35–49. [Google Scholar] [CrossRef]
- Gaines, R.E.; Mawhin, J.L. Coincidence Degree and Nonlinear Differential Equations; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Agarwal, R.P.; Hristova, S. Ulam-type stability for a boundary-value problem for multi-term delay fractional differential equations of Caputo type. Axioms 2022, 11, 742. [Google Scholar] [CrossRef]
- Wattanakejorn, V.; Karthikeyann, P.; Poornima, S.; Karthikeyan, K.; Sitthiwirattham, T. Existence solutions for implicit fractional relaxation differential equations with impulsive delay boundary conditions. Axioms 2022, 11, 611. [Google Scholar] [CrossRef]
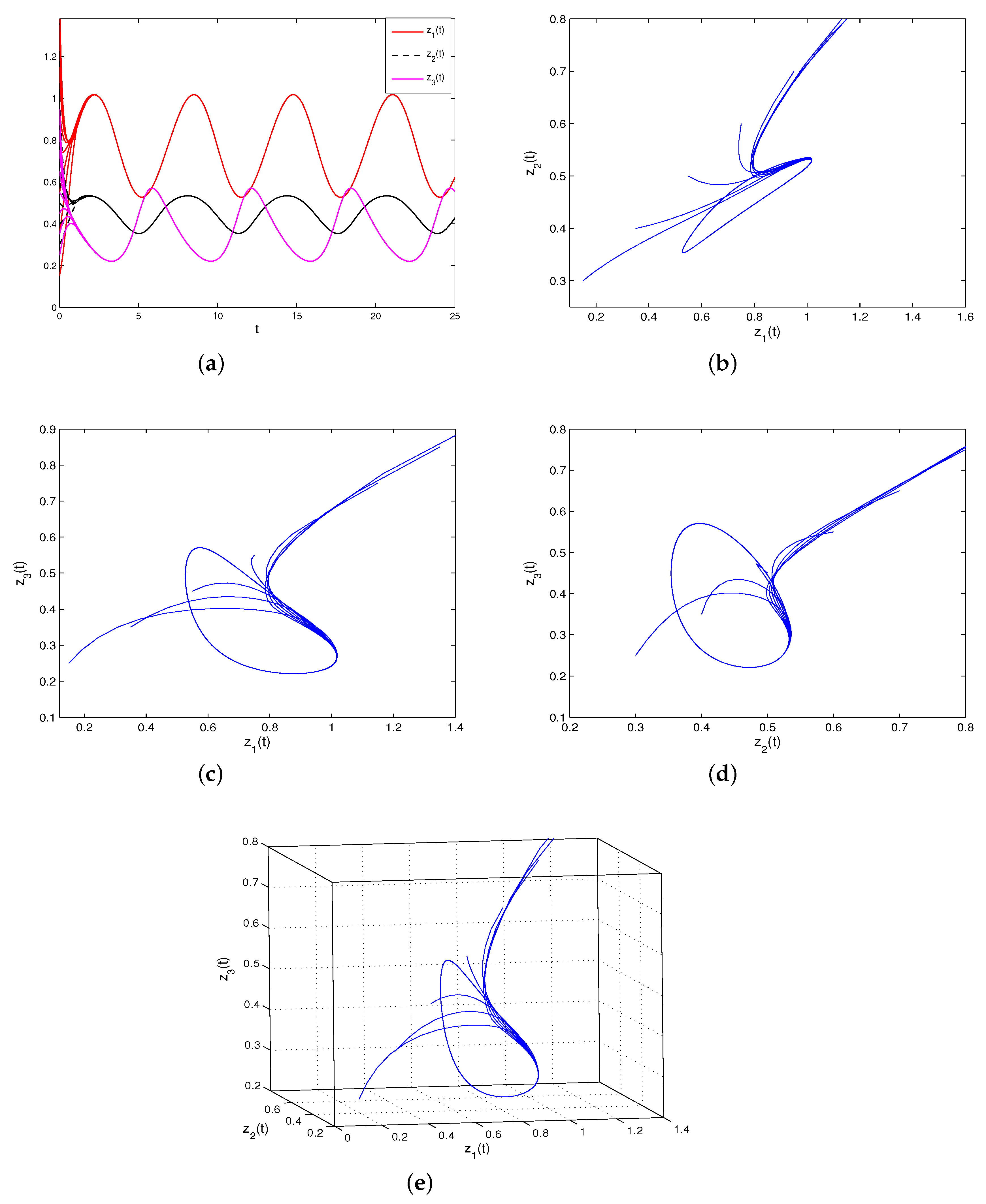
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, Z.; Halik, A.; Muhammadhaji, A. Dynamics in an n-Species Lotka–Volterra Cooperative System with Delays. Axioms 2023, 12, 501. https://doi.org/10.3390/axioms12050501
Jiang Z, Halik A, Muhammadhaji A. Dynamics in an n-Species Lotka–Volterra Cooperative System with Delays. Axioms. 2023; 12(5):501. https://doi.org/10.3390/axioms12050501
Chicago/Turabian StyleJiang, Zhao, Azhar Halik, and Ahmadjan Muhammadhaji. 2023. "Dynamics in an n-Species Lotka–Volterra Cooperative System with Delays" Axioms 12, no. 5: 501. https://doi.org/10.3390/axioms12050501
APA StyleJiang, Z., Halik, A., & Muhammadhaji, A. (2023). Dynamics in an n-Species Lotka–Volterra Cooperative System with Delays. Axioms, 12(5), 501. https://doi.org/10.3390/axioms12050501





