Two Novel Computational Techniques for Solving Nonlinear Time-Fractional Lax’s Korteweg-de Vries Equation
Abstract
1. Introduction
2. Preliminaries
3. General Implementation of HPTM
4. General Implementation of the YTDM
5. Application
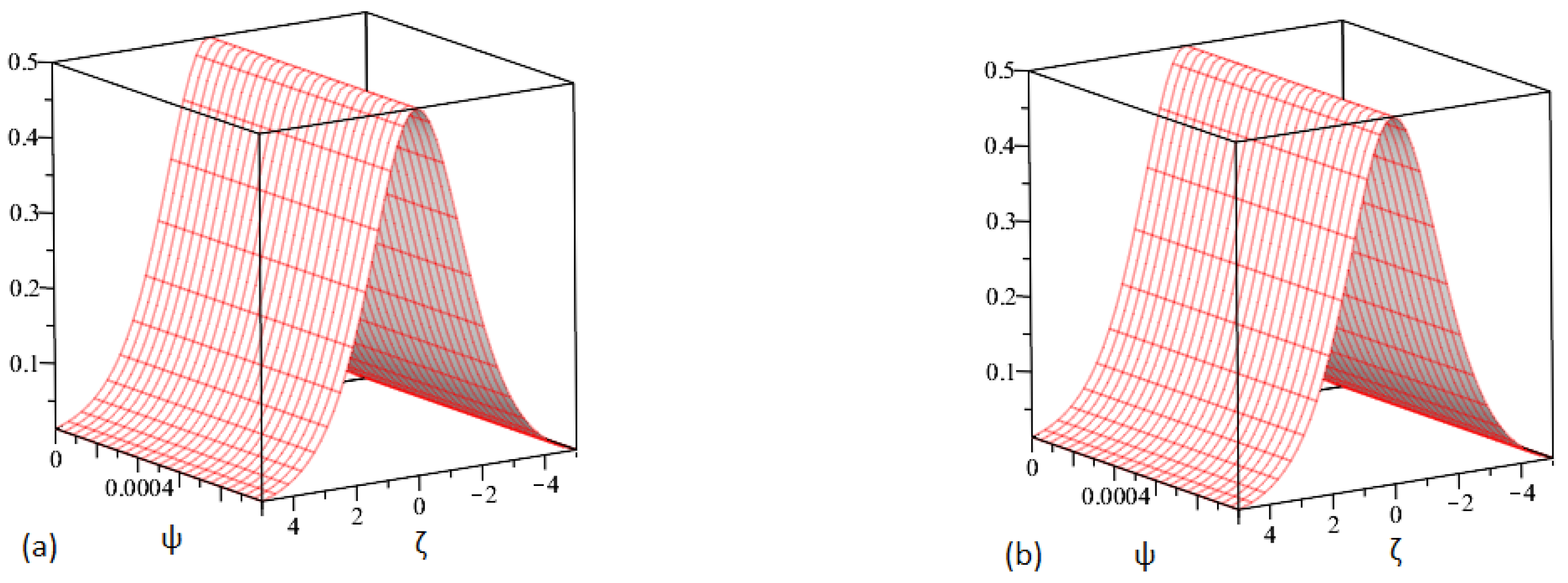
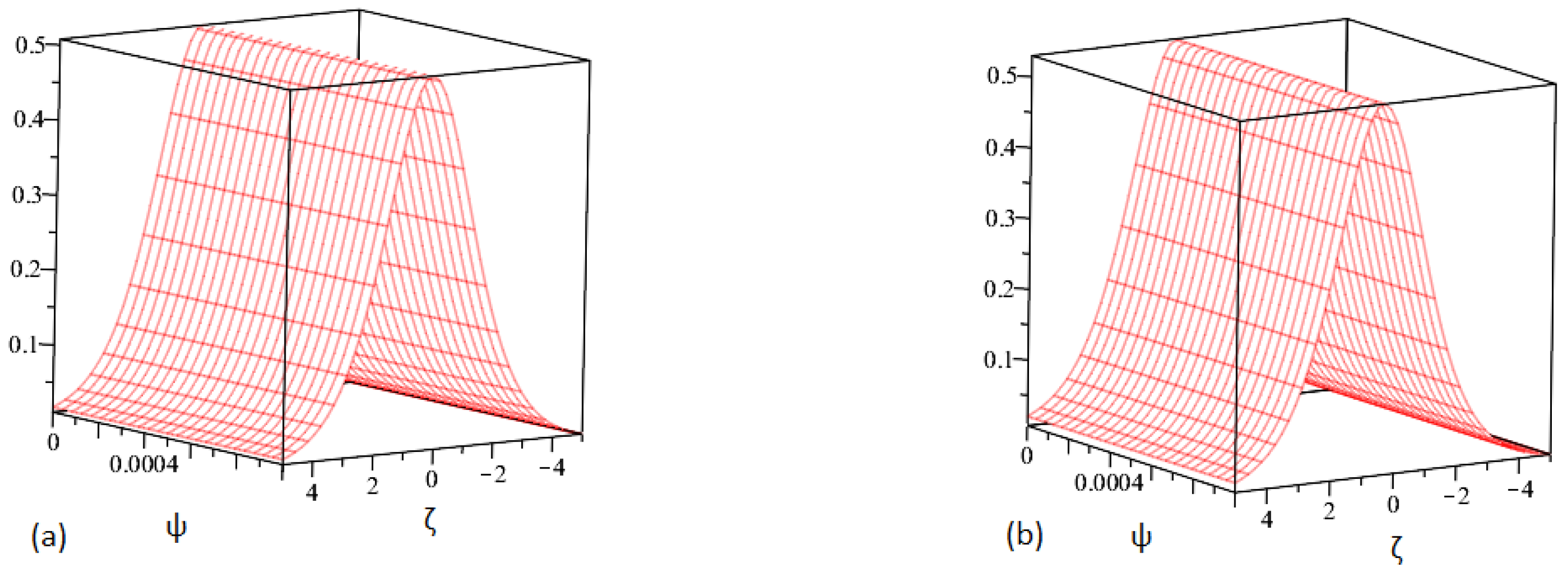
6. Numerical Simulation Studies
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Goyal, M.; Baskonus, H.M.; Prakash, A. An efficient technique for a time fractional model of lassa hemorrhagic fever spreading in pregnant women. Eur. Phys. J. Plus 2019, 134, 482. [Google Scholar] [CrossRef]
- Alaoui, K.M.; Nonlaopon, K.; Zidan, A.M.; Khan, A.; Shah, R. Analytical investigation of fractional-order Cahn-Hilliard and gardner equations using two novel techniques. Mathematics 2022, 10, 1643. [Google Scholar] [CrossRef]
- Ganie, A.H. New Bounds For Variables of Fractional Order. Pak. J. Stat. 2022, 38, 211–218. [Google Scholar]
- Podlubny, I. Fractional Differential Equations; Academic Press: New York, NY, USA, 1999. [Google Scholar]
- Hilfer, R. (Ed.) Applications of Fractional Calculus in Physics; World Scientific Publishing Company: Singapore; Hackensack, NJ, USA; Hong Kong, China, 2000; pp. 87–130. [Google Scholar]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations; Elsevier: Amsterdam, The Netherlands, 2006. [Google Scholar]
- Chen, W. An Intuitive Study of Fractional Derivative Modeling and Fractional Quantum in Soft Matter. J. Vib. Control 2008, 14, 1651–1657. [Google Scholar] [CrossRef]
- Acioli, P.S.; Xavier, F.A.; Moreira, D.M. Mathematical Model Using Fractional Derivatives Applied to the Dispersion of Pollutants in the Planetary Boundary Layer. Bound.-Layer Meteorol. 2019, 170, 285–304. [Google Scholar] [CrossRef]
- Jamil, B.; Anwar, M.S.; Rasheed, A.; Irfan, M. MHD Maxwell flow modeled by fractional derivatives with chemical reaction and thermal radiation. Chin. J. Phys. 2020, 67, 512–533. [Google Scholar] [CrossRef]
- Fellah, M.; Fellah, Z.E.A.; Mitri, F.; Ogam, E.; Depollier, C. Transient ultrasound propagation in porous media using Biot theory and fractional calculus: Application to human cancellous bone. J. Acoust. Soc. Am. 2013, 133, 1867–1881. [Google Scholar] [CrossRef]
- Yadav, R.P.; Verma, R. A numerical simulation of fractional order mathematical modeling of COVID-19 disease in case of Wuhan China. Chaos Solitons Fractals 2020, 140, 110124. [Google Scholar] [CrossRef]
- Esen, A.; Sulaiman, T.A.; Bulut, H.; Baskonus, H.M. Optical solitons and other solutions to the conformable space-time fractional Fokas-Lenells equation. Optik 2018, 167, 150–156. [Google Scholar] [CrossRef]
- Baleanu, D.; Guvenc, Z.B.; Tenreiro Machado, J.A. New Trends in Nanotechnology and Fractional Calculus Applications; Springer: Dordrecht, The Netherlands; Heidelberg, Germany; London, UK; New York, NY, USA, 2010. [Google Scholar]
- Baleanu, D.; Wu, G.-C.; Zeng, S.-D. Chaos analysis and asymptotic stability of generalized Caputo fractional differential equations. Chaos Solitons Fractals 2017, 102, 99–105. [Google Scholar] [CrossRef]
- Eslami, M.; Vajargah, B.F.; Mirzazadeh, M.; Biswas, A. Application of first integral method to fractional partial differential equations. Indian J. Phys. 2014, 88, 177–184. [Google Scholar] [CrossRef]
- Younis, M.; Iftikhar, M. Computational examples of a class of fractional order nonlinear evolution equations using modified extended direct algebraic method. J. Comput. Methods 2015, 15, 359–365. [Google Scholar] [CrossRef]
- Gaber, A.A.; Aljohani, A.F.; Ebaid, A.; Machado, J.T. The generalized Kudryashov method for nonlinear space-time fractional partial differential equations of Burgers type. Nonlinear Dyn. 2019, 95, 361–368. [Google Scholar] [CrossRef]
- Meerschaert, M.M.; Tadjeran, C. Finite difference approximations for two-sided space-fractional partial differential equations. Appl. Numer. Math. 2006, 56, 80–90. [Google Scholar] [CrossRef]
- Sarwar, S.; Alkhalaf, S.; Iqbal, S.; Zahid, M.A. A note on optimal homotopy asymptotic method for the solutions of fractional order heat-and wave-like partial differential equations. Comput. Math. Appl. 2015, 70, 942–953. [Google Scholar] [CrossRef]
- El-Wakil, S.A.; Elhanbaly, A.; Abdou, M.A. Adomian decomposition method for solving fractional nonlinear differential equations. Appl. Math. Comput. 2006, 182, 313–324. [Google Scholar] [CrossRef]
- Uddin, M.F.; Hafez, M.G.; Hwang, I.; Park, C. Effect of Space Fractional Parameter on Nonlinear Ion Acoustic Shock Wave Excitation in an Unmagnetized Relativistic Plasma. Front. Phys. 2022, 9, 766035. [Google Scholar] [CrossRef]
- Mishra, N.K.; AlBaidani, M.M.; Khan, A.; Ganie, A.H. Numerical Investigation of Time-Fractional Phi-Four Equation via Novel Transform. Symmetry 2023, 15, 687. [Google Scholar] [CrossRef]
- Fathima, D.; Alahmadi, R.A.; Khan, A.; Akhter, A.; Ganie, A.H. An Efficient Analytical Approach to Investigate Fractional Caudrey-Dodd-Gibbon Equations with Non-Singular Kernel Derivatives. Symmetry 2023, 15, 850. [Google Scholar] [CrossRef]
- Nonlaopon, K.; Alsharif, A.M.; Zidan, A.M.; Khan, A.; Hamed, Y.S.; Shah, R. Numerical investigation of fractional-order Swift-Hohenberg equations via a Novel transform. Symmetry 2021, 13, 1263. [Google Scholar] [CrossRef]
- Wang, L.; Ma, Y.; Meng, Z. Haar wavelet method for solving fractional partial differential equations numerically. Appl. Math. Comput. 2014, 227, 66–76. [Google Scholar] [CrossRef]
- Zheng, B.; Wen, C. Exact solutions for fractional partial differential equations by a new fractional sub-equation method. Adv. Differ. Equ. 2013, 2013, 1–12. [Google Scholar] [CrossRef]
- Odibat, Z.; Momani, S. A generalized differential transform method for linear partial differential equations of fractional order. Appl. Math. Lett. 2008, 21, 194–199. [Google Scholar] [CrossRef]
- Ali, U.; Naeem, M.; Alahmadi, R.; Abdullah, F.A.; Khan, M.A.; Ganie, A.H. An investigation of a closed-form solution for non-linear variable-order fractional evolution equations via the fractional Caputo derivative. Front. Phys. 2023, 11, 73. [Google Scholar] [CrossRef]
- Alyobi, S.; Shah, R.; Khan, A.; Shah, N.A.; Nonlaopon, K. Fractional Analysis of Nonlinear Boussinesq Equation under Atangana-Baleanu-Caputo Operator. Symmetry 2022, 14, 2417. [Google Scholar] [CrossRef]
- Korteweg, D.J.; de Vries, G. On the change of form of long waves advancing in a rectangular canal and on a new type of long stationary waves. Philos. Mag. 1895, 39, 422–443. [Google Scholar] [CrossRef]
- Fung, M.K. KdV equation as an Euler–Poincare equation. Chin. J. Phys. 1997, 35, 789–796. [Google Scholar]
- Elwakil, S.A.; Abulwafa, E.M.; Zahran, M.A.; Mahmoud, A.A. Time-fractional KdV equation: Formulation and solution using variational methods. Nonlinear Dyn. 2011, 65, 55–63. [Google Scholar] [CrossRef]
- Sukhinov, A. The Construction and Research of the Modified Upwind Leapfrog Difference Scheme with Improved Dispersion Properties for the Korteweg-de Vries Equation. Mathematics 2022, 10, 2922. [Google Scholar] [CrossRef]
- Salnikov, N.N. On construction of finite-dimensional mathematical model of convection-diffusion process with usage of the PetrovGalerkin method. J. Autom. Inf. Sci. 2010, 42, 67–83. [Google Scholar] [CrossRef]
- Salnikov, N.N. Analysis of lumped approximations in the finite-element method for convection-diffusion problems. Cybern. Syst. Anal. 2013, 49, 774–784. [Google Scholar]
- Siryk, S.V.; Salnikov, N.N. Numerical solution of Burgers equation by Petrov-Galerkin method with adaptive weighting functions. J. Autom. Inf. Sci. 2012, 44, 50–67. [Google Scholar] [CrossRef]
- Kashkool, H.A. Space-Time Petrov-Discontinuous Galerkin Finite Element Method for Solving Linear Convection-Diffusion Problems. J. Phys. Conf. Ser. 2022, 2322, 012007. [Google Scholar]
- Kashkool, H.A. hp-discontinuous Galerkin Finite Element Method for Incompressible Miscible Displacement in Porous Media. J. Phys. Conf. Ser. 2020, 1530, 012001. [Google Scholar] [CrossRef]
- Akinyemi, L. q-Homotopy analysis method for solving the seventh-order time-fractional Lax’s Korteweg-de Vries and Sawada-Kotera equations. Comput. Appl. Math. 2019, 38, 191. [Google Scholar] [CrossRef]
- Soliman, A.A. A numerical simulation and explicit solutions of KdV-Burgers and Lax’s seventh-order KdV equations. Chaos Solitons Fractals 2006, 29, 294–302. [Google Scholar] [CrossRef]
- Salas, A.H.; Gómez, S.; Cesar, A. Application of the Cole-Hopf transformation for finding exact solutions to several forms of the seventh-order KdV equation. Math. Probl. Eng. 2010, 2010, 194329. [Google Scholar] [CrossRef]
- Senol, M.; Tasbozan, O.; Kurt, A. Comparison of two reliable methods to solve fractional Rosenau-Hyman equation. Math. Methods Appl. Sci. 2021, 44, 7904–7914. [Google Scholar] [CrossRef]
- He, J.H. Homotopy perturbation technique. Compt. Meth. Appl. Mech. Eng. 1999, 178, 257–262. [Google Scholar] [CrossRef]
- He, J.H. A new perturbation technique which is also valid for large parameters. J. Sound Vib. 2000, 229, 1257–1263. [Google Scholar] [CrossRef]
- Rashidi, M.M.; Ganji, D.D.; Dinarvand, S. Explicit analytical solutions of the generalized Burger and Burger-Fisher equations by homotopy perturbation method. Numer. Meth. 2009, 25, 409–417. [Google Scholar] [CrossRef]
- Rashidi, M.M.; Ganji, D.D. Homotopy Perturbation Combined with Padé Approximation for Solving Two Dimensional Viscous Flow in the Extrusion Process. Int. J. Nonlinear Sci. 2009, 7, 387–394. [Google Scholar]
- Yildirim, A. An algorithm for solving the fractional nonlinear Schrödinger equation by means of the homotopy perturbation method. Int. J. Nonlinear Sci. Numer. Simul. 2009, 10, 445–451. [Google Scholar] [CrossRef]
- Kumar, S.; Singh, O.P. Numerical Inversion of the Abel Integral Equation using Homotopy Perturbation Method. Z. Naturforschung 2010, 65, 677–682. [Google Scholar] [CrossRef]
- Alaoui, M.K.; Fayyaz, R.; Khan, A.; Shah, R.; Abdo, M.S. Analytical investigation of Noyes-Field model for time-fractional Belousov-Zhabotinsky reaction. Complexity 2021, 2021, 3248376. [Google Scholar] [CrossRef]
- Sunthrayuth, P.; Alyousef, H.A.; El-Tantawy, S.A.; Khan, A.; Wyal, N. Solving Fractional-Order Diffusion Equations in a Plasma and Fluids via a Novel Transform. J. Funct. Spaces 2022, 2022, 1899130. [Google Scholar] [CrossRef]
- Zidan, A.M.; Khan, A.; Shah, R.; Alaoui, M.K.; Weera, W. Evaluation of time-fractional Fisher’s equations with the help of analytical methods. AIMS Math. 2022, 7, 18746–18766. [Google Scholar] [CrossRef]
- Yang, X.J.; Baleanu, D.; Srivastava, H.M. Local Fractional Integral Transforms and Their Applications; Academic Press: Cambridge, MA, USA, 2015. [Google Scholar]


| 0.2 | 0.31959022 | 0.31904353 | 0.31850296 | 0.31796602 | 0.31796602 | |
| 0.4 | 0.31512294 | 0.31405704 | 0.31300306 | 0.31195618 | 0.31195618 | |
| 0.01 | 0.6 | 0.30682292 | 0.30528988 | 0.30377397 | 0.30226828 | 0.30226828 |
| 0.8 | 0.29509495 | 0.29316615 | 0.29125890 | 0.28936453 | 0.28936453 | |
| 1 | 0.28048302 | 0.27824242 | 0.27602684 | 0.27382622 | 0.27382622 | |
| 0.2 | 0.31961056 | 0.31905702 | 0.31851156 | 0.31797133 | 0.31797133 | |
| 0.4 | 0.31516260 | 0.31408335 | 0.31301984 | 0.31196655 | 0.31196655 | |
| 0.02 | 0.6 | 0.30687997 | 0.30532771 | 0.30379811 | 0.30228320 | 0.30228320 |
| 0.8 | 0.29516673 | 0.29321375 | 0.29128927 | 0.28938330 | 0.28938330 | |
| 1 | 0.28056640 | 0.27829770 | 0.27606212 | 0.27384803 | 0.27384803 | |
| 0.2 | 0.31962793 | 0.31906917 | 0.31851973 | 0.31797664 | 0.31797664 | |
| 0.4 | 0.31519647 | 0.31410702 | 0.31303577 | 0.31197691 | 0.31197691 | |
| 0.03 | 0.6 | 0.30692867 | 0.30536176 | 0.30382101 | 0.30229811 | 0.30229811 |
| 0.8 | 0.29522800 | 0.29325659 | 0.29131809 | 0.28940207 | 0.28940207 | |
| 1 | 0.28063758 | 0.27834747 | 0.27609560 | 0.27386984 | 0.27386984 | |
| 0.2 | 0.31964361 | 0.31908051 | 0.31852763 | 0.31798194 | 0.31798194 | |
| 0.4 | 0.31522704 | 0.31412914 | 0.31305116 | 0.31198727 | 0.31198727 | |
| 0.04 | 0.6 | 0.30697264 | 0.30539357 | 0.30384315 | 0.30231302 | 0.30231302 |
| 0.8 | 0.29528332 | 0.29329662 | 0.29134595 | 0.28942083 | 0.28942083 | |
| 1 | 0.28070184 | 0.27839397 | 0.27612796 | 0.27389165 | 0.27389165 | |
| 0.2 | 0.31965814 | 0.31909129 | 0.31853532 | 0.31798723 | 0.31798723 | |
| 0.4 | 0.31525537 | 0.31415017 | 0.31306617 | 0.31199762 | 0.31199762 | |
| 0.05 | 0.6 | 0.30701339 | 0.30542381 | 0.30386474 | 0.30232792 | 0.30232792 |
| 0.8 | 0.29533460 | 0.29333466 | 0.29137311 | 0.28943959 | 0.28943959 | |
| 1 | 0.28076141 | 0.27843817 | 0.27615951 | 0.27391345 | 0.27391345 |
| 0.2 | 1.6241980000 | 1.0775117000 | 5.3693380000 | 3.5000000000 | 3.5000000000 | |
| 0.4 | 3.1667607000 | 2.1008643000 | 1.0468777000 | 3.0000000000 | 3.0000000000 | |
| 0.01 | 0.6 | 4.5546412000 | 3.0215991000 | 1.5056865000 | 2.8000000000 | 2.8000000000 |
| 0.8 | 5.7304317000 | 3.8016311000 | 1.8943820000 | 2.3000000000 | 2.3000000000 | |
| 1 | 6.6568000000 | 4.4161937000 | 2.2006227000 | 1.7000000000 | 1.7000000000 | |
| 0.2 | 1.6392279000 | 1.0856886000 | 5.4022870000 | 1.3800000000 | 1.3800000000 | |
| 0.4 | 3.1960548000 | 2.1167969000 | 1.0532916000 | 1.2700000000 | 1.2700000000 | |
| 0.02 | 0.6 | 4.5967682000 | 3.0445088000 | 1.5149057000 | 1.1000000000 | 1.1000000000 |
| 0.8 | 5.7834303000 | 3.8304514000 | 1.9059776000 | 9.1000000000 | 9.100000000 | |
| 1 | 6.7183635000 | 4.4496702000 | 2.2140901000 | 6.9000000000 | 6.9000000000 | |
| 0.2 | 1.6512893000 | 1.0925259000 | 5.4309060000 | 3.0900000000 | 3.0900000000 | |
| 0.4 | 3.2195538000 | 2.1301101000 | 1.0588540000 | 2.8400000000 | 2.8400000000 | |
| 0.03 | 0.6 | 4.6305573000 | 3.0636480000 | 1.5228971000 | 2.4900000000 | 2.4900000000 |
| 0.8 | 5.8259361000 | 3.8545254000 | 1.9160259000 | 2.0500000000 | 2.0500000000 | |
| 1 | 6.7677359000 | 4.4776312000 | 2.2257581000 | 1.5400000000 | 1.5400000000 | |
| 0.2 | 1.6616697000 | 1.0985706000 | 5.4568620000 | 5.4900000000 | 5.4900000000 | |
| 0.4 | 3.2397683000 | 2.1418713000 | 1.0638902000 | 5.0700000000 | 5.0700000000 | |
| 0.04 | 0.6 | 4.6596185000 | 3.0805511000 | 1.5301280000 | 4.4300000000 | 4.4300000000 |
| 0.8 | 5.8624910000 | 3.8757835000 | 1.9251149000 | 3.6400000000 | 3.6400000000 | |
| 1 | 6.8101937000 | 4.5023194000 | 2.2363100000 | 2.7300000000 | 2.7300000000 | |
| 0.2 | 1.6709106000 | 1.1040627000 | 5.4809170000 | 8.5800000000 | 8.5800000000 | |
| 0.4 | 3.2577540000 | 2.1525477000 | 1.0685486000 | 7.9200000000 | 7.9200000000 | |
| 0.05 | 0.6 | 4.6854706000 | 3.0958905000 | 1.5368119000 | 6.9300000000 | 6.9300000000 |
| 0.8 | 5.8950058000 | 3.8950718000 | 1.9335132000 | 5.6800000000 | 5.6800000000 | |
| 1 | 6.8479565000 | 4.5247175000 | 2.2460576000 | 4.2700000000 | 4.2700000000 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mishra, N.K.; AlBaidani, M.M.; Khan, A.; Ganie, A.H. Two Novel Computational Techniques for Solving Nonlinear Time-Fractional Lax’s Korteweg-de Vries Equation. Axioms 2023, 12, 400. https://doi.org/10.3390/axioms12040400
Mishra NK, AlBaidani MM, Khan A, Ganie AH. Two Novel Computational Techniques for Solving Nonlinear Time-Fractional Lax’s Korteweg-de Vries Equation. Axioms. 2023; 12(4):400. https://doi.org/10.3390/axioms12040400
Chicago/Turabian StyleMishra, Nidhish Kumar, Mashael M. AlBaidani, Adnan Khan, and Abdul Hamid Ganie. 2023. "Two Novel Computational Techniques for Solving Nonlinear Time-Fractional Lax’s Korteweg-de Vries Equation" Axioms 12, no. 4: 400. https://doi.org/10.3390/axioms12040400
APA StyleMishra, N. K., AlBaidani, M. M., Khan, A., & Ganie, A. H. (2023). Two Novel Computational Techniques for Solving Nonlinear Time-Fractional Lax’s Korteweg-de Vries Equation. Axioms, 12(4), 400. https://doi.org/10.3390/axioms12040400







