Turbulent Free Convection and Thermal Radiation in an Air-Filled Cabinet with Partition on the Bottom Wall
Abstract
1. Introduction
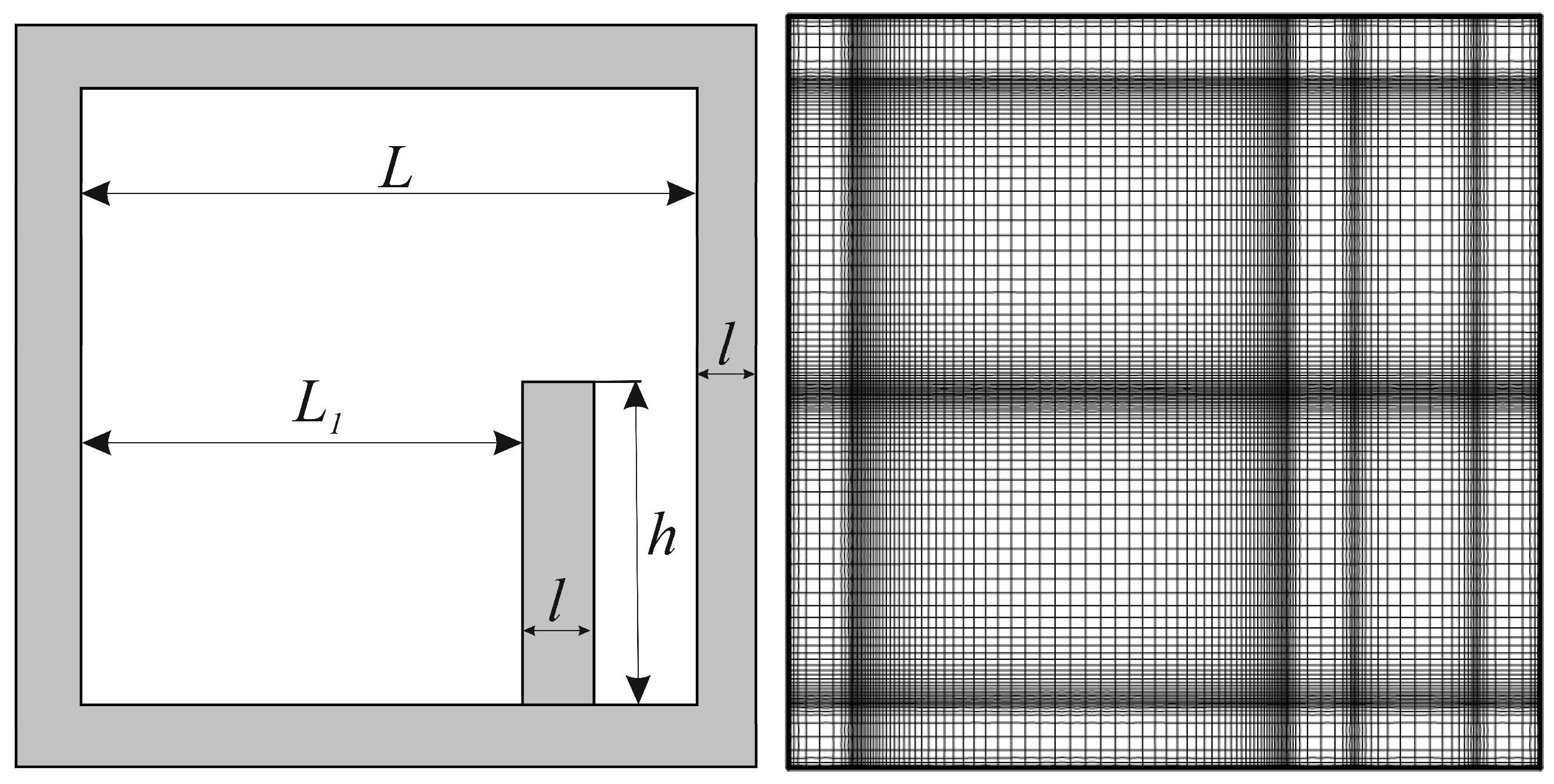
2. Governing Equations and Numerical Method
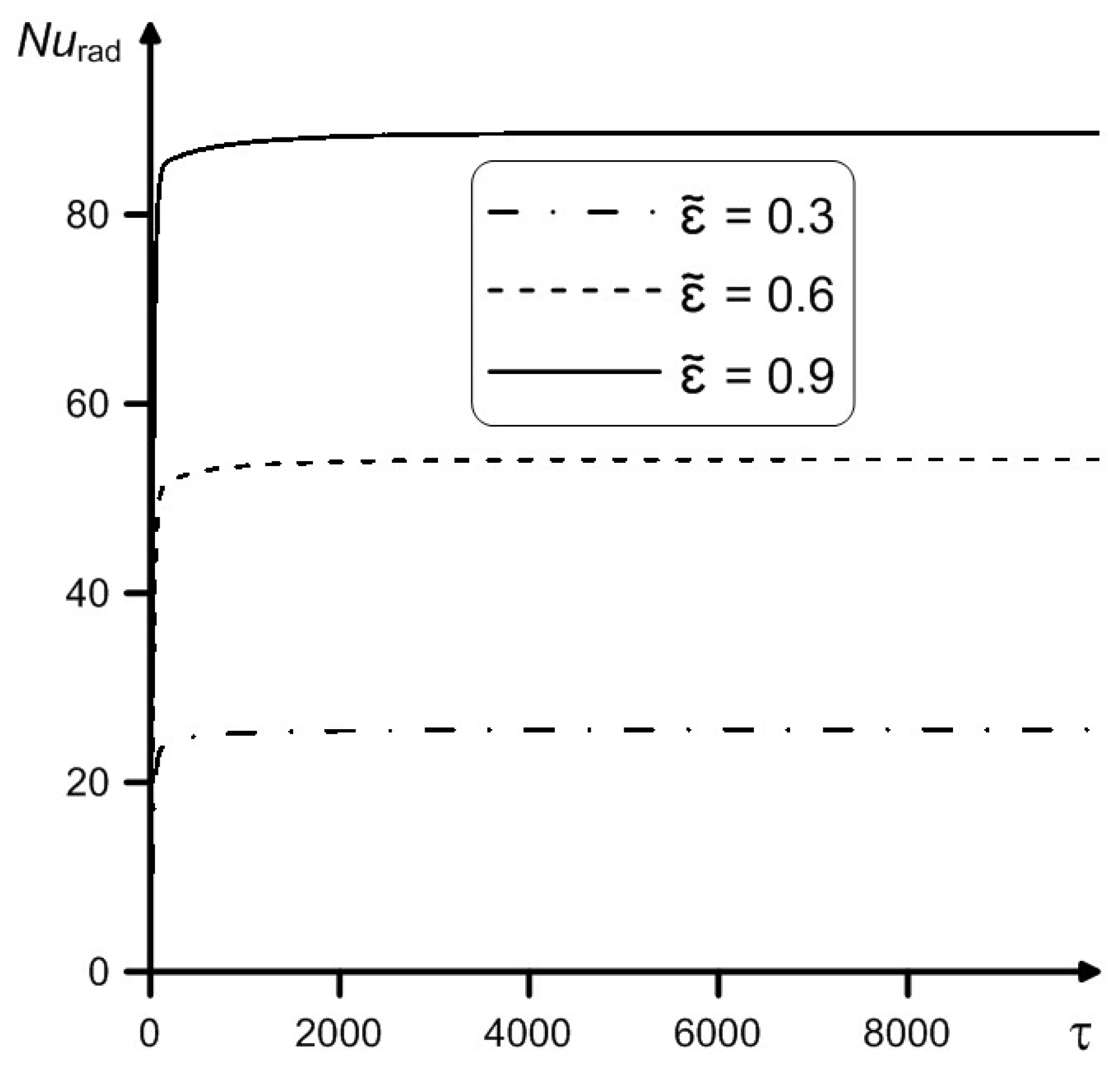
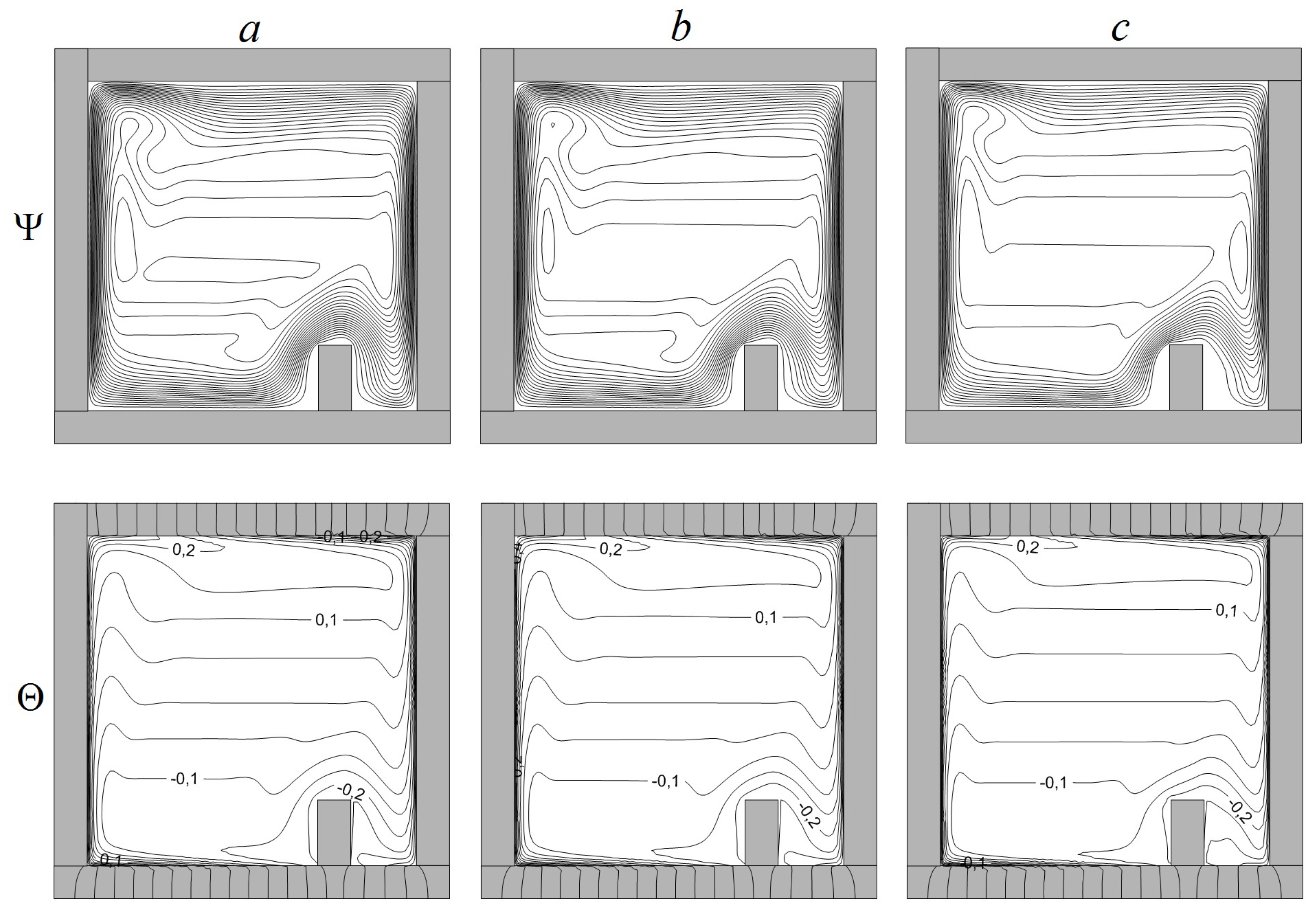
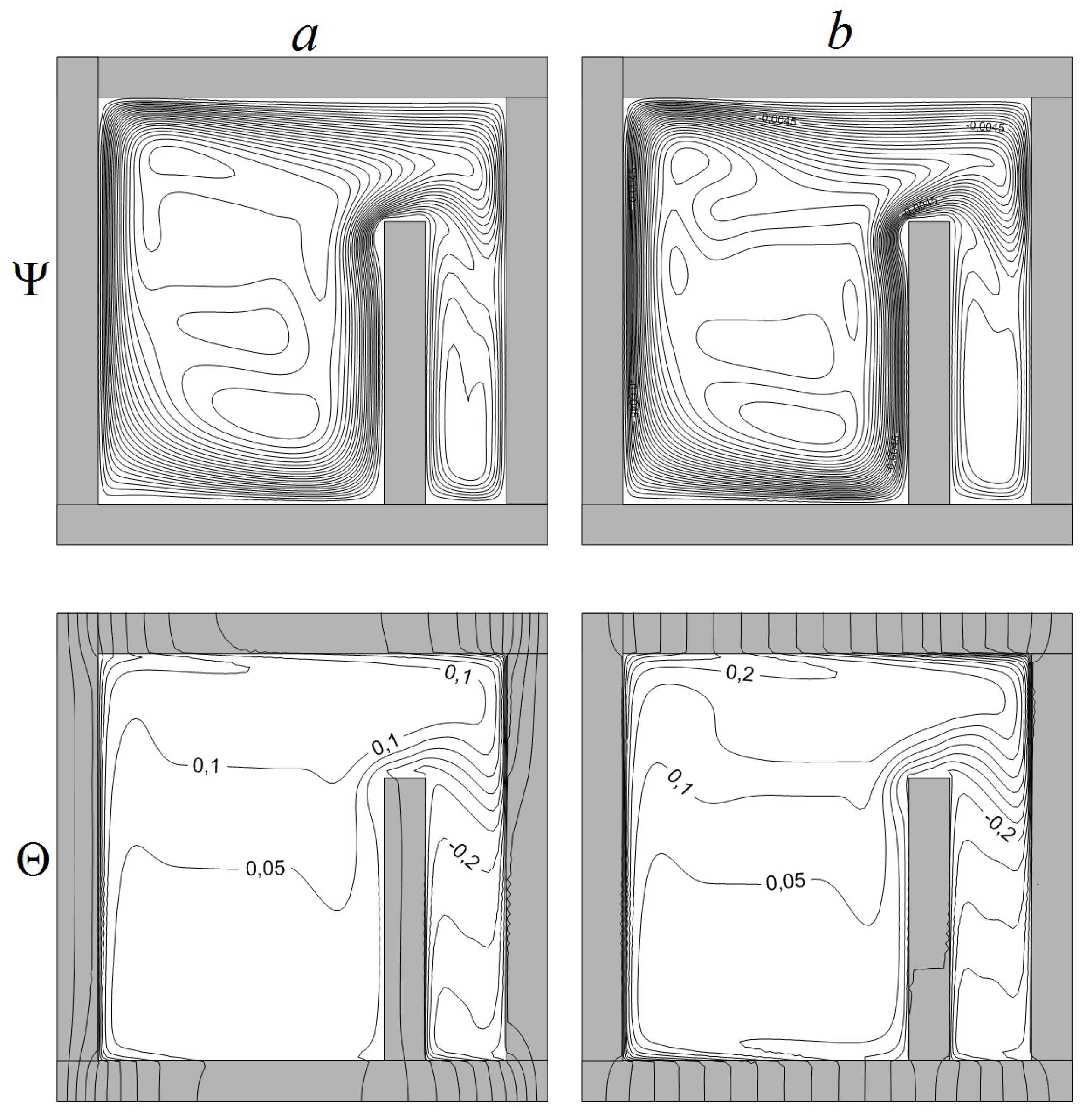
3. Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Fk–i | view factor between k-th and i-th elements of a cavity |
| L | size (m) |
| g | gravity acceleration (m/s2) |
| k | turbulence kinetic energy (m2/s2) |
| h | partition heights (m) |
| K | non-dimensional turbulent kinetic energy |
| generation/destruction of buoyancy turbulent kinetic energy | |
| E | non-dimensional dissipation rate of turbulent kinetic energy |
| Rayleigh number | |
| average convective Nusselt number | |
| radiation parameter | |
| Prandtl number | |
| average radiative Nusselt number | |
| shearing production | |
| turbulent Prandtl number | |
| Rk | non-dimensional radiosity of the k-th element of an enclosure |
| Qrad | non-dimensional net radiative heat flux |
| Th | temperature at the left border (K) |
| t | time (s) |
| Tc | temperature at the right border (K) |
| T | temperature (K) |
| Θf | non-dimensional temperature of fluid |
| Θ | non-dimensional temperature |
| u1, u2 | velocity components for x and y axis (m/s) |
| U1, U2 | non-dimensional velocity components for X and Y axis |
| Θw | non-dimensional temperature of walls |
| X, Y | non-dimensional Cartesian coordinates |
| ε | dissipation rate of turbulent kinetic energy (m2/s3) |
| temperature parameter | |
| β | factor of volumetric heat expansion (1/K) |
| thermal diffusivity of the wall material (m2/s) | |
| air thermal diffusivity (m2/s) | |
| thermal diffusivity ratio | |
| surface emissivity of inner surfaces | |
| air heat conductivity (W/mK) | |
| heat conductivity of the wall material (W/mK) | |
| heat conductivity ratio | |
| ν | kinematic viscosity (m2/s) |
| ψ | stream function (m2/s) |
| ω | vorticity (s−1) |
| turbulent viscosity (m2/s) | |
| Ψ | non-dimensional stream function |
| new dimensionless independent variables | |
| Ω | non-dimensional vorticity |
| τ | non-dimensional time |
| σ | Stefan–Boltzmann constant (W/m2K4) |
References
- Sharma, A.K.; Velusamy, K.; Balaji, C. Turbulent Natural Convection in an Enclosure with Localized Heating from Below. Int. J. Therm. Sci. 2007, 46, 1232–1241. [Google Scholar] [CrossRef]
- Dixit, H.N.; Babu, V. Simulation of High Rayleigh Number Natural Convection in a Square Cavity Using the Lattice Boltzmann Method. Int. J. Heat Mass Transf. 2006, 49, 727–739. [Google Scholar] [CrossRef]
- Zhuo, C.; Zhong, C. LES-Based Filter-Matrix Lattice Boltzmann Model for Simulating Turbulent Natural Convection in a Square Cavity. Int. J. Heat Fluid Flow 2013, 42, 10–22. [Google Scholar] [CrossRef]
- Miroshnichenko, I.V.; Sheremet, M.A.; Mohamad, A.A. The influence of surface radiation on the passive cooling of a heat-generating element. Energies 2019, 12, 980. [Google Scholar] [CrossRef]
- Baudoin, A.; Saury, D.; Boström, C. Optimized Distribution of a Large Number of Power Electronics Components Cooled by Conjugate Turbulent Natural Convection. Appl. Therm. Eng. 2017, 124, 975–985. [Google Scholar] [CrossRef]
- Ibrahim, A.; Saury, D.; Lemonnier, D. Coupling of Turbulent Natural Convection with Radiation in an Air-Filled Differentially-Heated Cavity at Ra = 1.5 × 109. Comput. Fluids 2013, 88, 115–125. [Google Scholar] [CrossRef]
- Shati, A.K.A.; Blakey, S.G.; Beck, S.B.M. A dimensionless solution to radiation and turbulent natural convection in square and rectangular enclosures. J. Eng. Sci. Technol. 2012, 7, 257–279. [Google Scholar]
- Tian, Y.S.; Karayiannis, T.G. Low turbulence natural convection in an air filled square cavity Part I: The thermal and fluid flow fields. Int. J. Heat Mass Transf. 2000, 43, 849–866. [Google Scholar] [CrossRef]
- Tian, Y.S.; Karayiannis, T.G. Low turbulence natural cosnvection in an air filled square cavity Part II: The turbulence quantities. Int. J. Heat Mass Transf. 2000, 43, 867–884. [Google Scholar] [CrossRef]
- Zhang, X.; Su, G.; Yu, J.; Yao, Z.; He, F. PIV measurement and simulation of turbulent thermal free convection over a small heat source in a large enclosed cavity. Build. Environ. 2015, 90, 105–113. [Google Scholar] [CrossRef]
- Altac, Z.; Ugurlubilek, N. Assessment of turbulence models in natural convection from two- and three-dimensional rectangular enclosures. Int. J. Therm. Sci. 2016, 107, 237–246. [Google Scholar] [CrossRef]
- Shati, A.K.A.; Blakey, S.G.; Beck, S.B.M. An empirical solution to turbulent natural convection and radiation heat transfer in square and rectangular enclosures. Appl. Therm. Eng. 2013, 51, 364–370. [Google Scholar] [CrossRef]
- Miroshnichenko, I.V.; Toilibayev, A.A.; Sheremet, M.A. Simulation of Thermal Radiation and Turbulent Free Convection in an Enclosure with a Glass Wall and a Local Heater. Fluids 2021, 6, 91. [Google Scholar] [CrossRef]
- Cui, H.; An, H.; Wang, W.; Han, Z.; Hu, B.; Xu, F.; Liu, Q.; Saha, S.C. Numerical Study of Mixed Convection and Heat Transfer in Arc-Shaped Cavity with Inner Heat Sources. Appl. Sci. 2023, 13, 1029. [Google Scholar] [CrossRef]
- Serrano-Arellano, J.M.; Belman-Flores, J.; Xamán, J.; M. Aguilar-Castro, K.; V. Macías-Melo, E. Numerical Study of the Double Diffusion Natural Convection inside a Closed Cavity with Heat and Pollutant Sources Placed near the Bottom Wall. Energies 2020, 13, 3085. [Google Scholar] [CrossRef]
- Balam, N.B.; Alam, T.; Gupta, A.; Blecich, P. Higher Order Accurate Transient Numerical Model to Evaluate the Natural Convection Heat Transfer in Flat Plate Solar Collector. Processes 2021, 9, 1508. [Google Scholar] [CrossRef]
- Yao, J.; Yao, Y. Unsteady Flow Oscillations in a 3-D Ventilated Model Room with Convective Heat Transfer. Fluids 2022, 7, 192. [Google Scholar] [CrossRef]
- Ovando-Chacon, G.E.; Rodríguez-León, A.; Ovando-Chacon, S.L.; Hernández-Ordoñez, M.; Díaz-González, M.; Pozos-Texon, F.d.J. Computational Study of Thermal Comfort and Reduction of CO2 Levels inside a Classroom. Int. J. Environ. Res. Public Health 2022, 19, 2956. [Google Scholar] [CrossRef]
- Miroshnichenko, I.V.; Sheremet, M.A. Turbulent Natural Convection Heat Transfer in Rectangular Enclosures Using Experimental and Numerical Approaches: A Review. Renew. Sustain. Energy Rev. 2018, 82, 40–59. [Google Scholar] [CrossRef]
- Qaddah, B.; Soucasse, L.; Doumenc, F.; Mergui, S.; Riviиre, P.; Soufiani, A. Influence of turbulent natural convection on heat transfer in shallow caves. Int. J. Therm. Sci. 2022, 177, 107524. [Google Scholar] [CrossRef]
- Chai, X.; Li, W.; Chen, B.; Liu, X.; Xiong, J.; Cheng, X. Numerical simulation of turbulent natural convection in an enclosure with a curved surface heated from below. Prog. Nucl. Energy 2020, 126, 103392. [Google Scholar] [CrossRef]
- Benyahia, N.; Aksouh, M.; Mataoui, A.; Oztop, H.F. Coupling turbulent natural convection-radiation-conduction in differentially heated cavity with high aspect ratio. Int. J. Therm. Sci. 2020, 158, 106518. [Google Scholar] [CrossRef]
- Fabregat, A.; Pallarès, J. Heat transfer and boundary layer analyses of laminar and turbulent natural convection in a cubical cavity with differently heated opposed walls. Int. J. Heat Mass Transf. 2020, 151, 119409. [Google Scholar] [CrossRef]
- Ampofo, F. Turbulent natural convection of air in a non-partitioned or partitioned cavity with differentially heated vertical and conducting horizontal walls. Exp. Therm. Fluid Sci. 2005, 29, 137–157. [Google Scholar] [CrossRef]
- Priam, S.S.; Ikram, M.M.; Saha, S.; Saha, S.C. Conjugate natural convection in a vertically divided square enclosure by a corrugated solid partition into air and water regions. Therm. Sci. Eng. Prog. 2021, 25, 101036. [Google Scholar] [CrossRef]
- Saha, S.C.; Gu, Y.T. Transient air flow and heat transfer in a triangular enclosure with a conducting partition. Appl. Math. Model. 2014, 38, 3879–3887. [Google Scholar] [CrossRef]
- Kandaswamy, P.; Lee, J.; Hakeem, A.K.A.; Saravanan, S. Effect of baffle–cavity ratios on buoyancy convection in a cavity with mutually orthogonal heated baffles. Int. J. Heat Mass Transf. 2008, 51, 1830–1837. [Google Scholar] [CrossRef]
- Ramesh, N.; Venkateshan, S.P. Effect of surface radiation on natural convection in a square enclosure. J. Thermophys. Heat Transf. 1999, 13, 299–301. [Google Scholar] [CrossRef]
- Balaji, C.; Venkateshan, S.P. Correlations for free convection and surface radiation in a square cavity. Int. J. Heat Fluid Flow 1994, 15, 249–251. [Google Scholar] [CrossRef]
- Boukendil, M.; Abdelbaki, A.; Zrikem, Z. Detailed numerical simulation of coupled heat transfer by conduction, natural convection and radiation through double honeycomb walls. Build. Simul. 2012, 5, 337–344. [Google Scholar] [CrossRef]
- Khatamifar, M.; Lin, W.; Dong, L. Transient conjugate natural convection heat transfer in a differentially-heated square cavity with a partition of finite thickness and thermal conductivity. Case Stud. Therm. Eng. 2021, 25, 100952. [Google Scholar] [CrossRef]
- Wu, W.; Ching, C.Y. Laminar natural convection in an air-filled square cavity with partitions on the top wall. Int. J. Heat Mass Transf. 2010, 53, 1759–1772. [Google Scholar] [CrossRef]
- Costa, V.A.F. Natural convection in partially divided square enclosures: Effects of thermal boundary conditions and thermal conductivity of the partitions. Int. J. Heat Mass Transf. 2012, 55, 7812–7822. [Google Scholar] [CrossRef]
- Said, S.A.M.; Habib, M.A.; Khan, M.A.R. Turbulent natural convection flow in partitioned enclosure. Comput. Fluids 1997, 26, 541–563. [Google Scholar] [CrossRef]
- Xu, F. Unsteady coupled thermal boundary layers induced by a fin on the partition of a differentially heated cavity. Int. Commun. Heat Mass Transf. 2015, 67, 59–65. [Google Scholar] [CrossRef]
- Famouri, M.; Hooman, K. Entropy generation for natural convection by heated partitions in a cavity. Int. Commun. Heat Mass Transf. 2008, 35, 492–502. [Google Scholar] [CrossRef]
- Khatamifara, M.; Lina, W.; Armfieldc, S.W.; Holmesa, D.; Kirkpatrickc, M.P. Conjugate natural convection heat transfer in a partitioned differentially-heated square cavity. Int. Commun. Heat Mass Transf. 2017, 81, 92–103. [Google Scholar] [CrossRef]
- Al-Farhany, K.; Al-Muhja, B.; Ali, F.; Khan, U.; Zaib, A.; Raizah, Z.; Galal, A.M. The Baffle Length Effects on the Natural Convection in Nanofluid-Filled Square Enclosure with Sinusoidal Temperature. Molecules 2022, 27, 4445. [Google Scholar] [CrossRef] [PubMed]
- Kublbeck, K.; Merker, G.P.; Straub, J. Advanced numerical computation of two-dimensional time-dependent free convection in cavities. Int. J. Heat Mass Transf. 1980, 23, 203–217. [Google Scholar] [CrossRef]
- Howell, J.R.; Menguc, M.P.; Siegel, R. Thermal Radiation Heat Transfer, 6th ed.; CRC Press: New York, NY, USA, 2015. [Google Scholar] [CrossRef]
- Ampofo, F.; Karayiannis, T.G. Experimental benchmark data for turbulent natural convection in an air filled square cavity. Int. J. Heat Mass Transf. 2003, 46, 3551–3572. [Google Scholar] [CrossRef]
- Kogawa, T.; Okajima, J.; Sakurai, A.; Komiya, A.; Maruyama, S. Influence of radiation effect on turbulent natural convection in cubic cavity at normal temperature atmospheric gas. Int. J. Heat Mass Transf. 2017, 104, 456–466. [Google Scholar] [CrossRef]
- Martyushev, S.G.; Sheremet, M.A. Conjugate natural convection combined with surface thermal radiation in an air filled cavity with internal heat source. Int. J. Therm. Sci. 2014, 76, 51–67. [Google Scholar] [CrossRef]
- Miroshnichenko, I.V.; Gibanov, N.S.; Sheremet, M.A. Numerical Analysis of Heat Transfer through Hollow Brick Using Finite-Difference Method. Axioms 2022, 11, 37. [Google Scholar] [CrossRef]






| Authors | Number of Partitions | Flow Regime | Aspect Ratio | Medium |
|---|---|---|---|---|
| Ampofo [24] | 5 | Turbulent | 2 | Air |
| Priam et at. [25] | 1 | Laminar | 1 | Air and water |
| Saha and Gu [26] | 1 | Laminar | 0.2, 0.5, 1 | Air |
| Kandaswamy et al. [27] | 2 | Laminar | 1 | Air |
| Khatamifar et al. [31] | 1 | Laminar | 1 | Air |
| Wu and Ching [32] | 2 | Laminar | 0.6–0.1 | Air |
| Costa [33] | 2 | Laminar | 1 | Air |
| Said et al. [34] | 1 | Turbulent | 10 | Air |
| Xu [35] | 1 | Laminar | 1 | Water |
| Famouri and Hooman [36] | 1 | Laminar | 1 | Air |
| Khatamifar et al. [37] | 1 | Laminar | 1 | Air |
| Al-Farhany et al. [38] | 1 | Laminar | 1 | Nanofluid |
| Physical Properties | Symbol | Value |
|---|---|---|
| Thermal expansion coefficient | 3.67 × 10–3 K−1 | |
| Kinematic viscosity | 14.61 × 10–6 m2∙s−1 | |
| Thermal diffusivity | 20.72 × 10–6 m2∙s−1 | |
| Density | 1.226 kg∙m−3 |
| at internal solid-fluid interfaces |
| Surface Emissivity Value | ||
|---|---|---|
| 0.011325 | 56.02 | |
| 0.011316 | 55.99 | |
| 0.011301 | 55.96 | |
| 0.011292 | 55.93 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Miroshnichenko, I.V.; Sheremet, M.A. Turbulent Free Convection and Thermal Radiation in an Air-Filled Cabinet with Partition on the Bottom Wall. Axioms 2023, 12, 213. https://doi.org/10.3390/axioms12020213
Miroshnichenko IV, Sheremet MA. Turbulent Free Convection and Thermal Radiation in an Air-Filled Cabinet with Partition on the Bottom Wall. Axioms. 2023; 12(2):213. https://doi.org/10.3390/axioms12020213
Chicago/Turabian StyleMiroshnichenko, Igor V., and Mikhail A. Sheremet. 2023. "Turbulent Free Convection and Thermal Radiation in an Air-Filled Cabinet with Partition on the Bottom Wall" Axioms 12, no. 2: 213. https://doi.org/10.3390/axioms12020213
APA StyleMiroshnichenko, I. V., & Sheremet, M. A. (2023). Turbulent Free Convection and Thermal Radiation in an Air-Filled Cabinet with Partition on the Bottom Wall. Axioms, 12(2), 213. https://doi.org/10.3390/axioms12020213






