A Study of Monotonicity Analysis for the Delta and Nabla Discrete Fractional Operators of the Liouville–Caputo Family
Abstract
:1. Introduction
2. Preliminaries
- We here use the standard extensions of the domains of and to define these to be zero when and are well defined, but and are not defined;
- They are both increasing. Further, we have
3. Delta and Nabla Monotonicity Results
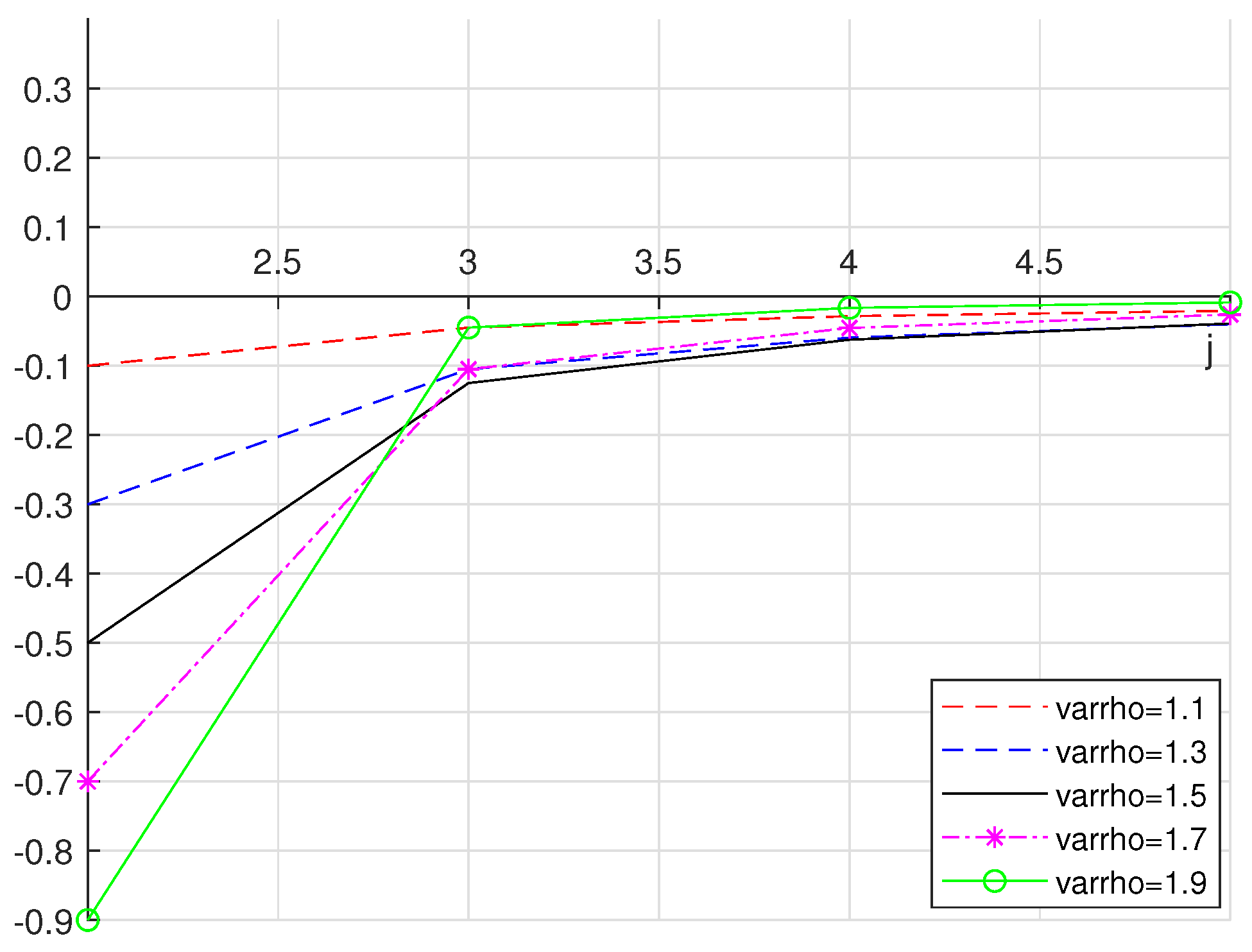
4. Example
5. Conclusions and Future Directions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Atici, F.M.; Eloe, P.W. A transform method in discrete fractional calculus. Int. J. Differ. Equ. 2007, 2, 165–176. [Google Scholar]
- Atici, F.M.; Eloe, P.W. Discrete fractional calculus with the nabla operator. Electron. J. Qual. Theory Differ. Equ. 2009, 2009, 1–12. [Google Scholar] [CrossRef]
- Atici, F.M.; Eloe, P.W. Initial value problems in discrete fractional calculus. Proc. Am. Math. Soc. 2009, 137, 981–989. [Google Scholar] [CrossRef]
- Abdeljawad, T.; Al-Mdallal, Q.M.; Hajji, M.A. Arbitrary order fractional difference operators with discrete exponential kernels and applications. Discrete Dyn. Nat. Soc. 2017, 2017, 4149320. [Google Scholar] [CrossRef] [Green Version]
- Abdeljawad, T.; Atici, F. On the definitions of nabla fractional operators. Abstr. Appl. Anal. 2012, 2012, 406757. [Google Scholar] [CrossRef] [Green Version]
- Abdeljawad, T.; Baleanu, D. Monotonicity analysis of a nabla discrete fractional operator with discrete Mittag-Leffler kernel. Chaos Solitons Fractals 2017, 102, 106–110. [Google Scholar] [CrossRef]
- Abdeljawad, T.; Madjidi, F. Lyapunov-type inequalities for fractional difference operators with discrete Mittag-Leffler kernel of order 2 < α < 5/2. Eur. Phys. J. Spec. Top. 2017, 226, 3355–3368. [Google Scholar]
- Chen, C.R.; Bohner, M.; Jia, B.G. Ulam-Hyers stability of Caputo fractional difference equations. Math. Methods Appl. Sci. 2019, 42, 7461–7470. [Google Scholar] [CrossRef]
- Ferreira, R.A.C.; Torres, D.F.M. Fractional h-difference equations arising from the calculus of variations. Appl. Anal. Discret. Math. 2011, 5, 110–121. [Google Scholar] [CrossRef] [Green Version]
- Lizama, C. The Poisson distribution, abstract fractional difference equations, and stability. Proc. Am. Math. Soc. 2017, 145, 3809–3827. [Google Scholar] [CrossRef]
- Wu, G.; Baleanu, D. Discrete chaos in fractional delayed logistic maps. Nonlinear Dyn. 2015, 80, 1697–1703. [Google Scholar] [CrossRef]
- Goodrich, C.S.; Peterson, A.C. Discrete Fractional Calculus; Springer: Berlin, Germany, 2015. [Google Scholar]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations; North-Holland Mathematics Studies; Elsevier: Amsterdam, The Netherlands, 2006; Volume 204. [Google Scholar]
- Wu, G.C.; Song, T.T.; Wang, S.Q. Caputo-Hadamard fractional differential equation on time scales: Numerical scheme, asymptotic stability, and chaos. Chaos 2022, 32, 093143. [Google Scholar] [CrossRef]
- Song, T.T.; Wu, G.C.; Wei, J.L. Hadamard fractional calculus on time scales. Fractals 2022, 30, 2250145. [Google Scholar] [CrossRef]
- Dahal, R.; Goodrich, C.S. A monotonicity result for discrete fractional difference operators. Arch. Math. 2014, 102, 293–299. [Google Scholar] [CrossRef]
- Atici, F.; Uyanik, M. Analysis of discrete fractional operators. Appl. Anal. Discrete Math. 2015, 9, 139–149. [Google Scholar] [CrossRef]
- Baoguo, J.; Erbe, L.; Peterson, A. Some relations between the Caputo fractional difference operators and integer-order differences. Electron. J. Differ. Equ. 2015, 2015, 1–7. [Google Scholar]
- Bravo, J.; Lizama, C.; Rueda, S. Second and third order forward difference operator: What is in between? Rev. R. Acad. Cienc. Exactas Fis. Nat. Ser. A Mat. RACSAM 2021, 115, 86. [Google Scholar] [CrossRef]
- Bravo, J.; Lizama, C.; Rueda, S. Qualitative properties of nonlocal discrete operators. Math. Methods Appl. Sci. 2022, 45, 6346–6377. [Google Scholar] [CrossRef]
- Dahal, R.; Goodrich, C.S. Mixed order monotonicity results for sequential fractional nabla differences. J. Differ. Equ. Appl. 2019, 25, 837–854. [Google Scholar] [CrossRef]
- Du, F.; Jia, B.; Erbe, L.; Peterson, A. Monotonicity and convexity for nabla fractional (q, h)-differences. J. Differ. Equ. Appl. 2016, 22, 1224–1243. [Google Scholar] [CrossRef]
- Wang, M.; Jia, B.G.; Du, F.F.; Liu, X. Asymptotic stability of fractional difference equations with bounded time delays. Fract. Calc. Appl. Anal. 2020, 23, 571–590. [Google Scholar] [CrossRef]
- Du, F.F.; Lu, J.G. Finite-time stability of neutral fractional order time delay systems with Lipschitz nonlinearities. Appl. Math. Comput. 2020, 375, 125079. [Google Scholar] [CrossRef]
- Goodrich, C.S. A sharp convexity result for sequential fractional delta differences. J. Differ. Equ. Appl. 2017, 23, 1986–2003. [Google Scholar] [CrossRef]
- Baoguo, J.; Erbe, L.; Peterson, A. Convexity for nabla and delta fractional differences. J. Differ. Equ. Appl. 2015, 21, 360–373. [Google Scholar] [CrossRef]
- Goodrich, C.S.; Lizama, C. Positivity, monotonicity, and convexity for convolution operators. Discret. Contin. Dyn. Syst. 2020, 40, 4961–4983. [Google Scholar] [CrossRef]
- Baoguo, J.; Erbe, L.; Peterson, A. Monotonicity and convexity for nabla fractional q-differences. Dynam. Syst. Appl. 2016, 25, 47–60. [Google Scholar]
- Abdeljawad, T.; Abdalla, B. Monotonicity results for delta and nabla Caputo and Riemann fractional differences via dual identities. Filomat 2017, 31, 3671–3683. [Google Scholar] [CrossRef]
- Chen, C.R.; Bohner, M.; Jia, B.G. Methods of upper and lower solutions for nonlinear Caputo fractional difference equations and its applications. Frac. Calc. Appl. Anal. 2019, 22, 1307–1320. [Google Scholar] [CrossRef]
- Mohammed, P.O.; Abdeljawad, T.; Hamasalh, F.K. On discrete delta Caputo-Fabrizio fractional operators and monotonicity analysis. Fractal Fract. 2021, 5, 116. [Google Scholar] [CrossRef]
- Mohammed, P.O.; Srivastava, H.M.; Baleanu, D.; Elattar, E.E.; Hamed, Y.S. Positivity analysis for the discrete delta fractional differences of the Riemann-Liouville and Liouville-Caputo types. Electron. Res. Arch. 2022, 30, 3058–3070. [Google Scholar] [CrossRef]
- Liu, X.; Du, F.; Anderson, D.; Jia, B. Monotonicity results for nabla fractional h-difference operators. Math. Methods Appl. Sci. 2020, 44, 1207–1218. [Google Scholar] [CrossRef]
- Mohammed, P.O.; Almutairi, O.; Agarwal, R.P.; Hamed, Y.S. On convexity, monotonicity and positivity analysis for discrete fractional operators defined using exponential kernels. Fractal Fract. 2022, 6, 55. [Google Scholar] [CrossRef]
- Mohammed, P.O.; Srivastava, H.M.; Baleanu, D.; Jan, R.; Abualnaja, K.M. Monotonicity results for nabla Riemann-Liouville fractional differences. Mathematics 2022, 10, 2433. [Google Scholar] [CrossRef]
- Atici, F.; Şengül, S. Modeling with discrete fractional equations. J. Math. Anal. Appl. 2010, 369, 1–9. [Google Scholar] [CrossRef] [Green Version]
- Atici, F.M.; Atici, M.; Nguyen, N.; Zhoroev, T.; Koch, G. A study on discrete and discrete fractional pharmacokinetics-pharmacodynamics models for tumor growth and anti-cancer effects. Comput. Math. Biophys. 2019, 7, 10–24. [Google Scholar] [CrossRef] [Green Version]
- Atici, F.M.; Atici, M.; Belcher, M.; Marshall, D. A new approach for modeling with discrete fractional equations. Fund. Inform. 2017, 151, 313–324. [Google Scholar]
- Abdeljawad, T. Different type kernel h–fractional differences and their fractional h-sums. Chaos Solit. Fract. 2018, 116, 146–156. [Google Scholar] [CrossRef]
- Abdeljawad, T. On delta and nabla Liouville-Caputo fractional differences and dual identities. Discret. Dyn. Nat. Soc. 2013, 2013, 12. [Google Scholar] [CrossRef] [Green Version]
- Gray, H.L.; Zhang, N.F. On a new definition of the fractional difference. Math. Comp. 1988, 50, 513–529. [Google Scholar] [CrossRef]
- Rahmat, M.R.S.; Noorani, M.S.M. Caputo type fractional difference operator and its application on discrete time scales. Adv. Diff. Equ. 2015, 2015, 160. [Google Scholar] [CrossRef] [Green Version]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mohammed, P.O.; Goodrich, C.S.; Srivastava, H.M.; Al-Sarairah, E.; Hamed, Y.S. A Study of Monotonicity Analysis for the Delta and Nabla Discrete Fractional Operators of the Liouville–Caputo Family. Axioms 2023, 12, 114. https://doi.org/10.3390/axioms12020114
Mohammed PO, Goodrich CS, Srivastava HM, Al-Sarairah E, Hamed YS. A Study of Monotonicity Analysis for the Delta and Nabla Discrete Fractional Operators of the Liouville–Caputo Family. Axioms. 2023; 12(2):114. https://doi.org/10.3390/axioms12020114
Chicago/Turabian StyleMohammed, Pshtiwan Othman, Christopher S. Goodrich, Hari Mohan Srivastava, Eman Al-Sarairah, and Y. S. Hamed. 2023. "A Study of Monotonicity Analysis for the Delta and Nabla Discrete Fractional Operators of the Liouville–Caputo Family" Axioms 12, no. 2: 114. https://doi.org/10.3390/axioms12020114
APA StyleMohammed, P. O., Goodrich, C. S., Srivastava, H. M., Al-Sarairah, E., & Hamed, Y. S. (2023). A Study of Monotonicity Analysis for the Delta and Nabla Discrete Fractional Operators of the Liouville–Caputo Family. Axioms, 12(2), 114. https://doi.org/10.3390/axioms12020114









