Design, Analysis and Comparison of a Nonstandard Computational Method for the Solution of a General Stochastic Fractional Epidemic Model
Abstract
:1. Introduction
2. Mathematical Models
3. Mathematical Analysis
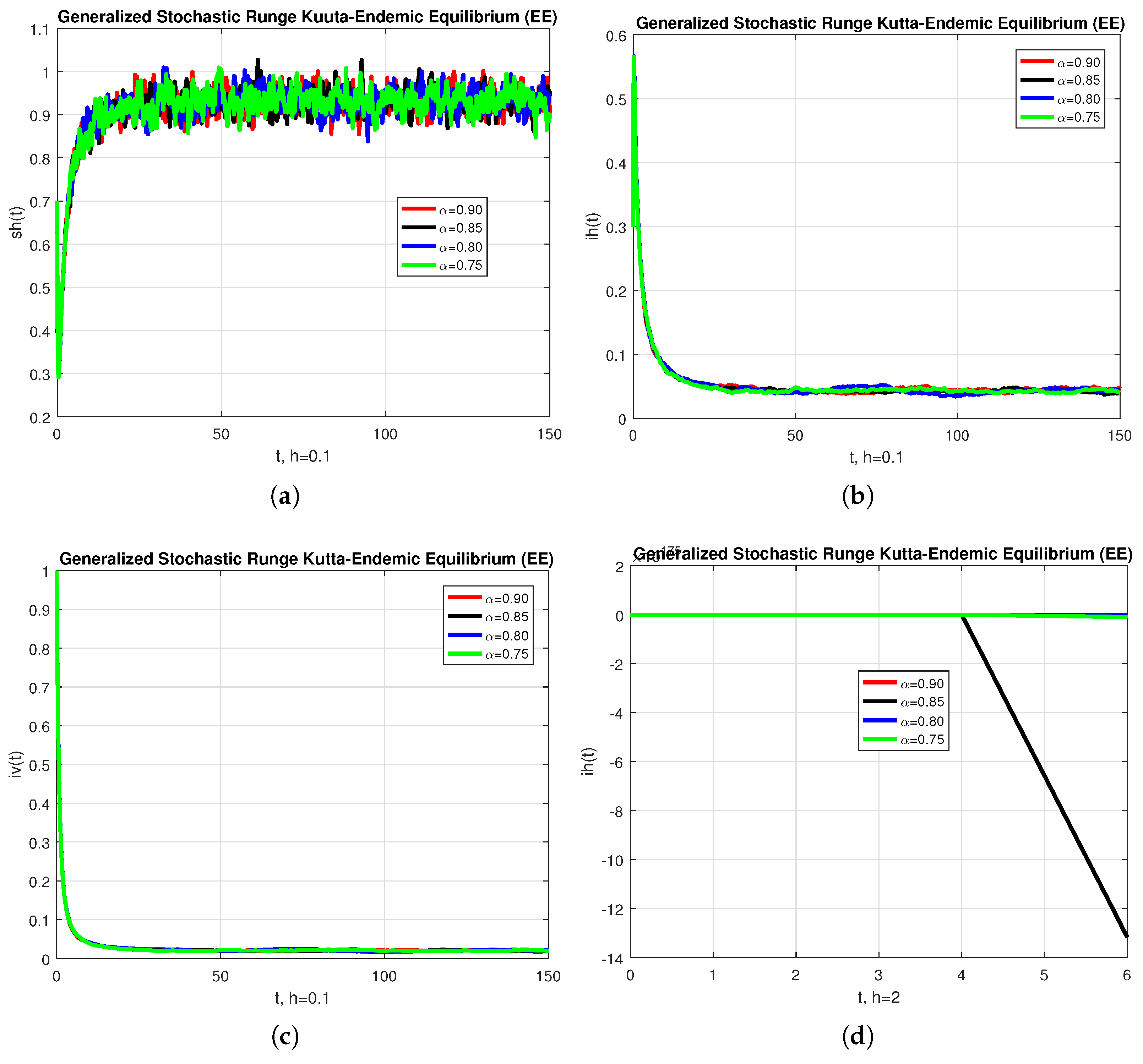
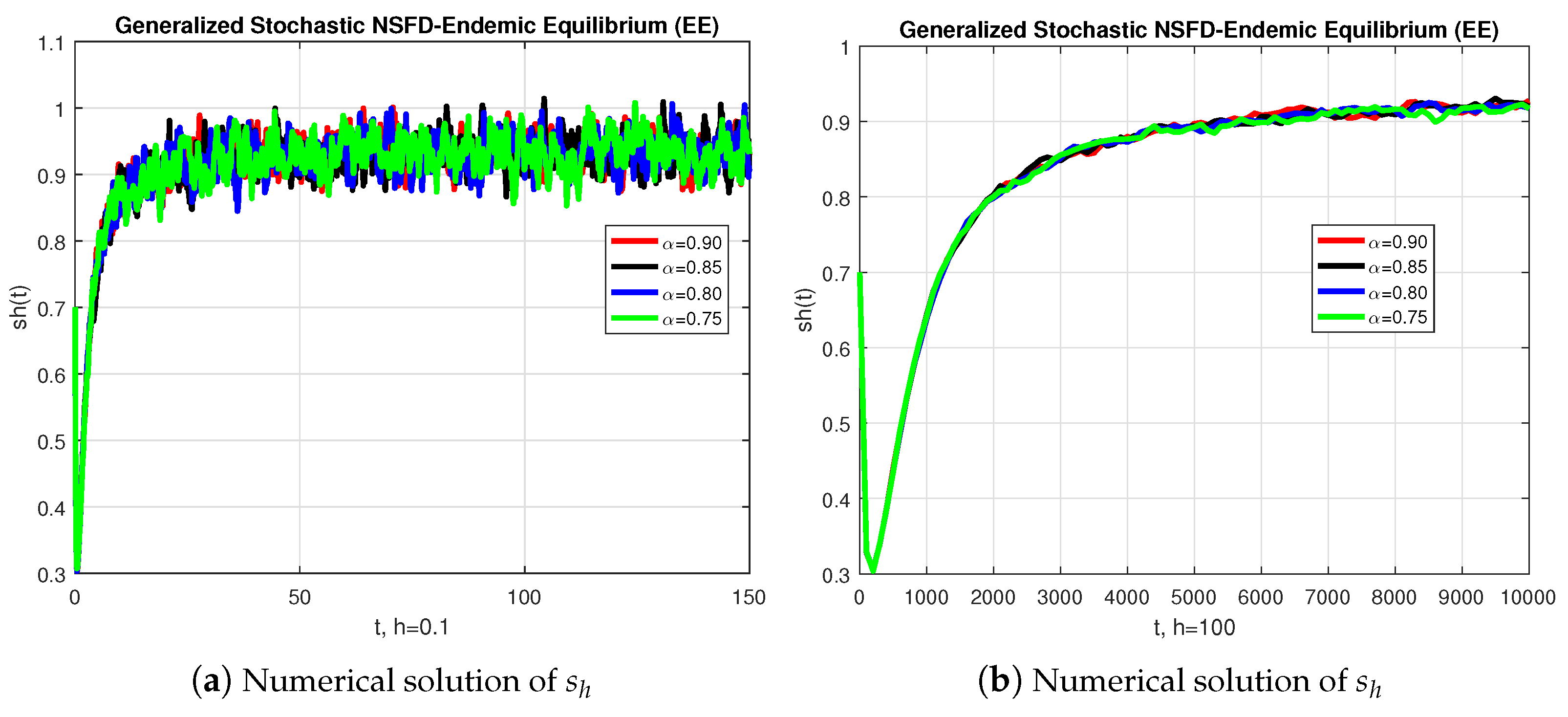
4. Numerical Model
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Montoya, C.; Romero-Leiton, J.P. Analysis and optimal control of a malaria mathematical model under resistance and population movement. arXiv 2020, arXiv:2002.00070. [Google Scholar]
- Olaniyi, S.; Okosun, K.O.; Adesanya, S.O.; Lebelo, R.S. Modelling malaria dynamics with partial immunity and protected travellers: Optimal control and cost-effectiveness analysis. J. Biol. Dyn. 2020, 14, 90–115. [Google Scholar] [CrossRef] [Green Version]
- Kim, S.; Byun, J.H.; Park, A.; Jung, I.H. A mathematical model for assessing the effectiveness of controlling relapse in Plasmodium vivax malaria endemic in the Republic of Korea. PLoS ONE 2020, 15, e0227919. [Google Scholar] [CrossRef]
- Ibrahim, M.M.; Kamran, M.A.; Naeem Mannan, M.M.; Kim, S.; Jung, I.H. Impact of Awareness to Control Malaria Disease: A Mathematical Modeling Approach. Complexity 2020, 2020, 8657410. [Google Scholar] [CrossRef]
- Baihaqi, M.A.; Adi-Kusumo, F. Modelling malaria transmission in a population with SEIRSp method. In AIP Conference Proceedings; AIP Publishing LLC: Melville, NY, USA, 2020; Volume 2264, pp. 1–13. [Google Scholar]
- Traoré, B.; Koutou, O.; Sangaré, B. A global mathematical model of malaria transmission dynamics with structured mosquito population and temperature variations. Nonlinear Anal. Real World Appl. 2020, 53, 103081. [Google Scholar] [CrossRef]
- Djidjou-Demasse, R.; Abiodun, G.J.; Adeola, A.M.; Botai, J.O. Development and analysis of a malaria transmission mathematical model with seasonal mosquito life-history traits. Stud. Appl. Math. 2020, 144, 389–411. [Google Scholar] [CrossRef]
- Pandey, R. Mathematical Model for Malaria Transmission and Chemical Control with Human-Related Activities. Natl. Acad. Sci. Lett. 2020, 43, 59–65. [Google Scholar] [CrossRef]
- Song, T.; Wang, C.; Tian, B. Mathematical models for within-host competition of malaria parasites. Math. Biosci. Eng. 2020, 16, 6623–6653. [Google Scholar] [CrossRef] [PubMed]
- Ogunmiloro, O.M. Mathematical Modeling of the Coinfection Dynamics of Malaria-Toxoplasmosis in the Tropics. Biom. Lett. 2019, 56, 139–163. [Google Scholar] [CrossRef] [Green Version]
- Koutou, O.; Traoré, B.; Sangaré, B. Mathematical modeling of malaria transmission global dynamics: Taking into account the immature stages of the vectors. Adv. Differ. Equ. 2018, 2018, 220. [Google Scholar] [CrossRef]
- Bakary, T.; Boureima, S.; Sado, T. A mathematical model of malaria transmission in a periodic environment. J. Biol. Dyn. 2018, 12, 400–432. [Google Scholar] [CrossRef] [Green Version]
- Beretta, E.; Capasso, V.; Garao, D.G. A mathematical model for malaria transmission with asymptomatic carriers and two age groups in the human population. Math. Biosci. 2018, 300, 87–101. [Google Scholar] [CrossRef]
- Rafia, G.; He, J.; Sana, D.; Ebrahim, A.S. A Simple SIR Mathematical Model of Malaria Transmission with the Efficacy of the Vaccine. In Proceedings of the 2018 2nd International Conference on Computational Biology and Bioinformatics, Bari, Italy, 26–28 December 2018; Volume 1145, pp. 6–11. [Google Scholar]
- Traoré, B.; Sangaré, B.; Traoré, S. A mathematical model of malaria transmission with structured vector population and seasonality. J. Appl. Math. 2017, 2017, 6754097. [Google Scholar] [CrossRef] [Green Version]
- Mojeeb, A.L.; Adu, I.K. Simple mathematical model for malaria transmission. J. Adv. Math. Comput. Sci. 2017, 25, 1–24. [Google Scholar]
- Olaniyi, S.; Obabiyi, O.S. Mathematical model for malaria transmission dynamics in human and mosquito populations with nonlinear forces of infection. Int. J. Pure Appl. Math. 2013, 88, 125–156. [Google Scholar] [CrossRef] [Green Version]
- Mandal, S.; Sarkar, R.R.; Sinha, S. Mathematical models of malaria—A review. Malar. J. 2011, 10, 202. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chitnis, N.; Cushing, J.M.; Hyman, J.M. Bifurcation analysis of a mathematical model for malaria transmission. SIAM J. Appl. Math. 2006, 67, 24–45. [Google Scholar] [CrossRef] [Green Version]
- Smith, T.; Killeen, G.F.; Maire, N.; Ross, A.; Molineaux, L.; Tediosi, F.; Tanner, M. Mathematical modeling of the impact of malaria vaccines on the clinical epidemiology and natural history of Plasmodium falciparum malaria: Overview. Am. J. Trop. Med. Hyg. 2006, 75, 1–10. [Google Scholar] [CrossRef] [PubMed]
- Arenas, A.J.; González-Parra, G.; Chen-Charpentier, B.M. Construction of nonstandard finite difference schemes for the SI and SIR epidemic models of fractional order. Math. Comput. Simul. 2015, 121, 48–63. [Google Scholar] [CrossRef]
- Iqbal, Z.; Ahmad, N.; Baleanu, D.; Adel, W.; Rqfiq, M.; Rehman, M.A.; Alshomrani, A.S. Positivity and boundedness preserving numerical algorithm for the solution of fractional nonlinear epidemic model of HIV/AIDS transmission. Chaos Solitons Fractals 2020, 134, 109706. [Google Scholar] [CrossRef]
- Iqbal, Z.; Ahmad, N.; Baleanu, D.; Rqfiq, M.; Iqbal, M.S.; Rehman, M.A. Structure preserving computational technique for fractional order Schnakenberg model. Comput. Appl. Math. 2020, 39, 61. [Google Scholar] [CrossRef]
- Macías-Dxixaz, J.E.; Hendy, A.S.; Markov, N.S. A bounded numerical solver for a fractional FitzHugh-Nagumo equation and its high-performance implementation. Eng. Comput. 2021, 37, 1593–1609. [Google Scholar] [CrossRef]
- Iqbal, Z.; Rehman, M.A.; Baleanu, D.; Ahmed, N.; Raza, A.; Rafiq, M. Mathematical and numerical investigations of the fractional-order epidemic model with constant vaccination strategy. Rom. Rep. Phys. 2021, 73, 112. [Google Scholar]
- Gebremeskel, A.A.; Krogstad, H.E. Mathematical modelling of endemic malaria transmission. Am. J. Appl. Math. 2015, 3, 36–46. [Google Scholar] [CrossRef]
- Sweilam, N.H.; Al-Mekhlafi, S.M.; Baleanu, D. A hybrid stochastic fractional order Coronavirus (2019-nCov) mathematical model. Chaos Solitons Fractals 2021, 145, 110762. [Google Scholar] [CrossRef]
- Omar, O.A.M.; Elbarkouky, R.A.; Ahmed, H.M. Fractional stochastic models for COVID-19: Case study of Egypt. Results Phys. 2021, 23, 104018. [Google Scholar] [CrossRef] [PubMed]
- Alkahtani, B.S.T.; Koca, I. Fractional stochastic sir model. Results Phys. 2021, 24, 104124. [Google Scholar] [CrossRef]
- Akinlar, M.A.; Inc, M.; Gómez-Aguilar, J.F.; Boutarfa, B. Solutions of a disease model with fractional white noise. Chaos Solitons Fractals 2020, 137, 109840. [Google Scholar] [CrossRef]
- Leon, C.V. Volterra Lyapunov functions for fractional-order epidemic systems. Commun. Nonlinear Sci. Numer. Simul. 2015, 24, 75–85. [Google Scholar] [CrossRef]
- Milici, C.; Machado, J.T.; Draganescu, G. Application of the Euler and Runge-Kutta generalized methods for FDE and symbolic packages in the analysis of some fractional attractors. Int. J. Nonlinear Sci. Numer. Simul. 2019, 21, 159–170. [Google Scholar] [CrossRef]
- Sweilama, N.H.; Al-Mekhlafi, S.M.; Almutairi, A.; Baleanu, D. A hybrid fractional COVID-19 model with general population mask use. Numer. Treat. Alex. Eng. J. 2021, 60, 3219–3232. [Google Scholar] [CrossRef]
- Mickens, R.E. Nonstandard Finite Difference Models of Differential Equations; World Scientific: Singapore, 1994. [Google Scholar]
- Macías-Díaz, J.E.; Puri, A. A numerical method for computing radially symmetric solutions of a dissipative nonlinear modified Klein-Gordon equation. Numer. Methods Partial Differ. Equ. Int. J. 2005, 21, 998–1015. [Google Scholar] [CrossRef] [Green Version]
- Macías-Díaz, J.E.; Anna, S. Existence and uniqueness of monotone and bounded solutions for a finite-difference discretization à la Mickens of the generalized Burgers–Huxley equation. J. Differ. Equ. Appl. 2014, 20, 989–1004. [Google Scholar] [CrossRef]
- Macías-Díaz, J.E.; González, A.E. A convergent and dynamically consistent finite-difference method to approximate the positive and bounded solutions of the classical Burgers–Fisher equation. J. Comput. Appl. Math. 2017, 318, 604–615. [Google Scholar] [CrossRef]
- Din, A.; Khan, T.; Li, Y.; Tahir, H.; Khan, A.; Ali Khan, W. Mathematical analysis of dengue stochastic epidemic model. Results Phys. 2021, 20, 103719. [Google Scholar] [CrossRef]


| Parameters | Values |
|---|---|
| 1 | |
| (DFE) | 3 |
| (EE) | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ahmed, N.; Macías-Díaz, J.E.; Raza, A.; Baleanu, D.; Rafiq, M.; Iqbal, Z.; Ahmad, M.O. Design, Analysis and Comparison of a Nonstandard Computational Method for the Solution of a General Stochastic Fractional Epidemic Model. Axioms 2022, 11, 10. https://doi.org/10.3390/axioms11010010
Ahmed N, Macías-Díaz JE, Raza A, Baleanu D, Rafiq M, Iqbal Z, Ahmad MO. Design, Analysis and Comparison of a Nonstandard Computational Method for the Solution of a General Stochastic Fractional Epidemic Model. Axioms. 2022; 11(1):10. https://doi.org/10.3390/axioms11010010
Chicago/Turabian StyleAhmed, Nauman, Jorge E. Macías-Díaz, Ali Raza, Dumitru Baleanu, Muhammad Rafiq, Zafar Iqbal, and Muhammad Ozair Ahmad. 2022. "Design, Analysis and Comparison of a Nonstandard Computational Method for the Solution of a General Stochastic Fractional Epidemic Model" Axioms 11, no. 1: 10. https://doi.org/10.3390/axioms11010010
APA StyleAhmed, N., Macías-Díaz, J. E., Raza, A., Baleanu, D., Rafiq, M., Iqbal, Z., & Ahmad, M. O. (2022). Design, Analysis and Comparison of a Nonstandard Computational Method for the Solution of a General Stochastic Fractional Epidemic Model. Axioms, 11(1), 10. https://doi.org/10.3390/axioms11010010










