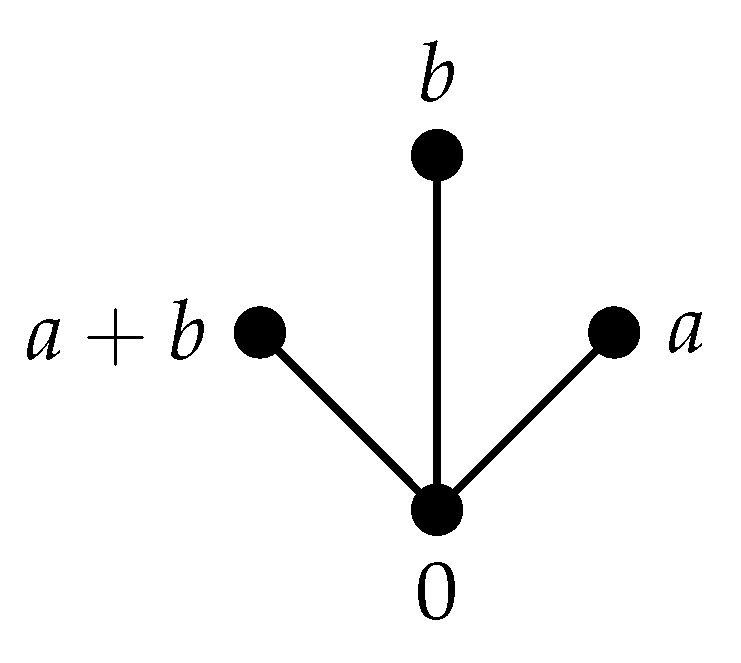On r-Noncommuting Graph of Finite Rings

Abstract

Let R be a finite ring and $r\in R$. The r-noncommuting graph of R, denoted by ${\mathsf{\Gamma }}_R^r$, is a simple undirected graph whose vertex set is R and two vertices x and y are adjacent if and only if $[x,y]\ne r$ and $[x,y]\ne -r$. In this paper, we obtain expressions for vertex degrees and show that ${\mathsf{\Gamma }}_R^r$ is neither a regular graph nor a lollipop graph if R is noncommutative. We characterize finite noncommutative rings such that ${\mathsf{\Gamma }}_R^r$ is a tree, in particular a star graph. It is also shown that ${\mathsf{\Gamma }}_{R_1}^r$ and ${\mathsf{\Gamma }}_{R_2}^{\psi \left(r\right)}$ are isomorphic if $R_1$ and $R_2$ are two isoclinic rings with isoclinism $(\varphi ,\psi )$. Further, we consider the induced subgraph ${\Delta }_R^r$ of ${\mathsf{\Gamma }}_R^r$ (induced by the non-central elements of R) and obtain results on clique number and diameter of ${\Delta }_R^r$ along with certain characterizations of finite noncommutative rings such that ${\Delta }_R^r$ is n-regular for some positive integer n. As applications of our results, we characterize certain finite noncommutative rings such that their noncommuting graphs are n-regular for $n\le 6$.

1. Introduction

Throughout the paper, R denotes a finite ring and $r\in R$. Let $Z\left(R\right):=\{z\in R:zr=rz\mathrm{for}\mathrm{all}r\in R\}$ be the center of R. For any element $x\in R$, the centralizer of x in R is a subring given by $C_R\left(x\right):=\{y\in R:xy=yx\}$. Clearly, $Z\left(R\right)=\underset{x\in R}{\cap }{C}_R\left(x\right)$. For any two elements x and y of R, $[x,y]:=xy-yx$ is called the additive commutator of x and y. Let $K\left(R\right)=\left\{\right[x,y]:x,y\in R\}$ and $[R,R]$ and $[x,R]$ for $x\in R$ denote the additive subgroups of $(R,+)$ generated by the sets $K\left(R\right)$ and $\left\{\right[x,y]:y\in R\}$, respectively.

The study of graphs defined on algebraic structures has been an active topic of research in the last few decades. The main question in this area is to recognize finite groups/rings through the properties of various graphs defined on it. In 2015, Erfanian, Khashyarmanesh and Nafar [1] considered noncommuting graphs of finite rings. Recall that the noncommuting graph of a finite noncommutative ring R is a simple undirected graph whose vertex set is $R\backslash Z\left(R\right)$ and two vertices x and y are adjacent if and only if $xy\ne yx$. The complement of noncommuting graph, called commuting graph, of a finite noncommutative ring is considered in [2,3,4,5]. The motivation for studying commuting/noncommuting graphs of finite rings comes from the study of commuting/noncommuting graphs of finite groups. Many interesting results on commuting/noncommuting graphs of finite groups can be found in [6,7,8,9,10,11,12,13,14,15,16]. There are many generalizations of noncommuting graphs of finite groups. The g-noncommuting graph of a finite group, studied extensively in [17,18,19,20], is a kind of generalization of noncommuting graph of a finite group. It is worth mentioning that commuting/noncommuting graphs and their generalizations for finite rings are not much studied. Some people want to know whether commuting/noncommuting graphs and their generalizations for finite rings possess results analogous to the results for finite groups.

In this paper, we introduce and study the r-noncommuting graph of a finite ring R for any given element $r\in R$ analogous to g-noncommuting graph of a finite group. The r-noncommuting graph of R, denoted by ${\mathsf{\Gamma }}_R^r$, is a simple undirected graph whose vertex set is R and two vertices x and y are adjacent if and only if $[x,y]\ne r$ and $[x,y]\ne -r$. Clearly, ${\mathsf{\Gamma }}_R^r={\mathsf{\Gamma }}_R^{-r}$. If $r=0$, then the induced subgraph of ${\mathsf{\Gamma }}_R^r$ with vertex set $R\backslash Z\left(R\right)$, denoted by ${\Delta }_R^r$, is nothing but the noncommuting graph of R. Note that ${\mathsf{\Gamma }}_R^r$ is a 0-regular graph if $r=0$ and R is commutative. In addition, ${\mathsf{\Gamma }}_R^r$ is complete if $r\notin K\left(R\right)$. Thus, for $r\notin K\left(R\right)$, ${\mathsf{\Gamma }}_R^r$ is n-regular if and only if R is of order $n+1$. Therefore throughout the paper we shall consider $r\in K\left(R\right)$.

In Section 2, we first compute degree of any vertex of ${\mathsf{\Gamma }}_R^r$ in terms of its centralizers. Then we characterize R if ${\mathsf{\Gamma }}_R^r$ is a tree, in particular a star graph. We further show that ${\mathsf{\Gamma }}_R^r$ is not a regular graph (if $r\in K\left(R\right)$) or a lollipop graph for any noncommutative ring R. We conclude this section by showing that ${\mathsf{\Gamma }}_{R_1}^r$ is isomorphic to ${\mathsf{\Gamma }}_{R_2}^{\psi \left(r\right)}$ if $(\varphi ,\psi )$ is an isoclinism between two finite rings $R_1$ and $R_2$ such that $|Z\left(R_1\right)|=|Z\left(R_2\right)|$. In Section 3, we consider the induced subgraph ${\Delta }_R^r$ of ${\mathsf{\Gamma }}_R^r$, induced by $R\backslash Z\left(R\right)$, and obtain results on clique number and diameter of ${\Delta }_R^r$ along with certain characterizations of finite noncommutative rings such that ${\Delta }_R^r$ is n-regular for some positive integer n. As applications of our results, we characterize certain finite noncommutative rings such that their noncommuting graphs are n-regular for $n\le 6$.

It was shown in [21] that there are only two noncommutative rings (up to isomorphism) having order $p^2$, where p is a prime, and the rings are given by

$$E\left(p^2\right)=\langle a,b:pa=pb=0,a^2=a,b^2=b,ab=a,ba=b\rangle$$

$$\mathrm{and}F\left(p^2\right)=\langle x,y:px=py=0,x^2=x,y^2=y,xy=y,yx=x\rangle .$$

The following figures (Figure 1, Figure 2, Figure 3 and Figure 4) show the graphs ${\mathsf{\Gamma }}_{E\left(p^2\right)}^r$ for $p=2,3$.

It is worth noting here that the graphs ${\mathsf{\Gamma }}_{F\left(4\right)}^0,{\mathsf{\Gamma }}_{F\left(4\right)}^{x+y},{\mathsf{\Gamma }}_{F\left(9\right)}^0$ and ${\mathsf{\Gamma }}_{F\left(9\right)}^{x+2y}$ are isomorphic to ${\mathsf{\Gamma }}_{E\left(4\right)}^0,{\mathsf{\Gamma }}_{E\left(4\right)}^{a+b},{\mathsf{\Gamma }}_{E\left(9\right)}^0$ and ${\mathsf{\Gamma }}_{E\left(9\right)}^{a+2b}$, respectively.

2. Some Properties

In this section, we characterize R when ${\mathsf{\Gamma }}_R^r$ is a tree or a star graph. We also show the non-existence of finite noncommutative rings R whose r-noncommuting graph is a regular graph (if $r\in K\left(R\right)$), a lollipop graph or a complete bipartite graph. However, we first compute degree of any vertex in the graph ${\mathsf{\Gamma }}_R^r$. For any two given elements x and r of R, we write $T_{x,r}$ to denote the generalized centralizer $\{y\in R:[x,y]=r\}$ of x. The following proposition gives the degree of any vertex of ${\mathsf{\Gamma }}_R^r$ in terms of its generalized centralizers.

Proposition 1.

Let x be any vertex in ${\mathsf{\Gamma }}_R^r$. Then

(a)

$deg\left(x\right)=\left|R\right|-|C_R\left(x\right)|$ if $r=0$.

(b)

If $r\ne 0$ then $deg\left(x\right)=\left\{\begin{array}{cc}\left|R\right|-|T_{x,r}|-1,\hfill & if 2r=0\hfill \\ \left|R\right|-2|T_{x,r}|-1,\hfill & if 2r\ne 0.\hfill \end{array}\right.$

Proof. 

(a) If $r=0$, then $deg\left(x\right)$ is the number of $y\in R$ such that $xy\ne yx$. Note that $|C_R\left(x\right)|$ gives the number of elements that commute with x. Hence, $deg\left(x\right)=\left|R\right|-|C_R\left(x\right)|$.

(b) Consider the case when $r\ne 0$. If $2r=0$ then $r=-r$. Note that $y\in R$ is not adjacent to x if and only if $y=x$ or $y\in T_{x,r}$. Therefore, $deg\left(x\right)=\left|R\right|-|T_{x,r}|-1$. If $2r\ne 0$ then $r\ne -r$. It is easy to see that $T_{x,r}\cap T_{x,-r}=\varnothing$ and $y\in T_{x,r}$ if and only if $-y\in T_{x,-r}$. Therefore, $|T_{x,r}|=|T_{x,-r}|$. Note that $y\in R$ is not adjacent to x if and only if $y=x$ or $y\in T_{x,r}$ or $y\in T_{x,-r}$. Therefore, $deg\left(x\right)=\left|R\right|-|T_{x,r}|-|T_{x,-r}|-1$. Hence the result follows. □

The following corollary gives degree of any vertex of ${\mathsf{\Gamma }}_R^r$ in terms of its centralizers.

Corollary 1.

Let x be any vertex in ${\mathsf{\Gamma }}_R^r$.

(a)

If $r\ne 0$ and $2r=0$ then $deg\left(x\right)=\left\{\begin{array}{cc}\left|R\right|-1,\hfill & if T_{x,r}=\varnothing \hfill \\ \left|R\right|-|C_R\left(x\right)|-1,\hfill & otherwise.\hfill \end{array}\right.$

(b)

If $r\ne 0$ and $2r\ne 0$ then $deg\left(x\right)=\left\{\begin{array}{cc}\left|R\right|-1,\hfill & if T_{x,r}=\varnothing \hfill \\ \left|R\right|-2|C_R\left(x\right)|-1,\hfill & otherwise.\hfill \end{array}\right.$

Proof. 

Notice that $T_{x,r}\ne \varnothing$ if and only if $r\in [x,R]$. Suppose that $T_{x,r}\ne \varnothing$. Let $t\in T_{x,r}$ and $p\in t+C_R\left(x\right)$. Then $[x,p]=r$ and so $p\in T_{x,r}$. Therefore, $t+C_R\left(x\right)\subseteq T_{x,r}$. Again, if $y\in T_{x,r}$ then $(y-t)\in C_R\left(x\right)$ and so $y\in t+C_R\left(x\right)$. Therefore, $T_{x,r}\subseteq t+C_R\left(x\right)$. Thus, $|T_{x,r}|=|C_R\left(x\right)|$ if $T_{x,r}\ne \varnothing$. Hence, the result follows from Proposition 1. □

We now present some results regarding realization of the graph ${\mathsf{\Gamma }}_R^r$ and characterization of R through certain properties of ${\mathsf{\Gamma }}_R^r$ as applications of Proposition 1.

Proposition 2.

Let R be a ring with unity. The r-noncommuting graph ${\mathsf{\Gamma }}_R^r$ is a tree if and only if $\left|R\right|=2$ and $r\ne 0$.

Proof. 

If $r=0$ then, by Proposition 1(a), we have $deg\left(r\right)=0$. Hence, ${\mathsf{\Gamma }}_R^r$ is not a tree. Suppose that $r\ne 0$. If R is commutative, then $r\notin K\left(R\right)$. Hence, ${\mathsf{\Gamma }}_R^r$ is a complete graph. Therefore ${\mathsf{\Gamma }}_R^r$ is a tree if and only if $\left|R\right|=2$. If R is noncommutative, then $[x,0]\ne r,-r$ and $[x,1]\ne r,-r$ for any $x\in R$. Therefore $deg\left(x\right)\ge 2$ for all $x\in R$. Hence, ${\mathsf{\Gamma }}_R^r$ is not a tree. □

Proposition 3.

Let R be a noncommutative ring. If ${\mathsf{\Gamma }}_R^r$ has an end vertex then $r\ne 0$ and ${\mathsf{\Gamma }}_R^{r\ne 0}$ is a star graph if and only if R is isomorphic to $E\left(4\right)=\langle a,b:2a=2b=0,a^2=a,b^2=b,ab=a,ba=b\rangle$ or $F\left(4\right)=\langle a,b:2a=2b=0,a^2=a,b^2=b,ab=b,ba=a\rangle$. Hence, ${\mathsf{\Gamma }}_R^r$ is not a lollipop graph.

Proof. 

Let $x\in R$ be an end vertex in ${\mathsf{\Gamma }}_R^r$. Then $deg\left(x\right)=1$. If $r=0$ then $x\notin Z\left(R\right)$ and so $|C_R\left(x\right)|\le \frac{\left|R\right|}{2}$. In addition, by Proposition 1(a), we have $deg\left(x\right)=\left|R\right|-|C_R\left(x\right)|$. These give $\left|R\right|-|C_R\left(x\right)|=1$. Hence, $\left|R\right|\le 2$, a contradiction. Therefore, $r\ne 0$. By Corollary 1, we have $deg\left(x\right)=\left|R\right|-1,\left|R\right|-|C_R\left(x\right)|-1$ or $\left|R\right|-2|C_R\left(x\right)|-1$. These give $\left|R\right|-|C_R\left(x\right)|=2$ or $\left|R\right|-2|C_R\left(x\right)|=2$. Clearly, $x\notin Z\left(G\right)$ and so $|C_R\left(x\right)|\le \frac{\left|R\right|}{2}$. Therefore, if $\left|R\right|-|C_R\left(x\right)|=2$, then $\left|R\right|\le 4$. If $\left|R\right|-2|C_R\left(x\right)|=2$, then $\left|R\right|$ is even and $|C_R\left(x\right)|\le \frac{\left|R\right|}{2}$. Therefore, $\left|R\right|\le 6$. Since R is noncommutative, we have $\left|R\right|=4$, and so R is isomorphic to either $E\left(4\right)$ or $F\left(4\right)$. In Figure 2, it is shown that ${\mathsf{\Gamma }}_{E\left(4\right)}^r$ is a star graph if $r\ne 0$. Furthermore, ${\mathsf{\Gamma }}_{E\left(4\right)}^r$ is isomorphic to ${\mathsf{\Gamma }}_{F\left(4\right)}^r$. Hence, the result follows. □

It follows that if R is noncommutative, having more than four elements, then there is no vertex of degree one in ${\mathsf{\Gamma }}_R^r$.

It is observed that ${\mathsf{\Gamma }}_R^r$ is $\left(\right|R|-1)$-regular if $r\notin K\left(R\right)$. Additionally, if $r=0$ and R is commutative, then ${\mathsf{\Gamma }}_R^r$ is 0-regular. In the following proposition, we show that ${\mathsf{\Gamma }}_R^r$ is not regular if $r\in K\left(R\right)$.

Proposition 4.

Let R be a noncommutative ring and $r\in K\left(R\right)$. Then ${\mathsf{\Gamma }}_R^r$ is not regular.

Proof. 

If $r=0$, then, by Proposition 1(a), we have $deg\left(r\right)=0$. Let $x\in R$ be a non-central element. Then $|C_R\left(x\right)|\ne |R|$. Therefore, by Proposition 1(a), $deg\left(x\right)\ne 0=deg\left(r\right)$. This shows that ${\mathsf{\Gamma }}_R^r$ is not regular. If $r\ne 0$ then $T_{0,r}=\varnothing$. Therefore, by Corollary 1, we have $deg\left(0\right)=\left|R\right|-1$. Since $r\in K\left(R\right)$, there exists $0\ne x\in R$ such that $T_{x,r}\ne \varnothing$. Therefore, by Corollary 1, we have $deg\left(x\right)=\left|R\right|-|C_R\left(x\right)|-1$ or $\left|R\right|-2|C_R\left(x\right)|-1$. If ${\mathsf{\Gamma }}_R^r$ is regular, then $deg\left(x\right)=deg\left(0\right)$. Therefore,

$$\left|R\right|-|C_R\left(x\right)|-1=|R|-2|C_R\left(x\right)|-1=|R|-1$$

which gives $|C_R\left(x\right)|=0$, a contradiction. Hence, ${\mathsf{\Gamma }}_R^r$ is not regular. This completes the proof. □

The following result shows that ${\mathsf{\Gamma }}_R^r$ is not complete bipartite if $\left|R\right|\ge 3$ and $\left|Z\right(R\left)\right|\ge 2$.

Proposition 5.

Let R be a finite ring.

(a)

If $r=0$ then, ${\mathsf{\Gamma }}_R^r$ is not complete bipartite.

(b)

If $r\ne 0$ then, ${\mathsf{\Gamma }}_R^r$ is not complete bipartite for $\left|R\right|\ge 3$ with $\left|Z\right(R\left)\right|\ge 2$.

Proof. 

Let ${\mathsf{\Gamma }}_R^r$ be complete bipartite. Then there exist subsets $V_1$ and $V_2$ of R such that $V_1\cap V_2=\varnothing ,V_1\cup V_2=R$ and if $x\in V_1$ and $y\in V_2$ then x and y are adjacent.

(a) If $r=0$, then for $x\in V_1$ and $y\in V_2$, we have $[x,y]\ne 0$. Therefore, $[x,x+y]\ne 0$, which implies $x+y\in V_2$. Again $[y,x+y]\ne 0$, which implies $x+y\in V_1$. Thus, $x+y\in V_1\cap V_2$, a contradiction. Hence ${\mathsf{\Gamma }}_R^r$ is not complete bipartite.

(b) If $r\ne 0,\left|R\right|\ge 3$ and $\left|Z\right(R\left)\right|\ge 2$, then for any $z_1,z_2\in Z\left(R\right)$, $z_1$ and $z_2$ are adjacent. Let us take $z_1\in V_1$ and $z_2\in V_2$. Since $\left|R\right|\ge 3$ we have $x\in R$ such that $x\ne z_1$ and $x\ne z_2$. Furthermore, $[x,z_1]=0=[x,z_2]$. Therefore, x is adjacent to both $z_1$ and $z_2$. Therefore, $x\notin V_1\cup V_2=R$, a contradiction. Hence ${\mathsf{\Gamma }}_R^r$ is not complete bipartite. □

In 1940, Hall [22] introduced isoclinism between two groups. Recently, Buckley et al. [23] introduced isoclinism between two rings. Let $R_1$ and $R_2$ be two rings. A pair of additive group isomorphisms $(\varphi ,\psi )$ where $\varphi :\frac{R_1}{Z\left(R_1\right)}\to \frac{R_2}{Z\left(R_2\right)}$ and $\psi :[R_1,R_1]\to [R_2,R_2]$ is called an isoclinism between $R_1$ to $R_2$ if $\psi \left([u,v]\right)=[u^{\prime },v^{\prime }]$ whenever $\varphi (u+Z\left(R_1\right))=u^{\prime }+Z\left(R_2\right)$ and $\varphi (v+Z\left(R_1\right))=v^{\prime }+Z\left(R_2\right)$. Two rings are called isoclinic if there exists an isoclinism between them. If $R_1$ and $R_2$ are two isomorphic rings and $\alpha :R_1\to R_2$ is an isomorphism, then it is easy to see that ${\mathsf{\Gamma }}_{R_1}^r\cong {\mathsf{\Gamma }}_{R_2}^{\alpha \left(r\right)}$. In the following proposition, we show that ${\mathsf{\Gamma }}_{R_1}^r\cong {\mathsf{\Gamma }}_{R_2}^{\psi \left(r\right)}$ if $R_1$ and $R_2$ are two isoclinic rings with isoclinism $(\varphi ,\psi )$.

Proposition 6.

Let $R_1$ and $R_2$ be two finite rings such that $|Z\left(R_1\right)|=|Z\left(R_2\right)|$. If $(\varphi ,\psi )$ is an isoclinism between $R_1$ and $R_2$, then

$${\mathsf{\Gamma }}_{R_1}^r\cong {\mathsf{\Gamma }}_{R_2}^{\psi \left(r\right)}.$$

Proof. 

Since $\varphi :\frac{R_1}{Z\left(R_1\right)}\to \frac{R_2}{Z\left(R_2\right)}$ is an isomorphism, $\frac{R_1}{Z\left(R_1\right)}$ and $\frac{R_2}{Z\left(R_2\right)}$ have the same number of elements. Let $\left|\frac{R_1}{Z\left(R_1\right)}\right|=\left|\frac{R_2}{Z\left(R_2\right)}\right|=n$. Again, since $|Z\left(R_1\right)|=|Z\left(R_2\right)|$, there exists a bijection $\theta :Z\left(R_1\right)\to Z\left(R_2\right)$. Let $\{r_i:1\le i\le n\}$ and $\{s_j:1\le j\le n\}$ be two transversals of $\frac{R_1}{Z\left(R_1\right)}$ and $\frac{R_2}{Z\left(R_2\right)}$, respectively. Let $\varphi :\frac{R_1}{Z\left(R_1\right)}\to \frac{R_2}{Z\left(R_2\right)}$ and $\psi :[R_1,R_1]\to [R_2,R_2]$ be defined as $\varphi (r_i+Z\left(R_1\right))=s_i+Z\left(R_2\right)$ and $\psi \left([r_i+z_1,r_j+z_2]\right)=[s_i+z_1^{\prime },s_j+z_2^{\prime }]$ for some $z_1,z_2\in Z\left(R_1\right)$, $z_1^{\prime },z_2^{\prime }\in Z\left(R_2\right)$ and $1\le i,j\le n$.

Let us define a map $\alpha :R_1\to R_2$ such that $\alpha (r_i+z)=s_i+\theta \left(z\right)$ for $z\in Z\left(R\right)$. Clearly, $\alpha$ is a bijection. We claim that $\alpha$ preserves adjacency. Let x and y be two elements of $R_1$ such that x and y are adjacent. Then $[x,y]\ne r,-r$. We have $x=r_i+z_i$ and $y=r_j+z_j$ where $z_i,z_j\in Z\left(R_1\right)$ and $1\le i,j\le n$. Therefore,

$$\begin{array}{cc}\hfill & [r_i+z_i,r_j+z_j]\ne r,-r\hfill \\ \hfill \Rightarrow & \psi \left([r_i+z_i,r_j+z_j]\right)\ne \psi \left(r\right),-\psi \left(r\right)\hfill \\ \hfill \Rightarrow & [s_i+\theta \left(z_i\right),s_j+\theta \left(z_j\right)]\ne \psi \left(r\right),-\psi \left(r\right)\hfill \\ \hfill \Rightarrow & [\alpha (r_i+z_i),\alpha (r_j+z_j)]\ne \psi \left(r\right),-\psi \left(r\right)\hfill \\ \hfill \Rightarrow & \left[\alpha \right(x),\alpha (y\left)\right]\ne \psi \left(r\right),-\psi \left(r\right).\hfill \end{array}$$

This shows that $\alpha \left(x\right)$ and $\alpha \left(y\right)$ are adjacent. Hence the result follows. □

3. An Induced Subgraph

We write ${\Delta }_R^r$ to denote the induced subgraph of ${\mathsf{\Gamma }}_R^r$ with vertex set $R\backslash Z\left(R\right)$. It is worth mentioning that ${\Delta }_R^0$ is the noncommuting graph of R. If $r\ne 0$, then it is easy to see that the commuting graph of R is a spanning subgraph of ${\Delta }_R^r$. The following result gives a condition such that ${\Delta }_R^r$ is the commuting graph of R.

Proposition 7.

Let R be a noncommutative ring and $r\ne 0$. If $K\left(R\right)=\{0,r,-r\}$ then ${\Delta }_R^r$ is the commuting graph of R.

Proof. 

The result follows from the fact that two vertices $x,y$ in ${\Delta }_R^r$ are adjacent if and only if $xy=yx$. □

Let $\omega \left({\Delta }_R^r\right)$ be the clique number of ${\Delta }_R^r$. The following result gives a lower bound for $\omega \left({\Delta }_R^r\right)$.

Proposition 8.

Let R be a noncommutative ring and $r\ne 0$. If S is a commutative subring of R with maximal order, then $\omega \left({\Delta }_R^r\right)\ge \left|S\right|-|S\cap Z\left(R\right)|$.

Proof. 

The result follows from the fact that the subset $S\backslash S\cap Z\left(R\right)$ of $R\backslash Z\left(R\right)$ is a clique of ${\Delta }_R^r$. □

By ([1] Theorem 2.1), it follows that the diameter of ${\Delta }_R^0$ is less than or equal to 2. The next result gives some information regarding diameter of ${\Delta }_R^r$ when $r\ne 0$. We write $diam\left({\Delta }_R^r\right)$ and $d(x,y)$ to denote the diameter of ${\Delta }_R^r$ and the distance between x and y in ${\Delta }_R^r$, respectively. For any two vertices x and y, we write $x\sim y$ to denote x and y are adjacent; otherwise $x\nsim y$.

Theorem 1.

Let R be a noncommutative ring and $r\in R\backslash Z\left(R\right)$ such that $2r\ne 0$.

(a)

If $3r\ne 0$, then $diam\left({\Delta }_R^r\right)\le 3$.

(b)

If $\left|Z\right(R\left)\right|=1$, $|C_R\left(r\right)|\ne 3$ and $3r=0$, then $diam\left({\Delta }_R^r\right)\le 3$.

Proof. 

(a) If $x\sim r$ for all $x\in R\backslash Z\left(R\right)$ such that $x\ne r$, then it is easy to see that $diam\left({\Delta }_R^r\right)\le 2$. Suppose there exists a vertex $x\in R\backslash Z\left(R\right)$ such that $x\nsim r$. Then $[x,r]=r$ or $-r$. We have

$$[x,2r]=2[x,r]=\left\{\begin{array}{cc}2r,\hfill & \mathrm{if}[x,r]=r\hfill \\ -2r,\hfill & \mathrm{if}[x,r]=-r.\hfill \end{array}\right.$$

Since $2r\ne 0$, we have $[x,2r]\ne 0$, and hence $2r\in R\backslash Z\left(R\right)$. Furthermore, $2r\ne r,-r$. Therefore, $[x,2r]\ne r,-r$, and so $x\sim 2r$. Let $y\in R\backslash Z\left(R\right)$ such that $y\ne x$. If $y\sim r$, then $d(x,y)\le 3$, noting that $r\sim 2r$. If $y\nsim r$, then $y\sim 2r$ (as shown above). In this case, $d(x,y)\le 2$. Hence, $diam\left({\Delta }_R^r\right)\le 3$.

(b) If $x\sim r$ for all $x\in R\backslash Z\left(R\right)$ such that $x\ne r$, then it is easy to see that $diam\left({\Delta }_R^r\right)\le 2$. Suppose there exists a vertex $x\in R\backslash Z\left(R\right)$ such that $x\nsim r$. Let $y\in R\backslash Z\left(R\right)$ such that $y\ne x$. We consider the following two cases.

Case 1:$x\nsim r$ and $x\sim 2r$.

If $y\sim r$, then $d(x,y)\le 3$; note that $r\sim 2r$. Therefore, $diam\left({\Delta }_R^r\right)\le 3$. If $y\nsim r$ but $y\sim 2r$, then $d(x,y)\le 2$. Consider the case when $y\nsim r$ as well as $y\nsim 2r$. Therefore $[y,r]=r$ or $-r$. If $[y,r]=r$, then $[y,2r]=2[y,r]=2r=-r$; otherwise $y\sim 2r$, a contradiction. Let $a\in C_R\left(r\right)$ such that $a\ne 0,r,-r$ (such an element exists, since $|C_R\left(r\right)|>3$). Clearly $a\in R\backslash Z\left(R\right)$. Suppose $y\sim a$. Then $x\sim 2r\sim a\sim y$, and so $d(x,y)\le 3$. Suppose $y\nsim a$. Then $[y,a]=r$ or $-r$. If $[y,a]=r$, then

$$[y,r-a]=[y,r]-[y,a]=r-r=0.$$

Note that $r-a\in R\backslash Z\left(R\right)$; otherwise $a=r$, a contradiction. Therefore, $y\sim r-a$. Furthermore,

$$[r-a,2r]=2[r,a]=0.$$

That is, $r-a\sim 2r$. Thus, $x\sim 2r\sim r-a\sim y$. Therefore, $d(x,y)\le 3$. If $[y,a]=-r$, then

$$[y,2r-a]=[y,2r]-[y,a]=-r-(-r)=0.$$

Note that $2r-a\in R\backslash Z\left(R\right)$; otherwise $a=2r=-r$, a contradiction. Therefore, $y\sim 2r-a$. Furthermore,

$$[2r-a,2r]=2[r,a]=0.$$

That is, $2r-a\sim 2r$. Thus, $x\sim 2r\sim 2r-a\sim y$. Therefore, $d(x,y)\le 3$.

If $[y,r]=-r$ then $[y,2r]=2[y,r]=-2r=r$; otherwise $y\sim 2r$, a contradiction. Let $a\in C_R\left(r\right)$ such that $a\ne 0,r,-r$. Suppose $y\sim a$. Then $x\sim 2r\sim a\sim y$ and so $d(x,y)\le 3$. Suppose $y\nsim a$. Then $[y,a]=r$ or $-r$. If $[y,a]=r$ then

$$[y,r+a]=[y,r]+[y,a]=-r+r=0.$$

Note that $r+a\in R\backslash Z\left(R\right)$; otherwise $a=-r$, a contradiction. Therefore, $y\sim r+a$. Furthermore,

$$[r+a,2r]=2[a,r]=0.$$

That is, $r+a\sim 2r$. Thus, $x\sim 2r\sim r+a\sim y$. Therefore, $d(x,y)\le 3$. If $[y,a]=-r$ then

$$[y,2r+a]=[y,2r]+[y,a]=r+(-r)=0.$$

Note that $2r+a\in R\backslash Z\left(R\right)$; otherwise $a=-2r=r$, a contradiction. Therefore, $y\sim 2r+a$. Furthermore,

$$[2r+a,2r]=2[a,r]=0.$$

That is, $2r+a\sim 2r$. Thus, $x\sim 2r\sim 2r+a\sim y$. Therefore, $d(x,y)\le 3$, and hence $diam\left({\Delta }_R^r\right)\le 3$.

Case 2:$x\nsim r$ and $x\nsim 2r$.

Let $a\in C_R\left(r\right)$ such that $a\ne 0,r,-r$.

Subcase 2.1:$x\sim a$

If $y\sim r$, then $y\sim r\sim a\sim x$. Therefore, $d(x,y)\le 3$. If $y\nsim r$ but $y\sim 2r$, then $y\sim 2r\sim a\sim x$. Therefore, $d(x,y)\le 3$. Consider the case when $y\nsim r$ as well as $y\nsim 2r$. Therefore $[y,r]=r$ or $-r$. If $[y,r]=r$, then $[y,2r]=2[y,r]=2r=-r$; otherwise $y\sim 2r$, a contradiction. Suppose $y\sim a$. Then $y\sim a\sim x$ and so $d(x,y)\le 2$. Suppose $y\nsim a$. Then $[y,a]=r$ or $-r$. If $[y,a]=r$ then $[y,r-a]=0$. Therefore, $y\sim r-a\sim a\sim x$. Therefore, $d(x,y)\le 3$. If $[y,a]=-r$, then $[y,2r-a]=0$. Therefore, $y\sim 2r-a\sim a\sim x$ and so $d(x,y)\le 3$.

If $[y,r]=-r$, then $[y,2r]=2[y,r]=-2r=r$; otherwise $y\sim 2r$, a contradiction. Suppose $y\sim a$. Then $y\sim a\sim x$ and so $d(x,y)\le 2$. Suppose $y\nsim a$. Then $[y,a]=r$ or $-r$. If $[y,a]=r$ then $[y,r+a]=0$. Therefore, $y\sim r+a\sim a\sim x$. Therefore, $d(x,y)\le 3$. If $[y,a]=-r$, then $[y,2r+a]=0$. Therefore, $y\sim 2r+a\sim a\sim x$ and so $d(x,y)\le 3$. Hence, $diam\left({\Delta }_R^r\right)\le 3$.

Subcase 2.2:$x\nsim a$

In this case we have $x\nsim r$ and $x\nsim 2r$. It can be seen that $[x,r]=r$ implies $[x,2r]=-r$, and $[x,r]=-r$ implies $[x,2r]=r$.

Suppose $[x,r]=r$ and $[x,a]=r$. Then $[x,r-a]=[x,r]-[x,a]=0$. Hence, $x\sim r-a$. Now, we have the following cases.

(i)

$x\sim r-a\sim r\sim y$ if $y\sim r$.

(ii)

$x\sim r-a\sim 2r\sim y$ if $y\nsim r$ but $y\sim 2r$.

Suppose $y\nsim r$ as well as $y\nsim 2r$. Then, proceeding as in Subcase 2.1, we get the following cases:

(iii)

$x\sim r-a\sim a\sim y$ if $y\nsim r$ and $2r$ but $y\sim a$.

(iv)

$y\sim r-a\sim x$ if $[y,r]=r$ and $[y,a]=r$.

(v)

$y\sim 2r-a\sim r-a\sim x$ if $[y,r]=r$ and $[y,a]=-r$.

(vi)

$y\sim r+a\sim r-a\sim x$ if $[y,r]=-r$ and $[y,a]=r$.

(vii)

$y\sim 2r+a\sim r-a\sim x$ if $[y,r]=-r$ and $[y,a]=-r$.

Therefore, $d(x,y)\le 3$.

Suppose $[x,r]=r$ and $[x,a]=-r$. Then

$$[x,2r-a]=[x,2r]-[x,a]=-r-(-r)=0.$$

Hence, $x\sim 2r-a$. Now, proceeding as above, we get the following cases:

(i)

$x\sim 2r-a\sim r\sim y$ if $y\sim r$.

(ii)

$x\sim 2r-a\sim 2r\sim y$ if $y\nsim r$ but $y\sim 2r$.

(iii)

$x\sim 2r-a\sim a\sim y$ if $y\nsim r$ and $2r$ but $y\sim a$.

(iv)

$y\sim r-a\sim 2r-a\sim x$ if $[y,r]=r$ and $[y,a]=r$.

(v)

$y\sim 2r-a\sim x$ if $[y,r]=r$ and $[y,a]=-r$.

(vi)

$y\sim r+a\sim 2r-a\sim x$ if $[y,r]=-r$ and $[y,a]=r$.

(vii)

$y\sim 2r+a\sim 2r-a\sim x$ if $[y,r]=-r$ and $[y,a]=-r$.

Therefore, $d(x,y)\le 3$.

Suppose $[x,r]=-r$ and $[x,a]=r$. Then

$$[x,r+a]=[x,r]+[x,a]=-r+r=0.$$

Hence, $x\sim r+a$. Proceeding as above, we get the following similar cases:

(i)

$x\sim r+a\sim r\sim y$ if $y\sim r$.

(ii)

$x\sim r+a\sim 2r\sim y$ if $y\nsim r$ but $y\sim 2r$.

(iii)

$x\sim r+a\sim a\sim y$ if $y\nsim r$ and $2r$ but $y\sim a$.

(iv)

$y\sim r-a\sim r+a\sim x$ if $[y,r]=r$ and $[y,a]=r$.

(v)

$y\sim 2r-a\sim r+a\sim x$ if $[y,r]=r$ and $[y,a]=-r$.

(vi)

$y\sim r+a\sim x$ if $[y,r]=-r$ and $[y,a]=r$.

(vii)

$y\sim 2r+a\sim r+a\sim x$ if $[y,r]=-r$ and $[y,a]=-r$.

Therefore, $d(x,y)\le 3$.

Suppose $[x,r]=-r$ and $[x,a]=-r$. Then

$$[x,2r+a]=[x,2r]+[x,a]=r+(-r)=0.$$

Hence, $x\sim 2r+a$, so we get the the following similar cases:

(i)

$x\sim 2r+a\sim r\sim y$ if $y\sim r$.

(ii)

$x\sim 2r+a\sim 2r\sim y$ if $y\nsim r$ but $y\sim 2r$.

(iii)

$x\sim 2r+a\sim a\sim y$ if $y\nsim r$ and $2r$ but $y\sim a$.

(iv)

$y\sim r-a\sim 2r+a\sim x$ if $[y,r]=r$ and $[y,a]=r$.

(v)

$y\sim 2r-a\sim 2r+a\sim x$ if $[y,r]=r$ and $[y,a]=-r$.

(vi)

$y\sim r+a\sim 2r+a\sim x$ if $[y,r]=-r$ and $[y,a]=r$.

(vii)

$y\sim 2r+a\sim x$ if $[y,r]=-r$ and $[y,a]=-r$.

Therefore, $d(x,y)\le 3$. Hence, in all the cases, $diam\left({\Delta }_R^r\right)\le 3$. This completes the proof. □

As a consequence of Proposition 1(a) and Corollary 1, we get the following result.

Corollary 2.

Let x be any vertex in ${\Delta }_R^r$.

(a)

If $r=0$ then $deg\left(x\right)=\left|R\right|-|C_R\left(x\right)|$.

(b)

If $r\ne 0$ and $2r=0$ then

$$deg\left(x\right)=\left\{\begin{array}{cc}\left|R\right|-\left|Z\right(R\left)\right|-1,\hfill & if T_{x,r}=\varnothing \hfill \\ \left|R\right|-\left|Z\left(R\right)\right|-|C_R\left(x\right)|-1,\hfill & otherwise.\hfill \end{array}\right.$$

(c)

If $r\ne 0$ and $2r\ne 0$ then

$$deg\left(x\right)=\left\{\begin{array}{cc}\left|R\right|-\left|Z\right(R\left)\right|-1,\hfill & if T_{x,r}=\varnothing \hfill \\ \left|R\right|-\left|Z\left(R\right)\right|-2|C_R\left(x\right)|-1,\hfill & otherwise.\hfill \end{array}\right.$$

Some applications of Corollary 2 are given below.

Theorem 2.

Let R be a noncommutative ring such that $\left|R\right|\ne 8$ and let $K_n$ be the complete graph on n-vertices. If ${\Delta }_R^r$ has an end vertex then $r\ne 0$ and ${\Delta }_R^{r\ne 0}=4K_2$ if and only if R is isomorphic to $E\left(9\right)$ or $F\left(9\right)$. Hence, ${\mathsf{\Gamma }}_R^r$ is neither a tree nor a lollipop graph.

Proof. 

Let $x\in R\backslash Z\left(R\right)$ be an end vertex in ${\Delta }_R^r$. Then $deg\left(x\right)=1$. If $r=0$ then, by Corollary 2(a), we have $deg\left(x\right)=\left|R\right|-|C_R\left(x\right)|$. Therefore, $\left|R\right|-|C_R\left(x\right)|=1$, and hence $|C_R\left(x\right)|=1$, a contradiction. Therefore, $r\ne 0$. Now, we consider the following cases.

Case 1:$r\ne 0$ and $2r=0$.

By Corollary 2(b), we have $deg\left(x\right)=\left|R\right|-\left|Z\right(R\left)\right|-1$ or $\left|R\right|-\left|Z\left(R\right)\right|-|C_R\left(x\right)|-1$. Hence $\left|R\right|-\left|Z\right(R\left)\right|-1=1$ or $\left|R\right|-\left|Z\left(R\right)\right|-|C_R\left(x\right)|-1=1$.

Subcase 1.1:$\left|R\right|-\left|Z\right(R\left)\right|=2$.

In this case, we have $\left|Z\right(R\left)\right|=1$ or 2. If $\left|Z\right(R\left)\right|=1$ then $\left|R\right|=3$, a contradiction. If $\left|Z\right(R\left)\right|=2$ then $\left|R\right|=4$. Therefore, the additive quotient group $\frac{R}{Z\left(R\right)}$ is cyclic. Hence, R is commutative; a contradiction.

Subcase 1.2:$\left|R\right|-\left|Z\left(R\right)\right|-|C_R\left(x\right)|=2$.

In this case, $\left|Z\right(R\left)\right|=1$ or 2. If $\left|Z\right(R\left)\right|=1$, then $\left|R\right|-|C_R\left(x\right)|=3$. Therefore, $|C_R\left(x\right)|=3$ and hence $\left|R\right|=6$. Therefore, R is commutative; a contradiction. If $\left|Z\right(R\left)\right|=2$, then $\left|R\right|-|C_R\left(x\right)|=4$. Therefore, $|C_R\left(x\right)|=4$ and so $\left|R\right|=8$, a contradiction.

Case 2:$r\ne 0$ and $2r\ne 0$.

By Corollary 2(c), we have $deg\left(x\right)=\left|R\right|-\left|Z\right(R\left)\right|-1$ or $\left|R\right|-\left|Z\left(R\right)\right|-2|C_R\left(x\right)|-1$. Hence, $\left|R\right|-\left|Z\right(R\left)\right|-1=1$ or $\left|R\right|-\left|Z\left(R\right)\right|-2|C_R\left(x\right)|-1=1$. If $\left|R\right|-\left|Z\right(R\left)\right|=2$, then, as shown in subcase 2.1, we get a contradiction. If $\left|R\right|-\left|Z\left(R\right)\right|-2|C_R\left(x\right)|=2$ then $\left|Z\right(R\left)\right|=1$ or 2.

Subcase 2.1:$\left|Z\right(R\left)\right|=1$.

In this case, $\left|R\right|-2|C_R\left(x\right)|=3$. Therefore, $|C_R\left(x\right)|=3$ and so $\left|R\right|=9$. Hence, R is isomorphic to either $E\left(9\right)$ or $F\left(9\right)$. It follows from Figure 4 that ${\Delta }_R^r=4K_2$, noting that ${\Delta }_{E\left(9\right)}^r$ and ${\Delta }_{F\left(9\right)}^r$ are isomorphic.

Subcase 2.2:$\left|Z\right(R\left)\right|=2$.

In this case, $\left|R\right|-2|C_R\left(x\right)|=4$. Therefore, $|C_R\left(x\right)|=4$ and so $\left|R\right|=12$. It follows that the additive quotient group $\frac{R}{Z\left(R\right)}$ is cyclic. Hence, R is commutative; a contradiction. Hence, the result follows. □

We have the following corollary to Theorem 2.

Corollary 3.

Let R be a noncommutative ring such that $\left|R\right|\ne 8$. Then

(a)

${\Delta }_R^r$ is 1-regular if and only if $r\ne 0$ and R is isomorphic to $E\left(9\right)$ or $F\left(9\right)$.

(b)

The noncommuting graph of R does not have any end vertex. In particular, noncommuting graph of such ring is neither a tree nor a lollipop graph.

Proof. 

The results follow from Theorem 2; note that any 1-regular graph has end vertices and noncommuting graph of R is the graph ${\Delta }_R^0$. □

Theorem 3.

Let R be a noncommutative ring such that $\left|R\right|\ne 8,12$. If ${\Delta }_R^r$ has a vertex of degree 2 then $r=0$ and ${\Delta }_R^0$ is a triangle if and only if R is isomorphic to $E\left(4\right)$ or $F\left(4\right)$.

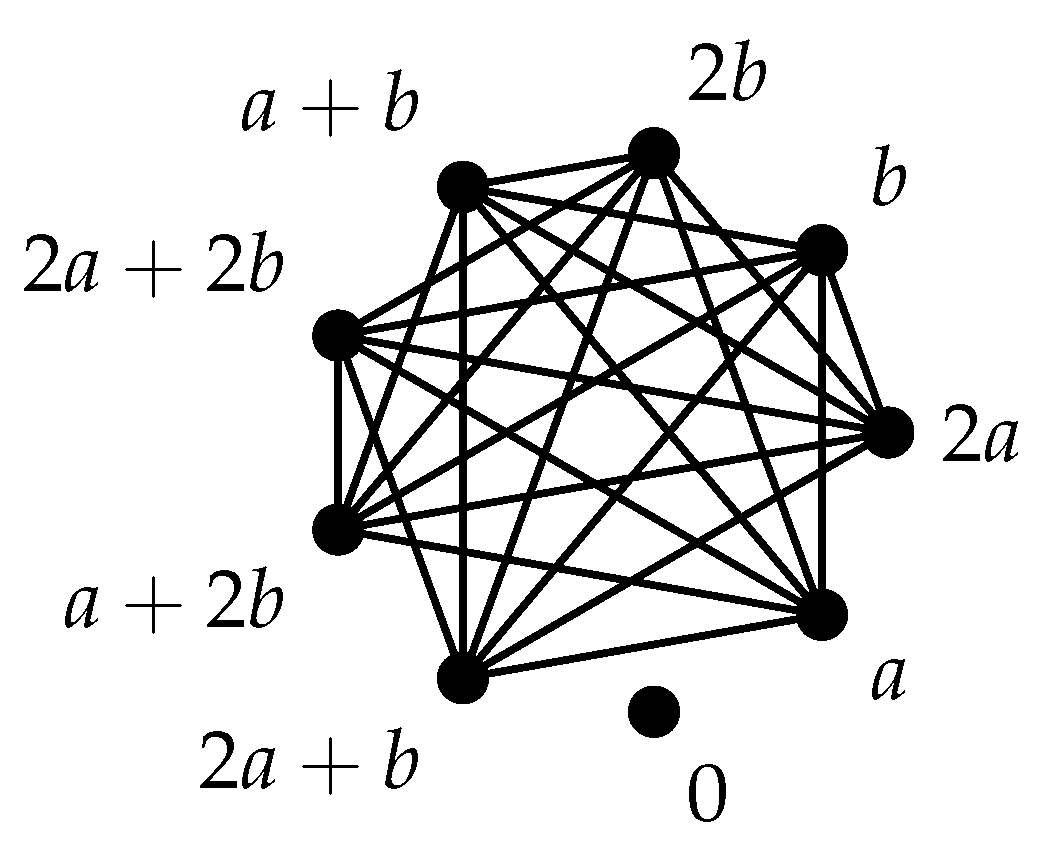
Proof. 

Suppose ${\Delta }_R^r$ has a vertex x of degree 2. Consider the following cases.

Case 1:$r=0$.

By Corollary 2(a), we have $deg\left(x\right)=\left|R\right|-|C_R\left(x\right)|$. Therefore, $\left|R\right|-|C_R\left(x\right)|=2$, and hence $|C_R\left(x\right)|=2$. Therefore, $\left|R\right|=4$ and so R is isomorphic to $E\left(4\right)$ or $F\left(4\right)$. Hence, ${\Delta }_R^r$ is a triangle (as shown in Figure 1; note that ${\Delta }_{E\left(4\right)}^r$ and ${\Delta }_{F\left(4\right)}^r$ are isomorphic).

Case 2:$r\ne 0$ and $2r=0$.

By Corollary 2(b), we have $deg\left(x\right)=\left|R\right|-\left|Z\right(R\left)\right|-1$ or $deg\left(x\right)=\left|R\right|-\left|Z\left(R\right)\right|-|C_R\left(x\right)|-1$. Therefore, $\left|R\right|-\left|Z\right(R\left)\right|-1=2$ or $\left|R\right|-\left|Z\left(R\right)\right|-|C_R\left(x\right)|-1=2$.

Subcase 2.1:$\left|R\right|-\left|Z\right(R\left)\right|=3$.

In this case we have $\left|Z\right(R\left)\right|=1$ or 3. If $\left|Z\right(R\left)\right|=1$, then $\left|R\right|=4$. As shown in Figure 2, ${\Delta }_R^r$ is a null graph on three vertices. Therefore, it has no vertex of degree 2, which is a contradiction. If $\left|Z\right(R\left)\right|=3$ then $\left|R\right|=6$. Therefore, R is commutative; a contradiction.

Subcase 2.2:$\left|R\right|-\left|Z\left(R\right)\right|-|C_R\left(x\right)|=3$.

In this case, $\left|Z\right(R\left)\right|=1$ or 3. If $\left|Z\right(R\left)\right|=1$, then $\left|R\right|-|C_R\left(x\right)|=4$. Therefore, $|C_R\left(x\right)|=2$ or 4 and hence $\left|R\right|=6$ or 8; a contradiction. If $\left|Z\right(R\left)\right|=3$, then $\left|R\right|-|C_R\left(x\right)|=6$. Therefore, $|C_R\left(x\right)|=6$ and so $\left|R\right|=12$, which contradicts our assumption.

Case 3:$r\ne 0$ and $2r\ne 0$.

By Corollary 2(c), we have $deg\left(x\right)=\left|R\right|-\left|Z\right(R\left)\right|-1$ or $\left|R\right|-\left|Z\left(R\right)\right|-2|C_R\left(x\right)|-1$. Hence, $\left|R\right|-\left|Z\right(R\left)\right|-1=2$ or $\left|R\right|-\left|Z\left(R\right)\right|-2|C_R\left(x\right)|-1=2$.

If $\left|R\right|-\left|Z\right(R\left)\right|=3$, then, as shown in Subcase 2.1, we get a contradiction. If $\left|R\right|-\left|Z\left(R\right)\right|-2|C_R\left(x\right)|=3$, then $\left|Z\right(R\left)\right|=1$ or 3.

Subcase 3.1:$\left|Z\right(R\left)\right|=1$.

In this case, $\left|R\right|-2|C_R\left(x\right)|=4$. Therefore, $|C_R\left(x\right)|=2$ or 4 and hence $\left|R\right|=8$ or 12, which is a contradiction.

Subcase 3.2:$\left|Z\right(R\left)\right|=3$.

In this case, $\left|R\right|-2|C_R\left(x\right)|=6$. Therefore, $|C_R\left(x\right)|=6$ and so $\left|R\right|=18$. It follows that the additive quotient group $\frac{R}{Z\left(R\right)}$ is cyclic. Hence, R is commutative; a contradiction. Hence, the result follows. □

We have the following corollary to Theorem 3.

Corollary 4.

Let R be a noncommutative ring such that $\left|R\right|\ne 8,12$. Then

(a)

${\Delta }_R^r$ is 2-regular if and only if $r=0$ and R is isomorphic to $E\left(4\right)$ or $F\left(4\right)$.

(b)

The noncommuting graph of R is 2-regular if and only if R is isomorphic to $E\left(4\right)$ or $F\left(4\right)$.

Proof. 

The results follow from Theorem 3 noting the facts that any 2-regular graph has vertices of degree 2 and noncommuting graph of R is the graph ${\Delta }_R^0$. □

Theorem 4.

Let R be a noncommutative ring such that $\left|R\right|\ne 16,18$. Then the graph ${\Delta }_R^r$ has no vertex of degree 3.

Proof. 

Suppose ${\Delta }_R^r$ has a vertex x of degree 3.

Case 1:$r=0$.

By Corollary 2(a), we have $deg\left(x\right)=\left|R\right|-|C_R\left(x\right)|$. Therefore, $\left|R\right|-|C_R\left(x\right)|=3$ and hence $|C_R\left(x\right)|=3$. Therefore, $\left|R\right|=6$ and hence R is commutative; a contradiction.

Case 2:$r\ne 0$ and $2r=0$.

By Corollary 2(b), we have $deg\left(x\right)=\left|R\right|-\left|Z\right(R\left)\right|-1$ or $deg\left(x\right)=\left|R\right|-\left|Z\left(R\right)\right|-|C_R\left(x\right)|-1$. Therefore, $\left|R\right|-\left|Z\right(R\left)\right|-1=3$ or $\left|R\right|-\left|Z\left(R\right)\right|-|C_R\left(x\right)|-1=3$.

Subcase 2.1:$\left|R\right|-\left|Z\right(R\left)\right|=4$.

In this case, we have $\left|Z\right(R\left)\right|=1$ or 2 or 4. If $\left|Z\right(R\left)\right|=1$ or 2, then $\left|R\right|=5$ or 6 and hence R is commutative; a contradiction. If $\left|Z\right(R\left)\right|=4$, then $\left|R\right|=8$. Therefore, the additive quotient group $\frac{R}{Z\left(R\right)}$ is cyclic. Hence, R is commutative; a contradiction.

Subcase 2.2:$\left|R\right|-\left|Z\left(R\right)\right|-|C_R\left(x\right)|=4$.

In this case, $\left|Z\right(R\left)\right|=1$ or 2 or 4. If $\left|Z\right(R\left)\right|=1$, then $\left|R\right|-|C_R\left(x\right)|=5$. Therefore, $|C_R\left(x\right)|=5$ and hence $\left|R\right|=10$. Therefore, R is commutative; a contradiction. If $\left|Z\right(R\left)\right|=2$, then $\left|R\right|-|C_R\left(x\right)|=6$. Therefore, $|C_R\left(x\right)|=6$ and so $\left|R\right|=12$. It follows that the additive quotient group $\frac{R}{Z\left(R\right)}$ is cyclic. Hence, R is commutative; a contradiction. If $\left|Z\right(R\left)\right|=4$, then $\left|R\right|-|C_R\left(x\right)|=8$. Therefore, $|C_R\left(x\right)|=8$ and so $\left|R\right|=16$; a contradiction.

Case 3:$r\ne 0$ and $2r\ne 0$.

By Corollary 2(c), we have $deg\left(x\right)=\left|R\right|-\left|Z\right(R\left)\right|-1$ or $\left|R\right|-\left|Z\left(R\right)\right|-2|C_R\left(x\right)|-1$. Hence, $\left|R\right|-\left|Z\right(R\left)\right|-1=3$ or $\left|R\right|-\left|Z\left(R\right)\right|-2|C_R\left(x\right)|-1=3$.

If $\left|R\right|-\left|Z\right(R\left)\right|=4$, then, as shown in Subcase 2.1, we get a contradiction. If $\left|R\right|-\left|Z\left(R\right)\right|-2|C_R\left(x\right)|=4$, then $\left|Z\right(R\left)\right|=1$ or 2 or 4.

Subcase 3.1:$\left|Z\right(R\left)\right|=1$.

In this case, $\left|R\right|-2|C_R\left(x\right)|=5$. Therefore, $|C_R\left(x\right)|=5$ then $\left|R\right|=15$. Therefore, R is commutative; a contradiction.

Subcase 3.2:$\left|Z\right(R\left)\right|=2$.

In this case, $\left|R\right|-2|C_R\left(x\right)|=6$. Therefore, $|C_R\left(x\right)|=6$ and so $\left|R\right|=18$; a contradiction.

Subcase 3.3:$\left|Z\right(R\left)\right|=4$.

In this case, $\left|R\right|-2|C_R\left(x\right)|=8$. Therefore, $|C_R\left(x\right)|=8$ and so $\left|R\right|=24$. It follows that the additive quotient group $\frac{R}{Z\left(R\right)}$ is cyclic. Hence, R is commutative; a contradiction. This completes the proof. □

Corollary 5.

Let R be a noncommutative ring such that $\left|R\right|\ne 16,18$. Then ${\Delta }_R^r$ is not 3-regular. In particular, the noncommuting graph of such R is not 3-regular.

Theorem 5.

Let R be a noncommutative ring such that $\left|R\right|\ne 8,12,18,20$. Then ${\Delta }_R^r$ has no vertex of degree 4.

Proof. 

Suppose ${\Delta }_R^r$ has a vertex x of degree 4.

Case 1:$r=0$.

By Corollary 2(a), we have $deg\left(x\right)=\left|R\right|-|C_R\left(x\right)|$. Therefore, $\left|R\right|-|C_R\left(x\right)|=4$ and hence $|C_R\left(x\right)|=2$ or 4. If $|C_R\left(x\right)|=2$, then $\left|R\right|=6$ and hence R is commutative; a contradiction. If $|C_R\left(x\right)|=4$, then $\left|R\right|=8$; a contradiction.

Case 2:$r\ne 0$ and $2r=0$.

By Corollary 2(b), we have $deg\left(x\right)=\left|R\right|-\left|Z\right(R\left)\right|-1$ or $deg\left(x\right)=\left|R\right|-\left|Z\left(R\right)\right|-|C_R\left(x\right)|-1$. Therefore, $\left|R\right|-\left|Z\right(R\left)\right|-1=4$ or $\left|R\right|-\left|Z\left(R\right)\right|-|C_R\left(x\right)|-1=4$.

Subcase 2.1:$\left|R\right|-\left|Z\right(R\left)\right|=5$.

In this case we have $\left|Z\right(R\left)\right|=1$ or 5. Then $\left|R\right|=6$ or 10 and hence R is commutative; a contradiction.

Subcase 2.2:$\left|R\right|-\left|Z\left(R\right)\right|-|C_R\left(x\right)|=5$.

In this case, $\left|Z\right(R\left)\right|=1$ or 5. If $\left|Z\right(R\left)\right|=1$, then $\left|R\right|-|C_R\left(x\right)|=6$. Therefore, $|C_R\left(x\right)|=2$ or 3 or 6. If $|C_R\left(x\right)|=2$, then $\left|R\right|=8$; a contradiction. If $|C_R\left(x\right)|=3$, then $\left|R\right|=9$. It follows from Figure 4 that ${\Delta }_R^r=4K_2$, which is a contradiction. If $|C_R\left(x\right)|=6$, then $\left|R\right|=12$; a contradiction. If $\left|Z\right(R\left)\right|=5$, then $\left|R\right|-|C_R\left(x\right)|=10$. Therefore, $|C_R\left(x\right)|=10$ and so $\left|R\right|=20$; a contradiction.

Case 3:$r\ne 0$ and $2r\ne 0$.

By Corollary 2(c), we have $deg\left(x\right)=\left|R\right|-\left|Z\right(R\left)\right|-1$ or $\left|R\right|-\left|Z\left(R\right)\right|-2|C_R\left(x\right)|-1$. Hence, $\left|R\right|-\left|Z\right(R\left)\right|-1=4$ or $\left|R\right|-\left|Z\left(R\right)\right|-2|C_R\left(x\right)|-1=4$.

If $\left|R\right|-\left|Z\right(R\left)\right|=5$, then, as shown in Subcase 2.1, we get a contradiction. If $\left|R\right|-\left|Z\left(R\right)\right|-2|C_R\left(x\right)|=5$, then $\left|Z\right(R\left)\right|=1$ or 5.

Subcase 3.1:$\left|Z\right(R\left)\right|=1$.

In this case, $\left|R\right|-2|C_R\left(x\right)|=6$. Therefore, $|C_R\left(x\right)|=2$ or 3 or 6. If $|C_R\left(x\right)|=2$, then $\left|R\right|=10$. Therefore R is commutative; a contradiction. If $|C_R\left(x\right)|=3$ or 6, then $\left|R\right|=12$ or 18; a contradiction.

Subcase 3.2:$\left|Z\right(R\left)\right|=5$.

In this case, $\left|R\right|-2|C_R\left(x\right)|=10$. Therefore, $|C_R\left(x\right)|=10$ and so $\left|R\right|=30$. It follows that the additive quotient group $\frac{R}{Z\left(R\right)}$ is cyclic. Hence, R is commutative; a contradiction. This completes the proof. □

Corollary 6.

Let R be a noncommutative ring such that $\left|R\right|\ne 8,12,18,20$. Then ${\Delta }_R^r$ is not 4-regular. In particular, the noncommuting graph of such R is not 4-regular.

Theorem 6.

Let R be a noncommutative ring such that $\left|R\right|\ne 8,16,24,27$. Then ${\Delta }_R^r$ has no vertex of degree 5.

Proof. 

Suppose ${\Delta }_R^r$ has a vertex x of degree 5.

Case 1:$r=0$.

By Corollary 2(a), we have $deg\left(x\right)=\left|R\right|-|C_R\left(x\right)|$. Therefore, $\left|R\right|-|C_R\left(x\right)|=5$, and hence $|C_R\left(x\right)|=5$. Then $\left|R\right|=10$ and hence R is commutative; a contradiction.

Case 2:$r\ne 0$ and $2r=0$.

By Corollary 2(b), we have $deg\left(x\right)=\left|R\right|-\left|Z\right(R\left)\right|-1$ or $deg\left(x\right)=\left|R\right|-\left|Z\left(R\right)\right|-|C_R\left(x\right)|-1$. Therefore, $\left|R\right|-\left|Z\right(R\left)\right|-1=5$ or $\left|R\right|-\left|Z\left(R\right)\right|-|C_R\left(x\right)|-1=5$.

Subcase 2.1:$\left|R\right|-\left|Z\right(R\left)\right|=6$.

In this case we have $\left|Z\right(R\left)\right|=1$ or 2 or 3 or 6. If $\left|Z\right(R\left)\right|=1$, then $\left|R\right|=7$ and hence R is commutative; a contradiction. If $\left|Z\right(R\left)\right|=2$, then $\left|R\right|=8$; a contradiction. If $\left|Z\right(R\left)\right|=3$, then $\left|R\right|=9$. It follows from Figure 4 that ${\Delta }_R^r=4K_2$, which is a contradiction. If $\left|Z\right(R\left)\right|=6$, then $\left|R\right|=12$. Therefore, the additive quotient group $\frac{R}{Z\left(R\right)}$ is cyclic. Hence, R is commutative; a contradiction.

Subcase 2.2:$\left|R\right|-\left|Z\left(R\right)\right|-|C_R\left(x\right)|=6$.

In this case, $\left|Z\right(R\left)\right|=1$ or 2 or 3 or 6. If $\left|Z\right(R\left)\right|=1$, then $\left|R\right|-|C_R\left(x\right)|=7$. Therefore, $|C_R\left(x\right)|=7$ then $\left|R\right|=14$, and hence R is commutative; a contradiction. If $\left|Z\right(R\left)\right|=2$, then $\left|R\right|-|C_R\left(x\right)|=8$. Therefore, $|C_R\left(x\right)|=4$ or 8. If $|C_R\left(x\right)|=4$, then $\left|R\right|=12$. Therefore, the additive quotient group $\frac{R}{Z\left(R\right)}$ is cyclic. Hence, R is commutative; a contradiction. If $|C_R\left(x\right)|=8$, then $\left|R\right|=16$; a contradiction. If $\left|Z\right(R\left)\right|=3$, then $\left|R\right|-|C_R\left(x\right)|=9$. Therefore, $|C_R\left(x\right)|=9$, so $\left|R\right|=18$. It follows that the additive quotient group $\frac{R}{Z\left(R\right)}$ is cyclic. Hence, R is commutative; a contradiction. If $\left|Z\right(R\left)\right|=6$, then $\left|R\right|-|C_R\left(x\right)|=12$. Therefore, $|C_R\left(x\right)|=12$, so $\left|R\right|=24$; a contradiction.

Case 3:$r\ne 0$ and $2r\ne 0$.

By Corollary 2(c), we have $deg\left(x\right)=\left|R\right|-\left|Z\right(R\left)\right|-1$ or $\left|R\right|-\left|Z\left(R\right)\right|-2|C_R\left(x\right)|-1$. Hence, $\left|R\right|-\left|Z\right(R\left)\right|-1=5$ or $\left|R\right|-\left|Z\left(R\right)\right|-2|C_R\left(x\right)|-1=5$.

If $\left|R\right|-\left|Z\right(R\left)\right|=6$, then, as shown in Subcase 2.1, we get a contradiction. If $\left|R\right|-\left|Z\left(R\right)\right|-2|C_R\left(x\right)|=6$, then $\left|Z\right(R\left)\right|=1$ or 2 or 3 or 6.

Subcase 3.1:$\left|Z\right(R\left)\right|=1$.

Here we have, $\left|R\right|-2|C_R\left(x\right)|=7$. Therefore, $|C_R\left(x\right)|=7$ then $\left|R\right|=21$ and hence R is commutative; a contradiction.

Subcase 3.2:$\left|Z\right(R\left)\right|=2$.

In this case, $\left|R\right|-2|C_R\left(x\right)|=8$. Therefore, $|C_R\left(x\right)|=4$ or 8. If $|C_R\left(x\right)|=4$ or 8, then $\left|R\right|=16$ or 24; a contradiction.

Subcase 3.3:$\left|Z\right(R\left)\right|=3$.

In this case, $\left|R\right|-2|C_R\left(x\right)|=9$. Therefore, $|C_R\left(x\right)|=9$ and so $\left|R\right|=27$; a contradiction.

Subcase 3.4:$\left|Z\right(R\left)\right|=6$.

In this case, $\left|R\right|-2|C_R\left(x\right)|=12$. Therefore, $|C_R\left(x\right)|=12$ and so $\left|R\right|=36$. It follows that the additive quotient group $\frac{R}{Z\left(R\right)}$ is cyclic. Hence, R is commutative; a contradiction. This completes the proof. □

Corollary 7.

Let R be a noncommutative ring such that $\left|R\right|\ne 8,16,24,27$. Then ${\Delta }_R^r$ is not 5-regular. In particular, the noncommuting graph of this R is not 5-regular.

We conclude this section with the following characterization of R.

Theorem 7.

Let R be a noncommutative ring such that $\left|R\right|\ne 8,12,16$, $24,28$. Then ${\Delta }_R^r$ has a vertex of degree 6 if and only if $r=0$ and R is isomorphic to $E\left(9\right)$ or $F\left(9\right)$.

Proof. 

Suppose ${\Delta }_R^r$ has a vertex x of degree 6.

Case 1:$r=0$.

By Corollary 2(a), we have $deg\left(x\right)=\left|R\right|-|C_R\left(x\right)|$. Therefore, $\left|R\right|-|C_R\left(x\right)|=6$ and hence $|C_R\left(x\right)|=2$ or 3 or 6. If $|C_R\left(x\right)|=2$, then $\left|R\right|=8$; a contradiction. If $|C_R\left(x\right)|=3$, then $\left|R\right|=9$. Therefore, ${\Delta }_R^r$ is a 6-regular graph (as shown in Figure 3). If $|C_R\left(x\right)|=6$, then $\left|R\right|=12$; a contradiction.

Case 2:$r\ne 0$ and $2r=0$.

By Corollary 2(b), we have $deg\left(x\right)=\left|R\right|-\left|Z\right(R\left)\right|-1$ or $deg\left(x\right)=\left|R\right|-\left|Z\left(R\right)\right|-|C_R\left(x\right)|-1$. Therefore $\left|R\right|-\left|Z\right(R\left)\right|-1=6$ or $\left|R\right|-\left|Z\left(R\right)\right|-|C_R\left(x\right)|-1=6$.

Subcase 2.1:$\left|R\right|-\left|Z\right(R\left)\right|=7$.

In this case we have $\left|Z\right(R\left)\right|=1$ or 7. If $\left|Z\right(R\left)\right|=1$, then $\left|R\right|=8$; a contradiction. If $\left|Z\right(R\left)\right|=7$, then $\left|R\right|=14$ and hence R is commutative; a contradiction.

Subcase 2.2:$\left|R\right|-\left|Z\left(R\right)\right|-|C_R\left(x\right)|=7$.

In this case, $\left|Z\right(R\left)\right|=1$ or 7. If $\left|Z\right(R\left)\right|=1$, then $\left|R\right|-|C_R\left(x\right)|=8$. Therefore, $|C_R\left(x\right)|=2$ or 4 or 8. If $|C_R\left(x\right)|=2$, then $\left|R\right|=10$. Thus, R is commutative; a contradiction. If $|C_R\left(x\right)|=4$ or 8, then $\left|R\right|=12$ or 16; which are contradictions. If $\left|Z\right(R\left)\right|=7$, then $\left|R\right|-|C_R\left(x\right)|=14$. Therefore, $|C_R\left(x\right)|=14$ and so $\left|R\right|=28$; a contradiction.

Case 3:$r\ne 0$ and $2r\ne 0$.

By Corollary 2(c), we have $deg\left(x\right)=\left|R\right|-\left|Z\right(R\left)\right|-1$ or $\left|R\right|-\left|Z\left(R\right)\right|-2|C_R\left(x\right)|-1$. Hence, $\left|R\right|-\left|Z\right(R\left)\right|-1=6$ or $\left|R\right|-\left|Z\left(R\right)\right|-2|C_R\left(x\right)|-1=6$.

If $\left|R\right|-\left|Z\right(R\left)\right|=7$, then as shown in Subcase 2.1, we get a contradiction. If $\left|R\right|-\left|Z\left(R\right)\right|-2|C_R\left(x\right)|=7$, then $\left|Z\right(R\left)\right|=1$ or 7.

Subcase 3.1:$\left|Z\right(R\left)\right|=1$.

In this case, $\left|R\right|-2|C_R\left(x\right)|=8$. Therefore, $|C_R\left(x\right)|=2$ or 4 or 8, and then $\left|R\right|=12$ or 16 or 24; all are contradictions to the order of R.

Subcase 3.2:$\left|Z\right(R\left)\right|=7$.

In this case, $\left|R\right|-2|C_R\left(x\right)|=14$. Therefore, $|C_R\left(x\right)|=14$ and so $\left|R\right|=42$. It follows that the additive quotient group $\frac{R}{Z\left(R\right)}$ is cyclic. Hence, R is commutative; a contradiction. This completes the proof. □

Corollary 8.

Let R be a noncommutative ring such that $\left|R\right|\ne 8,12,16$, $24,28$. Then ${\Delta }_R^r$ is 6-regular if and only if $r=0$ and R is isomorphic to $E\left(9\right)$ or $F\left(9\right)$. In particular, the noncommuting graph of such R is 6-regular if and only if R is isomorphic to $E\left(9\right)$ or $F\left(9\right)$.

4. Concluding Remarks

In this paper, we have obtained results on ${\mathsf{\Gamma }}_R^r$ and ${\Delta }_R^r$ analogous to certain results for g-noncommuting graphs of finite groups obtained in [18,20]. Of course, we have obtained results not analogous to the results for g-noncommuting graphs of finite groups. However, it will be interesting to discover more properties of ${\mathsf{\Gamma }}_R^r$ and ${\Delta }_R^r$ different from the case of groups. Many of our results that characterize finite noncommutative rings such that the graph ${\Delta }_R^r$ is n-regular for $1\le n\le 6$ involve conditions on $\left|R\right|$. Therefore, the question of recognizing rings with these graphs is still not clear for such cases. One may continue further research to remove those conditions on $\left|R\right|$ and recognize the rings clearly. It is also worth determining all the positive integers n such that ${\Delta }_R^r$ is n-regular.