1. Introduction
Hypercompositional algebra is the modern theory of hypercompositional structures, which are algebraic structures having at least one hyperoperation. The output of a hyperoperation on a set H is not just an element, as the result of classical operation, but a subset of H. The firstly introduced hypercompositional structure was the hypergroup, defined by F. Marty in 1934, as a natural generalization of group, proving that the quotient of a group by any of its subgroups (not necessarily normal) is a hypergroup.
In 1956, Krasner [
1] solved a problem in the approximation of a complete valued field by a sequence of such fields by a using a new hypercompositional structure, which he called hyperfield. Many years later, the same Krasner [
2] introduced the notion of hyperring, as the hypercompositional structure that we now call
Krasner hyperring. In the same paper, he also gave the definition of a hypermodule over a hyperring, now called
Krasner hypermodule. The additive part of all these structures is a canonical hypergroup, with many applications in hypercompositional algebra. At the beginning, Krasner hyperrings, hyperfields, and hypermodules were studied by Krasner himself and their students Mittas and Stratigopoulos, mostly for their applications in the classical algebra. The theoretical basis of hypermodules has been settled by Massouros [
3], when he gave important examples of hypermodules and introduced free and cyclic hypermodules. In 2008, Anvariyeh et al. [
4,
5] studied the fundamental relation
defined on a hypermodule, in the same way that Vougiouklis [
6] defined fundamental relations on hyperrings and Koskas [
7] on hypergroups. Details about fundamental relations in hypercompositional structures can be read in [
8], while new aspects of this theory are collected in [
9,
10,
11]. The study of the categorial aspects of the theory of Krasner hypermodules was initiated by Madanshekaf [
12] and deepened by Shojaei and Ameri [
13,
14,
15]. The latter authors have recently defined [
16] several types of
projective and
injective hypermodules based on different kinds of epimorphisms and monomorphisms that exist in Krasner hypermodule category. We explain them in the next section.
In this paper, we focus our study on a particular type of homomorphisms between hypermodules, called
normal homomorphisms, and consequently on the normal projective and normal injective hypermodules. The main aim of the manuscript is to give an equivalent definition of these hypermodules by using exact chains of Krasner hypermodules and normal homomorphisms. This new approach will permit us to also obtain new results in other categories, because the injectivity plays a fundamental role not only in Krasner hypermodule category, but also in other categories. For example, in the category of Boolean algebras, a complete Boolean algebra is injective [
17]. In the category of posets, the injective objects are the Dedekind-MacNeille completions [
18], while the field of real numbers is injective in the category of Banach spaces.
The rest of the paper is organized as follows. In
Section 2, we fix the notation and explain the terminology, as well as we provide the basic definitions and results concerning Krasner hypermodules.
Section 3 is dedicated to the study of various chains of Krasner hypermodules. This is based on the family
of all normal homomorphisms between two
R-hypermodules
M and
N over a Krasner hyperring
R, which we first endow with an
R- hypermodule structure. Then we establish a relationship between the exactness of a chain of
R-hypermodules and the corresponding chain of the sets of all normal
R-homomorphisms obtained by a fixed
R-hypermodule. In
Section 4, we re-define the normal injective and projective Krasner
R-hypermodules by using exact chains of Krasner hypermodules, showing that the new definitions are equivalent to those given in [
16]. Moreover, we present a new characterization of normal injective
R-hypermodules by considering an arbitrary hyperideal of
R as a Krasner hypermodule. Concluding remarks and future works are gathered in the last section of the paper.
2. Preliminaries
Throughout this paper, unless stated otherwise, R denotes a Krasner hyperring, which we call here, for short, hyperring, and the family of all non-empty subsets of R.
Definition 1 ([
1])
. A (Krasner) hyperring is a hyperstructure where is a canonical hypergroup, i.e.,
- (a)
- (b)
- (c)
- (d)
- (e)
- (f)
is a semigroup with a bilaterally absorbing element 0, i.e.,
- (a)
- (b)
- (c)
The product distributes from both sides over the hyperaddition, i.e.,
- (a)
Moreover, a hyperring is called commutative if is commutative, i.e.,
- 4.
.
Finally, if is a monoid, i.e.,
- 5.
, then we say that R is with a unit element, or a unitary hyperring.
Definition 2. A hyperring homomorphism is a mapping f from a hyperring to a hyperring with the unit elements and such that
The concept of hypermodule over a Krasner hyperring was introduced by Krasner himself and studied later more in detail for their algebraic properties in [
3,
19]. In 2016, Shojeai et al. [
13] named this hypermodule a
Krasner hypermodule and started their categorical study.
Definition 3. Let R be a hyperring with the unit element 1. A canonical hypergroup together with a left external map defined bysuch that for all and , we have .
,
which is called a left Krasner hypermodule over R, or for short, a left R-hypermodule. Similarly, one may define a right R-hypermodule. For simplicity, in this paper, we consider only left R-hypermodules, which we call R-hypermodules.
Definition 4. A subhypermodule N of Mis a subhypergroup of M that is also closed under multiplication by elements of R.
As already mentioned by Krasner and then very clearly explained by Massouros [
3], we may define more types of homomorphisms between
R-hypermodules.
Definition 5 ([
3])
. Let M and N be two R-hypermodules. A multivalued function is called an R-homomorphism if:- (i)
- (ii)
while f is called strong homomorphism if instead of we have
- (i′)
A singlevalued function is called a strict R-homomorphism if the axioms and are valid, and it is called a normal R-homomorphism if and are valid.
Notice that in the more recently published papers [
13,
14,
16], a similar terminology is used, but here we want to keep the original one. This is why the next definitions are slightly changed with respect to their form in [
16].
Definition 6. Let R be a hyperring and M and N be R-hypermodules. The family of all normal R-homomorphisms from M to N is denoted by , while the family of all strict homomorphisms from M to N is denoted by .
Definition 7. Let (respectively ). Then, f is called
- (i)
a surjective (normal) R-homomorphism if ;
- (ii)
an injective (normal) R-homomorphism if for all , implies ;
- (iii)
(normal) R-isomorphism if it is a bijective (normal) R-homomorphism.
Definition 8. For a normal homomorphism , the set is called the kernel of f and denoted by .
Then, it is easy to see that the R-normal homomorphism f is injective if and only if , so the zero subhypermodule of M.
Inspired by the similar notions defined in the category of modules, in [
16], the authors introduced different types of projectivity and injectivity for Krasner hypermodules. Herein, we recall with our notations those connected with normal
R-homomorphisms, consequently called
normal projectivity and injectivity, which we will use in
Section 4.
Definition 9 ([
16])
. Let R be a hyperring.- (i)
An R-hypermodule P is called normal projective if for every surjective and every , there exist such that .
- (ii)
An R-hypermodule E is called normal injective if for every injective and every , there exists such that
3. Chains of R-Hypermodules
In this section, we first introduce the notion of exact chain of R-hypermodules and normal R-homomorphisms. Then, we prove that the set of all normal R-homomorphisms between two R-hypermodules M and N, denoted by , is an R-hypermodule only when R is commutative. Finally, we study the relationship between the exactness of the chains of R-hypermodules and the corresponding sets of all normal R-homomorphisms obtained by a fixed R-hypermodule.
Note that the hyperring R needs not to be a commutative one, unless this is stated.
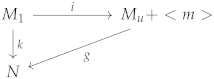
Proposition 1. Let R be a hyperring and an injective normal R-homomorphism. Then, there exists an R-hypermodule that is an extension of M (i.e., there exists an inclusion mapping from M to ) and a normal R-isomorphism such that for every , .
Proof. Set
. Define
, and for each
, consider the map
Then g is a bijective map and an extension of f, meaning that for every , .
By the help of
g, we show that
has an
R-hypermodule structure. Suppose that
, and
are arbitrary elements. Then
. Since
is an
R-hypermodule, it follows that
and
. Now we define
Using these definitions for + and ·, the set has the structure of an R-hypermodule and clearly it is an extension of M.
Furthermore, from (
3), we have
Thus, g is a normal R-homomorphism and a bijective map. Therefore g is a normal R-isomorphism and the proof is complete. □
Definition 10. Let , , and be R-hypermodules and consider the following chain of normal R-homomorphismsIf , then the chain in (4) is said to be a zero chain.
Moreover, if , then the chain in (4) is said to be an exact chain.
Lemma 1. The chainis an exact chain of R-hypermodules if f is an injective normal R-homomorphism, while the chainis an exact chain of R-hypermodules if g is a surjective normal R-homomorphism. Proof. The proof is straightforward. □
Based on Lemma 1, the following result is obvious.
Corollary 1. The chain of R-hypermodulesis an exact chain if f is an injective normal R-homomorphism, g is a surjective normal R-homomorphism, and . Example 1. Suppose that M is an R-hypermodule and N is a subhypermodule of M. Then, the following chainis an exact one, where i is the inclusion function and ρ is the projection function, i.e., . Example 2. Let R be a hyperring, M be an R-hypermodule and be a family of subhypermodules of M. Then, the sum
of this family is denoted by , and it is the family of the sets , where for every , . More specifically,where is a set (in particular a subset of M) and not only an element, since + is a hyperoperation on M, whilewhere the set can be written as the union . Clearly, the structure is a subhypermodule of M, and it is the smallest subhypermodule of M containing every . Moreover, the intersection of the family , denoted by , is the largest subhypermodule of M, which is contained in every .
Suppose now that and are R-hypermodules and is their sum as defined by (9). Then the following chainis an exact one, where i is the injection function defined as , i.e., the set containing only the element , and p is the projection function, such that . In the following, we endow the set of all normal R-homomorphisms between two R-hypermodules M and N with an R-hypermodule structure.
Definition 11. Let M and N be R-hypermodules. For any two normal R-homomorphisms and , definewhere is the hyperaddition on the R-hypermodule N. This means thatthus, ⊕ is a hyperoperation on the set . Using the associativity, commutativity, and reproduction axiom of the hypergroup , we can easily verify that the hypergroupoid () is a canonical hypergroup. Remark 1. The zero mapping is the identity element of this hypergroup, i.e., for , . If either or , then is a trivial hypergroup and clearly .
Theorem 1. Let R be a commutative hyperring and M, N be R-hypermodules. Then, is also an R-hypermodule.
Proof. Using Definition 11, it follows that
is a canonical hypergroup. Now, for any
and
, define the
R-multiplication ⊗ as follows:
Then since for , and , we have
- (i)
- (ii)
since
R is a commutative hyperring,
It remains to prove that is an R-hypermodule. For and , we have the following assertions, for an arbitrary element :
- (i)
- (ii)
- (iii)
- (iv)
For the zero element 0 of
, there is
- (v)
We can conclude now that is an R-hypermodule. □
Suppose that
R is a hyperring and
M is an
R-hypermodule. Every element of the
R-hypermodule
is called a normal
R-
endomorphism of
M and it is a normal
R-homomorphism from
M into itself. Accordingly, we denote
by
. For an arbitrary element
and
, define the multiplication on
by
With this operation, we endow with a hyperring structure, as explained in the following result.
Lemma 2. Let R be a commutative hyperring and M be an R-hypermodule. Then, is a hyperring with the hyperoperation ⊕ defined in (11) and the operation · defined by (14). Proof. Using Definition 11, it follows that is a commutative hypergroup. It is a routine to check that the multiplication operation is associative and distributive over the hyperoperation ⊕. Additionally, the hyperring has a unit element. This is the identity mapping . □
Definition 12. is called the hyperring of R-endomorphisms of M.
We shall now define a normal
R-homomorphism between two
R-hyper-modules
and
. Thus, let
,
and
M be
R-hypermodules and
be a normal
R-homomorphism. Define the map
F as follows:
where
is defined by
for any
. Since
R is a commutative hyperring, using Theorem 1, we conclude that
and
are
R-hypermodules. In addition, we get
When
, we conclude that for any
, there is
Clearly, for any , . Therefore, F is a normal R-homomorphism.
The next step in our study is to define chains of
R-hypermodules of normal
R-homomorphisms. Therefore, from the chain of
R-hypermodules
,
, and normal
R-homomorphisms
f and
g
we can derive the following chain of
R-hypermodules and normal
R-homomorphisms
F and
G
where for every
, we have
and for every
, it holds that
.
The next result states a relationship between the exactness of the chains defined in (
15) and (
16).
Proposition 2. If the chain defined by (15) is an exact one, then the chain in (16) is exact, too. Proof. Suppose that the chain defined by (
15) is exact. Then, using Lemma 1, it follows that
and
f is a normal monomorphism. In order to show that the chain in (
16) is exact, it is enough to prove that the homomorphism
F is a normal monomorphism (i.e.,
) and then that
.
Suppose that
such that
, i.e.,
. Then
,meaning that that, for any element
,
. Since the chain defined in (
15) is exact, the results show that
f is a normal monomorphism, so
and then
, for every element
. Thereby,
and thus
F is a normal monomorphism.
Let us prove now that
. Take
. Then
and
. So, for every
, we have
. Thus,
. Hence, there exists
such that
. Since
f is a normal monomorphism, it follows that there is only one
with the property that
. Now define
such that
. Clearly,
is a normal homomorphism, i.e.,
. Then for any element
,
Thus, . Hence, .
Conversely, suppose that
. Then there exists
such that
. Hence,
. Now consider
, and since the chain in (
15) is exact, it follows that
and
. This implies that the chain defined by (
16) is exact as well. □
We continue by defining another type of chains of
R-hypermodules. Suppose that
,
, and
N are
R-hypermodules and
is a normal
R-homomorphism. Define the map
as follows:
where
and for
,
. Since
R is a commutative hyperring, using Theorem 1, it follows that
and
are hypermodules and
is a normal homomorphism.
Now consider the following chain of
R-hypermodules
,
and
, and normal
R-homomorphisms
and
,
From this chain and based on the above discussion, we can derive the following chain of
R-hypermodules and normal
R-homomorphisms:
where for every
,
and for every
,
.
Similarly to Proposition 2, we obtain the following result.
Proposition 3. If the chain defined by (17) is exact, then the chain in (18) is also exact. Proof. Suppose that the chain defined by (
17) is exact. Then, by Lemma 1, the results show that
and
is a surjective normal
R-homomorphism.
Let k be an arbitrary element in and . Then . Now suppose that is an arbitrary element. Since is a surjective normal R-homomorphism, there exists such that . Hence, . Thus, for every element , we have , which means that and is an injective normal R-homomorphism.
Suppose that
. Then
. We should find
such that
. Suppose that
is an arbitrary element. Then, since
is a normal
R-homomorphism, there exists
such that
. Now, define
k as follows:
Then clearly
, and for any element
we have
Thus, .
It remains to show that the function in (
19) is well defined. For doing this, suppose that
and
. Then
We know that
. Thus,
. Therefore, there exists
such that
. Therefore,
and thus there exists
such that
. Hence,
Therefore,
, and since
is a canonical hypergroup, using Definition 1 part (e) of 1, we conclude that
. Therefore, the relation (
19) is well defined and
. This means that
and
.
Now we have to prove that
. Suppose that
. Then, there exists
such that
. So, for
we have
The last equation follows because the chain in (
17) is exact and so
. Thus,
. Therefore,
and the chain defined in (
18) is exact as requested. □
4. Normal Injective and Projective R-Hypermodules
The aim of this section is to provide an alternative definition of the normal injective and projective
R-hypermodules introduced in [
16], based on the notion of exact chains, which will permit us to better understand their relationships with the hyperring
R and the hyperideals of
R.
Consider the chain
of
R-hypermodules
,
and normal
R-homomorphisms
f and
g. In Proposition 2, we proved that the exactness of the above chain implies the exactness of the following chain
It is worth noticing that, generally, the exactness of the chain of
R-hypermodules
and
does not imply the exactness of the chain
In order to give a positive answer to this general problem, we introduce a particular class of R-hypermodules.
Definition 13. Let R be a hyperring and M be an R-hypermodule. If the exactness of the chain defined by (20), for two arbitrary R-hypermodules and , implies the exactness of the chain defined in (21), then the R-hypermodule M is called a normal projective
R-hypermodule. Similarly, a dual concept can be defined, by reversing all the arrows. Consider the chain
of
R-hypermodules
,
, and normal
R-homomorphisms
and
. According to Proposition 3, the exactness of the above chain implies the exactness of the following chain
while generally, the exactness of the chain
of
R-hypermodules
and
does not imply the exactness of the chain
Definition 14. Let R be a hyperring and N be an R-hypermodule. If the exactness of the chain defined in (22) for any R-hypermodules and implies the exactness of the chain defined in (23), then the R-hypermodule N is called a normal injective
R-hypermodule. The notions of normal injective and projective
R-hypermodules have been recently introduced in [
16], as we recalled them in Definition 9. Here, we re-define them by the mean of exact chains of
R-hypermodules and we show that these definitions are equivalent.
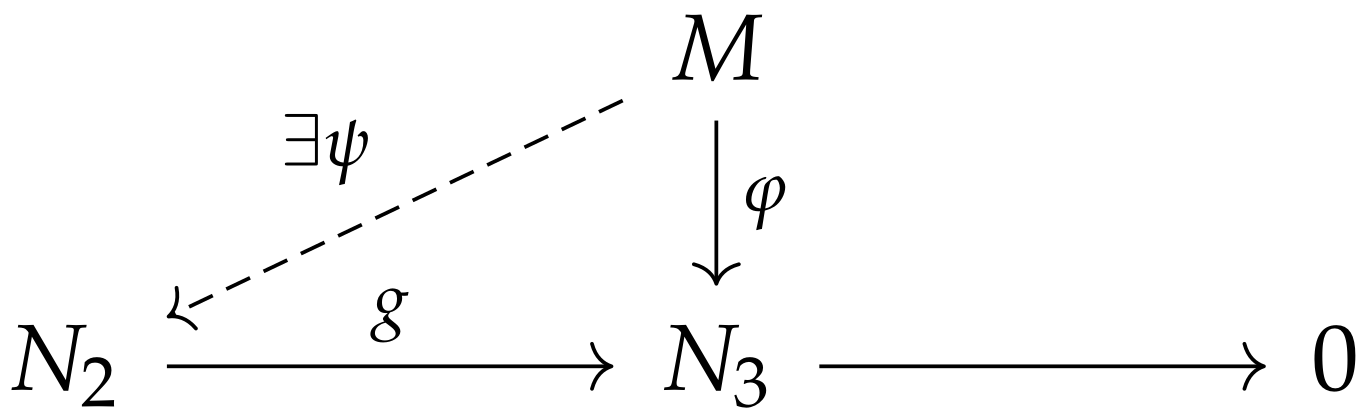
Theorem 2. Let R be a hyperring and N be an R-hypermodule. Then, the following statements are equivalent:
- (i)
For any exact chainof R-hypermodules and normal R-homomorphisms, the chainis also exact. - (ii)
For any R-hypermodules , and normal R-homomorphisms and such that the chain is exact, there exists a normal R-homomorphism such that the diagram in Figure 1 has the composition structure, i.e., .
Proof. Suppose that assertion
holds. Using Proposition 3 and Definition 14, we conclude that assertion
is equivalent to the fact that, if the chain
is exact, then the chain
is exact too, where the mapping
is defined as follows
Since the chain (
24) is exact, we have
This means that if we have the diagram in
Figure 1 with the exact row and
; then, there exists
such that
equivalently, the diagram has the composition structure.
Now suppose that assertion
holds. Since
is an arbitrary element of
, we conclude that for any
, if the chain
is exact, then there exists
such that
. This means that the normal
R-homomorphism
is surjective, and thus, using Proposition 3, assertion
also holds. □
Remark 2. By Lemma 1, it follows that assertion in Theorem 2 is equivalent with the definition of normal injectivity introduced in [16] (and recalled here in Definition 9), while assertion is equivalent with the same notion introduced in Definition 14. Therefore, we say that an R-hypermodule N is normal injective
if it satisfies the equivalent conditions and in Theorem 2. We may provide a similar characterization for normal projective R-hypermodules.
Theorem 3. Let R be a hyperring and M be an R-hypermodule. Then, the following statements are equivalent:
- (i)
For any exact chainof R-hypermodules and normal R-homomorphisms, the chainis also exact. - (ii)
For any R-hypermodules and normal R-homomorphisms and such that the chain is exact, there exists a normal R-homomorphism such that the diagram in Figure 2 has the composition structure, i.e., .
Proof. Similar to the proof of Theorem 2. □
Remark 3. An R-hypermodule M is called normal projective
if it satisfies the equivalent conditions and of Theorem 3, where the first one is equivalent with the notion defined in Definition 13, while the second one is exactly the definition given in [16] (see Definition 9). We conclude this section with a different characterization of normal injective R-hypermodules. One of the most commonly used equivalents of the axiom of choice is Zorn’s lemma. For a partially ordered set (also called a poset) P, a in P is a nonempty subset S of P such that S is totally ordered, meaning that any two elements of S are comparable.
Lemma 3. (Zorn’s lemma) If a poset P has the property that every chain in P has an upper bound, then P has a maximal element.
Based on Zorn’s lemma, the following theorem provides another equivalent definition of a normal injective
R-hypermodule by considering
R and an arbitrary hyperideal
I of
R as
R-hypermodules (for further details, refer to [
11]).
Theorem 4. Let R be a hyperring and N be an R-hypermodule. Then, the following statements are equivalent:
- (1)
N is a normal injective R-hypermodule.
- (2)
For any hyperideal I of R, an inclusion hyperring homomorphism , and a normal R-homomorphism , there exists a normal R-homomorphism such that the diagram in Figure 3 has the composition structure, i.e., .
Proof. Using Remark 2, it is enough to show that the assertion in Theorem 2 and the statement of this theorem are equivalent.
Assume that N is a normal injective R-hypermodule. Thus, the assertion in Theorem 2 holds. Since the hyperideal I can be considered as an R-hypermodule, statement is also true.
Now suppose that statement holds. Moreover, let , and N be arbitrary R-hypermodules and and be normal R-homomorphisms such that the chain is exact. Denote by ∑ the set of all pairs , where M is an R-hypermodule that contains and has the property that there exists an injective normal R-homomorphism from M to and is a normal R-homomorphism such that the following diagram has the composition structure, i.e., :
Then, ∑ is not empty, because . Define an order ≤ on ∑ such that if and only if is a subhypermodule of and is an extension of . This means that following diagram has the composition structure, i.e., .
Then is a partially ordered set. Suppose that is a totally ordered subset of ∑. Let and define by , where . Clearly, and it is an upper bound for an arbitrary chain . Thus, using Lemma 3, we conclude that ∑ has a maximal element . Now it is enough to show that .
If
, then there is an element
m such that
and
. Consider
I the set of all elements
such that
, i.e.,
By a routine verification, we can check that
I is a hyperideal. Now, define
such that for
,
Then
is a normal
R-homomorphism of
R-hypermodules because, for
and
, we have
and
By statement , there exists a normal R-homomorphism such that the following diagram has the composition structure, i.e., .
Consider the
R-hypermodule
and define the normal
R-homomorphism
such that, for
and
,
Since
, we conclude that
and there exists an injective normal R-homomorphism from
to
. Moreover, for
Thus, . Therefore, we have the following diagram with the composition structure.
This means that
, which is a contradiction because
is the maximal element of ∑. So,
. Hence, we proved that
and there exists the function
such that the diagram in
Figure 4 has a compositional structure.
Using Theorem 2, we conclude that N is a normal injective R-hypermodule. □
Finally, we may summarize the characterization of a normal injective R-hypermodule as follows.
Theorem 5. An R-hypermodule N is normal injective if it satisfies the following equivalent conditions.
- (i)
For any exact chainof R-hypermodules and normal R-homomorphisms, the chainis also exact. - (ii)
For any R-hypermodules , and normal R-homomorphisms and such that the chain is exact, there exists a normal R-homomorphism such that .
- (iii)
For any hyperideal I of R, any inclusion hyperring homomorphism , and normal R-homomorphism , there exists a normal R-homomorphism such that .