Applying a Simple Analytical Solution to Modelling Wind-Driven Coastal Upwelling of Two-Layered Fluid at the Head of Tokyo Bay, Japan
Abstract
:1. Introduction
2. Simple Explanation for Blue Tide Occurrence and Derivation of the Analytical Solution
- (1)
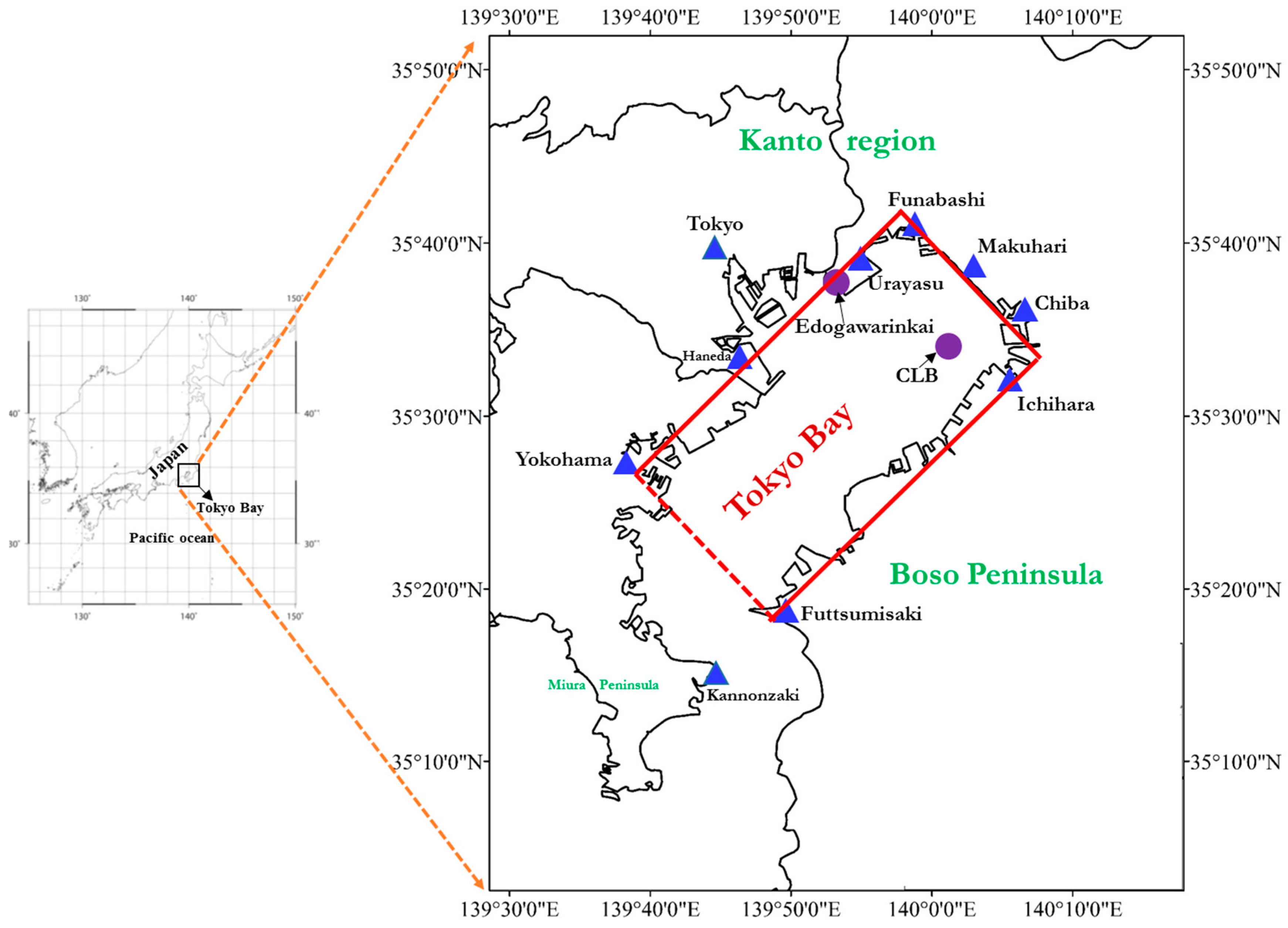
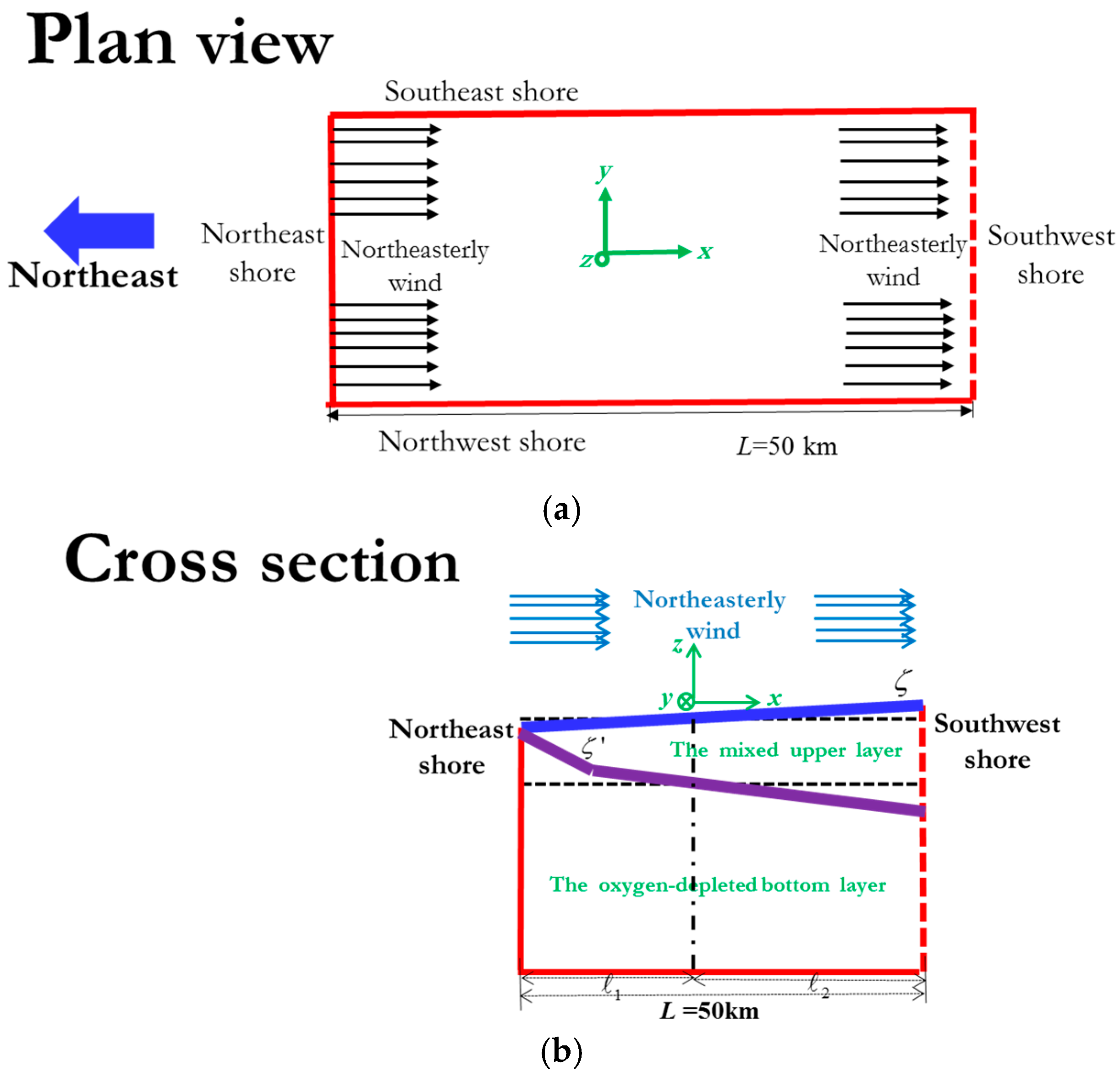
- Although the actual coastline of Tokyo Bay is irregular, the chosen domain was considered to be a rectangular flat domain 50 km in length, 20 km in width and with an average water depth of 15 m, as indicated by three solid red lines and a dashed red line showing the imaginary southwest shore in Figure 1. The remaining 10 km is the outer bay, which does not have not much exchange with the inner bay, as shown by many studies [15,22,26,28]. Figure 2a schematically shows the dimensions of the chosen research domain, where is the length, and in the right-handed Cartesian coordinate system, the axis follows the northeasterly wind, the axis is perpendicular to the length direction, and the axis is positive in the upward direction relative to the quiescent surface water level;
- (2)
- A imple two-layered fluid model consisting of an upper wind-driven water layer and a bottom oxygen-depleted water layer was adopted to represent the actual stratified flow in Tokyo Bay. Similar studies have been carried out by Otsubo et al. [5], Nakatsuji et al. [16] and Yoon et al. [17,18,19]. Figure 2b schematically shows this conceptual two-layered fluid model.
3. Comparison with Observational Data
3.1. Handling of the Observational Datasets
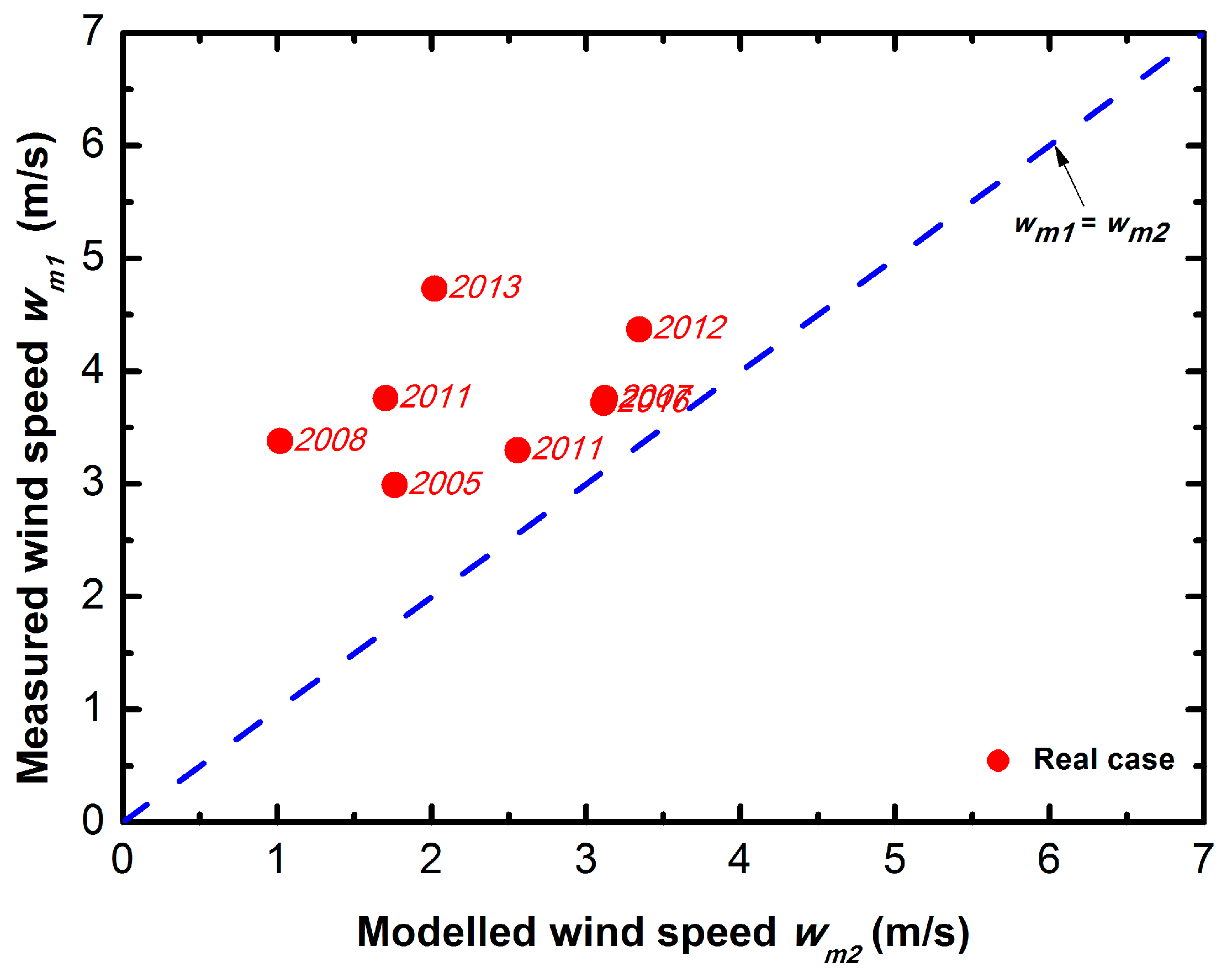
3.2. Comparison with Real Cases of Blue Tides on the Northeast Shore
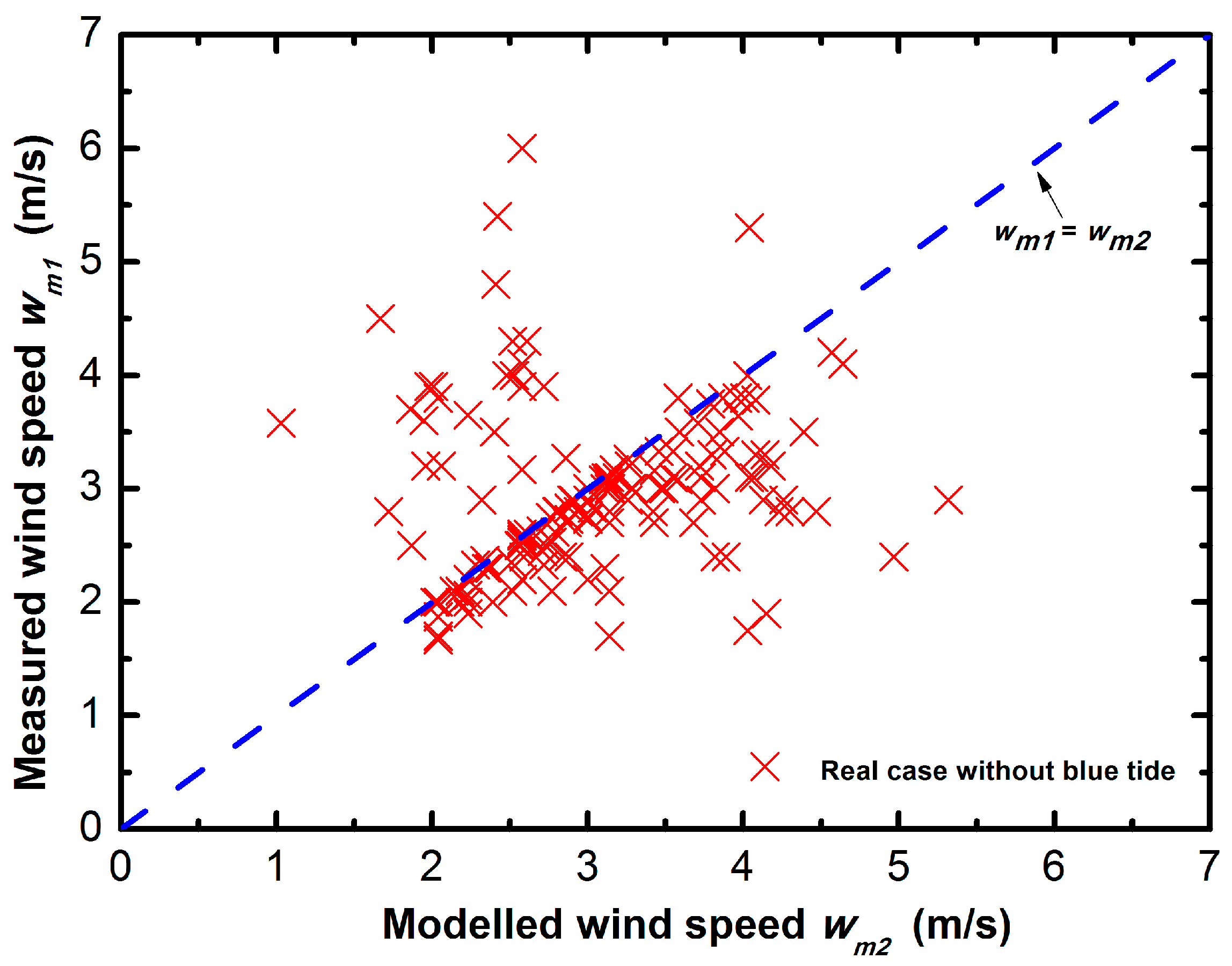
3.3. Comparison with Real Cases Lacking Blue Tides
4. Discussion and Concluding Remarks
4.1. Discussion
4.2. Concluding Remarks
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Yunus, A.P.; Dou, J.; Sravanthi, N. Remote sensing of chlorophyll-a as a measure of red tide in Tokyo Bay using hotspot analysis. Remote Sens. Appl. Soc. Environ. 2015, 2, 11–25. [Google Scholar] [CrossRef]
- Koibuchi, Y.; Isobe, M. Phytoplankton Bloom Mechanism in an Area Affected by Eutrophication: Tokyo Bay. Coast. Eng. J. 2007, 49, 461–479. [Google Scholar] [CrossRef]
- Higa, H.; Koibuchi, Y.; Kobayashi, H.; Toratani, M.; Sakuno, Y. Numerical Simulation and Remote Sensing for the Analysis of Blue Tide Distribution in Tokyo Bay in September 2012. J. Adv. Simul. Sci. Eng. 2015, 2, 1–15. [Google Scholar] [CrossRef]
- Sasaki, J. Coastal upwelling of water body at the head of Tokyo Bay and its influence to “Aoshio”. Proc. Coast. Eng. JSCE 1997, 44, 1101–1105. (In Japanese) [Google Scholar]
- Otsubo, K.; Harashima, A.; Miyazaki, T.; Yasuoka, Y.; Muraoka, K. Field survey and hydraulic study of “Aoshio” in Tokyo Bay. Mar. Pollut. Bull. 1991, 23, 51–55. [Google Scholar] [CrossRef]
- Kakino, J.; Matsuyama, S.; Sato, Y.; Kase, N. Relationship between Aoshio, blue-green turbid water and wind-driven current. Nippon Suisan Gakkaishi 1987, 53, 1475–1481. (In Japanese) [Google Scholar] [CrossRef]
- Kataoka, S.; Hirotaka, H.; Komatsu, A. On the occurrene condition of blue tide in the inner Tokyo Bay. Proc. Ocean Dev. JSCE 1989, 5, 233–238. (In Japanese) [Google Scholar]
- Zhu, Z. Minimum wind stress for the occurrence of blue tide on the southeast shore of Tokyo Bay. J. Geosci. Environ. Prot. 2014, 2, 126–134. [Google Scholar] [CrossRef]
- Zhu, Z.; Yu, J. Estimating the occurrence of wind-driven coastal upwelling associated with “aoshio” on the northeast shore of Tokyo Bay, Japan: An analytical model. Sci. World J. 2014, 2014. [Google Scholar] [CrossRef] [PubMed]
- Sasaki, J.; Isobe, M.; Watanabe, A.; Gomyo, M. The phenomenon regarding the anoxic water and a model of seasonal variations of temperature and DO in Tokyo Bay. Proc. Coast. Eng. JSCE 1993, 40, 1051–1055. (In Japanese) [Google Scholar]
- Sasaki, J.; Isobe, M.; Watanabe, A.; Gomyo, M. Study on scale of blue tide in Tokyo Bay. Proc. Coast. Eng. JSCE 1996, 43, 1111–1115. (In Japanese) [Google Scholar]
- Sasaki, J.; Isobe, M.; Huzimoto, H. Development of simple methods of predicting blue tide in Tokyo Bay. Proc. Coast. Eng. JSCE 1999, 46, 1006–1010. (In Japanese) [Google Scholar]
- Sasaki, J.; Koide, M.; Nagata, M.; Shibayama, T.; Isobe, M. Numerical study on improvement effect of blue tide water using microbubbles generator in Sanbanze of Tokyo Bay. Proc. Coast. Eng. JSCE 2003, 50, 981–985. (In Japanese) [Google Scholar]
- Takahashi, T.; Nakata, H.; Hirano, K.; Matsuoka, K.; Iwataki, M.; Yamaguchi, H.; Kasuya, T. Upwelling of oxygen-depleted water (Sumishio) in Omura Bay, Japan. J. Oceanogr. 2009, 65, 113–120. [Google Scholar] [CrossRef]
- Matsuyama, M.; Touma, K.; Ohwaki, A. Numerical experiments of upwelling in Tokyo Bay in relation to “Aoshio” (the upwelled anoxic blue-green turbid water). Bull. Coast. Oceanogr. 1990, 28, 63–74, (In Japanese and English Abstract). [Google Scholar]
- Nakatsuji, K.; Nagasaka, S.; Muraoka, K. Basic experiments on upwelling of anoxic water observed in Tokyo Bay, Japan. Proc. Hydraul. Eng. JSCE 1991, 35, 603–608. (In Japanese) [Google Scholar] [CrossRef]
- Yoon, J.S.; Nakatsuji, K.; Muraoka, K. Experimental study on wind-driven stratified flow in enclsoed water body. Proc. Hydraul. Eng. JSCE 1993, 37, 285–292. (In Japanese) [Google Scholar] [CrossRef]
- Yoon, J.S.; Nakatsuji, K.; Muraoka, K. Experimental study on wind-driven stratified flow in open water body. Proc. Coast. Eng. JSCE 1993, 40, 241–245. (In Japanese) [Google Scholar]
- Yoon, J.S.; Nakatsuji, K.; Muraoka, K. Density interface movement and mixing in a two-layered stratified flow system. Proc. Hydraul. Eng. JSCE 1995, 39, 811–818. (In Japanese) [Google Scholar] [CrossRef]
- Nakatsuji, K.; Yoon, J.S.; Yuasa, T.; Muraoka, K. The relationship between wind-driven stratified flow and occurrence mechanism of blue tide. Proc. Coast. Eng. JSCE 1995, 42, 1066–1070. (In Japanese) [Google Scholar]
- Tsukada, M.; Mimura, N.; Suzuki, M. Numerical simulation on formation, maintenance and attenuation of anoxic water body in Tokyo Bay. Proc. Coast. Eng. JSCE 1997, 44, 1086–1090. (In Japanese) [Google Scholar]
- Watanabe, M.; Amano, K.; Ishikawa, Y.; Kohata, K. Analysis of autumn upwelling of anoxic bottom water, distribution and vertical circulation induced by wind in Tokyo Bay. J. Jpn. Soc. Civ. Eng. 1998, 608, 13–29. (In Japanese) [Google Scholar]
- Koibuchi, Y.; Isobe, M. Study on “Aoshio” around Yokohama harbor at the western side of Tokyo Bay in 2004. Proc. Coast. Eng. JSCE 2005, 52, 896–900. (In Japanese) [Google Scholar]
- Sasaki, J.; Kawamoto, S.; Yoshimoto, Y.; Ishii, M.; Kakino, J. Evaluation of the effect of anoxic water in dredged trenches on blue tides in Tokyo Bay. Proc. Coast. Eng. JSCE 2007, 54, 1041–1045. (In Japanese) [Google Scholar] [CrossRef]
- Ichioka, S.; Sasaki, J.; Yoshimoto, Y.; Shimosako, K.; Kimura, S. Analysis of Sulfide Dynamics Including Outbreak of Blue Tide in Navigation Channels and Dredged Trench of Tokyo Bay. J. Jpn. Soc. Civ. Eng. Ser. B2 Coast. Eng. 2009, 65, 1041–1045. [Google Scholar] [CrossRef]
- Suzuki, T.; Matsuyama, M. Numerical Experiments on Stratified Wind-induced Circulation in Tokyo Bay, Japan. Estuar. Coast. Shelf Sci. 2000, 50, 17–25. [Google Scholar] [CrossRef]
- Sasaki, J.; Kawamoto, S.; Yoshimoto, Y.; Ishii, M.; Kakino, J. Evaluation of the Amount of Hydrogen Sulfide in a Dredged Trench of Tokyo Bay. J. Coast. Res. 2009, 1, 890–894. [Google Scholar]
- Ueno, S.; Nadaoka, K.; Ishimura, A.; Katsui, H. Analysis on the upwelling due to wind in Tokyo Bay based on NOAA-AVHRR data. Proc. Coast. Eng. JSCE 1992, 39, 256–260. (In Japanese) [Google Scholar]
- Csanady, G.T. Circulation in the Coastal Ocean; D. Reidel Publishing Company: Dordrecht, The Netherlands, 1982; pp. 25–63. ISBN 978-90-481-8372-2. [Google Scholar]
- Mccutcheon, S.C.; Martin, J.L.; Barnwell, T.O. Water quality. In Handbook of Hydrology, 1st ed.; Maidment, D.R., Ed.; McGraw-Hill: New York, NY, USA, 1993; pp. 191–197. ISBN 9780070397323. [Google Scholar]
- Angeli, C.; Leonardi, E.; Maciocco, L. A computational study of salt diffusion and heat extraction in solar pond plants. Sol. Energy 2006, 80, 1498–1508. [Google Scholar] [CrossRef]
- Mogollón, J.M.; Dale, A.W.; Jensen, J.B.; Schlüter, M.; Regnier, P. A method for the calculation of anaerobic oxidation of methane rates across regional scales: An example from the Belt Seas and The Sound (North Sea-Baltic Sea transition). Geo-Mar. Lett. 2013, 33, 299–310. [Google Scholar] [CrossRef]
- Stamou, A.I.; Nikiforakis, I.K. Integrated modelling of single port, steady-state thermal discharges in unstratified coastal waters. Environ. Fluid Mech. 2013, 13, 309–336. [Google Scholar] [CrossRef]
- Conroy, J.L.; Thompson, D.M.; Collins, A.; Overpeck, J.T.; Bush, M.B.; Cole, J.E. Climate influences on water and sediment properties of Genovesa Crater Lake, Galápagos. J. Paleolimnol. 2014, 52, 331–347. [Google Scholar] [CrossRef]
- Oh, S.H.; Kim, B.M.; Kang, N. Evaluation of changes in cylinder volume due to gas filling and subsequent release. Metrologia 2013, 50, 318–324. [Google Scholar] [CrossRef]
- Diamantopoulos, S.; Milickovic, N.; Butt, S.; Katsilieri, Z.; Kefala, V.; Zogal, P.; Sakas, G.; Baltas, D. Effect of using different U/S probe Standoff materials in image geometry for interventional procedures: The example of prostate. J. Contemp. Brachytherapy 2011, 3, 209–219. [Google Scholar] [CrossRef] [PubMed]



| Month | Year | Surface Temperature (°C) | Surface Salinity (psu) | The Upper Layer Density (kg/m3) | Bottom Temperature (°C) | Bottom Salinity (psu) | The Lower Layer Density (kg/m3) | Average Density Value of the Upper Layer (kg/m3) | Average Density Value of the Bottom Layer (kg/m3) |
|---|---|---|---|---|---|---|---|---|---|
| May | 2003 | 19.00 | 29.80 | 1021.08 | 16.80 | 32.20 | 1023.44 | 1021.12 | 1023.06 |
| 2004 | 18.00 | 30.20 | 1021.63 | 16.80 | 31.80 | 1023.13 | |||
| 2005 | 18.00 | 31.00 | 1022.24 | 16.80 | 32.60 | 1023.75 | |||
| 2006 | 18.00 | 30.20 | 1021.63 | 16.80 | 31.80 | 1023.13 | |||
| 2007 | 18.60 | 30.20 | 1021.48 | 18.00 | 31.40 | 1022.55 | |||
| 2008 | 16.80 | 31.00 | 1022.52 | 15.00 | 32.20 | 1023.84 | |||
| 2009 | 19.20 | 29.80 | 1021.03 | 16.80 | 31.40 | 1022.83 | |||
| 2010 | 18.00 | 29.00 | 1020.71 | 15.60 | 31.00 | 1022.79 | |||
| 2012 | 19.20 | 27.80 | 1019.51 | 16.80 | 31.00 | 1022.52 | |||
| 2014 | 20.40 | 29.40 | 1020.42 | 18.00 | 31.80 | 1022.85 | |||
| 2015 | 20.40 | 29.00 | 1020.12 | 18.00 | 31.80 | 1022.85 | |||
| June | 2003 | 22.80 | 29.00 | 1019.47 | 20.40 | 31.80 | 1022.25 | 1019.20 | 1022.75 |
| 2004 | 22.80 | 27.80 | 1018.57 | 20.40 | 31.00 | 1021.64 | |||
| 2005 | 21.60 | 29.80 | 1020.41 | 18.00 | 32.60 | 1023.46 | |||
| 2006 | 20.40 | 29.00 | 1020.12 | 16.80 | 33.00 | 1024.05 | |||
| 2007 | 22.80 | 29.00 | 1019.47 | 19.20 | 31.80 | 1022.56 | |||
| 2008 | 21.60 | 27.00 | 1018.29 | 19.20 | 31.80 | 1022.56 | |||
| 2009 | 21.60 | 27.00 | 1018.29 | 18.00 | 31.40 | 1022.55 | |||
| 2010 | 21.60 | 28.00 | 1019.04 | 19.20 | 31.00 | 1021.95 | |||
| 2012 | 21.60 | 29.00 | 1019.80 | 19.00 | 31.80 | 1022.61 | |||
| 2014 | 21.80 | 27.80 | 1018.84 | 19.20 | 32.20 | 1022.86 | |||
| 2015 | 21.60 | 27.80 | 1018.89 | 18.00 | 33.00 | 1023.77 | |||
| July | 2003 | 22.80 | 29.00 | 1019.47 | 19.60 | 32.60 | 1023.06 | 1017.67 | 1021.96 |
| 2004 | 26.40 | 28.20 | 1017.82 | 22.80 | 31.00 | 1020.99 | |||
| 2005 | 24.00 | 27.00 | 1017.63 | 20.40 | 32.20 | 1022.55 | |||
| 2006 | 24.00 | 28.20 | 1018.53 | 19.20 | 32.20 | 1022.86 | |||
| 2007 | 24.00 | 29.00 | 1019.14 | 20.40 | 32.60 | 1022.86 | |||
| 2008 | 26.40 | 25.00 | 1015.42 | 19.20 | 31.40 | 1022.25 | |||
| 2009 | 25.20 | 27.00 | 1017.28 | 21.60 | 30.20 | 1020.71 | |||
| 2010 | 26.40 | 27.00 | 1016.92 | 21.60 | 31.00 | 1021.32 | |||
| 2012 | 25.20 | 27.80 | 1017.88 | 20.40 | 31.00 | 1021.64 | |||
| 2014 | 26.40 | 26.60 | 1016.62 | 20.40 | 31.80 | 1022.25 | |||
| 2015 | 24.00 | 27.00 | 1017.63 | 20.40 | 30.20 | 1021.03 | |||
| August | 2003 | 26.40 | 27.00 | 1016.92 | 22.80 | 30.60 | 1020.69 | 1016.91 | 1020.91 |
| 2004 | 26.40 | 29.00 | 1018.42 | 25.20 | 31.00 | 1020.29 | |||
| 2005 | 27.60 | 25.00 | 1015.05 | 21.60 | 31.80 | 1021.93 | |||
| 2006 | 26.40 | 27.00 | 1016.92 | 19.20 | 31.80 | 1022.56 | |||
| 2007 | 28.20 | 27.00 | 1016.36 | 24.00 | 31.00 | 1020.65 | |||
| 2008 | 26.40 | 27.00 | 1016.92 | 24.00 | 31.00 | 1020.65 | |||
| 2009 | 26.40 | 27.80 | 1017.52 | 21.60 | 31.40 | 1021.62 | |||
| 2010 | 28.80 | 27.00 | 1016.16 | 25.20 | 31.00 | 1020.29 | |||
| 2012 | 27.80 | 27.80 | 1017.08 | 25.00 | 31.00 | 1020.35 | |||
| 2014 | 26.40 | 27.00 | 1016.92 | 24.00 | 29.80 | 1019.74 | |||
| 2015 | 27.60 | 28.60 | 1017.75 | 22.80 | 31.40 | 1021.29 | |||
| September | 2003 | 25.80 | 29.00 | 1018.60 | 22.80 | 31.00 | 1020.99 | 1018.93 | 1021.53 |
| 2004 | 25.20 | 30.20 | 1019.69 | 24.00 | 31.40 | 1020.95 | |||
| 2005 | 25.20 | 27.00 | 1017.28 | 21.60 | 31.00 | 1021.32 | |||
| 2006 | 24.00 | 29.00 | 1019.14 | 21.60 | 32.20 | 1022.23 | |||
| 2007 | 25.20 | 29.00 | 1018.78 | 22.80 | 31.00 | 1020.99 | |||
| 2008 | 25.20 | 29.00 | 1018.78 | 22.80 | 32.60 | 1022.20 | |||
| 2009 | 22.80 | 31.00 | 1020.99 | 21.60 | 32.60 | 1022.53 | |||
| 2010 | 27.60 | 29.00 | 1018.05 | 24.00 | 32.20 | 1021.55 | |||
| 2012 | 27.60 | 29.00 | 1018.05 | 25.20 | 31.40 | 1020.59 | |||
| 2014 | 22.80 | 29.80 | 1020.08 | 21.60 | 32.60 | 1022.53 | |||
| 2015 | 24.00 | 28.60 | 1018.83 | 22.80 | 31.00 | 1020.99 | |||
| October | 2003 | 20.40 | 31.00 | 1021.64 | 19.80 | 32.20 | 1022.71 | 1020.92 | 1022.30 |
| 2004 | 21.60 | 29.00 | 1019.80 | 21.60 | 32.20 | 1022.23 | |||
| 2005 | 21.60 | 31.00 | 1021.32 | 21.60 | 32.20 | 1022.23 | |||
| 2006 | 21.00 | 29.80 | 1020.57 | 21.00 | 32.20 | 1022.39 | |||
| 2007 | 21.60 | 29.00 | 1019.80 | 21.60 | 32.20 | 1022.23 | |||
| 2008 | 21.60 | 30.20 | 1020.71 | 21.60 | 32.60 | 1022.53 | |||
| 2009 | 21.60 | 31.00 | 1021.32 | 21.60 | 32.20 | 1022.23 | |||
| 2010 | 21.60 | 31.00 | 1021.32 | 21.60 | 33.00 | 1022.84 | |||
| 2012 | 20.40 | 31.40 | 1021.94 | 20.40 | 32.20 | 1022.55 | |||
| 2014 | 21.60 | 29.80 | 1020.41 | 21.60 | 32.60 | 1022.53 | |||
| 2015 | 20.40 | 30.60 | 1021.33 | 20.40 | 31.80 | 1022.25 | |||
| November | 2003 | 18.00 | 31.00 | 1022.24 | 18.00 | 32.60 | 1023.46 | 1021.94 | 1023.10 |
| 2004 | 19.20 | 29.00 | 1020.42 | 19.20 | 31.80 | 1022.56 | |||
| 2005 | 18.00 | 31.00 | 1022.24 | 19.20 | 32.60 | 1023.17 | |||
| 2006 | 18.00 | 31.00 | 1022.24 | 18.00 | 32.20 | 1023.16 | |||
| 2007 | 18.00 | 31.00 | 1022.24 | 18.00 | 32.60 | 1023.46 | |||
| 2008 | 19.20 | 31.40 | 1022.25 | 19.20 | 32.20 | 1022.86 | |||
| 2009 | 19.20 | 30.60 | 1021.64 | 19.20 | 31.80 | 1022.56 | |||
| 2010 | 18.00 | 29.80 | 1021.32 | 18.00 | 31.00 | 1022.24 | |||
| 2012 | 16.80 | 30.60 | 1022.21 | 15.60 | 31.80 | 1023.40 | |||
| 2014 | 18.00 | 31.40 | 1022.55 | 16.80 | 32.60 | 1023.75 | |||
| 2015 | 20.20 | 31.40 | 1021.99 | 18.00 | 32.60 | 1023.46 |
| Month | Year | The Thickness of the Upper Layer (m) | Average Thickness Value of the Upper Layer (m) | Month | Year | The Thickness of the Upper Layer (m) | Average Thickness Value of the Upper Layer (m) |
|---|---|---|---|---|---|---|---|
| May | 2003 | 4.00 | 5.00 | September | 2003 | 3.00 | 3.41 |
| 2004 | 5.00 | 2004 | 5.00 | ||||
| 2005 | 5.00 | 2005 | 3.00 | ||||
| 2006 | 5.00 | 2006 | 3.00 | ||||
| 2007 | 5.00 | 2007 | 3.00 | ||||
| 2008 | 5.00 | 2008 | 3.00 | ||||
| 2009 | 5.00 | 2009 | 2.00 | ||||
| 2010 | 5.00 | 2010 | 3.00 | ||||
| 2012 | 5.00 | 2012 | 3.00 | ||||
| 2014 | 6.00 | 2014 | 5.00 | ||||
| 2015 | 5.00 | 2015 | 4.50 | ||||
| June | 2003 | 5.00 | 4.50 | October | 2003 | 4.00 | 3.95 |
| 2004 | 4.50 | 2004 | 4.00 | ||||
| 2005 | 5.00 | 2005 | 5.00 | ||||
| 2006 | 3.00 | 2006 | 3.00 | ||||
| 2007 | 5.50 | 2007 | 3.00 | ||||
| 2008 | 4.00 | 2008 | 3.00 | ||||
| 2009 | 5.00 | 2009 | 4.00 | ||||
| 2010 | 5.00 | 2010 | 3.00 | ||||
| 2012 | 4.00 | 2012 | 5.00 | ||||
| 2014 | 4.00 | 2014 | 4.50 | ||||
| 2015 | 4.50 | 2015 | 5.00 | ||||
| July | 2003 | 3.00 | 4.09 | November | 2003 | 5.00 | 5.05 |
| 2004 | 4.00 | 2004 | 4.00 | ||||
| 2005 | 4.00 | 2005 | 5.00 | ||||
| 2006 | 3.00 | 2006 | 5.00 | ||||
| 2007 | 3.00 | 2007 | 5.00 | ||||
| 2008 | 4.50 | 2008 | 5.00 | ||||
| 2009 | 5.00 | 2009 | 5.00 | ||||
| 2010 | 5.50 | 2010 | 5.00 | ||||
| 2012 | 5.00 | 2012 | 5.50 | ||||
| 2014 | 4.00 | 2014 | 5.50 | ||||
| 2015 | 4.00 | 2015 | 5.50 | ||||
| August | 2003 | 4.00 | 4.05 | ||||
| 2004 | 5.00 | ||||||
| 2005 | 3.50 | ||||||
| 2006 | 3.00 | ||||||
| 2007 | 5.00 | ||||||
| 2008 | 4.00 | ||||||
| 2009 | 3.00 | ||||||
| 2010 | 5.00 | ||||||
| 2012 | 4.00 | ||||||
| 2014 | 4.00 | ||||||
| 2015 | 4.00 |
| Blue Tide on the Northeast Shore of Tokyo Bay | Northeasterly Wind | Stratification Condition | The Analytical Solution | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Occurrence Date | Occurrence Area | Average Wind Speed (m/s) | Surface Temperature//Surface Salinity (°C)//(psu) | Bottom Temperature//Bottom Salinity (°C)//(psu) | The Upper Layer Density (kg/m3) | The Bottom Layer Density (kg/m3) | The Thickness of the Upper Layer (m) | ||
| 2005 | 16–17 May | Urayasu~Ichigawa~Funabashi | 2.99 | 15.6//32.2 | 15.6//33.4 | 1023.71 | 1024.63 | 5.00 | w ≥ 1.76 m/s |
| 2007 | 1–2 October | Funabashi | 3.76 | 21.6//29 | 21.6//32.8 | 1019.80 | 1022.69 | 5.00 | w ≥ 3.12 m/s |
| 2008 | 13–14 November | Funabashi~Ichigawa | 3.38 | 18//32.2 | 19.2//33.00 | 1023.16 | 1023.47 | 5.00 | w ≥ 1.02 m/s |
| 2011 | 31 May–2 June | Funabashi | 3.30 | - | - | 1021.12 | 1023.06 | 5.00 | w ≥ 2.56 m/s |
| 2011 | 21–24 October | Funabashi~Makuhari | 3.76 | - | - | 1020.92 | 1022.30 | 3.95 | w ≥ 1.70 m/s |
| 2012 | 23–25 May | Makuhari | 4.37 | 19.2//28.2 | 16.8//31.8 | 1019.81 | 1023.13 | 5.00 | w ≥ 3.35 m/s |
| 2013 | 24–27 September | Makuhari~Funabashi | 4.73 | - | - | 1018.93 | 1021.53 | 3.41 | w ≥ 2.02 m/s |
| 2014 | 27 August–3 September | Makuhari~Funabashi | - | 26.4//27 | 22.8//31.8 | 1016.92 | 1021.59 | 4.00 | w ≥ 3.18 m/s |
| 2016 | 14–15 June | Funabashi | 3.72 | - | - | 1019.20 | 1022.75 | 4.50 | w ≥ 3.11 m/s |
| Blue Tide on the Northeast Shore During 1978–2002 | Northeasterly Wind | Stratification Condition Using Representative Values | The Analytical Solution | ||||
|---|---|---|---|---|---|---|---|
| Occurrence Date | Occurrence Area | Average Wind Speed (m/s) | The Upper Layer Density (kg/m3) | The Bottom Layer Density (kg/m3) | The Thickness of the Upper Layer (m) | ||
| 1978 | 31 May | Urayasu~Funabashi | 3.73 | 1021.12 | 1023.06 | 5.00 | w ≥ 2.56 m/s |
| 1978 | 13 June | Funabashi | 3.36 | 1019.20 | 1022.75 | 4.50 | w ≥ 3.11 m/s |
| 1978 | 16–17 July | Makuhari | 4.30 | 1017.67 | 1021.96 | 4.09 | w ≥ 3.11 m/s |
| 1978 | 14–16 August | Makuhari | 4.25 | 1016.91 | 1020.91 | 4.05 | w ≥ 2.98 m/s |
| 1980 | 2–5 August | Funabashi~Makuhari | 5.80 | 1016.91 | 1020.91 | 4.05 | w ≥ 2.98 m/s |
| 1980 | 19 September | Funabashi | 3.96 | 1018.93 | 1021.53 | 3.41 | w ≥ 2.02 m/s |
| 1982 | 21–23 May | Ichigawa | 4.55 | 1021.12 | 1023.06 | 5.00 | w ≥ 2.56 m/s |
| 1982 | 27–29 July | Ichigawa~Makuhari | 3.50 | 1017.67 | 1021.96 | 4.09 | w ≥ 3.11 m/s |
| 1982 | 6 September | Funabashi | 5.03 | 1018.93 | 1021.53 | 3.41 | w ≥ 2.02 m/s |
| 1984 | 24–26 August | Funabashi~Makuhari | 3.82 | 1016.91 | 1020.91 | 4.05 | w ≥ 2.98 m/s |
| 1984 | 9 September | Funabashi | 3.12 | 1018.93 | 1021.53 | 3.41 | w ≥ 2.02 m/s |
| 1985 | 15–18 June | Funabashi | 6.07 | 1019.20 | 1022.75 | 4.50 | w ≥ 3.11 m/s |
| 1986 | 4 August | Urayasu~Ichigawa | 3.94 | 1016.91 | 1020.91 | 4.05 | w ≥ 2.98 m/s |
| 1988 | 24 May | Funabashi | 4.94 | 1021.12 | 1023.06 | 5.00 | w ≥ 2.56 m/s |
| 1988 | 3–8 September | Funabashi | 3.75 | 1018.93 | 1021.53 | 3.41 | w ≥ 2.02 m/s |
| 1989 | 20 June | Funabashi | 4.28 | 1019.20 | 1022.75 | 4.50 | w ≥ 3.11 m/s |
| 1989 | 26–27 August | Funabashi | 4.50 | 1016.91 | 1020.91 | 4.05 | w ≥ 2.98 m/s |
| 1989 | 22–24 September | Funabashi~Makuhari | 3.92 | 1018.93 | 1021.53 | 3.41 | w ≥ 2.02 m/s |
| 1989 | 30 October | Funabashi~Makuhari | 3.35 | 1020.92 | 1022.30 | 3.95 | w ≥ 1.70 m/s |
| 1990 | 28 June–2 July | Funabashi~Makuhari | 4.18 | 1019.20 | 1022.75 | 4.50 | w ≥ 3.11 m/s |
| 1990 | 6 August | Ichigawa~Funabashi~Makuhari | 5.89 | 1016.91 | 1020.91 | 4.05 | w ≥ 2.98 m/s |
| 1990 | 7–8 September | Urayasu~Funabashi | 4.72 | 1018.93 | 1021.53 | 3.41 | w ≥ 2.02 m/s |
| 1990 | 27–29 September | Funabashi~Makuhari | 3.99 | 1018.93 | 1021.53 | 3.41 | w ≥ 2.02 m/s |
| 1992 | 1 June | Funabashi~Makuhari | 2.80 | 1019.20 | 1022.75 | 4.50 | w ≥ 3.11 m/s |
| 1992 | 2 July | Funabashi | 4.18 | 1017.67 | 1021.96 | 4.09 | w ≥ 3.11 m/s |
| 1992 | 3–6 August | Ichigawa~Funabashi | 5.12 | 1016.91 | 1020.91 | 4.05 | w ≥ 2.98 m/s |
| 1994 | 4–8 November | Makuhari~Funabashi | 3.45 | 1021.94 | 1023.10 | 5.05 | w ≥ 1.99 m/s |
| 1996 | 10–12 September | Funabashi~Makuhari | 3.71 | 1018.93 | 1021.53 | 3.41 | w ≥ 2.02 m/s |
| 1999 | 30 September | Funabashi | 2.97 | 1018.93 | 1021.53 | 3.41 | w ≥ 2.02 m/s |
| 1999 | 18–20 October | Funabashi | 3.46 | 1020.92 | 1022.30 | 3.95 | w ≥1.70 m/s |
| 2000 | 27–29 September | Funabashi | 2.85 | 1018.93 | 1021.53 | 3.41 | w ≥ 2.02 m/s |
| 2001 | 23–24 April | Funabashi | 4.39 | - | - | - | - |
| 2001 | 9–10 October | Makuhari and Funabashi and Ichigawa | 3.99 | 1021.94 | 1023.10 | 3.95 | w ≥ 1.56 m/s |
| Real Case without Blue Tide | Stratification Condition | The Analytical Solution | ||||||
|---|---|---|---|---|---|---|---|---|
| Date | Northeasterly Wind | |||||||
| Average Wind Speed (m/s) | Surface Temperature//Surface Salinity (°C)//(psu) | Bottom Temperature//Bottom Salinity (°C)//(psu) | The Upper Layer Density (kg/m3) | The Bottom Layer Density (kg/m3) | The Thickness of the Upper Layer (m) | |||
| 2003 | May | 4.00 | 19.2//29.8 | 16.8//32.2 | 1021.03 | 1023.44 | 7.00 | w ≥ 4.03 m/s |
| June | 3.16 | 24//29.4 | 20.4//32.6 | 1019.44 | 1022.86 | 5.00 | w ≥ 3.43 m/s | |
| July | 2.33,3.33,3,3.1 | 22.8//29.4,22.8//29.4,22.8//27.8,22.8//29.4 | 21.6//31.8,20//33,20//32.6,20//33 | 1019.78,1019.78,1018.57,1019.78 | 1021.93,1023.27,1022.96,1023.27 | 5,5,4.5,4.5 | w ≥ 2.72,3.46,3.49,3.12 m/s | |
| August | 3.08,3.17,3 | 26.4//27,22.8//27,22.8//27 | 24//31,21.6//32.6,20.4//32.6 | 1016.92,1017.96,1017.96 | 1020.65,1022.53,1022.86 | 5,4,4 | w ≥ 3.58,3.17,3.28 m/s | |
| September | 3,2.5 | 26.4//26.2,20.4//30.6 | 22//31.8,20//32.2 | 1016.32,1021.33 | 1021.82,1022.66 | 4,6 | w ≥ 3.47,2.57 m/s | |
| October | 3.90 | 19.2//31.4 | 19//32.6 | 1022.25 | 1023.22 | 5.50 | w ≥ 2.01 m/s | |
| November | 4.50 | 19.2//31.8 | 19//33.4 | 1022.56 | 1023.83 | 4.00 | w ≥ 1.67 m/s | |
| 2004 | May | 3.27,2.9,2.83 | 16.8//30.6,19.2//31,19//30.2 | 16//32.2,18//32.2,16.8//33 | 1022.21,1021.95,1021.39 | 1023.62,1023.16,1024.06 | 6.5,5.5,5 | w ≥ 2.86,2.32,3.03 m/s |
| June | 3.80 | 21.6//27.8 | 18//32.6 | 1018.89 | 1023.46 | 5.00 | w ≥ 3.96 m/s | |
| July | 3.50 | 25.2//28.2 | 20.4//31.4 | 1018.18 | 1021.94 | 5.00 | w ≥ 3.59 m/s | |
| September | 3.1,2.8,2.7,2.5 | 26//29,26//29,26//30.2,23//31.8 | 25.2//31,25.2//31,24//32.6,22.6//33 | 1018.54,1018.54,1019.45,1021.54 | 1020.29,1020.29,1021.85,1022.56 | 6.5,6,5,5 | w ≥ 3.19,2.94,2.87,1.87 m/s | |
| October | 3,2.8,2.1 | 20.4//29,22.6//28.6,20.4//29 | 21//33,22//31.4,20//31.8 | 1020.12,1019.23,1020.12 | 1023.00,1021.51,1022.35 | 5,5,5 | w ≥ 3.15,2.80,2.77 m/s | |
| November | 2.45 | 19.2//29.8 | 20.4//33 | 1021.03 | 1023.16 | 5.00 | w ≥ 2.71 m/s | |
| 2005 | May | 3.07,3.8,2.63 | 18//30,17//29.8,16.8//31 | 15.6//33,15.6//33.4,15.6//33.4 | 1021.48,1021.56,1022.52 | 1024.33,1024.64,1024.64 | 5,5.5,5 | w ≥ 3.13,3.58,2.70 m/s |
| July | 3.5,3.58 | 24//29,24//27 | 19.2//33,20.4//31 | 1019.14,1017.63 | 1023.47,1021.64 | 5,5 | w ≥ 3.85,3.71 m/s | |
| September | 3.20 | 26//25 | 24//29.8 | 1015.54 | 1019.74 | 5.50 | w ≥ 4.18 m/s | |
| October | 2.4,4.3 | 22//30.6,21.6//30.2 | 20.4//32.6,21//31.8 | 1020.91,1020.71 | 1022.86,1022.09 | 5,5 | w ≥ 2.59,2.61 m/s | |
| 2006 | May | 5.40 | 15.6//31.4 | 14.4//32.2 | 1023.10 | 1023.97 | 7.00 | w ≥ 2.42 m/s |
| June | 3.9,3,2.9 | 18//31.8,19.2//30.6,22.8//28 | 15.6//33.4,16//33,17//33 | 1022.85,1021.64,1018.72 | 1024.64,1024.24,1024.01 | 4,5.5,5 | w ≥ 1.98,3.29,4.26 m/s | |
| July | 2.4,3.1 | 27.6//26.2,25.2//27.8 | 19.2//32.6,18//33 | 1015.95,1017.88 | 1023.17,1023.17 | 5,4.5 | w ≥ 4.97,4.04 m/s | |
| September | 3.33,2.8,2.9,3.3 | 25.2//27.4,25.2//29.8,22.8//29.8,21.6//29 | 20.4//32.8,21.6//32.2,21.6//32.6,20.4//33 | 1017.58,1019.39,1020.08,1019.80 | 1023.01,1022.23,1022.53,1023.16 | 4.5,4.5,5,6 | w ≥ 3.88,2.81,2.90,4.08 m/s | |
| October | 2.62,2.9,4.3 | 22//30.2,21.6//27,21.6//29.8 | 20.4//32.6,20.4//31,20.4//31.8 | 1020.60,1018.29,1020.41 | 1022.86,1021.64,1022.25 | 5,5.5,5 | w ≥ 2.79,3.73,2.52 m/s | |
| November | 2.00 | 15.6//31 | 18//33 | 1022.79 | 1023.77 | 6.50 | w ≥ 2.39 m/s | |
| 2007 | July | 3,3.26,2.8,5.3 | 24//31,22.8//30.2,24//27,25.2//27.4 | 19.2//33,19.2//33,19.2//33,19.2//33 | 1020.65,1020.38,1017.63,1017.58 | 1023.47,1023.47,1023.47,1023.47 | 5,5,5,4.5 | w ≥ 3.11,3.26,4.47,4.04 m/s |
| August | 3.64 | 27.6//27.8 | 21.6//33 | 1017.15 | 1022.84 | 4.50 | w ≥ 3.97 m/s | |
| September | 2.9,3.5 | 25.2//25,25.2//25.8 | 24//31,21.6//30.6 | 1015.77,1016.38 | 1020.65 | 1021.02 | w ≥ 5.32,3.59 m/s | |
| October | 2.53 | 21.6//29.8 | 20.4//33 | 1020.41 | 1023.16 | 4.50 | w ≥ 2.77 m/s | |
| November | 2.75,4 | 18//31,18//30.6 | 19.2//32.2,19.2//32.6 | 1022.24,1021.93 | 1022.86,1023.17 | 6.5,6 | w ≥ 2.76,2.48 m/s | |
| 2008 | May | 3.75,4.8,2.6,2.6,6 | 17//29.8,18//29.8,16.8//31,16.8//31,16.8//31 | 15//33,16//31.4,15//33,15//33,15//33 | 1021.56,1021.32,1022.52,1022.52,1022.52 | 1024.46,1023.01,1024.46,1024.46,1024.46 | 6,5,5,5,5 | w ≥ 3.79,2.41,2.58,2.58,2.58m/s |
| June | 3,3.8,4.2 | 20.4//27,21.6//26.2,21.6//26.2 | 18//31.8,18//32.6,18//33 | 1018.60,1017.68,1017.67 | 1022.85,1023.46,1023.77 | 5,4.5,5 | w ≥ 3.82,4.01,4.57 m/s | |
| July | 2.40 | 27.6//25.8 | 19.2//31.8 | 1015.65 | 1022.56 | 4.00 | w ≥ 3.89 m/s | |
| September | 2.40 | 25.2//28.2 | 24//32.6 | 1018.18 | 1021.85 | 4.00 | w ≥ 2.84 m/s | |
| October | 3.58 | 21.6//32.8 | 21//33 | 1022.69 | 1023.00 | 5.00 | w ≥ 1.03 m/s | |
| 2009 | June | 3.1,2.8 | 21.6//28.6,21.6//29 | 18//32.2,17//33 | 1019.50,1019.80 | 1023.16,1024.01 | 5,4.5 | w ≥ 3.55,3.42 m/s |
| July | 2.3,3.1 | 24//29.8,26.4//27 | 19.2//31.8,20.4//31.4 | 1019.74,1016.92 | 1022.56,1021.94 | 5,4.5 | w ≥ 3.11,3.73 m/s | |
| August | 3.5,2.4 | 26.4//27,26.4//29 | 21.6//32.6,22.8//32.6 | 1016.92,1018.42 | 1022.53,1022.20 | 5,4 | w ≥ 4.39,2.88 m/s | |
| September | 3.7,2.3,2.6,2.1 | 22.8//31.4,23//31.4,22//32.2,22.8//31 | 21.6//32.6,21.6//33,20.4//32.8,21.6//33 | 1021.29,1021.24,1022.12,1020.99 | 1022.53,1022.84,1023.01,1022.84 | 4.5,5,5,5 | w ≥ 1.86,2.35,2.62,2.52 m/s | |
| October | 2.3,3.2 | 22//30.2,22//30.6 | 21.6//32.2,21.6//31.8 | 1020.60,1020.91 | 1022.23,1021.93 | 5,5.5 | w ≥ 2.37,2.06 m/s | |
| 2010 | May | 2.35,2.83 | 19.2//29.4,19.2//29.8 | 18//31,16.8//32.2 | 1020.73,1021.03 | 1022.24,1023.44 | 5.5,5 | w ≥ 2.51,2.88 m/s |
| June | 3.33,2.4 | 19.2//29.8,21.6//29 | 16.8//33,16.8//33 | 1021.03,1019.80 | 1024.06,1024.06 | 5.5,5 | w ≥ 3.55,3.82 m/s | |
| August | 3.08 | 27.6//28.6 | 23//32.6 | 1017.75 | 1022.14 | 4.00 | w ≥ 3.10 m/s | |
| October | 2.1,2,2.5,3.2 | 21.6//31,21.6//31,22.8//30.8,21.6//31.4 | 21//32.6,21//32.6,21//33,21//33 | 1021.32,1021.32,1020.84,1021.62 | 1022.70,1022.70,1023.00,1023.00 | 5,5,5,4.5 | w ≥ 2.18,2.18,2.73,1.96 m/s | |
| 2011 | May | 3.17,2.55,2.6 | - | - | 1021.12 | 1023.06 | 5.00 | w ≥ 2.58 m/s |
| June | 2.7,2.8,3,1.7 | - | - | 1019.20 | 1022.75 | 4.50 | w ≥ 3.14 m/s | |
| July | 3.00 | - | - | 1017.67 | 1021.96 | 4.09 | w ≥ 3.14 m/s | |
| August | 2.2,3,2.9 | - | - | 1016.91 | 1020.91 | 4.05 | w ≥ 3.00 m/s | |
| October | 2.80 | - | - | 1020.92 | 1022.30 | 3.95 | w ≥ 1.72 m/s | |
| November | 2.00 | - | - | 1021.94 | 1023.10 | 5.05 | w ≥ 2.02 m/s | |
| 2012 | June | 2.73,3.1,3.9 | 19.2//31,21.6//27,21.6//30.2 | 16.8//33.4,20.4//31,19.2//32.2 | 1021.95,1018.29,1020.71 | 1024.36,1021.64,1022.86 | 5,6,5 | w ≥ 2.88,4.07,2.72 m/s |
| July | 3.30 | 26.4//29 | 22.8//32.2 | 1018.42 | 1021.90 | 5.50 | w ≥ 3.80 m/s | |
| August | 3.20 | 27.6//29 | 22.8//32.2 | 1018.05 | 1021.90 | 4.50 | w ≥ 3.27 m/s | |
| September | 2.50 | 26.4//30 | 23//32.6 | 1019.17 | 1022.14 | 4.00 | w ≥ 2.56 m/s | |
| October | 2.33,4,2.37 | 23//31.4,22.8//31,20.4//31 | 22//32.2,21.6//32.6,20//32 | 1021.23,1020.99,1021.64 | 1022.12,1022.53,1022.50 | 6.5,5.5,6.8 | w ≥ 2.28,2.53,2.34 m/s | |
| November | 2.90 | 15.8//31 | 15//33 | 1022.75 | 1024.46 | 6.00 | w ≥ 2.91 m/s | |
| 2013 | May | 2.53,3.9 | - | - | 1021.12 | 1023.06 | 5.00 | w ≥ 2.58 m/s |
| July | 3,3.1 | - | - | 1017.67 | 1021.96 | 4.09 | w ≥ 3.14 m/s | |
| August | 3.00 | - | - | 1016.91 | 1020.91 | 4.05 | w ≥ 3.00 m/s | |
| September | 2.00 | - | - | 1018.93 | 1021.53 | 3.41 | w ≥ 2.04 m/s | |
| October | 3.65,2.2 | - | - | 1020.92 | 1022.30 | 3.95 | w ≥ 2.23 m/s | |
| 2014 | May | 3.20 | 19.2//29.4 | 16.8//33 | 1020.73 | 1024.06 | 5.50 | w ≥ 3.72 m/s |
| June | 2.8,2.7 | 24//27,22.8//28 | 18//33.4,18//33.4 | 1017.63,1018.72 | 1024.08,1024.08 | 4.5,4 | w ≥ 4.23,3.43 m/s | |
| July | 2.9,2.7 | 24//27,25.2//26 | 18//33,19.2//32 | 1017.63,1016.53 | 1023.77,1022.71 | 4.5,4 | w ≥ 4.13,3.68 m/s | |
| August | 3.80 | 26//27 | 24//31 | 1017.04 | 1020.65 | 5.50 | w ≥ 3.87 m/s | |
| September | 1.9,3.1 | 24//29,24//29 | 20//33,22//32 | 1019.13,1019.14 | 1023.27,1021.97 | 5.5,5 | w ≥ 4.15,3.12 m/s | |
| October | 3.50 | 21.6//30 | 21//32 | 1020.56 | 1022.24 | 5.00 | w ≥ 2.40 m/s | |
| 2015 | June | 2.80 | 24//28 | 18//33 | 1018.38 | 1023.77 | 5.00 | w ≥ 4.30 m/s |
| July | 1.75 | 22.8//26 | 19.2//31 | 1017.21 | 1021.95 | 5.00 | w ≥ 4.03 m/s | |
| August | 4.10 | 27.6//26 | 21.6//32 | 1015.80 | 1022.08 | 5.00 | w ≥ 4.64 m/s | |
| September | 3.78,3.3 | 25.2//25,24//27 | 24//29,22.8//32 | 1015.78,1017.63 | 1019.14,1021.75 | 6,5.5 | w ≥ 4.08,4.14 m/s | |
| October | 2.9,3.6,2.1 | 20.4//30.6,20.4//31.4,20.4//31.4 | 19.2//33,19.2//32,19.2//32 | 1021.33,1021.94,1021.94 | 1023.47,1022.71,1022.86 | 6,6,6 | w ≥ 3.26,1.95,2.14 m/s | |
| 2016 | May | 4.1,2.2,2.58 | - | - | 1021.12 | 1023.06 | 5.00 | w ≥ 2.58 m/s |
| June | 3.1,3.1,2.1 | - | - | 1019.20 | 1022.75 | 4.50 | w ≥ 3.14 m/s | |
| July | 3,3.05 | - | - | 1017.67 | 1021.96 | 4.09 | w ≥ 3.14 m/s | |
| August | 3,2.75,2.73 | - | - | 1016.91 | 1020.91 | 4.05 | w ≥ 3.00 m/s | |
| September | 3.8,1.87,1.7,1.67 | - | - | 1018.93 | 1021.53 | 3.41 | w ≥ 2.04 m/s | |
| October | 2,2.06,1.9 | - | - | 1020.92 | 1022.30 | 3.95 | w ≥ 2.23 m/s | |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, Z.; Bai, X.; Dou, J.; Hei, P. Applying a Simple Analytical Solution to Modelling Wind-Driven Coastal Upwelling of Two-Layered Fluid at the Head of Tokyo Bay, Japan. Water 2017, 9, 744. https://doi.org/10.3390/w9100744
Zhu Z, Bai X, Dou J, Hei P. Applying a Simple Analytical Solution to Modelling Wind-Driven Coastal Upwelling of Two-Layered Fluid at the Head of Tokyo Bay, Japan. Water. 2017; 9(10):744. https://doi.org/10.3390/w9100744
Chicago/Turabian StyleZhu, Zhongfan, Xiaomei Bai, Jie Dou, and Pengfei Hei. 2017. "Applying a Simple Analytical Solution to Modelling Wind-Driven Coastal Upwelling of Two-Layered Fluid at the Head of Tokyo Bay, Japan" Water 9, no. 10: 744. https://doi.org/10.3390/w9100744
APA StyleZhu, Z., Bai, X., Dou, J., & Hei, P. (2017). Applying a Simple Analytical Solution to Modelling Wind-Driven Coastal Upwelling of Two-Layered Fluid at the Head of Tokyo Bay, Japan. Water, 9(10), 744. https://doi.org/10.3390/w9100744







