Residential Water Scarcity in Cyprus: Impact of Climate Change and Policy Options

Abstract

This paper presents an assessment of the cost of water scarcity in Cyprus, today and in the next 20 years, taking into account the effect of projected climate change in the region. It focuses on the residential sector, accounting also for tourism and industry. Using a simple demand function, total scarcity costs in Cyprus are computed for the period 2010–2030, and three scenarios of future water demand are presented. The central estimate shows that the present value of total costs due to water shortages will amount to 72 million Euros (at 2009 prices), and, if future water demand increases a little faster, these costs may reach 200 million Euros. Using forecasts of regional climate models, costs are found to be about 20% higher in a “climate change” scenario. Compared to the loss of consumer surplus due to water shortages, desalination is found to be a costly solution, even if environmental damage costs from the operation of desalination plants are not accounted for. Finally, dynamic constrained optimization is employed and shows that efficient residential water prices should include a scarcity price of about 40 Eurocents per cubic meter at 2009 prices; this would constitute a 30–100% increase in current prices faced by residential consumers. Reductions in rainfall due to climate change would raise this price by another 2−3 Eurocents. Such a pricing policy would provide a clear long-term signal to consumers and firms and could substantially contribute to a sustainable use of water resources in the island.

1. Introduction

Cyprus is an island in the Eastern Mediterranean with an area of 9,250 square kilometers and a population of about 800,000, which became a member of the European Union (EU) in 2004; (the information provided here refers only to the area controlled by the government of the Republic of Cyprus. This section is based on [1] and the references contained therein). It has enjoyed sustained economic growth in the last three decades (averaging 5.8% and 3.1% per year over the last 30 and 10 years, respectively) mainly due to tourist income and the development of financial services. Its Gross Domestic Product (GDP) per capita exceeded 20,000 Euros in 2009.

Like other Mediterranean countries, Cyprus has a semi-arid climate associated with limited water resources. The principal cause of water scarcity is the combination of limited availability and excess demand of water among competing uses; this is clearly illustrated by the fact that Cyprus has the highest Water Exploitation Index. This index compares available water resources in a country to the amount of water used. An index above 20% indicates water scarcity (45%) in the EU [2]—which becomes much higher in years of excessive drought. Historically, droughts occur every two-to-three consecutive years as a result of large inter-annual decreases in precipitation. In the last four decades however, drought incidences have increased both in magnitude and frequency. The two main water-consuming sectors in the country are agriculture and households.

Water management has been problematic since the 1960s due to the limited development of water infrastructure for domestic and irrigation supply. The national government’s top priorities were to ensure food security and constant supply of good quality water so that the adverse effects of water scarcity do not impede socioeconomic development, given that agriculture was the backbone of the economy, contributing about 20% of the country’s GDP. Αs Cyprus gradually became service-dominated, the contribution of agriculture has decreased dramatically, and currently accounts for about 2% of GDP, employing 7% of the total workforce [3]. Despite such decreases, agriculture still remains the dominant water user in the country, accounting for 69% of total water use, while the domestic sector accounts for 25%, of which one fifth goes to tourism [4]. In order to store as much freshwater as possible, Cypriot governments have constructed numerous dams on key catchments over the course of the years. As a result, the water storage capacity of the island increased from six million cubic meters (m³) in 1960 to 327 million m³ in 2009, making Cyprus one of the most developed countries in terms of dam infrastructure [5,6].

The Eastern Mediterranean region is expected to be affected adversely by climate change. According to detailed regional climate models, which have been derived from global circulation models downscaled for regional application, maximum and minimum temperatures are projected to increase by about 3 °C in the mid-21st century and by more than 4 °C by the end of the century, with the strongest increases to be observed during summer months. Annual precipitation levels are forecasted to decline by 15%–25% in the same period (see the World Bank Climate Change Portal forecasts: http://sdwebx.worldbank.org/climateportal). Such projections illustrate that climate change will have serious consequences both for the (already scarce) water resources and for the energy needs of the country.

This paper describes an assessment of the social costs caused by water shortages in non-agricultural sectors in Cyprus for the entire period 2010–2030, and a comparison with the economic cost from the deployment of several desalination plants during the same period. It also reports on the results of the first attempt to assess the economic costs of climate change in Cyprus in the medium term (up to the year 2030) in non-agricultural water use. Although major climate changes are expected to happen later in the 21st century, the year 2030 is important because it constitutes the forecast horizon of several national and international studies and also enables more plausible scenarios of future economic development since forecasts into the longer term are fraught with much higher uncertainty. Finally, the efficient scarcity price of non-agricultural water is assessed which, if included in end-user water prices, may lead to sustainable utilization of the water resources of the island. As the island constitutes one single river basin and most regions are interconnected through water pipelines, this is effectively a nationwide assessment of scarcity prices; this makes the assessment presented here quite unique because in most cases reported in the literature scarcity costs and prices are calculated for a specific water basin or region [7,8]. Despite the fact that in principle total scarcity costs, optimal allocation of water resources and efficient water prices must be determined on the basis of a broader approach that includes the agricultural sector as well, this assessment is a first step towards this direction.

2. Background to the Calculation of Water Scarcity Costs

As mentioned in the introductory section, agriculture accounts for more than two thirds of total water consumption in Cyprus. However, when it comes to the consumption of freshwater collected in dams, the residential sector (including commercial and industrial consumers) is almost of equal importance to agriculture. The water stored in major dams is supplied to both residential and agricultural users; in recent years with adequate precipitation levels, agriculture has consumed about 60% of these water quantities, but during years with less rainfall more than 65% went to residential users, and this fraction increased further in years with extensive drought [6]. Apparently, apart from the fact that many irrigated farms can alternatively use groundwater from private boreholes in the absence of sufficient surface water, it is politically more difficult for the government to drastically reduce water supply to households, tourist accommodations and other enterprises.

Section 3 to Section 5 report on an assessment of the social costs of water scarcity in non-agricultural sectors of Cyprus. These sectors include households, tourism, industry and other commercial users. It should be pointed out that these costs—strictly speaking—do not constitute scarcity costs in the sense described in Section 7, i.e., they do not correspond to the opportunity cost caused by the fact that limited water quantities should be consumed each year in order to ensure water availability in the future. In fact, Section 4 and Section 5 calculate the economic losses from water shortages in Cyprus; a ‘genuine’ assessment of the price of scarcity is provided in Section 7.

Three further clarifications are necessary here:

(a)

Water use is addressed in all regions except that of Paphos (on the western part of the island accounting for about 11% of national non-agricultural water consumption) because all other regions are largely served by a common infrastructure, such as the Southern Conveyor Project, which transports water from relatively water-abundant southwestern areas to the rest of the country, and has direct connections between a desalination plant in the south and the capital city of Nicosia in the centre of the island. Hence, although each region possesses different amounts of water reserves, this whole area is treated like one common system.

(b)

The study is restricted to water supply by governmental water works which, according to [6], account for over 85% of total non-agricultural water consumption in this region.

(c)

The analysis is based on the residential sector. In the area under study, this sector (together with minor commercial users) is responsible for approximately 85% of non-agricultural water use; tourism accounts for 12% and industry for 3% [6]. These figures are confirmed in annual reports of the Municipal Water Boards of the two major cities Nicosia and Limassol, see www.wbn.org.cy and www.wbl.com.cy, respectively.

Although the above mentioned restrictions constitute a simplification in the analysis, it is questionable whether a more detailed examination would alter the results and the policy implications reported in this paper. This will be explained in Section 4 below.

When estimating available water quantities for residential users, one has to keep in mind that in recent years several wastewater treatment projects have been initiated, which aim at increasing water availability in agriculture. The supply of treated wastewater may in theory increase freshwater supply to non-agriculture sectors. This option was not considered in the water shortages scenarios used in this paper because (a) it is still unclear to what extent farmers will overcome reservations about the quality of treated water and use it; and (b) the use of wastewater may primarily be exploited in order to reduce groundwater extraction by farmers, which causes serious environmental degradation in underground reservoirs of the island.

The assessment of costs in the following sections includes a calculation of (a) costs of water shortage up to 2030 without climate change and (b) additional costs under the assumption of reduced freshwater supply due to climate change. Furthermore, a dynamic optimization procedure is applied in Section 7 in order to determine the marginal scarcity cost that should be included in residential water prices of Cypriot households.

3. Household Water Demand

To determine willingness to pay for water and the costs associated with reduced water deliveries due to scarcity, a demand function is necessary—at least an estimated price elasticity of water demand. Aggregate time series data do not allow identification of price effects on household water demand as variability of prices has been very limited over the last decades. Disaggregated time series data from individual Water Boards of Cypriot cities are burdensome to collect for the years prior to the late 1990s; since such data would be valuable in order to perform meaningful econometric analyses, there is currently a major data collection effort for this purpose. Until this effort enables the construction of a useful database, it is necessary to rely on data already available. The only empirical estimation currently available for Cyprus comes from a cross-sectional household water demand analysis that was carried out with the aid of Family Expenditure Surveys [9]. According to this, income elasticity was estimated at about 0.3, ranging from 0.22 at low income groups to 0.48 at the top 10 income percentile. Price elasticity was found to be in the range of −0.4 (for the highest income groups) to −0.8 (lowest income group), with a median value of −0.6.

Schleich and Hillenbrand [10] provide a comprehensive review of empirical findings from residential water demand studies in European countries. Although estimates of income and price elasticities vary greatly among studies and countries, the values for Cyprus estimated in [9] are at the higher end of the range of elasticities found in other countries. A price elasticity of −0.6 is quite high (in absolute terms) compared to most other European studies, and since per capita water use in Cyprus is comparable to that of other developed European countries it would be reasonable to expect similar elasticity levels. Therefore, the reference calculations in the rest of this paper have been conducted with a price elasticity of −0.3, which is approximately the average value from other European studies. Sensitivity analyses with lower and higher elasticity values (−0.15 and −0.6 respectively) are available elsewhere [12] and are briefly discussed in Section 4, Section 6 and Section 7. Water consumption in Germany was 128 liters per person per day in 2003 according to [10], and information from the Municipal Water Boards of Cyprus shows a daily per capita consumption of 120–145 liters in Cypriot cities throughout the 2000s. See also the review of OECD water consumption data in [11].

4. Scarcity Costs without Climate Change

As mentioned above, water scarcity is inherent in Cyprus and is expected to deteriorate under climate change conditions. It is therefore necessary, as a first step, to assess scarcity costs without climate change. These costs for the residential sector will be equal to the loss of consumer surplus due to reduced availability of water, i.e., the loss of economic benefits of consumers minus the expenditures that consumers avoid by not purchasing these quantities. 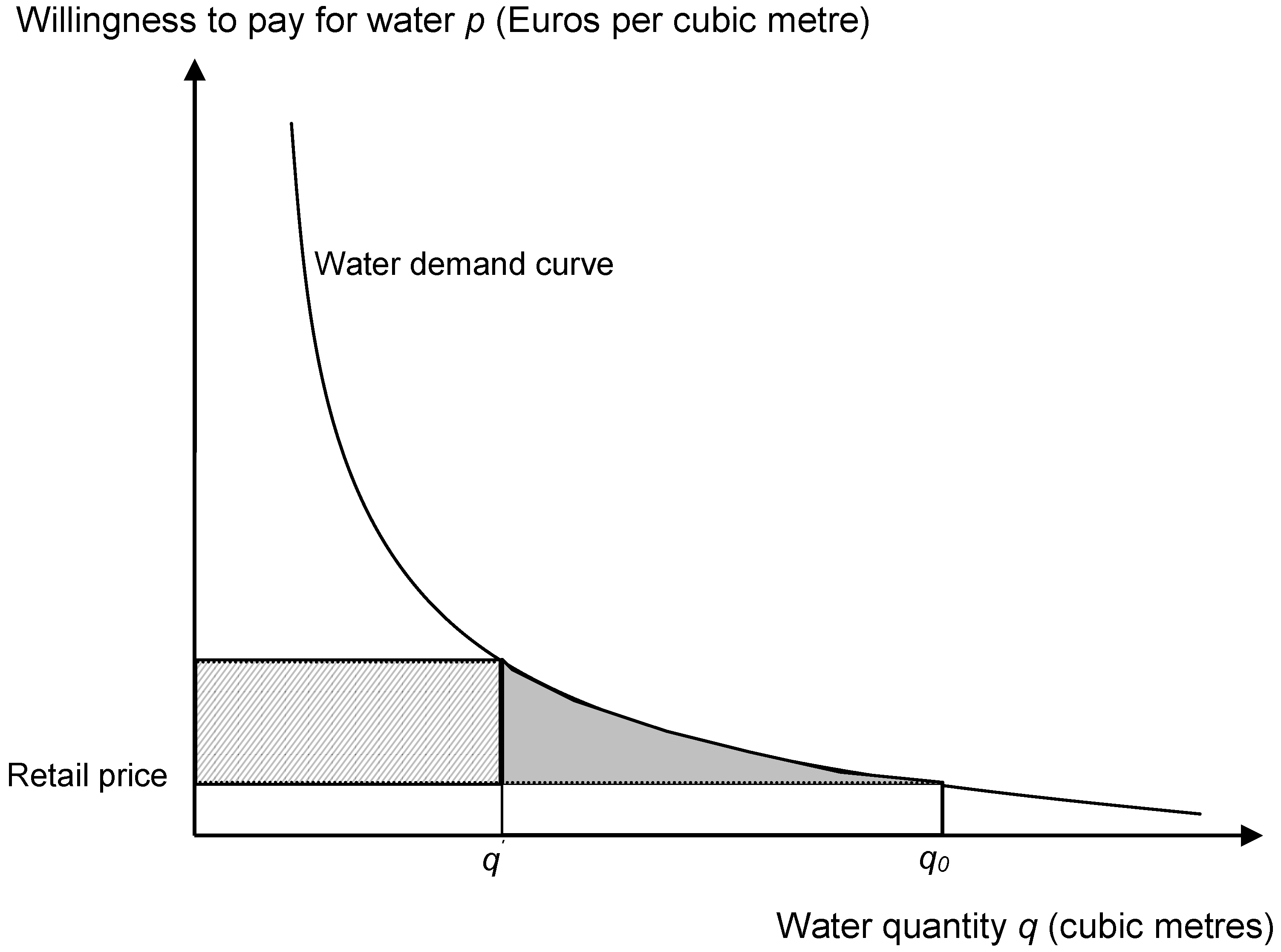Figure 1 illustrates graphically this cost, which is equal to the area between the demand curve and the price line. Hereby it is assumed that there is no producer surplus so that supply cost is equal to consumer price. This seems to be a reasonable assumption for modest changes in quantities (i.e., modest movements along the demand curve) for two reasons. Firstly, it is appropriate to consider marginal supply costs to remain constant because a) the governmental authority (the Water Development Department) currently sells water to resellers (local Water Boards and municipalities) at a fixed supply cost that does not change if resellers obtain additional quantities; b) the government has agreed with desalination plants to obtain additional water quantities, if needed, at a price that corresponds to the operating and energy costs of the desalination plants, which are very close to the marginal water supply costs of the existing quantities. Secondly, it is appropriate to assume a near-zero producer surplus because both the Water Development Department and water resellers are public nonprofit organizations so that retail water prices correspond approximately to their total costs. The lower the price elasticity of water demand (in absolute terms), the steeper the demand curve, which leads to higher economic losses.

Similar to studies such as [13], the following inverse demand function is formulated:

P_t = exp {[ln (q_t) / η] + c_t}

(1)

where P is marginal willingness to pay in Eurocents per cubic meter, q is annual water demand in thousand m³, η is the price elasticity assumed to remain constant over time, and c is a constant. Using 2006 as the base year because it is the most recent year for which all necessary data are available and which exhibited moderate rainfall patterns, the constant for that year c₂₀₀₆ can be calculated, so that the demand function for the base year is fully defined. For this purpose one needs to know q₂₀₀₆ and P₂₀₀₆, i.e., the water quantities consumed and the marginal water price in year 2006.

Figure 1. Illustration of economic losses associated with water shortage. q₀ = the quantity that would be consumed in the case of no shortages; q’ = the quantity actually consumed. The shaded area corresponds to the loss of consumer surplus due to reduced water availability. The rectangle with the diagonal pattern to the left of the shaded area corresponds to the scarcity rent to be paid by consumers due to water shortages; however, since water is supplied by governmental authorities, this scarcity rent remains at state ownership and hence is not considered a social cost.

Figure 1. Illustration of economic losses associated with water shortage. q₀ = the quantity that would be consumed in the case of no shortages; q’ = the quantity actually consumed. The shaded area corresponds to the loss of consumer surplus due to reduced water availability. The rectangle with the diagonal pattern to the left of the shaded area corresponds to the scarcity rent to be paid by consumers due to water shortages; however, since water is supplied by governmental authorities, this scarcity rent remains at state ownership and hence is not considered a social cost.

To determine q₂₀₀₆, one has to add up water sales by the three Municipal Water Boards of Cyprus, supplying the largest part of households and enterprises in the three major cities of Nicosia, Limassol and Larnaca. According to official data [6], during the period 2005–2007 60.9% of water to households, industry and tourism, was supplied by the Municipal Water Boards, while the rest was supplied directly by municipalities and village communities to end users. Hence the quantities of residential water were increased accordingly in order to account for effectively all non-agricultural water use in the country. For this procedure two reasonable assumptions have been made implicitly: First, that price elasticity of water use is the same in all regions of Cyprus; and second, that country-wide water distribution losses are similar to those recorded by the three Water Boards (which amounted to 21% of the total water quantities they supplied in year 2006). A third implicit assumption is that price elasticity of demand is similar across all non-agricultural sectors (households, industries and tourist enterprises). Although it might be justifiable to criticize this assumption, noting also the possibility that water demand may be very inelastic in hotels and industry, one has to keep in mind that (a) households, as outlined above, consume the vast majority of these water quantities; and (b) a household price elasticity of −0.3 is already quite low, so that it would be surprising for non-residential users to exhibit much lower long-term elasticities. Long-term versus short-term elasticities are discussed in the last paragraph of this section. Note that water demand bears similarities with demand for automotive fuels: There is a general belief that demand for both water and fuels is inelastic to prices. However, this applies primarily to short-term elasticities, which may be in the order of −0.05 to −0.2; in the long run, elasticities in the order of −0.3 (for water) to −0.8 (for fuels) have been observed, which implies that if high prices persist over a long period then demand will exhibit a non-negligible adjustment to these price levels. See also [14].

To determine P₂₀₀₆, the sales-weighted average price of water sold from the Municipal Water Boards to end users has to be calculated. All three Water Boards implement block pricing (albeit each one at different tariff levels and for different consumption blocks), therefore it can be assumed that this price remains constant at the margin for moderate variations in the quantities consumed. It was assumed that this price reflects the average of all non-agricultural water users, i.e. including those supplied directly by municipalities and communities.

As regards future demand up to the year 2030, it is expected that the demand function will shift outwards as a result of increasing population and income. Based on official statistics of water sales from the Municipal Water Boards, per capita water use has increased by more than 2% annually over the last 15 years—excluding those years with water restrictions on households—and by about 1.5% annually during the period 2000–2008 [15]. Therefore, three scenarios were constructed on the future evolution of residential water demand, assuming annual growth rates of per capita water use of zero, 1% and 2% respectively, and were combined with official demographic statistics to arrive at forecasts of residential water use. Official GDP growth forecasts, provided by the Cypriot Ministry of Finance, were also used together with the income elasticities of [9] mentioned above: Using the average income elasticity of 0.3, yields similar water use forecasts with the zero per capita growth scenario; and using the upper-end income elasticity of 0.48 leads to similar forecasts with the 1% per capita growth scenario. An earlier study by WDD and the United Nations Food and Agriculture Organization [4] assumed a 2% annual growth rate of domestic water demand per capita. It was therefore deemed appropriate to retain all three per capita water demand scenarios mentioned above.

The fraction of water supplied by the three Municipal Water Boards of the country was kept constant for the period 2005−2007 (60.9%). It was further assumed that the water price will remain constant in real terms up to 2030—which is a reasonable assumption under ‘business as usual’ conditions. As a result of these assumptions, it is possible to obtain one demand function for each year from 2010 until 2030, i.e., to calculate c_t of equation (1) for each year t. In response to provisions of the EU Water Framework Directive, which will be further mentioned in Section 7, in early 2010, governmental authorities reassessed end-user water prices so as to achieve ‘full cost recovery’ as required by the Directive; this would entail a calculation of resource and environmental costs—as defined by the authorities—to be included in water prices. The analysis shown here does not take into account eventual future changes in water prices as a result of that governmental re-assessment because ‘resource costs’ in the governmental study are defined differently from our scarcity costs, so that it would be inconsistent to mix the two studies.

Although demand is expected to rise in the future, available water quantities will remain more or less constant (if one ignores both the periodic variability of rainfall patterns and the effects of climate change, and assuming that no further desalination plants will be installed). It is therefore possible to compute the decrease in economic benefits because of reduced water availability for each future year, by integrating the annual inverse demand Equation (1) from the given ‘normal’ level of water consumption in the base year q₂₀₀₆ to the consumption level of each future year q_t, which corresponds to the maximum demanded water quantity for that year. Economic losses, expressed in Euros per year, are thus given by Equation (2):

$${LOSS}_t=0.01·\frac{\exp (c_t)}{1/\eta +1}·(q_t^{\frac{1}{\eta }+1} - q_{2006}^{\frac{1}{\eta }+1})$$

(2)

For each one of the three water demand scenarios used, the results are shown in Table 1. The present value of costs is projected to range between 15 and 200 million Euros (at constant prices of the year 2009; called Euros’ 2009 here); according to the probably more realistic scenario 2, costs will approach 72 million Euros’ 2009.

Evidently this result depends to a large extent, apart from future water demand, on the value of the price elasticity used in the analysis. Because no dramatic changes in national income, prices or water consumption are assumed to happen during the forecast period, the initial assumption of constant price elasticity seems to be justified. However, if this elasticity is markedly higher (in absolute terms) than the value of −0.3 used here, scarcity costs will be significantly lower: consumers will have less willingness to pay in order to retain their water consumption levels. The opposite is the case if the elasticity is lower in absolute terms—scarcity costs are much higher. To illustrate this, costs according to water consumption scenario 2 were re-calculated, assuming price elasticities of −0.15 and −0.6; results are available in [12]. In the first case, costs reach 207 million Euros’ 2009 (almost three times higher than with an elasticity of −0.3), whereas in the second case costs decline to 31 million Euros’ 2009 (57% lower). According to empirical studies from other European countries mentioned in Section 3, elasticities greater than 0.6 in absolute terms should not be considered as plausible. Taking also into account that future water demand may increase faster than 1% per capita at least in the near future, in line with trends of the last decade, the cost of 72 million Euros’ 2009 has to be considered as a low-end ‘business as usual’ figure; judging from the difference between scenarios 2 and 3, costs can easily approach 150 million Euros.

A second comment on these results is that they reflect the long-term costs of water shortages because the price elasticity used is considered to be a long-term elasticity, in line with results from other European countries. It is well known that demand for many goods and services is much less elastic in the short term. For example, a household facing the price of water doubling cannot immediately make significant changes to its everyday preferences—it may only try to partly conserve water in order to mitigate the high increase in water expenditures. Over a period of some years, however, if high water prices persist, the household can adjust its daily routine by installing appliances which consume less water and adopting less water-intensive habits. This is also valid for aggregate water consumption: Facing a severe water shortage in 2008, governmental authorities reduced residential water supply and imported water quantities from abroad at comparatively very high prices, while at the same time re-scheduling their medium-term investment decisions in order to provide more desalinated water in a few years’ time. The short-term solution of water imports was very costly, reflecting the very low short-term elasticity of both water demand and supply. These remarks do not imply that the costs calculated here are necessarily lower than real-world costs, but they try to explain why short-term costs observed in Cyprus in recent years may have been considerably higher. This is another reason to emphasize the need for a long-term water policy which can tackle water scarcity in Cyprus in an economically efficient manner.

Table 1. Annual costs of residential water shortages in Cyprus.

Year	Scenario 1: Constant per capita water use		Scenario 2: Per capita water use grows 1% p.a.		Scenario 3: Per capita water use grows 2% p.a.
	Water demand	Cost	Water demand	Cost	Water demand	Cost
	(m m3)	(m €’2009)	(m m3)	(m €’2009)	(m m3)	(m €’2009)
2010	54.8	0.21	54.8	0.21	54.8	0.21
2011	55.4	0.33	56.0	0.46	56.5	0.60
2012	55.8	0.42	57.0	0.72	58.1	1.11
2013	56.2	0.52	57.9	1.05	59.7	1.81
2014	56.6	0.63	58.9	1.46	61.3	2.71
2015	57.0	0.75	60.0	1.95	63.0	3.84
2016	57.5	0.88	61.0	2.51	64.7	5.22
2017	57.7	0.97	61.9	3.07	66.3	6.71
2018	58.0	1.07	62.8	3.69	67.9	8.46
2019	58.2	1.17	63.7	4.38	69.6	10.48
2020	58.5	1.27	64.6	5.15	71.3	12.81
2021	58.8	1.39	65.6	6.00	73.1	15.47
2022	59.0	1.47	66.4	6.85	74.8	18.32
2023	59.1	1.55	67.3	7.77	76.5	21.52
2024	59.3	1.64	68.2	8.78	78.3	25.11
2025	59.5	1.73	69.1	9.86	80.1	29.12
2026	59.7	1.82	70.0	11.02	82.0	33.59
2027	59.8	1.87	70.8	12.12	83.7	38.16
2028	59.9	1.91	71.6	13.28	85.5	43.20
2029	60.0	1.96	72.5	14.52	87.4	48.73
2030	60.1	2.01	73.3	15.84	89.3	54.80
Total economic loss, 2010–2030		25.57		130.69		381.97
Present value of economic loss, 2010–2030		15.20		71.96		204.21

Notes:

-

The above water quantities refer to water actually consumed by households, i.e., do not include water distribution losses.

-

Present value of costs has been computed with a social discount rate of 4%.

-

m = million.

Table 1. Annual costs of residential water shortages in Cyprus.

Year	Scenario 1: Constant per capita water use		Scenario 2: Per capita water use grows 1% p.a.		Scenario 3: Per capita water use grows 2% p.a.
	Water demand	Cost	Water demand	Cost	Water demand	Cost
	(m m3)	(m €’2009)	(m m3)	(m €’2009)	(m m3)	(m €’2009)
2010	54.8	0.21	54.8	0.21	54.8	0.21
2011	55.4	0.33	56.0	0.46	56.5	0.60
2012	55.8	0.42	57.0	0.72	58.1	1.11
2013	56.2	0.52	57.9	1.05	59.7	1.81
2014	56.6	0.63	58.9	1.46	61.3	2.71
2015	57.0	0.75	60.0	1.95	63.0	3.84
2016	57.5	0.88	61.0	2.51	64.7	5.22
2017	57.7	0.97	61.9	3.07	66.3	6.71
2018	58.0	1.07	62.8	3.69	67.9	8.46
2019	58.2	1.17	63.7	4.38	69.6	10.48
2020	58.5	1.27	64.6	5.15	71.3	12.81
2021	58.8	1.39	65.6	6.00	73.1	15.47
2022	59.0	1.47	66.4	6.85	74.8	18.32
2023	59.1	1.55	67.3	7.77	76.5	21.52
2024	59.3	1.64	68.2	8.78	78.3	25.11
2025	59.5	1.73	69.1	9.86	80.1	29.12
2026	59.7	1.82	70.0	11.02	82.0	33.59
2027	59.8	1.87	70.8	12.12	83.7	38.16
2028	59.9	1.91	71.6	13.28	85.5	43.20
2029	60.0	1.96	72.5	14.52	87.4	48.73
2030	60.1	2.01	73.3	15.84	89.3	54.80
Total economic loss, 2010–2030		25.57		130.69		381.97
Present value of economic loss, 2010–2030		15.20		71.96		204.21

Notes:

-

The above water quantities refer to water actually consumed by households, i.e., do not include water distribution losses.

-

Present value of costs has been computed with a social discount rate of 4%.

-

m = million.

5. Additional Scarcity Costs due to Climate Change

Households in Cyprus are supplied with water from both dams and desalination plants. Obviously, the amount of water stored in dams (and subsequently available for residential, agricultural and other uses) depends on precipitation levels. Future precipitation levels can be forecast through climate simulations. Since global climate models cannot simulate the climate of small regions with sufficient accuracy, regional models are used, which represent further refinements of global models at a regional scale.

To assess water scarcity costs under climate change conditions, results of regional climate simulations were used, some of which are widely available and some were provided to the author by climate researchers. Information on climate modeling results in this paper is based on regional model forecasts available at the World Bank Climate Change Portal (http://sdwebx.worldbank.org/climateportal) as well as on work currently carried out at the Cyprus Institute (http://www.cyi.ac.cy). Results of the latter are still tentative. According to these forecasts, precipitation levels are expected to decline by approximately 10% in the period 2021–2040 compared to the most recent twenty year period 1987–2006. In line with suggestions from the climate modelers, this 10% reduction was considered to apply for the year 2030 (the middle of the period 2021–2040), and it was assumed that precipitation will decrease linearly between 2010 and 2030; although the latter assumption is not realistic because rainfall levels may fluctuate considerably from year to year, it was considered appropriate because of the uncertainties of climate models and the short period examined in this analysis. Note that these models project a further decrease in precipitation levels towards the end of the 21st century. It is important to underline that the relationship between precipitation and water availability is not straightforward; depending on changes in the intensity and the duration of rainfall that may be caused by climate change, a 10% reduction in rainfall levels may lead to a higher or lower reduction in the amount of available surface water. In the absence of more detailed information, this 10% precipitation decrease was considered to cause an equal reduction in water stocks; such an assumption seems to be a reasonable starting point.

As mentioned earlier in Section 2, in years with serious water shortage, authorities prefer to continue supplying the residential and tourist sector as smoothly as possible, and reduce the quantities of water supplied to farmers. In this study, however, it was assumed that reduced freshwater availability would hurt farmers and households alike, thereby allocating climate change costs to each sector according to the stress that each sector puts to the island’s water resources today. ‘Today’ in this case refers to the years 2005–2006, when the available freshwater quantities were not abundant but were not under stress either.

Evidently the projected reduction in precipitation levels is moderate, and becomes even less important for non-agricultural water use in Cyprus since about 60% of the quantities supplied to these sectors comes from desalination plants that provide water irrespective of climate conditions. Therefore, additional water scarcity costs associated with climate change up to 2030 turn out to be quite modest; presented in Table 2. The present value of additional costs for the entire period 2010–2030 ranges between 6 and 31 million Euros at 2009 prices, 15−40% more than costs due to the already existing water scarcity in the country; the central estimate (scenario 2) shows a 22% cost increase—or 15.7 million Euros.

Table 2. Annual costs of residential water shortages in Cyprus under climate change assumptions.

		Additional scarcity cost due to climate change (m Euros’2009)
Year	Difference in water availability due to climate change	Scenario 1: Constant per capita water use	Scenario 2: Per capita water use grows 1% p.a.	Scenario 3: Per capita water use grows 2% p.a.
2010	0.0%	0.00	0.00	0.00
2015	−0.9%	0.17	0.28	0.41
2020	−1.9%	0.46	1.00	1.74
2025	−2.8%	0.85	2.27	4.58
2030	−3.7%	1.28	4.17	9.72
Total additional economic loss, 2010–2030		11.11	29.42	60.19
Present value of economic loss, 2010–2030		6.12	15.69	31.49

Table 2. Annual costs of residential water shortages in Cyprus under climate change assumptions.

		Additional scarcity cost due to climate change (m Euros’2009)
Year	Difference in water availability due to climate change	Scenario 1: Constant per capita water use	Scenario 2: Per capita water use grows 1% p.a.	Scenario 3: Per capita water use grows 2% p.a.
2010	0.0%	0.00	0.00	0.00
2015	−0.9%	0.17	0.28	0.41
2020	−1.9%	0.46	1.00	1.74
2025	−2.8%	0.85	2.27	4.58
2030	−3.7%	1.28	4.17	9.72
Total additional economic loss, 2010–2030		11.11	29.42	60.19
Present value of economic loss, 2010–2030		6.12	15.69	31.49

6. Comparison of Scarcity Costs with Desalination Costs

In response to increasing water demand and stagnating or decreasing supply of freshwater, and like other governments in water scarce regions of the world such as Middle East and Australia, Cypriot authorities have promoted the operation of desalination plants. By 2008 two plants were in operation, with a nominal water production capacity of 92,000 m³ per day; the capacity of both plants has recently been expanded by about 30%. Moreover, two temporary desalination units started operating in 2009, and significant new investments are under way. Table 3 shows the desalination units currently planned. As these investments are based on the BOOT (Build, Own, Operate and Transfer) system, private investors operating each plant incur investment and operating costs and sell water to the authorities (the Cyprus Water Development Department) at an agreed price so as to cover their costs; Table 3 also displays these prices.

Table 3. Desalination plants in operation or scheduled to operate in Cyprus (except in the area of Paphos).

Location	Start year	Capacity (m3/day)	Price of water sold to WDD (Euros/m3)
Dekelia	1997	40,000	0.6424
Larnaca	2001	52,000	0.6817
Moni (mobile)	2009	20,000	1.3870
Garyllis aquifer	2009	9,000	0.2992
Dekelia (expansion)	2009	20,000	0.7800
Larnaca (expansion)	2009	10,000	1.3200
Limassol	2012	40,000	0.8725
Vasilikos	2012	60,000	0.8130
Limassol (expansion)	2015	20,000	0.8725

Source: Cyprus Water Development Department (website www.moa.gov.cy/wdd and official announcements, February 2010).Note: The mobile plant of Moni is scheduled to stop operating at the end of 2011, and desalination at Garyllis aquifer is planned to cease operation at the end of 2014.

Table 3. Desalination plants in operation or scheduled to operate in Cyprus (except in the area of Paphos).

Location	Start year	Capacity (m3/day)	Price of water sold to WDD (Euros/m3)
Dekelia	1997	40,000	0.6424
Larnaca	2001	52,000	0.6817
Moni (mobile)	2009	20,000	1.3870
Garyllis aquifer	2009	9,000	0.2992
Dekelia (expansion)	2009	20,000	0.7800
Larnaca (expansion)	2009	10,000	1.3200
Limassol	2012	40,000	0.8725
Vasilikos	2012	60,000	0.8130
Limassol (expansion)	2015	20,000	0.8725

Source: Cyprus Water Development Department (website www.moa.gov.cy/wdd and official announcements, February 2010).Note: The mobile plant of Moni is scheduled to stop operating at the end of 2011, and desalination at Garyllis aquifer is planned to cease operation at the end of 2014.

The schedule shown in Table 3 has been designed in order to ensure that virtually all urban residential water needs in Cyprus can be met by desalination generated water, so that a) water supply to households and firms becomes independent of weather conditions and b) all freshwater reserves are supplied to the agricultural sector in order to restore groundwater reserves, which are currently being depleted due to over-exploitation by farmers. As Table 4 shows, even under scenario 3, which assumes rapid increase in water demand, and despite climate change, desalination can more or less satisfy all water demand of households, industry and tourism until 2030 (this may not be exactly accurate because the number of municipalities or communities supplied with desalinated water may increase in the future).

On the basis of these data, it is possible to calculate the costs to the governmental authorities associated with the operation of all these desalination plants. It has to be reminded that desalination is an energy-intensive process requiring large amounts of electricity—about 4.5 kilowatt-hours of electricity per cubic meter of water produced. This explains to a large extent the quite high prices of desalinated water purchased by authorities.

Table 5 demonstrates the resulting costs from the operation of all new desalination plants (i.e., except the already existing plants of Larnaca and Dekelia). These are the costs of purchasing desalinated water plus operation and maintenance costs of governmental authorities that supply this water to consumers, minus the current country-average water price; this means that what is calculated here are only the desalination costs incurred in addition to current cost levels, because it is appropriate to compare only these additional costs with the loss of consumer surplus due to water shortages. According to Table 5, these additional costs are pretty high, exceeding 400 million Euros’ 2009, and thus seem to be considerably higher than the social costs of water shortages shown in Table 1 and Table 2. A sensitivity analysis of the results of the previous section reveals that only under assumptions of high growth of water demand and at a rather low price elasticity (below 0.2 in absolute terms) can desalination costs become comparable to the social costs reported above.

Three aspects of this calculation have to be kept in mind:

-

First, the calculated desalination costs do not include costs from eventual local environmental degradation due to a) the potentially negative impact of desalination plants on marine ecosystems and b) local air pollution caused by emissions of sulfur dioxide, nitrogen oxides or particulate matter from power plants that have to operate more intensively in order to fulfill the electricity needs of desalination plants. Specifically as regards air pollution, European studies of oil-fired power plants, such as the ones operating in Cyprus, have found external costs in the order of 1–3 Eurocents per kilowatt-hour of electricity generated [16]. Since power plants in Cyprus do not operate very close to densely populated areas and hence air pollution affects a relatively small population, a lower-end estimate of these externalities would be appropriate. Still, with 4.5 kilowatt-hours of electricity demanded per cubic meter of desalinated water, external costs may lie around 5 Eurocents per m³ and hence should not be neglected.

-

Second, the analysis has not taken into account the fact that electricity prices will increase due to the obligation of the Electricity Authority of Cyprus (EAC), the major power company in the island, to purchase carbon dioxide permits because of its participation in the EU Emissions Trading System. This extra cost will be passed from desalination plant operators through to the government. For the period 2010–2012, the costs of permits to be purchased by EAC because it will exceed the free emission allowances provided by the government of Cyprus, at a current price of 10–20 Euros per tonne of CO₂, will amount to an increased cost of production for desalinated water of 3.5–6.5 Eurocents per m^3. From 2013 onwards, when the EAC will have to purchase all its emission permits in auctions, purchase costs for desalination water shown in Table 3 may rise by about 5 to 10 Eurocents per m³—at an assumed permit price of 20–30 Euros per tonne of CO₂—so that desalination costs may be 5–8% higher than those shown in Table 5. This cost calculation depends on the following assumptions: a) the government intends to exploit an option provided by EU legislation and distribute 70% of emission allowances for free in 2013 and gradually increase the amount auctioned until it reaches 100% in 2020; b) from 2014 onwards a substantial portion of electricity generated is scheduled to come from modern combined cycle gas turbine plants burning natural gas, which emit about half the amount of CO₂ emitted by conventional fuel oil fired plants. According to official forecasts up to 2020, and extrapolations made by the author, the share of natural gas powered electricity is expected to be about 50% in 2015 and rise to 80% by 2020 and 90% in 2030. If we include only the additional production cost in line with assumptions a) and b) above, then post-2012 desalination costs will increase by 3–6 Eurocents per m^3. If, however, we account for the full environmental costs of CO₂ emissions, regardless of whether EAC pay for all emission allowances, then these costs rise up to 10 Eurocents per m³ for the year 2013, and then gradually fall to 5–7.5 Eurocents per m³ for subsequent years as a result of the penetration of natural gas in the electricity system

-

Third, these costs have partly been adjusted for inflation, assuming an annual GDP deflator of 3%. The initially agreed price at which the WDD purchases desalinated water will increase over the years because the contracts signed between the government and desalination plant owners allow for changes in prices due to changes in a desalination plant’s labor and energy costs. This will affect both energy costs and operation and maintenance (O&M) costs, which represent about 45% and 20% of total desalination costs, respectively [17].

The result shown in Table 5 illustrates that if no new desalination plants are built, the costs from the resulting water shortages in non-agricultural sectors would be considerably lower. This finding implies that, instead of desalination, a less costly approach to water scarcity in Cyprus would be the increase in end-user water prices in order to cover the cost of this scarcity and encourage water conservation. This possibility is analyzed in the following section.

Table 4. Water surplus (annual demand minus annual supply in million m³ per year) in the residential sector due to new desalination plants, by scenario.

Year	Without climate change effects			Including climate change effects
	Scenario 1	Scenario 2	Scenario 3	Scenario 1	Scenario 2	Scenario 3
2010	12.6	12.6	12.6	12.6	12.6	12.6
2011	12.0	11.4	10.9	11.9	11.3	10.8
2012	32.3	31.2	30.0	32.1	31.0	29.8
2013	31.9	30.2	28.4	31.6	29.9	28.2
2014	31.5	29.2	26.8	31.1	28.8	26.4
2015	33.9	31.0	28.0	33.4	30.5	27.5
2016	33.5	30.0	26.3	32.9	29.4	25.7
2017	33.3	29.1	24.7	32.6	28.4	24.0
2018	33.0	28.2	23.0	32.2	27.4	22.3
2019	32.7	27.3	21.4	31.9	26.4	20.5
2020	32.5	26.3	19.7	31.5	25.4	18.7
2021	32.2	25.4	17.9	31.1	24.3	16.8
2022	32.0	24.5	16.2	30.9	23.4	15.0
2023	31.8	23.7	14.5	30.6	22.4	13.2
2024	31.6	22.8	12.7	30.3	21.4	11.3
2025	31.4	21.9	10.9	30.0	20.4	9.4
2026	31.3	20.9	9.0	29.7	19.4	7.4
2027	31.2	20.1	7.2	29.5	18.5	5.6
2028	31.1	19.3	5.4	29.3	17.6	3.7
2029	31.0	18.5	3.6	29.1	16.7	1.7
2030	30.9	17.7	1.7	29.0	15.7	-0.2

Note: It is assumed that plants will operate at 90% of their capacity.

Table 4. Water surplus (annual demand minus annual supply in million m³ per year) in the residential sector due to new desalination plants, by scenario.

Year	Without climate change effects			Including climate change effects
	Scenario 1	Scenario 2	Scenario 3	Scenario 1	Scenario 2	Scenario 3
2010	12.6	12.6	12.6	12.6	12.6	12.6
2011	12.0	11.4	10.9	11.9	11.3	10.8
2012	32.3	31.2	30.0	32.1	31.0	29.8
2013	31.9	30.2	28.4	31.6	29.9	28.2
2014	31.5	29.2	26.8	31.1	28.8	26.4
2015	33.9	31.0	28.0	33.4	30.5	27.5
2016	33.5	30.0	26.3	32.9	29.4	25.7
2017	33.3	29.1	24.7	32.6	28.4	24.0
2018	33.0	28.2	23.0	32.2	27.4	22.3
2019	32.7	27.3	21.4	31.9	26.4	20.5
2020	32.5	26.3	19.7	31.5	25.4	18.7
2021	32.2	25.4	17.9	31.1	24.3	16.8
2022	32.0	24.5	16.2	30.9	23.4	15.0
2023	31.8	23.7	14.5	30.6	22.4	13.2
2024	31.6	22.8	12.7	30.3	21.4	11.3
2025	31.4	21.9	10.9	30.0	20.4	9.4
2026	31.3	20.9	9.0	29.7	19.4	7.4
2027	31.2	20.1	7.2	29.5	18.5	5.6
2028	31.1	19.3	5.4	29.3	17.6	3.7
2029	31.0	18.5	3.6	29.1	16.7	1.7
2030	30.9	17.7	1.7	29.0	15.7	-0.2

Note: It is assumed that plants will operate at 90% of their capacity.

Table 5. Future annual desalination costs for the government of Cyprus (million Euros) due to desalination plants starting operation after 2008.

Year	Desalinated water quantity (m m3)	Net desalinated water quantity (m m3)*	Costs at current prices	Costs at 2009 prices**
2010	19.4	15.3	18.3	18.1
2011	19.4	15.3	18.3	17.8
2012	45.7	36.0	35.2	33.7
2013	45.7	36.0	35.2	33.2
2014	45.7	36.0	35.2	32.7
2015	49.3	38.8	39.8	36.4
2016	49.3	38.8	39.8	35.9
2017	49.3	38.8	39.8	35.4
2018	49.3	38.8	39.8	34.9
2019	49.3	38.8	39.8	34.3
2020	49.3	38.8	39.8	33.8
2021	49.3	38.8	39.8	33.3
2022	49.3	38.8	39.8	32.8
2023	49.3	38.8	39.8	32.3
2024	49.3	38.8	39.8	31.9
2025	49.3	38.8	39.8	31.4
2026	49.3	38.8	39.8	30.9
2027	49.3	38.8	39.8	30.5
2028	49.3	38.8	39.8	30.0
2029	49.3	38.8	39.8	29.6
2030	49.3	38.8	39.8	29.1
Total costs, 2010–2030			779.9	658.1
Present value of costs, 2010–2030			506.6	435.7

* Quantity available from desalination plants minus water distribution losses.** Assuming that half of the costs have to be adjusted for inflation and using a GDP deflator of 3% p.a.

Table 5. Future annual desalination costs for the government of Cyprus (million Euros) due to desalination plants starting operation after 2008.

Year	Desalinated water quantity (m m3)	Net desalinated water quantity (m m3)*	Costs at current prices	Costs at 2009 prices**
2010	19.4	15.3	18.3	18.1
2011	19.4	15.3	18.3	17.8
2012	45.7	36.0	35.2	33.7
2013	45.7	36.0	35.2	33.2
2014	45.7	36.0	35.2	32.7
2015	49.3	38.8	39.8	36.4
2016	49.3	38.8	39.8	35.9
2017	49.3	38.8	39.8	35.4
2018	49.3	38.8	39.8	34.9
2019	49.3	38.8	39.8	34.3
2020	49.3	38.8	39.8	33.8
2021	49.3	38.8	39.8	33.3
2022	49.3	38.8	39.8	32.8
2023	49.3	38.8	39.8	32.3
2024	49.3	38.8	39.8	31.9
2025	49.3	38.8	39.8	31.4
2026	49.3	38.8	39.8	30.9
2027	49.3	38.8	39.8	30.5
2028	49.3	38.8	39.8	30.0
2029	49.3	38.8	39.8	29.6
2030	49.3	38.8	39.8	29.1
Total costs, 2010–2030			779.9	658.1
Present value of costs, 2010–2030			506.6	435.7

* Quantity available from desalination plants minus water distribution losses.** Assuming that half of the costs have to be adjusted for inflation and using a GDP deflator of 3% p.a.

When interpreting the results, it is important to keep in mind that these calculations assume that, regardless of the water quantities delivered to non-agricultural consumers, the availability of water to agriculture will not be affected. As already mentioned, in periods of intense water scarcity it is standard practice of governmental authorities to restrict the amount of freshwater supplied to farmers, which leads to overexploitation of groundwater reservoirs. Calculations shown here do not deal with this problem because the analysis shown here attempts to assess the costs of water scarcity to non‑agricultural sectors all else being equal, i.e., assuming that agriculture will not be better off or worse off after the implementation of one or other of the measures.

7. Efficient Residential Water Pricing to Account for Scarcity

As a European Union member, Cyprus has to comply with the requirements set out by the EU Water Framework Directive (WFD) [18], which is considered the most important landmark in the history of the EU’s water policy. The WFD builds on previous legislation and aims to achieve a “good ecological status” of water resources within the EU by 2015. Among other provisions, the Directive introduces new water management approaches with particular emphasis on the role of economic tools. More specifically it requires full cost recovery to be the guiding principle for water pricing: end-user water prices should incorporate not only the cost of water service provision, but also environmental and resource costs. The latter costs correspond approximately to the scarcity costs discussed in previous paragraphs. For a detailed analysis of the policy implications of the WFD in Cyprus see [1].

Based on the information collected to calculate scarcity costs in Section 4 to Section 6, it is possible to use annual residential water demand functions and the restrictions in water availability discussed above in order to estimate the marginal user cost for residential water in Cyprus through a dynamic optimization approach. This user cost corresponds to the shadow price of scarcity, which shows how much end-user water prices of households, industry and tourism should increase in order to account for water scarcity in the country and ensure sustainable use of water resources over the longer term, until an alternative ‘inexhaustible’ technology (i.e., desalination) becomes less costly. This shadow price reflects the opportunity cost of scarcity, i.e., the economic benefits foregone due to the water quantities not consumed each year in order to ensure water availability in the future. The optimization is carried out assuming that no new desalination plants will operate from 2009 onwards unless their marginal water production cost becomes cheaper than the sum of marginal conventional water production cost plus scarcity cost. In other words this assessment attempts to answer the question ‘how much should residential water prices increase, and at what rate should available water quantities be used, in order to maximize net social benefits and manage water sustainably’. This optimization method determines also at what time in the future new desalination plants should start operating.

To assess the scarcity price, the annual demand functions—Equation (1) in Section 4—were used. As for supply costs, since the analysis is carried out at the consumer level, the costs of water supply to end-users is of interest—and not the cost of water distribution from the main governmental authority (WDD) to individual water boards that further sell water to end-users. Based on data from the annual accounts of the three Municipal Water Boards serving the main urban areas of Cyprus, omitting fixed water charges (in order to obtain only the variable portion of water charges), and assuming that similar cost levels apply for the rest of municipalities and communities of the country, a weighted average end-user price for the period 2005–2007 was calculated. This may reasonably be considered as the marginal cost of water supply to consumers. Marginal supply cost is assumed to remain constant for modest variations in quantities consumed because the contracts signed by the government with desalination plant operators foresee that, for every additional cubic meter of water to be provided by these plants, the government will pay the sum of operation/maintenance costs and energy costs, which, for the current desalination plants, is very close to the average supply cost mentioned above. In other words, the supply cost curve is almost horizontal at these water quantity levels (see also Section 4).

It was further assumed, in the scenarios without climate change, that stocks of freshwater in dams that are available for residential water use each year for the period 2010–2030 remain constant at the average level of years 2005–2006. Those were two years with sufficient to moderate water storage in dams, and with more or less an acceptable supply of water to agriculture. For the climate change scenarios, the 10% reduction factor (mentioned in Section 5) was used for freshwater availability in 2030, and linear interpolations between the 2010 figure and the 2030 figure for intermediate years. Water distribution losses were considered to remain constant as a fraction of total water supply. Obviously, water supplied from desalination plants that existed before 2009 was also taken into account.

As regards the alternative inexhaustible option, i.e., new desalination plants, its marginal production cost was assumed to be 150 Eurocents per cubic meter at 2009 prices and to remain constant over the years in real terms. This assumption was based on the current costs of desalinated water production by plants that are currently constructed in Cyprus, which, according to Table 3, are in the range of 0.8–0.9 Euros per m³. On top of these costs, one has to add the additional operating costs of governmental authorities, i.e., the WDD and the Municipal Water Boards, which amount to at least 0.5−0.6 Euros per m³ at 2009 prices, plus environmental costs which, according to Section 6, amount to more than 0.1 Euro per m³ [17]. Evidently this cost reflects the costs of plants applying today’s desalination technology used in Cyprus (reverse osmosis) and ignoring eventual future cost reductions due to technical progress. Currently installed mobile desalination plants exhibit higher operation costs but are not considered here because a long-term planning process is simulated, which does not rely on urgent short-term measures such as mobile desalination.

Calculation of efficient water prices was carried out by maximizing the present value of total net benefits to consumers due to water consumption for the entire period 2010–2030, subject to the constraint of water availability and in the presence of two technologies: a ‘conventional’ water supply technology and an alternative inexhaustible technology, i.e., new desalination plants, at a higher cost [19]. Following the notation of equation (1), the present value of net benefits is given by Equation (3):

$${\displaystyle \sum _{i=2010}^{2030}}\frac{\exp (c_t)·\frac{1}{\frac{1}{\eta }+1}·{(q_i+q_{\mathrm{di}})}^{(\frac{1}{\eta }+1)} - s_i·q_i - d_i·q_{di}}{{(1+r)}^{i-2010}}$$

(3)

and the constraint is

$${\displaystyle \sum _{i=2010}^{2030}}{q}_i\le Q$$

(4)

where s_i is the marginal water supply cost, assumed to be constant and equal to 84.7 Eurocents’ 2009 per m³; d_i is the marginal desalination cost, assumed to be constant and equal to 150 Eurocents’ 2009 per m³; and r is the social discount rate, assumed to be 4%. q_i and q_di are water quantities provided in year i from currently existing dams and plants and from new desalination plants respectively—obviously the total amount of water consumed in a year is q_i + q_di.

Optimization involves maximizing Z in the following expression:

$$Z={\displaystyle \sum _{i=2010}^{2030}} \frac{\exp (c_i)·\frac{1}{\frac{1}{\eta }+1}·{(q_i+q_{di})}^{(\frac{1}{\eta }+1)} - s_i·q_i - d_i·q_{di}}{{(1+r)}^{i-2010}}-\lambda ·({\displaystyle \sum _{i=2010}^{2030}}{q}_i - Q)$$

(5)

where λ is the Lagrangian multiplier, expressing in this case the shadow price of water scarcity, and Q is the total stock of water during the period 2010–2030, estimated as described above. Note that Q varies in each scenario because it represents the sum of the stock of available freshwater in each year for those years where existing dams and plants are utilized. If, for example, new desalination plants replace existing water supply methods in year x, Q is the sum of available quantities from year 2010 up to year x−1; freshwater that becomes available from year x onwards is not relevant because it will not be used for residential water supply, hence it does not enter in the calculation of Q.

Table 6 presents the resulting shadow prices λ and quantities q_i and q_di for each one of the three scenarios used earlier in this paper, with and without climate change assumptions. According to the central estimate, prices for residential water users—including industry and tourism that are supplied with water from the same sources—should currently rise by about 40 Eurocents per cubic meter to account for scarcity; for each consecutive year this amount should increase by 4% (the social discount rate) and should be further adjusted for inflation (since the shadow price is expressed in constant Eurocents of year 2009). The discount rate reflects the notion that today’s consumption is somewhat more valuable than future consumption because, among other reasons, the future is fraught with uncertainty and because society will be richer in the future, so that an additional Euro today will contribute more to welfare than an additional Euro in the future. The ‘social’ discount rate expresses the preference of society as a whole—private discount rates are usually higher. We use a social discount rate of 4%, in line with guidance from governments of developed countries [20] and assuming that, as in the last three decades, the annual real economic growth rate in Cyprus will be somewhat higher than that of other developed economies. It is usual to apply declining discount rates when an analysis extends into the longer term (e.g., about 30 years), but this is not the case in our study. At an annual inflation of 3% this might lead to a water price increase of over 80 Eurocents per m³ in year 2020 and over 1.60 Euros per m³ in 2030 at nominal prices. Compared to current tariff levels in Cypriot cities, the additional 40 Eurocents per m³ would amount to an increase in end-user price of 30−50% for the cities of Nicosia and Larnaca and up to more than 100% for the city of Limassol. Evidently the value of λ varies according to how binding the constraint becomes in the future, which in turn depends on the water demand scenario. If per capita water demand rises at a faster rate in the future, as assumed in scenario 3, i.e., at 2% per year, the scarcity price reaches 43 Eurocents per cubic meter. Conversely, if per capita water demand remains stable as in scenario 1, the scarcity price falls to 27 Eurocents per m³. As discussed earlier, using the climate model predictions up to 2030 provided to the author, it seems that climate change is expected to have a moderate effect, in the order of less than 2 Eurocents’2009 per m³ for scenario 2.

Table 6 demonstrates what the ‘appropriate’ timing would be for introduction of new desalination plants under the different demand scenarios. If per capita water demand remains constant in the future (scenarios 1 and 4), new desalination remains costly throughout the period up to 2030: the total marginal cost (supply cost plus scarcity cost) of existing water supply options remains below 150 Eurocents’ 2009 per m³ even in 2030, despite the fact that the real scarcity price increases by the discount rate every year. The temporal evolution of marginal costs is illustrated in Figure 2 in the case of this paper’s central scenario 2, according to which new desalination options become economically preferable in year 2025, whereas under stronger water demand assumptions (scenario 3), new desalination should enter the market in 2023. Under increased water shortages due to climate change, the entry year for the new plants moves one or two years earlier (2024 and 2022 for scenarios 5 and 6 respectively). In any case, the model indicates that under a long-term water resource planning schedule in Cyprus, extensive use of desalination would have to wait until after 2020—in contrast to the current policies that promote such plants already in 2010 and which, as shown in section 6, constitute clearly a costly solution.

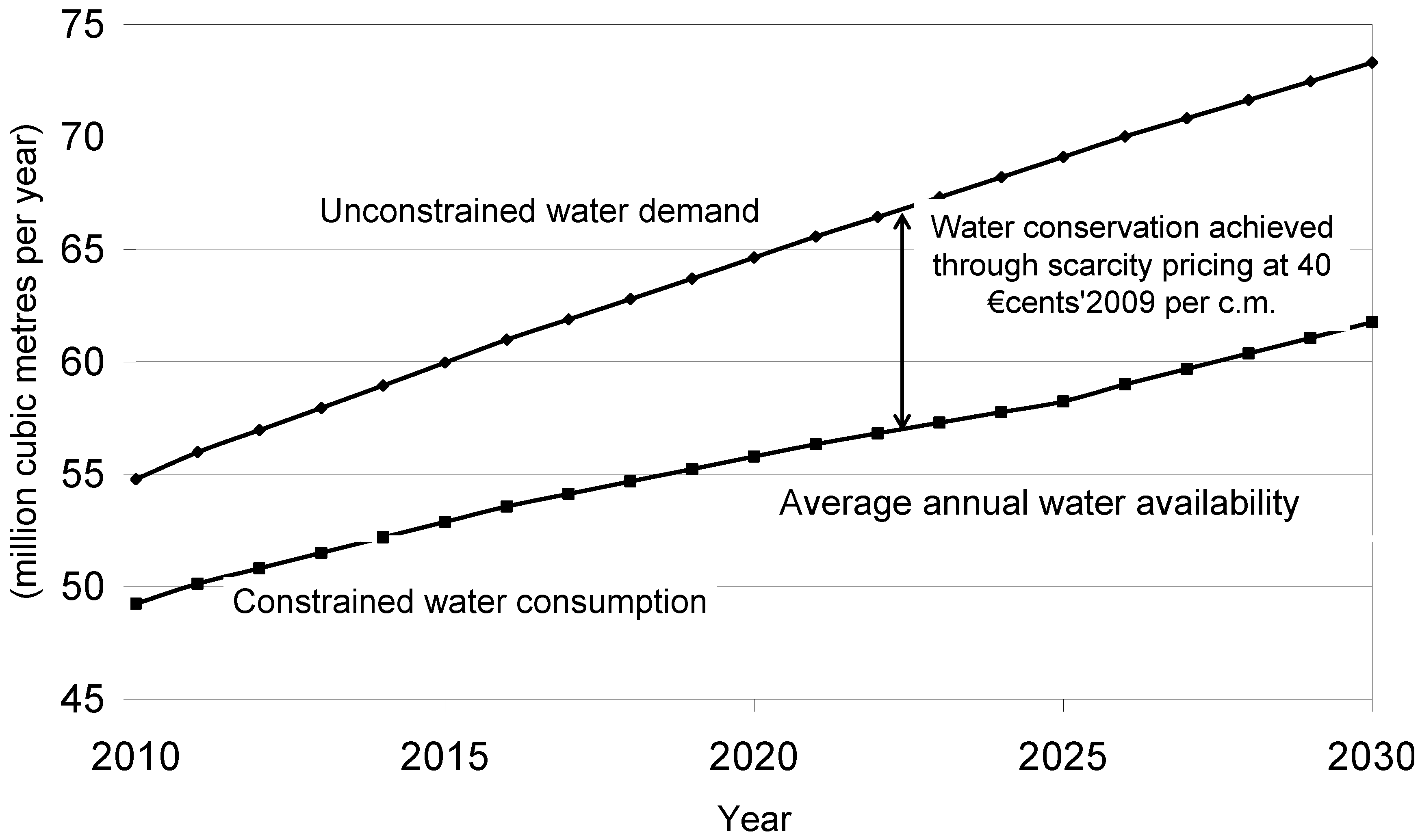
Figure 3 illustrates the evolution of optimal annual water consumption quantities in the period 2010–2030 according to scenario 2, using the central elasticity assumption of −0.3. The upper curve shows the evolution of water demand in the absence of any constraint; this is the evolution shown in Table 1 for scenario 2. The lower curve represents the solution of the constrained optimization problem; the quantities are the totals shown in Table 6 for scenario 2. The difference between these two curves represents the water savings that can be attained if the scarcity price of 40 Eurocents’ 2009 per m³ is implemented throughout the whole period. In fact, water conservation will be attained until 2025 because from that year onwards new desalination plants replace the existing water supply methods; scarcity will no longer be a concern and hence water consumption will start rising at a higher rate. Obviously this approach is somewhat simplified since a price increase today will not be fully effective in the short run but within a period of a few years; nevertheless this graph demonstrates both the large adjustment that is necessary in order to bring future consumption in line with water reserves and the potentially great contribution of an appropriate pricing mechanism towards sustainable water management.

The calculated shadow price rises or falls, respectively, if lower or higher values for the demand elasticity are used. At an elasticity of −0.6 the value of λ for scenario 2 is 25 Eurocents per m³, almost half of the reference case, and climate change adds only another 3 Eurocents to this price. On the other hand, in the extreme assumption of an elasticity of −0.15 (which may be close to reality for the short run but perhaps unrealistically low in absolute terms for the long run), the shadow price increases by 3−4 Eurocents’2009. Since, however, the calculations presented here make sense for the design of a long-term pricing strategy, long-term elasticity values should be used; hence Table 6 seems to present plausible scarcity price levels which, if implemented gradually over a few years and then adjusted annually as explained above (to account for both inflation and the discount rate) might provide adequate incentives for water conservation in households over the medium and longer term.

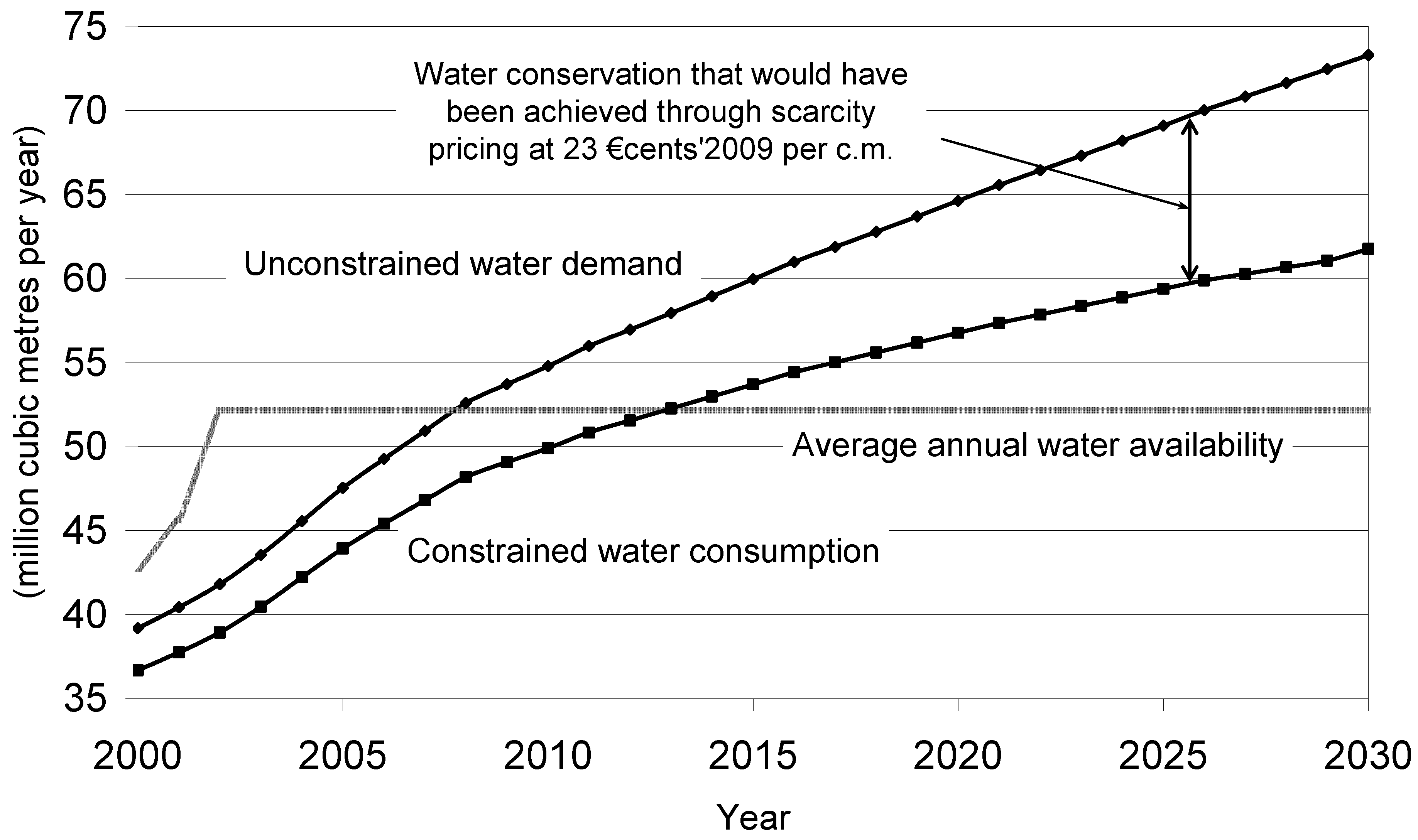
If such a pricing policy had been implemented a decade ago, it might have been sufficient to address the water scarcity problem of Cyprus without the need for extensive use of desalination; apart from the two desalination plants that have been operating for some years now, the construction of new plants might not have been necessary until 2030. To quantify this, a retrospective assessment was made for the entire period 2000–2030, illustrated in Figure 4: A policymaker trying to assess the efficient scarcity price back in the year 2000—at water consumption levels of that period, assuming an increase in water consumption until 2030 similar to that of scenario 2 and with one desalination plant operating and the second one under construction—would have estimated this price at about 23 Eurocents per cubic meter at 2009 prices. The model shows that new desalination plants would become economically preferable only in the year 2030. This means that if this price had been implemented in the year 2000 for the entire 30 year period until 2030, available water quantities might have been able to meet demand without further desalination plants.

Table 6. Marginal scarcity price (λ) and optimal annual water quantities to be supplied by existing sources or new desalination plants in Cyprus.

Year	Optimal water quantities, without climate change (m m3)
	Scenario 1			Scenario 2			Scenario 3
	From existing dams and plants	From new desalination plants	Total	From existing dams and plants	From new desalination plants	Total	From existing dams and plants	From new desalination plants	Total
2010	50.8	0.0	50.8	49.2	0.0	49.2	48.9	0.0	48.9
2011	51.2	0.0	51.2	50.1	0.0	50.1	50.3	0.0	50.3
2012	51.5	0.0	51.5	50.8	0.0	50.8	51.4	0.0	51.4
2013	51.7	0.0	51.7	51.5	0.0	51.5	52.6	0.0	52.6
2014	51.9	0.0	51.9	52.2	0.0	52.2	53.9	0.0	53.9
2015	52.1	0.0	52.1	52.9	0.0	52.9	55.1	0.0	55.1
2016	52.3	0.0	52.3	53.6	0.0	53.6	56.3	0.0	56.3
2017	52.4	0.0	52.4	54.1	0.0	54.1	57.5	0.0	57.5
2018	52.5	0.0	52.5	54.7	0.0	54.7	58.6	0.0	58.6
2019	52.5	0.0	52.5	55.2	0.0	55.2	59.8	0.0	59.8
2020	52.6	0.0	52.6	55.8	0.0	55.8	61.0	0.0	61.0
2021	52.6	0.0	52.6	56.3	0.0	56.3	62.2	0.0	62.2
2022	52.6	0.0	52.6	56.8	0.0	56.8	63.3	0.0	63.3
2023	52.6	0.0	52.6	57.3	0.0	57.3	51.1	13.3	64.5
2024	52.5	0.0	52.5	57.8	0.0	57.8	0.0	66.0	66.0
2025	52.5	0.0	52.5	25.8	32.4	58.2	0.0	67.5	67.5
2026	52.4	0.0	52.4	0.0	59.0	59.0	0.0	69.1	69.1
2027	52.3	0.0	52.3	0.0	59.7	59.7	0.0	70.6	70.6
2028	52.2	0.0	52.2	0.0	60.4	60.4	0.0	72.1	72.1
2029	52.0	0.0	52.0	0.0	61.1	61.1	0.0	73.6	73.6
2030	51.8	0.0	51.8	0.0	61.8	61.8	0.0	75.2	75.2
λ (€ cents’2009):	27.0			39.9			43.1
2010	50.1	0.0	50.1	49.1	0.0	49.1	48.7	0.0	48.7
2011	50.5	0.0	50.5	50.0	0.0	50.0	50.1	0.0	50.1
2012	50.7	0.0	50.7	50.6	0.0	50.6	51.2	0.0	51.2
2013	50.9	0.0	50.9	51.3	0.0	51.3	52.4	0.0	52.4
2014	51.1	0.0	51.1	52.0	0.0	52.0	53.6	0.0	53.6
2015	51.3	0.0	51.3	52.7	0.0	52.7	54.9	0.0	54.9
2016	51.5	0.0	51.5	53.3	0.0	53.3	56.1	0.0	56.1
2017	51.5	0.0	51.5	53.9	0.0	53.9	57.2	0.0	57.2
2018	51.5	0.0	51.5	54.4	0.0	54.4	58.4	0.0	58.4
2019	51.6	0.0	51.6	55.0	0.0	55.0	59.5	0.0	59.5
2020	51.6	0.0	51.6	55.5	0.0	55.5	60.7	0.0	60.7
2021	51.6	0.0	51.6	56.1	0.0	56.1	61.9	0.0	61.9
2022	51.6	0.0	51.6	56.5	0.0	56.5	56.4	6.6	63.0
2023	51.5	0.0	51.5	57.0	0.0	57.0	0.0	64.5	64.5
2024	51.5	0.0	51.5	24.5	33.0	57.5	0.0	66.0	66.0
2025	51.4	0.0	51.4	0.0	58.2	58.2	0.0	67.5	67.5
2026	51.3	0.0	51.3	0.0	59.0	59.0	0.0	69.1	69.1
2027	51.1	0.0	51.1	0.0	59.7	59.7	0.0	70.6	70.6
2028	51.0	0.0	51.0	0.0	60.4	60.4	0.0	72.1	72.1
2029	50.8	0.0	50.8	0.0	61.1	61.1	0.0	73.6	73.6
2030	50.6	0.0	50.6	0.0	61.8	61.8	0.0	75.2	75.2
λ (€ cents’2009):	32.7			41.4			44.8

Table 6. Marginal scarcity price (λ) and optimal annual water quantities to be supplied by existing sources or new desalination plants in Cyprus.

Year	Optimal water quantities, without climate change (m m3)
	Scenario 1			Scenario 2			Scenario 3
	From existing dams and plants	From new desalination plants	Total	From existing dams and plants	From new desalination plants	Total	From existing dams and plants	From new desalination plants	Total
2010	50.8	0.0	50.8	49.2	0.0	49.2	48.9	0.0	48.9
2011	51.2	0.0	51.2	50.1	0.0	50.1	50.3	0.0	50.3
2012	51.5	0.0	51.5	50.8	0.0	50.8	51.4	0.0	51.4
2013	51.7	0.0	51.7	51.5	0.0	51.5	52.6	0.0	52.6
2014	51.9	0.0	51.9	52.2	0.0	52.2	53.9	0.0	53.9
2015	52.1	0.0	52.1	52.9	0.0	52.9	55.1	0.0	55.1
2016	52.3	0.0	52.3	53.6	0.0	53.6	56.3	0.0	56.3
2017	52.4	0.0	52.4	54.1	0.0	54.1	57.5	0.0	57.5
2018	52.5	0.0	52.5	54.7	0.0	54.7	58.6	0.0	58.6
2019	52.5	0.0	52.5	55.2	0.0	55.2	59.8	0.0	59.8
2020	52.6	0.0	52.6	55.8	0.0	55.8	61.0	0.0	61.0
2021	52.6	0.0	52.6	56.3	0.0	56.3	62.2	0.0	62.2
2022	52.6	0.0	52.6	56.8	0.0	56.8	63.3	0.0	63.3
2023	52.6	0.0	52.6	57.3	0.0	57.3	51.1	13.3	64.5
2024	52.5	0.0	52.5	57.8	0.0	57.8	0.0	66.0	66.0
2025	52.5	0.0	52.5	25.8	32.4	58.2	0.0	67.5	67.5
2026	52.4	0.0	52.4	0.0	59.0	59.0	0.0	69.1	69.1
2027	52.3	0.0	52.3	0.0	59.7	59.7	0.0	70.6	70.6
2028	52.2	0.0	52.2	0.0	60.4	60.4	0.0	72.1	72.1
2029	52.0	0.0	52.0	0.0	61.1	61.1	0.0	73.6	73.6
2030	51.8	0.0	51.8	0.0	61.8	61.8	0.0	75.2	75.2
λ (€ cents’2009):	27.0			39.9			43.1
2010	50.1	0.0	50.1	49.1	0.0	49.1	48.7	0.0	48.7
2011	50.5	0.0	50.5	50.0	0.0	50.0	50.1	0.0	50.1
2012	50.7	0.0	50.7	50.6	0.0	50.6	51.2	0.0	51.2
2013	50.9	0.0	50.9	51.3	0.0	51.3	52.4	0.0	52.4
2014	51.1	0.0	51.1	52.0	0.0	52.0	53.6	0.0	53.6
2015	51.3	0.0	51.3	52.7	0.0	52.7	54.9	0.0	54.9
2016	51.5	0.0	51.5	53.3	0.0	53.3	56.1	0.0	56.1
2017	51.5	0.0	51.5	53.9	0.0	53.9	57.2	0.0	57.2
2018	51.5	0.0	51.5	54.4	0.0	54.4	58.4	0.0	58.4
2019	51.6	0.0	51.6	55.0	0.0	55.0	59.5	0.0	59.5
2020	51.6	0.0	51.6	55.5	0.0	55.5	60.7	0.0	60.7
2021	51.6	0.0	51.6	56.1	0.0	56.1	61.9	0.0	61.9
2022	51.6	0.0	51.6	56.5	0.0	56.5	56.4	6.6	63.0
2023	51.5	0.0	51.5	57.0	0.0	57.0	0.0	64.5	64.5
2024	51.5	0.0	51.5	24.5	33.0	57.5	0.0	66.0	66.0
2025	51.4	0.0	51.4	0.0	58.2	58.2	0.0	67.5	67.5
2026	51.3	0.0	51.3	0.0	59.0	59.0	0.0	69.1	69.1
2027	51.1	0.0	51.1	0.0	59.7	59.7	0.0	70.6	70.6
2028	51.0	0.0	51.0	0.0	60.4	60.4	0.0	72.1	72.1
2029	50.8	0.0	50.8	0.0	61.1	61.1	0.0	73.6	73.6
2030	50.6	0.0	50.6	0.0	61.8	61.8	0.0	75.2	75.2
λ (€ cents’2009):	32.7			41.4			44.8

Figure 2. Evolution of marginal water supply costs in the period 2010–2030 according to results of scenario 2; from 2025 onwards existing water supply options are substituted by new desalination plants.

Figure 2. Evolution of marginal water supply costs in the period 2010–2030 according to results of scenario 2; from 2025 onwards existing water supply options are substituted by new desalination plants.

Figure 3. Evolution of optimal water consumption quantities throughout the period 2010–2030 according to scenario 2, at a price elasticity of −0.3. Note: Annual water availability takes into account the existing desalination plants of Dekelia and Larnaca, without their capacity expansions that became operational in 2009.

Figure 3. Evolution of optimal water consumption quantities throughout the period 2010–2030 according to scenario 2, at a price elasticity of −0.3. Note: Annual water availability takes into account the existing desalination plants of Dekelia and Larnaca, without their capacity expansions that became operational in 2009.

Figure 4. Evolution of optimal water consumption quantities for the period 2000–2030 if efficient pricing had been implemented in the year 2000. Note: Annual water availability takes into account the desalination plants of Dekelia (which started operating in 1997) and Larnaca (which became fully operational in 2002).

Figure 4. Evolution of optimal water consumption quantities for the period 2000–2030 if efficient pricing had been implemented in the year 2000. Note: Annual water availability takes into account the desalination plants of Dekelia (which started operating in 1997) and Larnaca (which became fully operational in 2002).

As things currently stand (at the beginning of 2010 with several desalination plants operating or are in construction), an implementation of the pricing policy described above might mitigate the additional needs for desalination in the future, thereby reducing the costs of adapting to water scarcity.

It is understandable that, apart from economic efficiency, equity is also a priority for governmental authorities. A substantial increase in the end-user price of water might put a burden on low-income households whose water demand, as mentioned in Section 3, seems to be the most inelastic. According to the Family Expenditure Survey carried out by the Statistical Service of Cyprus for the year 2003, domestic water expenditures represent less than 0.5% of total household expenditures on average, and this fraction becomes somewhat higher—but still less than 1%—for the poorest 20% of households [21]. Therefore, a doubling in the price of water would increase the cost of living for these households by 80–120 Euros per year at 2009 prices; if governmental authorities wish to provide a compensation for these extra costs, a direct payment to households in the form of a lump sum would be preferable. In any case, it is not advisable to subsidize water use on equity grounds, e.g., by reducing water prices for low-income households or waiving the extra scarcity price for low water consumption levels. Although access to clean water is essential for human life, current water use levels in Cyprus are far higher than the minimum amount needed: Savvides et al. [4] estimated water demand at over 200 liters per person per day in Cyprus, and official data show a daily per capita consumption of 120–150 liters, whereas basic water needs for human subsistence (drinking, cooking and basic sanitation) are estimated at 20−50 liters per person per day [22,23]. Therefore, in an economically developed country like Cyprus, it is essential to calculate water prices on the basis of marginal willingness of consumers to pay for water services—which leads to the implementation of the scarcity pricing system proposed above.

8. Conclusions and Outlook

This paper has presented an assessment of the costs of water scarcity in Cyprus, today and in the next twenty years, accounting also for the effect of projected climate change in the region. The focus was on the residential sector (including tourism and industry since these sectors are largely supplied with water from the same sources—freshwater from dams and desalination plants). Using a simple demand function in the absence of more detailed national data, assuming a price elasticity comparable to that of other European countries, and taking 2006 as a reference year because it was a period without serious water scarcity, total scarcity costs in Cyprus were computed for the entire period 2010–2030 for three scenarios of future water demand. The central estimate shows that the present value of total scarcity costs in this period will amount to 72 million Euros (at 2009 prices), and, if future water demand increases somewhat faster, these costs may reach 200 million Euros’ 2009. Regional climate models forecast annual rainfall levels to decrease by 10% at about 2030, with more serious decreases happening later in the 21st century; using this forecast, scarcity costs up to 2030 were found to be 15 million Euros (or 22%) higher than the cost due to the already existing water scarcity in the country.

In response to increasing water demand and stagnating or decreasing supply of freshwater, Cypriot authorities have promoted the operation of desalination plants. Official plans for new desalination plants have been designed in order to ensure that all residential water needs in Cyprus can be met by desalination generated water in the coming years. As governmental authorities have agreed to purchase pre-determined amounts of desalinated water at specific prices, desalination costs were compared with the social costs of water scarcity calculated earlier. Although securing a reliable water supply to all residential, industrial and commercial users, is a legitimate policy objective, results of this analysis indicate the desalination-only option currently promoted by the government of the country seems to be a costly solution under all scenarios, even if environmental costs from the operation of desalination plants are not taken into account.

Finally, to illustrate the alternative to desalination, the paper computed the efficient levels that water prices in all non-agricultural sectors should reach in order to account for water scarcity in Cyprus. As the country constitutes one single river basin and most of its regions are interconnected through water pipelines, this is effectively a nationwide assessment of scarcity prices, quite a rare case in the literature. The central estimate of an appropriate scarcity price is 40 Eurocents per cubic meter at 2009 prices; this would constitute a 30–100% increase in current prices faced by residential consumers and an even higher increase in future decades. If per capita water demand increases faster than assumed in the central scenario, the scarcity price becomes higher. Modest reductions in rainfall levels due to climate change would raise this price by a further 2−3 Eurocents’ 2009. Although politically difficult to implement, such costs should be incorporated in end-user prices in order to provide a clear long-term price signal to consumers and firms, with the aim to attain a sustainable use of water resources in the island—instead of depending entirely on more costly and energy-intensive desalination.

Such a pricing policy might provide adequate incentives for water conservation in households over the medium and longer term. If a similar policy had been implemented a decade ago, with a considerably lower scarcity price, it might have been sufficient to address the water scarcity problem of Cyprus without the need for extensive use of desalination. Apart from the two desalination plants that have been operating for some years now, the construction of new plants might not have been necessary until after the year 2020. Even temporary prolonged drought episodes, such as the one experienced in Cyprus during years 2007–2008, could have been addressed without the need to resort to very costly solutions—such as importing water from abroad at a cost of over four Euros per cubic meter.

A substantial increase in the end-user price of water might put a burden on low-income households. If governmental authorities wish to provide a compensation for these extra costs, a direct payment to households in the form of a lump sum would be preferable. In any case, it is not advisable to subsidize water use on equity grounds, e.g., by reducing water prices for low-income households or waiving the extra scarcity price for low water consumption levels, because such subsidies would weaken the overall target of water conservation.

The calculations presented here are meant to assess the economic effect of different policies on non‑agricultural water use, assuming that the agricultural sector will not be better-off or worse-off after the implementation of one or other measure. This was one of the reasons why 2006 was selected as a reference year, which was not a bad year in terms of supply of irrigation water to agriculture.

To arrive at the assessments shown in this paper, a number of assumptions had to be made which have been reported in detail. In the case of a few crucial parameters—such as the price elasticity and the growth rate of water demand in the future—alternative values have been tested and results have been briefly presented. Despite the uncertainty associated with some of the underlying assumptions, the overall policy implications of this analysis seem to be valid even if different values are assumed for some parameters.

Further research on these issues will focus on the collection of water consumption data from the country’s Municipal Water Boards. If it is possible to gather sufficient official time series, it may be feasible to estimate residential water demand functions with panel econometric methods. Moreover, it will be attempted to assess climate change impacts in Cyprus over the longer term. However, the major policy challenge for Cyprus, as for all other Mediterranean countries, is to determine the appropriate long-run water price levels for all end-users so that marginal benefits are equalized across all sectors. It is therefore particularly important to carry out efficient pricing studies, also for the agricultural sector, which consumes most of the water in the country with questionable economic benefits; to what extent adequate agricultural water supply should be ensured (and at what prices) is a major topic for further research.