The Impact of the Water Tariff on the Economic Feasibility of Rainwater Harvesting for Use in Residential Buildings
Abstract
1. Introduction
2. Materials and Methods
2.1. Study Area
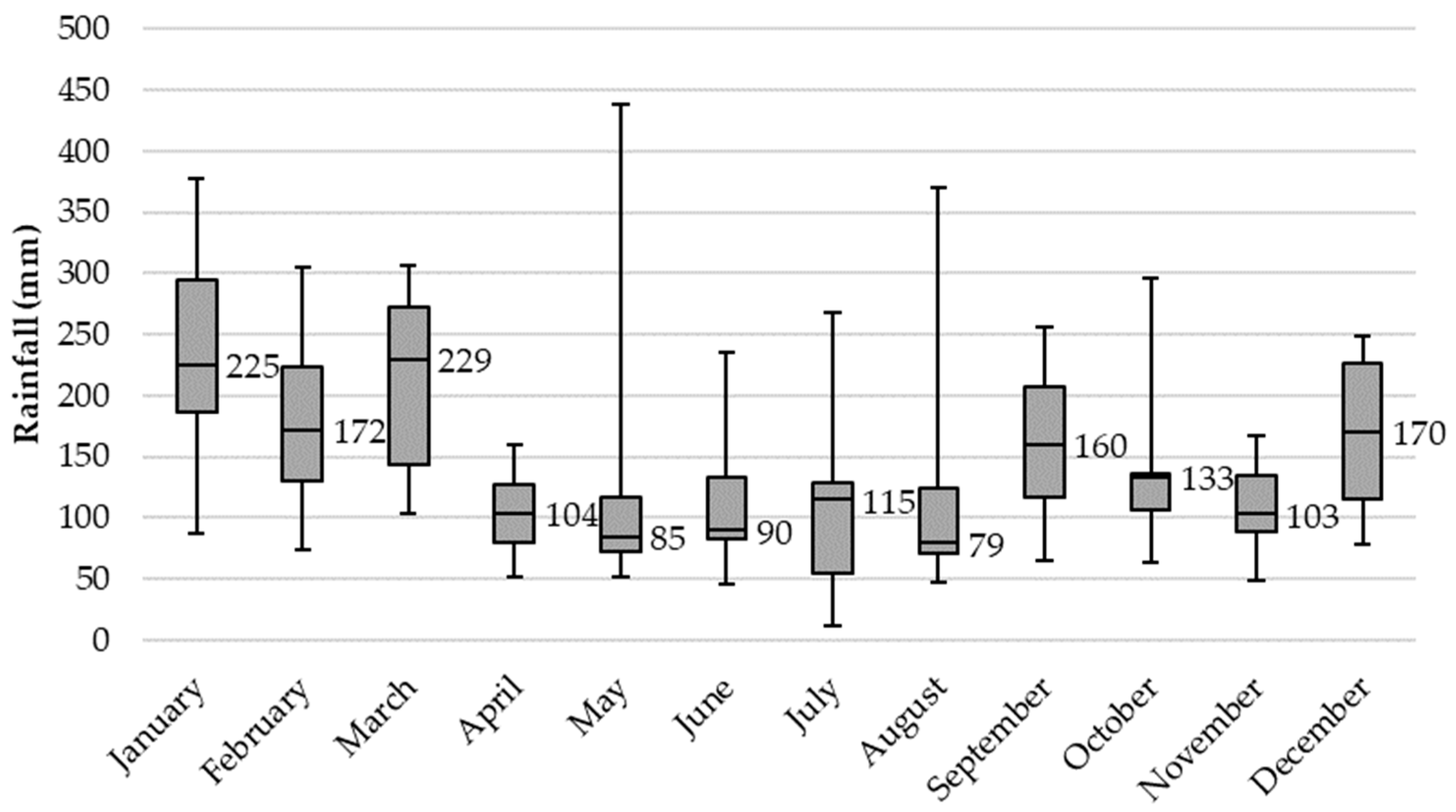
2.2. Rainfall Data
2.3. Simulation Scenarios
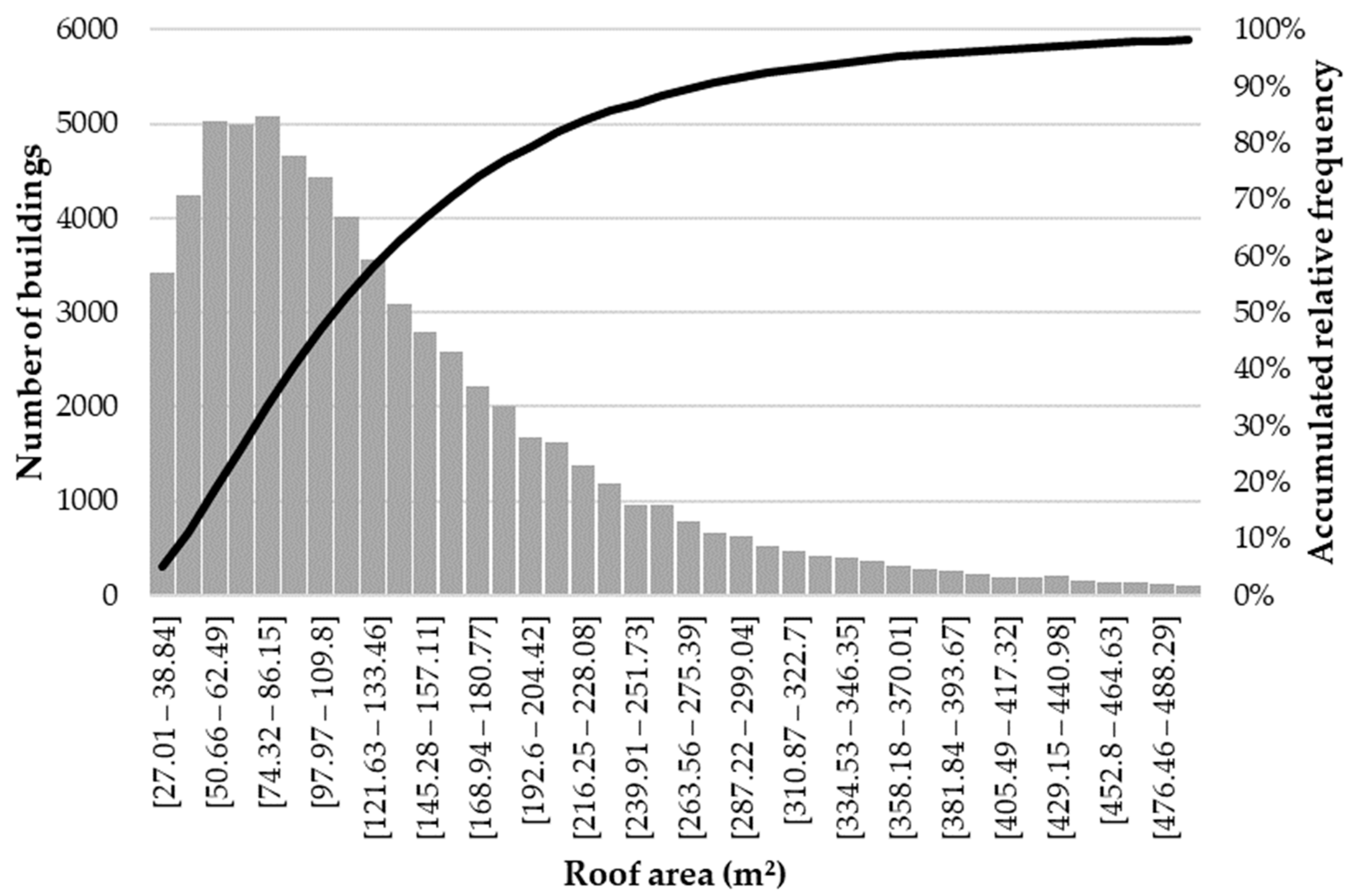
2.4. Representative Buildings
- is the number of categories;
- is the number of buildings in the group.
- is the number of categories;
- is the number of buildings in the subgroup.
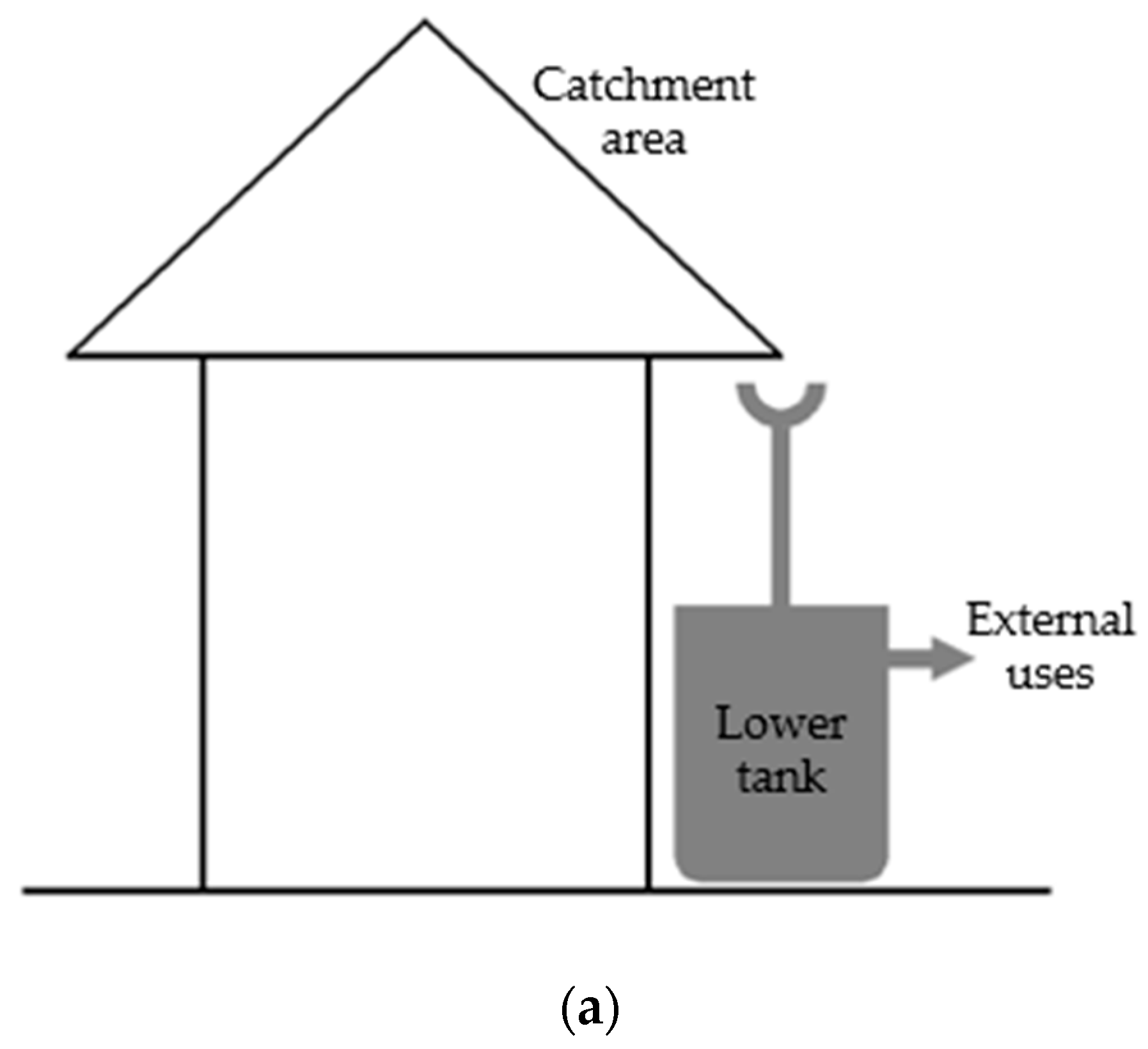
2.5. Rainwater HarvestingRainwater-harvesting Systems’ Simulation
2.6. Technical Viability Assessment
- is the catchment area per cubic metre of rainwater (m2/m3);
- is the roof area of the representative building simulated (m2);
- is the number of residents in the scenario simulated;
- is the total daily per capita water consumption in the scenario simulated (litres);
- is the potential for potable-water savings (%).
2.7. Economic Feasibility Assessment
- is the net present value (R$);
- is the total period of the investment (years);
- is the year of the cashflow (years);
- is the cash flow for the tth period (R$);
- is the rate of return (%).
3. Results
3.1. Representative Buildings
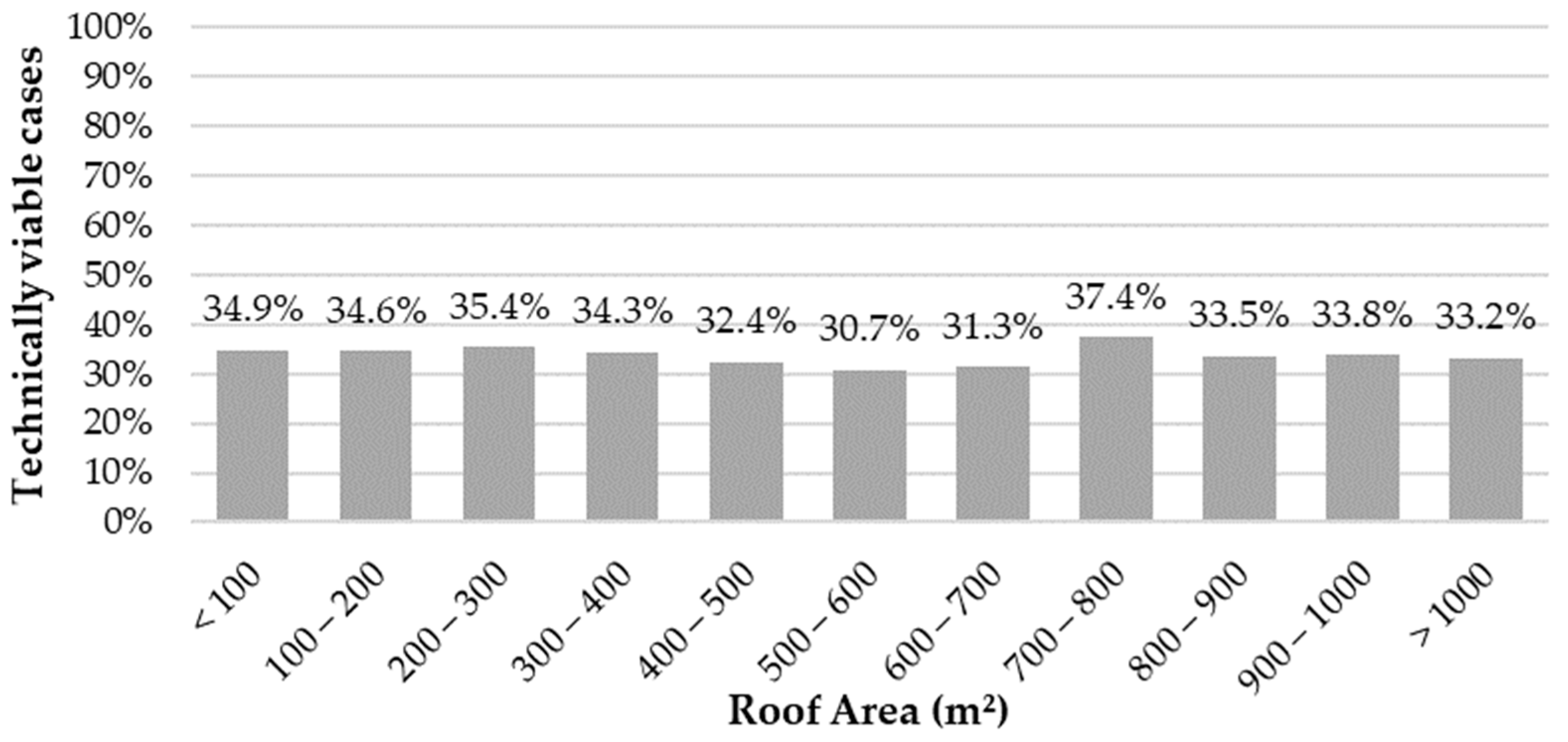
3.2. Potential for Potable-water Savings
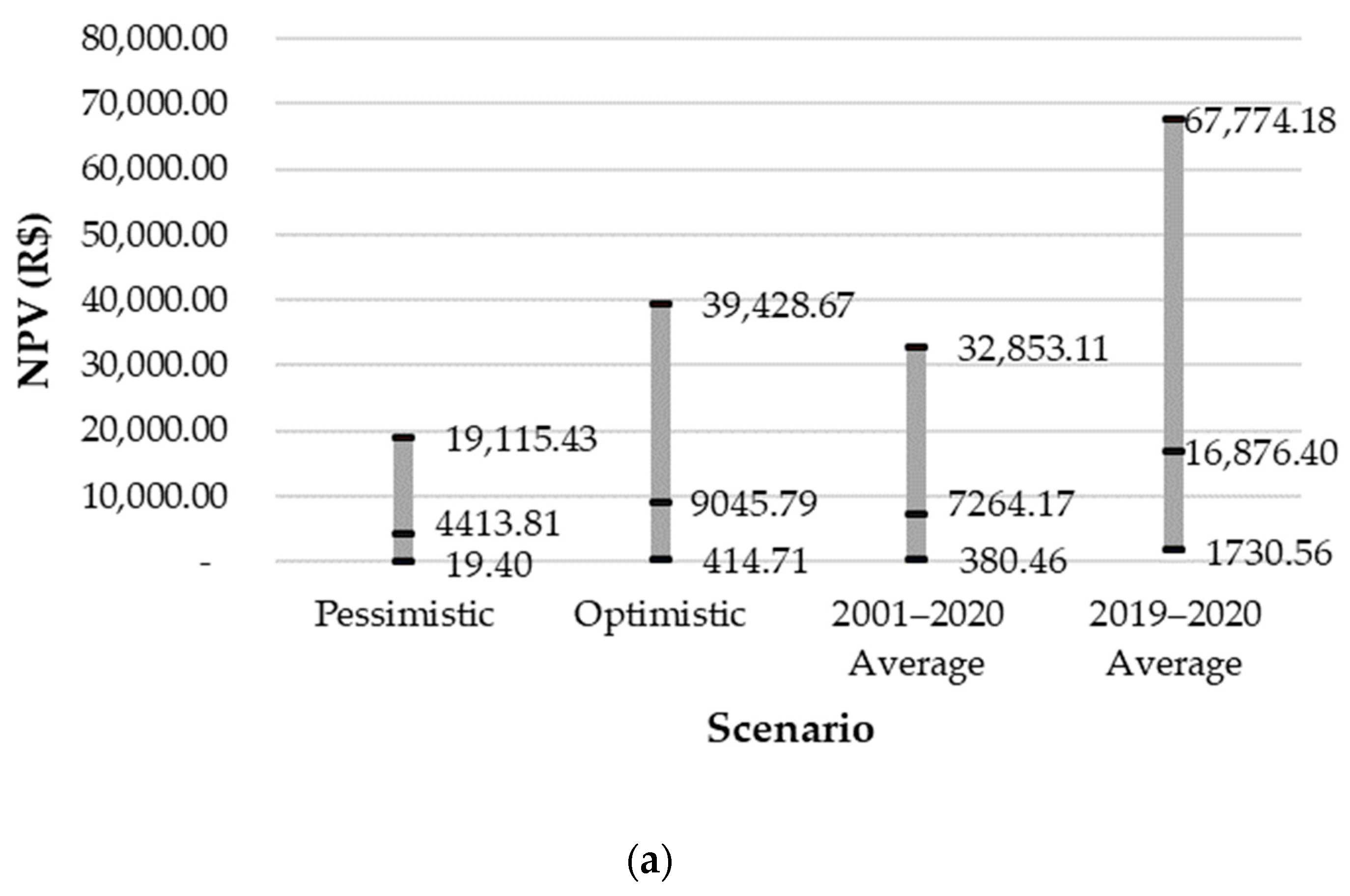
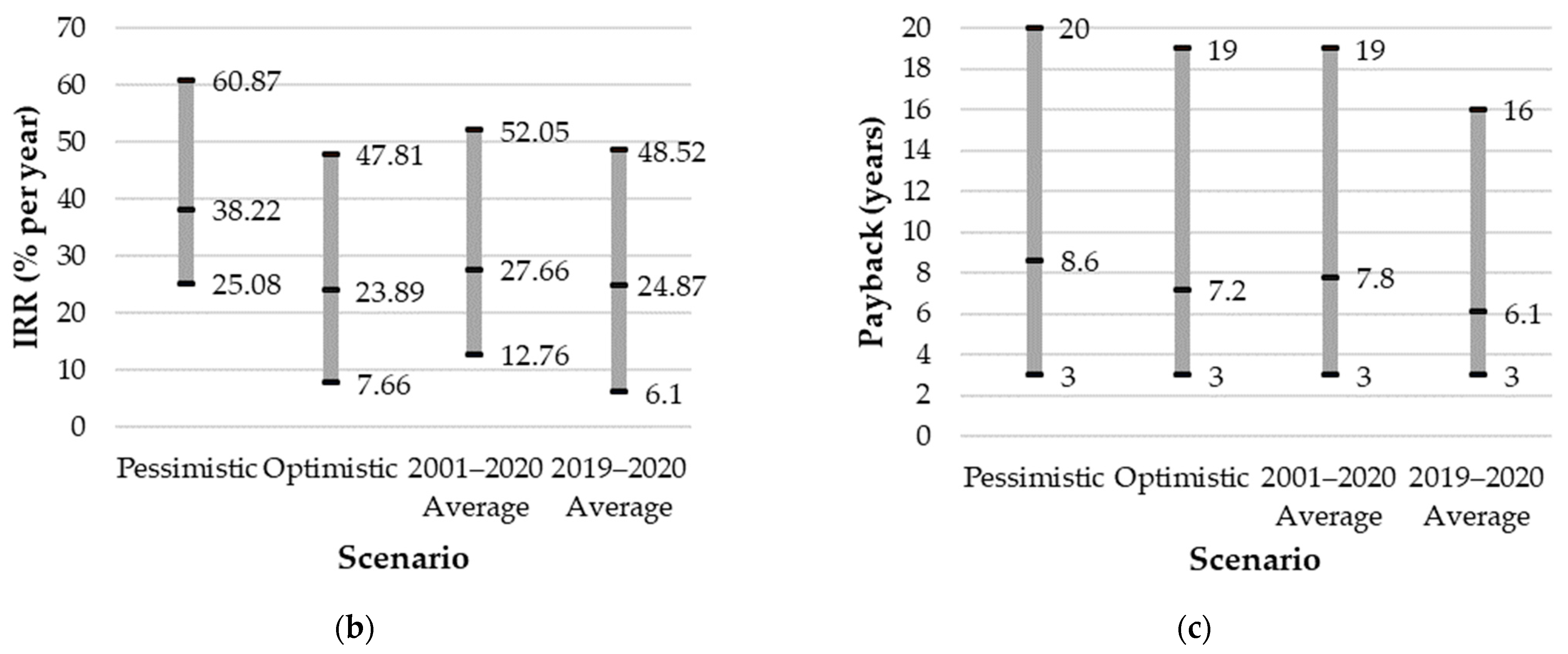
3.3. Economic Feasibility
3.4. Tariff-format Comparison
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- United Nations Environment Programme. Freshwater. In Global Environment Outlook, 6th ed.; United Nations Environment Programme: Cambridge, UK, 2019; pp. 236–269. [Google Scholar]
- Rygaard, M.; Binning, P.J.; Albrechtsen, H.J. Increasing urban water self-sufficiency: New era, new challenges. J. Environ. Manag. 2011, 92, 185–194. [Google Scholar] [CrossRef] [PubMed]
- Abdulla, F.A.; AL-Shareef, A.W. Roof rainwater harvesting systems for household water supply in Jordan. Desalination 2009, 243, 195–207. [Google Scholar] [CrossRef]
- Marinoski, A.K.; Ghisi, E. Environmental performance of hybrid rainwater-greywater systems in residential buildings. Resour. Conserv. Recycl. 2019, 144, 100–114. [Google Scholar] [CrossRef]
- Palla, A.; Gnecco, I.; Lanza, L.G.; Labarbera, P. Performance analysis of domestic rainwater harvesting systems under various European climate zones. Resour. Conserv. Recycl. 2012, 62, 71–80. [Google Scholar] [CrossRef]
- Pavolová, H.; Bakalár, T.; Kudelas, D.; Pułkárová, P. Environmental and economic assessment of rainwater application in households. J. Clean. Prod. 2019, 209, 1119–1125. [Google Scholar] [CrossRef]
- Souza, E.L.; Ghisi, E. Potable Water Savings by Using Rainwater for Non-Potable Uses in Houses. Water 2012, 4, 607–628. [Google Scholar] [CrossRef]
- Freitas, D.A.; Ghisi, E. Economic feasibility analysis of rainwater harvesting: A case study in Imbituba, Brazil. Urban Water J. 2020, 17, 905–911. [Google Scholar] [CrossRef]
- Vieira, A.S. Uso Racional de Água em Habitações de Interesse Social Como Estratégia Para a Conservação de Energia em Florianópolis, Santa Catarina. Masters’s Thesis, Federal University of Santa Catarina, Florianópolis, Brazil, 2012. [Google Scholar]
- Ghisi, E.; Rupp, R.F.; Triska, Y. Comparing indicators to rank strategies to save potable water in buildings. Resour. Conserv. Recycl. 2014, 87, 137–144. [Google Scholar] [CrossRef]
- Proença, L.C.; Ghisi, E.; Tavares, D.F.; Coelho, G.M. Potential for electricity savings by reducing potable water consumption in a city scale. Resour. Conserv. Recycl. 2011, 55, 960–965. [Google Scholar] [CrossRef]
- Gonçalves, R.F. Uso Racional da Água em Edificações; ABES: Rio de Janeiro, Brazil, 2006. [Google Scholar]
- Teston, A.; Teixeira, C.A.; Ghisi, E.; Cardoso, E.B. Impact of rainwater harvesting on the drainage system: Case study of a condominium of houses in Curitiba, Southern Brazil. Water 2018, 10, 1100. [Google Scholar] [CrossRef]
- Teston, A.; Geraldi, M.; Colasio, B.; Ghisi, E. Rainwater Harvesting in Buildings in Brazil: A literature review. Water 2018, 10, 471. [Google Scholar] [CrossRef]
- Ghisi, E.; Montibeller, A.; Schmidt, R.W. Potential for potable water savings by using rainwater: An analysis over 62 cities in southern Brazil. Build. Environ. 2006, 41, 204–210. [Google Scholar] [CrossRef]
- Ghisi, E.; Ferreira, D.F. Potential for potable water savings by using rainwater and greywater in a multi-storey residential building in southern Brazil. Build. Environ. 2007, 42, 2512–2522. [Google Scholar] [CrossRef]
- Ghisi, E.; Oliveira, S.M. Potential for potable water savings by combining the use of rainwater and greywater in houses in southern Brazil. Build. Environ. 2007, 42, 1731–1742. [Google Scholar] [CrossRef]
- Ghisi, E.; Thives, L.P.; Meincheim, D.L. Potential for potable water savings by using rainwater in a house in southern Brazil. In Proceedings of the Iwa Efficient 2017—9th Biennial Specialist Conference on Efficient Use and Management of Water, Bath, UK, 18–20 July 2017. [Google Scholar]
- Marinoski, D.L.; Ghisi, E.; Gómez, L.A. Aproveitamento de água pluvial e dimensionamento de reservatório para fins não potáveis: Estudo de caso em um conjunto residencial localizado em Florianópolis-SC. In Proceedings of the Conferência Latino-Americana de Construção Sustentável, 1, Encontro Nacional de Tecnologia do Ambiente Construído, 10, São Paulo, Brazil, 18–21 July 2004. [Google Scholar]
- Maykot, J.K.; Ghisi, E. Assessment of A Rainwater Harvesting System in A Multi-Storey Residential Building in Brazil. Water 2020, 12, 546. [Google Scholar] [CrossRef]
- Abas, P.G.E.; Mahlia, T.M.I. Techno-Economic and Sensitivity Analysis of Rainwater Harvesting System as Alternative Water Source. Sustainability 2019, 11, 2365. [Google Scholar] [CrossRef]
- Rahman, A.; Keane, J.; Imteaz, M.A. Rainwater harvesting in Greater Sydney: Water savings, reliability and economic benefits. Resour. Conserv. Recycl. 2012, 61, 16–21. [Google Scholar] [CrossRef]
- Ghisi, E.; Cordova, M.M. Netuno 4. Programa computacional. Universidade Federal de Santa Catarina, Departamento de Engenharia Civil. Available online: https://labeee.ufsc.br/downloads/softwares/netuno (accessed on 27 March 2024).
- Ghisi, E. (Universidade Federal de Santa Catarina, Florianópolis, Santa Catarina, Brasil); Cordova, M.M (Universidade Federal de Santa Catarina, Florianópolis, Santa Catarina, Brasil). Personal Communication. 2014. Available online: https://labeee.ufsc.br/sites/default/files/Manual-Netuno-4_Junho2014.pdf (accessed on 27 March 2024).
- Cordova, M.M.; Ghisi, E. Analysis of Potable Water Savings Using Behavioural Models. In Water Conservation, 1st ed.; Jha, M., Ed.; InTech Open: London, UK, 2011; Volume 1, pp. 89–104. Available online: https://www.intechopen.com/chapters/24681 (accessed on 27 March 2024).
- IBGE—Instituto Brasileiro de Geografia e Estatística. Portal Cidades. Available online: https://cidades.ibge.gov.br/brasil/sc/florianopolis/panorama (accessed on 25 October 2021).
- Pandolfo, C.; Braga, H.J.; Silva, V.P., Jr.; Massignam, A.M.; Pereira, E.S.; Thomé, V.M.R.; Valci, F.V. Atlas climatológico do Estado de Santa Catarina. Florianópolis: Epagri. 2002. Available online: https://ciram.epagri.sc.gov.br/ciram_arquivos/atlasClimatologico/atlasClimatologico.pdf (accessed on 25 October 2021).
- INMET—Instituto Nacional de Metereologia. Banco de Dados Meteorológicos. Available online: https://bdmep.inmet.gov.br/# (accessed on 25 February 2021).
- Geraldi, M.S.; Ghisi, E. Influence of the length of rainfall time series on rainwater harvesting systems: A case study in Berlin. Resour. Conserv. Recycl. 2017, 125, 169–180. [Google Scholar] [CrossRef]
- Geraldi, M.S.; Ghisi, E. Assessment of the length of rainfall time series for rainwater harvesting in buildings. Resour. Conserv. Recycl. 2018, 133, 231–241. [Google Scholar] [CrossRef]
- Ghisi, E.; Cardoso, K.A.; Rupp, R.F. Short-term versus long-term rainfall time series in the assessment of potable water savings by using rainwater in houses. J. Environ. Manag. 2012, 100, 109–119. [Google Scholar] [CrossRef]
- SNIS—Sistema Nacional de Informações Sobre Saneamento. In Diagnóstico dos Serviços de Água e Esgotos, 25th ed.; SNS/MDR: Brasília, Brazil, 2020.
- Marinoski, A.K.; Vieira, A.S.; Silva, A.S.; Ghisi, E. Water End-Uses in Low-Income Houses in Southern Brazil. Water 2014, 6, 1985–1999. [Google Scholar] [CrossRef]
- Hammes, G.; Ghisi, E.; Thives, L.P. Water end-uses and rainwater harvesting: A case study in Brazil. Urban Water J. 2020, 17, 177–183. [Google Scholar] [CrossRef]
- Costa, F.M. Estatística, 1st ed.; Universidade do Estado do Pará: Belém-Pará, Brasil, 2011; p. 27. Available online: https://ccse.uepa.br/downloads/material_2010/LIVRO_ESTATISTICA.pdf (accessed on 27 March 2024).
- Basu, A.; Basu, S. A User’s Guide to Analytics, 1st ed.; Taylor & Francis: Boca Raton, FL, USA, 2016; p. 31. [Google Scholar]
- Real Statistics Using Excel. Available online: https://www.real-statistics.com/multivariate-statistics/cluster-analysis/jenks-natural-breaks/ (accessed on 26 November 2021).
- ABNT—Associação Brasileira de Normas Técnicas. NBR 15527: Água de Chuva—Aproveitamento de Coberturas em Áreas Urbanas Para Fins Não Potáveis—Requisitos, 2nd ed.; ABNT—Associação Brasileira de Normas Técnicas: Rio de Janeiro, Brazil, 2019; pp. 1–10. [Google Scholar]
- Plínio Tomáz Engenharia—Aproveitamento de Água de Chuva. Available online: https://www.pliniotomaz.com (accessed on 2 February 2021).
- Severis, R.M.; Silva, F.A.; Wahrlich, J.; Skoronski, E.; Simioni, F.J. Economic analysis and risk-based assessment of the financial losses of domestic rainwater harvesting systems. Resour. Conserv. Recycl. 2019, 146, 206–217. [Google Scholar] [CrossRef]
- CELESC—Centrais Elétricas de Santa Catarina. Tarifas e Taxas de Energia. Available online: https://www.celesc.com.br/tarifas-de-energia#tributos (accessed on 17 December 2021).
- CASAN—Companhia Catarinense de Água e Saneamento. Tarifas. Available online: https://www.casan.com.br/menu-conteudo/index/url/tarifas#0 (accessed on 17 December 2021).
- Casarotto Filho, N.; Kopittke, B.H. Análise de Investimentos, 10th ed.; Atlas: São Paulo, Brazil, 2007; p. 418. [Google Scholar]
- BCB—Banco Central do Brasil. Taxas de Juros Básicas: Histórico. Available online: https://www.bcb.gov.br/controleinflacao/historicotaxasjuros (accessed on 17 December 2021).
- BCB—Banco Central do Brasil. Histórico Das Metas Para a Inflação. Available online: https://www.bcb.gov.br/controleinflacao/historicometas (accessed on 17 December 2021).
- Ghisi, E. Potential for potable water savings by using rainwater in the residential sector of Brazil. Build. Environ. 2006, 41, 1544–1550. [Google Scholar] [CrossRef]
- Lopes, A.C.; Rupp, R.F.; Ghisi, E. Assessment of the potential for potable water savings by using rainwater in houses in southern Brazil. Water Sci. Technol. Water Supply 2016, 16, 533–541. [Google Scholar] [CrossRef]
- Istchuk, R.N.; Ghisi, E. Financial Feasibility Analysis of Residential Rainwater Harvesting in Maringá, Brazil. Sustainability 2022, 14, 12859. [Google Scholar] [CrossRef]
- Ghisi, E.; Schondermark, P.N. Investment Feasibility Analysis of Rainwater Use in Residences. Water Resour. Manag. 2013, 27, 2555–2576. [Google Scholar] [CrossRef]
- Berwanger, H.; Ghisi, E. Investment feasibility analysis of rainwater harvesting in the city of Itapiranga, Brazil. Int. J. Sustain. Hum. Dev. 2014, 2, 104–114. [Google Scholar]




| Scenario | Inflation (% per Year) | MARR (% per Year) |
|---|---|---|
| Pessimistic | 12.53 | 25.00 |
| Optimistic | 2.95 | 7.00 |
| 2001–2020 Average | 6.18 | 12.10 |
| 2019–2020 Average | 4.41 | 3.25 |
| System Design | Rainwater Demand (%) | Technically Viable Cases (%) | Average Potential for Potable-Water Savings for Unviable Cases (%) |
|---|---|---|---|
| 1 | 5 | 100.0 | - |
| 2 | 30 | 98.6 | 21.3 |
| 3 | 50 | 95.0 | 33.6 |
| Number of Flats in the Building | System Design | Technically Viable Cases (%) | Average Potential for Potable-water Savings for Unviable Cases (%) |
|---|---|---|---|
| 2 | 1 | 100.0 | - |
| 2 | 94.2 | 22.1 | |
| 3 | 83.3 | 34.3 | |
| 3–5 | 1 | 99.7 | 4.2 |
| 2 | 62.8 | 17.9 | |
| 3 | 42.0 | 26.7 | |
| 6–10 | 1 | 98.5 | 4.2 |
| 2 | 41.5 | 18.0 | |
| 3 | 17.8 | 26.0 | |
| 11–20 | 1 | 98.0 | 4.2 |
| 2 | 16.3 | 17.8 | |
| 3 | 3.3 | 23.1 | |
| 21–30 | 1 | 93.0 | 4.0 |
| 2 | 5.2 | 15.9 | |
| 3 | 0.0 | 19.1 | |
| 31–40 | 1 | 87.8 | 3.9 |
| 2 | 1.1 | 14.7 | |
| 3 | 0.0 | 16.6 | |
| 41–50 | 1 | 75.5 | 3.9 |
| 2 | 0.0 | 12.4 | |
| 3 | 0.0 | 13.1 | |
| 51–60 | 1 | 59.8 | 3.8 |
| 2 | 0.0 | 10.5 | |
| 3 | 0.0 | 10.7 | |
| 61–70 | 1 | 60.1 | 3.9 |
| 2 | 0.0 | 10.9 | |
| 3 | 0.0 | 10.8 | |
| 71–80 | 1 | 49.6 | 3.7 |
| 2 | 0.0 | 9.9 | |
| 3 | 0.0 | 9.5 | |
| 81–90 | 1 | 42.0 | 3.8 |
| 2 | 0.0 | 9.6 | |
| 3 | 0.0 | 8.9 | |
| 91–100 | 1 | 36.4 | 3.7 |
| 2 | 0.0 | 8.6 | |
| 3 | 0.0 | 7.3 | |
| 101–110 | 1 | 30.2 | 3.7 |
| 2 | 0.0 | 7.6 | |
| 3 | 0.0 | 6.0 | |
| Total | 1 | 78.9 | 3.8 |
| 2 | 14.4 | 13.7 | |
| 3 | 7.1 | 16.7 |
| Scenario | Economically Feasible Cases (%) | Average NPV (R$) | Average IRR (% per Year) | Average Payback (Years) |
|---|---|---|---|---|
| Pessimistic | 60.1 | 4413.81 | 38.22 | 8.6 |
| Optimistic | 74.6 | 9045.79 | 23.89 | 7.2 |
| 2001–2020 Average | 74.3 | 7264.17 | 27.66 | 7.8 |
| 2019–2020 Average | 74.8 | 16,876.40 | 24.87 | 6.2 |
| Number of Flats in the Building | Pessimistic Scenario | Optimistic Scenario | ||||||
|---|---|---|---|---|---|---|---|---|
| Economically Feasible Cases (%) | Average NPV (R$) | Average IRR (% per Year) | Average Payback (Years) | Economically Feasible Cases (%) | Average NPV (R$) | Average IRR (% per Year) | Average Payback (Years) | |
| 2 | 50.8 | 5602.96 | 44.00 | 6.4 | 75.0 | 8995.02 | 25.31 | 7.8 |
| 3–5 | 68.0 | 3551.30 | 45.02 | 7.2 | 75.0 | 7287.73 | 31.19 | 5.7 |
| 6–10 | 75.0 | 2972.33 | 52.56 | 5.0 | 75.0 | 6287.54 | 40.10 | 4.1 |
| 11–20 | 75.0 | 2354.00 | 58.90 | 4.1 | 75.0 | 4837.48 | 45.99 | 3.5 |
| 21–30 | 75.0 | 1687.50 | 58.53 | 4.2 | 75.0 | 3462.67 | 45.65 | 3.5 |
| 31–40 | 74.4 | 1208.76 | 54.20 | 4.5 | 74.9 | 2517.60 | 41.42 | 3.8 |
| 41–50 | 73.9 | 674.86 | 45.14 | 6.1 | 74.8 | 1509.25 | 32.92 | 4.8 |
| 51–60 | 64.7 | 375.04 | 37.41 | 8.9 | 72.6 | 871.57 | 24.46 | 6.9 |
| 61–70 | 39.6 | 260.58 | 33.63 | 10.9 | 66.9 | 510.24 | 17.91 | 9.6 |
| 71–80 | 6.5 | 385.57 | 39.09 | 8.3 | 23.2 | 366.05 | 14.97 | 12.6 |
| 81–90 | 4.3 | 302.81 | 38.26 | 7.1 | 5.0 | 709.53 | 24.76 | 6.5 |
| 91–100 | 2.9 | 93.35 | 29.96 | 12.2 | 3.7 | 336.81 | 17.76 | 8.7 |
| 101–110 | 0.0 | - | - | - | 1.2 | 81.19 | 10.49 | 14.7 |
| Total | 57.8 | 1883.49 | 51.34 | 5.6 | 62.2 | 3758.06 | 37.00 | 5.0 |
| Number of Flats in the Building | 2001–2020 Average Scenario | 2019–2020 Average Scenario | ||||||
|---|---|---|---|---|---|---|---|---|
| Economically Feasible Cases (%) | Average NPV (R$) | Average IRR (% per Year) | Average Payback (Years) | Economically Feasible Cases (%) | Average NPV (R$) | Average IRR (% per Year) | Average Payback (Years) | |
| 2 | 68.9 | 7925.15 | 30.61 | 7.7 | 79.4 | 15,535.05 | 25.08 | 7.0 |
| 3–5 | 74.6 | 5998.29 | 35.17 | 6.1 | 78.3 | 12,450.05 | 30.93 | 5.6 |
| 6–10 | 75.0 | 5214.49 | 44.16 | 4.3 | 75.1 | 10,898.51 | 40.85 | 3.8 |
| 11–20 | 75.0 | 4033.54 | 50.18 | 3.6 | 75.0 | 8302.91 | 46.71 | 3.3 |
| 21–30 | 75.0 | 2888.02 | 49.83 | 3.6 | 75.0 | 5939.71 | 46.37 | 3.3 |
| 31–40 | 74.9 | 2092.95 | 45.54 | 4.0 | 75.0 | 4352.61 | 42.18 | 3.6 |
| 41–50 | 74.8 | 1236.01 | 36.82 | 5.1 | 75.0 | 2682.64 | 33.77 | 4.4 |
| 51–60 | 71.6 | 704.55 | 28.38 | 7.4 | 74.4 | 1597.10 | 25.01 | 6.1 |
| 61–70 | 63.9 | 405.77 | 21.94 | 10.4 | 71.2 | 989.13 | 18.19 | 8.3 |
| 71–80 | 16.3 | 381.21 | 21.53 | 11.5 | 44.9 | 470.08 | 11.05 | 12.9 |
| 81–90 | 4.7 | 592.12 | 29.34 | 6.5 | 5.3 | 1262.75 | 24.46 | 6.3 |
| 91–100 | 3.5 | 260.49 | 21.74 | 9.0 | 4.4 | 612.62 | 16.59 | 8.7 |
| 101–110 | 0.9 | 58.28 | 14.92 | 15.6 | 2.4 | 167.69 | 8.44 | 14.0 |
| Total | 61.4 | 3147.13 | 41.38 | 5.1 | 64.2 | 6374.08 | 36.85 | 4.8 |
| Tariff Format | Economically Feasible Cases (%) | Average NPV (R$) | Average IRR (% per Year) | Average Payback (Years) |
|---|---|---|---|---|
| Former | 74.6 | 13,830.38 | 21.36 | 7.4 |
| Current | 74.8 | 16,893.73 | 24.95 | 6.1 |
| Number of Flats in the Building | Former Tariff | Current Tariff | ||||||
|---|---|---|---|---|---|---|---|---|
| Economically Feasible Cases (%) | Average NPV (R$) | Average IRR (% per Year) | Average Payback (Years) | Economically Feasible Cases (%) | Average NPV (R$) | Average IRR (% per Year) | Average Payback (Years) | |
| 2 | 75.0 | 13,498.46 | 22.35 | 7.6 | 79.4 | 15,535.05 | 25.08 | 7.0 |
| 3–5 | 75.0 | 10,783.31 | 27.81 | 5.9 | 78.3 | 12,450.05 | 30.93 | 5.6 |
| 6–10 | 75.0 | 9105.91 | 35.52 | 4.4 | 75.1 | 10,898.51 | 40.85 | 3.8 |
| 11–20 | 75.0 | 6882.59 | 40.23 | 3.9 | 75.0 | 8302.91 | 46.71 | 3.3 |
| 21–30 | 75.0 | 4806.77 | 39.09 | 4.0 | 75.0 | 5939.71 | 46.37 | 3.3 |
| 31–40 | 75.0 | 3372.48 | 34.35 | 4.4 | 75.0 | 4352.61 | 42.18 | 3.6 |
| 41–50 | 74.5 | 1919.04 | 26.11 | 5.7 | 75.0 | 2682.64 | 33.77 | 4.4 |
| 51–60 | 72.1 | 995.47 | 17.45 | 8.7 | 74.4 | 1597.10 | 25.01 | 6.1 |
| 61–70 | 46.9 | 570.01 | 11.52 | 12.7 | 71.2 | 989.13 | 18.19 | 8.3 |
| 71–80 | 5.3 | 1430.41 | 24.29 | 6.0 | 44.9 | 470.08 | 11.05 | 12.9 |
| 81–90 | 5.1 | 835.40 | 18.30 | 7.9 | 5.3 | 1262.75 | 24.46 | 6.3 |
| 91–100 | 3.4 | 283.93 | 10.20 | 12.3 | 4.4 | 612.62 | 16.59 | 8.7 |
| 101–110 | 0.0 | - | - | - | 2.4 | 67.69 | 8.44 | 14.0 |
| Total | 59.9 | 5506.89 | 31.97 | 5.4 | 64.2 | 6374.08 | 36.85 | 4.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Borgert, A.E.; Ghisi, E. The Impact of the Water Tariff on the Economic Feasibility of Rainwater Harvesting for Use in Residential Buildings. Water 2024, 16, 1058. https://doi.org/10.3390/w16071058
Borgert AE, Ghisi E. The Impact of the Water Tariff on the Economic Feasibility of Rainwater Harvesting for Use in Residential Buildings. Water. 2024; 16(7):1058. https://doi.org/10.3390/w16071058
Chicago/Turabian StyleBorgert, Aline Eloize, and Enedir Ghisi. 2024. "The Impact of the Water Tariff on the Economic Feasibility of Rainwater Harvesting for Use in Residential Buildings" Water 16, no. 7: 1058. https://doi.org/10.3390/w16071058
APA StyleBorgert, A. E., & Ghisi, E. (2024). The Impact of the Water Tariff on the Economic Feasibility of Rainwater Harvesting for Use in Residential Buildings. Water, 16(7), 1058. https://doi.org/10.3390/w16071058








