A Connectivity Metrics-Based Approach for the Prediction of Stress-Dependent Fracture Permeability
Abstract
1. Introduction
2. Materials and Methods
2.1. Fracture Aperture Generation
2.2. Fluid Flow along Fractures
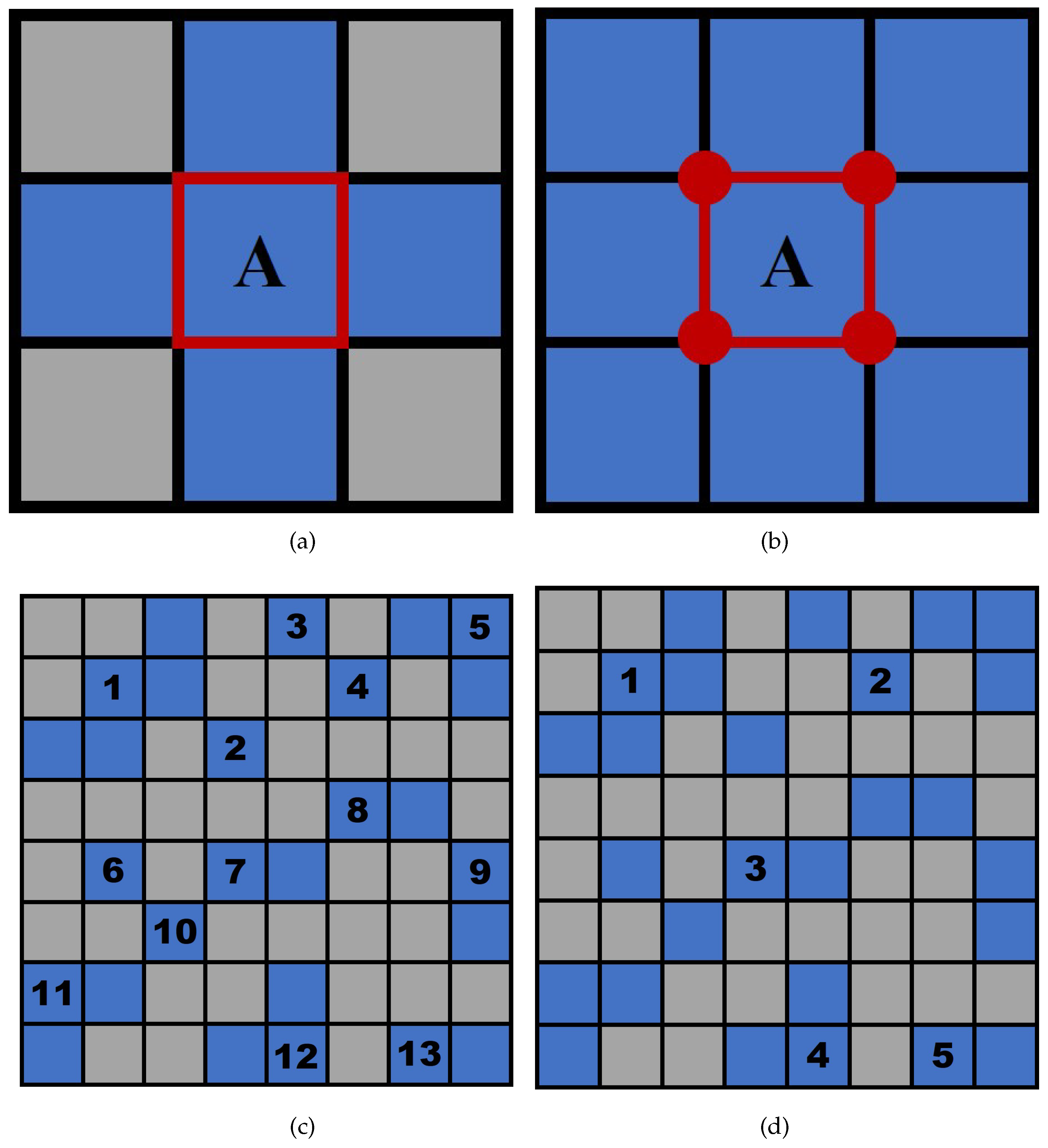
2.3. Percolation Theory and Connectivity Metrics
3. Results and Discussions
3.1. Fracture Permeability under Normal Stress from Numerical Simulations
3.2. Percolation Threshold of the Fracture Aperture Field
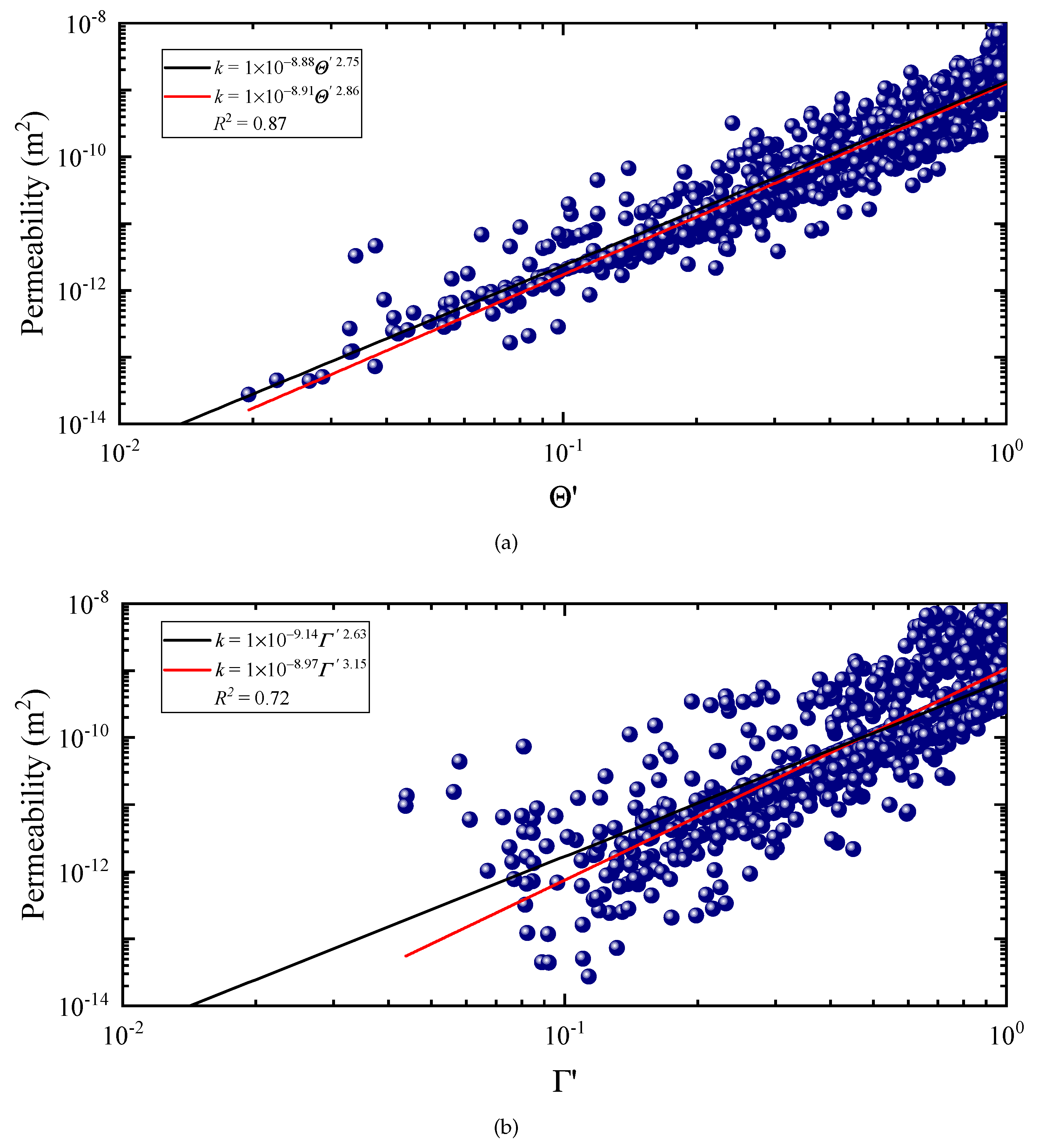
3.3. Fracture Permeability and Connectivity Metrics
4. Conclusions
- (1)
- With the increase in normal loading, the fracture permeability deviates from the cubic law to an increasing degree. For the generated fracture dataset in this study, common fracture permeability prediction models based on fracture geometrical parameters are established by laboratory experiments and numerical simulations with an accuracy of 75% to 88%;
- (2)
- The flow percolation threshold is affected by the fractal dimension of the fracture and the stress variations, independent of the mismatch length, and the connectivity metrics of the fracture aperture field based on the percolation theory can be quickly estimated, which is related to the set cutoff aperture threshold;
- (3)
- By fitting a power law model to permeability–connectivity metrics, similar permeability prediction results and accuracy to the previous study are obtained. Using the improved connectivity metrics, the permeability prediction accuracy is improved by 8 to 15 percentage points for different cutoff aperture thresholds, with a maximum fitting accuracy of 0.87. In particular, for the connectivity metric , the accuracy remains almost over 80%, comparable with common previous fracture permeability prediction models; however, the computation of our approach can be easily achieved, showing an advantage over previous methods.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| LCL | local cubic law |
| FFT | fast Fourier transform |
| BEM | boundary element method |
| NS | Navier–Stokes |
| FEM | finite element method |
| JRC | joint roughness coefficient |
References
- Pariseau, W.; Schmelter, S.; Sheik, A. Mine slope stability analysis by coupled finite element modelling. Int. J. Rock Mech. Min. Sci. 1997, 34, 242.e1–242.e17. [Google Scholar] [CrossRef]
- Xu, G.; Luxbacher, K.D.; Ragab, S.; Xu, J.; Ding, X. Computational fluid dynamics applied to mining engineering: A review. Int. J. Min. Reclam. Environ. 2017, 31, 251–275. [Google Scholar] [CrossRef]
- Yang, T.; Liu, J.; Zhu, W.; Elsworth, D.; Tham, L.; Tang, C. A coupled flow-stress-damage model for groundwater outbursts from an underlying aquifer into mining excavations. Int. J. Rock Mech. Min. Sci. 2007, 44, 87–97. [Google Scholar] [CrossRef]
- Blöcher, G.; Reinsch, T.; Henninges, J.; Milsch, H.; Regenspurg, S.; Kummerow, J.; Francke, H.; Kranz, S.; Saadat, A.; Zimmermann, G.; et al. Hydraulic history and current state of the deep geothermal reservoir Groß Schönebeck. Geothermics 2016, 63, 27–43. [Google Scholar] [CrossRef]
- Zamehrian, M.; Sedaee, B. Underground hydrogen storage in a naturally fractured gas reservoir: The role of fracture. Int. J. Hydrogen Energy 2022, 47, 39606–39618. [Google Scholar] [CrossRef]
- Guan, Y.; Zhou, Z.; Ge, Z.; Deng, Q.; Jia, Y.; Huang, S.; Chen, C.; Gong, S. Effect of ScCO2-H2O treatment duration on the microscopic structure of coal reservoirs: Implications for CO2 geological sequestration in coal. Int. J. Coal Geol. 2024, 282, 104439. [Google Scholar] [CrossRef]
- Zimmerman, R.W.; Chen, D.W.; Cook, N.G. The effect of contact area on the permeability of fractures. J. Hydrol. 1992, 139, 79–96. [Google Scholar] [CrossRef]
- Zimmerman, R.W.; Bodvarsson, G.S. Hydraulic conductivity of rock fractures. Transp. Porous Media 1996, 23, 1–30. [Google Scholar] [CrossRef]
- Pyrak-Nolte, L.; Morris, J. Single fractures under normal stress: The relation between fracture specific stiffness and fluid flow. Int. J. Rock Mech. Min. Sci. 2000, 37, 245–262. [Google Scholar] [CrossRef]
- Wang, L.; Cardenas, M.B. Development of an empirical model relating permeability and specific stiffness for rough fractures from numerical deformation experiments. J. Geophys. Res. Solid Earth 2016, 121, 4977–4989. [Google Scholar] [CrossRef]
- Li, B.; Cui, X.; Zou, L.; Cvetkovic, V. On the relationship between normal stiffness and permeability of rock fractures. Geophys. Res. Lett. 2021, 48, e2021GL095593. [Google Scholar] [CrossRef]
- Zimmerman, R.; Kumar, S.; Bodvarsson, G. Lubrication theory analysis of the permeability of rough-walled fractures. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1991, 28, 325–331. [Google Scholar]
- Méheust, Y.; Schmittbuhl, J. Geometrical heterogeneities and permeability anisotropy of rough fractures. J. Geophys. Res. Solid Earth 2001, 106, 2089–2102. [Google Scholar] [CrossRef]
- Petrovitch, C.; Pyrak-Nolte, L.; Nolte, D. Combined scaling of fluid flow and seismic stiffness in single fractures. Rock Mech. Rock Eng. 2014, 47, 1613–1623. [Google Scholar] [CrossRef]
- Deng, Q.; Blöcher, G.; Cacace, M.; Schmittbuhl, J. Hydraulic Diffusivity of a Partially Open Rough Fracture. Rock Mech. Rock Eng. 2021, 54, 5493–5515. [Google Scholar] [CrossRef]
- Javanmard, H.; Saar, M.O.; Vogler, D. On the applicability of connectivity metrics to rough fractures under normal stress. Adv. Water Resour. 2022, 161, 104122. [Google Scholar] [CrossRef]
- Bour, O.; Davy, P. On the connectivity of three-dimensional fault networks. Water Resour. Res. 1998, 34, 2611–2622. [Google Scholar] [CrossRef]
- Sadeghnejad, S.; Masihi, M.; Pishvaie, M.; Shojaei, A.; King, P. Utilization of percolation approach to evaluate reservoir connectivity and effective permeability: A case study on North Pars gas field. Sci. Iran. 2011, 18, 1391–1396. [Google Scholar] [CrossRef][Green Version]
- Schmittbuhl, J.; Vilotte, J.P.; Roux, S. Percolation through self-affine surfaces. J. Phys. A Math. Gen. 1993, 26, 6115. [Google Scholar] [CrossRef]
- Mourzenko, V.; Thovert, J.F.; Adler, P. Geometry of simulated fractures. Phys. Rev. E 1996, 53, 5606. [Google Scholar] [CrossRef]
- Mourzenko, V.V.; Thovert, J.F.; Adler, P.M. Permeability of self-affine fractures. Transp. Porous Media 2001, 45, 89–103. [Google Scholar] [CrossRef]
- Harter, T. Finite-size scaling analysis of percolation in three-dimensional correlated binary Markov chain random fields. Phys. Rev. E 2005, 72, 026120. [Google Scholar] [CrossRef]
- Knusby, C.; Carrera, J.; Bumgardner, J.D.; Fogg, G.E. Binary upscaling—The role of connectivity and a new formula. Adv. Water Resour. 2006, 29, 590–604. [Google Scholar]
- Hovadik, J.M.; Larue, D.K. Static characterizations of reservoirs: Refining the concepts of connectivity and continuity. Pet. Geosci. 2007, 13, 195–211. [Google Scholar] [CrossRef]
- Tyukhova, A.R.; Willmann, M. Connectivity metrics based on the path of smallest resistance. Adv. Water Resour. 2016, 88, 14–20. [Google Scholar] [CrossRef]
- Marrink, S.; Paterson, L.; Knackstedt, M.A. Definition of percolation thresholds on self-affine surfaces. Phys. A Stat. Mech. Its Appl. 2000, 280, 207–214. [Google Scholar] [CrossRef][Green Version]
- Renard, P.; Allard, D. Connectivity metrics for subsurface flow and transport. Adv. Water Resour. 2013, 51, 168–196. [Google Scholar] [CrossRef]
- Ogilvie, S.R.; Isakov, E.; Glover, P.W. Fluid flow through rough fractures in rocks. II: A new matching model for rough rock fractures. Earth Planet. Sci. Lett. 2006, 241, 454–465. [Google Scholar] [CrossRef]
- Phillips, T.; Bultreys, T.; Bisdom, K.; Kampman, N.; Offenwert, S.V.; Mascini, A.; Cnudde, V.; Busch, A. A Systematic Investigation Into the Control of Roughness on the Flow Properties of 3D-Printed Fractures. Water Resour. Res. 2021, 57, ewrcr-25233. [Google Scholar] [CrossRef]
- Candela, T.; Renard, F.; Klinger, Y.; Mair, K.; Schmittbuhl, J.; Brodsky, E.E. Roughness of fault surfaces over nine decades of length scales. J. Geophys. Res. Solid Earth 2012, 117, B08409. [Google Scholar] [CrossRef]
- Schmittbuhl, J.; Gentier, S.; Roux, S. Field measurements of the roughness of fault surfaces. Geophys. Res. Lett. 1993, 20, 639–641. [Google Scholar] [CrossRef]
- Schmittbuhl, J.; Vilotte, J.P.; Roux, S. Reliability of self-affine measurements. Phys. Rev. E 1995, 51, 131. [Google Scholar] [CrossRef] [PubMed]
- Candela, T.; Renard, F.; Bouchon, M.; Brouste, A.; Marsan, D.; Schmittbuhl, J.; Voisin, C. Characterization of fault roughness at various scales: Implications of three-dimensional high resolution topography measurements. Pure Appl. Geophys. 2009, 166, 1817–1851. [Google Scholar] [CrossRef]
- Renard, F.; Voisin, C.; Marsan, D.; Schmittbuhl, J. High resolution 3D laser scanner measurements of a strike-slip fault quantify its morphological anisotropy at all scales. Geophys. Res. Lett. 2006, 33. [Google Scholar] [CrossRef]
- Briggs, S.; Karney, B.W.; Sleep, B.E. Numerical modelling of flow and transport in rough fractures. J. Rock Mech. Geotech. Eng. 2014, 6, 535–545. [Google Scholar] [CrossRef]
- Press, W.; Teukolsky, S.; Vetterling, W.; Flannery, B. Numerical Recipes in C; Cambridge University Press: Cambridge, UK, 1992. [Google Scholar]
- Brown, S.R.; Scholz, C.H. Broad bandwidth study of the topography of natural rock surfaces. J. Geophys. Res. Solid Earth 1985, 90, 12575–12582. [Google Scholar] [CrossRef]
- Bouchaud, E. Scaling properties of cracks. J. Phys. Condens. Matter 1997, 9, 4319. [Google Scholar] [CrossRef]
- Keller, A. High resolution, non-destructive measurement and characterization of fracture apertures. Int. J. Rock Mech. Min. Sci. 1998, 35, 1037–1050. [Google Scholar] [CrossRef]
- Méheust, Y.; Schmittbuhl, J. Scale effects related to flow in rough fractures. Pure Appl. Geophys. 2003, 160, 1023–1050. [Google Scholar] [CrossRef]
- Brown, S.R. Simple mathematical model of a rough fracture. J. Geophys. Res. Solid Earth 1995, 100, 5941–5952. [Google Scholar] [CrossRef]
- Plouraboué, F.; Kurowski, P.; Hulin, J.P.; Roux, S.; Schmittbuhl, J. Aperture of rough cracks. Phys. Rev. E 1995, 51, 1675. [Google Scholar] [CrossRef]
- Hopkins, D.; Myer, L.; Cook, N. Fracture Stiffness and Aperture as a Function of Applied Stress and Contact Geometry. 1987. Available online: https://escholarship.org/uc/item/6ht8f5w9 (accessed on 4 January 2024).
- Röttger, M.C.; Sanner, A.; Thimons, L.A.; Junge, T.; Gujrati, A.; Monti, J.M.; Nöhring, W.G.; Jacobs, T.D.; Pastewka, L. Contact. engineering–Create, analyze and publish digital surface twins from topography measurements across many scales. arXiv 2022, arXiv:2203.13606. [Google Scholar]
- Kling, T.; Vogler, D.; Pastewka, L.; Amann, F.; Blum, P. Numerical simulations and validation of contact mechanics in a granodiorite fracture. Rock Mech. Rock Eng. 2018, 51, 2805–2824. [Google Scholar] [CrossRef]
- Zou, L.; Cvetkovic, V. Impact of normal stress-induced closure on laboratory-scale solute transport in a natural rock fracture. J. Rock Mech. Geotech. Eng. 2020, 12, 732–741. [Google Scholar] [CrossRef]
- Jaeger, J.C.; Cook, N.G.; Zimmerman, R. Fundamentals of Rock Mechanics; John Wiley & Sons: Hoboken, NJ, USA, 2009. [Google Scholar]
- Jacquey, A.B.; Cacace, M.; Blöcher, G. Modelling coupled fluid flow and heat transfer in fractured reservoirs: Description of a 3D benchmark numerical case. Energy Procedia 2017, 125, 612–621. [Google Scholar] [CrossRef]
- Watanabe, N.; Hirano, N.; Tsuchiya, N. Determination of aperture structure and fluid flow in a rock fracture by high-resolution numerical modeling on the basis of a flow-through experiment under confining pressure. Water Resour. Res. 2008, 44, W06412. [Google Scholar] [CrossRef]
- Brown, S.R. Fluid Flow Through Rock Joints: The Effect of Surface Roughnes. J. Geophys. Res. Solid Earth 1987, 92, 1337–1347. [Google Scholar] [CrossRef]
- Allen, H.; Ewing, R.; Ghanbarian, B. Percolation Theory for Flow in Porous Media; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Sisavath, S.; Al-Yaarubi, A.; Pain, C.C.; Zimmerman, R.W. A simple model for deviations from the cubic law for a fracture undergoing dilation or closure. Pure Appl. Geophys. 2003, 160, 1009–1022. [Google Scholar] [CrossRef]
- Xiong, X.; Li, B.; Jiang, Y.; Koyama, T.; Zhang, C. Experimental and numerical study of the geometrical and hydraulic characteristics of a single rock fracture during shear. Int. J. Rock Mech. Min. Sci. 2011, 48, 1292–1302. [Google Scholar] [CrossRef]
- Javanmard, H.; Ebigbo, A.; Walsh, S.D.; Saar, M.O.; Vogler, D. No-Flow Fraction (NFF) permeability model for rough fractures under normal stress. Water Resour. Res. 2021, 57, e2020WR029080. [Google Scholar] [CrossRef]
- Walsh, J. Effect of pore pressure and confining pressure on fracture permeability. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1981, 18, 429–435. [Google Scholar]
- Barton, N.; Bandis, S.; Bakhtar, K. Strength, deformation and conductivity coupling of rock joints. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1985, 22, 121–140. [Google Scholar] [CrossRef]
- Witherspoon, P.A.; Wang, J.S.; Iwai, K.; Gale, J.E. Validity of cubic law for fluid flow in a deformable rock fracture. Water Resour. Res. 1980, 16, 1016–1024. [Google Scholar] [CrossRef]
- Mourzenko, V.; Thovert, J.F.; Adler, P. Conductivity and transmissivity of a single fracture. Transp. Porous Media 2018, 123, 235–256. [Google Scholar] [CrossRef]
- Watanabe, N.; Hirano, N.; Tamagawa, T.; Tezuka, K.; Tsuchiya, N. Numerical estimation of aperture structure and flow wetted field in rock fracture. Trans.-Geotherm. Resour. Counc. 2005, 29, 431–436. [Google Scholar]
- Blöcher, G.; Kluge, C.; Milsch, H.; Cacace, M.; Jacquey, A.B.; Schmittbuhl, J. Permeability of matrix-fracture systems under mechanical loading–constraints from laboratory experiments and 3-D numerical modelling. Adv. Geosci. 2019, 49, 95–104. [Google Scholar] [CrossRef]
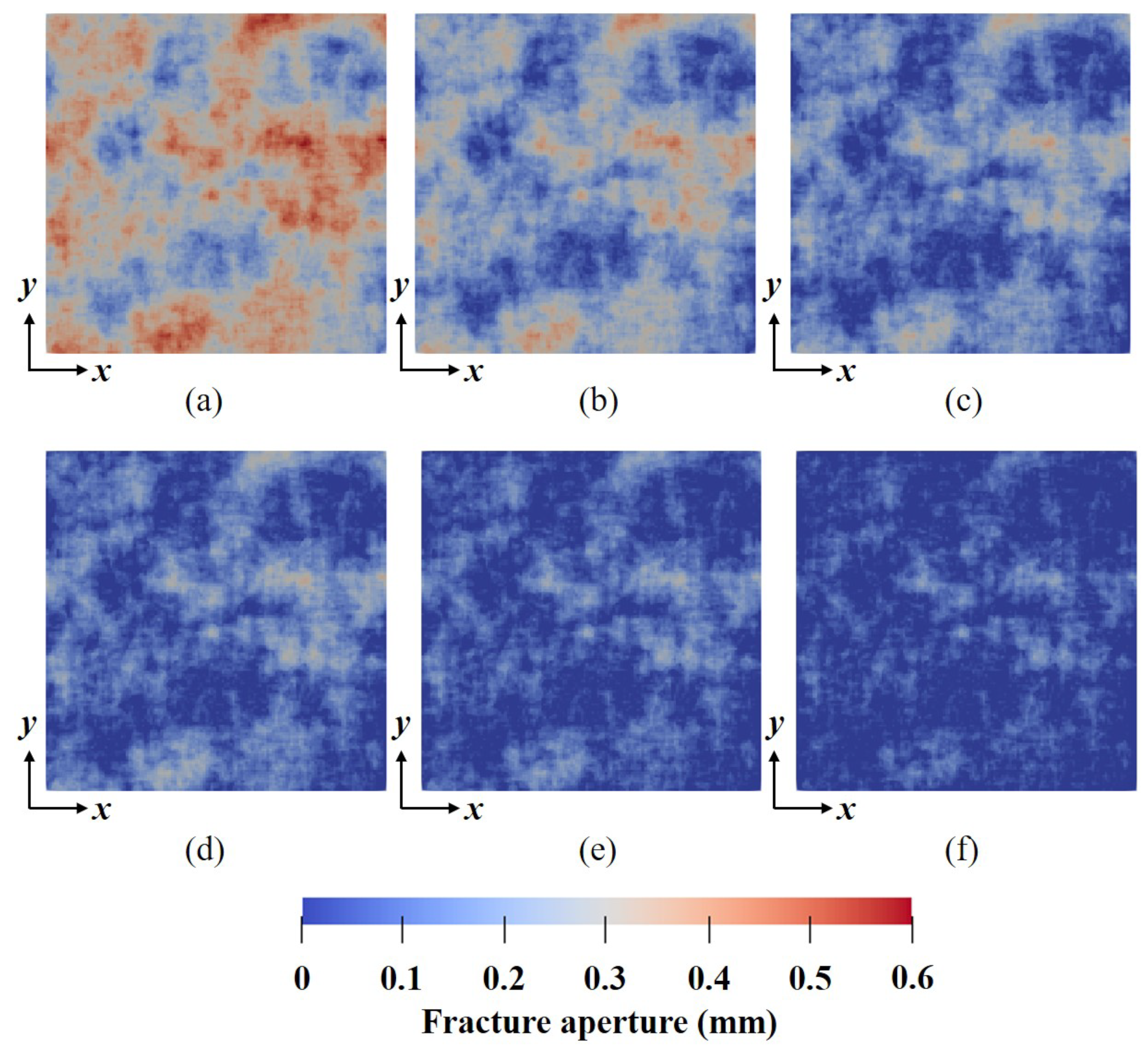



| Data | (μm) | ||||||
|---|---|---|---|---|---|---|---|
| a | b | a | b | ||||
| D1 D2 D3 | 0 | 25.47 26.21 27.15 | 0.69 0.71 0.79 | 220.53 210.57 206.88 | 0.45 0.50 0.62 | ||
| D1 D2 D3 | 10 | 7.37 6.71 7.22 | 0.72 0.74 0.82 | 8.32 8.09 8.07 | 0.36 0.51 0.60 | ||
| D1 D2 D3 | 20 | 3.70 3.55 3.63 | 0.70 0.75 0.84 | 3.69 3.76 3.89 | 0.48 0.55 0.67 | ||
| D1 D2 D3 | 30 | 2.55 2.75 2.86 | 0.72 0.77 0.87 | 2.65 2.63 3.15 | 0.55 0.58 0.72 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Deng, Q.; Shang, X.; He, P. A Connectivity Metrics-Based Approach for the Prediction of Stress-Dependent Fracture Permeability. Water 2024, 16, 697. https://doi.org/10.3390/w16050697
Deng Q, Shang X, He P. A Connectivity Metrics-Based Approach for the Prediction of Stress-Dependent Fracture Permeability. Water. 2024; 16(5):697. https://doi.org/10.3390/w16050697
Chicago/Turabian StyleDeng, Qinglin, Xueyi Shang, and Ping He. 2024. "A Connectivity Metrics-Based Approach for the Prediction of Stress-Dependent Fracture Permeability" Water 16, no. 5: 697. https://doi.org/10.3390/w16050697
APA StyleDeng, Q., Shang, X., & He, P. (2024). A Connectivity Metrics-Based Approach for the Prediction of Stress-Dependent Fracture Permeability. Water, 16(5), 697. https://doi.org/10.3390/w16050697








