The Correlation between Water–Sediment Index and Floodplain Transverse Slope Based on Wavelet Analysis
Abstract
1. Introduction
2. Materials and Methods
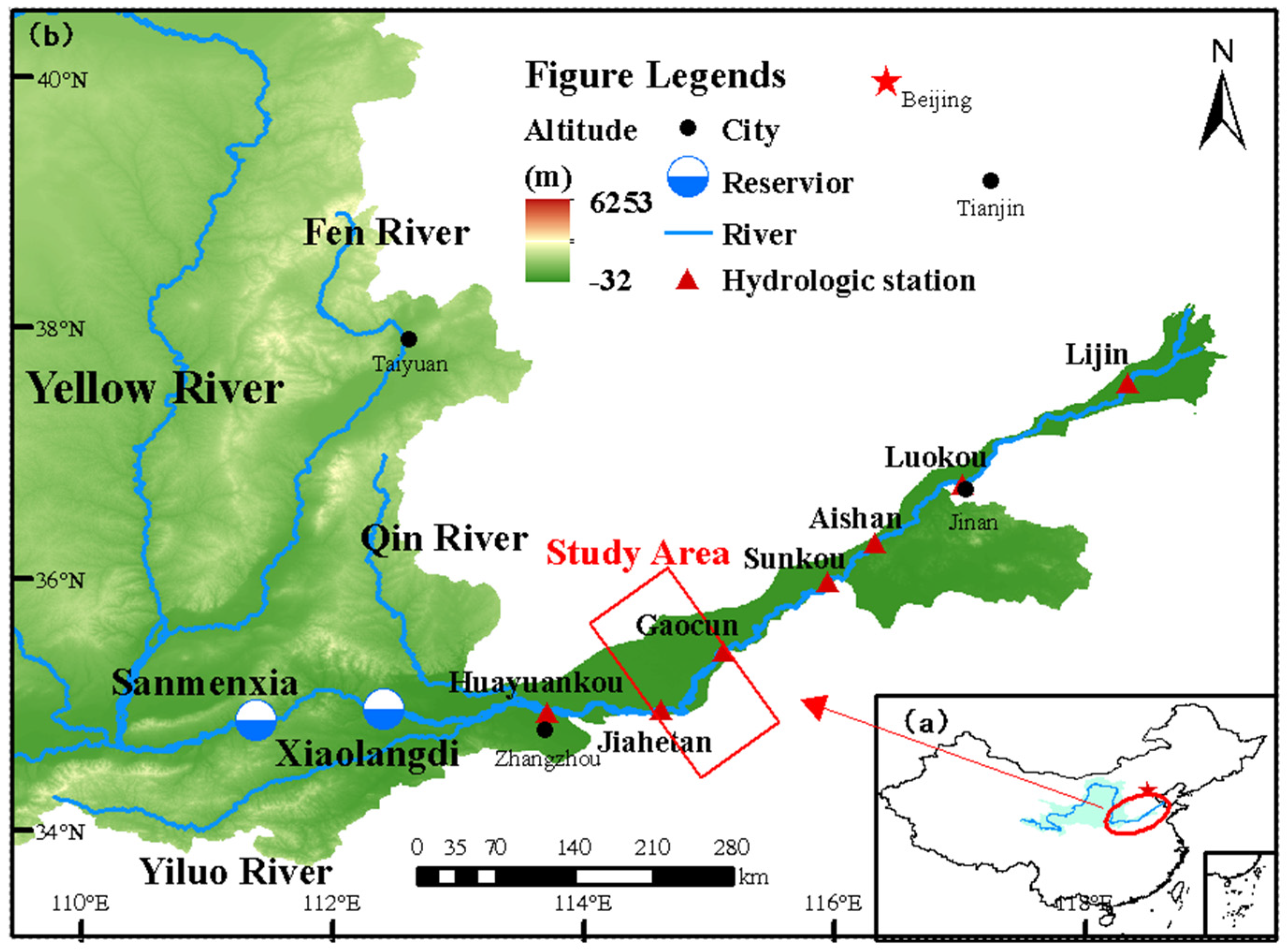
2.1. Study Area
2.2. Study Methods
2.2.1. Data Sources
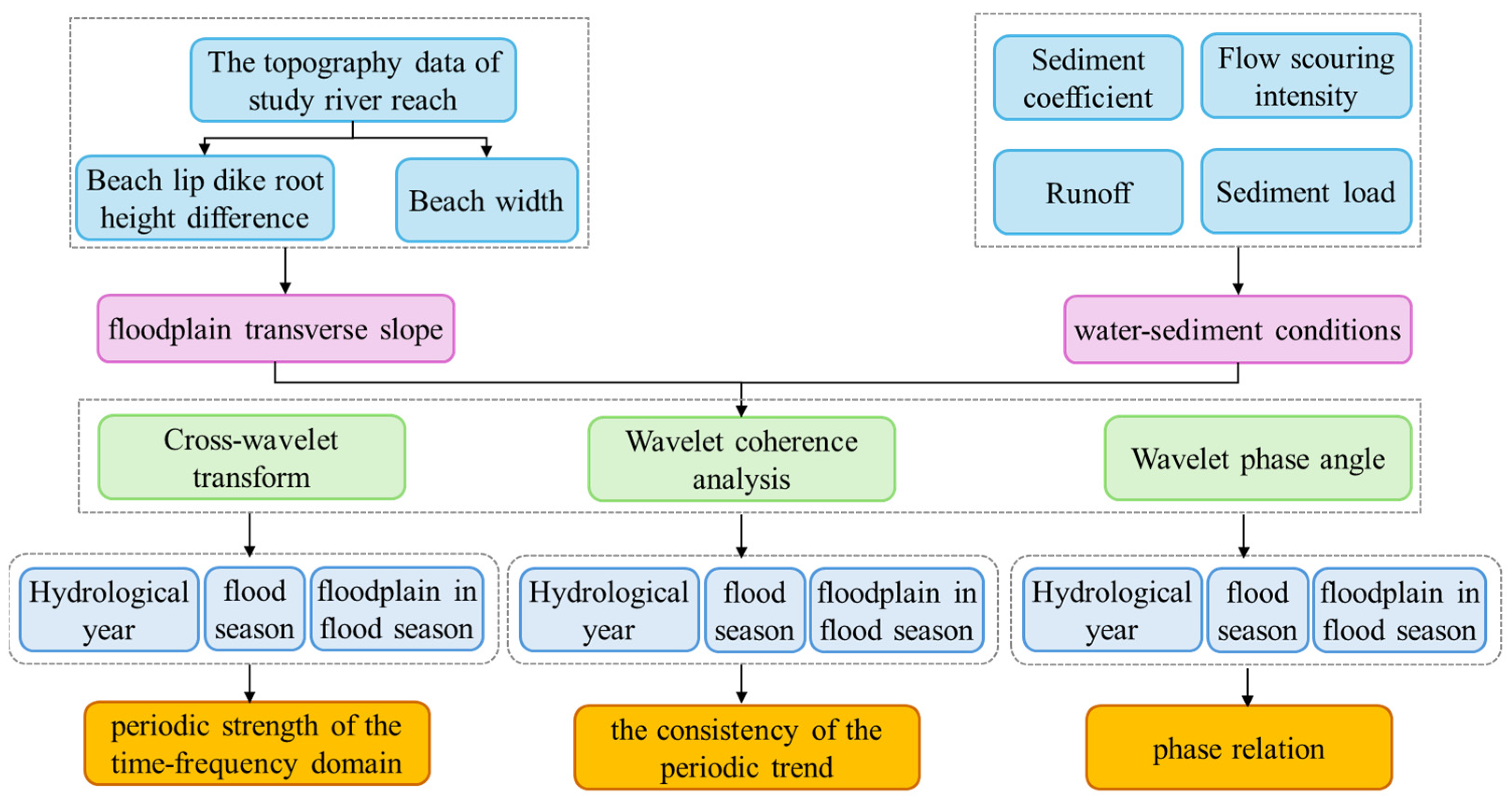
2.2.2. Data Processing Methods
- (1)
- Water–sediment conditions
- (2)
- Floodplain transverse slope [13]
- (3)
- Cross-wavelet transform (XWT)
- (4)
- Wavelet coherence analysis (WTC)
- (5)
- Wavelet phase angle
3. Results
3.1. Evolution Characteristics of Transverse Slope in Dongbatou–Gaocun River Section
3.2. Correlation Analysis of Different Water and Sediment Indexes and Transverse Slope
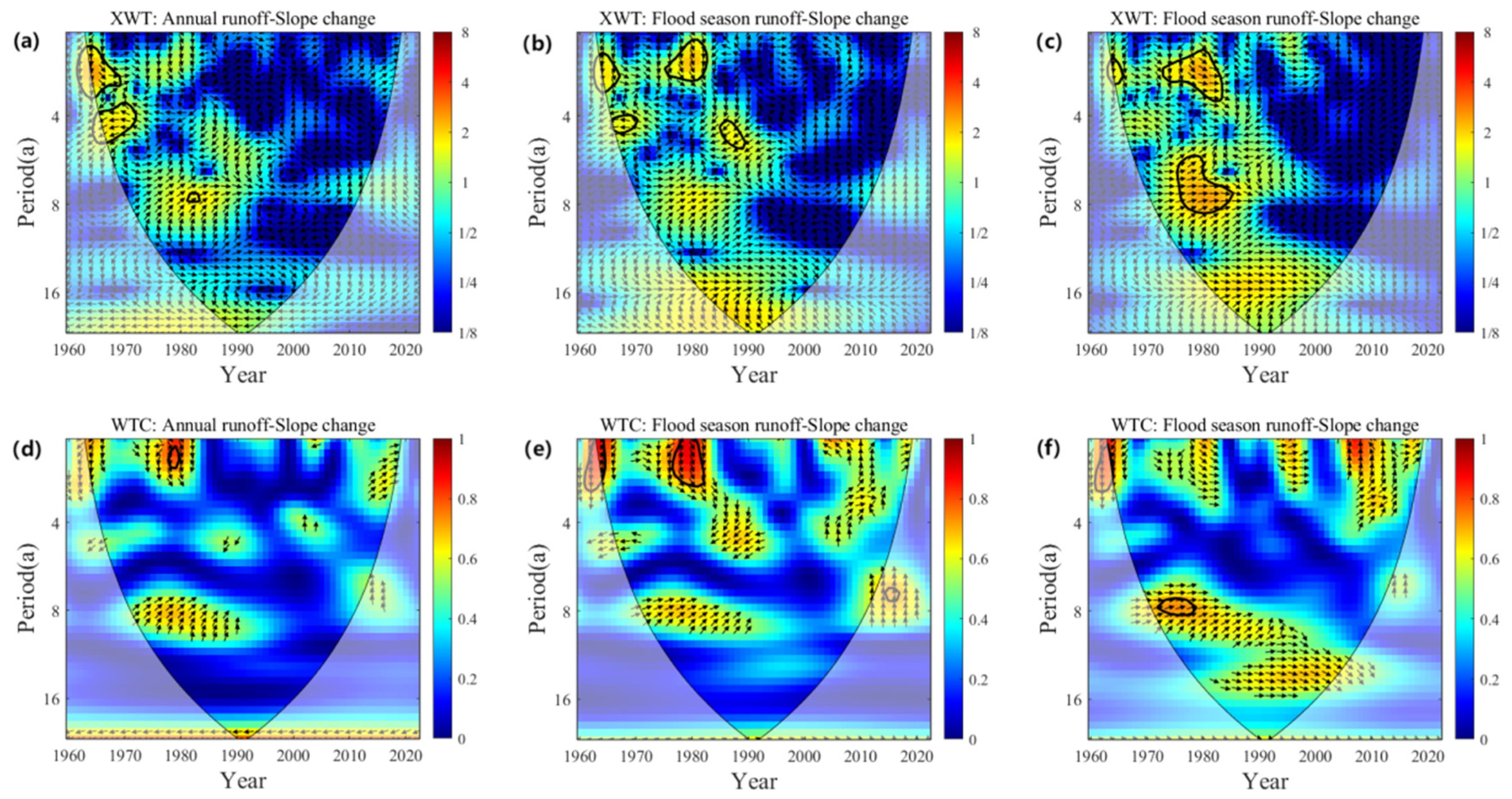
3.2.1. Correlation between Runoff and Changes in Transverse Slope
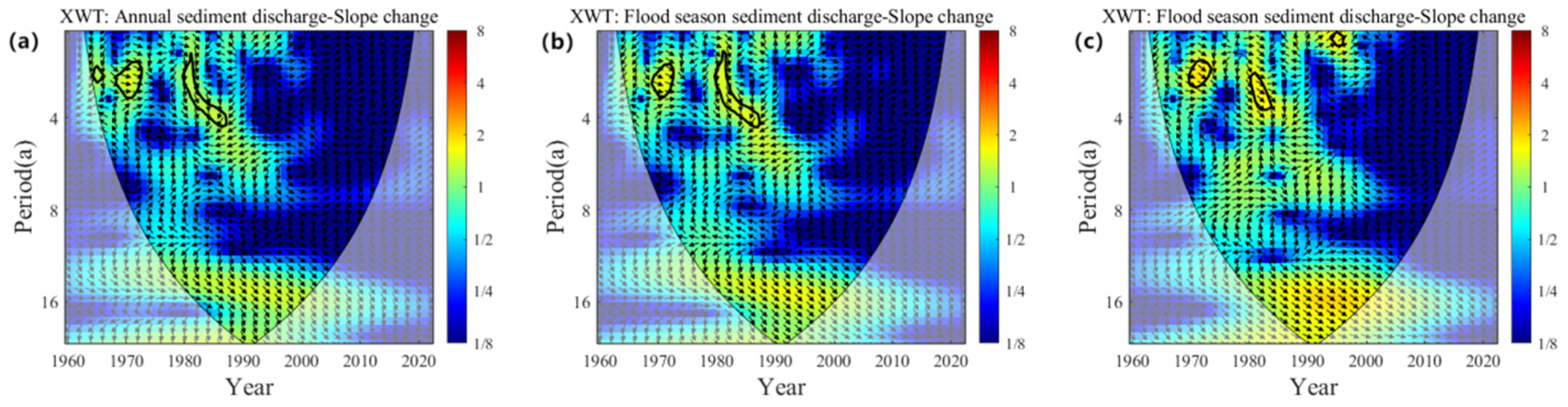
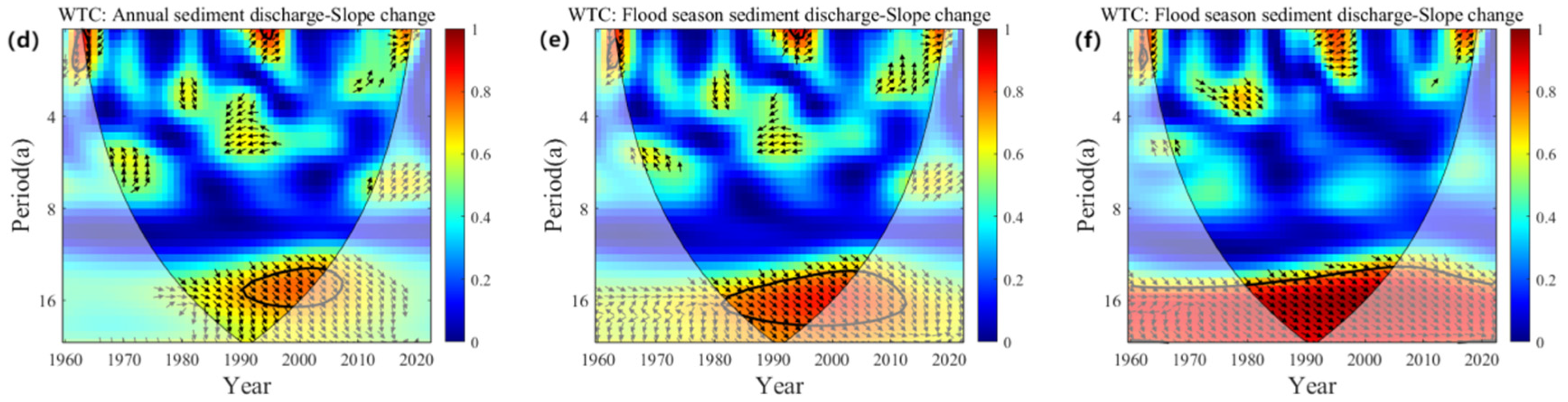
3.2.2. Correlation between Sediment Load and Changes in Transverse Slope
3.2.3. Correlation between Sediment Coefficient and Changes in Transverse Slope
3.2.4. Correlation between Flow Scouring Intensity and Changes in Transverse Slope
4. Discussion
5. Conclusions
- (1)
- From 1960 to 2022, the floodplain transverse slope in the Dongbatou–Gaocun section of the lower Yellow River generally showed an upward trend, particularly before 2000, the increase in the transverse slope was more significant. After 2000, the floodplain transverse slope exhibited relatively stable fluctuations. The amplitude of changes in the transverse slope varied in different periods.
- (2)
- Through the analysis of wavelet cross-transform and wavelet coherence spectrum of the time series of changes in transverse slope and the conditions of incoming water and sediment, both annual sediment load and annual sediment coefficient have a high correlation with the evolution cycle of the transverse slope, followed by the flow scouring intensity during the flood season. However, the correlation between annual runoff and the evolution cycle of the transverse slope is relatively small, indicating that compared to water flow conditions, the change in sediment load has a greater impact on the secondary suspended river. Through phase correlation analysis, it can be observed that there is a positive correlation between changes in transverse slope and water–sediment conditions, and the change in transverse slope lags behind the variation in incoming water and sediment conditions, which is consistent with the hysteresis of riverbed evolution.
- (3)
- Through a comparative analysis of the evolution of transverse slope under different modes, the change in transverse slope has a good correlation with the floodplain overflow mode during the flood season, indicating that the water–sediment characteristics of floodplain floods have a high similarity with the evolution cycle of the transverse slope; that is, the change in transverse slope is significantly influenced by individual floodplain floods.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhang, J.; Liu, J.; Lu, J.; Luo, Q. Theories and practices of sediment engineering control in the Yellow River. J. Sediment Res. 2023, 48, 1–8. [Google Scholar] [CrossRef]
- Hu, Y. Perched River Discussion. J. North China Univ. Water Resour. Electr. Power (Nat. Sci. Ed.) 2001, 3, 12–18. [Google Scholar] [CrossRef]
- Wang, K.; Wang, C.; Du, X.; Wang, G.; Zhang, J. Practice and evaluation of sediment control in the Yellow River Estuary. J. Sediment Res. 2024, 22, 12–18. [Google Scholar] [CrossRef]
- Zhang, C.; Wang, G. Thoughts on Ecological Protection and High-Quality Development in the Yellow River Basin. Yellow River 2024, 46, 271–277. [Google Scholar]
- Jing, L.; Wan, Z.; Chen, C. Significance of extending the service life of Xiaolangdi reservoir insediment retention for Yellow River flood control and security. Yellow River 2024, 46, 1–4+24. [Google Scholar] [CrossRef]
- Zhang, R. Suggestions on a General Plan for Lower Yellow River Management. Yellow River 2004, 26, 1–4. [Google Scholar] [CrossRef]
- Pang, J. Pondering over Issues of a General Plan of the Lower Yellow River Harnessing. Yellow River 2005, 27, 3–4. [Google Scholar] [CrossRef]
- Jiang, E.; Han, Q.; Li, J.; Cao, Y. Outline of Typical Phenomena of Bed Development of the Yellow River and Its Related Study. Yellow River 2010, 8, 5–7. [Google Scholar]
- Liu, X. The Water and Sediment Situation and Countermeasure. Yellow River 2020, 42, 34–40. [Google Scholar] [CrossRef]
- Zhang, C.; Zhang, M.; Yao, W.; Li, Y.; Ma, D. Mainstream swing rule and its driving mechanism in the Sanguanmiao to Weitan reach of the lower Yellow River. J. Basic Sci. Eng. Mech. 2023, 31, 1110–1124. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, Q.; Liu, Y.; Liu, G.; Jiang, E. Morphological effects of the operation of Xiaolangdi Reservoir on the Lower Yellow River in recent years. J. Hydraul. Eng. 2024, 55, 505–515. [Google Scholar] [CrossRef]
- Jiang, E.; Cao, Y.; Zhang, L. Study on the Evolution Law and Mechanism of River Regime in the Wandering Reach of the Lower Yellow River; China Water & Power Press: Beijing, China, 2006. [Google Scholar]
- Xu, L.; Li, J.; Xu, H.; Zhang, X.; Lai, R.; Zhang, X.; Gao, X. Evolution and drivers of secondary suspended rivers in typical wandering sections of the lower Yellow River from 1960–2021. Front. Ecol. Evol. 2023, 11, 1330749. [Google Scholar] [CrossRef]
- Wang, Y.; Xia, J.; Zhou, M.; Li, J. Characteristics of main channel migration in the braided reach of the Lower Yellow River after the Xiaolangdi Reservoir operation. Adv. Water Sci. 2019, 2, 198–209. [Google Scholar] [CrossRef]
- Zheng, H.; Wang, Y. Evolution characteristics of runoff and sediment fluxes of Yellow River, Yangtze River and Pearl River into sea during 1960-2020. Bull. Soil Water Conserv. 2024, 44, 147–157. [Google Scholar] [CrossRef]
- Zhang, B.; Wang, Z. Trend and multi-time scale cross-wavelet analysis on precipitation and temperature in Tianjin area. J. Tianjin Norm. Univ. (Nat. Sci. Ed.) 2016, 1, 32–39. [Google Scholar]
- Thompson, D.; Wallace, J. The Arctic oscillation signature in the wintertime geopotential height and temperature fields. Geophys. Res. Lett. 1998, 25, 1297–1300. [Google Scholar] [CrossRef]
- Firouzi, S.; Wang, X. A comparative study of exchange rates and order flow based on wavelet transform coherence and cross wavelet transform. Econ. Model. 2019, 82, 42–56. [Google Scholar] [CrossRef]
- Yellow River Conservancy Commission. Causes and Control Measures of the “Secondary Suspended River” in the Lower Yellow River; The Yellow River Water Conservancy Press: Zhengzhou, China, 2003. [Google Scholar]
- Xie, F.; Zhao, X.; Mu, X.; Gao, P.; Sun, W. The change of water-sediment relationship in the main stream of the Yellow River in the past 70 years. Sci. Soil Water Conserv. 2021, 19, 1–9. [Google Scholar] [CrossRef]
- Yu, H.; Zhang, Y.; Ma, J.; Guo, J.; Chen, P. Variation of Observed and Natural Runoff of Yellow River from 1969 to 2018. Bull. Soil Water Conserv. 2020, 40, 1–7. [Google Scholar] [CrossRef]
- Zhang, Y.; Kang, J.; Liu, C. Data on Annual and Interannual Changes in Runoff in the Yellow River Basin from 2015 to 2019; National Cryosphere Desert Data Center: Lanzhou, China, 2021; Available online: http://www.ncdc.ac.cn (accessed on 16 June 2024). [CrossRef]
- Hu, C.; Zhang, X.; Zhao, Y. Cause analysis of the centennial trend and recent fluctuation of the Yellow River sediment load. Adv. Water Sci. 2020, 31, 725–733. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, L.; Xu, H.; Yan, J.; Li, J.; Kou, Z.; Gao, X. The Correlation between Water–Sediment Index and Floodplain Transverse Slope Based on Wavelet Analysis. Water 2024, 16, 2418. https://doi.org/10.3390/w16172418
Xu L, Xu H, Yan J, Li J, Kou Z, Gao X. The Correlation between Water–Sediment Index and Floodplain Transverse Slope Based on Wavelet Analysis. Water. 2024; 16(17):2418. https://doi.org/10.3390/w16172418
Chicago/Turabian StyleXu, Linjuan, Haifan Xu, Jun Yan, Junhua Li, Zhao Kou, and Xiangyu Gao. 2024. "The Correlation between Water–Sediment Index and Floodplain Transverse Slope Based on Wavelet Analysis" Water 16, no. 17: 2418. https://doi.org/10.3390/w16172418
APA StyleXu, L., Xu, H., Yan, J., Li, J., Kou, Z., & Gao, X. (2024). The Correlation between Water–Sediment Index and Floodplain Transverse Slope Based on Wavelet Analysis. Water, 16(17), 2418. https://doi.org/10.3390/w16172418





