Simulation of Flow and Pressure Loss in the Example of the Elbow
Abstract
1. Introduction
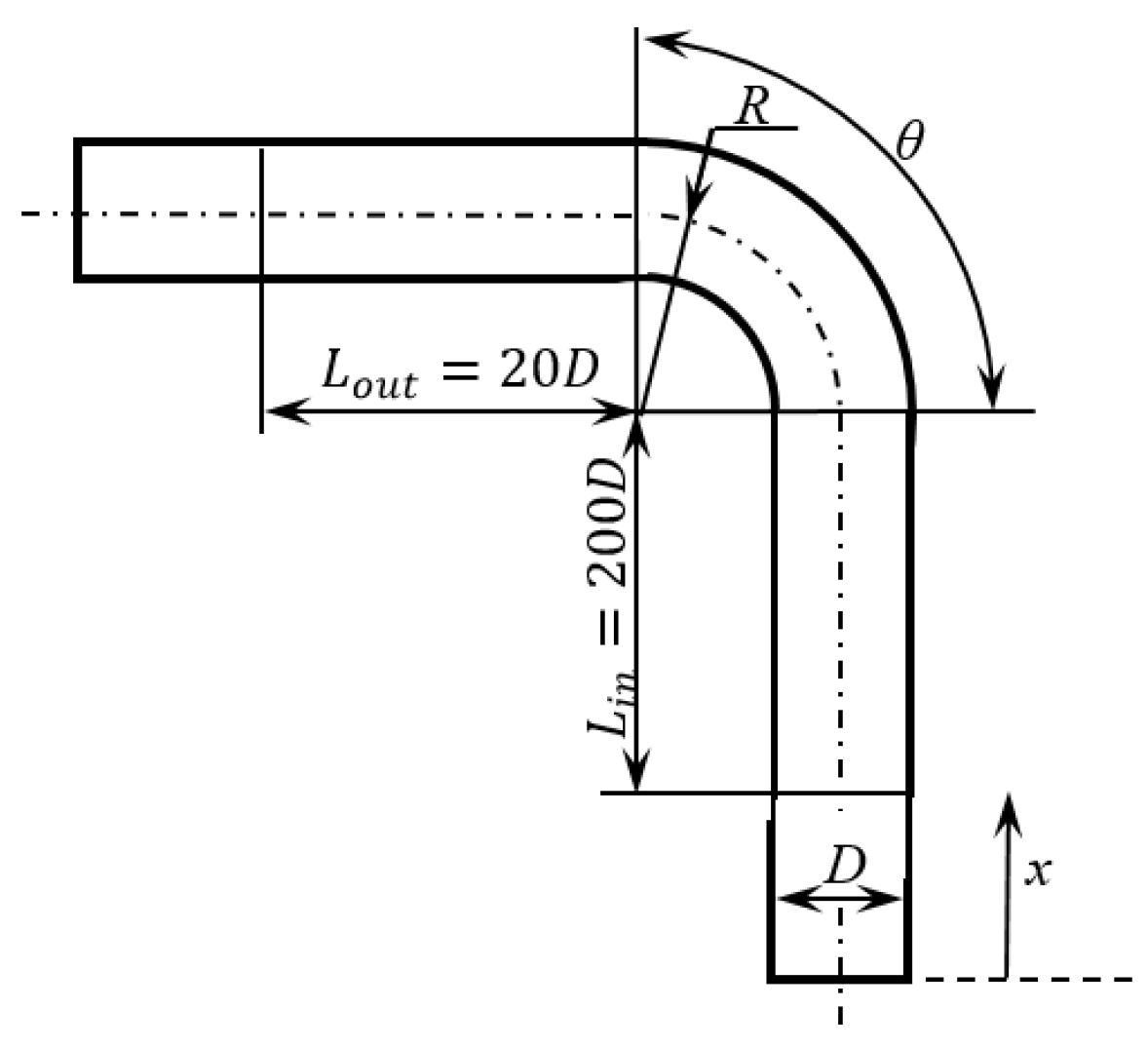
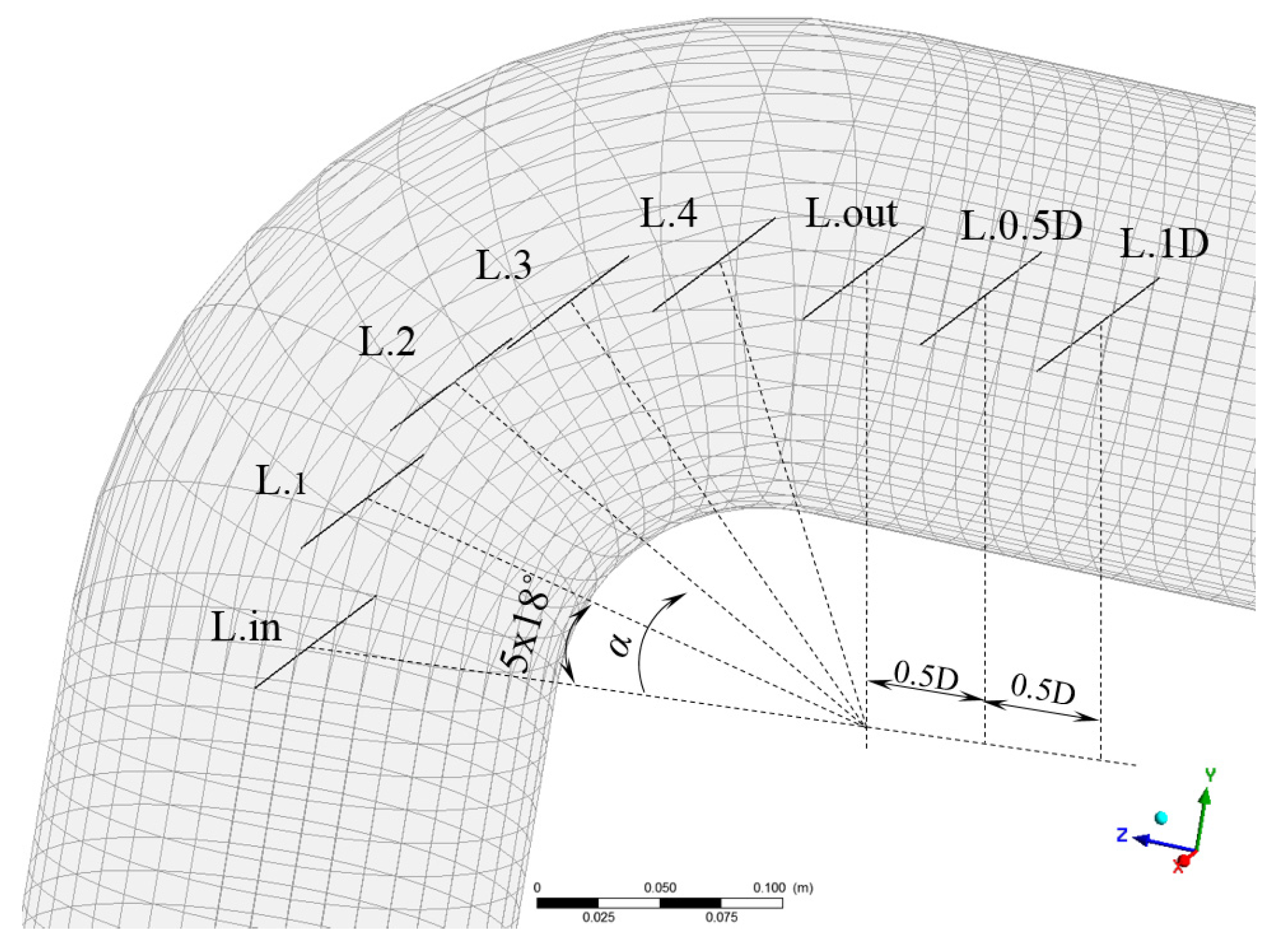
2. Materials and Methods
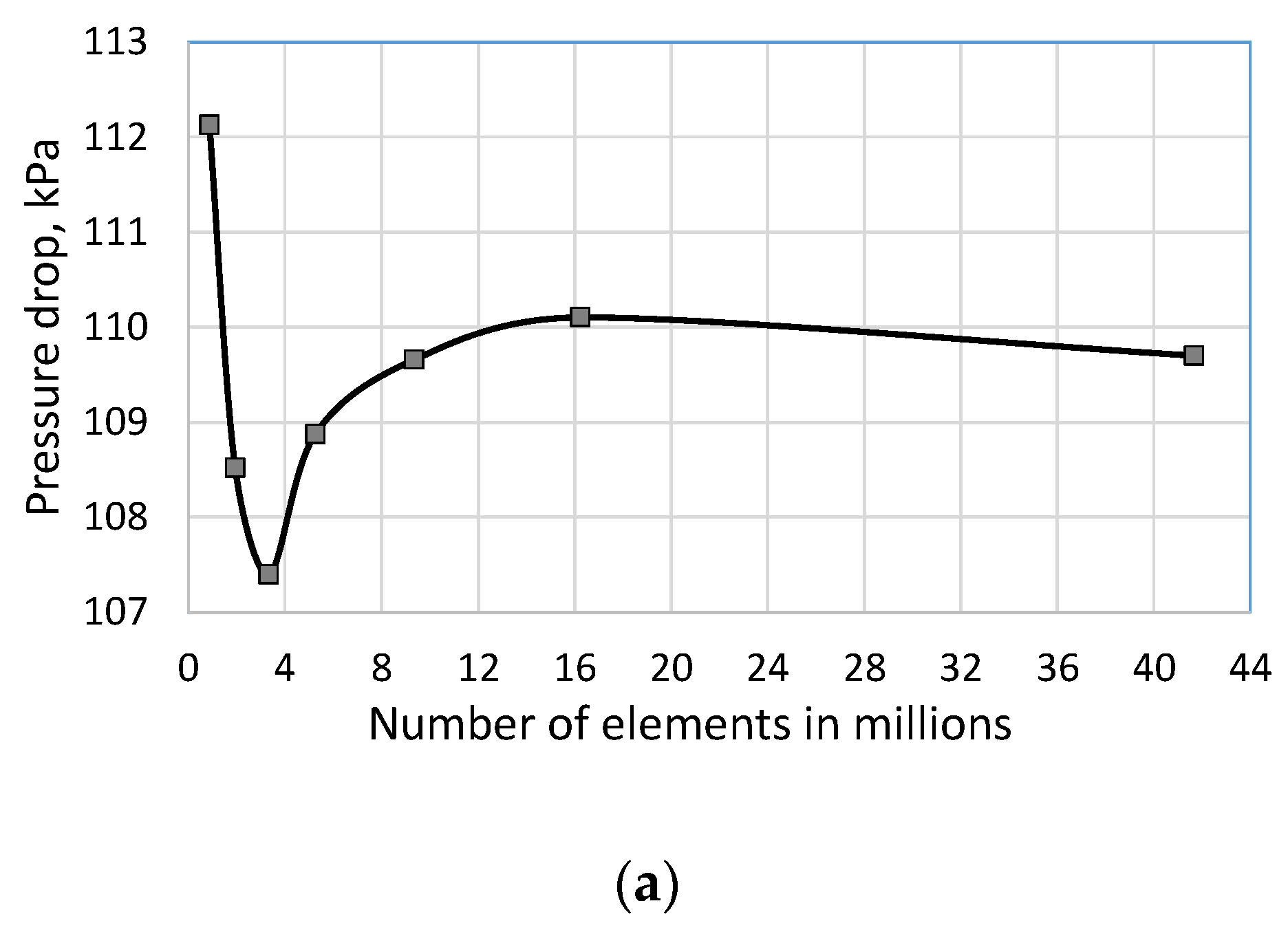
Grid Independence
3. Results and Discussion
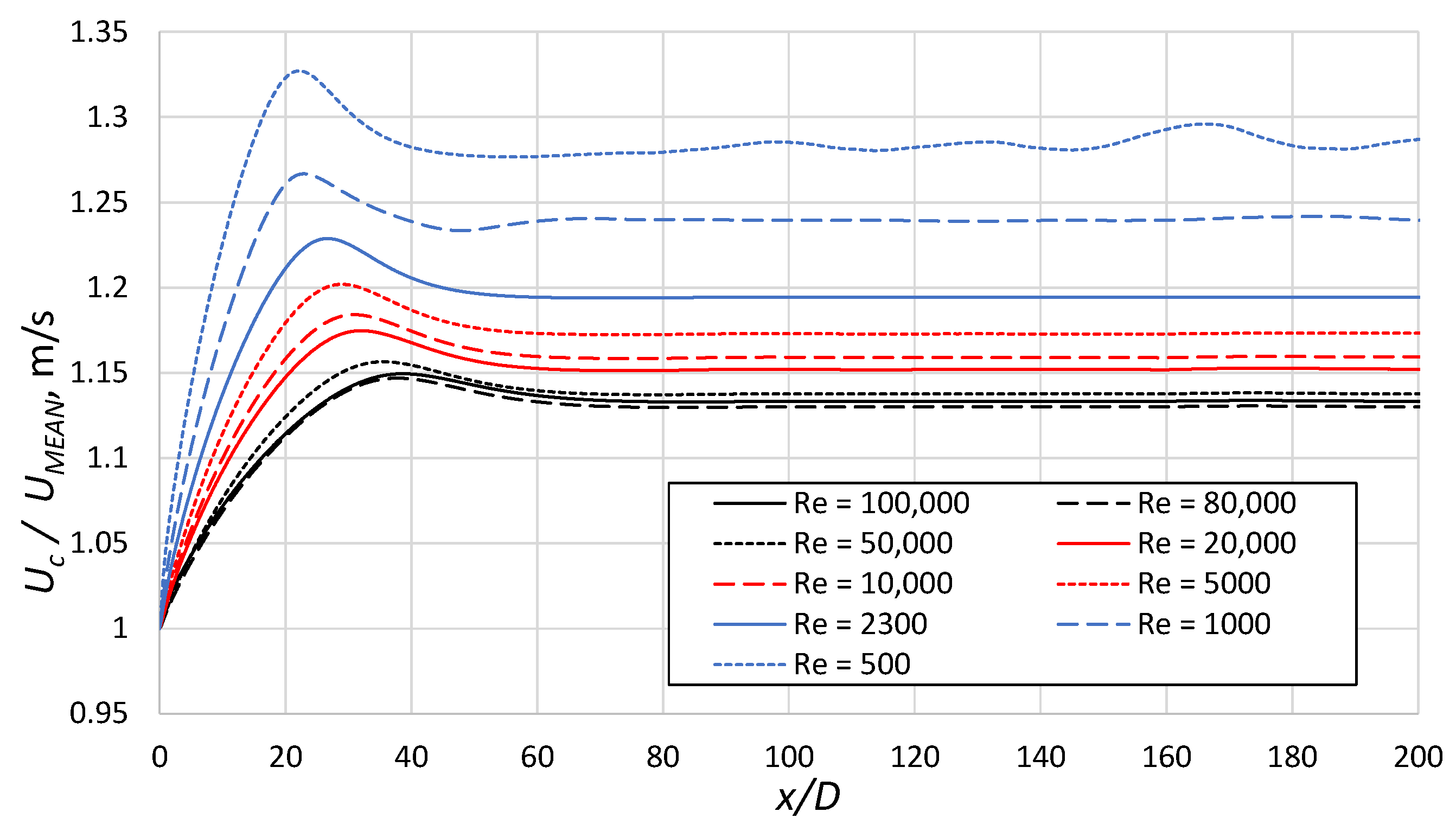
3.1. Hydrodynamic Entry Length and Head Loss
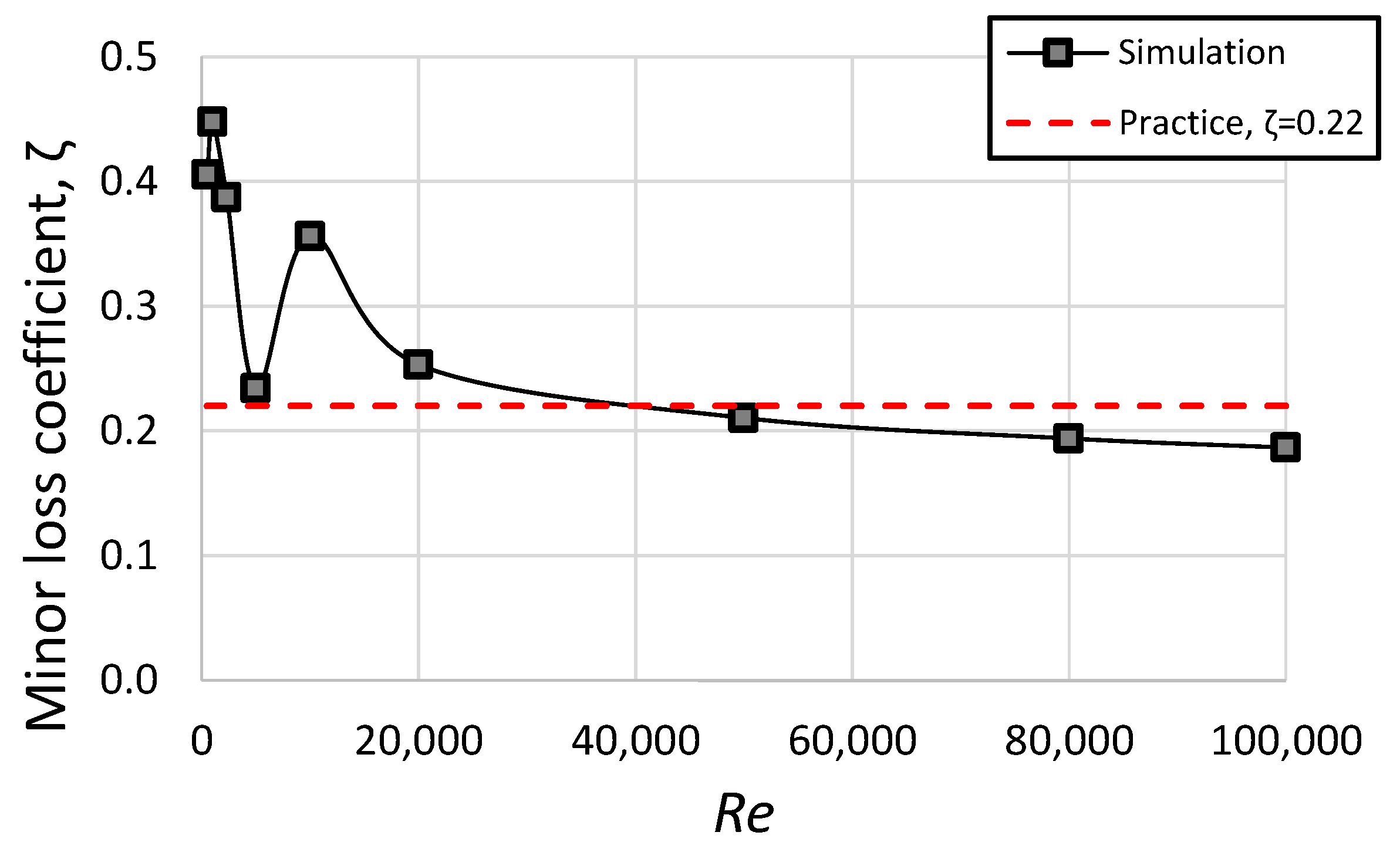
3.2. Minor Loss Coefficient
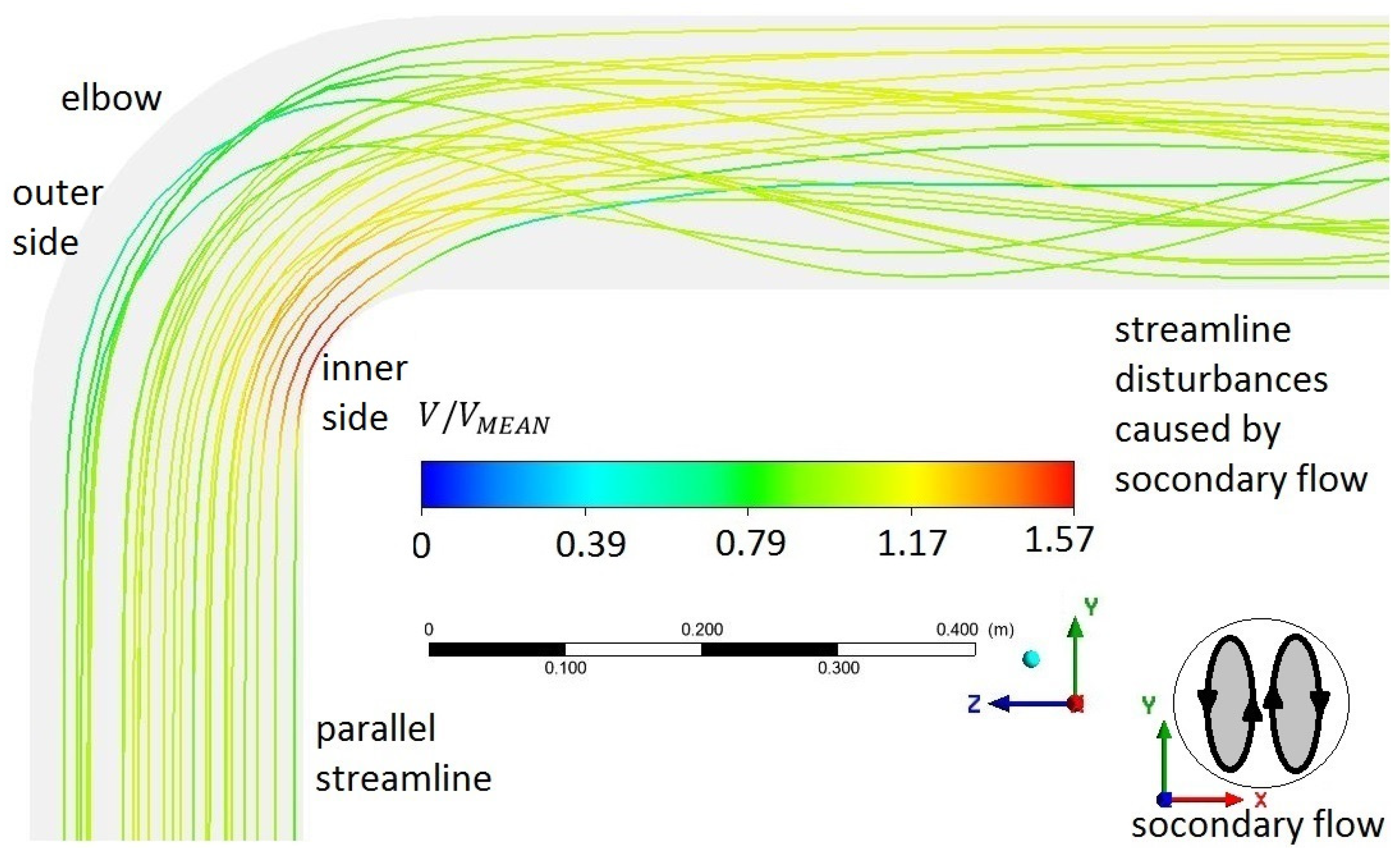
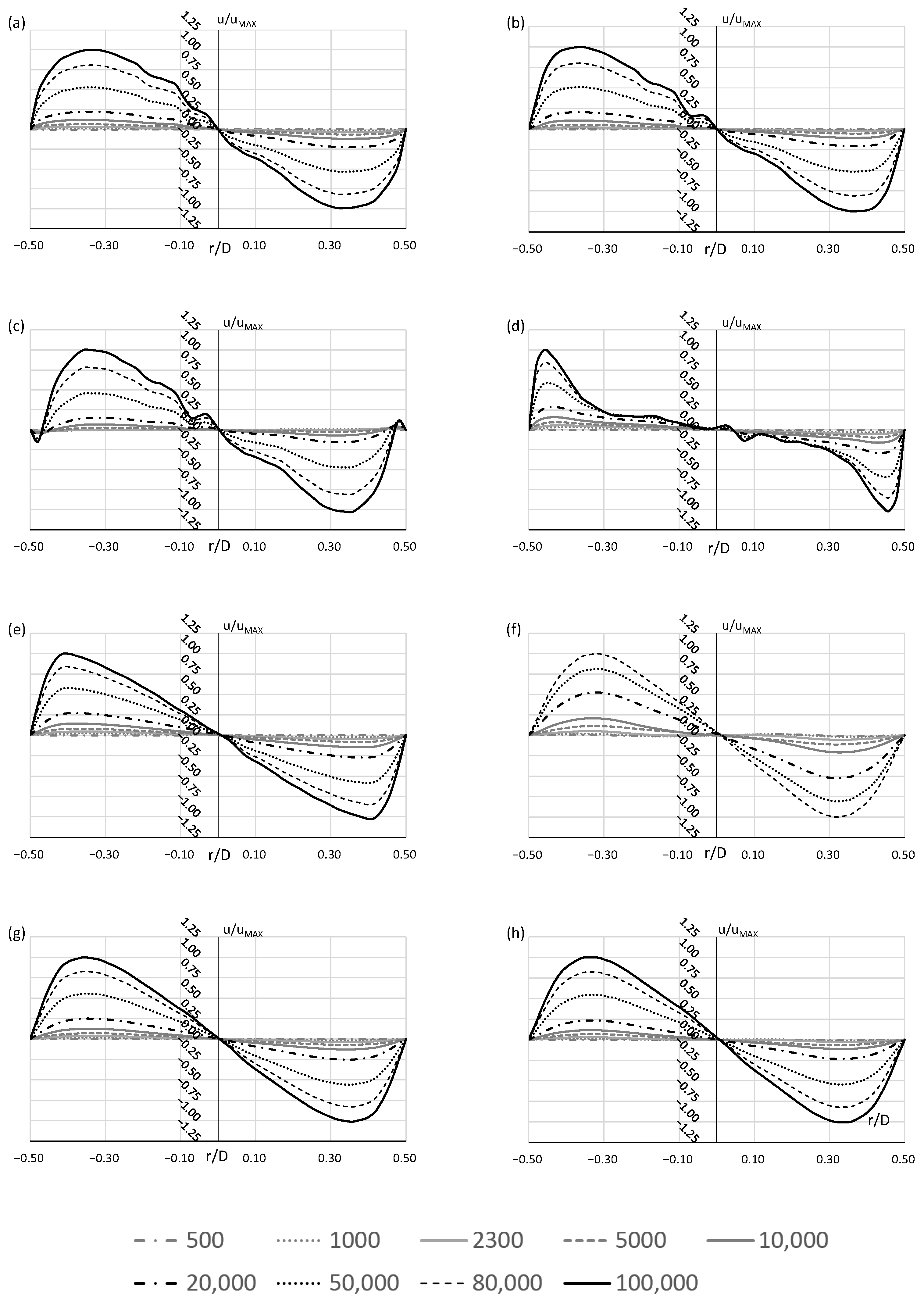
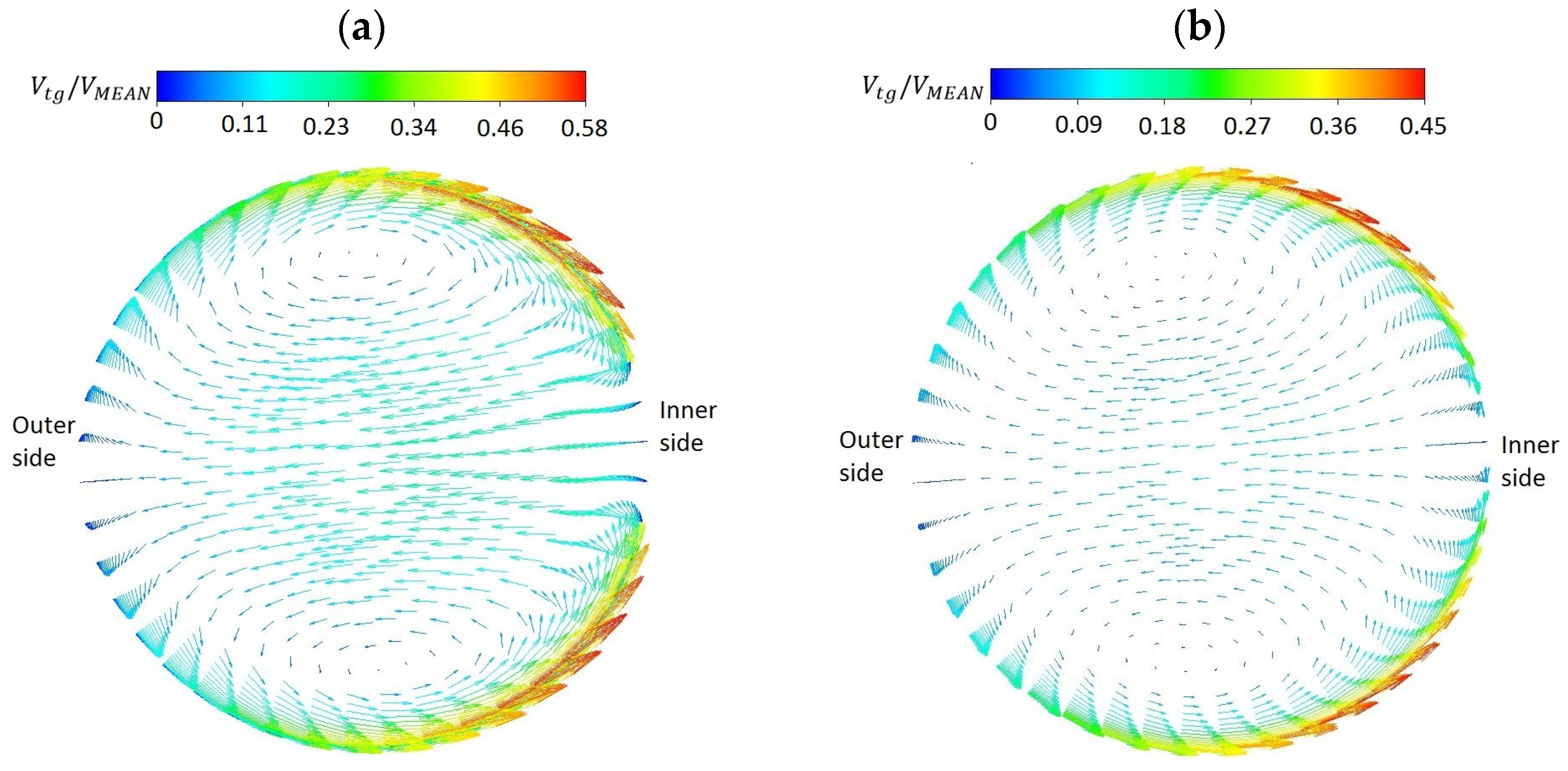
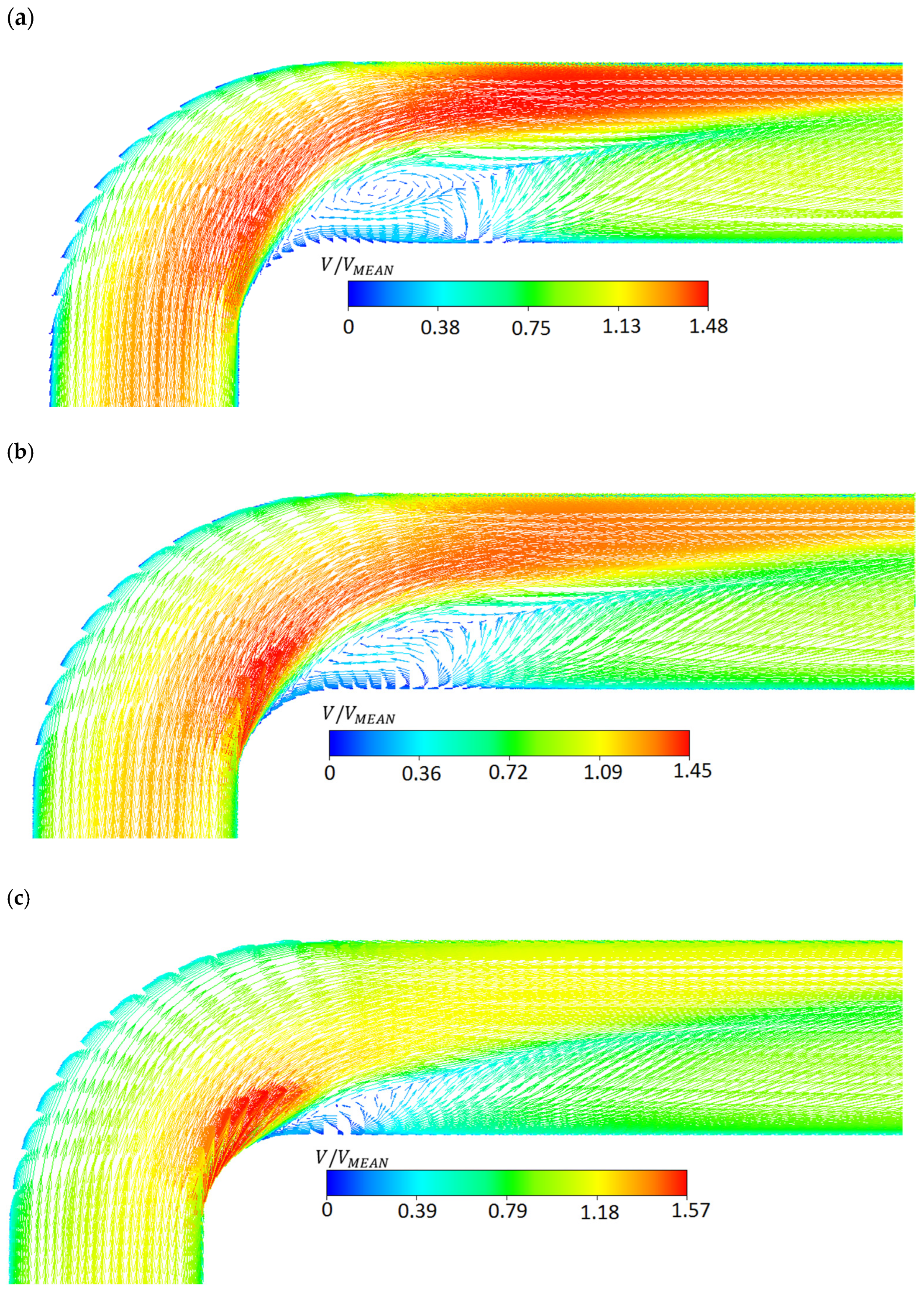
3.3. Flow Analysis
4. Conclusions
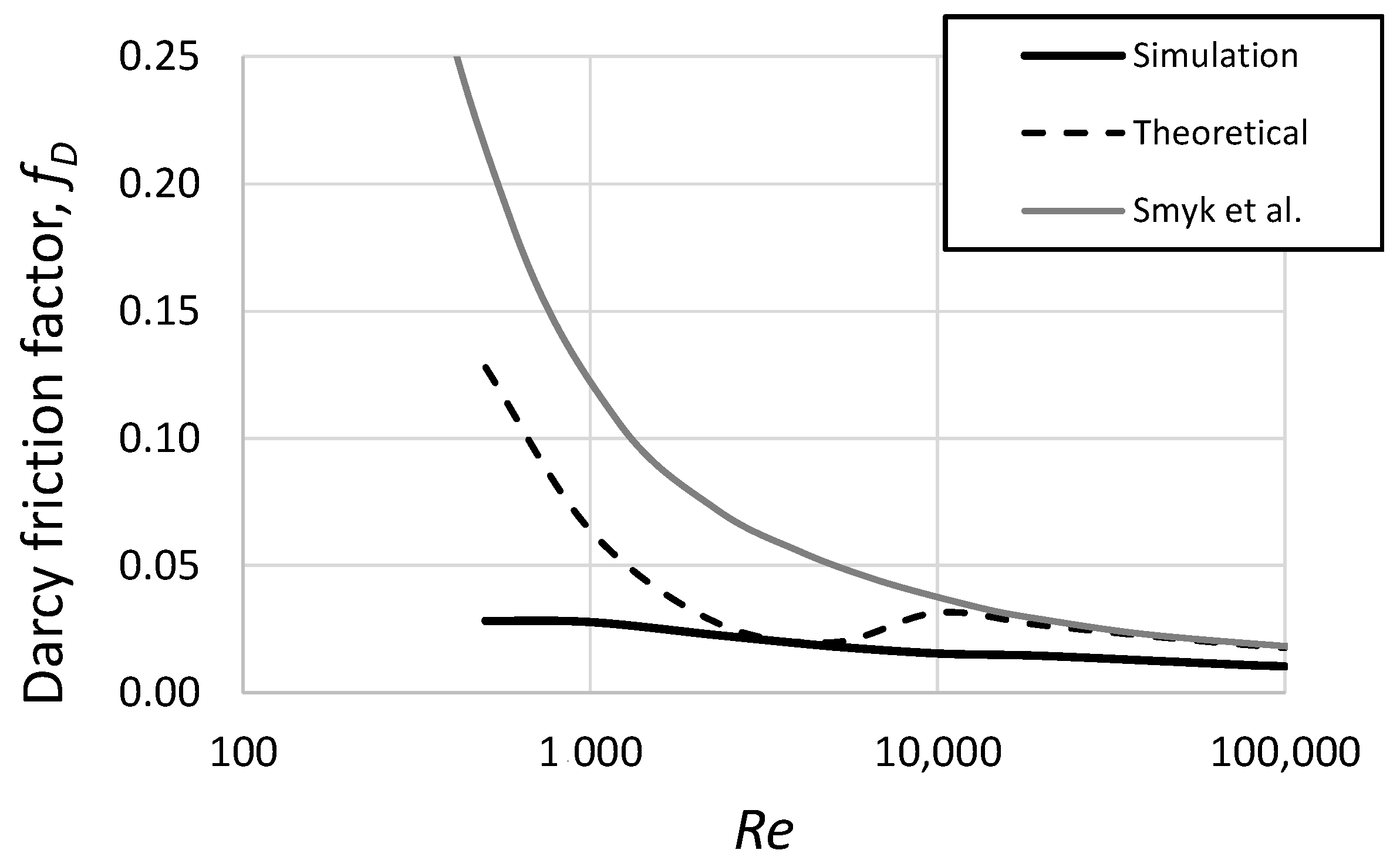
- The k-ω model can be used to calculate head pressure loss. However, the Darcy friction factor was similar to the theoretical value only in the case of the high Reynolds number ().
- The calculated minor loss coefficient depends on the Reynolds number. The calculated value of the minor loss coefficient was similar to the engineering/literature value at . The minor loss coefficient should be better investigated for Reynolds numbers .
- The secondary flow was observed in all investigated cases. It was observed also by the analysis of the velocity profile.
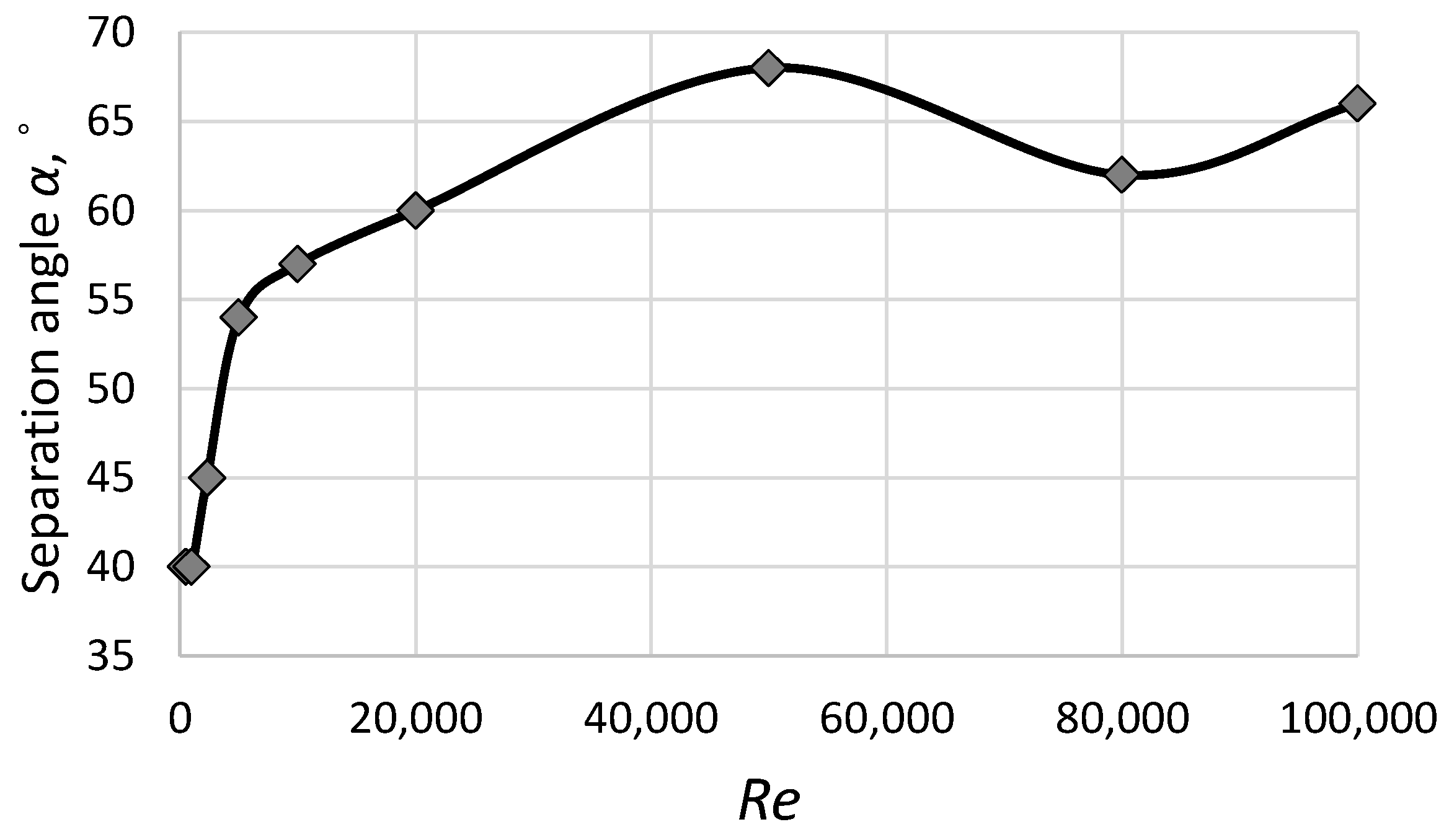
- The separation point location depends on the Reynolds number and is stabilized for , similar to the minor loss coefficient. The analysis of the velocity vectors showed the flow dead zone behind the elbow. The flow dead zones were bigger the lower the Reynolds number.
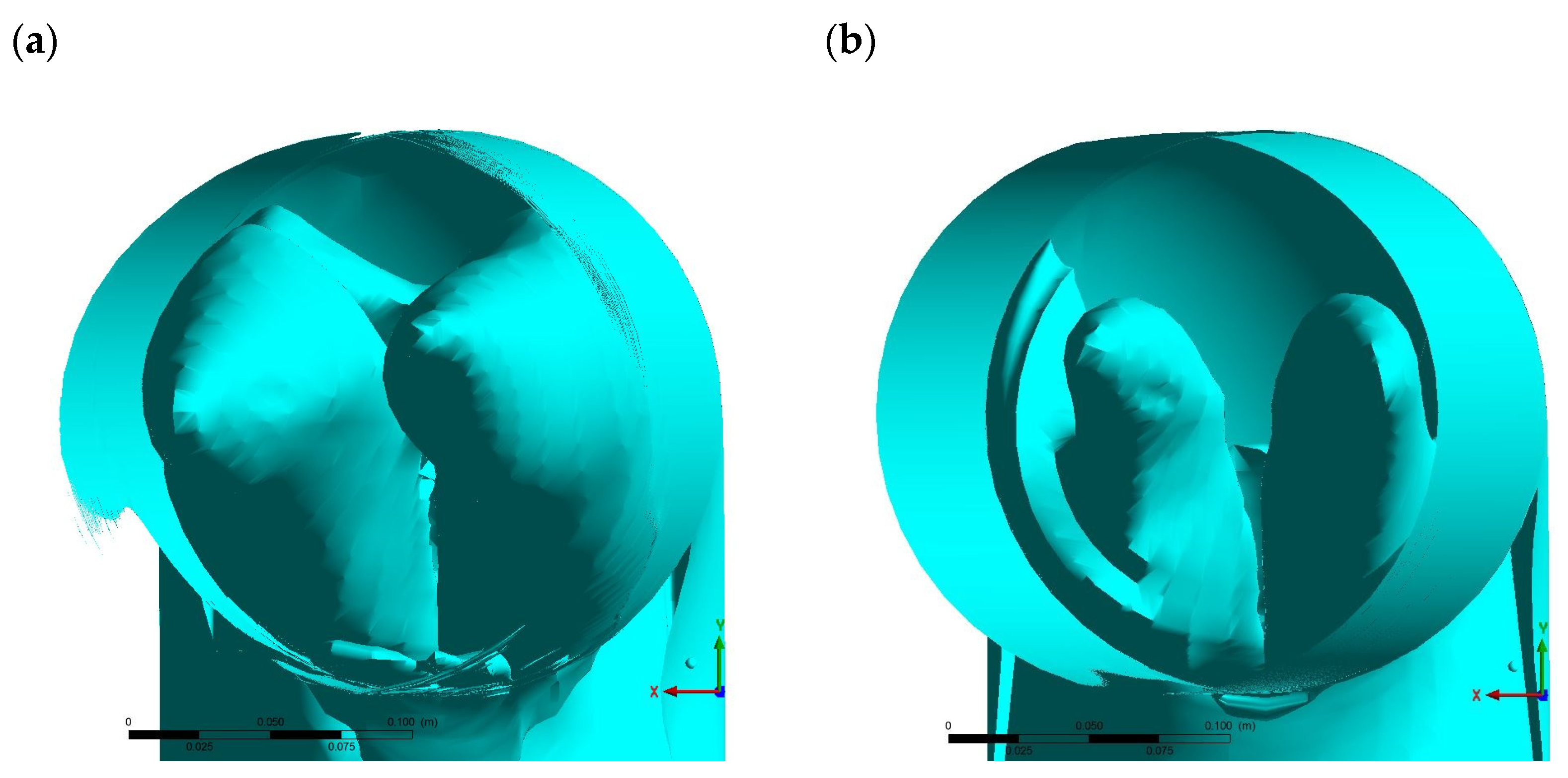
- The Q-criterion vortex identification method showed two vortex structures. They were the result of overlapping of the main flow and the secondary flow. The impact of the secondary flow decreased with the Reynolds number increasing.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- dos Santos, A.P.P.; Andrade, C.R.; Zaparoli, E.L. CFD Prediction of the Round Elbow Fitting Loss Coefficient. Int. J. Mech. Mechatron. Eng. 2014, 8, 743–747. [Google Scholar]
- Smyk, E.; Mrozik, D.; Wawrzyniak, S.; Peszyński, K. Tabular air deflector in ventilation ducts. In Proceedings of the 23rd International Conference Engineering Mechanics, Svratka, Czech Republic, 15–18 May 2017; pp. 882–885. [Google Scholar]
- Gallegos-Muñoz, A.; Uzárraga Rodríguez, N.C.; Belman Flores, J.M.; Rangel Hernández, V.H. Analysis of effect caused by fitting in the measurements of flow in air conditioning system. Appl. Therm. Eng. 2012, 33–34, 227–236. [Google Scholar] [CrossRef]
- Wang, J.; Shirazi, S.A. CFD based correlation for mass transfer coefficient in elbows. Int. J. Heat Mass Transf. 2001, 44, 1817–1822. [Google Scholar] [CrossRef]
- Spedding, P.L.; Benard, E.; Crawford, N.M. Fluid flow through a vertical to horizontal 90° elbow bend III three phase flow. Exp. Therm. Fluid Sci. 2008, 32, 827–843. [Google Scholar] [CrossRef][Green Version]
- Zahedi, R.; Babaee Rad, A. Numerical and experimental simulation of gas-liquid two-phase flow in 90-degree elbow. Alex. Eng. J. 2022, 61, 2536–2550. [Google Scholar] [CrossRef]
- Cao, X.; Zhang, P.; Li, X.; Li, Z.; Zhang, Q.; Bian, J. Experimental and numerical study on the flow characteristics of slug flow in a horizontal elbow. J. Pipeline Sci. Eng. 2022, 2, 100076. [Google Scholar] [CrossRef]
- Ono, A.; Kimura, N.; Kamide, H.; Tobita, A. Influence of elbow curvature on flow structure at elbow outlet under high Reynolds number condition. Nucl. Eng. Des. 2011, 241, 4409–4419. [Google Scholar] [CrossRef]
- Sudo, K.; Sumida, M.; Hibara, H. Experimental investigation on turbulent flow through a circular-sectioned 180° bend. Exp. Fluids 2000, 28, 51–57. [Google Scholar] [CrossRef]
- Röhrig, R.; Jakirlić, S.; Tropea, C. Comparative computational study of turbulent flow in a 90° pipe elbow. Int. J. Heat Fluid Flow 2015, 55, 120–131. [Google Scholar] [CrossRef]
- Kim, J.; Yadav, M.; Kim, S. Characteristics of secondary flow induced by 90-degree elbow in turbulent pipe flow. Eng. Appl. Comput. Fluid Mech. 2014, 8, 229–239. [Google Scholar] [CrossRef]
- Spedding, P.L.; Benard, E.; Mcnally, G.M. Fluid Flow through 90 Degree Bends. Dev. Chem. Eng. Miner. Process. 2010, 12, 107–128. [Google Scholar] [CrossRef]
- Dutta, P.; Nandi, N. Effect of Reynolds number and curvature ratio on single phase turbulent flow in pipe bends. Mech. Mech. Eng. 2015, 19, 5–16. [Google Scholar] [CrossRef]
- Jarrahi, M.; Castelain, C.; Peerhossaini, H. Secondary flow patterns and mixing in laminar pulsating flow through a curved pipe. Exp. Fluids 2011, 50, 1539–1558. [Google Scholar] [CrossRef]
- Rup, K.; Sarna, P. Analysis of turbulent flow through a square-sectioned duct with installed 90-degree elbow. Flow Meas. Instrum. 2011, 22, 383–391. [Google Scholar] [CrossRef]
- Patiño-Jaramillo, G.A.; Iglesias, I.; Vera, M. Laminar flow and pressure loss in planar Tee joints: Numerical simulations and flow analysis. Eur. J. Mech. B/Fluids 2022, 92, 75–89. [Google Scholar] [CrossRef]
- Sun, Q.L.; Xia, L.; Deng, L.; Wang, J.G.; Wang, G.L.; Feng, D. Experimental and Numerical Simulation Analyses of Elbow Erosion in Surface Process of Deepwater Gas Well Testing. J. Fail. Anal. Prev. 2024, 24, 202–215. [Google Scholar] [CrossRef]
- Liu, E.B.; Huang, S.; Tian, D.C.; Shi, L.M.; Peng, S.B.; Zheng, H. Experimental and numerical simulation study on the erosion behavior of the elbow of gathering pipeline in shale gas field. Pet. Sci. 2024, 21, 1257–1274. [Google Scholar] [CrossRef]
- Chen, M.; Tan, L. An improved method combined modified drag model and modified erosion model based on LES for solid-liquid multiphase flow and erosion with application in a 90° elbow. Wear 2024, 538–539, 205214. [Google Scholar] [CrossRef]
- Smyk, E.; Mrozik, D.; Łukasz, O.; Peszyński, K. Numerical simulation of minor losses coefficient on the example of elbows. EPJ Web Conf. 2018, 180, 02093. [Google Scholar] [CrossRef][Green Version]
- Salehi, M.; Sleiti, A.K.; Idem, S. Study to identify computational fluid dynamics models for use in determining HVAC duct fitting loss coefficients. Sci. Technol. Built Environ. 2017, 23, 181–191. [Google Scholar] [CrossRef]
- Liu, W.; Long, Z.; Chen, Q. A procedure for predicting pressure loss coefficients of duct fittings using computational fluid dynamics (RP-1493). HVAC R Res. 2012, 18, 1168–1181. [Google Scholar] [CrossRef]
- Sami, S.; Cui, J. Numerical study of pressure losses in close-coupled fittings. HVAC&R Res. 2004, 10, 539–552. [Google Scholar] [CrossRef]
- Zmrhal, V.; Schwarzer, J. Numerical simulation of local loss coefficients of ventilation duct fittings. In Proceedings of the Eleventh International IBPSA Conference, Glasgow, Scotland, 27–30 July 2009; pp. 1761–1766. [Google Scholar]
- Weissenbrunner, A.; Ekat, A.; Straka, M.; Schmelter, S. A virtual flow meter downstream of various elbow configurations. Metrologia 2023, 60, 5. [Google Scholar] [CrossRef]
- Durst, F.; Ray, S.; Ünsal, B.; Bayoumi, O.A. The development lengths of laminar pipe and channel flows. J. Fluids Eng. Trans. ASME 2005, 127, 1154–1160. [Google Scholar] [CrossRef]
- Kahramanoglu, E.; Sezen, S.; Bayraktar, S. Computational fluid dynamics analyses on the hydrodynamic entry length in internal flows. Pamukkale Univ. J. Eng. Sci. 2017, 23, 372–377. [Google Scholar] [CrossRef]
- Grossmann, S.; Lohse, D. Curvature effects on the velocity profile in turbulent pipe flow. Eur. Phys. J. E 2017, 40, 7–12. [Google Scholar] [CrossRef] [PubMed]
- Bariya, H.G.; Patel, M.P. On the Solution of Blasius Boundary Layer Equations of Prandtl-Eyring Fluid Flow Past a Stretching Sheet. Indian J. Sci. Technol. 2024, 17, 1237–1244. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, Z. Asymptotic behavior of the steady Prandtl equation. Math. Ann. 2023, 387, 1289–1331. [Google Scholar] [CrossRef]
- Cengel, Y.A.; Cimbala, J.M. Fluid Mechanics: Fundamentals and Applications; Mc Graw-Hill, Higher Education: New York, NY, USA, 2006. [Google Scholar]
- Turgut, O.E.; Asker, M.; Çoban, M.T. A review of non iterative friction factor correlations for the calculation of pressure drop in pipes. Bitlis Eren Univ. J. Sci. Technol. 2014, 4, 1–8. [Google Scholar] [CrossRef]
- Vatankhah, A.R. Approximate Analytical Solutions for the Colebrook Equation. J. Hydraul. Eng. 2018, 144, 1–8. [Google Scholar] [CrossRef]
- Kiijarvi, J. Darcy Friction Factor Formulae in Turbulent Pipe Flow. Lunowa Fluid Mech. Pap. 2011, 110727, 1–11. [Google Scholar]
- Hendiger, J.; Ziętek, P.; Chlidzinska, M. Wentylacja i klimatyzacja. Materiały pomocnicze do projektowania (From Polish: Ventilation and Air Conditioning. Support Materials for Design); Venture Industries Sp. z.o.o.: Warszawa, Poland, 2013. [Google Scholar]
- Dutta, P.; Nandi, N. Study on pressure drop characteristics of single phase turbulent flow in pipe bend for high reynolds number. ARPN J. Eng. Appl. Sci. 2015, 10, 2221–2226. [Google Scholar]
- Glenn, A.L.; Bulusu, K.V.; Shu, F.; Plesniak, M.W. Secondary flow structures under stent-induced perturbations for cardiovascular flow in a curved artery model. Int. J. Heat Fluid Flow 2012, 35, 76–83. [Google Scholar] [CrossRef]
- Kalpakli, A.; Örlü, R.; Alfredsson, P.H. Vortical patterns in turbulent flow downstream a 90° curved pipe at high Womersley numbers. Int. J. Heat Fluid Flow 2013, 44, 692–699. [Google Scholar] [CrossRef]
- Valeriu, D. A New Mathematical Model for Coandă Effect Velocity Approximation. Incas Bull. 2012, 4, 85–92. [Google Scholar] [CrossRef]
- Dumitrache, A.; Frunzulica, F.; Ionescu, T.C. Mathematical Modelling and Numerical Investigations on the Coanda Effect. In Nonlinearity, Bifurcation and Chaos-Theory and Applications; Hagedorn, P., Awrejcewicz, J., Eds.; InTech: London, UK, 2012; pp. 101–132. [Google Scholar]
- Jeong, J.; Hussain, F. On the identification of a vortex. J. Fluid Mech. 1995, 285, 69–94. [Google Scholar] [CrossRef]
- Peszynski, K.; Tesař, V.; Kuliś, E. Evaluation of linear losses in ventilation ducts with a rounded rectangle cross-section. MATEC Web Conf. 2019, 302, 01021. [Google Scholar] [CrossRef][Green Version]
- Jurga, A.P.; Janocha, M.J.; Ong, M.C.; Yin, G. Numerical Investigations of Turbulent Flow Through a 90-Degree Pipe Bend and Honeycomb Straightener. J. Fluids Eng. 2024, 146, 021307. [Google Scholar] [CrossRef]
- Puspitasari, D.; Pramadhony, P.; Ellyanie, E.; Marwani, M. The effects of flow straightener inclination on distribution of flue gas flow. AIP Conf. Proc. 2018, 2001, 060002. [Google Scholar]
- Scheiman, J. Comparison of Experimental and Theoretical Turbulence Reduction Characteristics for Screens, Honeycomb, and Honeycomb-Screen Combinations; NASA Technical Paper; NASA: Washington, DC, USA, 1981. [Google Scholar]

| Number | Re | VMEAN, m/s | De |
|---|---|---|---|
| 1 | 100,000 | 8.95 | 70,711 |
| 2 | 80,000 | 7.16 | 56,569 |
| 3 | 50,000 | 4.47 | 35,355 |
| 4 | 20,000 | 1.79 | 14,142 |
| 5 | 10,000 | 0.89 | 7071 |
| 6 | 5000 | 0.45 | 3536 |
| 7 | 2300 | 0.21 | 1626 |
| 8 | 1000 | 0.09 | 707 |
| 9 | 500 | 0.04 | 354 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Smyk, E.; Stopel, M.; Szyca, M. Simulation of Flow and Pressure Loss in the Example of the Elbow. Water 2024, 16, 1875. https://doi.org/10.3390/w16131875
Smyk E, Stopel M, Szyca M. Simulation of Flow and Pressure Loss in the Example of the Elbow. Water. 2024; 16(13):1875. https://doi.org/10.3390/w16131875
Chicago/Turabian StyleSmyk, Emil, Michał Stopel, and Mikołaj Szyca. 2024. "Simulation of Flow and Pressure Loss in the Example of the Elbow" Water 16, no. 13: 1875. https://doi.org/10.3390/w16131875
APA StyleSmyk, E., Stopel, M., & Szyca, M. (2024). Simulation of Flow and Pressure Loss in the Example of the Elbow. Water, 16(13), 1875. https://doi.org/10.3390/w16131875







