1. Introduction
The Ji’an area in Jiangxi Province is mostly mountainous and hilly landforms. Transmission line towers are usually located on ridges or slopes. During the excavation and construction of tower foundations, many artificial tower foundation slopes are formed. The geological conditions in this area are complex, the rainfall is abundant, and geological disasters occur frequently. Under the influence of natural disasters such as rainfall, typhoons, and earthquakes and human engineering activities, these artificial tower foundation slopes are prone to instability and damage problems such as erosion and landslides. The safe operation of the line poses hidden dangers. It is of great significance to analyze the influence of the position of the transmission line tower on the stability of the spoil slope of the tower foundation, which is of great significance to guiding the prevention and control of hidden dangers of the tower foundation slope and the operation and maintenance of the transmission line. The above research results have an important guiding role in improving the risk prevention and control of transmission line safety operation in terms of theory and technical methods. In extreme cases such as heavy rain, once the slope where the foundation of the transmission line tower is located is deformed and damaged, or even the overall landslide occurs, the line will not be able to transmit electricity normally and will cause serious regional social and economic losses [
1]. Therefore, it is of great practical significance to discuss the prevention and control effect of different protective measures on landslides of transmission line tower foundations for ensuring the continuous and safe operation of high-voltage transmission lines in our country. Predecessors have relatively few studies on the stability of transmission line tower foundations and slopes, prevention methods, and prevention measures, and they have only gradually begun to appear in recent years. For example, Huang et al. [
2] published “Landslide area under extreme conditions Evaluation and Analysis of Tower Foundation Instability of EHV Transmission Line”; Zhang et al. [
3] published the reference manuscript “Enhanced technology for sewage sludge advanced dewatering from an engineering practice perspective” and “Coupling analysis of the heat-water dynamics and frozen depth in a seasonally frozen zone” [
4]. The foundation instability of towers of EHV transmission lines in the region has been evaluated and analyzed. Liu et al. [
5], based on the evaluation of the slope stability of the transmission line tower foundation, suggested that targeted control measures should be taken for different risk levels; Yu et al. [
6] published “Selection and application of slope protection schemes for transmission line towers in mountainous areas; Long-term investigations on the pore pressure regime in saturated and unsaturated sloping soils”; Urciuoli et al. [
7] published “Passive soil pressure on sloping ground and design of retaining structures for slope stabilization”; Muraro et al. [
8] published “Triaxial creep tests of glacitectonically disturbed stiff clay–structural, strength, and slope stability aspects”; Kaczmarek et al. [
9] published “Advances of coupled water-heat-salt theory and test techniques for soils in cold and arid regions: A review”; and Shu et al. published work [
10]. After comparing various protection schemes, it is proposed that the pile-slab retaining wall protection has more advantages. These studies are mainly based on stability calculation, or numerical simulation methods, discussing the mode or probability of tower foundation slope instability and putting forward corresponding control countermeasures and schemes, but they have not used experimental methods to conduct in-depth research on the control mechanism or control effect.
At present, relevant scholars have carried out a lot of research on the instability process and mechanical mechanism of rainfall-type landslides by using methods such as physical models and numerical simulations. For example, a comprehensive study has been conducted on the triggering factors of landslides under extreme rainfall conditions. Heavy rainfall is the key factor in inducing shallow landslides [
11,
12,
13,
14,
15,
16]. For example, Chang et al. [
17], based on the Green-Ampt infiltration model, considered the effect of hydrodynamic pressure and established the shallow landslide under the condition of rainfall infiltration. The conceptual model of the landslide deduced the relationship expression between the slope safety factor and the rainfall time under the condition of having or not a groundwater level before the rainfall: Liang et al. [
18] established a landslide fluid–solid coupling calculation model based on the finite element method. Considering the deformation of the soil skeleton and the change in the permeability coefficient caused by the transient seepage of a landslide, the change law of seepage and the stress–strain and stability of landslides under rainfall conditions are analyzed. The excavation at the foot of the slope caused by road construction is also an important factor causing landslide deformation and damage [
19]. Peng et al. [
20] studied the law of deformation and failure of landslides with different excavation angles and different rainfall intensities and concluded that the greater the excavation angle of the landslide, the less affected the landslide is by rainfall.
In order to better study rainfall-induced shallow landslides, Tian et al. [
21], based on the Richards equation and finite element method, narrowed the calculation domain of landslide seepage to landslides, based on the horizontal length of the bedrock slope and the rainfall of landslides The saturation of the infiltration boundary and the rainfall infiltration boundary is revised, and the simplified numerical simulation of landslide rainfall infiltration considering runoff recharge is realized. Zhang et al. [
22] used a numerical simulation method to analyze the influence of the depth, location, and number of cracks on the revival of ancient landslides under rainfall conditions. It also becomes shorter and shorter; with the increase in the number of fractures, the influence range of the seepage field in the sliding body expands, and the saturation time shortens, obviously. For tower foundation landslides, Huang et al. [
23] considered extreme rainfall and local road excavation conditions, analyzed the stability and surface deformation characteristics of Yanzi tower foundation landslides, and explored the impact of landslide surface deformation on the foundation deformation of ultra-high-voltage transmission line towers on slopes. A quantitative calculation method for the inclination of towers under landslide hazards is proposed. However, the analysis of the instability process of the tower foundation landslide with cracks in different relative positions under the action of rainfall is less common.
Although the foundation of transmission line towers will choose a safe and stable area, because they are generally located in mountainous areas, they are extremely vulnerable to geological disasters such as landslides. Overhead transmission lines are “point-to-line” projects, mainly because the tower foundations are directly affected by geological disasters. Many scholars have conducted research on the treatment projects after the transmission line tower foundations are affected by geological disasters. For example, Chen et al. [
24] took the landslide disaster of the No. 157 tower base of Huang (Yan) Da (Zhou) line as an example and studied the characteristics and control measures of tower base landslide disasters of ultra-high voltage transmission lines; Guo et al. [
25] took the Tibet section of the Sichuan-Tibet interconnection transmission line project as an example and carried out an analysis of the mechanism of geological hazards affecting the stability of towers according to different types of geological hazards along the line; Zhang et al. [
26] summarized the impact of landslides on line engineering. Hazards and the calculation formula of the horizontal distance from the edge of rock landslides and soil landslides to the outer edge of the tower foundation bottom surface were obtained; Feng et al. [
27] published “Landslide Treatment and Analysis of Overhead Transmission Line Tower Foundation”, combined the characteristics of the tower foundation, analyzed the common landslide disaster forms at the tower foundation, and studied and analyzed landslide control measures. Zhang et al. [
28,
29] published “Finite element analysis in geotechnical engineering [
30], based on the advances of coupled water-heat-salt theory and test techniques for soils in cold and arid regions: A review”, “Numerical and Experimental Study on Water-Heat-Salt Transport Patterns in Shallow Bare Soil with Varying Salt Contents under Evaporative Conditions: A Comparative Investigation”, and “Theoretical and analytical solution on vacuum preloading consolidation of landfill sludge treated by freeze–thaw and chemical preconditioning”. The above research work provides a theoretical and practical basis for carrying out model experiments on different landslide prevention and control measures. This paper takes a residual soil landslide in Jiangxi Province as an example, based on field investigations and professional monitoring data, and uses numerical simulation methods to study the landslide under heavy rainfall conditions, the deformation and failure characteristics of towers and landslides, and their stability and influencing factors. The research results have important practical significance.
2. Project Overview
2.1. Hydrogeomorphology
Ji’an City is located in the middle reaches of Ganjiang River in central and western Jiangxi Province. It is located between 2558′32″~2757′50″ north latitude and 11,348′~11,556′ east longitude. It is a subtropical monsoon humid climate area with four distinct seasons, a mild climate, abundant rainfall, sufficient light, and a long frost-free period. It is 218 km long from north to south and 208 km wide from east to west, with a total land area of 25,283 square kilometers. Ji’an is a mountainous and hilly basin landform, with mountains and hills as the main terrain, surrounded by mountains on three sides in the east, south, and west.
According to the rainfall data of Ji’an Meteorological Bureau (Ji’an Station), the average annual rainfall is 1534.26 mm, the maximum annual rainfall is 2010.7 mm, the minimum annual rainfall is 897.5 mm, and the average annual rainfall day is 156.6 days. The maximum daily rainfall is 215.5 mm, the maximum rainfall within 1 h is 60.3 mm, and the maximum rainfall within 24 h is 187.3 mm. The distribution of rainfall in time is mostly concentrated in March to August, accounting for about 72.18% of the annual rainfall.
The landslide body is in the shape of a long tongue, the rear wall of the landslide is relatively steep, and the rear edge slope is relatively gentle. There are many jagged cracks on the road. The terrain at the trailing edge of the landslide is relatively gentle, and the possibility of sliding is small; the sides of the landslide perimeter are steep, and sliding may occur. The main influencing factors of landslide deformation in the study area include:
Rock and soil properties: According to the survey data, the landslide mass is composed of residual soil, gravel-containing silty clay, and fully weathered glutenite. The structure of the landslide is loose, and it is easy for the surface water to infiltrate under the condition of rainfall. The water flow will cause the clay in the soil to carry and lose continuously.
Rainfall factors: Rainfall infiltration reduces the shear strength of the rock and soil mass, leading to a rise in the groundwater level in the slope body, reducing the anti-sliding frictional resistance generated by the self-weight of the slide body, resulting in higher pore water pressure inside the slope body, and enhancing the slide of the slope body ability.
Human factors: The front edge of the landslide is excavated and cut to build houses, there are long-term vehicle loads passing by and vibrating, and the steep cutting slope changes the original balance conditions, providing sliding space for the landslide.
To sum up: the landslide in this project is a shallow soil landslide, and the main causes are: the structure of residual slope deposits is loose, it is easy for rainwater to infiltrate, and the volumetric water content increases; rainfall infiltration not only increases the weight of the soil but also reduces the soil. The shear strength of the body itself and the artificial slope change the original terrain.
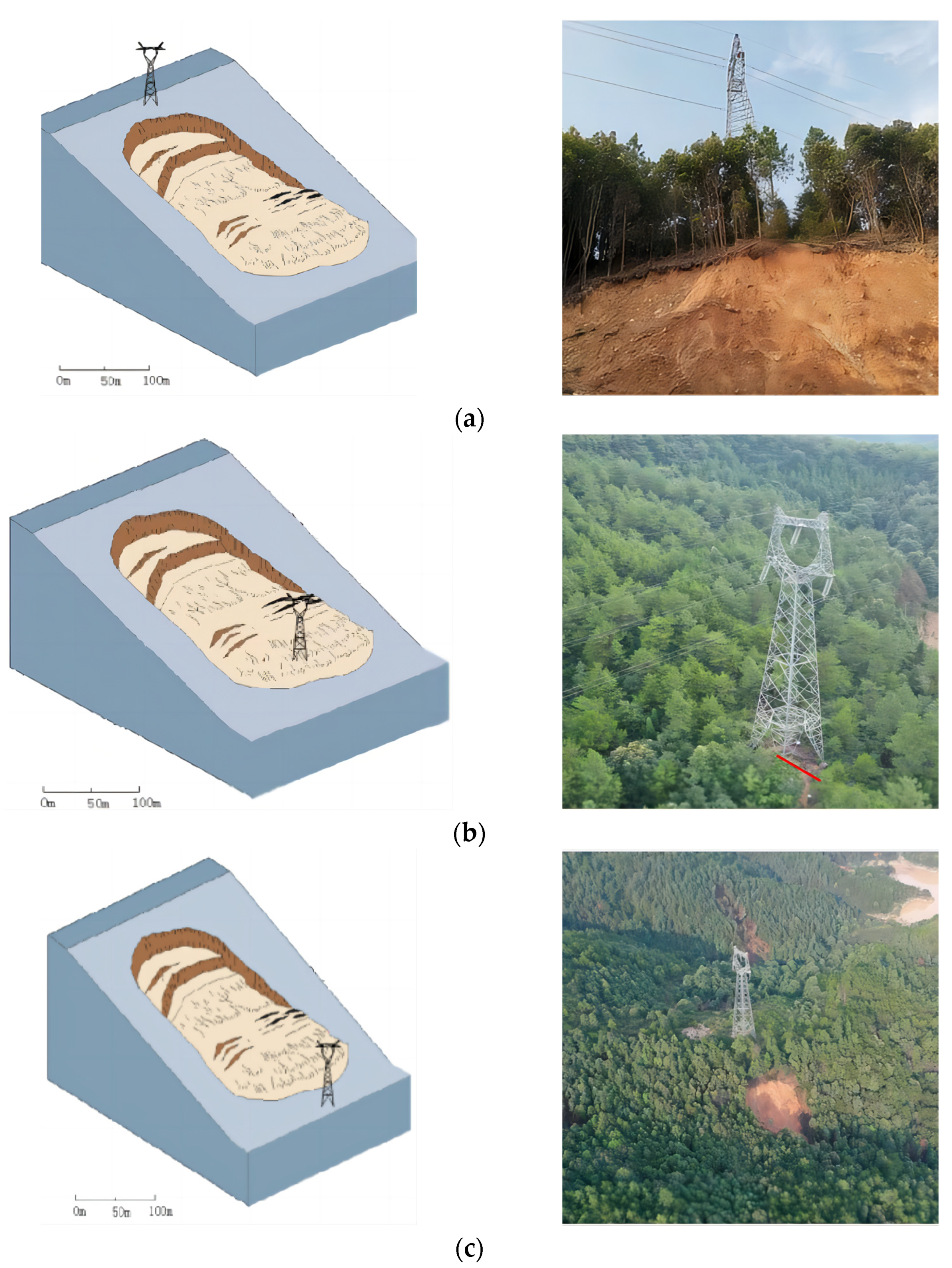
2.2. Relation between the Pole and Tower Position and the Landslide
Through the field investigation, the geological disasters along the overhead transmission line project are more serious. According to incomplete statistics, the number of poles and towers reported to be affected by geological disasters in 2019–2020 in the province is 35, and the number of poles and towers affected by flood disasters is 29. Jingge Line, Jingdiao Line, and Wenzhang Line in the study area have been affected by geological disasters frequently in the past two years, which has caused great hidden dangers to the safety of the power supply in Ji’an city and even in the province. Among them, the relative position relationship between the line tower and landslide disaster can be divided as in
Figure 1:
When the pole and tower are located within the influence range of the landslide, the stability of its foundation will be affected no matter what its relative position is, as long as the pole and tower are located within a certain range of the landslide.
In summary, there are two ways to affect the landslide on the tower: one is by acting on the foundation, and the other is directly acting on the tower member. Based on the failure types in this study area, the stability of poles and towers is closely related to different positions, different rainfall intensities, and different slopes. The following analysis and discussion are carried out to study the failure mechanism.
3. Model Introduction
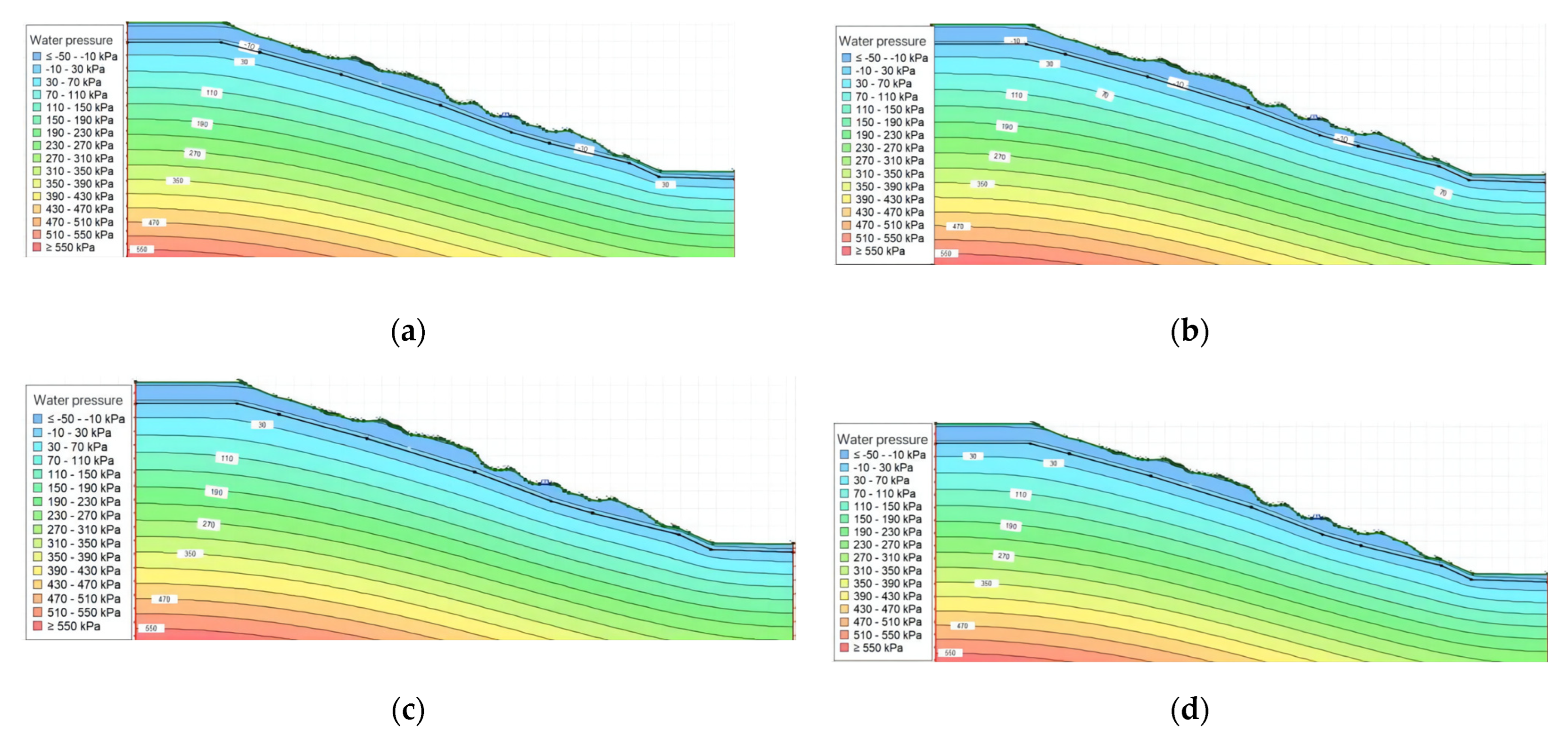
The finite element method can consider slope stability issues from the perspective of stress and strain and has outstanding advantages such as strong applicability and convenience in dealing with heterogeneity, nonlinearity, and complex boundaries. In this paper, the SIGMA/W module and SEEP/W module of Geo-Studio geotechnical engineering simulation software are used for rainfall (the soil–water characteristic curve suitable for engineering analysis can be added by itself, and the movement of the soaked surface and the dissipation process of pore water pressure can be calculated). Based on the simplified typical geological section, combined with the physical and mechanical properties of rock and soil, the load conditions of the tower are designed. Finally, through a variety of integrated analysis and comprehensive evaluation methods, the slope stability analysis and stress–deformation analysis of the slope and pile foundation are carried out.
Among them, there is the slope numerical model SEEP/W module: (1) It is used to analyze the seepage and pore water pressure of porous materials such as soil. (2) It is used for the seepage problem of unsaturated soil. The saturated–unsaturated calculation model in SEEP/W software allows the software to simulate steady-state and transient seepage processes. (3) The saturated–unsaturated calculation model in the software allows the software to simulate steady-state and transient seepage processes. It can add the soil–water characteristic curve suitable for engineering analysis by itself and calculate the movement of the soaked surface and the dissipation process of pore water pressure. SIGMA/W module: (1) With linear and nonlinear elastic models, elastoplastic models, and elastoviscosity models, it can perform stress–strain analysis on soils in different environments. (2) It can calculate the excess pore water pressure of the soil under a load and analyze the slope stability. The pore water pressure in SEEP/W can be called in SIGMA/W to simulate the generation and dissipation of pore water pressure, which is used for slope consolidation settlement and stress–strain analysis.
The slope angle of the slope model is 22°, the length is 200 m, and the height is 80 m. The pile length is 15 m and the pile spacing is 8 m. According to the actual situation, x is set as the fixed boundary on the right and left sides of the model, and X-Y is the fixed boundary on the bottom of the model. The parameters of the soil layer and the parameters of the pile foundation are shown in
Table 1.
5. Discussion
The study intends to focus on the typical unstable slope rock and soil along the transmission line project and intends to use numerical simulation, theoretical analysis and other technical means to carry out the impact of the landslide nature, scale, relative position relationship with the tower, distance, and other influencing factors on the foundation of the tower. The transmission line tower foundation has the characteristics of discontinuity. The significance of this feature is that when the landslide deforms and fails, the safety of the tower will be threatened only when the tower foundation is within the deformation range of the landslide, and the transmission line tower will fail when the landslide occurs. When stable failure occurs, it is impacted by the sliding body and fails. In addition, compared with the rainfall duration of 5 days, the rainfall intensity is: 20 mm/d, 45 mm/d, 90 mm/d, and 190 mm/d. The impact on slope stability: the greater the rainfall intensity, the greater the rainwater infiltration depth, the greater the pore water pressure and volumetric water content change, the greater the range of influence on the shear stress on the slope surface, and the greater the shear stress increment and displacement increment. In the case of the same rainfall intensity and different slope gradients, the smaller the slope gradient, the greater the pore water pressure change, the greater the infiltration depth, and the safety factor of the slope decreases accordingly, and eventually, the slope will fail. These results show that the relative position of the towers, rainfall intensity, and slope will affect the slope stability. Further research can be based on the results of this paper to carry out corresponding experimental analysis and verification work.
6. Conclusions
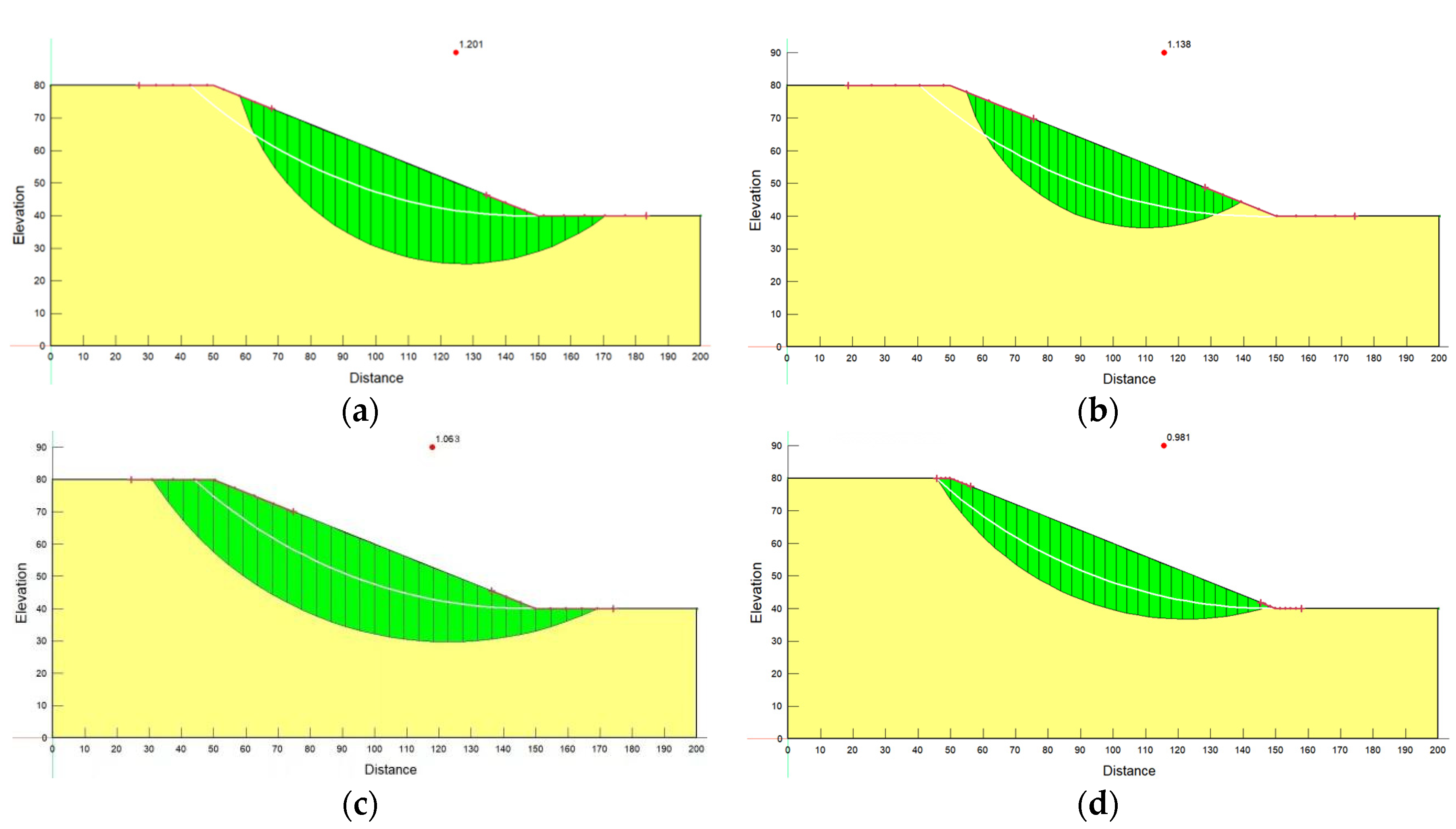
(1) The finite element analysis method is used to calculate the corresponding safety factors of different pole and tower positions, respectively. The results show that the slope and slope height have an important influence on the selection of a reasonable position of the pole and tower. The safety factor of the slope is the highest when the pole tower is located at the lower part of the slope, which may be because the effect of the pile foundation is equivalent to that of the retaining wall strengthening the slope. Second, the pole and tower are located at the bottom of the slope and the top of the slope, and the safety coefficient of the slope is similar. When the pole and tower are located at the upper part of the slope, the safety coefficient of the slope is the lowest, and there is the possibility of instability under some extreme conditions.
(2) The maximum total stress on the slope increases with the increase in the depth at different positions of the pole and tower, the contour line is basically parallel to the slope surface, and the stress concentration occurs in the downward direction of the extension pile. However, no matter where the tower is located on the slope, the stress concentration will occur at the downhill foot of the platform, and the range will expand from top to bottom, which may cause soil structure damage under the platform, resulting in instability.
(3) Under normal circumstances, when the rainfall intensity is the same and the slope is different, the smaller the slope is, the easier it is for rainwater to penetrate into the slope, the greater the change in the pore water pressure, the greater the depth of infiltration, the safety factor of the slope will also decrease, and the slope will eventually be destabilized and destroyed. In contrast, when the rainfall duration is 5 days, the rainfall intensity is 20 mm/d, 45 mm/d, 90 mm/d, and 190 mm/d, and the influence on slope stability is as follows: the greater the rainfall intensity, the greater the rainwater infiltration depth, the greater the pore water pressure and volume water content changes, the greater the influence range on the shear stress on the slope surface, and the greater the shear stress increment and displacement increment. The stability coefficient of the slope in the natural state is 1.221, which is in the stable state. The results provide a scientific basis for further understanding of the influence mechanism of the slope angle of a column pole on such landslides under rainfall conditions. Further research can be based on the results of this paper to carry out the corresponding experimental analysis and verification work.