An Analytical Model Coupled with Orthogonal Experimental Design Is Used to Analyze the Main Controlling Factors of Multi-Layer Commingled Gas Reservoirs
Abstract
:1. Introduction
2. Mathematical Model
2.1. Establishment of Constant Production Mathematical Model
2.2. Laplace Solution of Constant Production Equation
2.3. Real-Time Domain Solution of Constant Production Problem
3. Results and Discussion
3.1. Effect of Dimensionless Parameters on Layered Contribution Rate
3.1.1. Storage Coefficient
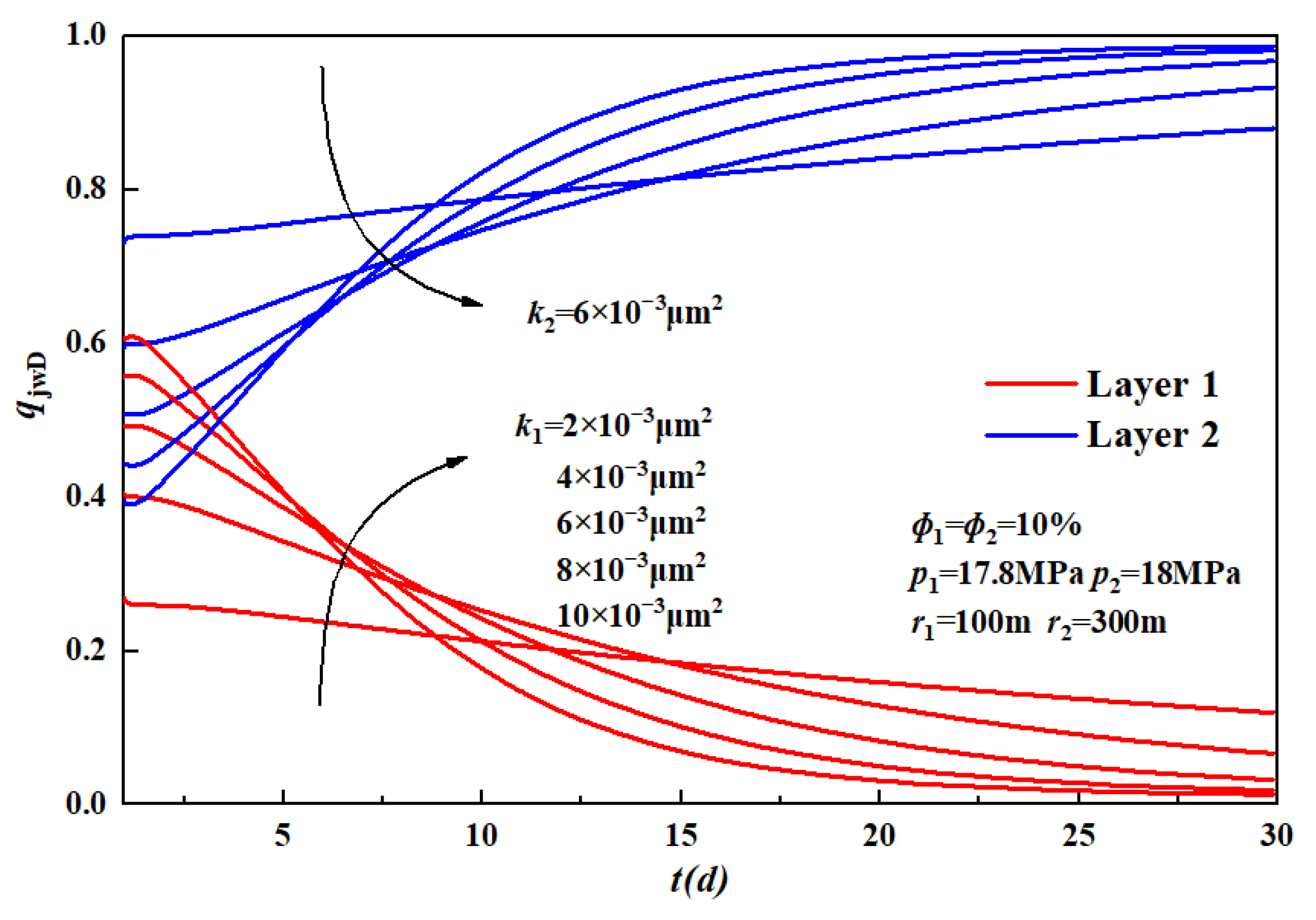
3.1.2. Permeability Coefficient
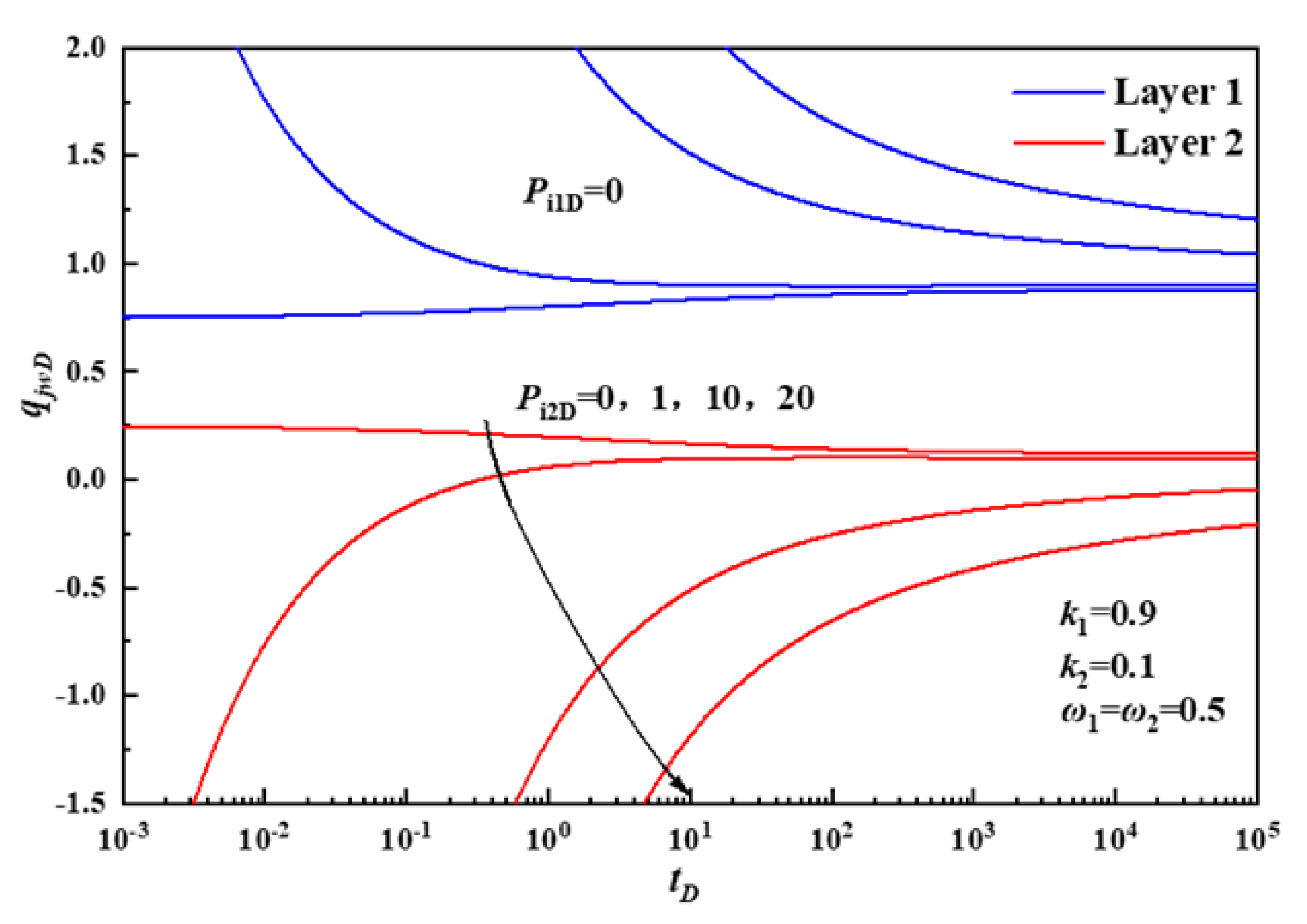
3.1.3. Dimensionless Initial Pressure
3.2. Case Example Analysis of Changqing Oil Field
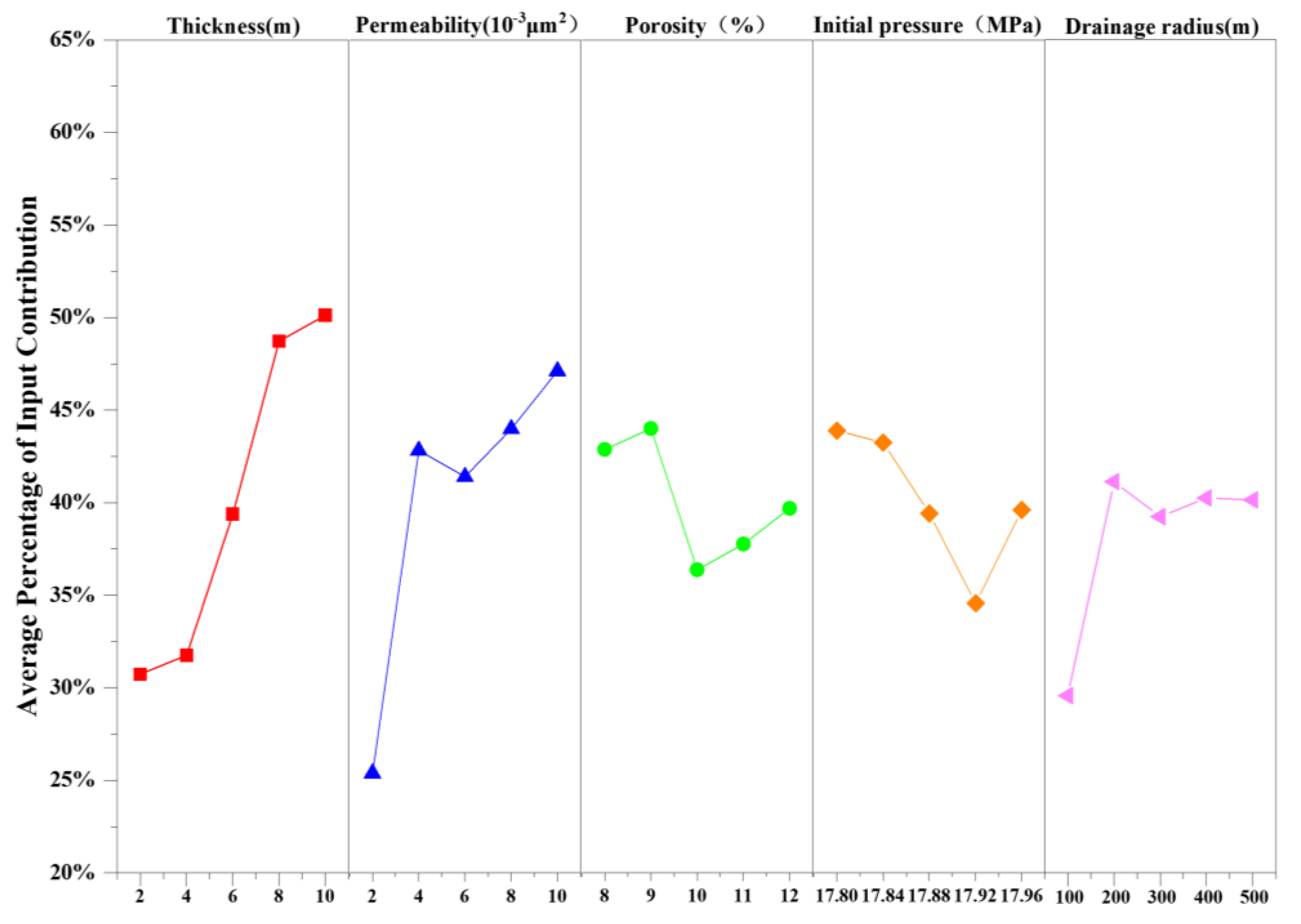
3.2.1. Effect of Formation Parameters on Layered Contribution Rate
- 1
- Effect of permeability on layered contribution rate.
- 2
- Effect of initial pressure on layered contribution rate.
- 3
- Effect of porosity on layered contribution rate.
- 4
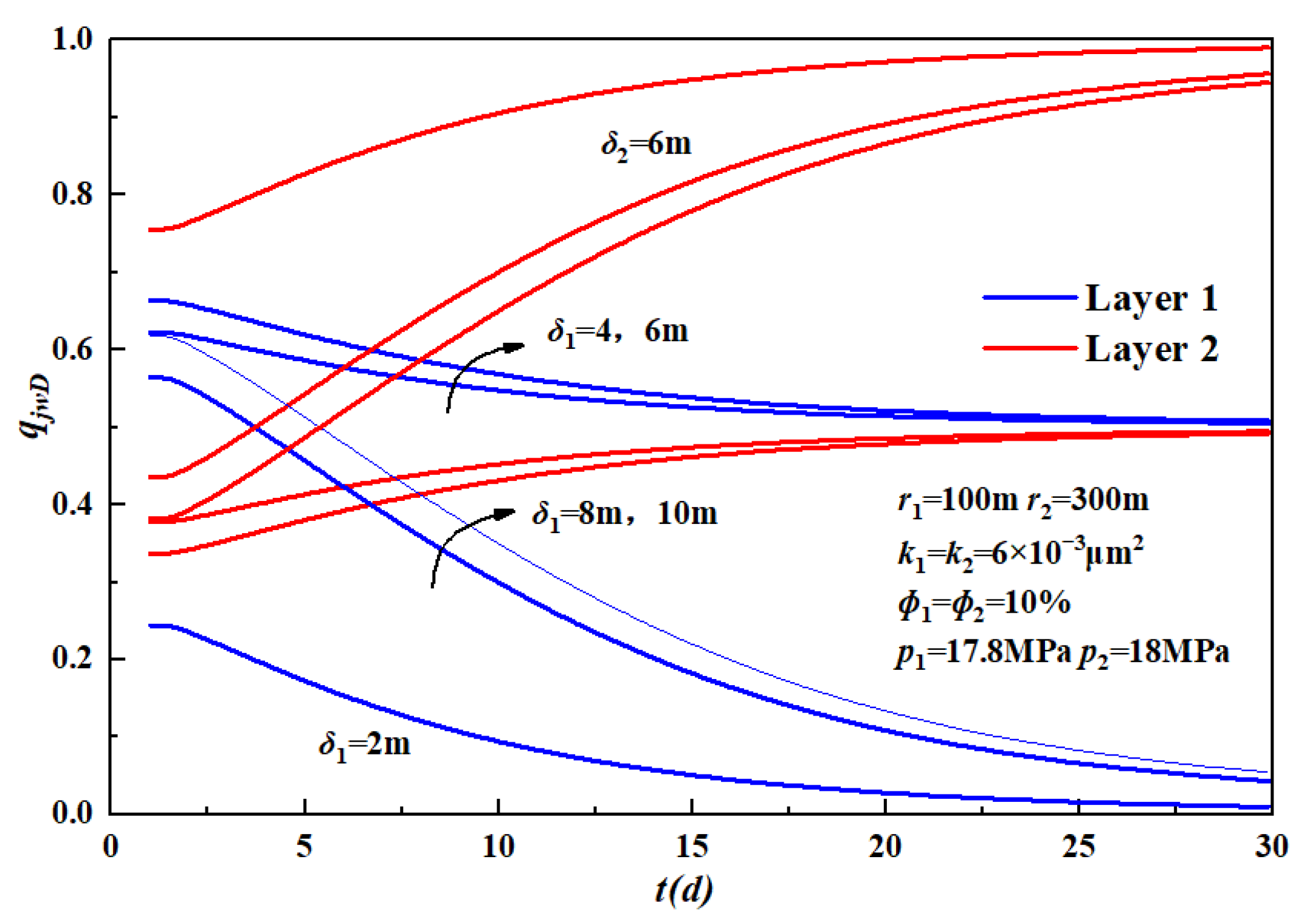
- Effect of formation thickness on layered contribution rate.
- 5
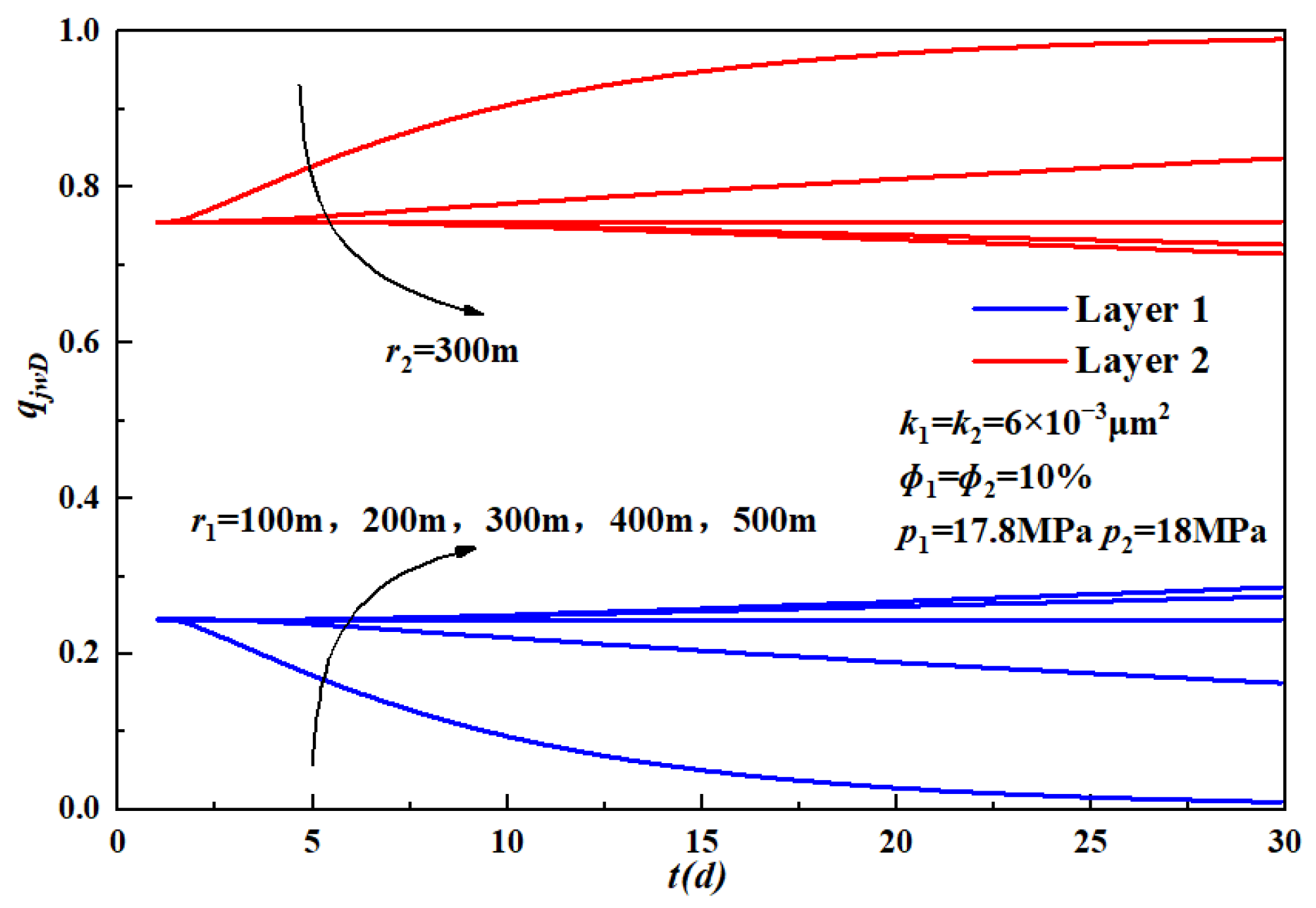
- Effect of drainage radius on layered contribution rate.
3.2.2. Multi-Factor Sensitivity Analysis
- 1
- Orthogonal experiment design.
- 2
- Range analysis of orthogonal test results.
- 3
- Variance analysis of Orthogonal test results.
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kong, X. Advanced Seepage Mechanics, 3rd ed.; University of Science and Technology of China Press: Hefei, China, 2020. [Google Scholar]
- Zhang, M.Y. Numerical Simulation of Factors Affecting Productivity of Multi-Layer Fractured Straight Wells in Tight Gas Reservoirs; Yangtze University: Wuhan, China, 2016. [Google Scholar]
- Lefkovits, H.C.; Hazebroek, P.; Allen, E.E.; Matthews, C.S. A study of the behavior of bounded reservoirs composed of stratified layers. Soc. Pet. Eng. J. 1961, 1, 43–58. [Google Scholar] [CrossRef]
- Russell, D.G.; Prats, M. Performance of Layered Reservoirs with Crossflow Single Compressible Fluid Case. Soc. Pet. Eng. J. 1962, 2, 53–67. [Google Scholar] [CrossRef]
- Russell, D.G.; Prats, M. The Practical Aspects of Interlayer Crossflow. J. Pet. Technol. 1962, 14, 589–594. [Google Scholar] [CrossRef]
- Papadopulos, I.S. Nonsteady flow to multiaquifer wells. J. Geophys. Res. 1966, 71, 4791–4797. [Google Scholar] [CrossRef]
- Kucuk, F.; Ayestaran, L. Analysis of simultaneously measured pressure and sandface flow rate in transient well testing (includes associated papers 13937 and 14693). J. Pet. Technol. 1985, 37, 323–334. [Google Scholar] [CrossRef]
- Kucuk, F.; Karakas, M.; Ayestaran, L. Well testing and analysis techniques for layered reservoirs. SPE Form. Eval. 1986, 1, 342–354. [Google Scholar] [CrossRef]
- Tariq, S.M.; Henry, J.R. Drawdown behavior of a well with storage and skin effect communicating with layers of different radii and other characteristics. In Proceedings of the SPE Annual Fall Technical Conference and Exhibition, Houston, TX, USA, 1–3 October 1978. [Google Scholar]
- Larsen, L. Wells producing commingled zones with unequal initial pressures and reservoir properties. In Proceedings of the SPE Annual Technical Conference and Exhibition, San Antonio, TX, USA, 4–7 October 1981. [Google Scholar]
- Bourdet, D. Pressure behavior of layered reservoirs with crossflow. In Proceedings of the SPE California Regional Meeting, Bakersfield, CA, USA, 27–29 March 1985. [Google Scholar]
- Mavor, M.J.; Walkup, G.W., Jr. Application of the parallel resistance concept to well test analysis of multilayered reservoirs. In Proceedings of the SPE California Regional Meeting, Oakland, CA, USA, 2–4 April 1986. [Google Scholar]
- Rahman, N.M.A.; Mattar, L. New analytical solution to pressure transient problems in commingled, layered zones with unequal initial pressures subject to step changes in production rates. J. Pet. Sci. Eng. 2007, 56, 283–295. [Google Scholar] [CrossRef]
- Spath, J.B.; Ozkan, E.; Raghavan, R. An efficient algorithm for computation of well responses in commingled reservoirs. SPE Form. Eval. 1994, 9, 115–121. [Google Scholar] [CrossRef]
- Milad, B.; Civan, F.; Devegowda, D.; Sigal, R.F. Modeling and simulation of production from commingled shale gas reservoirs. In Proceedings of the SPE/AAPG/SEG Unconventional Resources Technology Conference, Denver, CO, USA, 12–14 August 2013. [Google Scholar]
- Agarwal, B.; Chen, H.Y.; Raghavan, R. Buildup behaviors in commingled reservoir systems with unequal initial pressure distributions: Interpretation. In Proceedings of the SPE Annual Technical Conference and Exhibition, Washington, DC, USA, 4–7 October 1992. [Google Scholar]
- Hatzignatiou, D.G.; Ogbe, D.O. Interference pressure behavior in stratified reservoirs. In Proceedings of the SPE Western Regional Meeting, Anchorage, AK, USA, 26–28 May 1993. [Google Scholar]
- Sahni, A.; Hatzignatiou, D.G.; Ogbe, D.O. Interference pressure behavior in multilayered faulted reservoirs. In Proceedings of the SPE Western Regional Meeting, Anchorage, AK, USA, 22–24 May 1996. [Google Scholar]
- Ei-Banb, A.H.; Wattenbarger, R.A. Analysis of commingled tight gas reservoirs. In Proceedings of the SPE Annual Technical Conference and Exhibition, Denver, CO, USA, 6–9 October 1996. [Google Scholar]
- Arevalo-Villagran, J.A.; Wattenbarger, R.A.; El-Banbi, A.H. Production analysis of commingled gas reservoirs-case histories. In Proceedings of the SPE International Petroleum Conference and Exhibition in Mexico, Villahermosa, Mexico, 1–3 February 2000. [Google Scholar]
- Zhang, S.Q.; Zhang, W.J.; Zhang, S.G. Influence of interzonal interference on gas test. Oil Gas Well Test. 1996, 3, 42–45. [Google Scholar]
- Wang, Y.; He, Z.X.; Wang, R.C.; Li, X.C. Determination of interlayer interference coefficient of multi-layer combined production in low permeability gas reservoir. Sci. Technol. Eng. 2012, 20, 9163–9166. [Google Scholar]
- Zhang, S.; Liu, Z.; Shi, A.; Wang, X. Development of accurate well models for numerical reservoir simulation. Adv. Geo-Energy Res. 2019, 3, 250–257. [Google Scholar] [CrossRef]
- Xian, B.; Xiong, Y.; Shi, G.X.; Li, P.C.; Chen, M. Study on interlayer interference analysis and technical countermeasures of combined production in thin reservoir. Spec. Oil Gas Reserv. 2007, 14, 51–54. [Google Scholar]
- Liu, Q.G.; Wang, H.; Shi, G.X.; Wang, R.C.; Li, X.C. Calculation method and influencing factors of stratified production contribution of multi-layer gas reservoir. J. Southwest Pet. Univ. Nat. Sci. Ed. 2010, 32, 80. [Google Scholar]
- Luo, H.; Mahiya, G.F.F.; Pannett, S. The use of rate-transient-analysis modeling to quantify uncertainties in commingled tight gas production-forecasting and decline-analysis parameters in the alberta deep basin. SPE Reserv. Eval. Eng. 2014, 17, 209–219. [Google Scholar] [CrossRef]
- Yang, X.F.; Liu, Y.C.; Jin, L.; Ying, W.; Hui, D. Effect of separate layer recovery or multilayer commingled production and the optimal selection of development methods for two-layer gas reservoirs. Nat. Gas Ind. 2012, 32, 57–60. [Google Scholar]
- Santiago, V.; Ribeiro, A.; Hurter, S. Modeling the contribution of individual coal seams on commingled gas production. SPE Prod. Oper. 2021, 36, 245–261. [Google Scholar] [CrossRef]
- Huang, S.; Kang, B.; Cheng, L.; Zhou, W.; Chang, S. Quantitative characterization of interlayer interference and productivity prediction of directional wells in the multilayer commingled production of ordinary offshore heavy oil reservoirs. Pet. Explor. Dev. 2015, 42, 533–540. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhao, L.; Cheng, L.; Wang, Z.; Yang, H. Numerical simulation of multi-seam coalbed methane production using a gray. J. Pet. Sci. Eng. 2018, 175, 587–594. [Google Scholar] [CrossRef]
- Yan, B.; Wang, Y.; Killough, J.E. Beyond dual-porosity modeling for the simulation of complex flow mechanisms in shale reservoirs. Comput. Geoences 2016, 20, 69–91. [Google Scholar] [CrossRef]
- Wang, Y.; Yan, B.; John, K. Compositional Modeling of Tight Oil Using Dynamic Nanopore Properties. In Proceedings of the SPE Annual Technical Conference and Exhibition, New Orleans, LA, USA, 30 September–2 October 2013. [Google Scholar]
- Yan, B.; Li, C.; Killough, J.E. A Fully Compositional Model Considering the Effect of Nanopores in Tight Oil Reservoirs. J. Pet. Ence Eng. 2017, 152, 675–682. [Google Scholar] [CrossRef]
- Yan, B.; Wang, Y.; Tariq, Z.; Zhang, K. Estimation of heterogeneous permeability using pressure derivative data through an inversion neural network inspired by the Fast Marching Method. Geoenergy Sci. Eng. 2023, 228, 211982. [Google Scholar] [CrossRef]
- Tariq, Z.; Gudala, M.; Yan, B.; Sun, S.; Rui, Z. Optimization of Carbon-Geo Storage into Saline Aquifers: A Coupled Hydro-Mechanics-Chemo Process. In Proceedings of the SPE EuropEC—Europe Energy Conference featured at the 84th EAGE Annual Conference & Exhibition, Vienna, Austria, 5–8 June 2023. [Google Scholar]
- Xu, Z.; Yan, B.; Gudala, M.; Rui, Z.; Tariq, Z. A Robust General Physics-Informed Machine Learning Framework for Energy Recovery Optimization in Geothermal Reservoirs. In Proceedings of the SPE EuropEC—Europe Energy Conference featured at the 84th EAGE Annual Conference & Exhibition, Vienna, Austria, 5–8 June 2023. [Google Scholar]
- Tariq, Z.; Ertugrul, U.Y.; Gudala, M.; Yan, B.; Sun, S.; Hussein, H. Spatial–temporal prediction of minerals dissolution and precipitation using deep learning techniques: An implication to Geological Carbon Sequestration. Fuel 2023, 341, 127677. [Google Scholar] [CrossRef]
- Yan, B.; Xu, Z.; Gudala, M.; Tariq, Z.; Finkbeiner, T. Reservoir Modeling and Optimization Based on Deep Learning with Application to Enhanced Geothermal Systems. In Proceedings of the SPE Reservoir Characterisation and Simulation Conference and Exhibition, Abu Dhabi, UAE, 24–26 January 2023. [Google Scholar]
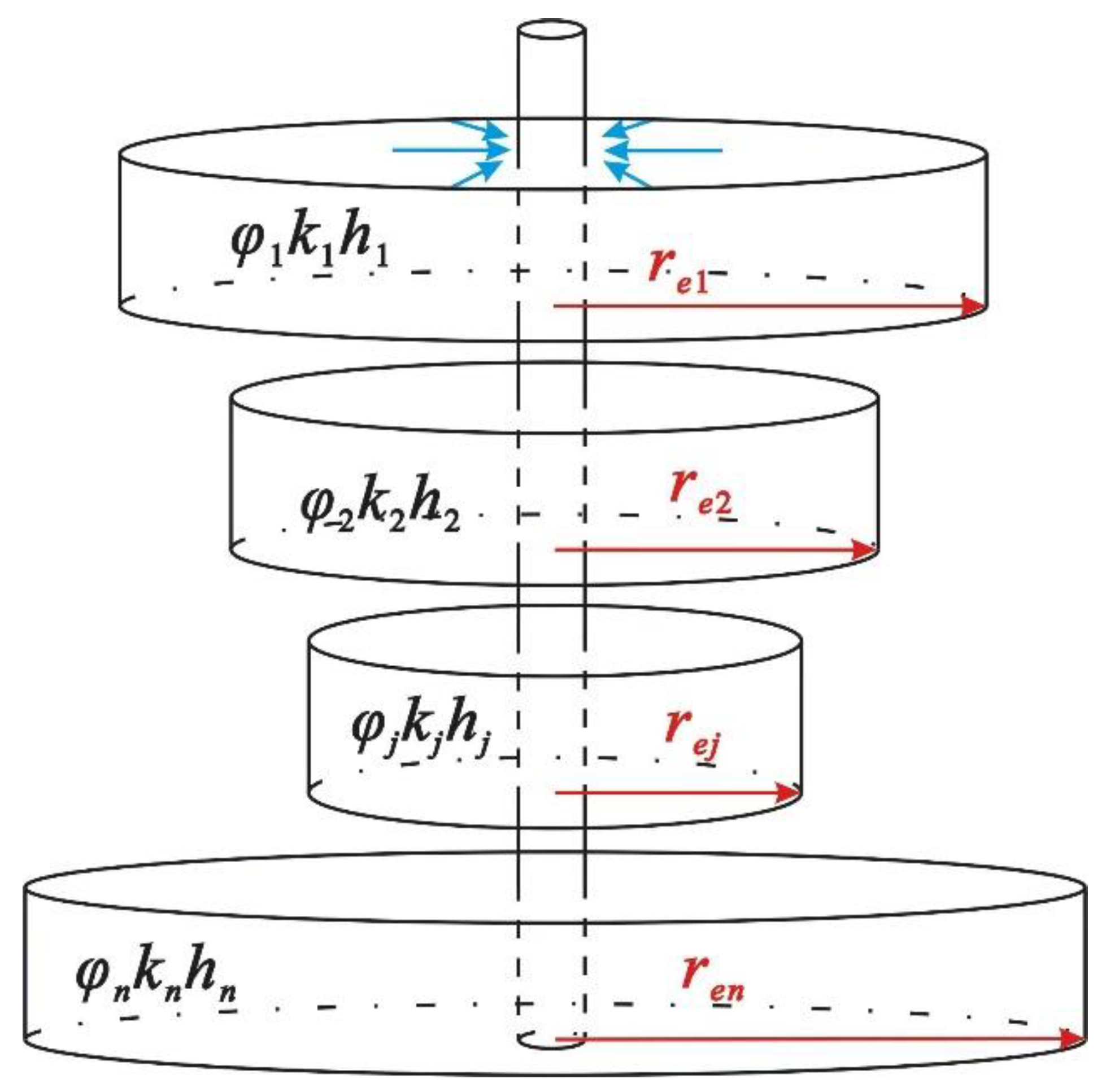
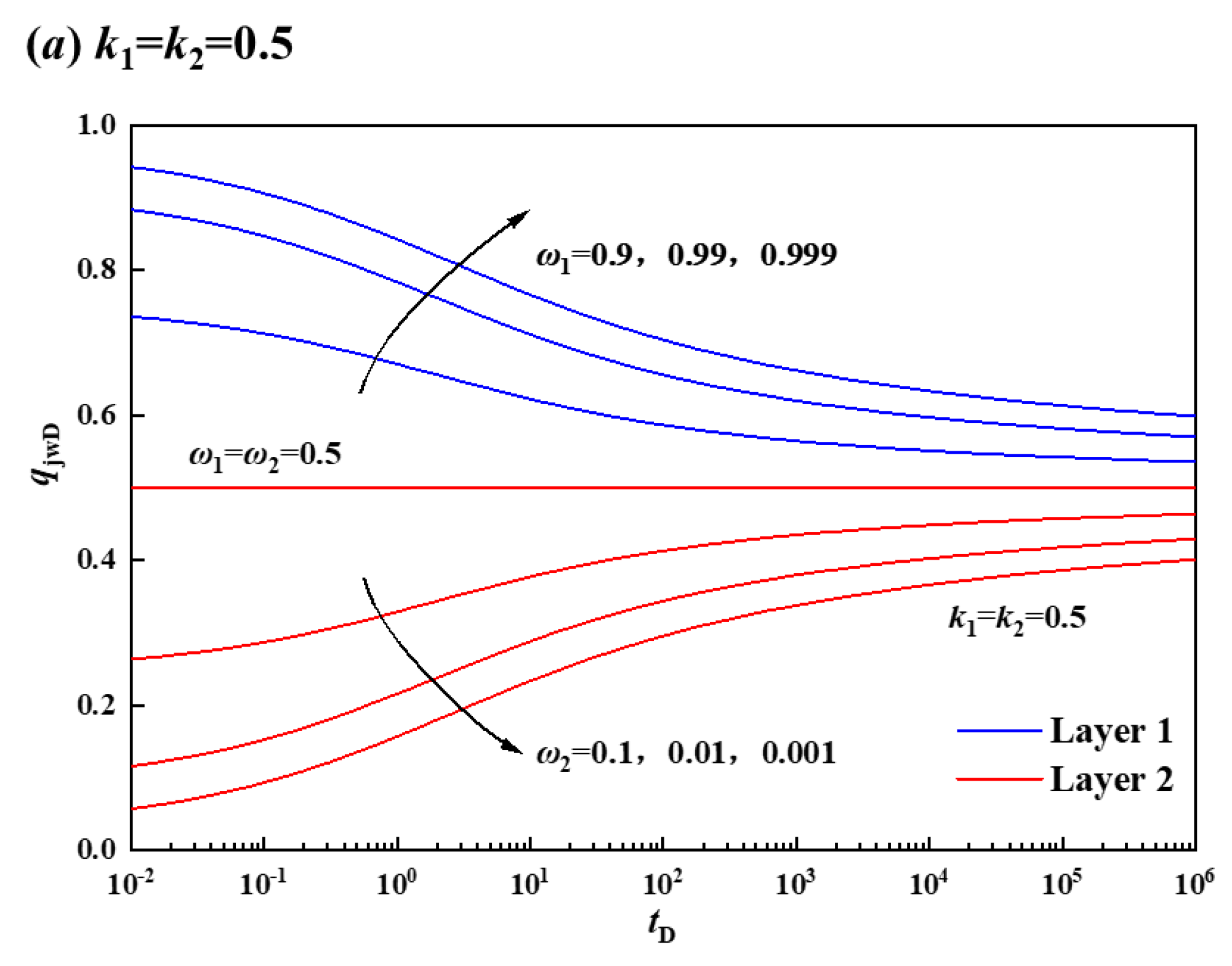
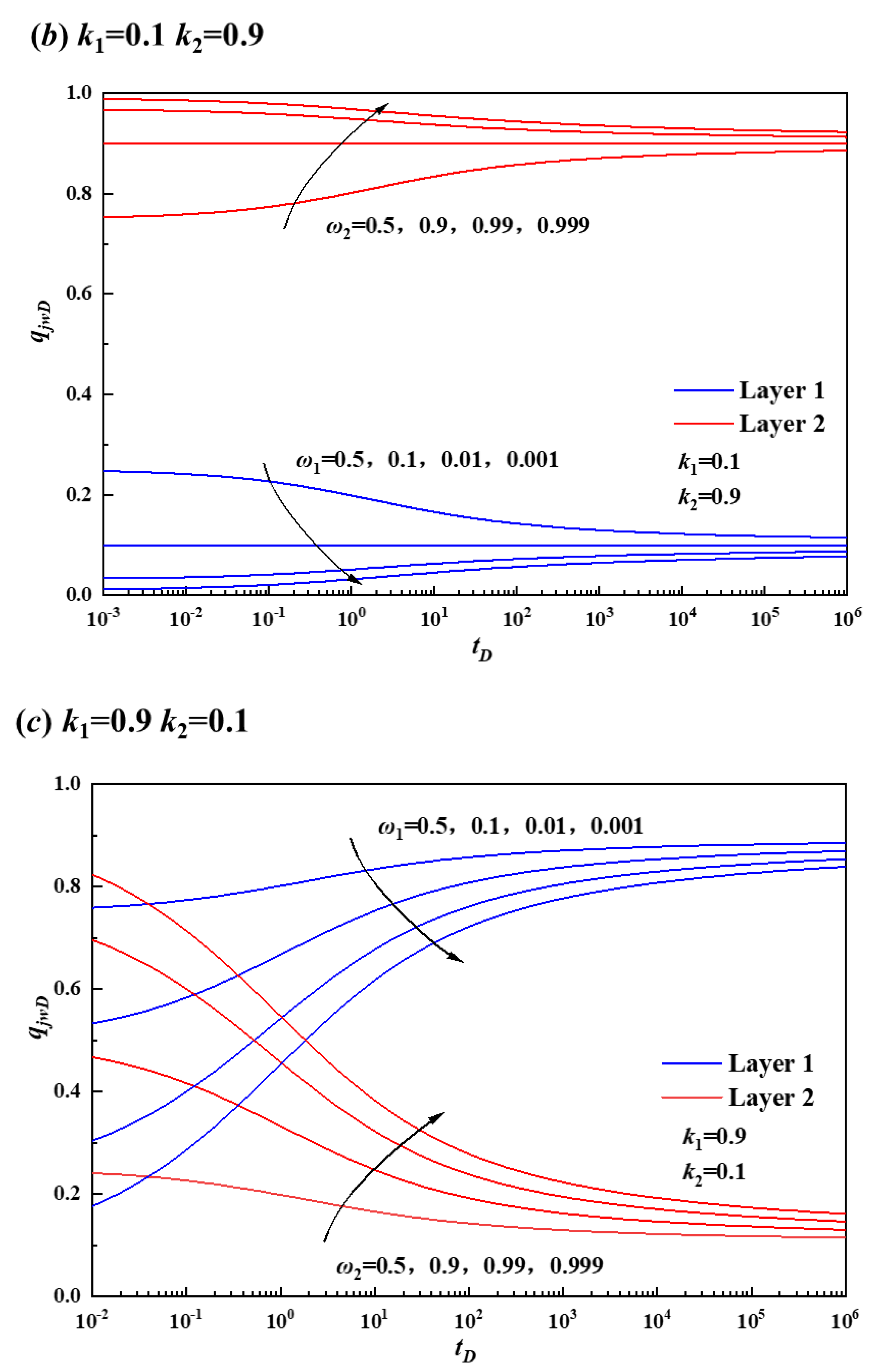
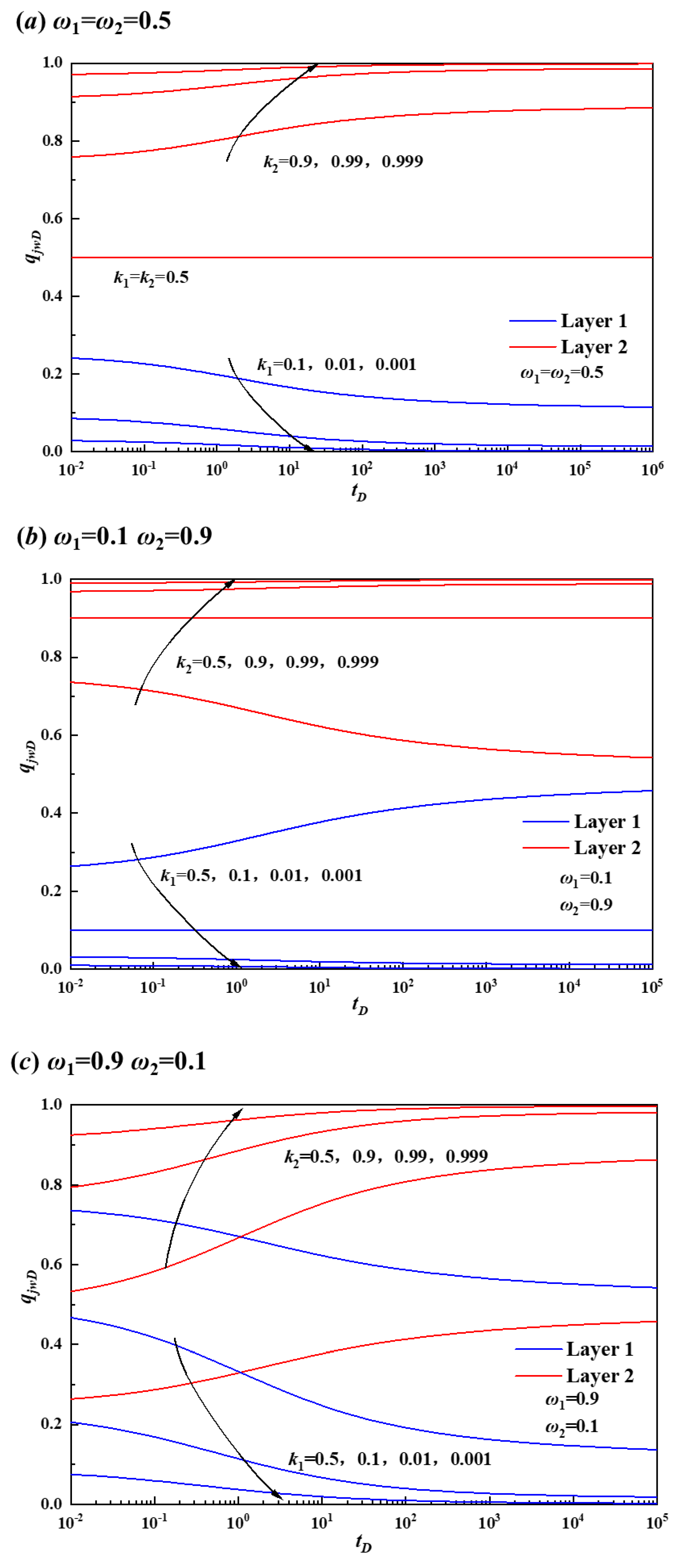




| Level | Thickness (m) | Permeability (10−3 μm2) | Porosity (%) | Initial Pressure (MPa) | Drainage Radius (m) |
|---|---|---|---|---|---|
| 1 | 2 | 2 | 8 | 17.80 | 100 |
| 2 | 4 | 4 | 9 | 17.84 | 200 |
| 3 | 6 | 6 | 10 | 17.88 | 300 |
| 4 | 8 | 8 | 11 | 17.92 | 400 |
| 5 | 10 | 10 | 12 | 17.96 | 500 |
| Thickness (m) | Porosity (%) | Initial Pressure (MPa) | Drainage Radius (m) | Permeability (10−3 μm2) | ||||
|---|---|---|---|---|---|---|---|---|
| 2 | 8 | 17.80 | 100 | 2 | 4 | 6 | 8 | 10 |
| Thickness (m) | Porosity (%) | Permeability (10−3 μm2) | Drainage Radius (m) | Initial Pressure (MPa) | ||||
|---|---|---|---|---|---|---|---|---|
| 2 | 8 | 6 | 100 | 17.8 | 17.84 | 17.88 | 17.92 | 17.96 |
| Thickness (m) | Initial Pressure (MPa) | Permeability (10−3 μm2) | Drainage Radius (m) | Porosity (%) | ||||
|---|---|---|---|---|---|---|---|---|
| 2 | 17.8 | 6 | 100 | 8 | 9 | 10 | 11 | 12 |
| Porosity (%) | Initial Pressure (MPa) | Permeability (10−3 μm2) | Drainage Radius (m) | Thickness (m) | ||||
|---|---|---|---|---|---|---|---|---|
| 8 | 17.8 | 6 | 100 | 2 | 4 | 6 | 8 | 10 |
| Porosity (%) | Initial Pressure (MPa) | Permeability (10−3 μm2) | Thickness (m) | Drainage Radius (m) | ||||
|---|---|---|---|---|---|---|---|---|
| 8 | 17.8 | 6 | 100 | 100 | 200 | 300 | 400 | 500 |
| The Case | Thickness (m) | Permeability (10−3 μm2) | Porosity (%) | Initial Pressure (MPa) | Drainage Radius (m) | Production Contribution |
|---|---|---|---|---|---|---|
| 1 | 2 | 2 | 8 | 17.8 | 100 | 6.74% |
| 2 | 2 | 4 | 9 | 17.84 | 200 | 54.53% |
| 3 | 2 | 6 | 10 | 17.88 | 300 | 24.67% |
| 4 | 2 | 8 | 11 | 17.92 | 400 | 31.18% |
| 5 | 2 | 10 | 12 | 17.96 | 500 | 36.56% |
| 6 | 4 | 2 | 9 | 17.88 | 400 | 20.21% |
| 7 | 4 | 4 | 10 | 17.92 | 500 | 32.87% |
| 8 | 4 | 6 | 11 | 17.96 | 100 | 18.59% |
| 9 | 4 | 8 | 12 | 17.8 | 200 | 40.24% |
| 10 | 4 | 10 | 8 | 17.84 | 300 | 46.90% |
| 11 | 6 | 2 | 10 | 17.96 | 200 | 26.84% |
| 12 | 6 | 4 | 11 | 17.8 | 300 | 41.26% |
| 13 | 6 | 6 | 12 | 17.84 | 400 | 51.32% |
| 14 | 6 | 8 | 08 | 17.88 | 500 | 57.22% |
| 15 | 6 | 10 | 09 | 17.92 | 100 | 20.31% |
| 16 | 8 | 2 | 11 | 17.84 | 500 | 33.86% |
| 17 | 8 | 4 | 12 | 17.88 | 100 | 31.09% |
| 18 | 8 | 6 | 8 | 17.92 | 200 | 49.14% |
| 19 | 8 | 8 | 9 | 17.96 | 300 | 61.67% |
| 20 | 8 | 10 | 10 | 17.8 | 400 | 67.88% |
| 21 | 10 | 2 | 12 | 17.92 | 300 | 39.30% |
| 22 | 10 | 4 | 8 | 17.96 | 400 | 54.40% |
| 23 | 10 | 6 | 9 | 17.8 | 500 | 63.31% |
| 24 | 10 | 8 | 10 | 17.84 | 100 | 29.62% |
| 25 | 10 | 10 | 11 | 17.88 | 200 | 63.96% |
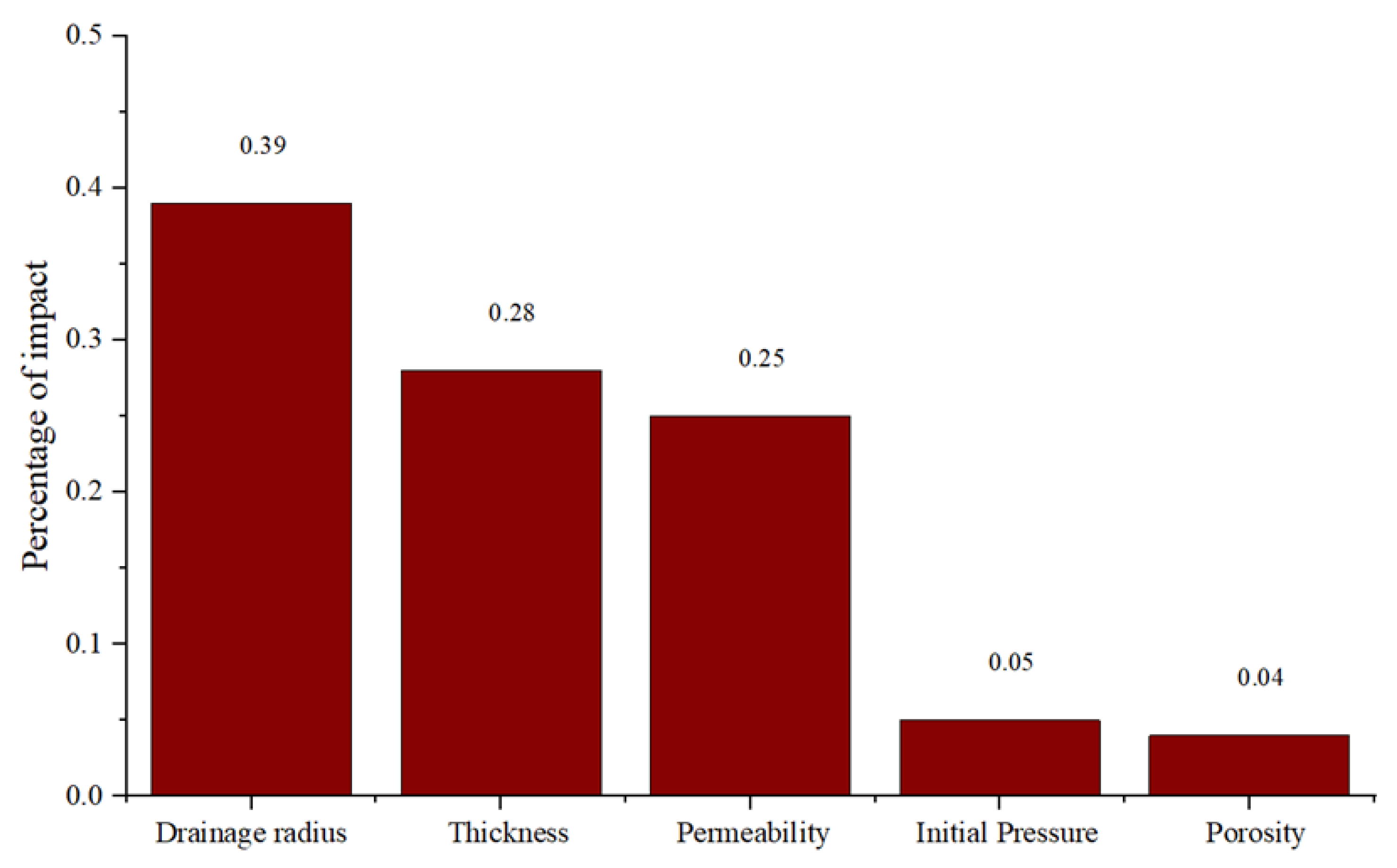
| Level | Thickness | Permeability | Porosity | Initial Pressure | Drainage Radius | |
|---|---|---|---|---|---|---|
| k value | 1 | 0.3074 | 0.2539 | 0.4288 | 0.4389 | 0.2127 |
| 2 | 0.3176 | 0.4283 | 0.4401 | 0.4325 | 0.4694 | |
| 3 | 0.3939 | 0.4141 | 0.3638 | 0.3943 | 0.4276 | |
| 4 | 0.4873 | 0.4399 | 0.3777 | 0.3456 | 0.4500 | |
| 5 | 0.5012 | 0.4712 | 0.3970 | 0.3961 | 0.4476 | |
| Range R | 0.1938 | 0.2173 | 0.0763 | 0.0933 | 0.2567 | |
| Ranking of impact degree | 3 | 2 | 5 | 4 | 1 | |
| Sources of Variance | Degrees of Freedom | Deviation Sum of Squares | Variance | F Value | p Value | Degree of Significance |
|---|---|---|---|---|---|---|
| Thickness | 4 | 0.16623 | 0.041557 | 2.84 | 0.168 | * |
| permeability | 4 | 0.14495 | 0.036237 | 2.48 | 0.200 | * |
| Porosity | 4 | 0.02121 | 0.005304 | 0.36 | 0.825 | Δ |
| Initial pressure | 4 | 0.02781 | 0.006953 | 0.48 | 0.755 | Δ |
| Drainage radius | 4 | 0.22711 | 0.056778 | 3.89 | 0.108 | * |
| Error | 4 | 0.05843 | 0.014607 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, L.; Xiang, Y.; Tao, H.; Kuang, J. An Analytical Model Coupled with Orthogonal Experimental Design Is Used to Analyze the Main Controlling Factors of Multi-Layer Commingled Gas Reservoirs. Water 2023, 15, 3052. https://doi.org/10.3390/w15173052
Wang L, Xiang Y, Tao H, Kuang J. An Analytical Model Coupled with Orthogonal Experimental Design Is Used to Analyze the Main Controlling Factors of Multi-Layer Commingled Gas Reservoirs. Water. 2023; 15(17):3052. https://doi.org/10.3390/w15173052
Chicago/Turabian StyleWang, Lei, Yangyue Xiang, Hongyan Tao, and Jiyang Kuang. 2023. "An Analytical Model Coupled with Orthogonal Experimental Design Is Used to Analyze the Main Controlling Factors of Multi-Layer Commingled Gas Reservoirs" Water 15, no. 17: 3052. https://doi.org/10.3390/w15173052
APA StyleWang, L., Xiang, Y., Tao, H., & Kuang, J. (2023). An Analytical Model Coupled with Orthogonal Experimental Design Is Used to Analyze the Main Controlling Factors of Multi-Layer Commingled Gas Reservoirs. Water, 15(17), 3052. https://doi.org/10.3390/w15173052






