A Comparative Analysis of Infiltration Models for Groundwater Recharge from Ephemeral Stream Beds: A Case Study in Al Madinah Al Munawarah Province, Saudi Arabia
Abstract
:1. Introduction
- -
- To estimate the soil hydrological parameters (such as hydraulic conductivity, soil sorptivity, and initial infiltration rates) of the ephemeral stream bed that are useful for flood prediction, groundwater recharge, and irrigation studies in the Al Madinah Al Munawarah Province;
- -
- To perform a simple statistical analysis to estimate the spatial variability of the soil parameters from the field tests and obtain an overview regarding the degree of this variability. This is important in sensitivity and uncertainty analyses in rainfall-runoff modeling;
- -
- To test the best infiltration model (Philip, Horton, Kostiakov, and Green and Ampt) suited to interpreting the infiltration process in the ephemeral stream bed, utilizing field data from double-ring infiltrometer tests;
- -
- To recommend the best one to use in hydrological modeling in the arid region of Saudi Arabia and similar regions.
2. Study Area and Data Collection
2.1. Climate
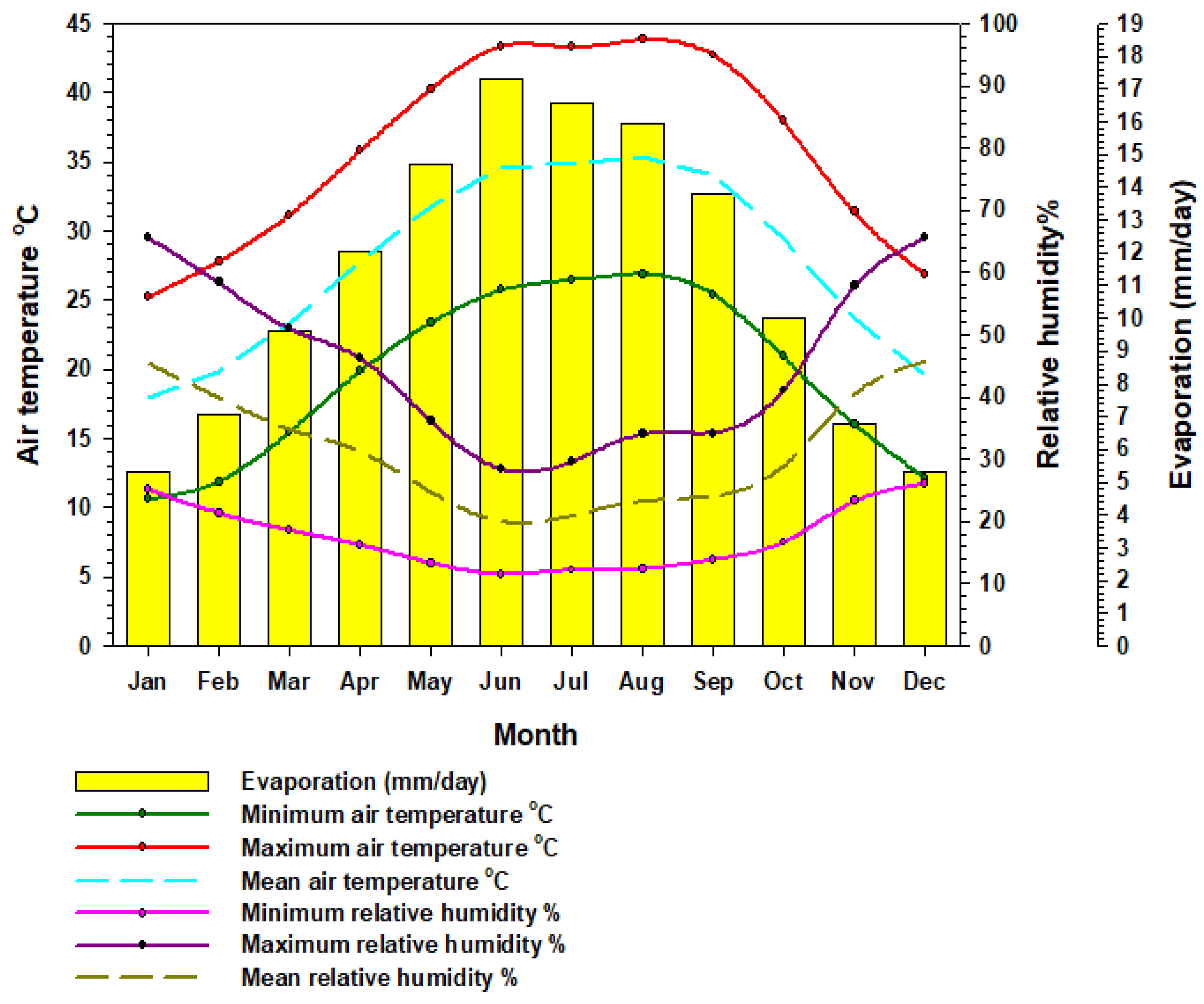
2.1.1. Air Temperature
2.1.2. Evaporation
2.1.3. Relative Humidity
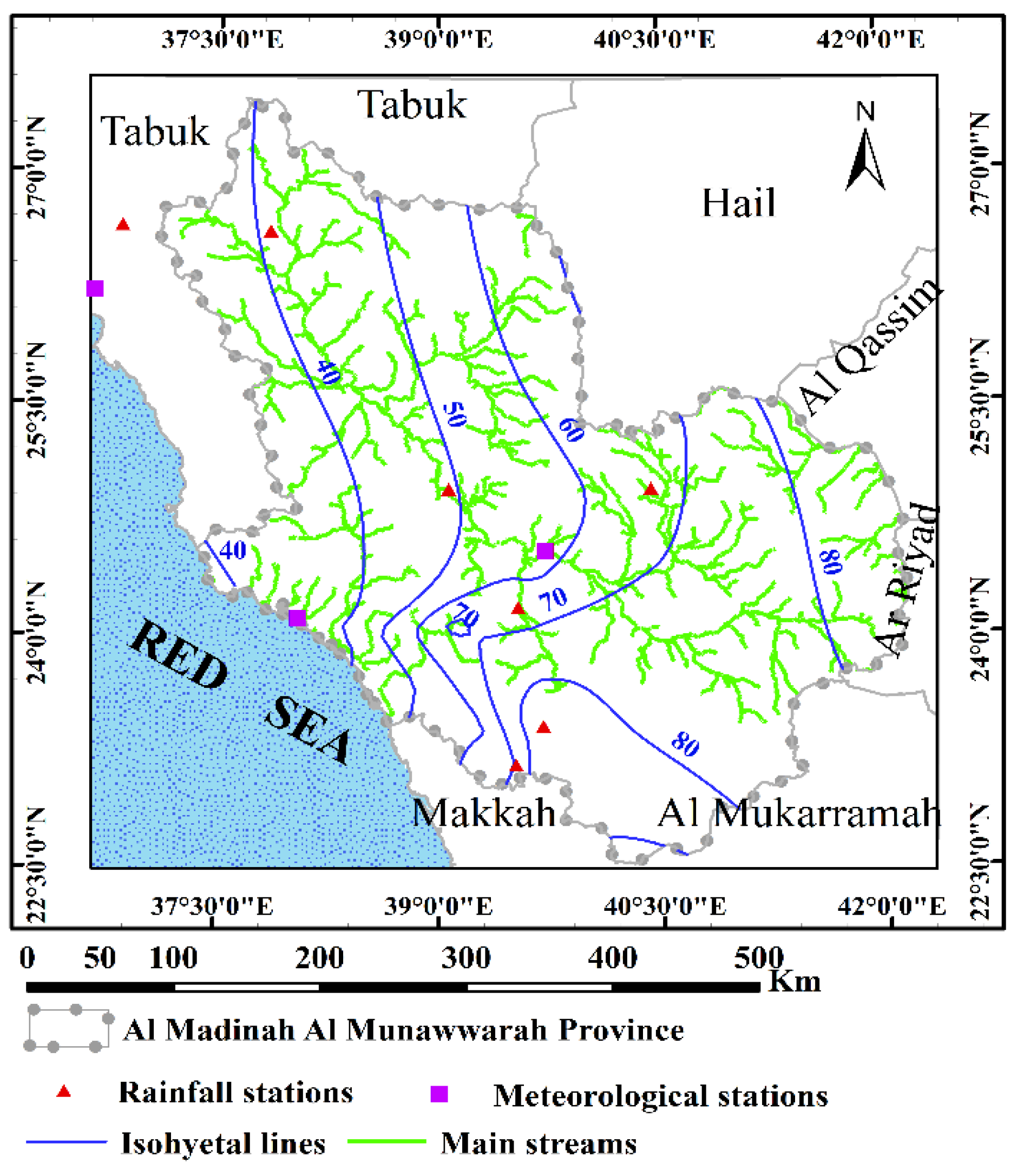
2.1.4. Rainfall
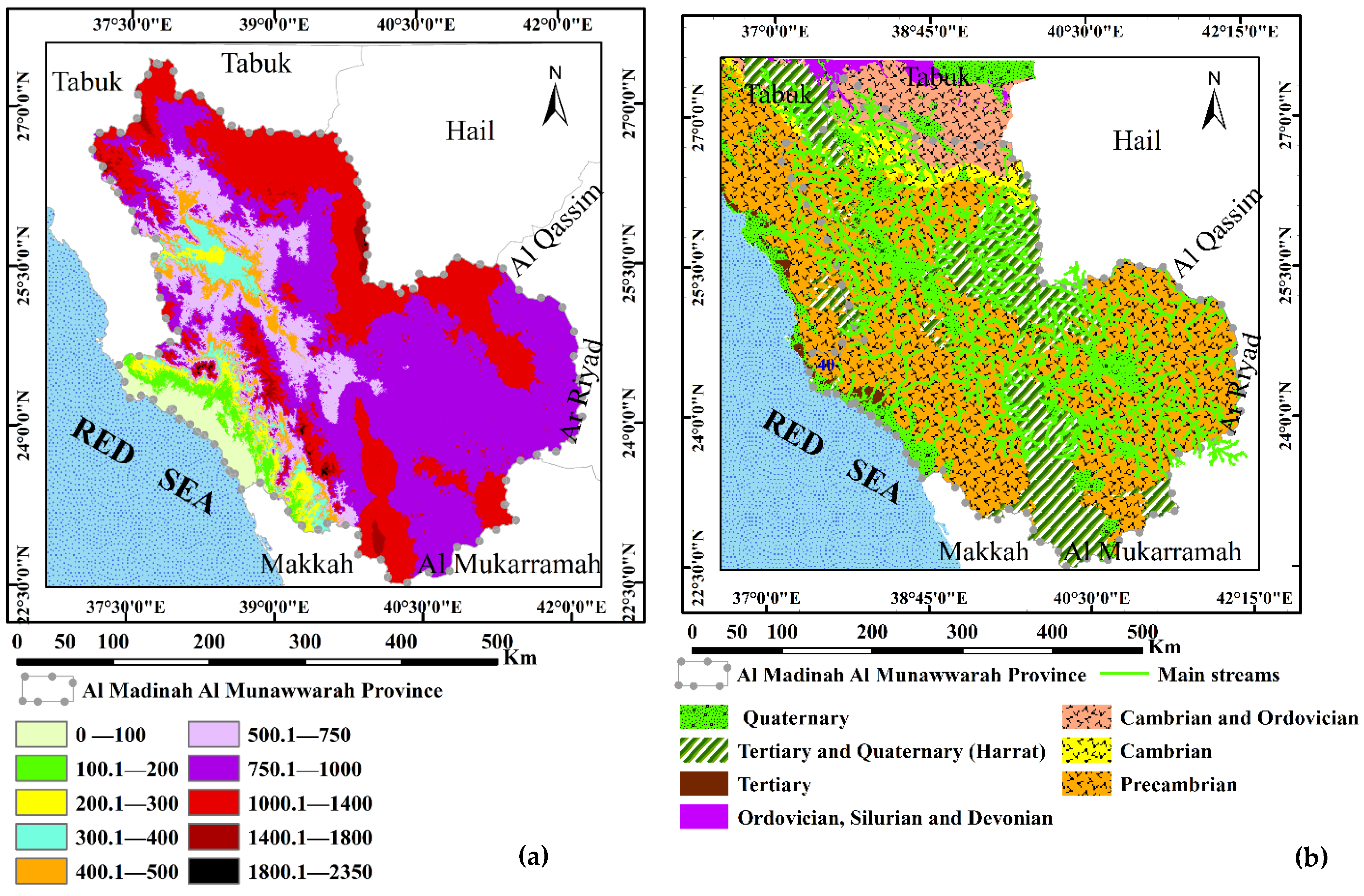
2.2. Geomorphology
- The coastal plain (lowland areas) is located between the sabkhas alongside the Red Sea shoreline and the foothills, with widths varying from 20 km to 100 km. Usually, the lowland areas are inundated by flash floods along the major valleys of the drainage basins which are cross the Red Sea’s direction. This part is characterized by alluvial deposits which are suitable for groundwater recharge of the unconfined aquifers [35,36].
- The foothills (hilly areas) extend from the coastal plain to the mountainous range, with widths ranging between 60 km and 150 km and elevations about 400 m above mean sea level. This area is gently sloping and partly plateaus, and it is composed of boulders and alluvial deposits which are characterized by high permeability for water infiltration and aquifer recharge. Most of the stream networks originate from the Hijaz mountainous series crossing the hilly areas to the coastal plain.
- The Hijaz Mountains (highland areas) extend east from the hilly areas parallel to the Red Sea and are characterized by sharply high elevations that reach 3000 m. The stream networks are initiated from these highland areas and cross toward the lowland areas. Many hydrologic basins are located in the study province, which is called a coastal basin, and draining their water toward the Red Sea.
2.3. Geology
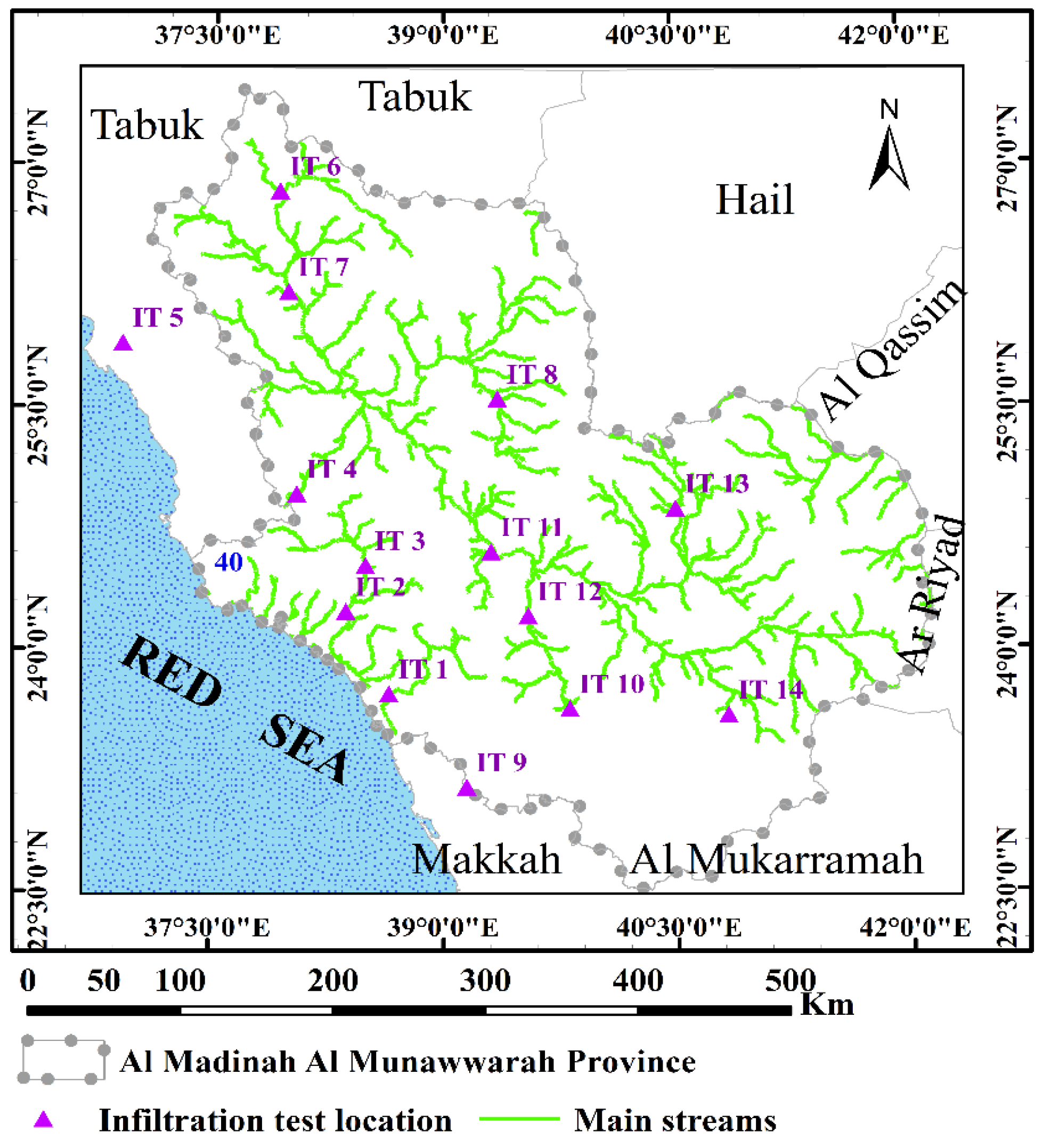
2.4. Infiltration Tests
3. Methodology
3.1. Field Infiltration Tests
3.2. Infiltration Models Used in the Analysis
3.2.1. Philip Model
3.2.2. Horton Model
3.2.3. Kostiakov Model
3.2.4. Green and Ampt Model
4. Results and Discussions
4.1. Comparison of the Various Infiltration Models
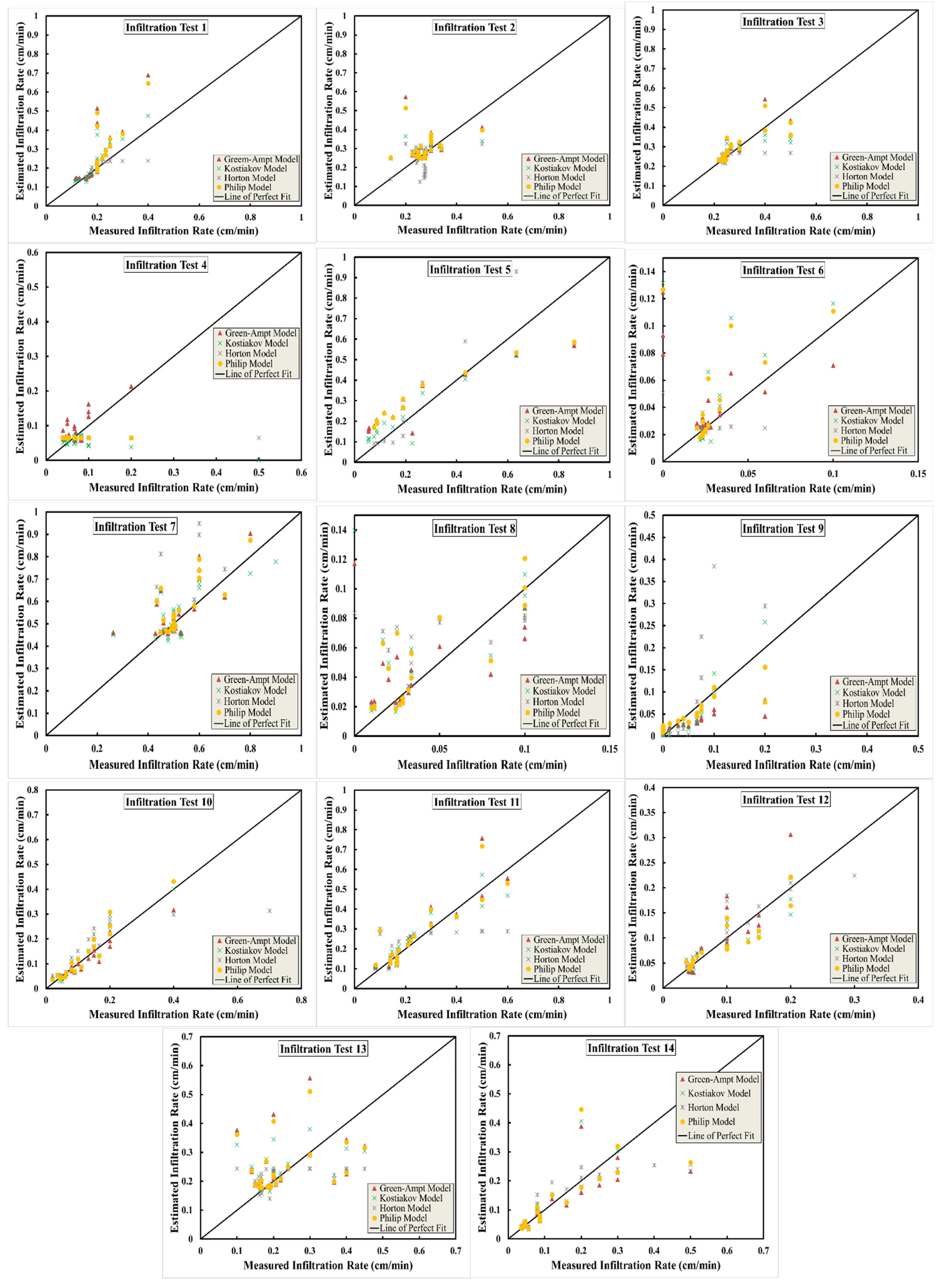
4.2. Estimated Versus Measured Infiltration Depths and Rates
5. Conclusions and Recommendations
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Garg, S.; Goel, A. Infiltration—A Critical Review. In Sustainable Engineering; Springer: Berlin/Heidelberg, Germany, 2019; pp. 111–120. [Google Scholar]
- Elfeki, A.M.; Ewea, H.A.R.; Bahrawi, J.A.; Al-Amri, N.S. Incorporating transmission losses in flash flood routing in ephemeral streams by using the three-parameter Muskingum method. Arab. J. Geosci. 2014, 8, 5153–5165. [Google Scholar] [CrossRef]
- Richards, L.A. Capillary conduction through porous mediums. Physics 1931, 1, 313–318. [Google Scholar] [CrossRef]
- Rawls, W.J.; Ahuja, L.R.; Brakensiek, D.L.; Shirmohammadi, A. Infiltration and soil water movement. In Handbook of Hydrology; McGraw-Hill, Inc.: New York, NY, USA, 1993. [Google Scholar]
- Li, W.; Pang, Y. Application of Adomian decomposition method to nonlinear systems. Adv. Differ. Equ. 2020, 1, 67. [Google Scholar] [CrossRef]
- Turkyilmazoglu, M. Nonlinear Problems via a Convergence Accelerated Decomposition Method of Adomian. J. Comput. Model. Eng. Sci. 2021, 127, 1–22. [Google Scholar] [CrossRef]
- Turkyilmazoglu, M. Accelerating the convergence of Adomian decomposition method (ADM). J. Comput. Sci. 2019, 31, 54–59. [Google Scholar] [CrossRef]
- García-Olivares, A. Analytical solution of nonlinear partial differential equations of physics. Kybernetes 2003, 32, 548–560. [Google Scholar] [CrossRef] [Green Version]
- Philip, J.R. The theory of Infiltration. Soil. Sci. 1957, 83, 345–357. [Google Scholar] [CrossRef]
- Green, W.H.; Ampt, G.A. Studies in soil physics—Part 1: The flow of air and water through soils. J. Agric. Sci. 1911, 4, 1–24. [Google Scholar]
- Horton, R.E. The role of infiltration in the hydrological cycle. EOS Trans. Am. Geophys. Union 1933, 14, 446–460. [Google Scholar] [CrossRef]
- Horton, R.E. Analysis of runoff-plot experiments with varying infiltration capacity. EOS Trans. Am. Geophys. Union 1939, 20, 693–711. [Google Scholar] [CrossRef]
- Kostiakov, A.N. On the dynamics of the coefficients of water percolation in soils and on the necessity of studying it from a dynamic point of view for purpose of amelioration. Trans. 6th Commun. Int. Soc. Soil Sci. 1932, 1, 17–21. [Google Scholar]
- Allam, M.N.; Balkhair, K.S. Case study evaluation of the geomorphologic instantaneous unit hydrograph. Water Resour. Manag. 1987, 1, 267–291. [Google Scholar] [CrossRef]
- Noor, K.; Elfeki, A.M. Development of a generalized Hayami solution for modelling of a diffusive flood wave in arid and non-arid regions. Nat. Hazards 2017, 88, 121–144. [Google Scholar] [CrossRef]
- Hayami, S. On the propagation of flood waves. Bull.-Disaster Prev. Res. Inst. Kyoto Univ. 1951, 1, 1–16. [Google Scholar]
- Elfeki, A.; Masoud, M.; Basahi, J.; Zaidi, S. A unified approach for hydrological modeling of arid catchments for flood hazards assessment: Case study of wadi Itwad, southwest of Saudi Arabia. Arab. J. Geosci. 2020, 13, 490. [Google Scholar] [CrossRef]
- Schaap, M.G.; Leij, F.J. Using neural networks to predict soil water retention and soil hydraulic conductivity. Soil Tillage Res. 1998, 47, 37–42. [Google Scholar] [CrossRef]
- Erzin, Y.; Gumaste, S.D.; Gupta, A.K.; Singh, D.N. Artificial neural network (ANN) models for determining hydraulic conductivity of compacted fine-grained soils. Can. Geotech. J. 2009, 46, 955–968. [Google Scholar] [CrossRef]
- Arshad, R.R.; Sayyad, G.; Mosaddeghi, M.; Gharabaghi, B. Predicting saturated hydraulic conductivity by artificial intelligence and regression models. ISRN Soil Sci. 2013, 2013, 308159. [Google Scholar] [CrossRef] [Green Version]
- Al-Sulaiman, M.A.; Aboukarima, A.M. Distribution of natural radionuclides in the surface soil in some areas of agriculture and grazing located in west of Riyadh, Saudi Arabia. J. Appl. Life Sci. Int. 2015, 7, 1–12. [Google Scholar] [CrossRef]
- Saito, T.; Yasuda, H.; Suganuma, H.; Inosako, K.; Abe, Y.; Kojima, T. Predicting Soil Infiltration and Horizon Thickness for a Large-Scale Water Balance Model in an Arid Environment. Water 2016, 8, 96. [Google Scholar] [CrossRef] [Green Version]
- Singh, B.; Sihag, P.; Singh, K. Modelling of impact of water quality on infiltration rate of soil by random forest regression. Modeling Earth Syst. Environ. 2017, 3, 999–1004. [Google Scholar] [CrossRef]
- Sihag, P. Prediction of unsaturated hydraulic conductivity using fuzzy logic and artificial neural network. Modeling Earth Syst. Environ. 2018, 4, 189–198. [Google Scholar] [CrossRef]
- Masoud, M.H.Z.; Basahi, J.M.; Zaidi, F.K. Assessment of artificial groundwater recharge potential through estimation of permeability values from infiltration and aquifer tests in unconsolidated alluvial formations in coastal areas. Environ. Monit. Assess 2019, 191, 31. [Google Scholar] [CrossRef] [PubMed]
- Huang, J.; Wu, P.; Zhao, X. Effects of rainfall intensity, underlying surface and slope gradient on soil infiltration under simulated rainfall experiments. Catena 2013, 104, 93–102. [Google Scholar] [CrossRef]
- Liu, Y.; Cui, Z.; Huang, Z.; López-Vicente, M.; Wu, G. Influence of soil moisture and plant roots on the soil infiltration capacity at different stages in arid grasslands of China. Catena 2019, 182, 104147. [Google Scholar] [CrossRef]
- Cheng, Y.; Yang, W.; Zhan, H.; Jiang, O.; Shi, M.; Wang, Y. On the Origin of Deep Soil Water Infiltration in the Arid Sandy Region of China. Water 2020, 12, 2409. [Google Scholar] [CrossRef]
- Almazroui, M.A.; Al Khalaf, A.K.; Abdel Basset, H.M.; Hasanean, H.M. Detecting Climate Change Signals in Saudi Arabia Using Surface Temperature; King Abdelaziz University: Jeddah, Saudi Arabia, 2009. [Google Scholar]
- Köppen, W. Das Geographische System der Klimate. In Handbuch der Klimatologie; Köppen, W., Geiger, R., Eds.; Gebrüder Borntraeger: Berlin, Germany, 1936; pp. 1–44. [Google Scholar]
- Trewartha, G.T. An Introduction to Climate, 3rd ed.; McGraw-Hill: New York, NY, USA, 1954. [Google Scholar]
- Hevesi, J.; Flint, A.; Istok, J. Precipitation estimation in mountainous terrain using multivariate geostatistics: Part 1. J. Appl. Meteorol. Climatol. 1992, 31, 661–688. [Google Scholar] [CrossRef]
- Al-Turki, S. Water Resources in Saudi Arabia with Particular Reference to Tihama Asir Province. Ph.D. Thesis, University of Durham, Durham, UK, 1995. Available online: http://core.kmi.open.ac.uk/download/pdf/9640285.pdf (accessed on 23 December 2013).
- Subyani, A.M. Topographic and seasonal influences on precipitation variability in southwest Saudi Arabia. J. King Abdulaziz Univ. 1999, 11, 89–102. [Google Scholar] [CrossRef]
- Sen, Z. Wadi Hydrology; CRC Press: Boca Raton, FL, USA, 2008; 368p. [Google Scholar]
- Abu-Alainine, H.A. Geomorphology, 5th ed.; Dar Al-Nahdah Al-Arabia: Beirut, Lebanon, 1979. [Google Scholar]
- Al-Sharif, A.S. Geography of Saudi Arabia; Dar Al Marrekh Press: Riyadh, Saudi Arabia, 1977; Volume 1. [Google Scholar]
- Burgy, R.H.; Luthin, J.N. A test of the single-and double-ring types of infiltrometers. EOS Trans. Am. Geophys. Union 1956, 37, 189–192. [Google Scholar] [CrossRef]
- Parr, J.R.; Bertrand, A.R. Water Infiltration into Soils. Adv. Agron. 1960, 12, 311–363. [Google Scholar] [CrossRef]
- Angelaki, A.; Sihag, P.; Sakellariou-Makrantonaki, M.; Tzimopoulos, C. The effect of sorptivity on cumulative infiltration. Water Supply 2021, 21, 606–614. [Google Scholar] [CrossRef]

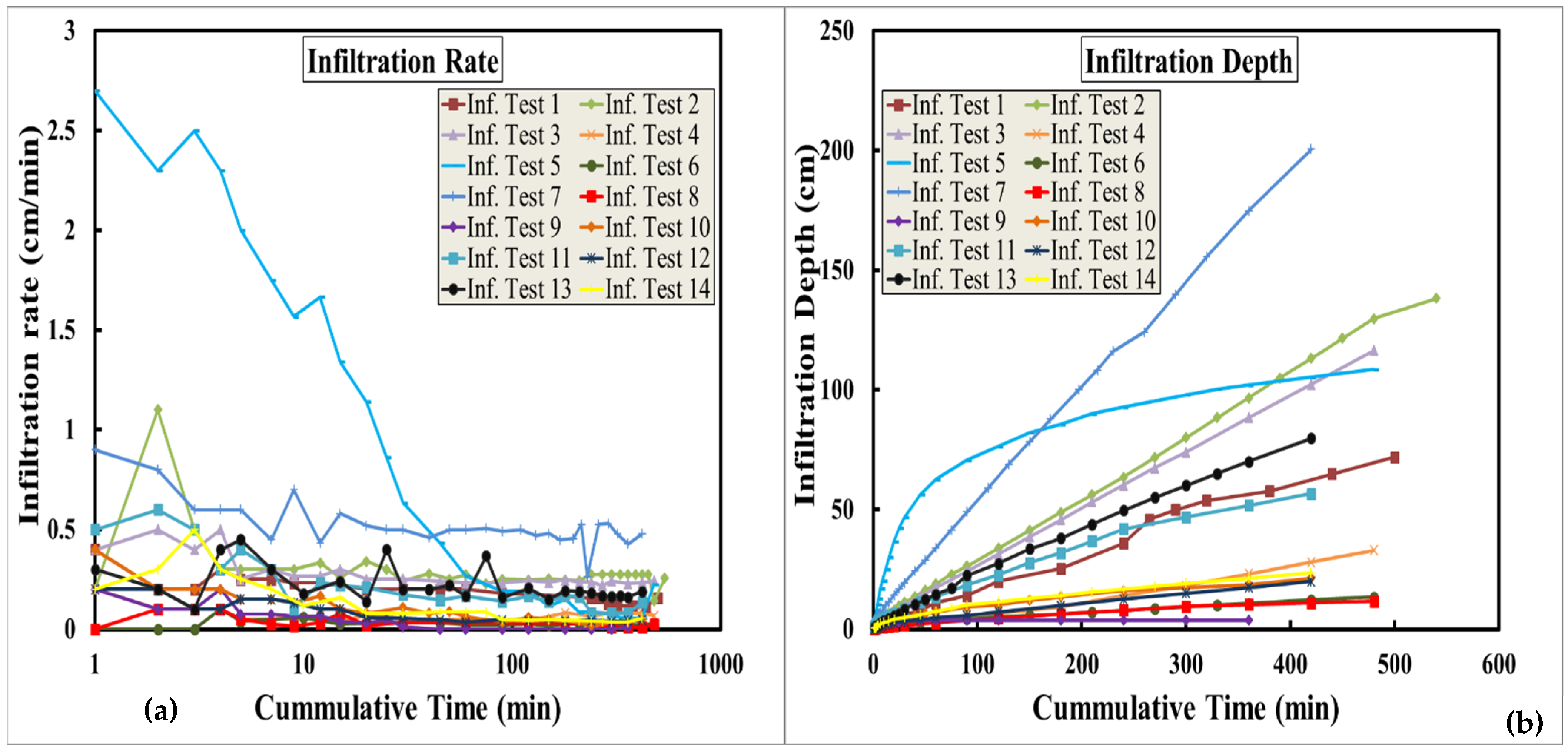


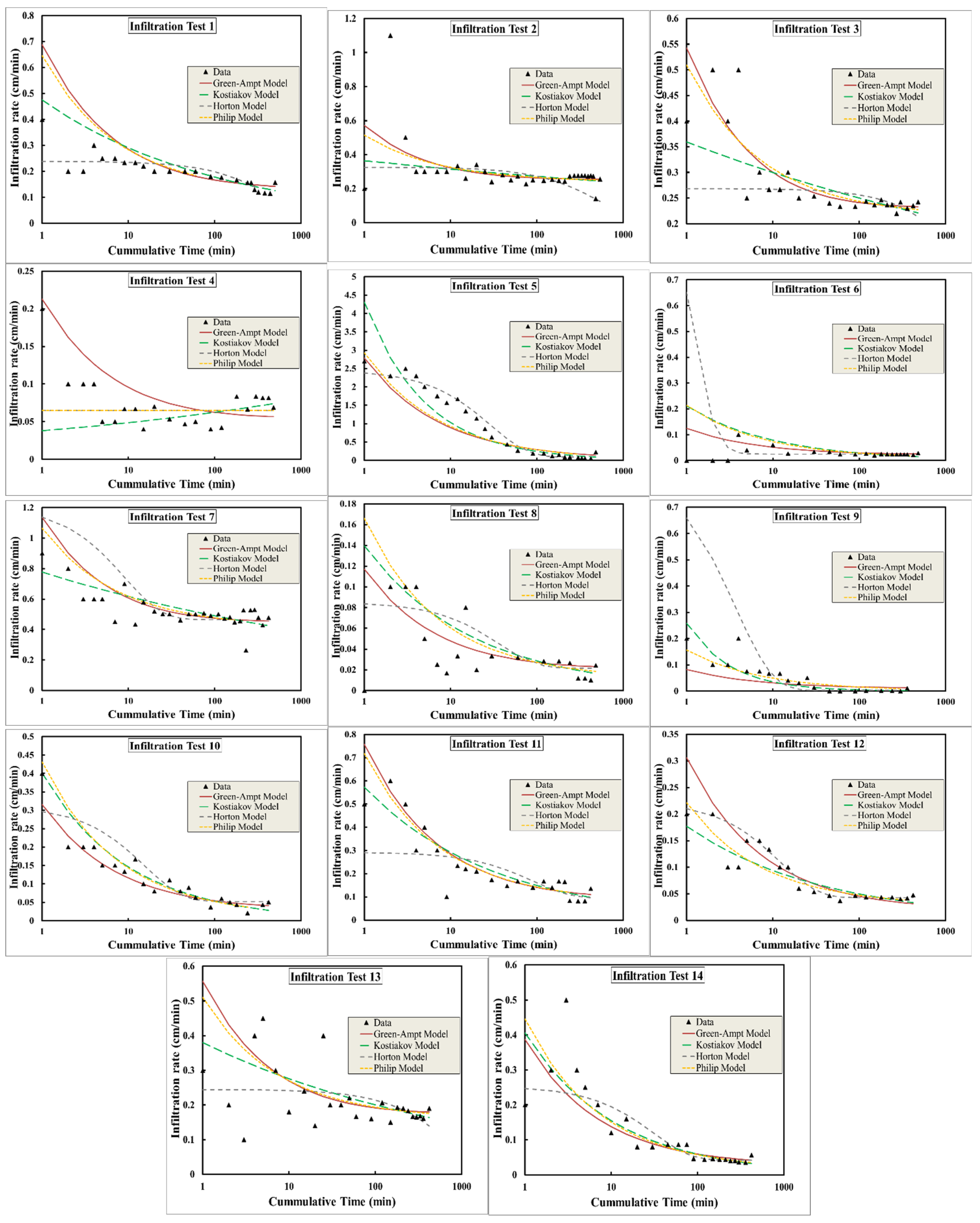
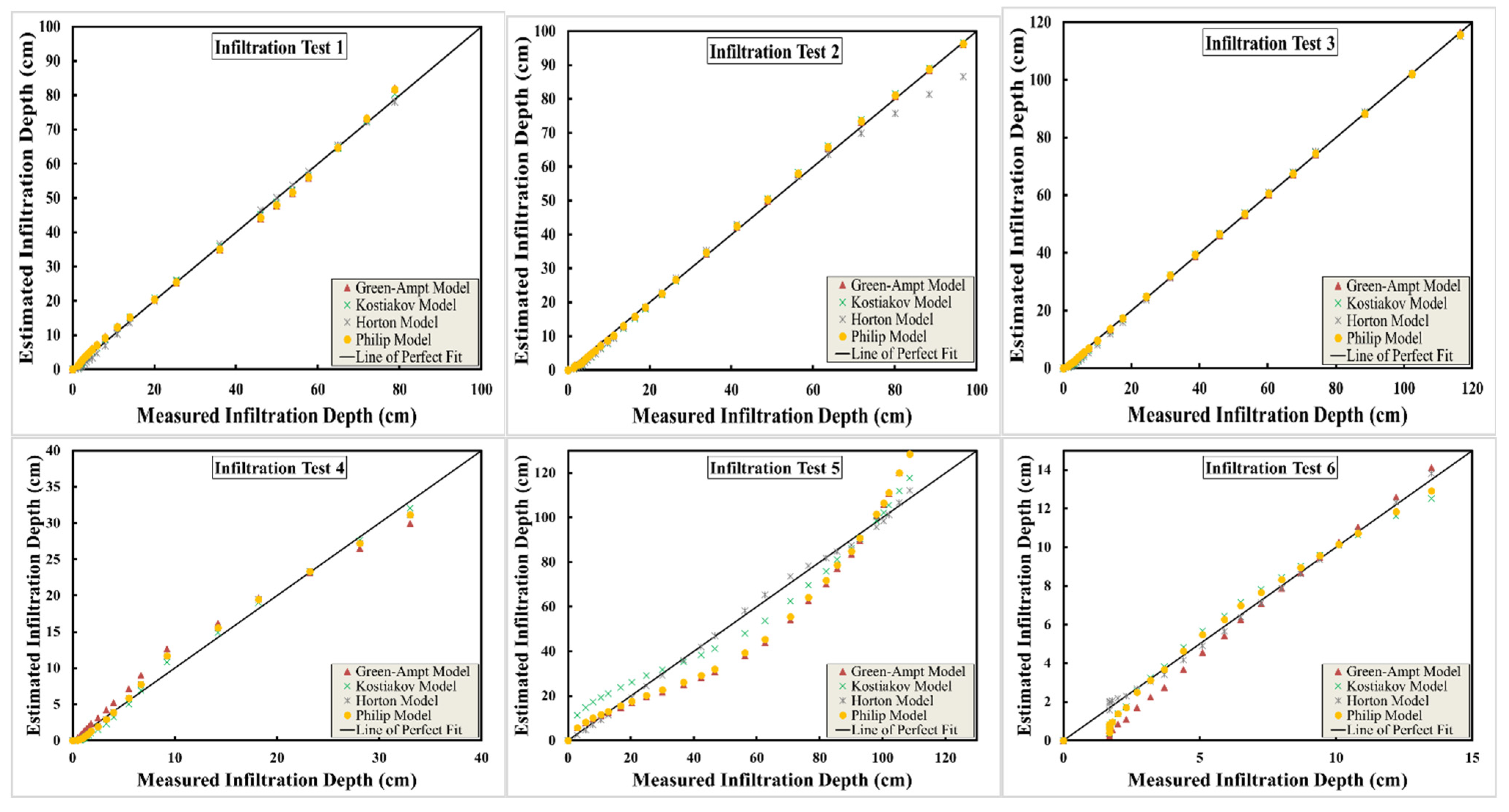
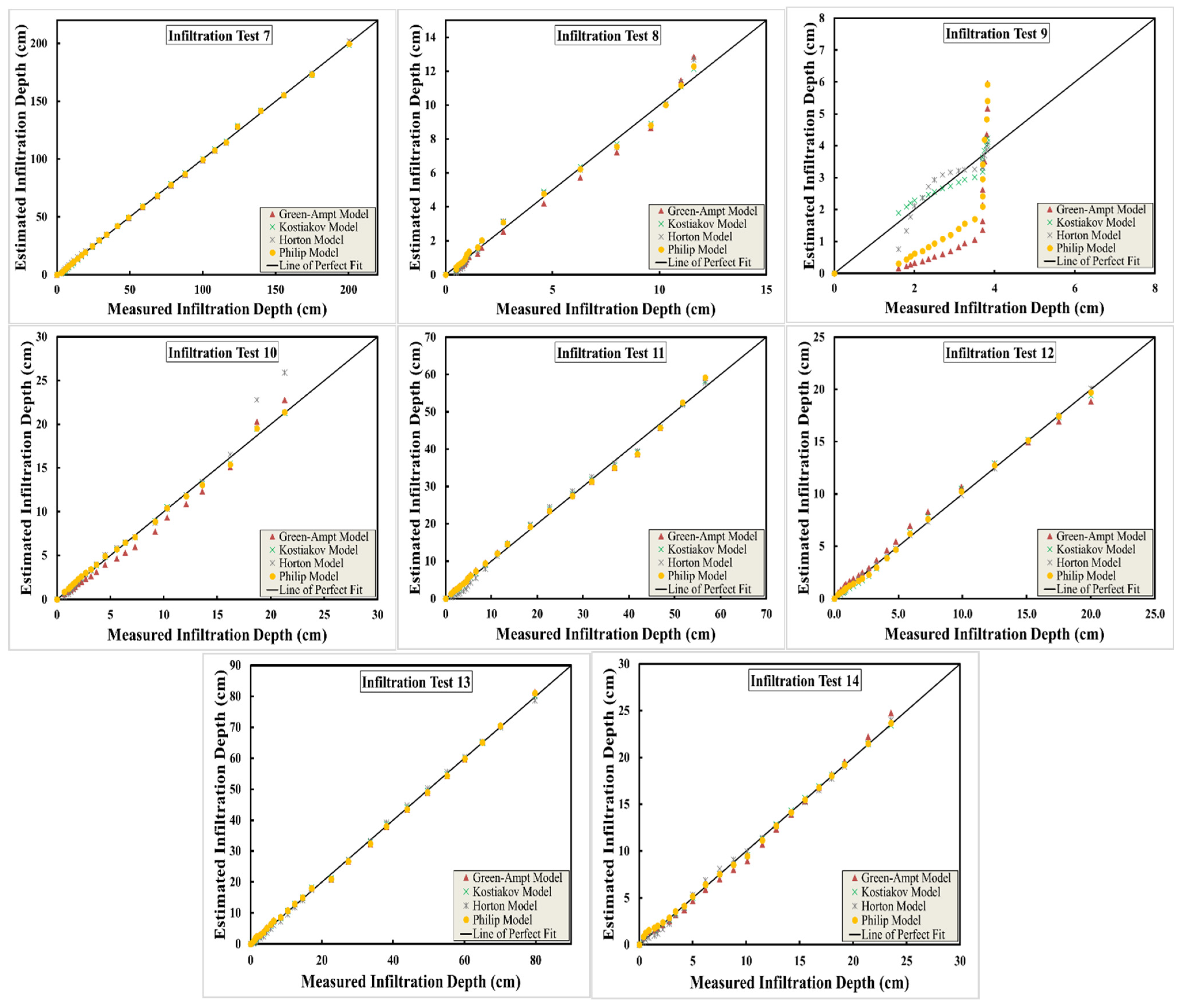
| Inf. Test No. | Horton | Philip | Kostiakov | Green-Ampt | Mean (K, cm/min) | SD (K, cm/min) | CV (K) | Max (K, cm/min) | Min (K, cm/min) | |||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| fc (cm/min) | fo (cm/min) | k (min−1) | S (cm/min.5) | Kp (cm/min) | a (cm/min b) | b | Ks (cm/min) | Ψ∆θ (cm) | ||||||
| 1 | 0.002 | 0.239 | 0.002 | 1.06 | 0.116 | 0.787 | 8.69 | 0.134 | 5.308 | 0.308 | 0.343 | 0.90 | 0.787 | 0.00200 |
| 2 | 0.003 | 0.326 | 0.002 | 0.552 | 0.238 | 0.389 | 0.94 | 0.253 | 1.21 | 0.215 | 0.160 | 1.34 | 0.389 | 0.00300 |
| 3 | 0.00026 | 0.269 | 0.00047 | 0.591 | 0.214 | 0.39 | 0.92 | 0.231 | 1.241 | 0.207 | 0.160 | 1.29 | 0.390 | 0.00026 |
| 4 | 0.00027 | 0.065 | 0.000001 | 0 | 0.065 | 0.034 | 1.10 | 0.055 | 1.127 | 0.030 | 0.023 | 1.32 | 0.055 | 0.00027 |
| 5 | 0.091 | 2.463 | 0.035 | 5.86 | 0 | 11.398 | 0.38 | 0.023 | 680.1 | 3.837 | 5.346 | 0.72 | 11.398 | 0.02300 |
| 6 | 0.025 | 3.11 | 1.594 | 0.411 | 0.008 | 0.371 | 0.57 | 0.024 | 0.985 | 0.140 | 0.163 | 0.86 | 0.371 | 0.02400 |
| 7 | 0.465 | 1.216 | 0.11 | 1.307 | 0.412 | 0.864 | 0.9 | 0.449 | 2.989 | 0.593 | 0.192 | 3.09 | 0.864 | 0.44900 |
| 8 | 0.022 | 0.085 | 0.027 | 0.309 | 0.012 | 0.213 | 0.65 | 0.021 | 0.986 | 0.085 | 0.090 | 0.95 | 0.213 | 0.02100 |
| 9 | 0.003 | 0.863 | 0.271 | 0.312 | 0 | 1.897 | 0.14 | 0.011 | 0.981 | 0.637 | 0.891 | 0.71 | 1.897 | 0.00300 |
| 10 | 0.052 | 0.313 | 0.064 | 0.843 | 0.009 | 0.713 | 0.56 | 0.033 | 5.12 | 0.266 | 0.316 | 0.84 | 0.713 | 0.03300 |
| 11 | 0.095 | 0.293 | 0.011 | 1.278 | 0.078 | 0.81 | 0.71 | 0.094 | 10.221 | 0.333 | 0.337 | 0.99 | 0.810 | 0.09400 |
| 12 | 0.042 | 0.225 | 0.082 | 0.387 | 0.029 | 0.245 | 0.72 | 0.022 | 7.622 | 0.103 | 0.101 | 1.02 | 0.245 | 0.02200 |
| 13 | 0.002 | 0.245 | 0.001 | 0.704 | 0.159 | 0.441 | 0.86 | 0.175 | 2.16 | 0.206 | 0.181 | 1.14 | 0.441 | 0.00200 |
| 14 | 0.041 | 0.254 | 0.032 | 0.864 | 0.014 | 0.697 | 0.58 | 0.031 | 8.609 | 0.256 | 0.312 | 0.82 | 0.697 | 0.03100 |
| Max | 0.47 | 3.11 | 1.59 | 5.86 | 0.41 | 11.40 | 8.69 | 0.45 | 680.06 | 3.84 | 5.35 | 3.09 | 11.40 | 0.45 |
| Min | 0.00026 | 0.07 | 0.000001 | 0.00 | 0.00 | 0.03 | 0.14 | 0.01 | 0.98 | 0.03 | 0.02 | 0.71 | 0.06 | 0.00026 |
| Mean | 0.06 | 0.71 | 0.16 | 1.03 | 0.10 | 1.37 | 1.27 | 0.11 | 52.04 | 0.52 | 0.62 | 1.14 | 1.38 | 0.05 |
| SD | 0.12 | 0.91 | 0.40 | 1.39 | 0.12 | 2.81 | 2.07 | 0.12 | 174.21 | 0.94 | 1.33 | 0.58 | 2.81 | 0.11 |
| CV | 1.93 | 1.27 | 2.53 | 1.34 | 1.21 | 2.05 | 1.64 | 1.10 | 3.35 | 1.82 | 2.16 | 0.50 | 2.04 | 2.24 |
| Inf. Test No. | Horton | Philip | Kostiakov | Green-Ampt | Max R and Min RMSE | Best Model | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| f (cm/min) | F (cm) | f (cm/min) | F (cm) | f (cm/min) | F (cm) | f (cm/min) | F (cm) | f (cm/min) | F (cm) | ||||||||||||
| R | RMSE | R | RMSE | R | RMSE | R | RMSE | R | RMSE | R | RMSE | R | RMSE | R | RMSE | R | RMSE | R | RMSE | ||
| 1 | 0.319 | 0.263 | 0.999 | 0.936 | 0.718 | 0.096 | 0.998 | 1.210 | 0.850 | 0.060 | 0.990 | 0.790 | 0.830 | 0.107 | 0.990 | 1.330 | 0.850 | 0.060 | 0.999 | 0.790 | Kostiakov |
| 2 | 0.294 | 0.178 | 0.994 | 8.680 | 0.468 | 0.157 | 0.999 | 1.390 | 0.452 | 0.164 | 0.999 | 1.674 | 0.460 | 0.157 | 0.999 | 1.288 | 0.468 | 0.157 | 0.999 | 1.288 | Philip/Green-Ampt |
| 3 | 0.325 | 0.079 | 1.000 | 1.401 | 0.816 | 0.047 | 1.000 | 0.563 | 0.775 | 0.056 | 1.000 | 1.020 | 0.811 | 0.049 | 0.999 | 0.411 | 0.816 | 0.047 | 1.000 | 0.411 | Philip |
| 4 | 0.156 | 0.034 | 0.995 | 0.951 | 0.000 | 0.034 | 0.995 | 0.951 | −0.781 | 0.042 | 0.998 | 0.803 | 0.732 | 0.032 | 0.991 | 1.399 | 0.732 | 0.032 | 0.998 | 0.803 | Kostiakov/Green-Ampt |
| 5 | 0.984 | 0.165 | 0.999 | 1.684 | 0.922 | 0.450 | 0.973 | 9.986 | 0.898 | 0.457 | 0.987 | 6.374 | 0.932 | 0.442 | 0.968 | 10.751 | 0.984 | 0.165 | 0.999 | 1.684 | Horton |
| 6 | 0.973 | 0.144 | 0.999 | 0.184 | −0.107 | 0.064 | 0.993 | 0.566 | −0.080 | 0.064 | 0.989 | 0.647 | −0.119 | 0.038 | 0.997 | 0.853 | 0.973 | 0.038 | 0.999 | 0.184 | Horton |
| 7 | 0.623 | 0.171 | 1.000 | 1.416 | 0.868 | 0.088 | 1.000 | 1.154 | 0.738 | 0.089 | 0.999 | 1.706 | 0.830 | 0.106 | 0.999 | 1.122 | 0.868 | 0.088 | 1.000 | 1.122 | Philip |
| 8 | 0.420 | 0.030 | 0.996 | 0.441 | 0.394 | 0.042 | 0.997 | 0.316 | 0.448 | 0.037 | 0.994 | 0.281 | 0.384 | 0.032 | 0.995 | 0.479 | 0.448 | 0.030 | 0.997 | 0.281 | Kostiakov |
| 9 | 0.753 | 0.153 | 0.953 | 0.318 | 0.892 | 0.031 | 0.774 | 1.445 | 0.842 | 0.034 | 0.961 | 0.281 | 0.890 | 0.050 | 0.744 | 1.830 | 0.892 | 0.031 | 0.961 | 0.281 | Kostiakov/Philip |
| 10 | 0.770 | 0.047 | 0.992 | 1.378 | 0.960 | 0.032 | 0.998 | 0.352 | 0.958 | 0.030 | 0.999 | 0.280 | 0.962 | 0.027 | 0.993 | 0.916 | 0.962 | 0.027 | 0.999 | 0.280 | Kostiakov/Green-Ampt |
| 11 | 0.591 | 0.105 | 0.998 | 1.329 | 0.885 | 0.070 | 0.998 | 1.113 | 0.893 | 0.063 | 0.999 | 0.941 | 0.897 | 0.076 | 0.998 | 1.180 | 0.897 | 0.063 | 0.999 | 0.941 | Kostiakov |
| 12 | 0.900 | 0.025 | 1.000 | 0.066 | 0.899 | 0.022 | 0.999 | 0.220 | 0.910 | 0.023 | 0.993 | 0.360 | 0.901 | 0.035 | 0.998 | 0.574 | 0.910 | 0.022 | 1.000 | 0.066 | Horton |
| 13 | 0.403 | 0.085 | 0.785 | 1.000 | 0.339 | 0.104 | 0.999 | 0.673 | 0.405 | 0.088 | 0.999 | 0.448 | 0.320 | 0.111 | 0.999 | 0.801 | 0.405 | 0.085 | 0.999 | 0.448 | Kostiakov |
| 14 | 0.858 | 0.063 | 0.999 | 0.352 | 0.774 | 0.073 | 0.999 | 0.299 | 0.795 | 0.069 | 1.000 | 0.215 | 0.773 | 0.073 | 0.998 | 0.541 | 0.858 | 0.063 | 1.000 | 0.215 | Horton |
| Max | 0.98 | 0.26 | 1.00 | 8.68 | 0.96 | 0.45 | 1.00 | 9.99 | 0.96 | 0.46 | 1.00 | 6.37 | 0.96 | 0.44 | 1.00 | 10.75 | |||||
| Min | 0.16 | 0.03 | 0.79 | 0.07 | −0.11 | 0.02 | 0.77 | 0.22 | −0.78 | 0.02 | 0.96 | 0.22 | −0.12 | 0.03 | 0.74 | 0.41 | |||||
| Mean | 0.60 | 0.11 | 0.98 | 1.44 | 0.63 | 0.09 | 0.98 | 1.45 | 0.58 | 0.09 | 0.99 | 1.13 | 0.69 | 0.10 | 0.98 | 1.68 | |||||
| SD | 0.27 | 0.07 | 0.06 | 2.07 | 0.34 | 0.10 | 0.06 | 2.40 | 0.47 | 0.11 | 0.01 | 1.53 | 0.30 | 0.10 | 0.06 | 2.55 | |||||
| CV | 0.45 | 0.62 | 0.06 | 1.44 | 0.54 | 1.12 | 0.06 | 1.66 | 0.81 | 1.17 | 0.01 | 1.35 | 0.44 | 1.08 | 0.07 | 1.52 | |||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Niyazi, B.; Masoud, M.; Elfeki, A.; Rajmohan, N.; Alqarawy, A.; Rashed, M. A Comparative Analysis of Infiltration Models for Groundwater Recharge from Ephemeral Stream Beds: A Case Study in Al Madinah Al Munawarah Province, Saudi Arabia. Water 2022, 14, 1686. https://doi.org/10.3390/w14111686
Niyazi B, Masoud M, Elfeki A, Rajmohan N, Alqarawy A, Rashed M. A Comparative Analysis of Infiltration Models for Groundwater Recharge from Ephemeral Stream Beds: A Case Study in Al Madinah Al Munawarah Province, Saudi Arabia. Water. 2022; 14(11):1686. https://doi.org/10.3390/w14111686
Chicago/Turabian StyleNiyazi, Burhan, Milad Masoud, Amro Elfeki, Natarajan Rajmohan, Abdulaziz Alqarawy, and Mohamed Rashed. 2022. "A Comparative Analysis of Infiltration Models for Groundwater Recharge from Ephemeral Stream Beds: A Case Study in Al Madinah Al Munawarah Province, Saudi Arabia" Water 14, no. 11: 1686. https://doi.org/10.3390/w14111686
APA StyleNiyazi, B., Masoud, M., Elfeki, A., Rajmohan, N., Alqarawy, A., & Rashed, M. (2022). A Comparative Analysis of Infiltration Models for Groundwater Recharge from Ephemeral Stream Beds: A Case Study in Al Madinah Al Munawarah Province, Saudi Arabia. Water, 14(11), 1686. https://doi.org/10.3390/w14111686







