Innovative Approaches to RC Deep Beam Strengthening: Evaluating Low-Cost Glass Fiber Wraps Against Traditional CFRP Solutions
Abstract
1. Introduction
2. Experimental Program
2.1. Summary of Tested Deep Beams
2.2. Details of Deep Beams
2.3. Material Properties
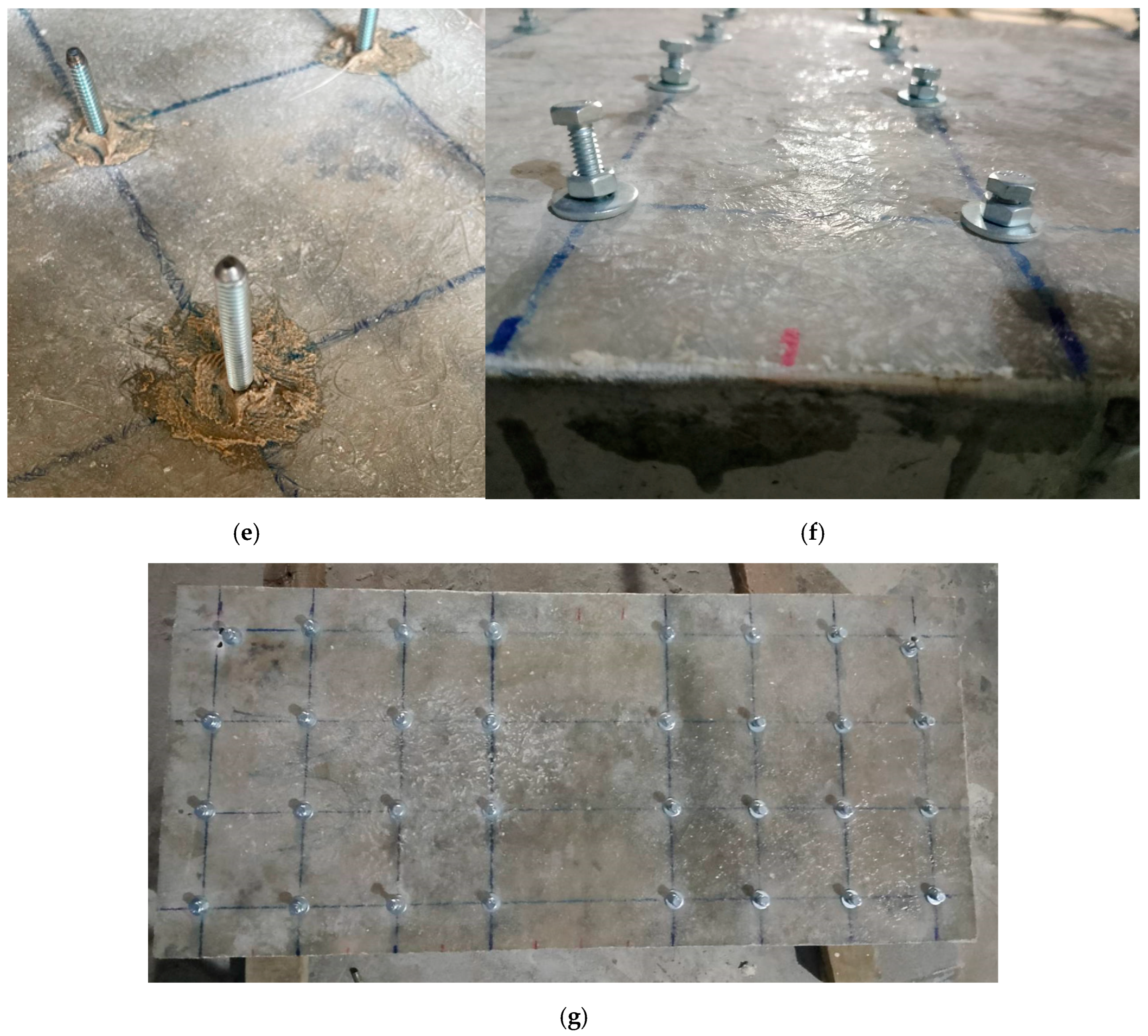
2.4. Construction and Strengthening of Beams
2.5. Test Setup
3. Experimental Results
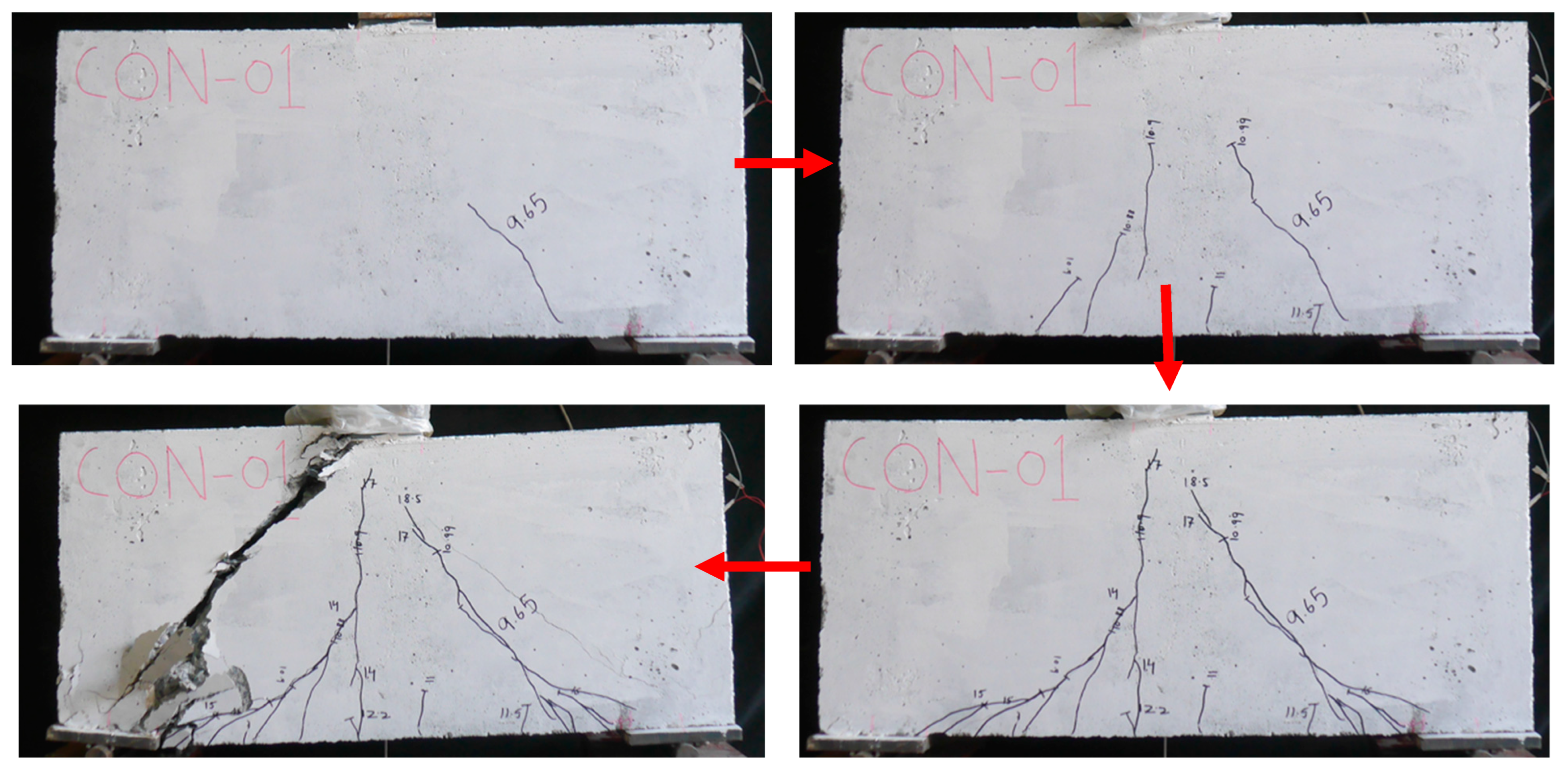
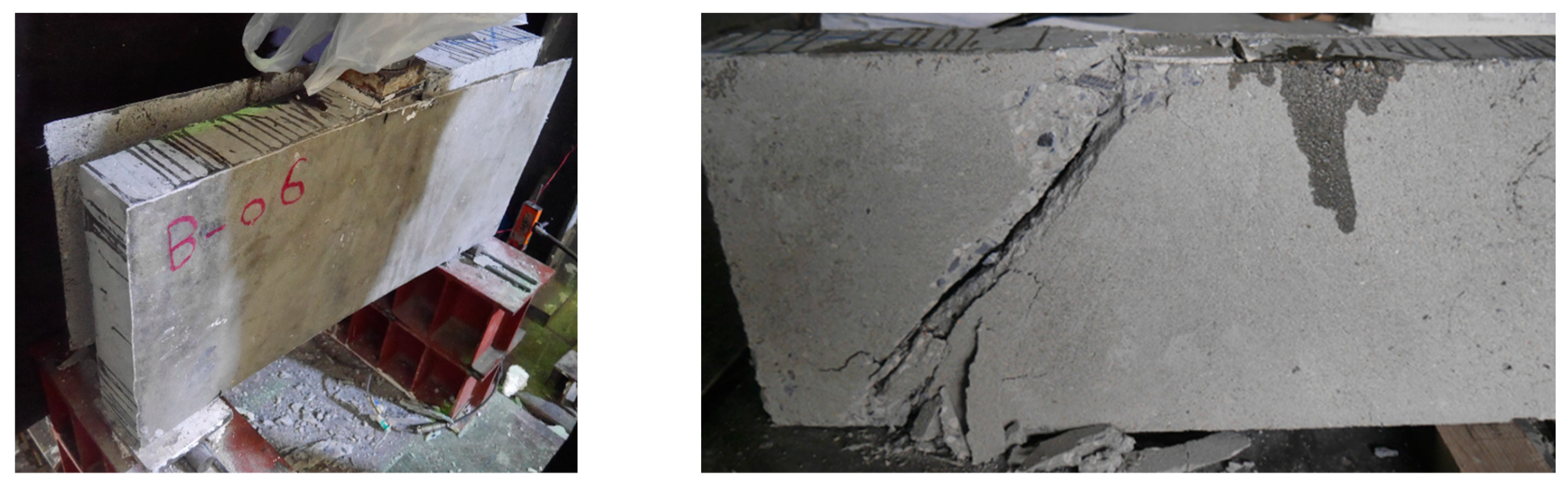
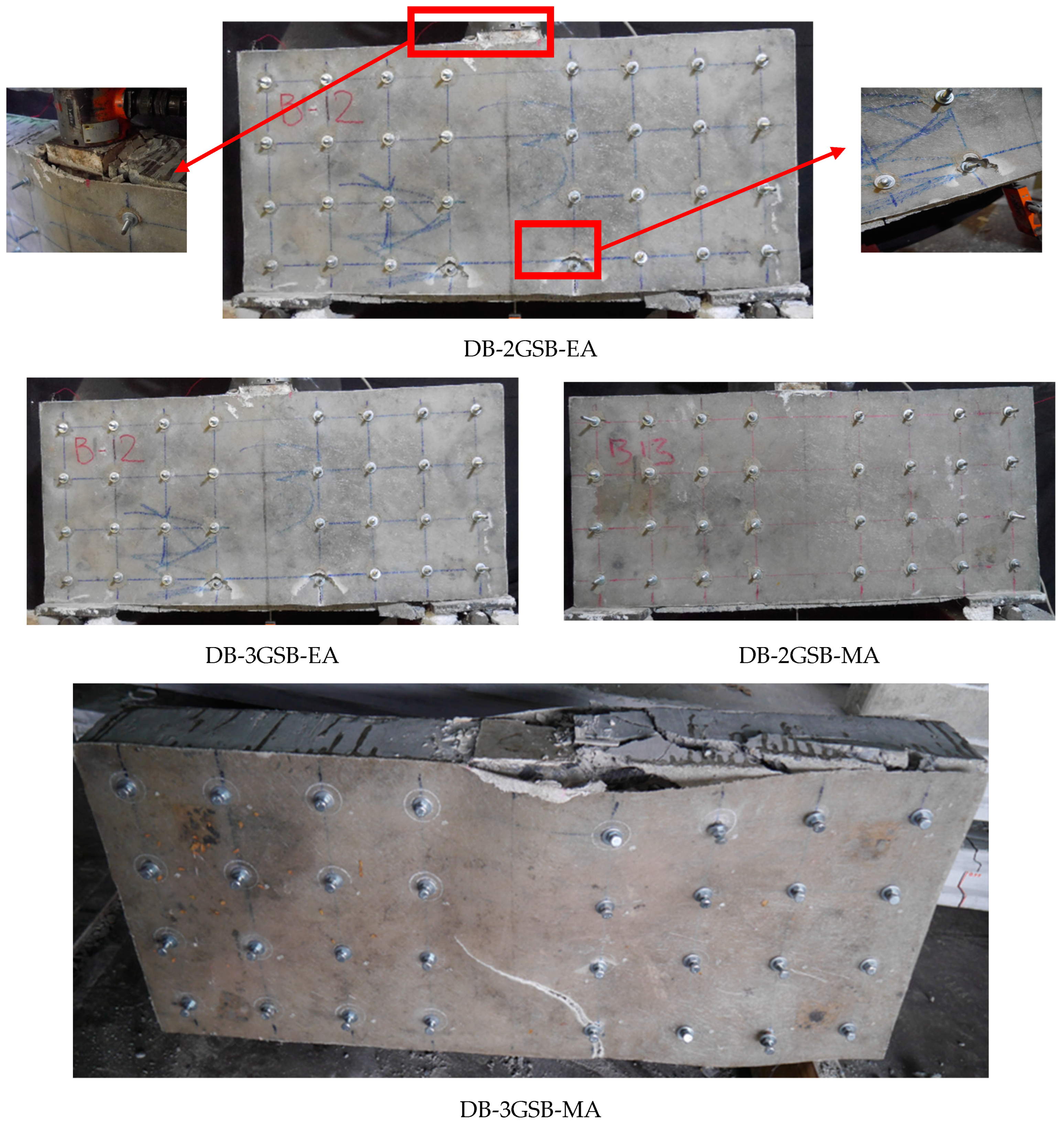
3.1. Failure Patterns of Deep Beams
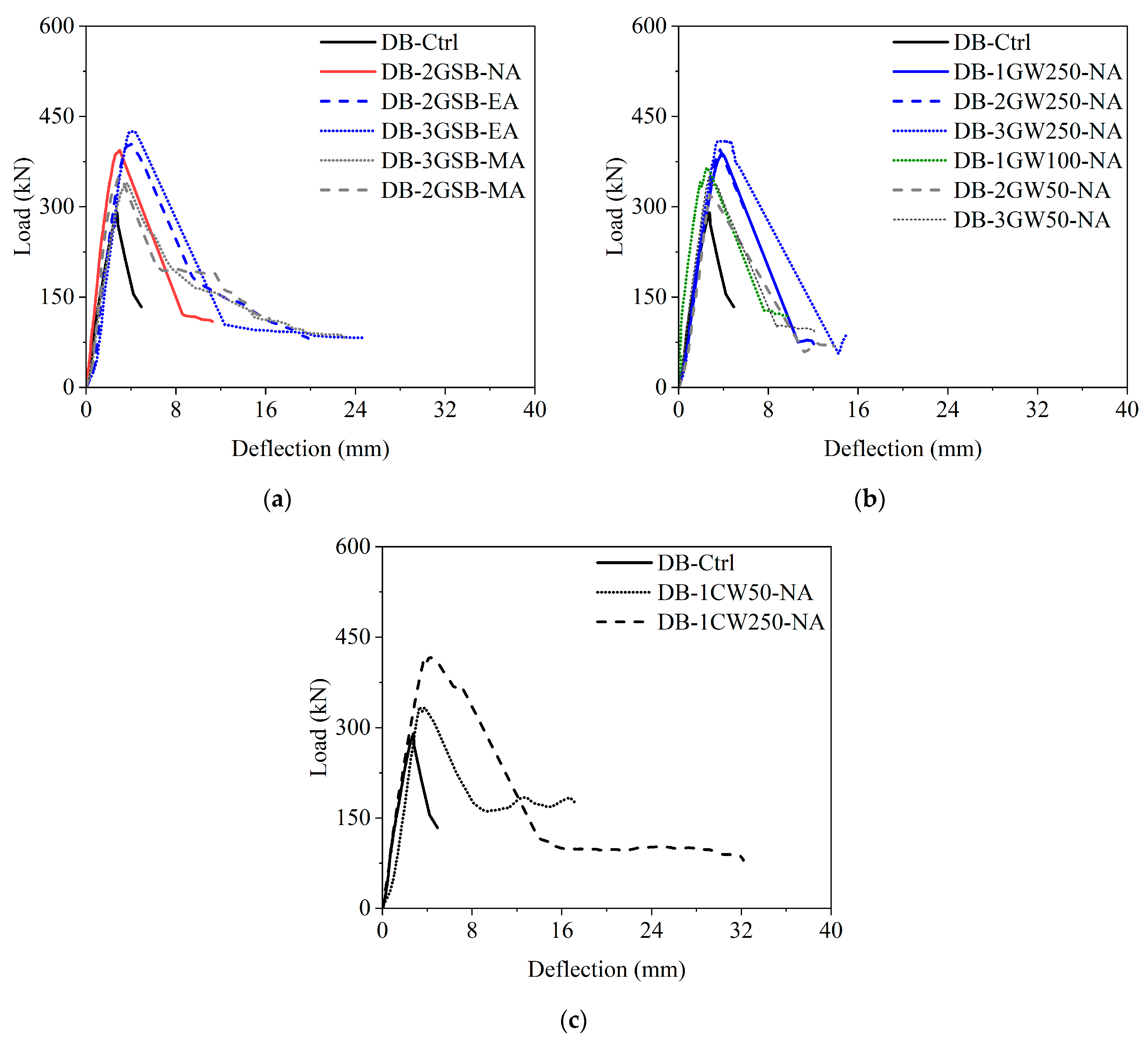
3.2. Load vs. Deflection Response
3.3. Peak Capacity, Ultimate Deflection, and Dissipated Energy
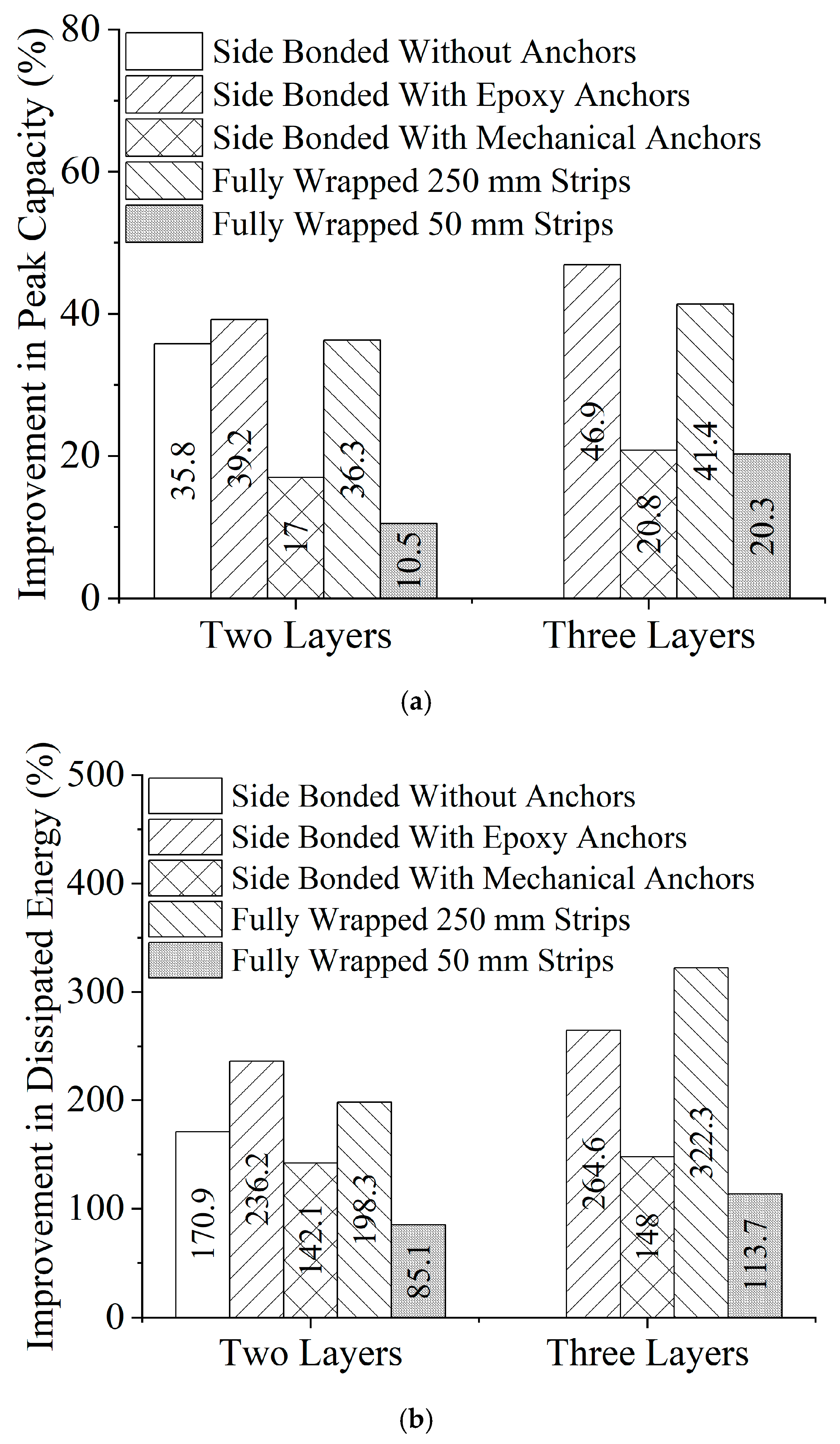
3.4. Effect of Strengthening Configuration
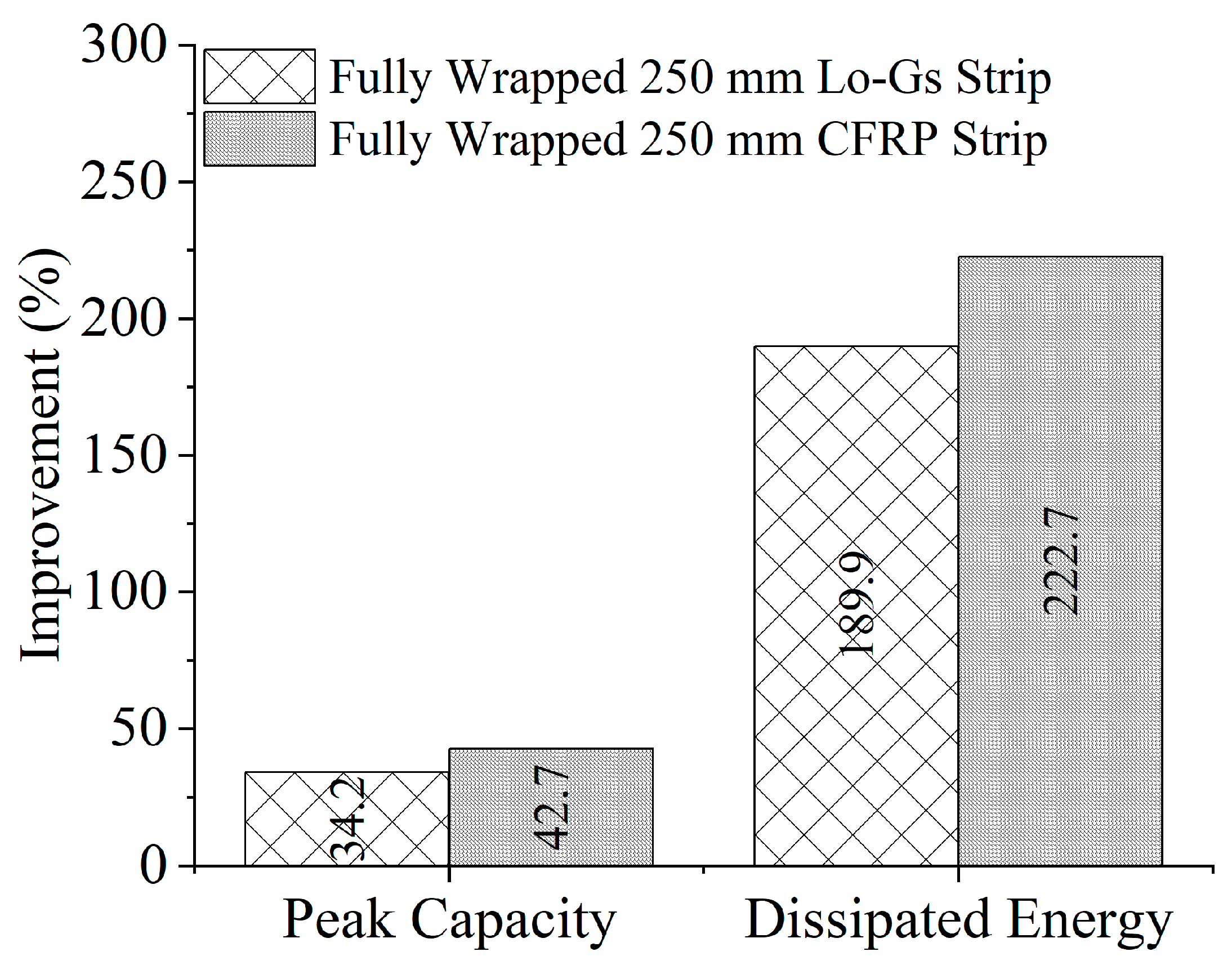
3.5. Effect of Strengthening Type
4. Performance Evaluation of Existing Model Expressions
Design Expressions for FRP Contribution to Shear Strength
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Rao, G.A.; Kunal, K.; Eligehausen, R. Shear strength of RC deep beams. In Proceedings of the 6th International Conference on Fracture Mechanics of Concrete and Concrete Structures, Taylor and Francis, Catania, Italy, 17–22 June 2007; pp. 693–699. [Google Scholar]
- Garcia, S.; Pereira, A.; Pierott, R. Shear Strength of Sand-Lightweight Concrete Deep Beams with Steel Fibers. ACI Struct. J. 2021, 118, 203–214. [Google Scholar] [CrossRef]
- Mattock, A.H.; Li, W.K.; Wang, T.C. Shear transfer in lightweight reinforced concrete. PCI J. 1976, 21, 20–39. [Google Scholar] [CrossRef]
- Sneed, L.H.; Krc, K.; Wermager, S.; Meinheit, D. Interface shear transfer of lightweight-aggregate concretes with different lightweight aggregates. PCI J. 2016, 61, 38–55. [Google Scholar] [CrossRef]
- Emiko, L.; Thamaraikkannan, V.; Huan, W.T.; Thangayah, T. Shear transfer in lightweight concrete. Mag. Concr. Res. 2015, 63, 393–400. [Google Scholar] [CrossRef]
- Floyd, R.W.; Hale, W.M.; Bymaster, J.C. Effect of aggregate and cementitious material on properties of lightweight self-consolidating concrete for prestressed members. Constr. Build. Mater. 2015, 85, 91–99. [Google Scholar] [CrossRef]
- Kong, F.K.; Robins, P.J. Web Reinforcement Effects on Lightweight Concrete Deep beams. ACI J. Proc. 1971, 68, 514–520. [Google Scholar] [CrossRef]
- Yang, K.H. Tests on Lightweight Concrete Deep Beams. ACI Struct. J. 2010, 107, 663–670. [Google Scholar] [CrossRef]
- Yang, K.H.; Ashour, A.F. Aggregate interlock in lightweight concrete continuous deep beams. Eng. Struct. 2011, 33, 136–145. [Google Scholar] [CrossRef]
- Manharawy, M.S.; Mahmoud, A.A.; El-Mahdy, O.O.; El-Diasity, M.H. Experimental and numerical investigation of lightweight foamed reinforced concrete deep beams with steel fibers. Eng. Struct. 2022, 260, 114202. [Google Scholar] [CrossRef]
- Ali, A.A.M.; Hussein, R.L.; Mohamad, R. Experimental Study of the Behaviour of Deep Beams Using Light-Weight Structural Leca Concrete, International Journal of Innovative Research in Science. Eng. Technol. 2016, 5, 428–436. [Google Scholar]
- ACI Committee 318. ACI 318-19: Building Code Requirements for Structural Concrete and Commentary; American Concrete Institute (ACI): Farmington Hills, MI, USA, 2019; Available online: https://www.concrete.org/store/productdetail.aspx?ItemID=318U19&Language=English (accessed on 27 December 2021).
- Wu, T.; Wei, H.; Liu, X. Experimental investigation of shear models for lightweight aggregate concrete deep beams. Adv. Struct. Eng. 2018, 21, 109–124. [Google Scholar] [CrossRef]
- Joyklad, P.; Ali, N.; Verre, S.; Magbool, H.M.; Elnemr, A.; Qureshi, M.I.; Hussain, Q.; Chaiyasarn, K. Experimental Study on the Out-of-Plane Behavior of Brick Masonry Walls Strengthened with Mortar and Wire Mesh: A Pioneer Study. Infrastructures 2021, 6, 165. [Google Scholar] [CrossRef]
- Anwar, A. The influence of waste glass powder as a pozzolanic material in concrete. Int. J. Civ. Eng. 2016, 7, 131–148. [Google Scholar]
- Bakir, P.G.; Boduroǧlu, H.M. Mechanical behaviour and non-linear analysis of short beams using softened truss and direct strut & tie models. Eng. Struct. 2005, 27, 639–651. [Google Scholar] [CrossRef]
- Rahal, K.N.; Al-Shaleh, K.S. Minimum Transverse Reinforcement in 65 Mpa Concrete Beams. ACI Struct. J. 2004, 101, 872–878. [Google Scholar] [CrossRef]
- Ashour, A.F.; Morley, C.T. Effectiveness Factor of Concrete in Continuous Deep Beams. J. Struct. Eng. 1996, 122, 169–178. [Google Scholar] [CrossRef]
- Ashour, A.F. Shear Capacity of Reinforced Concrete Deep Beams. J. Struct. Eng. 2000, 126, 1045–1052. [Google Scholar] [CrossRef]
- Russo, G.; Venir, R.; Pauletta, M. Reinforced Concrete Deep Beams- Shear Strength Model and Design Formula. ACI Struct. J. 2005, 102, 429–437. [Google Scholar] [CrossRef]
- Nishitha, N.; Kavitha, P.E. Effect of openings in deep beams using strut and tie model method. Int. J. Tech. Res. Appl. 2015, 3, 59–62. [Google Scholar]
- Cao, S.Y.; Chen, J.F.; Teng, J.G.; Hao, Z.; Chen, J. Debonding in RC Beams Shear Strengthened with Complete FRP Wraps. J. Compos. Constr. 2005, 9, 417–428. [Google Scholar] [CrossRef]
- Chrysanidis, T.; Tegos, I. Axial and transverse strengthening of R/C circular columns: Conventional and new type of steel and hybrid jackets using high-strength mortar. J. Build. Eng. 2020, 30, 101236. [Google Scholar] [CrossRef]
- Bukhari, I.A.; Vollum, R.; Ahmad, S.; Sagaseta, J. Shear Strengthening of Short Span Reinforced Concrete Beams with CFRP Sheets. Arab. J. Sci. Eng. 2013, 38, 523–536. [Google Scholar] [CrossRef]
- Alacali, S. A prediction model for strength and strain of CFRP-confined concrete cylinders using gene expression programming. Comput. Concr. 2022, 30, 377–391. [Google Scholar] [CrossRef]
- Cakir, F.; Aydin, M.R.; Acar, V.; Aksar, B.; Akkaya, H.C. An experimental study on RC beams shear-strengthened with Intraply Hybrid U-Jackets Composites monitored by digital image correlation (DIC). Compos. Struct. 2023, 323, 117503. [Google Scholar] [CrossRef]
- Cakir, F.; Acar, V.; Aydin, M.R.; Aksar, B.; Yildirim, P. Strengthening of reinforced concrete beams without transverse reinforcement by using intraply hybrid composites. Case Stud. Constr. Mater. 2021, 15, e00700. [Google Scholar] [CrossRef]
- Ulu, A.; Tutar, A.I.; Kurklu, A.; Cakir, F. Effect of excessive fiber reinforcement on mechanical properties of chopped glass fiber reinforced polymer concretes. Constr. Build. Mater. 2022, 359, 129486. [Google Scholar] [CrossRef]
- Cakir, F. Evaluation of mechanical properties of chopped glass/basalt fibers reinforced polymer mortars. Case Stud. Constr. Mater. 2021, 15, e00612. [Google Scholar] [CrossRef]
- Li, W.; Tang, S.; Huang, Z.; Yang, X.; Shi, T.; Xing, F. Shear behavior of concrete beam reinforced in shear with carbon fiber-reinforced polymer mesh fabric (CFRP-MF) configuration. Eng. Struct. 2020, 218, 110828. [Google Scholar] [CrossRef]
- Al-Rousan, R.Z. Impact of elevated temperature and anchored grooves on the shear behavior of reinforced concrete beams strengthened with CFRP composites. Case Stud. Constr. Mater. 2021, 14, e00487. [Google Scholar] [CrossRef]
- Mostofinejad, D.; Kashani, A.T.; Hosseini, A. Design model for shear capacity of RC beams strengthened with two-side CFRP wraps based on effective FRP strain concept. Eur. J. Environ. Civ. Eng. 2016, 20, 161–179. [Google Scholar] [CrossRef]
- Abid, S.R.; Al-Lami, K. Critical review of strength and durability of concrete beams externally bonded with FRP. Cogent Eng. 2018, 5, 1525015. [Google Scholar] [CrossRef]
- ACI. 440.2 R-02: Guide for the Design and Construction of Externally Bonded FRP Systems for Strengthening Concrete Structures; ACI: Farmington Hills, MI, USA, 1981; Available online: https://www.scribd.com/document/538872004/ACI-440-2R-02 (accessed on 26 October 2022).
- Ejaz, A.; Ruangrassamee, A.; Kruavit, P.; Udomworarat, P.; Wijeyewickrema, A.C. Strengthening of Substandard Lap Splices Using Hollow Steel Section (HSS) Collars. Structures 2022, 46, 128–145. [Google Scholar] [CrossRef]
- Lal, H.M.; Uthaman, A.; Li, C.; Xian, G.; Thomas, S. Combined effects of cyclic/sustained bending loading and water immersion on the interface shear strength of carbon/glass fiber reinforced polymer hybrid rods for bridge cable. Constr. Build. Mater. 2022, 314, 125587. [Google Scholar] [CrossRef]
- Duarte, P.; Correia, J.R.; Ferreira, J.G.; Nunes, F.; Arruda, M.R.T. Experimental and numerical study on the effect of repairing reinforced concrete cracked beams strengthened with carbon fibre reinforced polymer laminates. Can. J. Civ. Eng. 2014, 41, 222–231. [Google Scholar] [CrossRef]
- Yazdani, N.; Aljaafreh, T.; Beneberu, E. Concrete beam flexural strengthening with anchored pre-saturated CFRP laminates. Compos. Struct. 2020, 235, 111733. [Google Scholar] [CrossRef]
- Abed, F.; Al-Mimar, M.; Ahmed, S. Performance of BFRP RC beams using high strength concrete. Compos. Part C Open Access 2021, 4, 100107. [Google Scholar] [CrossRef]
- Azam, R.; Soudki, K.; West, J.S.; Noël, M. Strengthening of shear-critical RC beams: Alternatives to externally bonded CFRP sheets. Constr. Build. Mater. 2017, 151, 494–503. [Google Scholar] [CrossRef]
- Baggio, D.; Soudki, K.; Noël, M. Strengthening of shear critical RC beams with various FRP systems. Constr. Build. Mater. 2014, 66, 634–644. [Google Scholar] [CrossRef]
- Chaallal, O.; Nollet, M.-J.; Perraton, D. Shear strengthening of RC beams by externally bonded side CFRP strips. J. Compos. Constr. 1998, 2, 111–113. [Google Scholar] [CrossRef]
- Sen, T.; Reddy, H.J. Efficacy of bio derived jute FRP composite based technique for shear strength retrofitting of reinforced concrete beams and its comparative analysis with carbon and glass FRP shear retrofitting schemes. Sustain. Cities Soc. 2014, 13, 105–124. [Google Scholar] [CrossRef]
- Zwawi, M. A Review on Natural Fiber Bio-Composites, Surface Modifications and Applications. Molecules 2021, 26, 404. [Google Scholar] [CrossRef]
- Ivanova, I.; Assih, J.; Dontchev, D. Investigation of the mechanical behavior of natural vegetable fibers used in composite materials for structural strengthening. Key Eng. Mater. 2021, 888, 15–21. [Google Scholar] [CrossRef]
- Yang, K.; Wang, H.; Liu, Z. Evaluation on mechanical properties of high-performance biocomposite bridge deck structure: A review. Polym. Compos. 2021, 42, 6265–6297. [Google Scholar] [CrossRef]
- Zaman, A.; Gutub, S.A.; Wafa, M.A. A review on FRP composites applications and durability concerns in the construction sector. J. Reinf. Plast. Compos. 2013, 32, 1966–1988. [Google Scholar] [CrossRef]
- Yoddumrong, P.; Rodsin, K.; Katawaethwarag, S. Seismic Strengthening of Low-Strength RC Concrete Columns Using Low-Cost Glass Fiber Reinforced Polymers (GFRPs). Case Stud. Constr. Mater. 2020, 13, e00383. [Google Scholar] [CrossRef]
- Khaloo, A.; Tabatabaeian, M.; Khaloo, H. The axial and lateral behavior of low strength concrete confined by GFRP wraps: An experimental investigation. Structures 2020, 27, 747–766. [Google Scholar] [CrossRef]
- Lam, L.; Hussain, Q.; Joyklad, P.; Pimanmas, A. Behavior of RC Deep Beams Strengthened in Shear using Glass Fiber Reinforced Polymer with Mechanical Anchors. In Proceedings of the International Conference on Environment and Civil Engineering (ICEACE’2015), Pattaya, Thailand, 24–25 April 2015. [Google Scholar] [CrossRef]
- Rodsin, K.; Hussain, Q.; Suparp, S.; Nawaz, A. Compressive behavior of extremely low strength concrete confined with low-cost glass FRP composites. Case Stud. Constr. Mater. 2020, 13, e00452. [Google Scholar] [CrossRef]
- Rodsin, K.; Ejaz, A.; Hussain, Q.; Parichatprecha, R. Experimental and Analytical Studies on Low-Cost Glass-Fiber-Reinforced-Polymer-Composite-Strengthened Reinforced Concrete Beams: A Comparison with Carbon/Sisal Fiber-Reinforced Polymers. Polymers 2023, 15, 4027. [Google Scholar] [CrossRef]
- Rodsin, K.; Hussain, Q.; Joyklad, P.; Nawaz, A.; Fazliani, H. Seismic strengthening of nonductile bridge piers using low-cost glass fiber polymers. Bull. Pol. Acad. Sci. Tech. Sci. 2020, 68, 1457–1470. [Google Scholar] [CrossRef]
- Bukhari, I.A.; Vollum, R.L.; Ahmad, S.; Sagaseta, J. Shear strengthening of reinforced concrete beams with CFRP. Mag. Concr. Res. 2015, 62, 65–77. [Google Scholar] [CrossRef]
- Grande, E.; Imbimbo, M.; Rasulo, A. Effect of transverse steel on the response of RC beams strengthened in shear by FRP: Experimental study. J. Compos. Constr. 2009, 13, 405–414. [Google Scholar] [CrossRef]
- ASTM E8-04; Standard Test Methods for Tension Testing of Metallic Materials. ASTM International: Washington, DC, USA, 2004.
- ASTM D7565/D7565M-10(2017); Standard Test Method for Determining Tensile Properties of Fiber Reinforced Polymer Matrix Composites Used for Strengthening of Civil Structures. ASTM International: Washington, DC, USA, 2017. Available online: https://www.astm.org/d7565_d7565m-10r17.html (accessed on 28 November 2021).
- ACI SPEC-440.12-22; Strengthening of Concrete Structures with Externally Bonded Fiber-Reinforced Polymer (FRP) Materials Using the Wet Layup Method—Specification. ACI: Farmington Hills, MI, USA, 2022.
- Yang, K.H.; Chung, H.S.; Lee, E.T.; Eun, H.C. Shear characteristics of high-strength concrete deep beams without shear reinforcements. Eng. Struct. 2003, 25, 1343–1352. [Google Scholar] [CrossRef]
- Hadhood, A.; Agamy, M.H.; Abdelsalam, M.M.; Mohamed, H.M.; El-Sayed, T.A. Shear strengthening of hybrid externally-bonded mechanically-fastened concrete beams using short CFRP strips: Experiments and theoretical evaluation. Eng. Struct. 2019, 201, 109795. [Google Scholar] [CrossRef]
- Suparp, S.; Khan, I.; Ejaz, A.; Khan, K.; Weesakul, U.; Hussain, Q.; Saingam, P. Behavior of non-prismatic RC beams with conventional steel and green GFRP rebars for sustainable infrastructure. Sci. Rep. 2023, 13, 15733. [Google Scholar] [CrossRef]
- Aydemir, C.; Aydemir, M.E.; Arslan, G. Drift capacity and allowable axial load level of RC columns. Structures 2023, 48, 1072–1081. [Google Scholar] [CrossRef]
- Lehman, D.; Moehle, J.; Mahin, S.; Calderone, A.; Henry, L. Experimental evaluation of the seismic performance of reinforced concrete bridge columns. J. Struct. Eng. 2004, 130, 869–879. [Google Scholar] [CrossRef]
- Salama, A.S.D.; Hawileh, R.A.; Abdalla, J.A. Performance of externally strengthened RC beams with side-bonded CFRP sheets. Compos. Struct. 2019, 212, 281–290. [Google Scholar] [CrossRef]
- Chen, J.F.; Teng, J.G. Shear capacity of FRP-strengthened RC beams: FRP debonding. Constr. Build. Mater. 2003, 17, 27–41. [Google Scholar] [CrossRef]
- Khalifa, A.; Gold, W.J.; Nanni, A.; MI, A.A. Contribution of Externally Bonded FRP to Shear Capacity of RC Flexural Members. J. Compos. Constr. 1998, 2, 195–202. [Google Scholar] [CrossRef]
- Triantifillou, T.C. Shear strengthening of reinforced concrete beams using epoxy-bonded FRP composites. ACI Struct. J. 1998, 95, 107–115. Available online: https://www.researchgate.net/profile/Thanasis-Triantafillou/publication/247509718_Shear_Strengthening_Of_Reinforced_Concrete_Beams_Using_Epoxy-Bonded_FRP_Composites/links/5a8aecb20f7e9b1a9554c8c4/Shear-Strengthening-Of-Reinforced-Concrete-Beams-Using-Epoxy-Bonded-FRP-Composites.pdf (accessed on 7 July 2023).
- Zhang, Z.; Hsu, C.-T.T. Shear Strengthening of Reinforced Concrete Beams Using Carbon-Fiber-Reinforced Polymer Laminates. J. Compos. Constr. 2005, 9, 158–169. [Google Scholar] [CrossRef]
- Fib TG 9.3, Externally Bonded FRP Reinforcement for RC Structures, n.d. Available online: https://biblio.ugent.be/publication/323754 (accessed on 21 July 2023).
- Sengun, K.; Arslan, G. Investigation of the parameters affecting the behavior of RC beams strengthened with FRP. Front. Struct. Civ. Eng. 2022, 16, 729–743. [Google Scholar] [CrossRef]
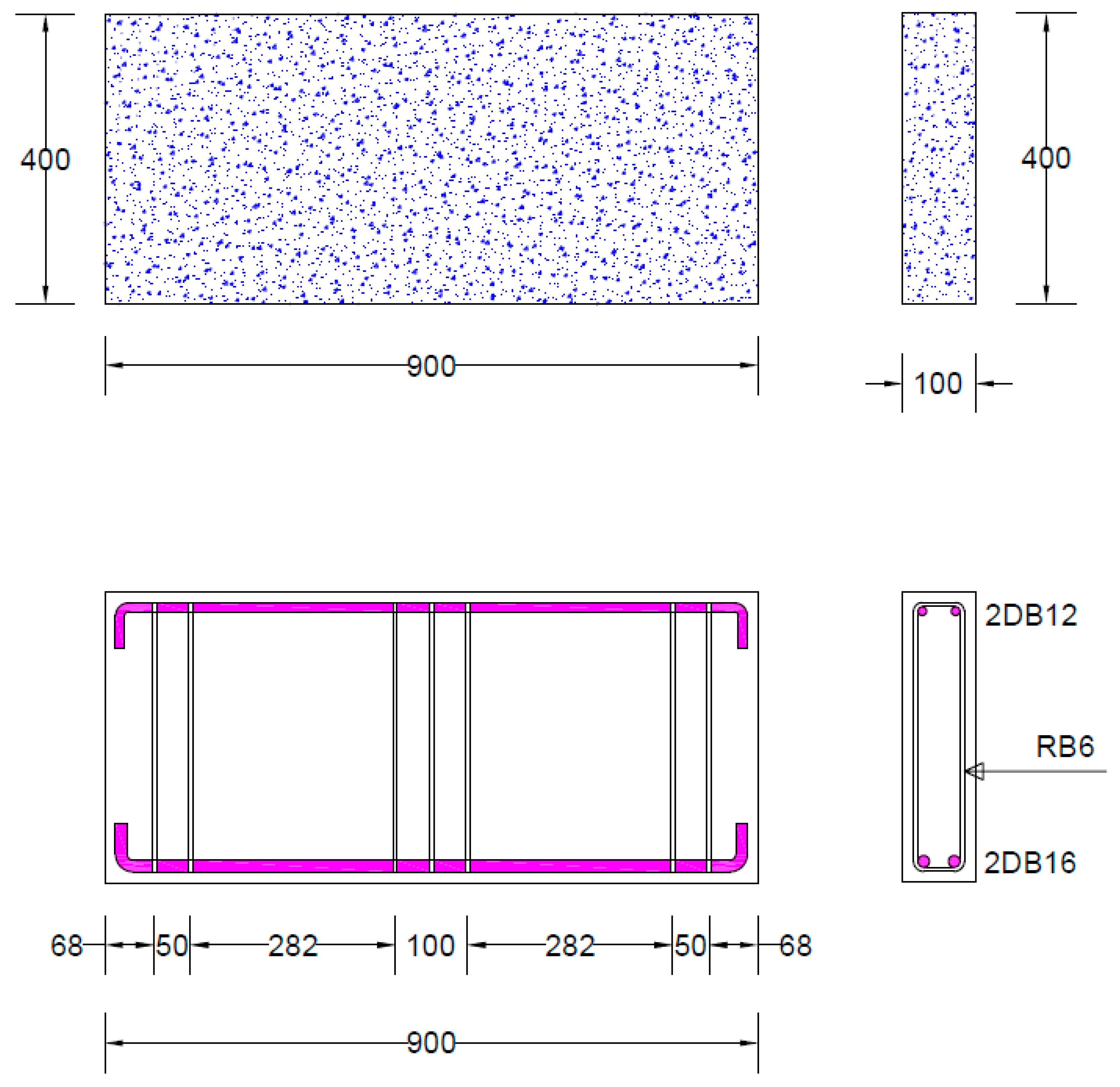


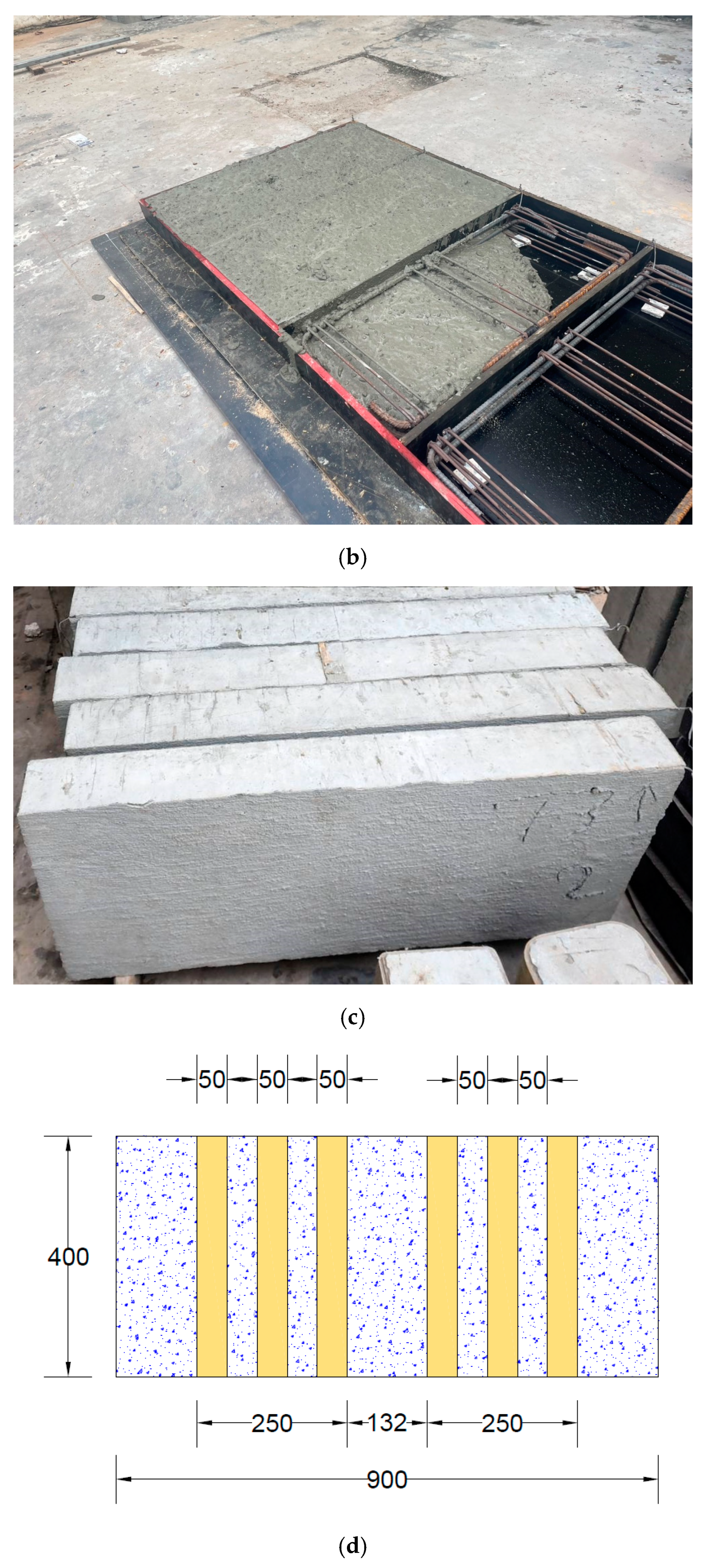
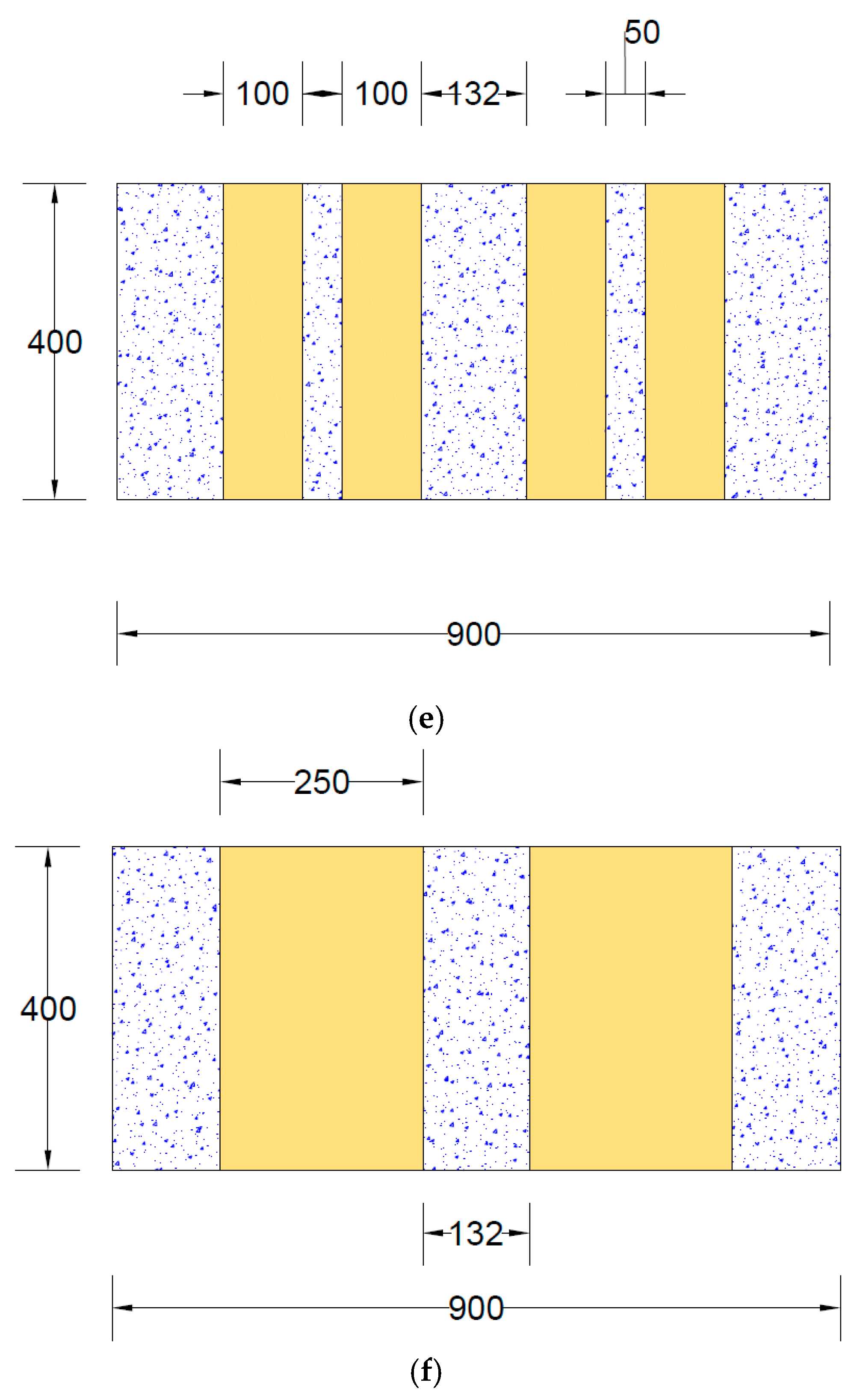
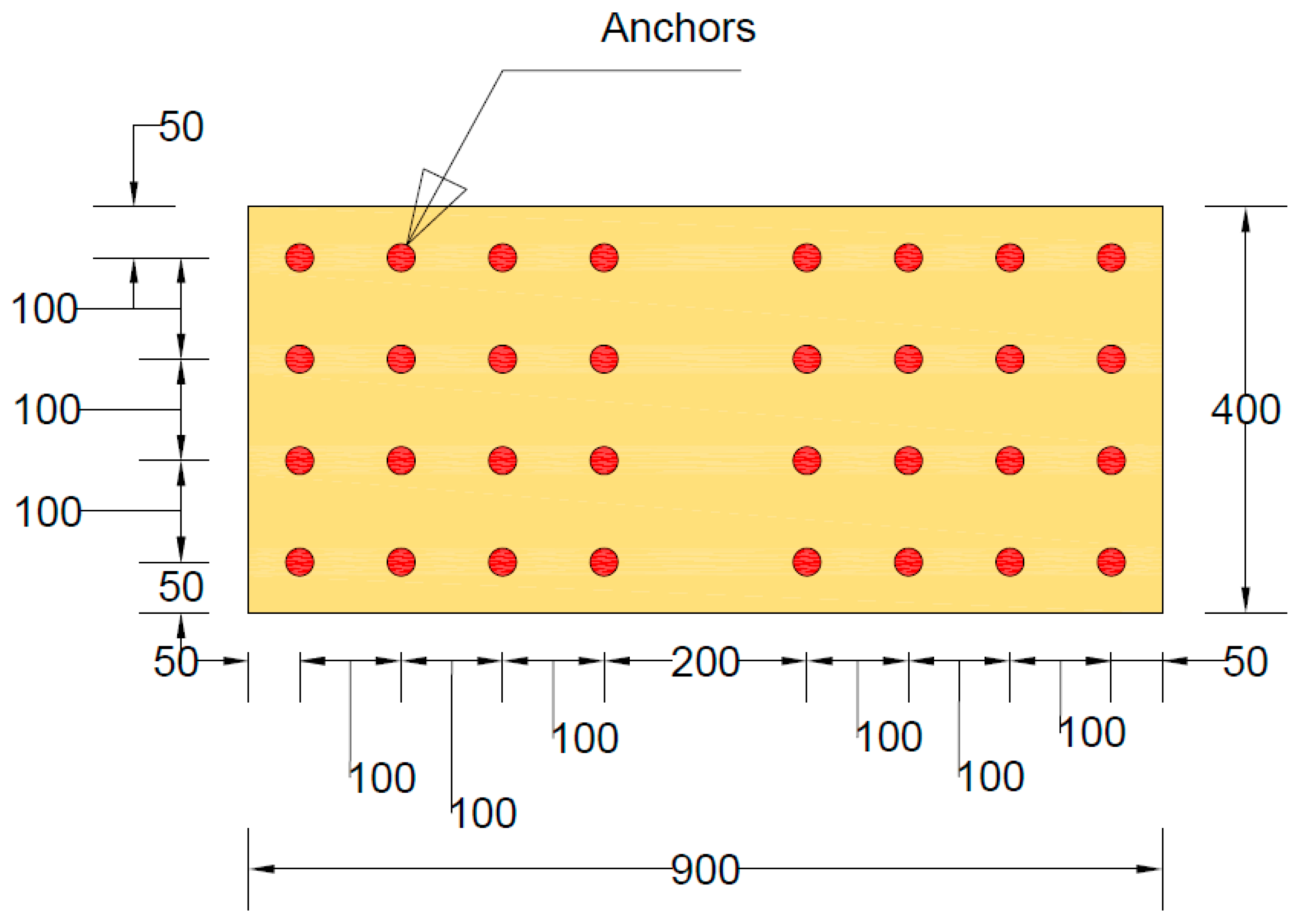


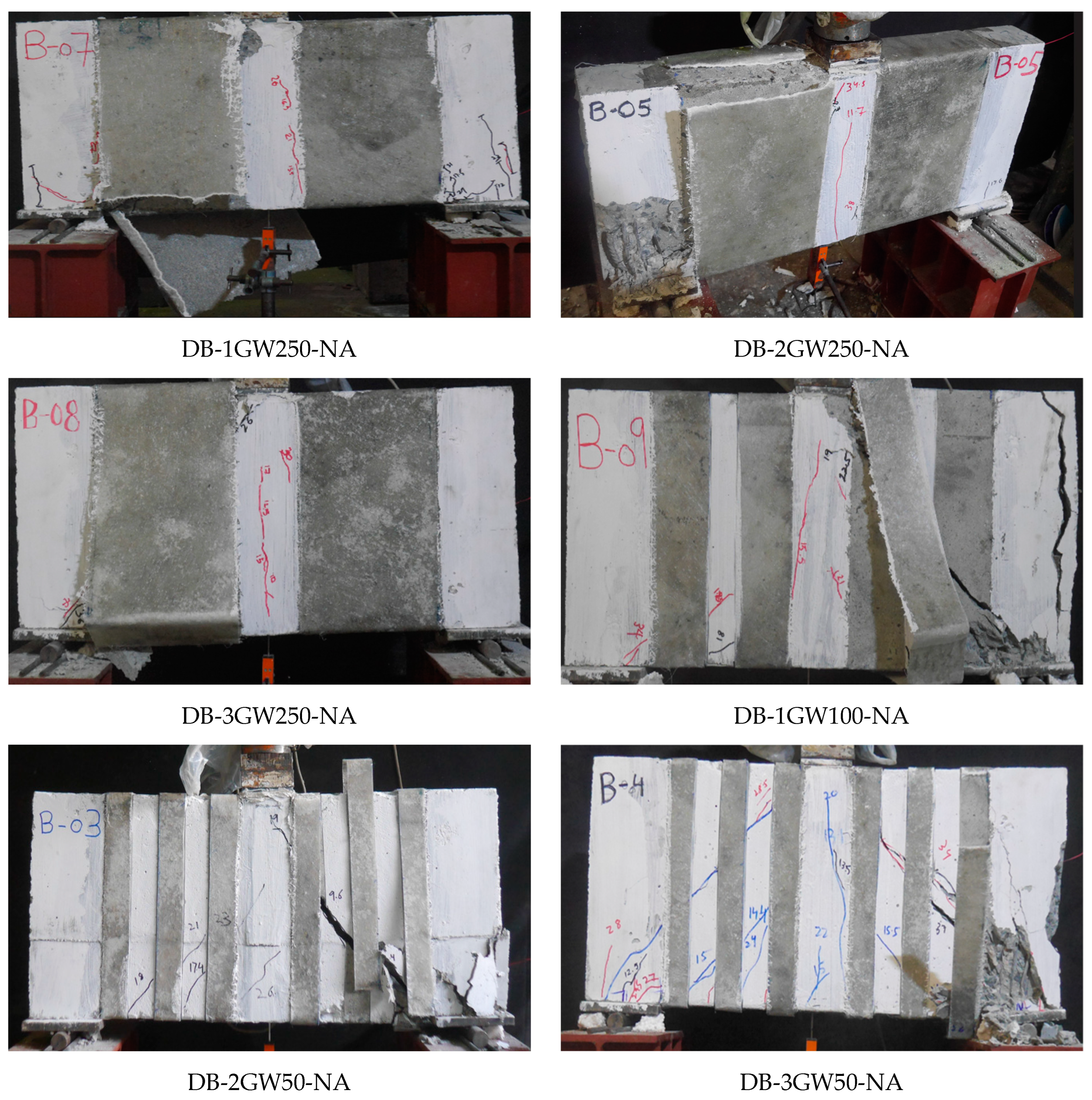

| Group | ID | Configuration | Anchorage | Quantity of Lo-G Wraps |
|---|---|---|---|---|
| 1 | DB-Ctrl | None | None | None |
| 2 | DB-2GSB-NA | Side-bonded | None | 2 |
| DB-2GSB-EA | Side-bonded | Epoxy | 2 | |
| DB-3GSB-EA | Side-bonded | Epoxy | 3 | |
| DB-2GSB-MA | Side-bonded | Mechanical | 2 | |
| DB-3GSB-MA | Side-bonded | Mechanical | 3 | |
| 3 | DB-1GW250-NA | Fully wrapped | None | 1 |
| DB-2GW250-NA | Fully wrapped | None | 2 | |
| DB-3GW250-NA | Fully wrapped | None | 3 | |
| DB-1GW100-NA | Fully wrapped | None | 1 | |
| DB-2GW50-NA | Fully wrapped | None | 2 | |
| DB-3GW50-NA | Fully wrapped | None | 3 | |
| 4 | DB-1CW50-NA | Fully wrapped | None | 1 |
| DB-1CW250-NA | Fully wrapped | None | 1 |
| Material | Thickness (mm) | Tensile Capacity (MPa) | Tensile Strain (%) | Elastic Modulus (GPa) |
|---|---|---|---|---|
| Lo-Gs | 0.50 | 377 | 2.04 | 19 |
| CFRP | 0.13 | 5241 | 2.00 | 30 |
| ID | Peak Capacity P (kN) | Increase in P (%) | (mm) | (%) | Dissipated Energy E (kN-mm) | Increase in E (%) | Failure Mode |
|---|---|---|---|---|---|---|---|
| DB-Ctrl | 290.1 | - | 2.9 | - | 475 | - | Shear |
| DB-2GSB-NA | 393.8 | 35.8 | 4.6 | 58.6 | 1287 | 170.9 | Shear, debonding |
| DB-2GSB-EA | 403.7 | 39.2 | 5.9 | 103.4 | 1597 | 236.2 | Shear, debonding |
| DB-3GSB-EA | 425.9 | 46.9 | 6.3 | 117.2 | 1732 | 264.6 | Shear, debonding |
| DB-2GSB-MA | 339.3 | 17.0 | 5.1 | 75.9 | 1150 | 142.1 | Shear, debonding |
| DB-3GSB-MA | 350.3 | 20.8 | 5.2 | 79.3 | 1178 | 148.0 | Shear, debonding |
| DB-1GW250-NA | 389.1 | 34.2 | 5.4 | 86.2 | 1377 | 189.9 | Fracture, shear, debonding |
| DB-2GW250-NA | 395.4 | 36.3 | 5.5 | 89.7 | 1417 | 198.3 | Fracture, shear, debonding |
| DB-3GW250-NA | 410.1 | 41.4 | 6.9 | 137.9 | 2006 | 322.3 | Fracture, shear, debonding |
| DB-1GW100-NA | 363.9 | 25.5 | 4.1 | 41.4 | 1144 | 140.8 | Fracture, shear, debonding |
| DB-2GW50-NA | 320.5 | 10.5 | 4.4 | 51.7 | 879 | 85.1 | Fracture, shear, debonding |
| DB-3GW50-NA | 349.0 | 20.3 | 4.3 | 48.3 | 1015 | 113.7 | Fracture, shear, debonding |
| DB-1CW50-NA | 334.6 | 15.4 | 5.6 | 93.1 | 1180 | 148.4 | Fracture, shear, debonding |
| DB-1CW250-NA | 413.8 | 42.7 | 7.1 | 144.8 | 1533 | 222.7 | Fracture, shear, debonding |
| ID | (kN) | Predicted Strength | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
(kN) | (kN) | (kN) | (kN) | (kN) | |||||||
| DB-2GSB-NA | 51.9 | 101.6 | 2.0 | 101.3 | 2.0 | 23.5 | 0.5 | 10.7 | 0.2 | 53.8 | 1.0 |
| DB-2GSB-EA | 56.9 | 101.6 | 1.8 | 101.3 | 1.8 | 23.5 | 0.4 | 10.7 | 0.2 | 53.8 | 0.9 |
| DB-3GSB-EA | 68.0 | 111.4 | 1.6 | 134.6 | 2.0 | 26.0 | 0.4 | 14.3 | 0.2 | 57.4 | 0.8 |
| DB-2GSB-MA | 24.7 | 101.6 | 4.1 | 101.3 | 4.1 | 23.5 | 0.9 | 10.7 | 0.4 | 53.8 | 2.2 |
| DB-3GSB-MA | 30.2 | 111.4 | 3.7 | 134.6 | 4.5 | 26.0 | 0.9 | 14.3 | 0.5 | 57.4 | 1.9 |
| DB-1GW250-NA | 49.6 | 67.5 | 1.4 | 62.4 | 1.3 | 19.6 | 0.4 | 6.6 | 0.1 | 48.1 | 1.0 |
| DB-2GW250-NA | 52.7 | 101.6 | 1.9 | 101.3 | 1.9 | 23.5 | 0.4 | 10.7 | 0.2 | 53.8 | 1.0 |
| DB-3GW250-NA | 60.1 | 111.4 | 1.9 | 134.6 | 2.2 | 26.0 | 0.4 | 14.3 | 0.2 | 57.4 | 1.0 |
| DB-1GW100-NA | 37.0 | 49.2 | 1.3 | 47.0 | 1.3 | 26.6 | 0.7 | 7.5 | 0.2 | 67.7 | 1.8 |
| DB-2GW50-NA | 15.3 | 67.5 | 4.4 | 62.4 | 4.1 | 39.4 | 2.6 | 13.2 | 0.9 | 96.3 | 6.3 |
| DB-3GW50-NA | 29.5 | 88.2 | 3.0 | 82.8 | 2.8 | 43.7 | 1.5 | 17.6 | 0.6 | 102.7 | 3.5 |
| Mean | 2.2 | 2.3 | 0.4 | 0.3 | 2.0 | ||||||
| Standard Deviation | 1.2 | 1.2 | 0.3 | 0.2 | 1.5 | ||||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Saingam, P.; Ejaz, A.; Gadagamma, C.K.; Hussain, Q.; Sua-iam, G.; Chatveera, B.; Maneengamlert, B.; Joyklad, P. Innovative Approaches to RC Deep Beam Strengthening: Evaluating Low-Cost Glass Fiber Wraps Against Traditional CFRP Solutions. Polymers 2025, 17, 807. https://doi.org/10.3390/polym17060807
Saingam P, Ejaz A, Gadagamma CK, Hussain Q, Sua-iam G, Chatveera B, Maneengamlert B, Joyklad P. Innovative Approaches to RC Deep Beam Strengthening: Evaluating Low-Cost Glass Fiber Wraps Against Traditional CFRP Solutions. Polymers. 2025; 17(6):807. https://doi.org/10.3390/polym17060807
Chicago/Turabian StyleSaingam, Panumas, Ali Ejaz, Chaitanya Krishna Gadagamma, Qudeer Hussain, Gritsada Sua-iam, Burachat Chatveera, Bodee Maneengamlert, and Panuwat Joyklad. 2025. "Innovative Approaches to RC Deep Beam Strengthening: Evaluating Low-Cost Glass Fiber Wraps Against Traditional CFRP Solutions" Polymers 17, no. 6: 807. https://doi.org/10.3390/polym17060807
APA StyleSaingam, P., Ejaz, A., Gadagamma, C. K., Hussain, Q., Sua-iam, G., Chatveera, B., Maneengamlert, B., & Joyklad, P. (2025). Innovative Approaches to RC Deep Beam Strengthening: Evaluating Low-Cost Glass Fiber Wraps Against Traditional CFRP Solutions. Polymers, 17(6), 807. https://doi.org/10.3390/polym17060807






