Damping Optimization and Energy Absorption of Mechanical Metamaterials for Enhanced Vibration Control Applications: A Critical Review
Abstract
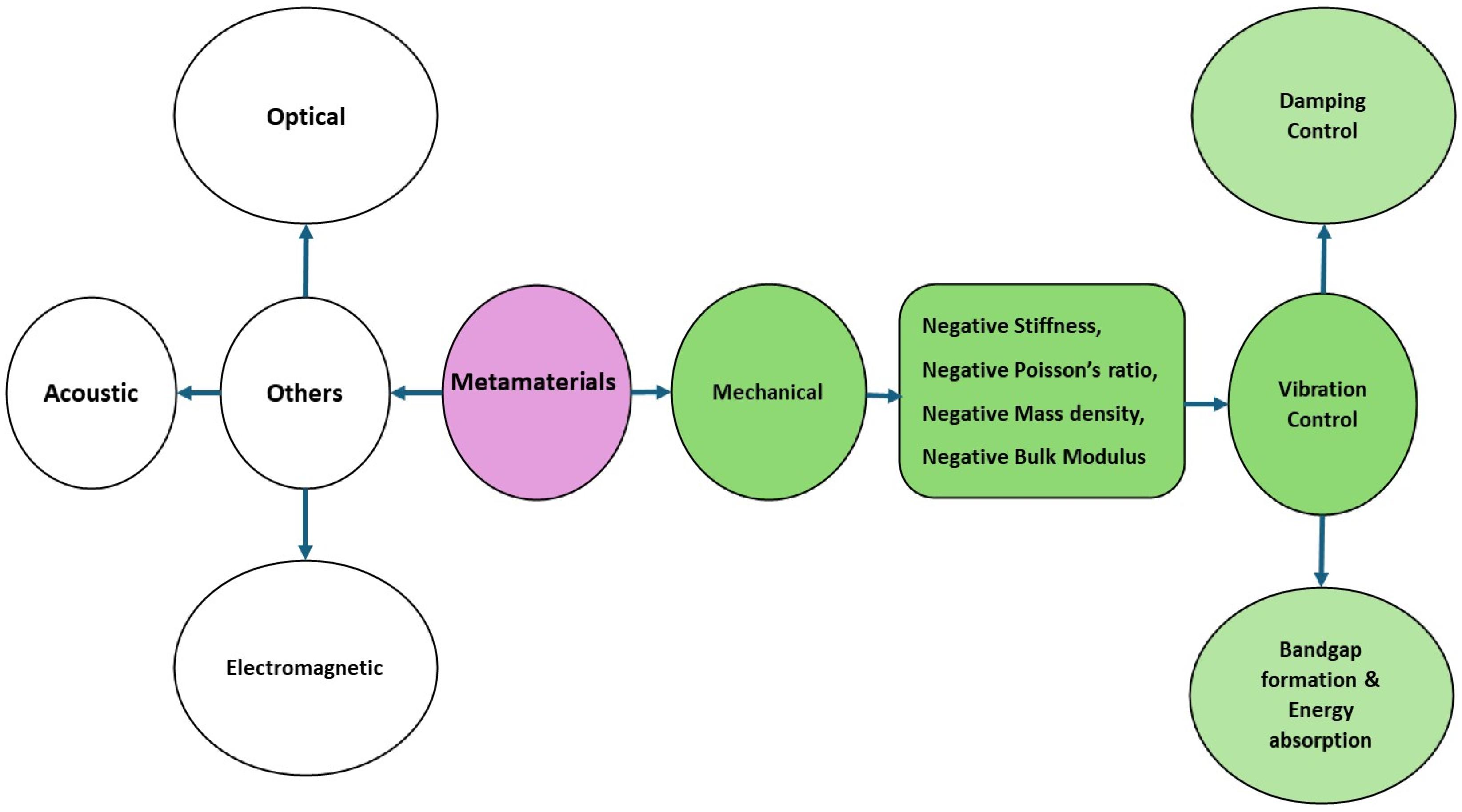
1. Introduction
2. Mechanical Metamaterial for Vibration Control
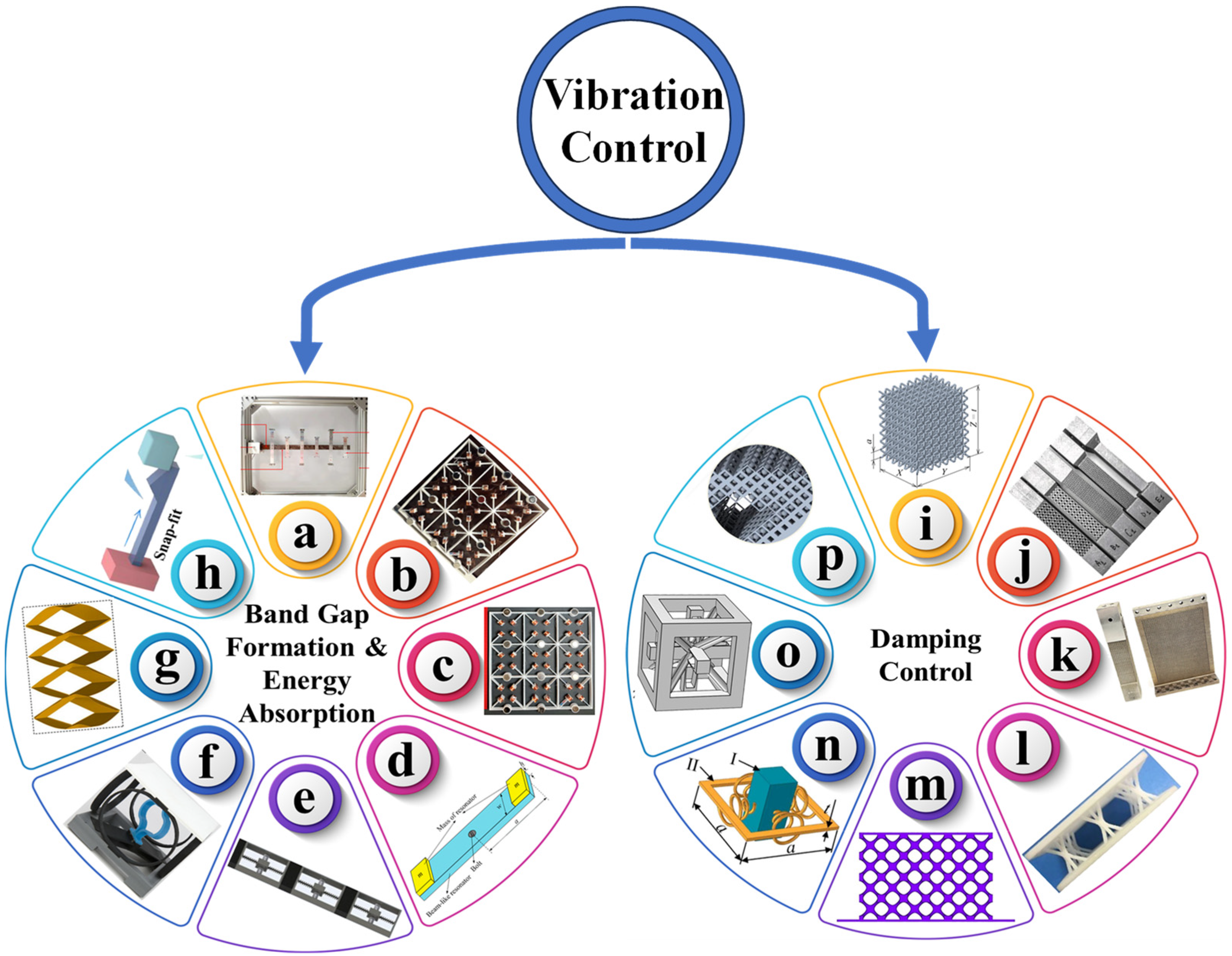
2.1. Bandgap Formation and Energy Absorption Using Mechanical Metamaterials
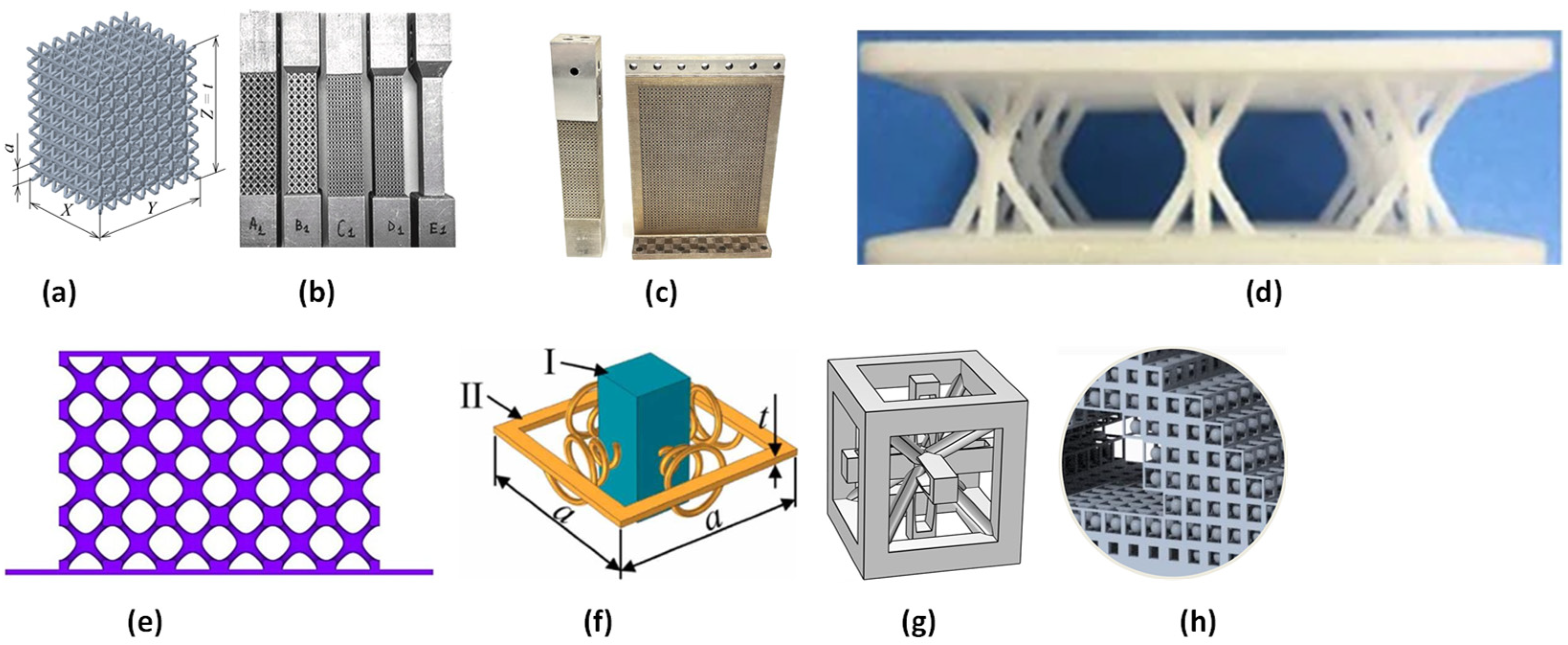
2.2. Damping Control Using Mechanical Metamaterials
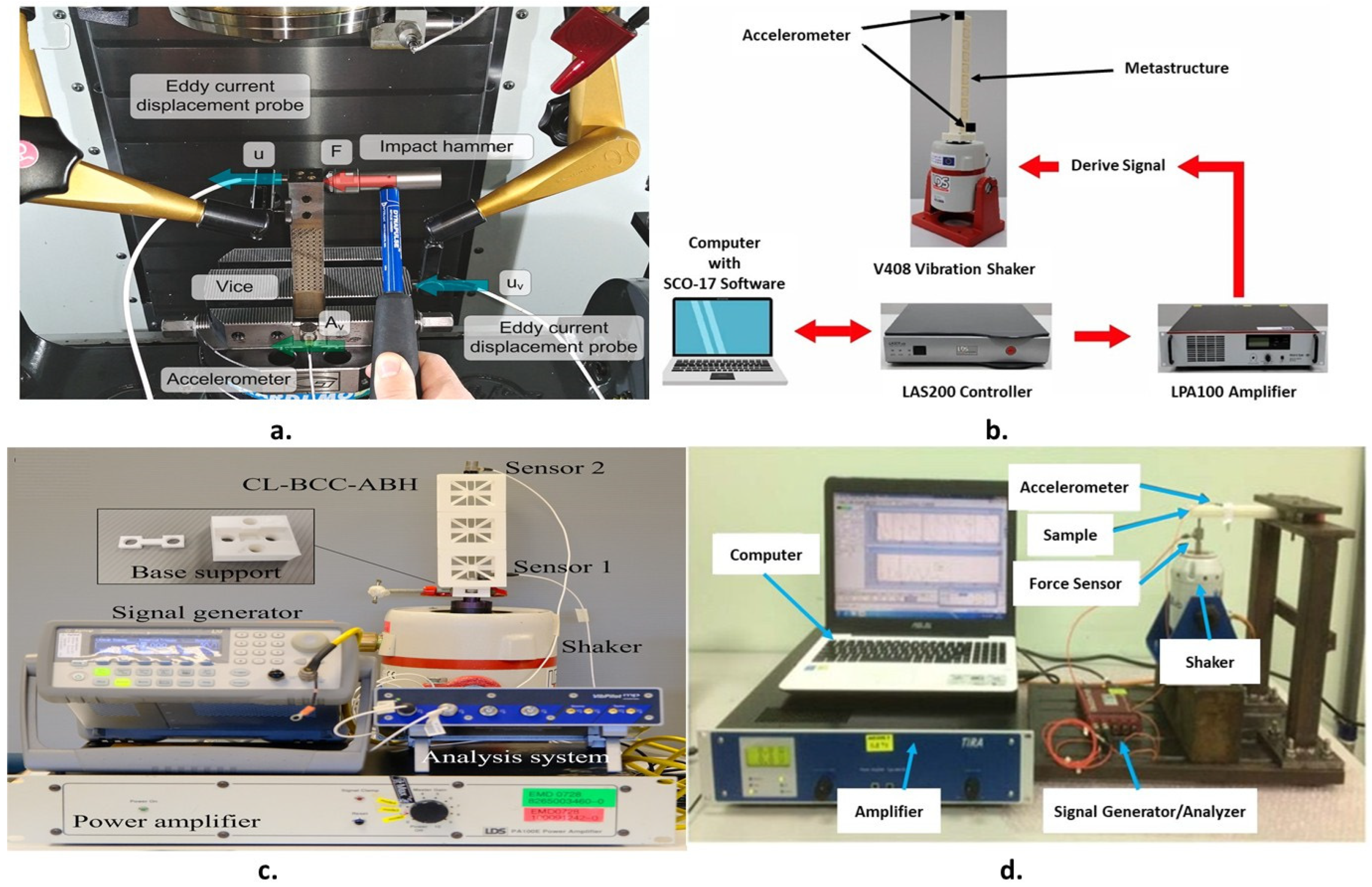
3. Vibration Damping Modeling and Experimental Measurement
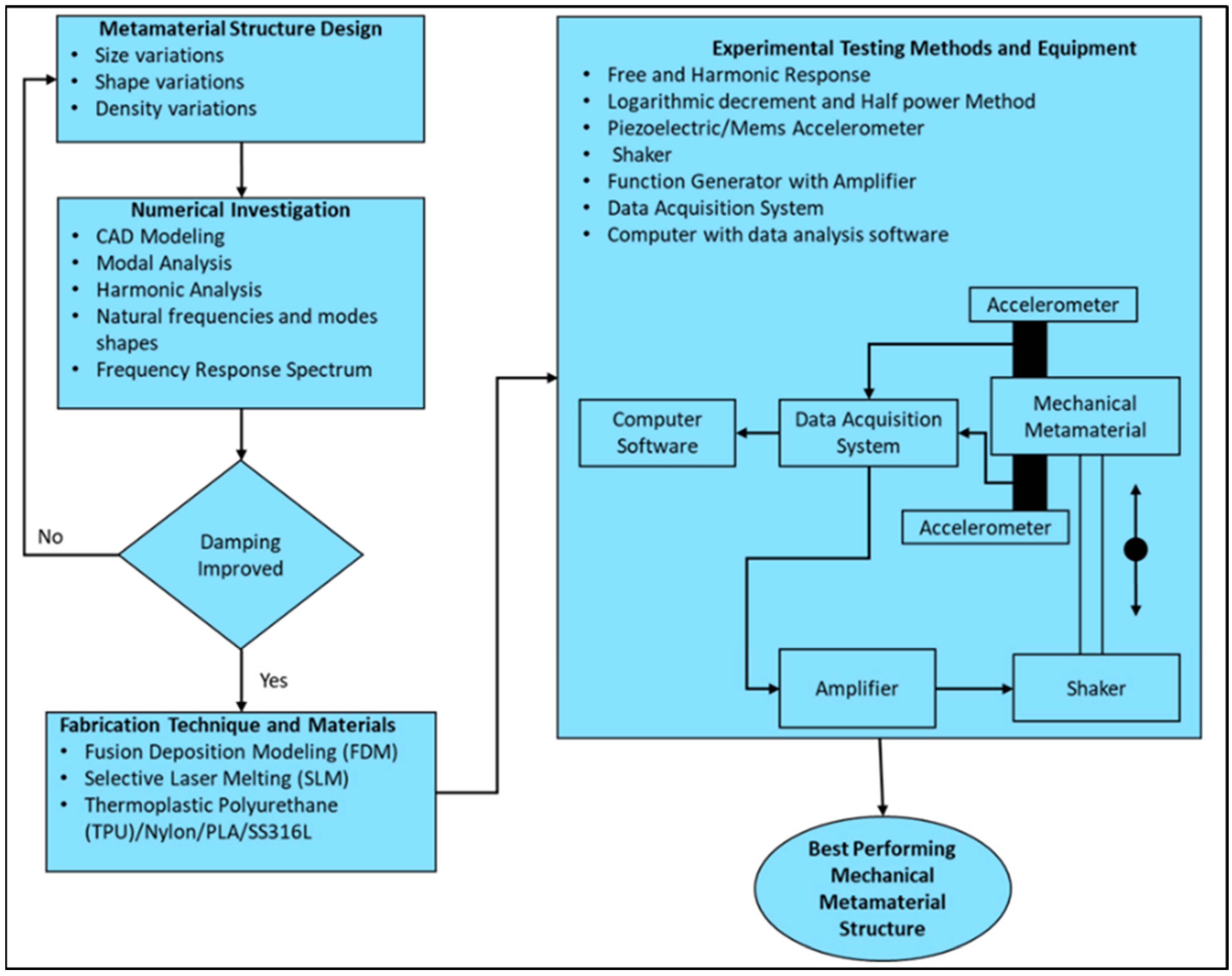
4. Possible Research Gaps and Future Research Systematic Procedure
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Fleck, N.A.; Deshpande, V.S.; Ashby, M.F. Micro-architectured materials: Past, present and future. Proc. R. Soc. A 2010, 466, 2495–2516. [Google Scholar] [CrossRef]
- Ashby, M.F.; Jones, D.R. Engineering Materials 1: An Introduction to Properties, Applications and Design; Elsevier: Amsterdam, The Netherlands, 2012; Volume 1. [Google Scholar]
- Libonati, F.; Buehler, M.J. Advanced structural materials by bioinspiration. Adv. Eng. Mater. 2017, 19, 1600787. [Google Scholar] [CrossRef]
- Levesque, J.N.; Shah, A.; Ekhtiari, S.; Yan, J.R.; Thornley, P.; Williams, D.S. Three-dimensional printing in orthopaedic surgery: A scoping review. EFORT Open Rev. 2020, 5, 430–441. [Google Scholar] [CrossRef]
- Nazir, A.; Abate, K.M.; Kumar, A.; Jeng, J.-Y. A state-of-the-art review on types, design, optimization, and additive manufacturing of cellular structures. Int. J. Adv. Manuf. Technol. 2019, 104, 3489–3510. [Google Scholar] [CrossRef]
- Bauer, J.; Schroer, A.; Schwaiger, R.; Kraft, O. Approaching theoretical strength in glassy carbon nanolattices. Nat. Mater. 2016, 15, 438–443. [Google Scholar] [CrossRef]
- Vyatskikh, A.; Delalande, S.; Kudo, A.; Zhang, X.; Portela, C.M.; Greer, J.R. Additive manufacturing of 3D nano-architected metals. Nat. Commun. 2018, 9, 593. [Google Scholar] [CrossRef]
- Nazir, A.; Gokcekaya, O.; Billah, K.M.M.; Ertugrul, O.; Jiang, J.; Sun, J.; Hussain, S. Multi-material additive manufacturing: A systematic review of design, properties, applications, challenges, and 3D printing of materials and cellular metamaterials. Mater. Des. 2023, 226, 111661. [Google Scholar] [CrossRef]
- Abate, K.M.; Nazir, A.; Jeng, J.-Y. Design, optimization, and selective laser melting of vin tiles cellular structure-based hip implant. Int. J. Adv. Manuf. Technol. 2021, 112, 2037–2050. [Google Scholar] [CrossRef]
- Monkova, K.; Vasina, M.; Zaludek, M.; Monka, P.P.; Tkac, J. Mechanical vibration damping and compression properties of a lattice structure. Materials 2021, 14, 1502. [Google Scholar] [CrossRef]
- Scalzo, F.; Totis, G.; Vaglio, E.; Sortino, M. Experimental study on the high-damping properties of metallic lattice structures obtained from SLM. Precis. Eng. 2021, 71, 63–77. [Google Scholar] [CrossRef]
- Wang, R.; Shang, J.; Li, X.; Luo, Z.; Wu, W. Vibration and damping characteristics of 3D printed Kagome lattice with viscoelastic material filling. Sci. Rep. 2018, 8, 9604. [Google Scholar] [CrossRef]
- Scalzo, F.; Totis, G.; Sortino, M. Influence of the Experimental Setup on the Damping Properties of SLM Lattice Structures. Exp. Mech. 2023, 63, 15–28. [Google Scholar] [CrossRef]
- Zhang, C.; Qiao, H.; Yang, L.; Ouyang, W.; He, T.; Liu, B.; Chen, X.; Wang, N.; Yan, C. Vibration characteristics of additive manufactured IWP-type TPMS lattice structures. Compos. Struct. 2024, 327, 117642. [Google Scholar] [CrossRef]
- Sheng, H.; He, M.-X.; Zhao, J.; Kam, C.T.; Ding, Q.; Lee, H.P. The ABH-based lattice structure for load bearing and vibration suppression. Int. J. Mech. Sci. 2023, 252, 108378. [Google Scholar] [CrossRef]
- Chen, S.; Liu, X.; Hu, J.; Wang, B.; Li, M.; Wang, L.; Zou, Y.; Wu, L. Elastic architected mechanical metamaterials with negative stiffness effect for high energy dissipation and low frequency vibration suppression. Compos. Part B Eng. 2023, 267, 111053. [Google Scholar] [CrossRef]
- Janousek, M.K.B.; Xu, D.; Sureshbabu, A.V.; Zimmermann, M. Vibration reduction by tuned mass dampers inside cavities of topology optimized lattice structures. Proc. Des. Soc. 2023, 3, 3791–3800. [Google Scholar] [CrossRef]
- Richard, S.D.; Liu, R.; Cui, T.J. (Eds.) Metamaterials: Theory, Design, and Applications; Springer: New York, NY, USA, 2010. [Google Scholar]
- Ankit, S. Elastic metamaterials and dynamic homogenization: A review. Int. J. Smart Nano Mater. 2015, 6, 41–60. [Google Scholar]
- Singh, G.; Ni, R.; Marwaha, A. A review of metamaterials and its applications. Int. J. Eng. Trends Technol. 2015, 19, 305–310. [Google Scholar] [CrossRef]
- Kim, K.; Ju, J. Mechanical metamaterials with 3D compliant porous structures. Compos. Struct. 2015, 132, 874–884. [Google Scholar] [CrossRef]
- Aydin, K.; Bulu, I.; Ozbay, E. Subwavelength resolution with a negative-index metamaterial superlens. Appl. Phys. Lett. 2007, 90, 254102. [Google Scholar] [CrossRef]
- Lu, D.; Liu, Z. Hyperlenses and metalenses for far-field super-resolution imaging. Nat. Commun. 2012, 3, 1205. [Google Scholar] [CrossRef] [PubMed]
- Yu, L.; Ji, F.; Guo, T.; Yan, Z.; Huang, Z.; Deng, J.; Tang, C. Ultraviolet thermally tunable silicon magnetic plasmon induced transparency. Opt. Commun. 2025, 575, 131312. [Google Scholar] [CrossRef]
- Tyszka-Zawadzka, A.; Janaszek, B.; Szczepański, P. Tunable slow light in graphene-based hyperbolic metamaterial waveguide operating in SCLU telecom bands. Opt. Express 2017, 25, 7263–7272. [Google Scholar] [CrossRef] [PubMed]
- AbuHussain, M.; Hasar, U.C. Design of X-bandpass waveguide Chebyshev filter based on CSRR metamaterial for telecommunication systems. Electronics 2020, 9, 101. [Google Scholar] [CrossRef]
- Dong, Y.; Itoh, T. Metamaterial-based antennas. Proc. IEEE 2012, 100, 2271–2285. [Google Scholar] [CrossRef]
- Schaedler, T.A.; Jacobsen, A.J.; Torrents, A.; Sorensen, A.E.; Lian, J.; Greer, J.R.; Valdevit, L.; Carter, W.B. Ultralight metallic microlattices. Science 2011, 334, 962–965. [Google Scholar] [CrossRef]
- Li, J.; Fok, L.; Yin, X.; Bartal, G.; Zhang, X. Experimental demonstration of an acoustic magnifying hyperlens. Nat. Mater. 2009, 8, 931–934. [Google Scholar] [CrossRef]
- Liu, Z.; Zhang, X.; Mao, Y.; Zhu, Y.Y.; Yang, Z.; Chan, C.T.; Sheng, P. Locally resonant sonic materials. Science 2000, 289, 1734–1736. [Google Scholar] [CrossRef]
- Yang, Z.; Mei, J.; Yang, M.; Chan, N.H.; Sheng, P. Membrane-type acoustic metamaterial with negative dynamic mass. Phys. Rev. Lett. 2008, 101, 204301. [Google Scholar] [CrossRef]
- Liu, Z.; Chan, C.T.; Sheng, P. Analytic model of phononic crystals with local resonances. Phys. Rev. B Condens. Matter Mater. Phys. 2005, 71, 014103. [Google Scholar] [CrossRef]
- Lei, H.; Li, C.; Meng, J.; Zhou, H.; Liu, Y.; Zhang, X.; Wang, P.; Fang, D. Evaluation of compressive properties of SLM-fabricated multi-layer lattice structures by experimental test and μ-CT-based finite element analysis. Mater. Des. 2019, 169, 107685. [Google Scholar] [CrossRef]
- Geng, L.; Wu, W.; Sun, L.; Fang, D. Damage characterizations and simulation of selective laser melting fabricated 3D re-entrant lattices based on in-situ CT testing and geometric reconstruction. Int. J. Mech. Sci. 2019, 157, 231–242. [Google Scholar] [CrossRef]
- Ma, S.; Tang, Q.; Feng, Q.; Song, J.; Han, X.; Guo, F. Mechanical behaviours and mass transport properties of bone-mimicking scaffolds consisted of gyroid structures manufactured using selective laser melting. J. Mech. Behav. Biomed. Mater. 2019, 93, 158–169. [Google Scholar] [CrossRef] [PubMed]
- Zhong, T.; He, K.; Li, H.; Yang, L. Mechanical properties of lightweight 316L stainless steel lattice structures fabricated by selective laser melting. Mater. Des. 2019, 181, 108076. [Google Scholar] [CrossRef]
- Leary, M.; Mazur, M.; Williams, H.; Yang, E.; Alghamdi, A.; Lozanovski, B.; Zhang, X.; Shidid, D.; Farahbod-Sternahl, L.; Witt, G.; et al. Inconel 625 lattice structures manufactured by selective laser melting (SLM): Mechanical properties, deformation and failure modes. Mater. Des. 2018, 157, 179–199. [Google Scholar] [CrossRef]
- Leary, M.; Mazur, M.; Elambasseril, J.; McMillan, M.; Chirent, T.; Sun, Y.; Qian, M.; Easton, M.; Brandt, M. Selective laser melting (SLM) of AlSi12Mg lattice structures. Mater. Des. 2016, 98, 344–357. [Google Scholar] [CrossRef]
- Che, K.; Yuan, C.; Wu, J.; Qi, H.J.; Meaud, J. Three-dimensional-printed multistable mechanical metamaterials with a deterministic deformation sequence. J. Appl. Mech. 2017, 84, 011004. [Google Scholar] [CrossRef]
- Izard, A.G.; Alfonso, R.F.; McKnight, G.; Valdevit, L. Optimal design of a cellular material encompassing negative stiffness elements for unique combinations of stiffness and elastic hysteresis. Mater. Des. 2017, 135, 37–50. [Google Scholar] [CrossRef]
- Duoss, E.B.; Weisgraber, T.H.; Hearon, K.; Zhu, C.; Small, W.; Metz, T.R.; Vericella, J.J.; Barth, H.D.; Kuntz, J.D.; Maxwell, R.S.; et al. Three-dimensional printing of elastomeric, cellular architectures with negative stiffness. Adv. Funct. Mater. 2014, 24, 4905–4913. [Google Scholar] [CrossRef]
- Frenzel, T.; Findeisen, C.; Kadic, M.; Gumbsch, P.; Wegener, M. Tailored Buckling Microlattices as Reusable Light-Weight Shock Absorbers. Adv. Mater. 2016, 28, 5865–5870. [Google Scholar] [CrossRef]
- Jiao, P.; Mueller, J.; Raney, J.R.; Zheng, X.; Alavi, A.H. Mechanical metamaterials and beyond. Nat. Commun. 2023, 14, 6004. [Google Scholar] [CrossRef]
- Ha, C.S.; Lakes, R.S.; Plesha, M.E. Design, fabrication, and analysis of lattice exhibiting energy absorption via snap-through behavior. Mater. Des. 2018, 141, 426–437. [Google Scholar] [CrossRef]
- Klatt, T.D. Extreme Energy Absorption: The Design, Modeling, and Testing of Negative Stiffness Metamaterial Inclusions. Ph.D. Thesis, The University of Texas at Austin, Austin, TX, USA, 2013. [Google Scholar]
- Shan, S.; Kang, S.H.; Raney, J.R.; Wang, P.; Fang, L.; Candido, F.; Lewis, J.A.; Bertoldi, K. Multistable architected materials for trapping elastic strain energy. Adv. Mater 2015, 27, 4296–4301. [Google Scholar] [CrossRef] [PubMed]
- Sarlis, A.A.; Pasala, D.T.R.; Constantinou, M.C.; Reinhorn, A.M.; Nagarajaiah, S.; Taylor, D.P. Negative stiffness device for seismic protection of structures. J. Struct. Eng. 2013, 139, 1124–1133. [Google Scholar] [CrossRef]
- Cortes, S.; Allison, J.; Morris, C.; Haberman, M.R.; Seepersad, C.C.; Kovar, D. Design, manufacture, and quasi-static testing of metallic negative stiffness structures within a polymer matrix. Exp. Mech. 2017, 57, 1183–1191. [Google Scholar] [CrossRef]
- A Ganilova, O.; Low, J.J. Application of smart honeycomb structures for automotive passive safety. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2018, 232, 797–811. [Google Scholar] [CrossRef]
- Wu, W.; Chen, X.; Shan, Y. Analysis and experiment of a vibration isolator using a novel magnetic spring with negative stiffness. J. Sound Vib. 2014, 333, 2958–2970. [Google Scholar] [CrossRef]
- Meirovitch, L. Fundamentals of Vibrations; Waveland Press: Long Grove, IL, USA, 2010. [Google Scholar]
- Thomson, W. Theory of Vibration with Applications; CRC Press: Boca Raton, FL, USA, 2018. [Google Scholar]
- Chu, J.; Zhou, G.; Liang, X.; Liang, H.; Yang, Z.; Chen, T. A metamaterial for low-frequency vibration damping. Mater. Today Commun. 2023, 36, 106464. [Google Scholar] [CrossRef]
- Zhao, C.; Zhong, J.; Wang, H.; Liu, C.; Li, M.; Liu, H. Impact behaviour and protection performance of a CFRP NPR skeleton filled with aluminum foam. Mater. Des. 2024, 246, 113295. [Google Scholar] [CrossRef]
- El-Borgi, S.; Fernandes, R.; Rajendran, P.; Yazbeck, R.; Boyd, J.; Lagoudas, D. Multiple bandgap formation in a locally resonant linear metamaterial beam: Theory and experiments. J. Sound Vib. 2020, 488, 115647. [Google Scholar] [CrossRef]
- Anigbogu, W.; Bardaweel, H. A metamaterial-inspired structure for simultaneous vibration attenuation and energy harvesting. Shock Vib. 2020, 2020, 4063025. [Google Scholar] [CrossRef]
- Anigbogu, W.; Nguyen, H.; Bardaweel, H. Layered Metamaterial Beam Structures with Local Resonators for Vibration Attenuation: Model and Experiment. Front. Mech. Eng. 2021, 7, 768508. [Google Scholar] [CrossRef]
- Lv, H.; Zhang, Y. A wave-based vibration analysis of a finite Timoshenko locally resonant beam suspended with periodic uncoupled force-moment type resonators. Crystals 2020, 10, 1132. [Google Scholar] [CrossRef]
- Wang, K.; Zhou, J.; Xu, D.; Ouyang, H. Lower band gaps of longitudinal wave in a one-dimensional periodic rod by exploiting geometrical nonlinearity. Mech. Syst. Signal Process. 2019, 124, 664–678. [Google Scholar] [CrossRef]
- Wen, G.; Chen, G.; Long, K.; Wang, X.; Liu, J.; Xie, Y.M. Stacked-origami mechanical metamaterial with tailored multistage stiffness. Mater. Des. 2021, 212, 110203. [Google Scholar] [CrossRef]
- Xu, R.; He, Y.; Li, X.; Lu, M.; Chen, Y. Snap-fit mechanical metamaterials. Appl. Mater. Today 2023, 30, 101714. [Google Scholar] [CrossRef]
- Jang, D.; Greer, J.R. Transition from a strong-yet-brittle to a stronger-and-ductile state by size reduction of metallic glasses. Nat. Mater. 2010, 9, 215–219. [Google Scholar] [CrossRef]
- Gao, H.; Ji, B.; Jäger, I.L.; Arzt, E.; Fratzl, P. Materials become insensitive to flaws at nanoscale: Lessons from nature. Proc. Natl. Acad. Sci. USA 2003, 100, 5597–5600. [Google Scholar] [CrossRef]
- Moruzzi, M.C.; Cinefra, M.; Bagassi, S. Vibroacoustic analysis of an innovative windowless cabin with metamaterial trim panels in regional turboprops. Mech. Adv. Mater. Struct. 2021, 28, 1509–1521. [Google Scholar] [CrossRef]
- Dalela, S.; Balaji, P.S.; Jena, D.P. A review on application of mechanical metamaterials for vibration control. Mech. Adv. Mater. Struct. 2022, 29, 3237–3262. [Google Scholar] [CrossRef]
- Lv, H.; Zhang, R.; Chen, C.; Ma, H.; Huang, X.; Yu, Z. Vibration Analysis of Locally Resonant Beams with L-Joint Using an Exact Wave-Based Vibration Approach. Materials 2023, 16, 2276. [Google Scholar] [CrossRef] [PubMed]
- Ji, J.; Luo, Q.; Ye, K. Vibration control based metamaterials and origami structures: A state-of-the-art review. Mech. Syst. Signal Process. 2021, 161, 107945. [Google Scholar] [CrossRef]
- Sebaq, M.H.; Liu, Z. Energy absorption and vibration mitigation performances of novel 2D auxetic metamaterials. Int. J. Comput. Mater. Sci. Eng. 2024, 13, 2350022. [Google Scholar] [CrossRef]
- Xu, C.; Li, Q.; Zhang, L.; Liu, Q.; Ren, L. Glass sponge-inspired auxetic mechanical metamaterials for energy absorption. J. Bionic Eng. 2024, 21, 2349–2365. [Google Scholar] [CrossRef]
- Saddek, A.A.; Lin, T.-K.; Chang, W.-K.; Chen, C.-H.; Chang, K.-C. Metamaterials of auxetic geometry for seismic energy absorption. Materials 2023, 16, 5499. [Google Scholar] [CrossRef]
- Hu, G.; Tang, L.; Das, R. Internally coupled metamaterial beam for simultaneous vibration suppression and low frequency energy harvesting. J. Appl. Phys. 2018, 123, 055107. [Google Scholar] [CrossRef]
- Zhang, Y.; Fan, X.; Li, J.; Li, F.; Yu, G.; Zhang, R.; Yuan, K. Low-frequency vibration insulation performance of the pyramidal lattice sandwich metamaterial beam. Compos. Struct. 2021, 278, 114719. [Google Scholar] [CrossRef]
- Correa, D.M.; Seepersad, C.C.; Haberman, M.R. Mechanical design of negative stiffness honeycomb materials. Integr. Mater. Manuf. Innov. 2015, 4, 165–175. [Google Scholar] [CrossRef]
- Ha, C.S.; Lakes, R.S.; Plesha, M.E. Cubic negative stiffness lattice structure for energy absorption: Numerical and experimental studies. Int. J. Solids Struct. 2019, 178, 127–135. [Google Scholar] [CrossRef]
- Al Rifaie, M.; Abdulhadi, H.; Mian, A. Advances in mechanical metamaterials for vibration isolation: A review. Adv. Mech. Eng. 2022, 14, 16878132221082872. [Google Scholar] [CrossRef]
- Wang, Y.; Yang, F.; Yang, J.-S.; Tong, L.-L.; Liu, Q.; Schmidt, R.; Schröder, K.-U. A new periodic seismic metamaterial with ultra-low bandgap for lamb waves. Mech. Adv. Mater. Struct. 2023, 31, 7742–7752. [Google Scholar] [CrossRef]
- Zhang, L.; Bai, Z.; Chen, Y. Multi-objective design and optimization of stretching-dominated plate-based mechanical metamaterials for simultaneous vibration insulation and energy absorption. Mech. Adv. Mater. Struct. 2022, 31, 2759–2771. [Google Scholar] [CrossRef]
- Lv, H.; Li, S.; Huang, X.; Yu, Z. Vibration analysis of a finite lightweight locally resonant beam suspended with periodic force-moment-type resonators inside using an exact wave-based approach. Symmetry 2022, 14, 1542. [Google Scholar] [CrossRef]
- Lv, H.; Li, S.; Huang, X.; Yu, Z. Band-gap properties of finite locally resonant beam suspended periodically with two-degree-of-freedom force type resonators. Crystals 2021, 11, 716. [Google Scholar] [CrossRef]
- Wu, Z.; Liu, W.; Li, F.; Zhang, C. Band-gap property of a novel elastic metamaterial beam with X-shaped local resonators. Mech. Syst. Signal Process. 2019, 134, 106357. [Google Scholar] [CrossRef]
- Li, Q.; Sheng, M. An improved method for bandgap calculation of a locally resonant plate with multi-periodic of multiple degree-of-freedom resonators. J. Appl. Phys. 2021, 129, 245110. [Google Scholar] [CrossRef]
- Zhu, R.; Liu, X.; Hu, G.; Sun, C.; Huang, G. A chiral elastic metamaterial beam for broadband vibration suppression. J. Sound Vib. 2014, 333, 2759–2773. [Google Scholar] [CrossRef]
- Jian, Y.; Hu, G.; Tang, L.; Tang, W.; Abdi, M.; Aw, K.C. Analytical and experimental study of a metamaterial beam with grading piezoelectric transducers for vibration attenuation band widening. Eng. Struct. 2023, 275, 115091. [Google Scholar] [CrossRef]
- Fabro, A.T.; Beli, D.; Ferguson, N.S.; Arruda, J.R.F.; Mace, B.R. Wave and vibration analysis of elastic metamaterial and phononic crystal beams with slowly varying properties. Wave Motion 2021, 103, 102728. [Google Scholar] [CrossRef]
- Chen, Y.; Li, T.; Scarpa, F.; Wang, L. Lattice metamaterials with mechanically tunable Poisson’s ratio for vibration control. Phys. Rev. Appl. 2017, 7, 024012. [Google Scholar] [CrossRef]
- Li, J.; Fan, X.; Li, F. Numerical and experimental study of a sandwich-like metamaterial plate for vibration suppression. Compos. Struct. 2020, 238, 111969. [Google Scholar] [CrossRef]
- Bukhari, M.A.; Barry, O.R. Towards a self tuning sliding mass metastructure. Sci. Rep. 2021, 11, 21630. [Google Scholar] [CrossRef] [PubMed]
- Bae, J.-S.; Hwang, J.-H.; Kwag, D.-G.; Park, J.; Inman, D.J. Vibration suppression of a large beam structure using tuned mass damper and eddy current damping. Shock Vib. 2014, 2014, 893914. [Google Scholar] [CrossRef]
- Chen, J.; Lu, G.; Li, Y.; Wang, T.; Wang, W.; Song, G. Experimental study on robustness of an eddy current-tuned mass damper. Appl. Sci. 2017, 7, 895. [Google Scholar] [CrossRef]
- Cheng, T.-H.; Oh, I.-K. Vibration suppression of flexible beam using electromagnetic shunt damper. IEEE Trans. Magn. 2009, 45, 2758–2761. [Google Scholar] [CrossRef]
- Chen, S.; Wang, B.; Zhu, S.; Tan, X.; Hu, J.; Lian, X.; Wang, L.; Wu, L. A novel composite negative stiffness structure for recoverable trapping energy. Compos. Part A Appl. Sci. Manuf. 2020, 129, 105697. [Google Scholar] [CrossRef]
- Tan, X.; Wang, B.; Chen, S.; Zhu, S.; Sun, Y. A novel cylindrical negative stiffness structure for shock isolation. Compos. Struct. 2019, 214, 397–405. [Google Scholar] [CrossRef]
- Kovacic, I.; Rakaric, Z.; Kanovic∗, Z.; Rajs, V. Metastructure with integrated internal oscillators of constant, linearly and nonlinearly varying natural frequency. Front. Phys. 2022, 10, 934998. [Google Scholar] [CrossRef]
- Tan, X.; Chen, S.; Wang, B.; Tang, J.; Wang, L.; Zhu, S.; Yao, K.; Xu, P. Real-time tunable negative stiffness mechanical metamaterial. Extrem. Mech. Lett. 2020, 41, 100990. [Google Scholar] [CrossRef]
- Jones, D.I. Handbook of Viscoelastic Vibration Damping; John Wiley & Sons: Hoboken, NJ, USA, 2001. [Google Scholar]
- Rao, S.S.; Yap, F.F. Mechanical Vibrations; Addison-Wesley: New York, NY, USA, 1995; Volume 4. [Google Scholar]
- Simsek, U.; Arslan, T.; Kavas, B.; Gayir, C.E.; Sendur, P. Parametric studies on vibration characteristics of triply periodic minimum surface sandwich lattice structures. Int. J. Adv. Manuf. Technol. 2021, 115, 675–690. [Google Scholar] [CrossRef]
- Carrella, A.; Brennan, M.J.; Waters, T.P.; Lopes, V., Jr. Force and displacement transmissibility of a nonlinear isolator with high-static-low-dynamic-stiffness. Int. J. Mech. Sci. 2012, 55, 22–29. [Google Scholar] [CrossRef]
- Ab Latif, N.; Rus, A.Z.M. Vibration transmissibility study of high density solid waste biopolymer foam. J. Mech. Eng. Sci. 2014, 6, 772–781. [Google Scholar] [CrossRef]
- Kelly. Mechanical Vibrations: Theory and Applications; Cengage Learning: Boston, MA, USA, 2012. [Google Scholar]
- Liu, K.; Liu, J. The damped dynamic vibration absorbers: Revisited and new result. J. Sound Vib. 2005, 284, 1181–1189. [Google Scholar] [CrossRef]
- Hadas, Z.; Ondrusek, C. Nonlinear spring-less electromagnetic vibration energy harvesting system. Eur. Phys. J. Spec. Top. 2015, 224, 2881–2896. [Google Scholar] [CrossRef]
- Ewins, D.J. Modal Testing: Theory, Practice and Application; John Wiley & Sons: Hoboken, NJ, USA, 2009. [Google Scholar]
- Medel, F.; Abad, J.; Esteban, V. Stiffness and damping behavior of 3D printed specimens. Polym. Test. 2022, 109, 107529. [Google Scholar] [CrossRef]
- Gietl, J.; Vignola, J.; Sterling, J.; Ryan, T. Characterization of damping properties in 3D printed structures. J. Phys. Conf. Ser. 2018, 1149, 012002. [Google Scholar] [CrossRef]
- Samimi, A.H.; Karamooz-Ravari, M.R.; Dehghani, R. Numerical and Experimental Investigation of Natural Frequency and Damping Coefficient of Flexible Cellular Lattice Structures. Int. J. Adv. Des. Manuf. Technol. 2024, 17, 29. [Google Scholar]
- He, F.; Ning, H.; Khan, M. Effect of 3D Printing Process Parameters on Damping Characteristic of Cantilever Beams Fabricated Using Material Extrusion. Polymers 2023, 15, 257. [Google Scholar] [CrossRef]
- Trujillo, M.; Corral, L.; Curtin, M.; Abdelkefi, A. Comparative investigations on the dynamical responses of ABS and PLA additively-manufactured beams. Int. J. Non-Linear Mech. 2023, 157, 104558. [Google Scholar] [CrossRef]
- Palmieri, M.; Zucca, G.; Morettini, G.; Landi, L.; Cianetti, F. Vibration fatigue of FDM 3D printed structures: The use of frequency domain approach. Materials 2022, 15, 854. [Google Scholar] [CrossRef]
- Nashif, A.D.; Jones, D.I.; Henderson, J.P. Vibration Damping; John Wiley & Sons: Hoboken, NJ, USA, 1991. [Google Scholar]
- Kovacic, I.; Teofanov, L.; Kanovic, Z.; Zhao, J.; Zhu, R.; Rajs, V. On the influence of internal oscillators on the performance of metastructures: Modelling and tuning conditions. Mech. Syst. Signal Process. 2023, 205, 110861. [Google Scholar] [CrossRef]
- Azmi, M.S.; Ismail, R.; Hasan, R. Investigation on the static and dynamic behavior of BCC lattice structure with quatrefoil node manufactured using fused deposition modelling additive manufacturing. IOP Conf. Ser. Mater. Sci. Eng. 2020, 788, 012008. [Google Scholar] [CrossRef]



| Reference | Structure(s) | Investigation/Applications | Key Findings |
|---|---|---|---|
| El-Borgi et al. [55] | Metamaterial beams | Bandgap formation | Observed two bandgaps from a numerical and experimental study |
| Ji et al. [67] | Metamaterials (auxetic, bandgap, and pentamode) and origami-based structures | Vibration mitigation and isolation | Reviewed advances in metamaterials and origami-based structures with future research directions |
| Anigbogu et al. [56] | Structure with periodic local resonators | Vibration attenuation and energy harvesting | Observed two bandgaps: 205–257 Hz and 587–639 Hz |
| Al Rifaie et al. [75] | Porous materials (PMs), periodic cellular structures (PCSs), and lattice cellular structures (LCSs) | Vibration isolation | Explored modeling, mechanical properties, and vibration techniques for PMs, PCSs, and LCSs with future proposed studies |
| Dalela et al. [65] | Star-shaped unit cell, HSLDS resonator, sinusoidal beam, and LR beam with X-shaped resonator | Stopband enhancement and broadening in varying frequency range | Explored MM development and focused on passive and active techniques to control vibration |
| Wang et al. [76] | Square cross-section with curved boundaries | Buildings’ seismic isolation and attenuation | Found that the proposed structure has excellent damping properties in a low-frequency band |
| Zhang et al. [77] | Stretch plate-based MM | Vibration isolation and energy absorption | Found that the metamaterial structure S8 is best for both vibration isolation and absorption |
| Anigbogu et al. [57] | Layered metamaterial structure | Frequency bandgap formation | Concluded that the local resonator’s length influences the shape and location of the local resonator frequency bandgap. |
| Hu et al. [71] | Internally coupled metamaterial | Energy harvesting and Vibration suppression in low-frequency | Observed that the new metamaterial model enhances energy harvesting and vibration suppression |
| Zhang et al. [72] | Pyramidal-truss metamaterial beam | Low-frequency vibration insulation | The position and bandgap width are affected by the resonator’s natural frequency, mass ratio, and the truss inclination angle; moreover, the proposed structure can obtain much broader bandgaps, with the same host beam structure, lattice constant, and resonator total masses in each unit cell |
| Lv et al. [78] | Finite lightweight locally resonant beam | Aerospace space-arm and antennas framework | The designed structure’s resonator mass and stiffness are more sensitive in achieving a broad-width frequency bandgap |
| Lv et al. [58] | Timoshenko beam with periodic 2-DOF uncoupled force-moment type resonators | Low-frequency range bandgaps | The study found an efficient approach to achieving broadband low-frequency bandgaps |
| Lv et al. [66] | L-joint LR beam | Vibration attenuation in lower-frequency range | Observed that the proposed structure can attenuate the propagation of the axial waves in the low-frequency range |
| Lv et al. [79] | Periodic 2-DOF force-type resonators | Vibration attenuation | Concluded that the resonator mass and stiffness affect the bandgap properties |
| Wang et al. [59] | Periodic rod with HSLDS resonator | Low-frequency bandgap | Creation of a very low-frequency bandgap for longitudinal waves propagating along the rod |
| Wu et al. [80] | Elastic metamaterial beam having X-shaped resonators | Bandgap adjustment and vibration control | The initial angle, length ratio, and layer number are important to manage the bandgap characteristics |
| Li et al. [81] | LR plate with multiple arrays of multi-DOF resonators | Broadband vibration suppression | The improved plane-wave expansion and extended plane-wave expansion approaches have outstanding efficiency and broad applicability for the proposed structure |
| Zhu et al. [82] | Chiral lattice elastic metamaterial beam | Vibration broadband suppression | The proposed structure’s experimental testing is performed to verify the design |
| Jian et al. [83] | Metamaterial-graded piezoelectric transducer beam | Vibration attenuation band broadening | Found that for the power spectral density of a random input, the excitation was 0.001 G2/Hz, and the RMS acceleration amplitude at the beam tip could be attenuated to 0.38 G |
| Fabro et al. [84] | Metamaterial and phononic crystals | Vibration suppression | Showed that the slowly changing method is appropriate to represent the ensemble statistics of bandgaps |
| Chen et al. [85] | Sinusoidally shaped lattice | Broadband vibration mitigation | Reported extreme Poisson’s ratio fluctuations between −0.7 and 0.5 over large tensile deformations of up to 50% for the proposed structure |
| Li et al. [86] | Sandwich-like metamaterial | Vibration attenuation and isolation | Showed that the stopband is affected by the resonator’s natural frequency and the mass ratio; the stopband width is mostly affected by the resonator’s damping ratio |
| Bukhari et al. [87] | Sliding mass metastructure | Wide-frequency-range vibration reduction | Showed that the resonator can adjust itself with external frequency once the slider achieves equilibrium position |
| Bae et al. [88] | Large beam structure | Vibration suppression | Showed that the present approach is effective in the vibration suppression of a large beam structure without the addition of significant weight |
| Chen et al. [89] | Cantilever beam | Vibration control | Found that the present structure with a tuned mass damper experiences 1.63 to 2.99 times the maximum vibration of that of an eddy current-tuned mass damper |
| Cheng et al. [90] | Flexible cantilever beams | Vibration suppression | Found that the magnet shunt damper can be used without any issue to reduce the vibration of flexible structures |
| Izard et al. [40] | Three-spring model | Energy dissipation | Observed that proposed materials show a very high Young’s modulus and damping combination, far better than those of the constituent phase |
| Correa et al. [73] | NS honeycomb structure | Mechanical energy dissipation | Found that the proposed structure may be modeled to dissipate mechanical energy comparable to traditional designs at relatively lower densities |
| Chen et al. [91] | Composite NS structure | Shock isolation and vibration control | Found from the impact tests that the proposed structure has good cushioning properties by tuning the acceleration threshold response and is reusable after snap-through behavior takes place |
| Ha et al. [74] | Cubic NS lattice structure | Energy absorption | Concluded that the proposed structure can absorb mechanical energy with full geometry recovery in all directions, and its energy absorption increases with its dimensions |
| Tan et al. [92] | Cylindrical NS structure | Shock isolation | Concluded from the impact tests that the cylindrical NS structure achieved good cushioning performance by adjusting the acceleration magnitude threshold after the snap-through behavior took place |
| Kovacic et al. [93] | Metastructure with integrated internal oscillators | Vibration attenuation | Observed that the proposed structure’s natural frequency is increased linearly and nonlinearly along the structure in line with new theoretical results |
| Xu et al. [61] | Polymeric snap-fit MM | Energy absorption | Found that the proposed structures achieve excellent impact resistance and energy absorption ability, so they can be assumed to be a suitable candidate for the development of shock absorbers |
| Tan et al. [94] | Pneumatically actuated tunable NS mechanical metamaterial | Vibration isolation and energy absorption | Mentioned that the multistage pattern transformation can be obtained through pneumatic actuation |
| Wen et al. [60] | ZBSO metamaterial | Energy absorption | Found that the proposed structure has many excellent advantages in comparison with the conventional mechanical metamaterials, i.e., material-independent, scale-invariant, and lightweight properties and excellent energy absorption capability |
| Reference | Study Nature | Lattice Structure | Manufacturing Technique/Material |
|---|---|---|---|
| Monkova et al. [10] | Experimental work on vibration damping compression properties | BCC lattice structure | FDM technique/polymer acrylonitrile butadiene styrene (ABS) |
| Scalzo et al. [11] | Experimental and numerical work on damping properties | FBCCZ lattice structure | SLM technique/AISI 316L, AlSi10Mg |
| Wang et al. [12] | Experimental and numerical vibration and damping characteristics bandgap design | Kagome lattice structure | SLM technique/polymer nylon PA6, thermoplastic polyurethane as viscoelastic filling material |
| Scalzo et al. [13] | Experimental work on damping properties | FBCCZ Lattice Structure | SLM Technique/AISI 316L |
| Zhang et al. [14] | Experimental work on compression testing and dynamic vibration rate transfer testing | IWP TPMS lattice structure | SLM technique |
| Chu et al. [53] | Numerical, low-frequency damping | Rectangular sheet with conical spring | Lead and polymer nylon |
| Sheng et al. [15] | Simulations and experiments on high load-bearing capability and vibration suppression | SC-BCC-ABH lattice structure SC latticeBCC supportABH acoustic black hole | SLS technique/polymer PA12 nylon powder |
| Chen et al. [16] | Numerical and experimental work on low-frequency vibration suppression | NS metamaterial | SLS technique/polymer thermoplastic urethane |
| Janousek et al. [17] | Simulations of vibration reduction | Unit cell cavity lattice with TMD; a robotic arm was made from it | SLA resin material TOUGH1500 |
| Simsek et al. [97] | Experimental work on compression testing and dynamic vibration rate transfer testing | Gyroid structures | Concept laser M2 (metal 3D-printing system) with high-temperature alloy named Haynes® 188 (HS188) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fayyaz; Bashmal, S.; Nazir, A.; Khan, S.; Alofi, A. Damping Optimization and Energy Absorption of Mechanical Metamaterials for Enhanced Vibration Control Applications: A Critical Review. Polymers 2025, 17, 237. https://doi.org/10.3390/polym17020237
Fayyaz, Bashmal S, Nazir A, Khan S, Alofi A. Damping Optimization and Energy Absorption of Mechanical Metamaterials for Enhanced Vibration Control Applications: A Critical Review. Polymers. 2025; 17(2):237. https://doi.org/10.3390/polym17020237
Chicago/Turabian StyleFayyaz, Salem Bashmal, Aamer Nazir, Sikandar Khan, and Abdulrahman Alofi. 2025. "Damping Optimization and Energy Absorption of Mechanical Metamaterials for Enhanced Vibration Control Applications: A Critical Review" Polymers 17, no. 2: 237. https://doi.org/10.3390/polym17020237
APA StyleFayyaz, Bashmal, S., Nazir, A., Khan, S., & Alofi, A. (2025). Damping Optimization and Energy Absorption of Mechanical Metamaterials for Enhanced Vibration Control Applications: A Critical Review. Polymers, 17(2), 237. https://doi.org/10.3390/polym17020237







