1. Introduction
High-density polyethylene (HDPE) has emerged as a cornerstone material in modern engineering, renowned for its versatility and reliability across a broad spectrum of applications. Its use in constructing pressure-bearing infrastructure, including natural gas pipelines, district heating systems, and medical-grade fluid transfer lines, is a direct result of its exceptional mechanical properties, ease of processing, and robust corrosion resistance [
1]. The mechanical characteristics such as strength and elastic modulus affect the structural strength and service life of HDPE-based pressure equipment. As such, understanding its failure mechanisms and mechanical behaviors has become a central focus of research in both academic and industrial settings.
In recent years, significant strides have been made in the study of HDPE’s mechanical properties. For example, through advanced spectroscopic techniques, researchers like Reis et al. [
2] have demonstrated the effect of strain rate and temperature on the mechanical properties of HDPE through a series of tensile tests. In another study, Briody et al. [
3] reported the applicability of the superelastic and viscoelastic constitutive laws. Additionally, Grytten et al. [
4] employed digital image correlation methods to precisely measure the surface strains of HDPE samples under various loading conditions, providing valuable experimental data for validating theoretical models.
However, despite these achievements, there are still several critical gaps in our current knowledge. In the realm of testing methods, the uniaxial tensile test remains a widely used standard for determining mechanical properties. While it offers simplicity and convenience, it is deeply rooted in the assumptions of classical material mechanics and fails to mimic the complex stress states that HDPE experiences in real-world applications [
5,
6]. In practical scenarios, pressure-bearing structures such as pipelines and storage vessels are often subjected to multi-axial and symmetric constraints, placing the HDPE material in a plane-stress state. The mechanical parameters obtained from uniaxial tensile tests, such as Young’s modulus and ultimate tensile strength, can deviate significantly from the values relevant to actual service conditions [
7,
8]. This discrepancy can lead to inaccurate predictions of material behavior and potential safety risks in engineering designs.
The small punch test (SPT) was developed to address the limitations of uniaxial testing for small specimens under plane-stress conditions. Nevertheless, it is not without its own problems. One of the major challenges is the friction between the specimen and the loading device. This friction can introduce additional forces and moments during the test, complicating the stress distribution within the specimen and making it difficult to accurately interpret the test results. Moreover, numerical analysis of SPT data is often hindered by the complex nature of friction-related effects, which are difficult to model precisely [
9,
10]. Some alternative methods, like the one proposed by Wang [
11], have attempted to overcome these issues but are limited in their applicability. Wang’s method, for instance, is only suitable for metallic materials due to the unique challenges posed by polymers, such as their susceptibility to chemical degradation under certain testing conditions and their sensitivity to over-pressurization [
12,
13,
14].
Regarding constitutive models, HDPE exhibits a highly complex mechanical response that defies simple characterization. The traditional linear elastic constitutive model, based on Young’s modulus and Poisson’s ratio, is inadequate for capturing the nonlinear elastic and plastic behaviors of HDPE, especially during large deformation and post-yielding processes [
15]. More advanced constitutive models, such as the Marlow model and the Arruda–Boyce model [
16], have been proposed to account for the nonlinearity. However, these models still struggle to incorporate all the factors that influence HDPE’s mechanical behavior. For example, the effects of temperature, strain rate, and material microstructure on HDPE’s mechanical properties are not fully considered in these models. Temperature can significantly alter the viscoelastic properties of HDPE, while strain rate can affect its yield strength and ductility. The complex microstructure of HDPE, including its degree of crystallinity and the distribution of crystallites, also plays a crucial role in determining its mechanical response but is often oversimplified in existing models [
17,
18].
Against this backdrop, the present study aims to develop an improved testing method, the small specimen bulging test (SBT), for evaluating the mechanical properties of small HDPE specimens. The main advantage of this method is its ability to evaluate the mechanical properties of materials under symmetric constraint conditions. Compared to traditional uniaxial tensile tests, the underlying micromechanisms of deformation under symmetric constraints are different, leading to significant variations in the resulting mechanical performance assessments. This approach more closely simulates the actual service conditions of equipment such as pipelines and pressure vessels, which operate under plane-stress states, thereby enabling a more accurate prediction of material behavior under real-world operating conditions. By carefully selecting appropriate constitutive relations and deformation parameters for fitting and using the finite element simulation software ABAQUS to simulate the experimental process, we seek to establish a more accurate mechanical response model for HDPE. This model will not only better capture the material’s behavior under realistic stress conditions but also provide a more reliable basis for engineering design and material selection, thereby filling in the existing scientific gaps and addressing the practical needs in the field.
2. Experimental Section
2.1. Specimen Preparation
In accordance with the ASTM F 2183-02 standards [
19], we prepared high-density polyethylene (HDPE) specimens for the experiments. The HDPE raw material used was M7600 supplied by SINOPEC (Beijing, China). Specimens were produced via a bar-extruded process at 165 °C. The HDPE material was first processed into 1 mm thick sheets. Then, using a 10 mm diameter punch, circular disk specimens with a diameter of approximately 10 mm and a thickness of approximately 1.0 mm were carefully cut from the sheets to ensure accurate dimensions. After cutting, the edges of the specimens were polished to achieve a smooth surface finish. Surface roughness was measured and recorded to ensure that it fell within the specified range. A total of three specimens were prepared in this manner to ensure the statistical significance of the experimental results.
2.2. Experimental Setup
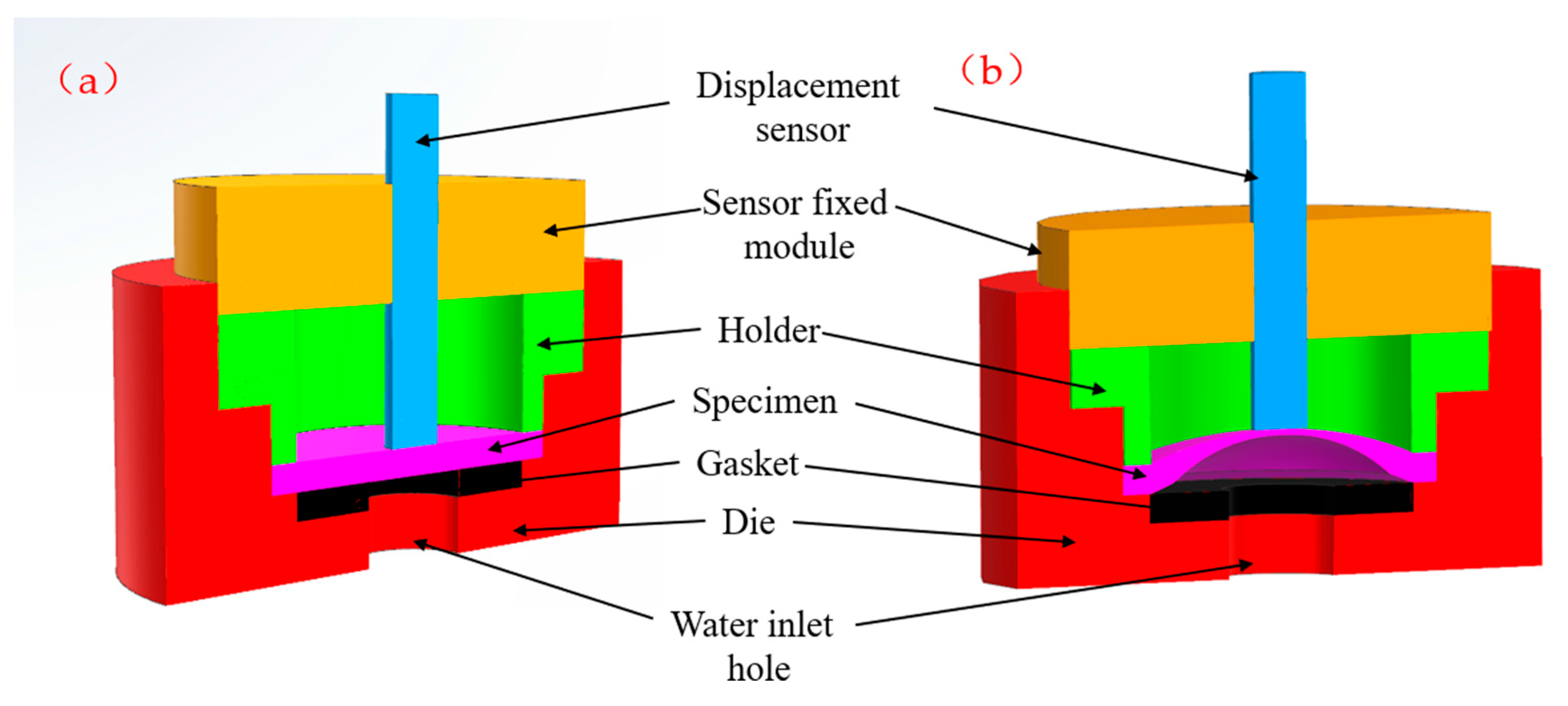
The experimental setup consisted of a hydraulic system, a high-precision pressure sensor, a high-accuracy displacement sensor, and a data-acquisition system. The hydraulic system was used to apply pressure to the specimens.
Figure 1 presents the experimental device, clearly showing its key components and how it is connected to the rest of the experimental setup. The machine is self-developed. The test parameters are set as follows: initial pressure of 0.5 MPa, test temperature at room temperature, and pressure increase rate of 0.2 MPa/s. The high-precision pressure sensor, with an accuracy of ±0.05 MPa and a working range up to 150 MPa, was installed in the hydraulic line to measure the pressure applied to the specimen precisely. The high-accuracy displacement sensor, with a resolution of 0.001 mm and a range of 7.5 mm, was positioned to measure the displacement of the specimen at the center point during the test. Additionally, a pressure pump capable of providing a maximum pressure of 25 MPa with an accuracy of ±0.05 MPa was used. The data-acquisition system, with a sampling rate of 200 Hz, was used to collect the data from the pressure and displacement sensors in real time.
2.3. Experimental Procedure
Before the test, each specimen was carefully placed in the testing apparatus, ensuring proper alignment and secure fixation. The holder and die, made of steel and aluminum alloy, respectively, were designed to provide the necessary support and confinement for the specimen during the hydraulic bulging process. The contact between the specimen and the holder/die was modeled as a contact-pair with appropriate friction coefficients in the subsequent finite element method (FEM) simulations.
During the test, the hydraulic pressure gradually increased at a controlled rate. The pressure-increasing rate was set based on preliminary tests, ensuring that the deformation process of the specimen was quasi-static. The pressure–displacement data were continuously recorded by the data-acquisition system throughout the test until the specimen was fractured. The fracture area of the specimens was consistently located at the center, indicating a relatively uniform stress distribution on the surface and a tendency towards a state of minimum surface strain.
2.4. FEM Model Integration
A detailed FEM model should be determined by the experimental results, including FEM mesh, boundary conditions, and a description of the used settings and elements. The FEM model scheme is as follows.
Boundary Conditions
Displacement Boundary Conditions:
At the bottom surface of the holder, all degrees of freedom (translational in the
x,
y, and
z directions and rotational around the
x,
y, and
z axes) are constrained, as shown in Equations (1) and (2).
where
u is the displacement and
θ is the angle of rotation.
This mimics the fixed-base condition in the actual experimental setup where the holder is firmly mounted.
For the die, the outer-most surface that is not in contact with the specimen is also fixed in all degrees of freedom, ensuring its stability during the simulation and enabling it to apply the required pressure on the specimen.
Pressure Boundary Conditions:
A uniformly distributed pressure load, equivalent to the hydraulic pressure in the experiment, is applied on the inner surface of the die that contacts the HDPE specimen. The pressure value is based on the experimental data and gradually increased during the simulation to replicate the loading process.
Supports:
The holder acts as the main support for the specimen. In the FEM model, its support function is represented by the fixed-displacement boundary conditions at its bottom surface.
Small support pads are assumed between the specimen and the holder at the specimen’s edges. In the FEM model, the interaction between the specimen and these support pads is modeled as a contact-pair with a high-friction coefficient to prevent relative motion.
Load Application:
The load on the HDPE specimen is the hydraulic pressure transmitted through the die. It is applied step-by-step, with each step corresponding to a specific time increment in the simulation.
The pressure load direction is always normal to the inner surface of the die in contact with the specimen, consistent with the physical hydraulic bulging process. A small random variation in the pressure distribution, based on experimental measurements of pressure uniformity, is introduced to make the simulation more realistic.
2.5. Experimental Data Analysis
The recorded pressure–displacement curves were analyzed in detail. We calculated various parameters from these curves, such as the initial elastic modulus, yield point, and ultimate strength of the HDPE specimens. The thickness reduction–pressure curve was also obtained and found to be fitted well by an exponential equation. This finding not only validates the feasibility of the numerical analysis but also provides valuable insights into the material’s behavior under loading.
To ensure the reliability of the experimental results, we conducted a comprehensive statistical analysis. For each measured parameter, we calculated the minimum, maximum, median, mean, range, standard deviation, and measurement uncertainty. The results of this statistical analysis were presented in a table, which clearly shows the distribution and variability of the data.
2.6. Comparison with FEM Simulations
The experimental data were compared with the results obtained from the FEM simulations. Both the pressure–displacement curves from the experiments and simulations exhibited a rise–fall–rise process, indicating that the FEM simulations could adequately describe the experimental data. However, some minor differences were also observed, which were further analyzed in the
Section 4. These differences were mainly attributed to factors such as the simplification of the FEM model, the assumptions in the material constitutive model, and the manufacturing tolerances of the specimens.
4. Discussion
In this paper, the overestimation of the early-stage displacement growth rate is mainly due to the simplification of the finite element model, like treating HDPE’s non-uniform microstructure as homogeneous, and assumptions in the Marlow constitutive model, which fails to capture the complex elastic–plastic transition at the start of loading. This overestimation distorts the analysis of the experimental results, such as miscalculating the modulus of elasticity, and undermines the accuracy of the conclusions, potentially misguiding product safety assessment and future research. To rectify this, we propose model refinement by incorporating detailed micro-structural information and adjusting mesh density, modifying the constitutive model by adding parameters or coupling with other models, and conducting extensive calibration and validation using diverse experimental data and in situ microscopy techniques.
4.1. Experimental Method and Analysis of Deformation Mechanism
Figure 8 shows the pressure–displacement curves of the HDPE sample obtained by the finite element simulation and corresponding experimental results. As can be observed, both methodologies exhibit the same trend prior to failure. Based on the definition of the parameter Ꞁ determined by Equation (3), Ꞁ goes through the process of rising–descending–rising in both curves. This indicates that the finite element simulation can describe the experimental data well, along with revealing the characteristics of the above stages. The main error between the simulation and experimental results is that the finite element method overestimates the top displacement growth rate during the initial loading stage. The predicted value is slightly higher than the experimental data at the end of stage 1 for the different pressurization conditions. However, the observed error is less than 7% and has been effectively corrected in the subsequent analysis. Therefore, the simulation results obtained in this study can be confirmed to be accurate.
Based on
Figure 3 and
Figure 4, the behavior of the pressure–displacement curve obtained from the hydraulic bulging test can be analyzed as follows:
In stage 1 (A–B section), owing to the initial loading stage, the bulging height and Ꞁ rise rapidly with pressure, thus indicating that in the initial stage of stage 1 (A–B section), the bulge height increases slowly with the increase in pressure and the growth rate accelerates in the later stage. In stage 1 (A–B section), the deformation of the material basically conforms to Hooke’s law [
27,
28]. Therefore, the material exhibits a linear elastic deformation at this stage. The deformation mechanism is mainly the internal rotation of the flexible macromolecular chain, leading to the reduced chain motion and conformational number [
29,
30].
In stage 2 (B–C section), the displacement of the sample is linear as a function of pressure. However, at this stage, Ꞁ is lower than stage 1, and the bulging height of the material gradually slows down on increasing the pressure. Thus, the deformation of the material cannot be recovered, thus indicating an increment in the modulus of the material, with the sample entering the plastic deformation zone. In addition to the movement of the chain segments, the deformation mechanism of the sample also includes the orientation change, slip, lamellae fracture, and recrystallization in the crystalline region [
31,
32].
In stage 3 (C–D section), the degree of bulging of the sample no longer conforms to the linear relationship with pressure, and Ꞁ is observed to increase with pressure. The sample exhibits an obvious crazing morphology, and the macromolecular chains are highly oriented with stress direction. Thus, a high degree of plastic and viscoelastic deformation appears inside the sample [
33]. As the craze modulus is lower than that of HDPE, the deformation is mainly concentrated in the craze area. As the bulging stress increases, the sample undergoes a crazing–crack transition. It should be noted that the delamination debonding behavior of the crack position and non-obvious microfibers in
Figure 4 are related to the high constraint of the plane-stress state on the deformation of the sample.
Figure 9 shows the damage distribution nephogram of the bulging specimen prior to fracture. As can be observed, the position of the maximum damage is located at the top of the specimen and expands in an annular shape, which is consistent with the test results. As compared with other locations, the large deformation area observed during the experiment, especially the white area where the color changes in
Figure 9, has a significantly high damage value
D (between 0.7 and 1).
As the fixing and sealing of the sample and fixture mainly rely on friction, the sample will inevitably slide relative to the fixture during the bulging test, especially near the filet and cavity, thus leading to a deviation in the process analysis. In addition,
Figure 9 shows a certain degree of damage at the contact position of the blank holder. In order to prevent the non-top fracture during the experiment, particular attention must be paid to the blank holder of the fixture.
4.2. Analysis of Sample Thinning
In the experiment, an obvious local thinning was observed at the top of the hydraulically bulging sample, i.e., the top thickness
H1 was significantly smaller than the side thickness
H2, as shown in
Figure 10.
The thinning process forms the basis for the bulging and rupture of the samples. To effectively analyze the process,
H1 and
H2 can be defined as the thicknesses of the specimen at a distance of 0 mm and 2 mm from the center point, respectively, and the thinning thickness
DH can be defined as follows:
where
DH is the thinning thickness, mm;
H1 is the thickness at 0 mm from the center point, mm; and
H2 is the thickness at 2 mm from the center point, mm.
Figure 11 shows the variation in the thinning thickness as a function of load based on the finite element simulation results. The results exhibit a nonlinear relationship between
DH and applied pressure. At the early stage of loading, the specimen sheet is in the elastic deformation stage. At this stage, the degree of thinning is less, thus indicating that the specimen is bulging nearly uniformly. As the pressure reaches 10 MPa, the thinning thickness shows an upward trend, resulting from the large plastic deformation of the local material during the middle and late loading stages [
34].
Based on the finite element calculation results, the exponential equation (Equation (8)) can be used to fit the relationship between the pressure and thinning thickness, as follows:
where
DH is the thinning thickness, mm;
ρ is the loading pressure, bar.; and
A and
B are the correction coefficients for the curves in
Figure 11, where A is −7.64 and B is 0.29.
In our study on the mechanical properties of high-density polyethylene (HDPE) using a numerical model, the influence of mesh size on the model’s results is a crucial aspect. By varying the mesh size in our simulations, we were able to observe distinct trends. When a coarse mesh was employed, computational efficiency increased, yet the precision of the outcomes was compromised. For example, in the hydraulic bulging test simulation involving HDPE specimens, a coarse mesh inadequately resolved the stress and strain distributions in key regions, resulting in discrepancies in the anticipated deformation profiles. On the other hand, a fine mesh provided more accurate results, closely resembling the experimental observations, but it significantly increased computational time. Regarding the multi-level Monte Carlo simulation method, we explored its application in reliability analysis. This method is based on generating multiple levels of approximations of the numerical model, each with a different mesh size [
35]. By doing so, it can efficiently estimate the variance and uncertainty in the model’s output. In the context of our HDPE research, this method can be used to assess the reliability of our numerical model in predicting the mechanical properties of HDPE under different conditions. For example, it can help us determine the probability of the failure of HDPE in pressure-bearing applications by considering the uncertainties associated with the mesh size and other model parameters. This not only enhances the reliability evaluation of our numerical model but also broadens the scope of our research, allowing for a more comprehensive understanding of the behavior of HDPE in real-world scenarios. We have successfully tested and analyzed the mechanical properties of HDPE materials under symmetric constraints using the hydraulic bulging method. Next, we will optimize the fixture structure and specimen size and explore the effectiveness of this method in testing various flexible non-metallic materials.