Evaluating Effects of Wrinkle Defects on Impact Response and Residual Compressive Strength After Impact in CFRP
Abstract
1. Introduction
2. Materials and Methods
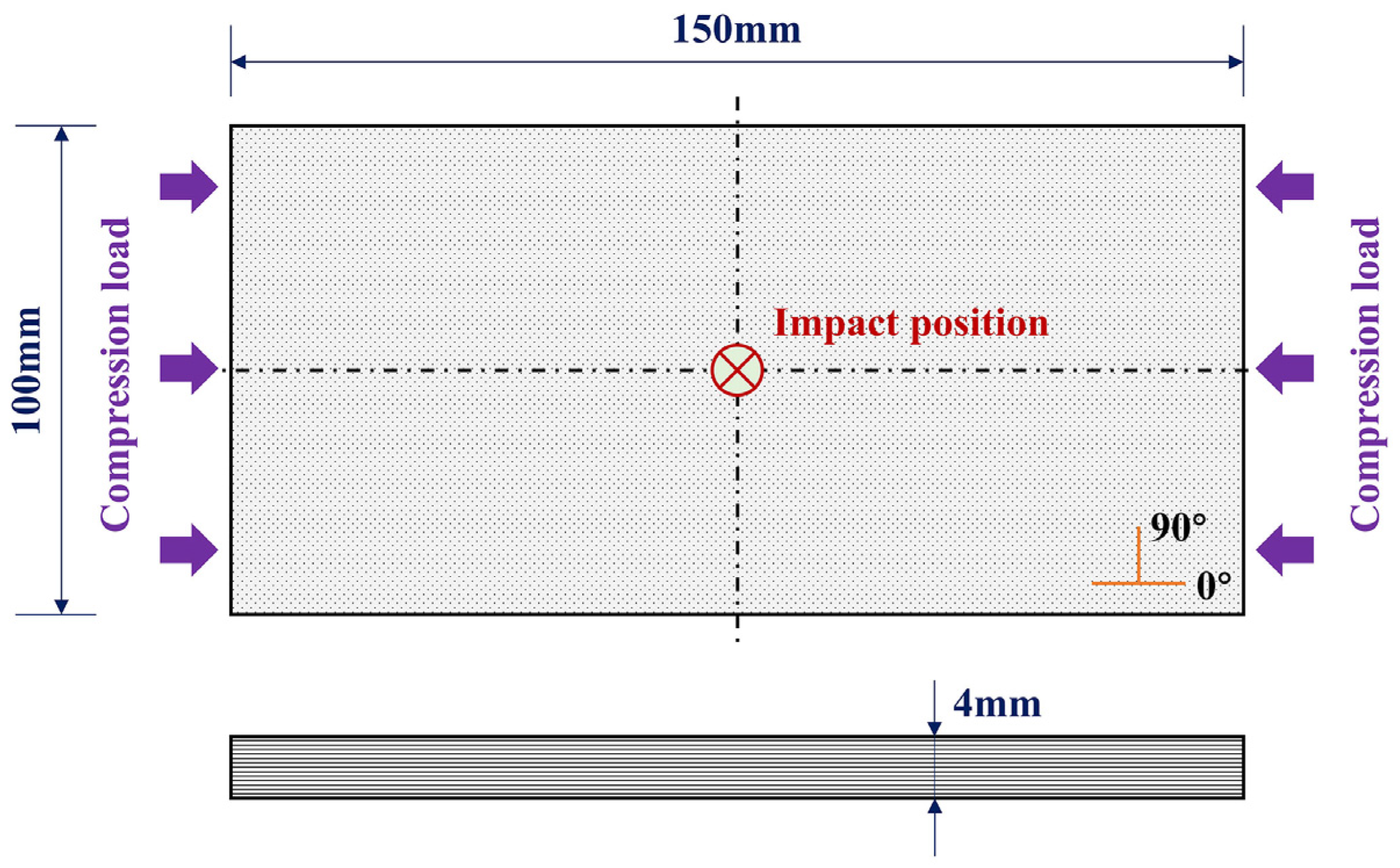
2.1. Test Matrix and Wrinkle Defect Characterization
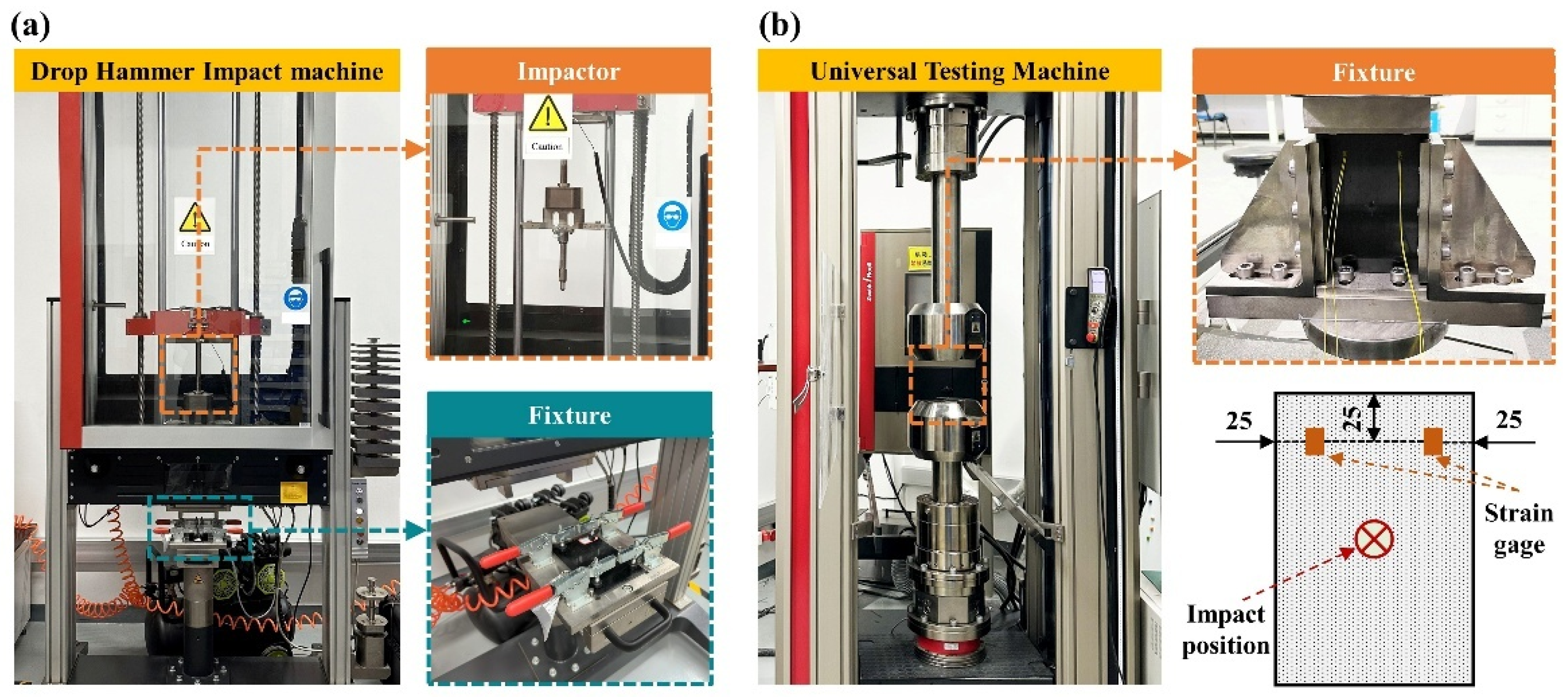
2.2. Experimental Setup
3. Effect of Wrinkle Defects on Impact Response
3.1. Numerical Model
3.2. Impact Response
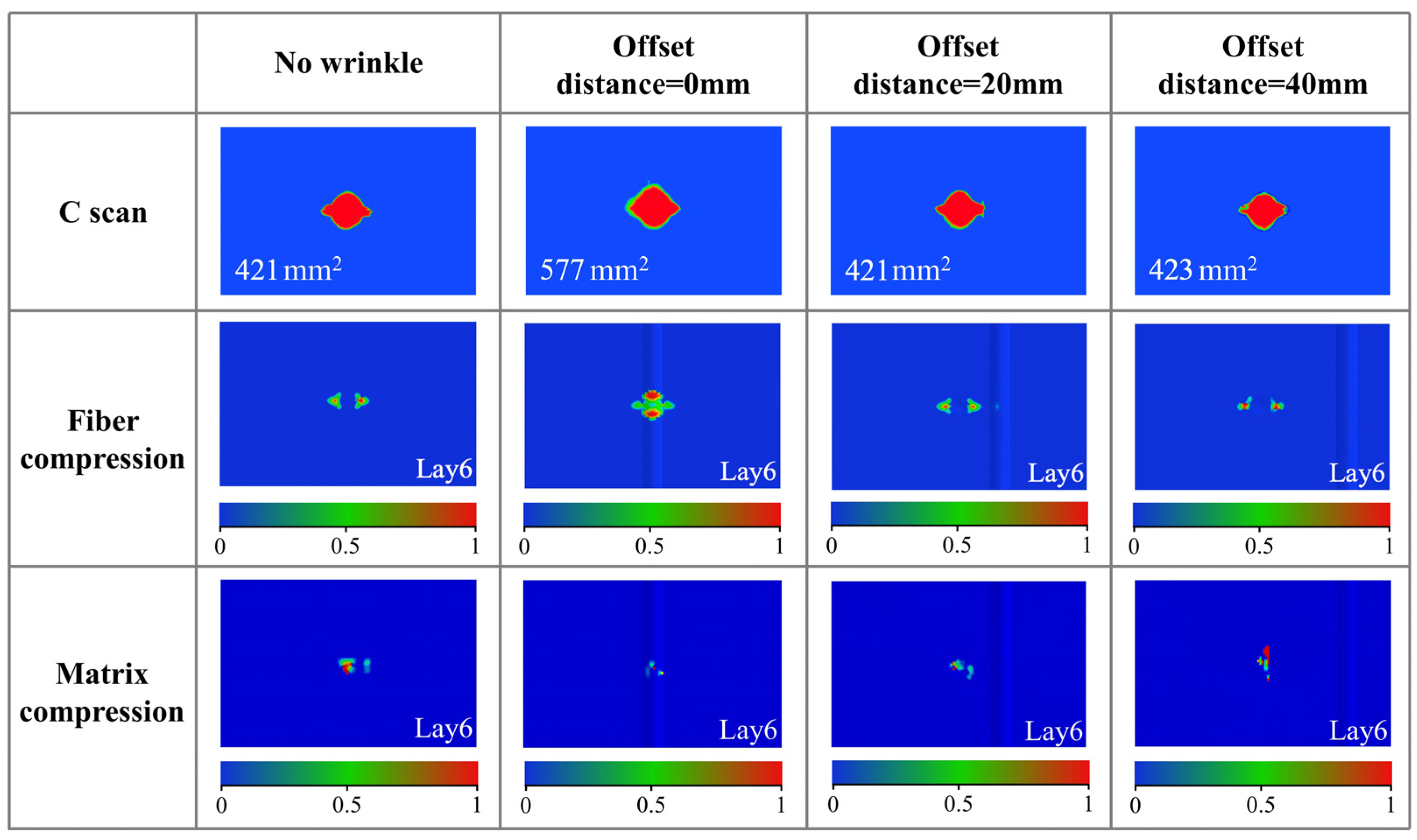
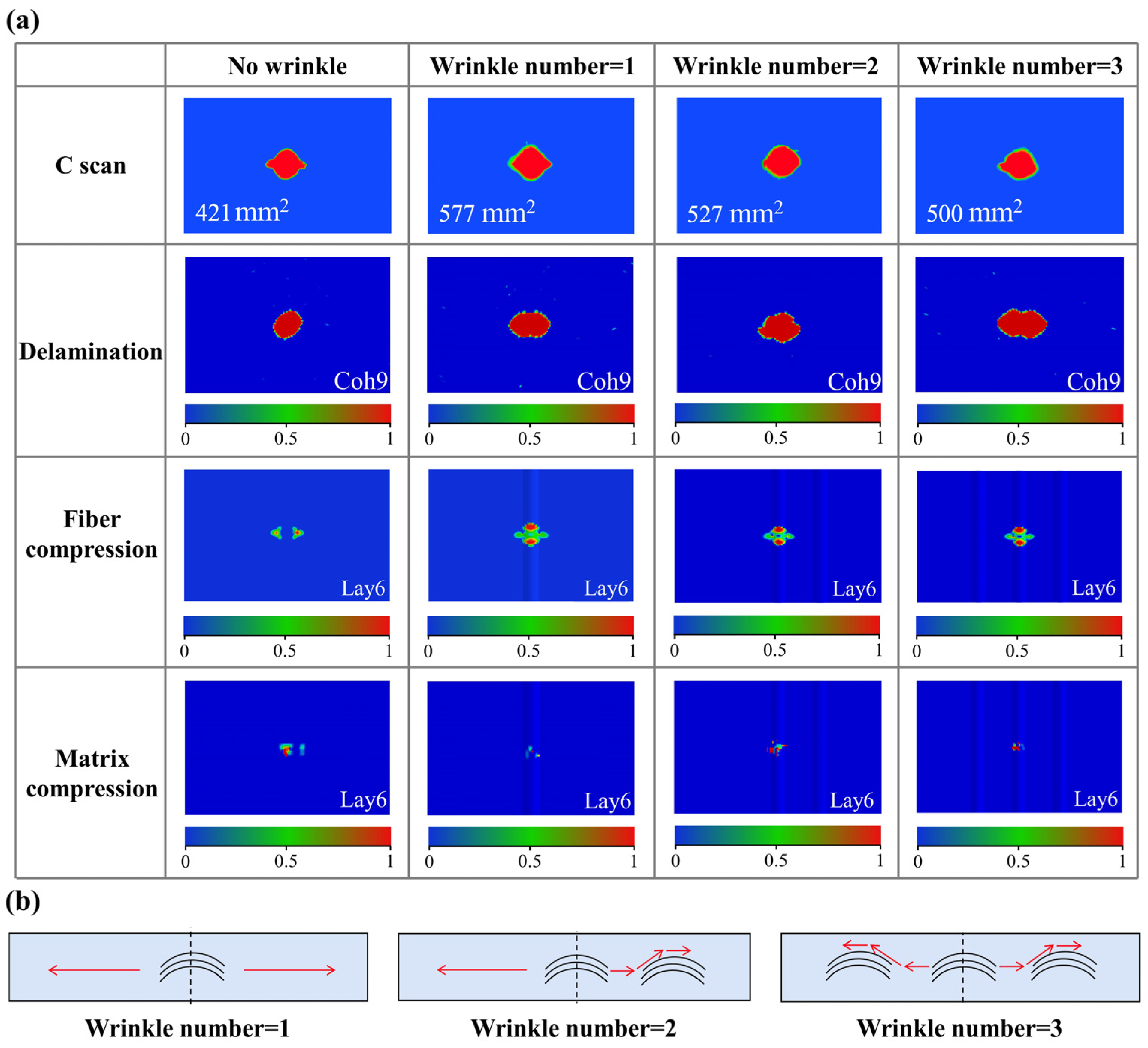
3.3. Damage Analysis
4. Effects of Wrinkle Defects on CAI
4.1. Compressive Response
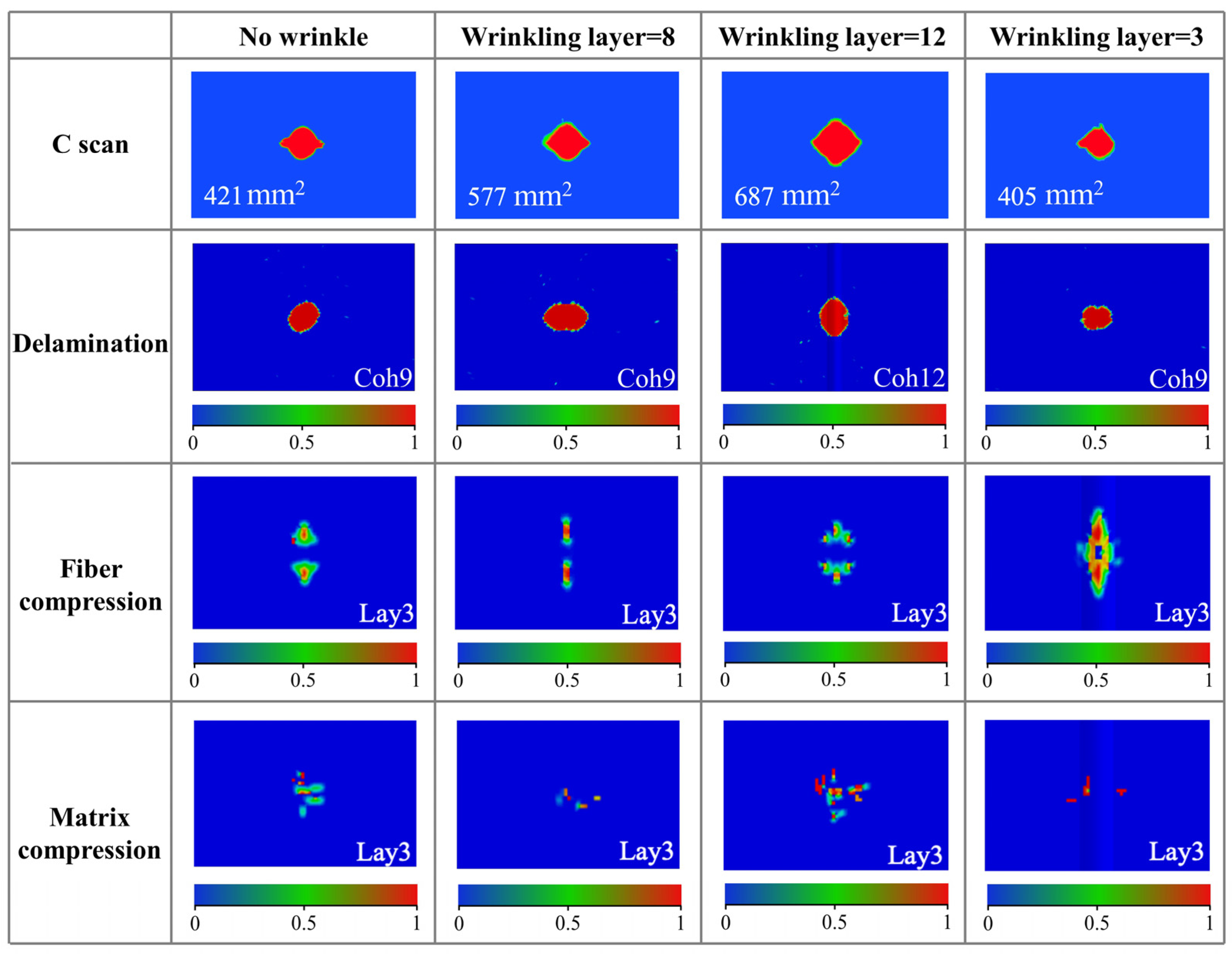
4.2. Damage Analysis
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Symbols and Abbreviations
| C | curvature percentage of specimen |
| CAI | compression after impact |
| CFRP | carbon fiber-reinforced polymer |
| E1, E2, E3 | elastic modulus of composite |
| F | compression load |
| G12, G13, G23 | shear modulus of composite |
| G1t, G1c | 0° fracture toughness of composite |
| G2t, G2c | 90° fracture toughness of composite |
| Gnn, Gss, Gtt | fracture toughness of cohesive model |
| h | wrinkle amplitude |
| hs | thickness of compressive specimen |
| k | wrinkle ratio |
| Knn, Kss, Ktt | stiffness of cohesive model |
| L | wrinkle width |
| S12, S13, S23 | shear strength of composite |
| w | width of compressive specimen |
| XT, XC | 0° strength of composite |
| YT, YC | 90° strength of composite |
| ZT, ZC | out-of-plane strength of composite |
| ε1, ε2 | surface strain of specimen |
| ν12, ν13, ν23 | Poisson’s ratio of composite |
| σCAI | residual compressive strength |
| σnn, σss, σtt | strength of cohesive model |
Appendix A
| Specimen ID | Maximum Contact Force (N) | ||
|---|---|---|---|
| Experiment | Simulation | Error | |
| L-NW | 5895.43 | 6334.56 | 7.45% |
| L-LW-O1 | 5923.10 | 6296.07 | 6.30% |
| L-LW-O2 | 5946.52 | 6468.11 | 8.77% |
| L-LW-L1 | 5679.55 | 6236.76 | 9.81% |
| L-LW-L2 | 6109.57 | 6421.03 | 5.10% |
| L-LW-N2 | 5879.21 | 6396.62 | 8.80% |
| L-LW-N3 | 5797.89 | 6442.37 | 11.12% |
| L-LW-F | 5934.73 | 6368.15 | 7.30% |
| L-LW-R | 5899.54 | 6272.70 | 6.33% |

References
- Zhang, J.; Lin, G.; Vaidya, U.; Wang, H. Past, present and future prospective of global carbon fibre composite developments and applications. Compos. Part B Eng. 2023, 250, 110463. [Google Scholar] [CrossRef]
- Ayyappan, V.; Tengsuthiwat, J.; Raghunathan, V.; Mavinkere Rangappa, S.; Siengchin, S. Quasi-static-cyclic and fatigue properties of carbon-innegra/pineapple multi-material laminates. Ind. Crops Prod. 2024, 222, 119894. [Google Scholar] [CrossRef]
- Mavinkere Rangappa, S.; Ayyappan, V.; Tengsuthiwat, J.; Siengchin, S. Innovations in Woven and Non-Woven Fabrics Based Laminated Composites; Springer: Berlin/Heidelberg, Germany, 2025. [Google Scholar]
- Yashas Gowda, T.G.; Ayyappan, V.; Madhu, P.; Mavinkere Rangappa, S.; Siengchin, S.; Jawaid, M. Mechanical and thermal properties of flax/carbon/kevlar based epoxy hybrid composites. Polym. Compos. 2022, 43, 5649. [Google Scholar]
- Qi, L.; Bai, J.; Wu, H.; Xu, G.; Xiong, H.; Yang, Y. The first engineering application of 10MN CFRP cables in cable-stayed bridge in China. Structures 2024, 68, 107199. [Google Scholar] [CrossRef]
- Kupski, J.; Teixeira de Freitas, S. Design of adhesively bonded lap joints with laminated CFRP adherends: Review, challenges and new opportunities for aerospace structures. Compos. Struct. 2021, 268, 113923. [Google Scholar] [CrossRef]
- Collinson, M.; Swait, T.; Bower, M.; Nuhiji, B.; Hayes, S. Development and implementation of direct electric cure of plain weave CFRP composites for aerospace. Compos. Part A Appl. Sci. Manuf. 2023, 172, 107615. [Google Scholar] [CrossRef]
- Fu, Y.; Yao, X. A review on manufacturing defects and their detection of fiber reinforced resin matrix composites. Compos. Part C Open Access 2022, 8, 100276. [Google Scholar] [CrossRef]
- Zarei, A.; Farahani, S.; Pilla, S. An experimental study on the manufacturing of engineered defects in composite plates. Compos. Part C Open Access 2022, 9, 100327. [Google Scholar] [CrossRef]
- Zhou, W.; Zhu, W.; Xu, Q.; Ke, Y. Out-of-plane tensile failure behavior of fiber reinforced composites due to lay-up temperature induced intra-ply and inter-ply voids. Compos. Struct. 2021, 271, 114150. [Google Scholar] [CrossRef]
- Mendoza, I.; Lamberson, L. Damage tolerancing in carbon fiber-reinforced polymer (CFRP) laminates under combined impact fatigue and environmental conditioning. Compos. Part A Appl. Sci. Manuf. 2024, 180, 108062. [Google Scholar] [CrossRef]
- Xu, Y.; Zhu, P.; Wang, W. Study of multiple impact behaviors of CFRP based on peridynamics. Compos. Struct. 2023, 322, 117380. [Google Scholar] [CrossRef]
- Shukla, B.; Naresh, L.; Raju, G. Experimental studies of CFRP laminates under repetitive low-velocity impact loading. Mater. Today Proc. 2024, 108, 123–129. [Google Scholar] [CrossRef]
- Arifin, H.; Noor, N.; Yahya, N.; Lim, K.; Ahmad Shah, M.; Umar, S.; Jay Kim, J. Influence of defect geometry on putty performance in pipeline composite repair assessments. Int. J. Press. Vessel. Pip. 2024, 209, 105190. [Google Scholar] [CrossRef]
- Du, J.; Wang, H.; Chen, C.; Ni, M.; Guo, C.; Zhang, S.; Ding, H.; Wang, H.; Bi, Y. Damage classification and evolution in composite under low-velocity impact using acoustic emission, machine learning and wavelet packet decomposition. Eng. Fract. Mech. 2024, 306, 110238. [Google Scholar] [CrossRef]
- Kulkarni, P.; Mali, K.; Singh, S. An overview of the formation of fibre waviness and its effect on the mechanical performance of fibre reinforced polymer composites. Compos. Part A Appl. Sci. Manuf. 2020, 137, 106013. [Google Scholar] [CrossRef]
- Codolini, A.; Chen, S.; Lawrence, G.; Harper, L.; Sutcliffe, M. Characterisation of process-induced variability in wrinkle defects during double diaphragm forming of non-crimp fabric. Compos. Part B Eng. 2024, 281, 111549. [Google Scholar] [CrossRef]
- Li, X.; Ge, J.; Chen, G.; Zhang, B.; Liang, J. The effects of wrinkle distributions on the mechanical characteristics of unidirectional glass fiber-reinforced composites. Compos. Sci. Technol. 2024, 256, 110762. [Google Scholar] [CrossRef]
- Bloom, L.; Wang, J.; Potter, K. Damage progression and defect sensitivity: An experimental study of representative wrinkles in tension. Compos. Part B Eng. 2013, 45, 449–458. [Google Scholar] [CrossRef]
- Mukhopadhyay, S.; Jones Mi Hallett, S. Tensile failure of laminates containing an embedded wrinkle; numerical and experimental study. Compos. Part A Appl. Sci. Manuf. 2015, 77, 219–228. [Google Scholar] [CrossRef]
- Liu, C.; Li, X.; Ge, J.; Liu, X.; Li, B.; Liu, Z.; Liang, J. A deep learning framework based on attention mechanism for predicting the mechanical properties and failure mode of embedded wrinkle fiber-reinforced composites. Compos. Part A Appl. Sci. Manuf. 2024, 186, 108401. [Google Scholar] [CrossRef]
- Krishnappa, S.; Gururaja, S. Compressive failure mechanisms in unidirectional fiber reinforced polymer composites with embedded wrinkles. Compos. Part B Eng. 2024, 284, 111688. [Google Scholar] [CrossRef]
- Davidson, P.; Waas, A. The effects of defects on the compressive response of thick carbon composites: An experimental and computational study. Compos. Struct. 2017, 176, 582–596. [Google Scholar] [CrossRef]
- Li, X.; Ge, J.; Zhang, B.; He, C.; Liu, S.; Li, Y.; Liang, J. An experimental and computational investigation of embedded wrinkle’s impacts on the compressive responses of thick unidirectional glass fiber-reinforced composites. Mater. Des. 2023, 231, 112079. [Google Scholar] [CrossRef]
- Chen, L.; Miao, L.; Xu, Q.; Yang, Q.; Zhu, W.; Ke, Y. Damage and failure mechanisms of CFRP due to manufacturing induced wrinkling defect. Compos. Struct. 2023, 326, 117624. [Google Scholar] [CrossRef]
- Mukhopadhyay, S.; Nixon-Pearson, O.; Hallett, S. An experimental and numerical study on fatigue damage development in laminates containing embedded wrinkle defects. Int. J. Fatigue 2018, 107, 1–12. [Google Scholar] [CrossRef]
- Miao, X.; Chen, X.; Rasmussen, S.; McGugan, M. Compression–compression fatigue damage of wrinkled carbon/glass hybrid composite laminates. Compos. Struct. 2024, 346, 118443. [Google Scholar] [CrossRef]
- Hörrmann, S.; Adumitroaie, A.; Viechtbauer, C.; Schagerl, M. The effect of fiber waviness on the fatigue life of CFRP materials. Int. J. Fatigue 2016, 90, 139–147. [Google Scholar] [CrossRef]
- Maragoni, L.; Modenato, G.; De Rossi, N.; Vescovi, L.; Quaresimin, M. Effect of fibre waviness on the compressive fatigue behavior of woven carbon/epoxy laminates. Compos. Part B Eng. 2020, 199, 108282. [Google Scholar] [CrossRef]
- D7136M-15 AD; Standard Test Method for Measuring the Damage Resistance of a Fiber-Reinforced Polymer Matrix Composite to a Drop-Weight Impact Event. ASTM International: West Conshohocken, PA, USA, 2015.
- Wang, J.; Zhu, C.; Yang, Y.; Zhang, Y.; Bi, Y. Effect of riveting displacement on the mechanical behavior of CFRP bolted joints with elliptical-head non-lug self-locking rivet nut. Int. J. Adv. Manuf. Technol. 2023, 125, 2161–2182. [Google Scholar] [CrossRef]
- D7137M-12 A; Standard Test Method for Compressive Residual Strength Properties of Damaged Polymer Matrix Composite Plates. ASTM International: West Conshohocken, PA, USA, 2012.
- Hsiao, H.; Daniel, I. Nonlinear elastic behavior of unidirectional composites with fiber waviness under compressive loading. J. Eng. Mater. Technol. 1996, 118, 561–570. [Google Scholar] [CrossRef]
- Zhang, H.; Li, M.; Zhou, J.; Yang, D.; Wang, H.; Song, X.; Ke, Y. Applicability evaluation of damage evolution models in simulating repeated low-velocity impact behavior of composite laminates. J. Reinf. Plast. Compos. 2024, 1–22. [Google Scholar] [CrossRef]
- Li, F.; Jin, S.; Li, W.; Luo, Z. Assessment of damage prediction models for composite laminates under single and repeated low-velocity impacts. Aerosp. Sci. Technol. 2024, 155, 109633. [Google Scholar] [CrossRef]
- Cai, L.; Qian, S.; Wang, H.; Ding, H.; Xu, Q. Effect of laying parameters on mechanical behavior of composite in thickness direction. Acta Aeronaut. Astronaut. Sin. 2021, 42, 423821. [Google Scholar]
- Ma, J.; Xu, Q.; Zhu, W.; Ke, Y. Translaminar enveloping ply for CFRP interlaminar toughening. Compos. Part B Eng. 2023, 250, 110464. [Google Scholar] [CrossRef]
- Zhang, F.; Liu, X.; Ye, Y.; Chen, Q.; Xu, Q.; Bi, Y. Effect of gap and overlap on mode II interlaminar fracture toughness of automated fiber placement prepreg laminates. Chin. J. Aeronaut. 2023, 36, 390–401. [Google Scholar] [CrossRef]
- Qian, S.; Liu, X.; Ye, Y.; Xu, Q.; Zhang, T.; Li, X. Effect of gap and overlap fiber placement defects on the delamination behavior of L-shaped composite laminates. Compos. Struct. 2021, 268, 113963. [Google Scholar] [CrossRef]
- Turon, A.; Camanho, P.; Costa, J.; Renart, J. Accurate simulation of delamination growth under mixed-mode loading using cohesive elements: Definition of interlaminar strengths and elastic stiffness. Compos. Struct. 2010, 92, 1857–1864. [Google Scholar] [CrossRef]







| ID | Wrinkle Offset Distance (mm) | Wrinkling Layer | Wrinkle Number | Impact Direction |
|---|---|---|---|---|
| L-NW | - | - | - | Forward |
| L-LW-O1 | 20 | 8 | 1 | Forward |
| L-LW-O2 | 40 | 8 | 1 | Forward |
| L-LW-L1 | 0 | 12 | 1 | Forward |
| L-LW-L2 | 0 | 3 | 1 | Forward |
| L-LW-N2 | 0 | 8 | 2 | Forward |
| L-LW-N3 | 0 | 8 | 3 | Forward |
| L-LW-F | 0 | 8 | 1 | Forward |
| L-LW-R | 0 | 8 | 1 | Reverse |
| E1 /GPa | E2 /GPa | E3 /GPa | ν12 | ν13 | ν23 | G12/GPa | G13 /GPa | G23 /GPa | XT /MPa | XC /MPa |
| 120 | 8 | 8 | 0.25 | 0.25 | 0.33 | 4.5 | 4.5 | 3 | 1600 | 1200 |
| YT /MPa | YC /MPa | ZT /MPa | ZC /MPa | S12 /MPa | S13 /MPa | S23 /MPa | G1t N/mm | G1c N/mm | G2t N/mm | G2c N/mm |
| 55 | 200 | 55 | 200 | 100 | 100 | 90 | 133 | 40 | 0.3 | 1 |
| Parameter | Value |
|---|---|
| Stiffness (MPa) | Knn = Kss = Ktt = 50,000 |
| Strength (MPa) | σnn = 35, σss = σtt = 60 |
| Fracture toughness (N/mm) | Gnn = 0.6, Gss = Gtt = 2.1 |
| ID | Offset Distance (mm) | Wrinkling Layer | Wrinkle Number | Impact Direction | Strength (MPa) | Variation |
|---|---|---|---|---|---|---|
| L-NW | - | - | - | Forward | 174.79 ± 8.15 | - |
| L-LW-O1 | 20 | 8 | 1 | Forward | 148.45 ± 15.23 | −15.10% |
| L-LW-O2 | 40 | 8 | 1 | Forward | 161.06 ± 13.62 | −7.90% |
| L-LW-L1 | 0 | 12 | 1 | Forward | 129.32 ± 10.08 | −26.00% |
| L-LW-L2 | 0 | 3 | 1 | Forward | 183.24 ± 12.36 | 4.80% |
| L-LW-N2 | 0 | 8 | 2 | Forward | 167.38 ± 9.87 | −4.20% |
| L-LW-N3 | 0 | 8 | 3 | Forward | 128.65 ± 12.81 | −26.40% |
| L-LW-F | 0 | 8 | 1 | Forward | 169.81 ± 19.14 | −2.90% |
| L-LW-R | 0 | 8 | 1 | Reverse | 126.65 ± 17.49 | −27.50% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, J.; Ding, H.; Zhang, S.; Wang, H.; Bi, Y.; Hua, Z. Evaluating Effects of Wrinkle Defects on Impact Response and Residual Compressive Strength After Impact in CFRP. Polymers 2025, 17, 1355. https://doi.org/10.3390/polym17101355
Wang J, Ding H, Zhang S, Wang H, Bi Y, Hua Z. Evaluating Effects of Wrinkle Defects on Impact Response and Residual Compressive Strength After Impact in CFRP. Polymers. 2025; 17(10):1355. https://doi.org/10.3390/polym17101355
Chicago/Turabian StyleWang, Jian, Huiming Ding, Shidi Zhang, Han Wang, Yunbo Bi, and Zhengli Hua. 2025. "Evaluating Effects of Wrinkle Defects on Impact Response and Residual Compressive Strength After Impact in CFRP" Polymers 17, no. 10: 1355. https://doi.org/10.3390/polym17101355
APA StyleWang, J., Ding, H., Zhang, S., Wang, H., Bi, Y., & Hua, Z. (2025). Evaluating Effects of Wrinkle Defects on Impact Response and Residual Compressive Strength After Impact in CFRP. Polymers, 17(10), 1355. https://doi.org/10.3390/polym17101355






