Modeling of Preforming Process for Unidirectional Prepreg Composites Using Simplified Linear Friction Model and Fiber-Tracking Method
Abstract
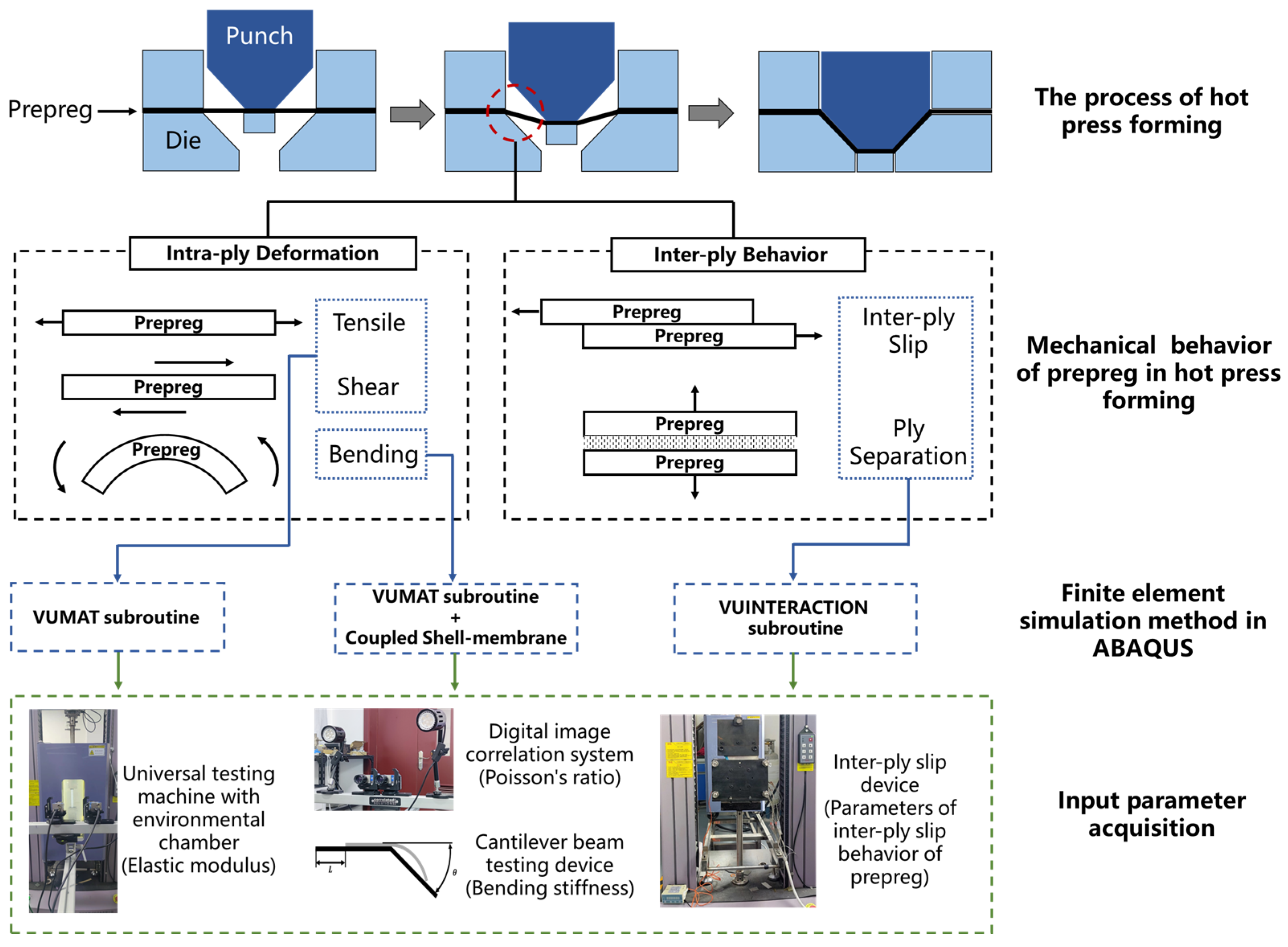
1. Introduction
2. Method
2.1. Intra-Ply Deformation
2.2. Inter-Ply Behavior
3. Experiments
3.1. Materials
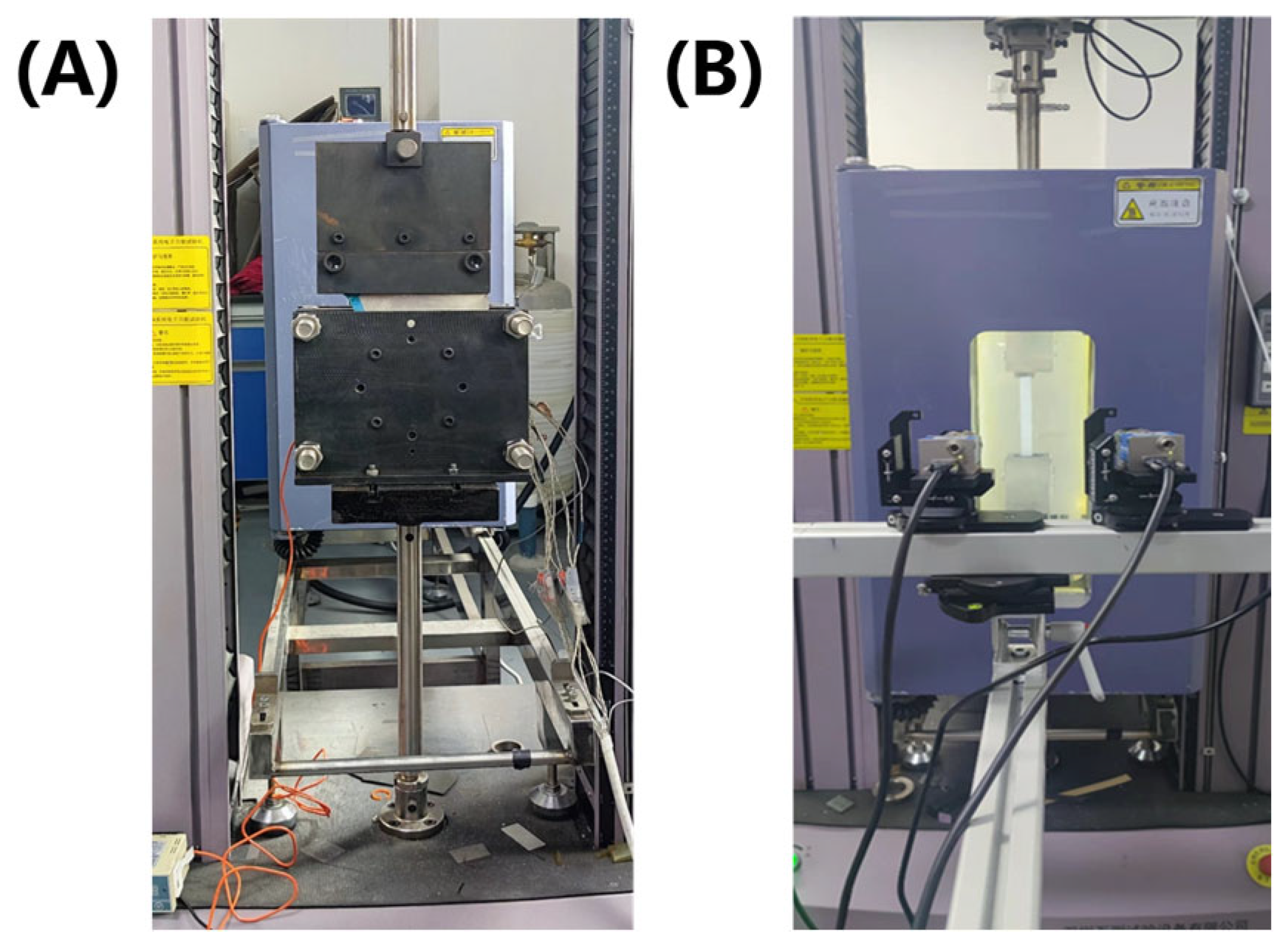
3.2. Inte-Ply Slip Testing
3.3. Mechanical Properties Testing
3.4. Flexural Stiffness Testing
3.5. Hot Press Preforming and Finite Element Analysis
4. Results and Discussion
5. Conclusions
- (1)
- This study elucidates the fundamental mechanisms governing the behavior of UD prepregs during hot press preforming by explicitly characterizing intra-ply deformations (in-plane tensile/shear and out-of-plane bending) and inter-ply interactions (slip and separation). It designs dedicated experimental methods to quantify these behaviors, including tensile testing, cantilever beam bending, and custom interfacial slip tests, and integrates the resulting insights into an integrated modeling approach that couples material anisotropy, friction dynamics, and deformation;
- (2)
- A simplified bilinear friction model was employed to capture inter-ply slip behavior under varying temperatures. Experimental validation confirmed that elevated temperatures (75 °C) reduce inter-ply friction and improve formability. The model showed good agreement with experimental data (RMSE < 9.5 × 10−7 MPa), demonstrating its capability in accurately simulating the frictional behavior of UD prepreg materials;
- (3)
- A fiber-tracking method was applied for UD prepreg in the process of hot press preform by updating material properties in real-time based on fiber reorientation. By decoupling in-plane (membrane elements) and out-of-plane (shell elements) behaviors, the model accurately predicts bending stiffness;
- (4)
- Experimental validation using a 2 m doubly curved Ω-stringer component confirmed the accuracy of the proposed integrated modeling approach. A comparison of the surface contour between simulation results and experimental measurements yielded a root mean square error (RMSE) of 1.27 mm, indicating good agreement. Elevated processing temperatures (75 °C) demonstrated significantly improved wrinkle suppression compared to conventional forming at 40 °C, consistent with simulation predictions.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Bakis, G.; Wendel, J.-F.; Zeiler, R.; Aksit, A.; Häublein, M.; Demleitner, M.; Benra, J.; Forero, S.; Schütz, W.; Altstädt, V. Mechanical properties of the carbon nanotube modified epoxy–carbon fiber unidirectional prepreg laminates. Polymers 2021, 13, 770. [Google Scholar] [CrossRef] [PubMed]
- Mukhopadhyay, S.; Jones, M.I.; Hallett, S.R. Compressive failure of laminates containing an embedded wrinkle; experimental and numerical study. Compos. Part A Appl. Sci. Manuf. 2015, 73, 132–142. [Google Scholar] [CrossRef]
- Baran, I.; Straumit, I.; Shishkina, O.; Lomov, S.V. X-ray computed tomography characterization of manufacturing induced defects in a glass/polyester pultruded profile. Compos. Struct. 2018, 195, 74–82. [Google Scholar] [CrossRef]
- Hörrmann, S.; Adumitroaie, A.; Viechtbauer, C.; Schagerl, M. The effect of fiber waviness on the fatigue life of CFRP materials. Int. J. Fatigue 2016, 90, 139–147. [Google Scholar] [CrossRef]
- Bae, D.; Kim, S.; Lee, W.; Yi, J.W.; Um, M.K.; Seong, D.G. Experimental and numerical studies on fiber deformation and formability in thermoforming process using a fast-cure carbon prepreg: Effect of stacking sequence and mold geometry. Materials 2018, 11, 857. [Google Scholar] [CrossRef]
- Wang, Z.; Luo, J.; Gong, Z.; Luo, Q.; Li, Q.; Sun, G. On correlation of stamping process with fiber angle variation and structural performance of thermoplastic composites. Compos. Part B Eng. 2022, 247, 110270. [Google Scholar] [CrossRef]
- Peng, X.; Cao, J. A continuum mechanics-based non-orthogonal constitutive model for woven composite fabrics. Compos. Part A Appl. Sci. Manuf. 2005, 36, 859–874. [Google Scholar] [CrossRef]
- Boisse, P.; Hamila, N.; Madeo, A. Modelling the development of defects during composite reinforcements and prepreg forming. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2016, 374, 20150269. [Google Scholar] [CrossRef]
- Thompson, A.J.; Belnoue, J.P.; Hallett, S.R. Modelling defect formation in textiles during the double diaphragm forming process. Compos. Part B Eng. 2020, 202, 108357. [Google Scholar] [CrossRef]
- Sun, J.; Li, M.; Gu, Y.; Zhang, D.; Li, Y.; Zhang, Z. Interply friction of carbon fiber/epoxy prepreg stacks under different processing conditions. J. Compos. Mater. 2014, 48, 515–526. [Google Scholar] [CrossRef]
- Dörr, D.; Faisst, M.; Joppich, T.; Poppe, C.; Henning, F.; Kärger, L. Modelling approach for anisotropic inter-ply slippage in finite element forming simulation of thermoplastic UD-tapes. In AIP Conference Proceedings; AIP Publishing LLC.: Melville, NY, USA, 2018; p. 020005. [Google Scholar]
- Wang, L.; Xu, P.; Peng, X.; Zhao, K.; Wei, R. Characterization of inter-ply slipping behaviors in hot diaphragm preforming: Experiments and modelling. Compos. Part A Appl. Sci. Manuf. 2019, 121, 28–35. [Google Scholar] [CrossRef]
- Li, Z.; Zhao, D.; Sun, B.; Liu, W.; Yue, G. Experimental characterization and modeling of the inter-ply sliding behavior of unidirectional prepreg in the preforming process. Mater. Res. Express 2023, 10, 035602. [Google Scholar] [CrossRef]
- Cline, J.; Yeager, M.; Bogetti, T. Determination of In-Plane Shear Properties of Ultra-High-Molecular-Weight Polyethylene (UHMWPE) Composites for Input into a Thermoforming Model; Army Research Laboratory: Adelphi, MD, USA, 2019. [Google Scholar]
- Ahn, H.; Kuuttila, N.E.; Pourboghrat, F. Mechanical analysis of thermo-hydroforming of a fiber-reinforced thermoplastic composite helmet using preferred fiber orientation model. J. Compos. Mater. 2018, 52, 3183–3198. [Google Scholar] [CrossRef]
- Harrison, P.; Campbell, I.; Guliyev, E.; McLelland, B.; Gomes, R.; Curado-Correia, N.; McGookin, E.; Mulvihill, D. Induction melt thermoforming of advanced multi-axial thermoplastic composite laminates. J. Manuf. Process. 2020, 60, 673–683. [Google Scholar] [CrossRef]
- Ahn, H.; Park, T.; Li, Y.; Yeo, S.Y.; Pourboghrat, F. Mechanical analysis of a thermo-hydroforming fiber-reinforced composite using a preferred fiber orientation model and considering viscoelastic properties. J. Manuf. Sci. Eng. 2022, 144, 021008. [Google Scholar] [CrossRef]
- Chen, B.; Colmars, J.; Naouar, N.; Boisse, P. A hypoelastic stress resultant shell approach for simulations of textile composite reinforcement forming. Compos. Part A Appl. Sci. Manuf. 2021, 149, 106558. [Google Scholar] [CrossRef]
- Pierce, R.S.; Falzon, B.G.; Thompson, M.C. A multi-physics process model for simulating the manufacture of resin-infused composite aerostructures. Compos. Sci. Technol. 2017, 149, 269–279. [Google Scholar] [CrossRef]
- Khan, M.; Mabrouki, T.; Vidal-Sallé, E.; Boisse, P. Numerical and experimental analyses of woven composite reinforcement forming using a hypoelastic behaviour. Application to the double dome benchmark. J. Mater. Process. Technol. 2010, 210, 378–388. [Google Scholar] [CrossRef]
- Peng, X.; Guo, Z.; Du, T.; Yu, W.-R. A simple anisotropic hyperelastic constitutive model for textile fabrics with application to forming simulation. Compos. Part B Eng. 2013, 52, 275–281. [Google Scholar] [CrossRef]
- Broberg, P.H.; Lindgaard, E.; Thompson, A.J.; Belnoue, J.P.-H.; Hallett, S.R.; Bak, B.L. An accurate forming model for capturing the nonlinear material behaviour of multilayered binder-stabilised fabrics and predicting fibre wrinkling. Compos. Part B Eng. 2024, 274, 111268. [Google Scholar] [CrossRef]
- Mulye, P.; Hemmer, J.; Morançay, L.; Binetruy, C.; Leygue, A.; Comas-Cardona, S.; Pichon, P.; Guillon, D. Numerical modeling of interply adhesion in composite forming of viscous discontinuous thermoplastic prepregs. Compos. Part B Eng. 2020, 191, 107953. [Google Scholar] [CrossRef]
- Wang, Y.; Mahapatra, S.; Belnoue, J.P.-H.; Ivanov, D.S.; Hallett, S.R. Understanding tack behaviour during prepreg-based composites’ processing. Compos. Part A Appl. Sci. Manuf. 2023, 164, 107284. [Google Scholar] [CrossRef]
- Wang, L.; Wang, J.; Liu, M.; Peng, X. Development and verification of a finite element model for double diaphragm preforming of unidirectional carbon fiber prepreg. Compos. Part A Appl. Sci. Manuf. 2020, 135, 105924. [Google Scholar] [CrossRef]
- ASTM D3039-00; Standard Test Methods for Tensile Properties of Polymer Matrix Composites. ASTM: Philadelphia, PA, USA, 2000.
- Margossian, A.; Bel, S.; Hinterhoelzl, R. On the characterisation of transverse tensile properties of molten unidirectional thermoplastic composite tapes for thermoforming simulations. Compos. Part A Appl. Sci. Manuf. 2016, 88, 48–58. [Google Scholar] [CrossRef]
- ISO 9073-7; Textiles. Test Methods for Nonwovens. Part 7: Determination of Bending Length. ISO: Geneva, Switzerland, 1998.
- Pilato, N.R.; Butler, R.; Trevarthen, J.; Lunt, A.J. An experimental and numerical study of industrially representative wrinkles in carbon fibre composite laminates. Mater. Des. 2022, 222, 111055. [Google Scholar] [CrossRef]




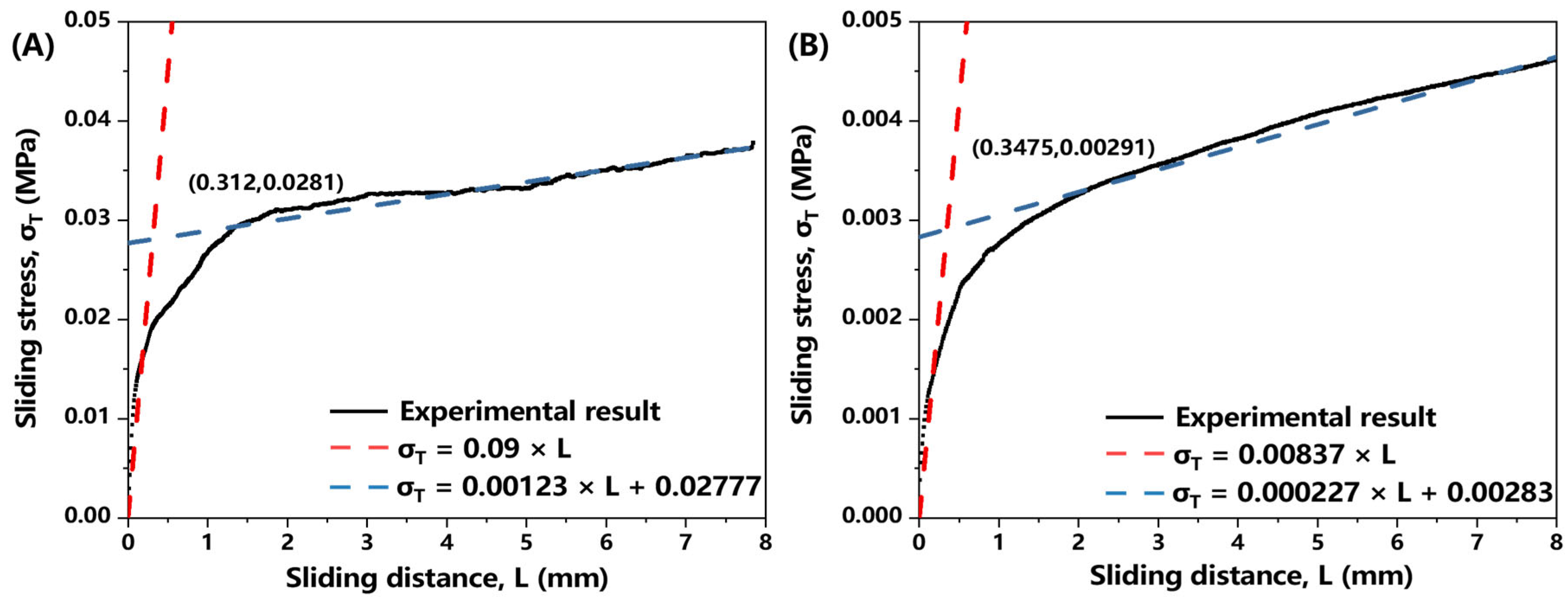




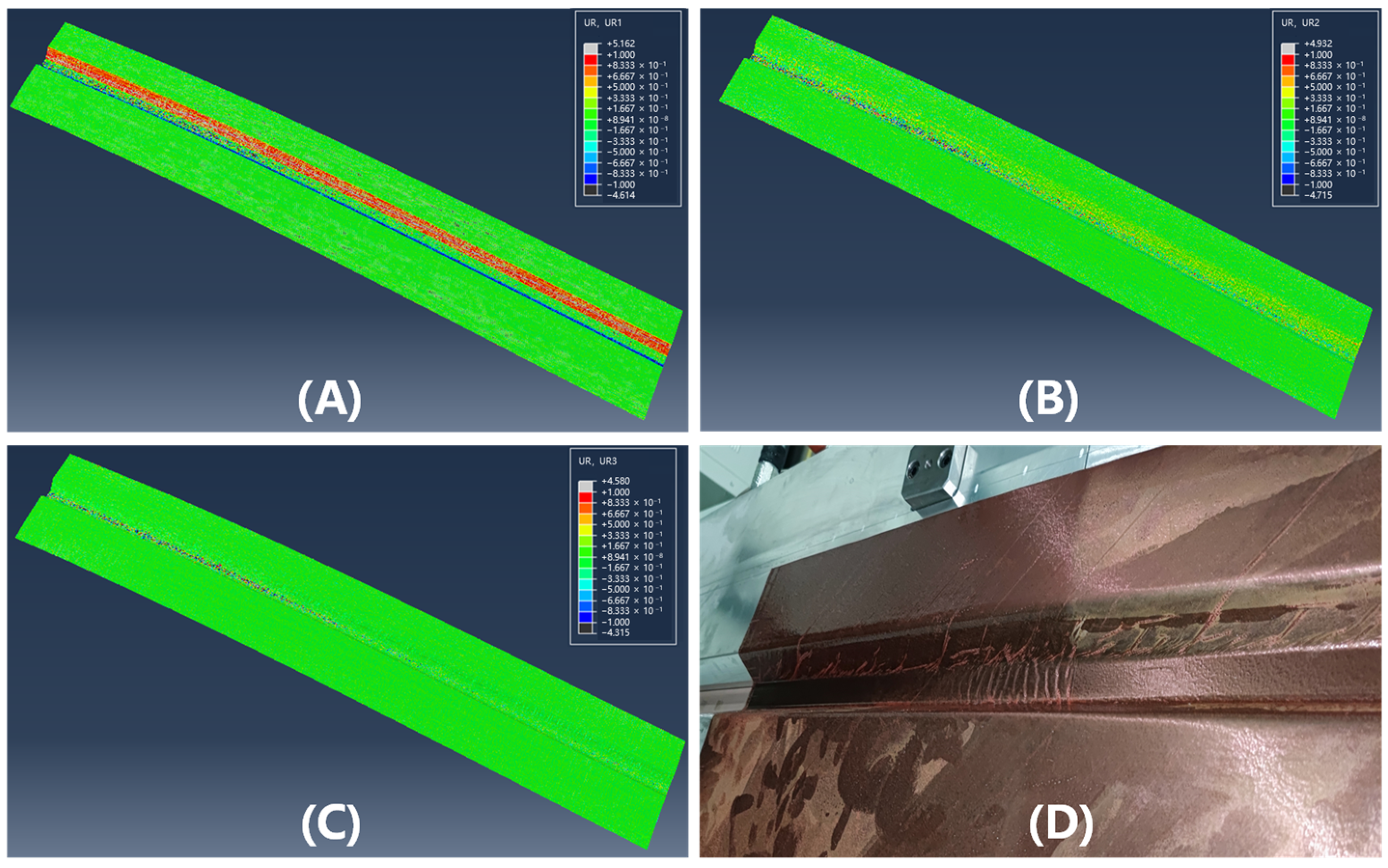
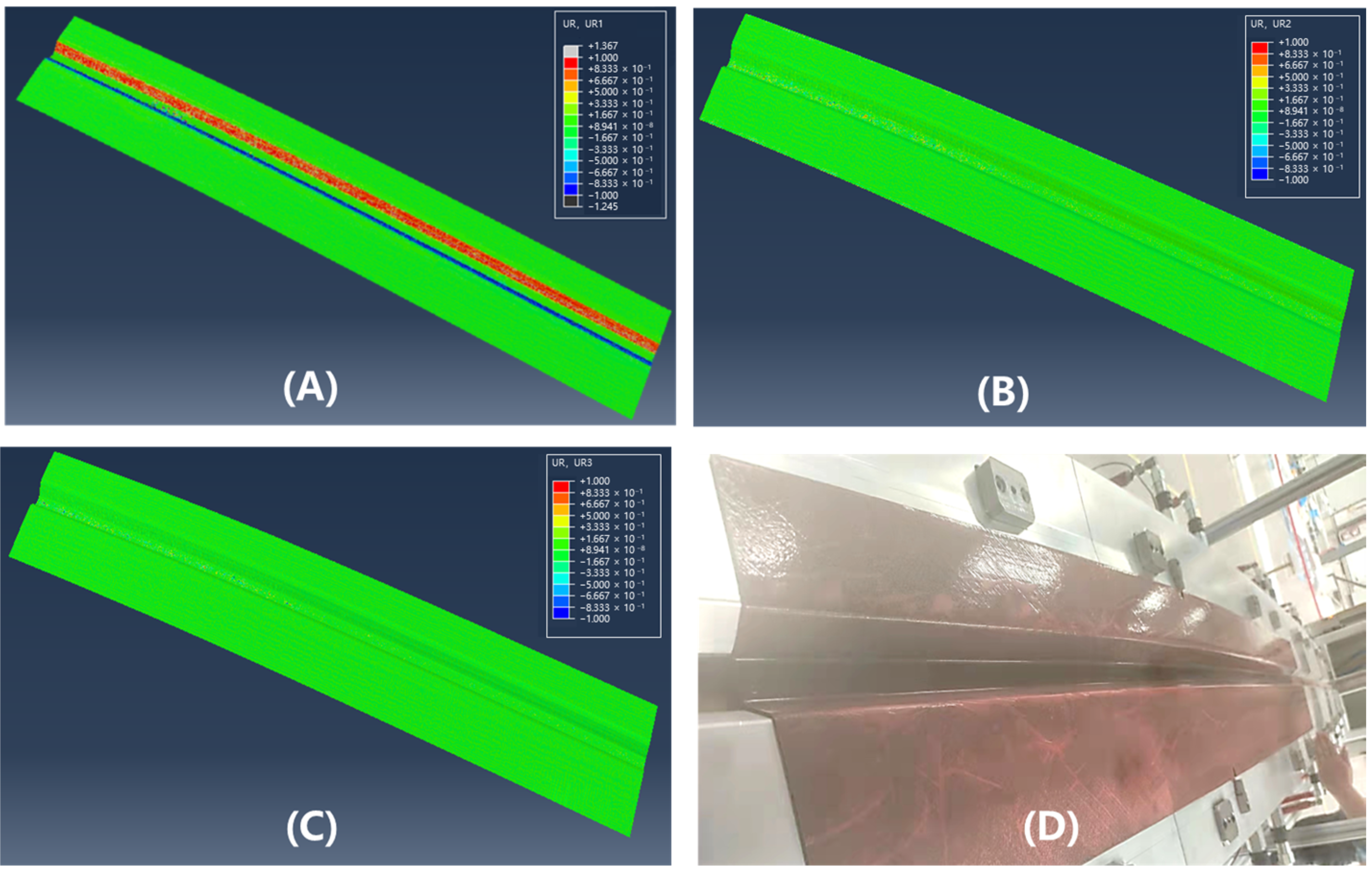

| Temperature (°C) | Initial Stiffness k1 (MPa/mm) | (MPa) | Hardening Stiffness k2 (MPa/mm) | RMSE |
|---|---|---|---|---|
| 40 | 0.09 | 0.0281 | 0.00123 | 9.45 × 10−7 |
| 75 | 0.00837 | 0.00291 | 0.000227 | 8.49 × 10−7 |
| Temperature (°C) | Sample Direction | Tensile Modulus (GPa) | Poisson’s Ratio |
|---|---|---|---|
| 40 | fiber direction | 141.98 | 0.3731 |
| 75 | fiber direction | 141.62 | 0.3775 |
| 40 | fiber’s normal direction | 0.815 | 0.0029 |
| 75 | fiber’s normal direction | 0.562 | 0.0035 |
| Temperature (°C) | Shear Modulus (MPa) |
| 40 | 0.2963 |
| 75 | 0.2046 |
| Temperature (°C) | 40 | 75 |
|---|---|---|
| Density (g/m2) | 294 | 294 |
| Displacement of fiber direction sample (cm) | 26.9 | 19.1 |
| Displacement of fiber’s normal direction sample (cm) | 2.0 | 1.6 |
| Bending stiffness in fiber direction (mN·cm) | 5722.7 | 2048.6 |
| Bending stiffness in fiber’s normal direction (mN·cm) | 2.4 | 1.2 |
| Equivalent tensile modulus in fiber direction (MPa) | 1177.5 | 421.5 |
| Equivalent tensile modulus in fiber’s normal direction (MPa) | 0.5 | 0.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Z.; Song, Q.; Liu, J.; Liu, W.; Chen, P.; Yue, G. Modeling of Preforming Process for Unidirectional Prepreg Composites Using Simplified Linear Friction Model and Fiber-Tracking Method. Polymers 2025, 17, 1321. https://doi.org/10.3390/polym17101321
Li Z, Song Q, Liu J, Liu W, Chen P, Yue G. Modeling of Preforming Process for Unidirectional Prepreg Composites Using Simplified Linear Friction Model and Fiber-Tracking Method. Polymers. 2025; 17(10):1321. https://doi.org/10.3390/polym17101321
Chicago/Turabian StyleLi, Zhefu, Qinghua Song, Jun Liu, Weiping Liu, Ping Chen, and Guangquan Yue. 2025. "Modeling of Preforming Process for Unidirectional Prepreg Composites Using Simplified Linear Friction Model and Fiber-Tracking Method" Polymers 17, no. 10: 1321. https://doi.org/10.3390/polym17101321
APA StyleLi, Z., Song, Q., Liu, J., Liu, W., Chen, P., & Yue, G. (2025). Modeling of Preforming Process for Unidirectional Prepreg Composites Using Simplified Linear Friction Model and Fiber-Tracking Method. Polymers, 17(10), 1321. https://doi.org/10.3390/polym17101321






