The Influence of Residual Stresses on the Curve Shape—Describing Interface Behavior in “Polymer–Fiber” Systems
Abstract
1. Introduction
- -
- Round fiber cross-section.
- -
- Constant diameter of the fiber immersed in resin.
- -
- Good wetting of the fiber by the binder, i.e., the absence of any discontinuities in the area of the fiber immersed in the binder, where the adhesive joint is formed. And, accordingly, the equality of the visible (measured when determining the adhesive strength) and the true area of contact between the fiber and the adhesive.
- -
- Uniform distribution of tangential stresses acting at the “adhesive–fiber” interface.
2. Materials
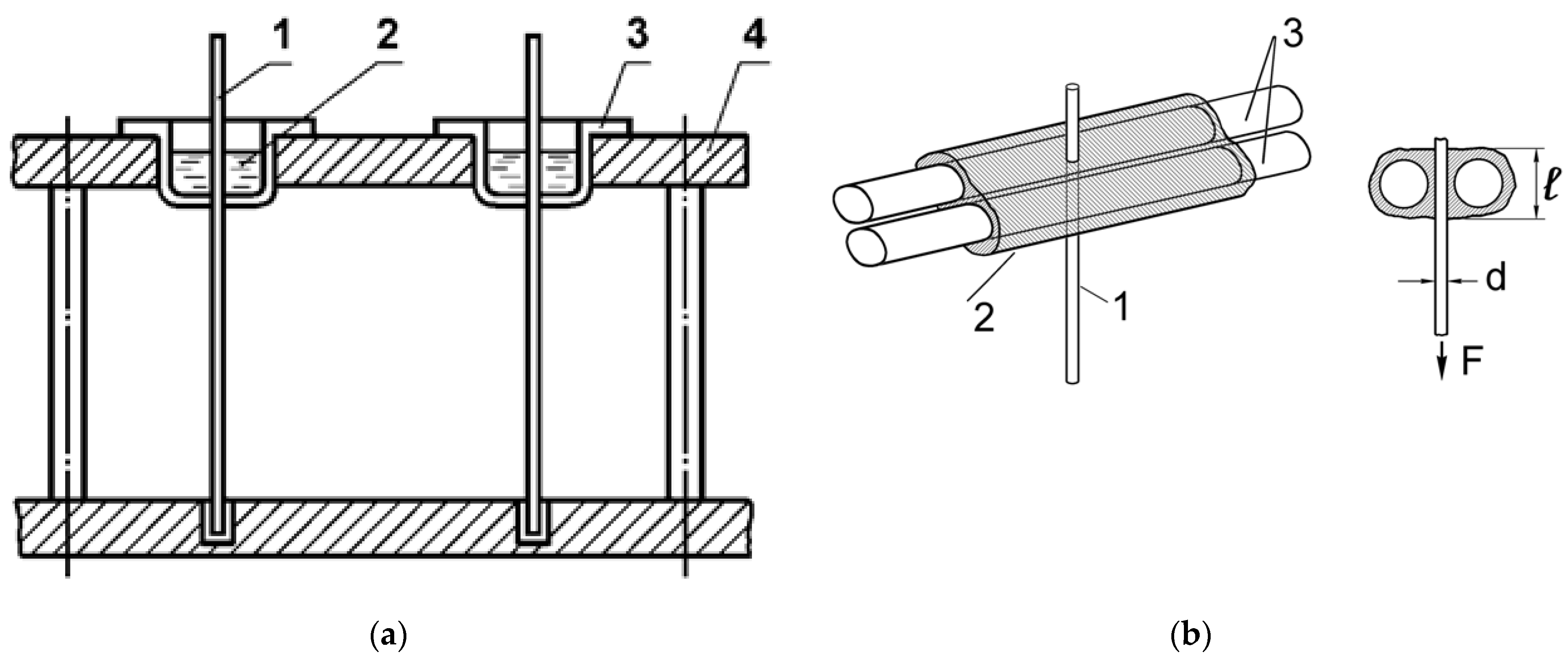
3. Methods
4. Results and Discussion
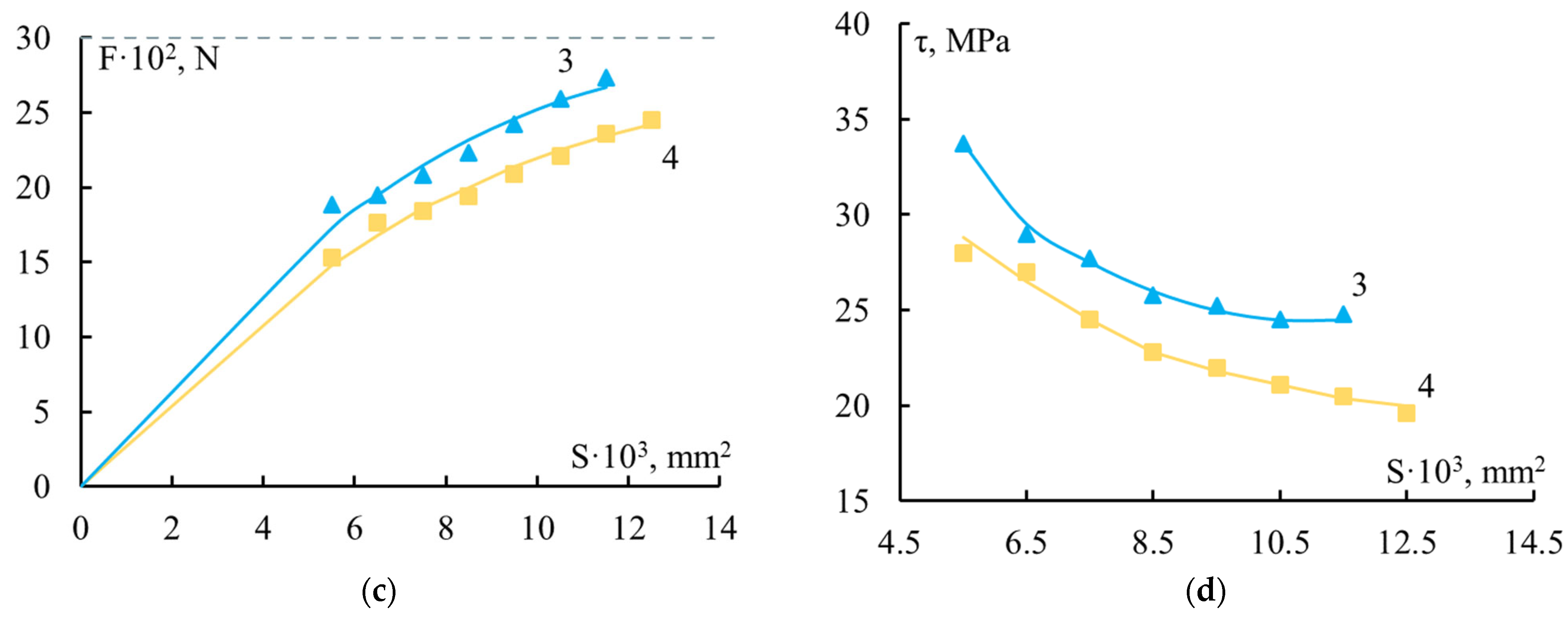
4.1. The Influence of Residual Stresses on the Dependences F–S and τ–S (Scale Dependence of Adhesive Strength)
- If measurements of τ are carried out in the glass transition region of the adhesive or at temperatures above it. This is observed, for example, when studying adhesion in polyolefin-fiber systems at room temperature. Residual stresses under these conditions are either zero or can relax.
- If measurements of τ are carried out in the glass transition region of the adhesive, but in a very narrow range of areas, especially if this interval corresponds to large values of S (l/d ≥ 7–10). Residual stresses in a narrow interval S change little and, accordingly, changes in the values of τ are difficult to notice since they lie within the limits of measurement errors.
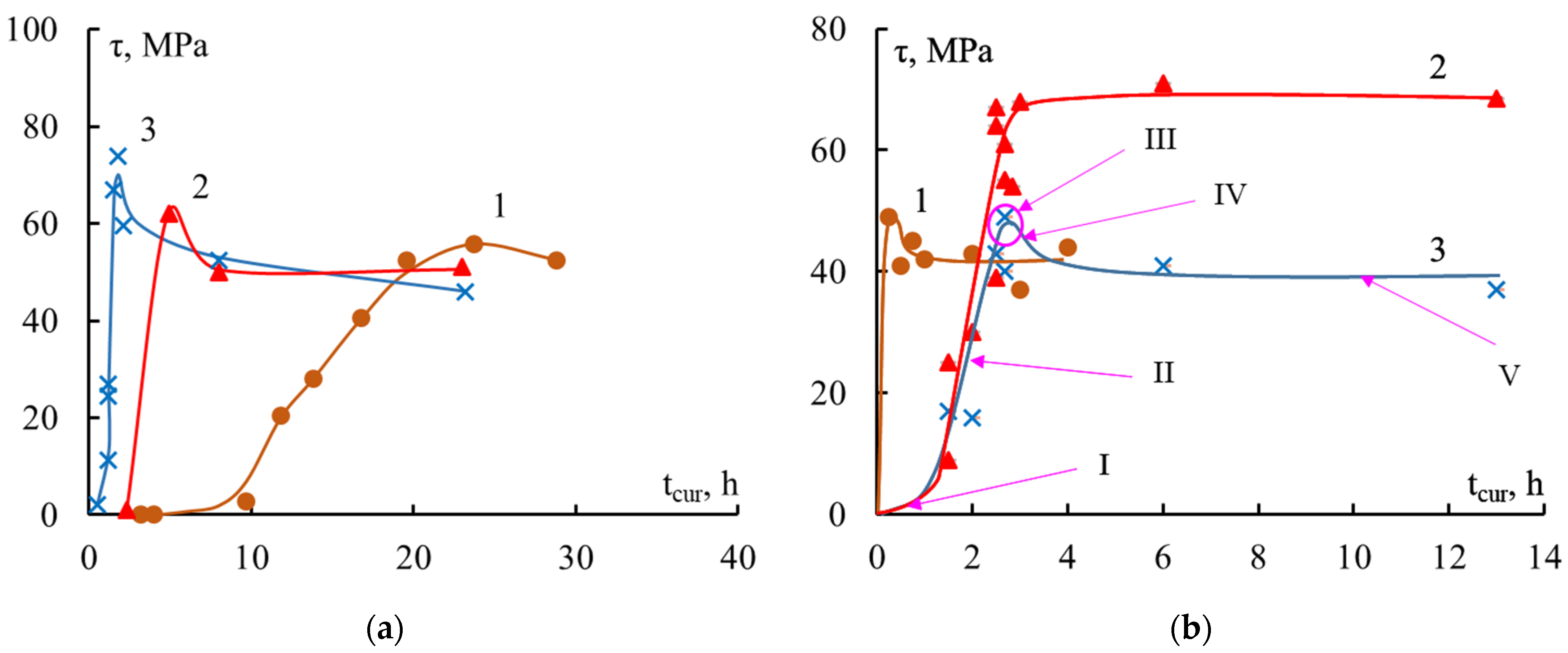
4.2. Influence of Residual Stresses on the Dependences τ–tcur (Adhesive Strength–Curing Time)
- The interface strength was formed.
- The adhesive structure was formed.
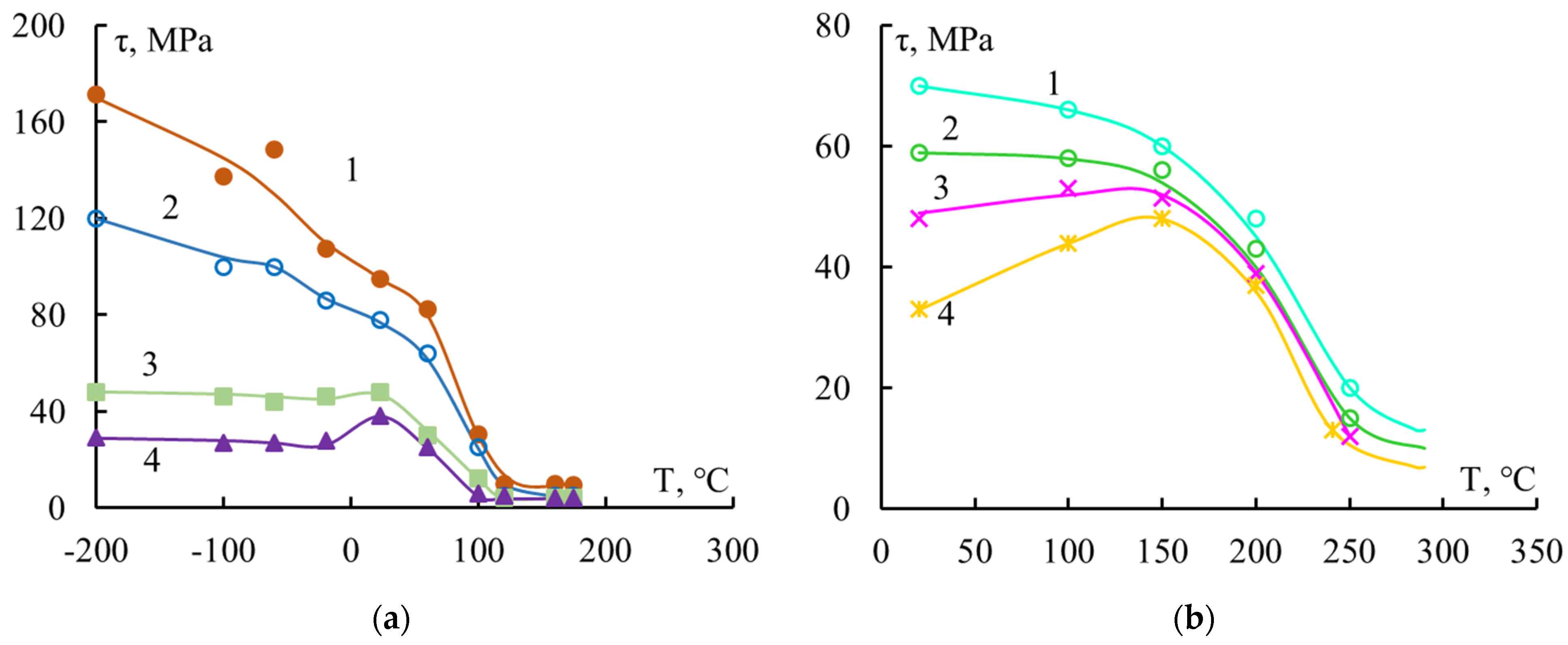
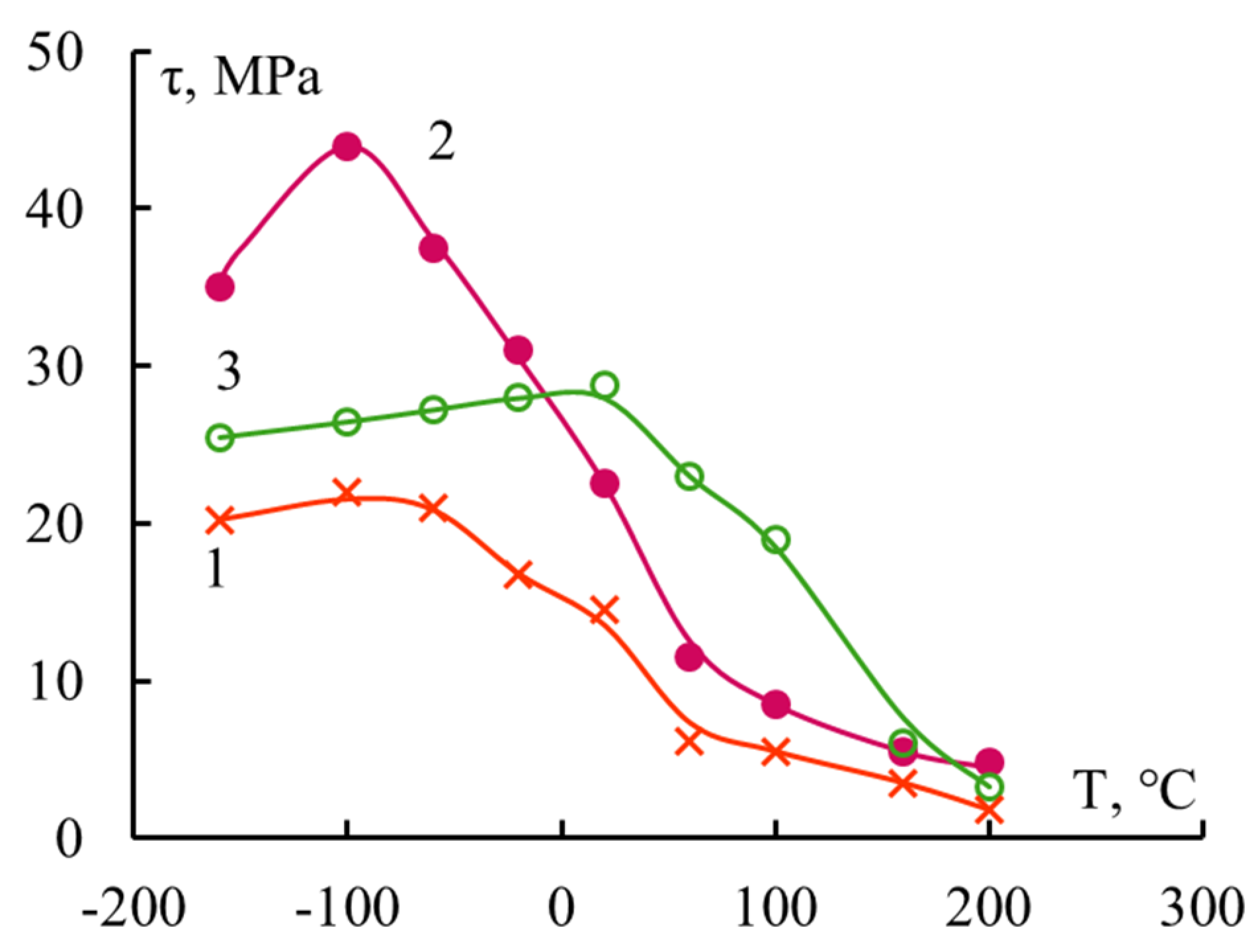
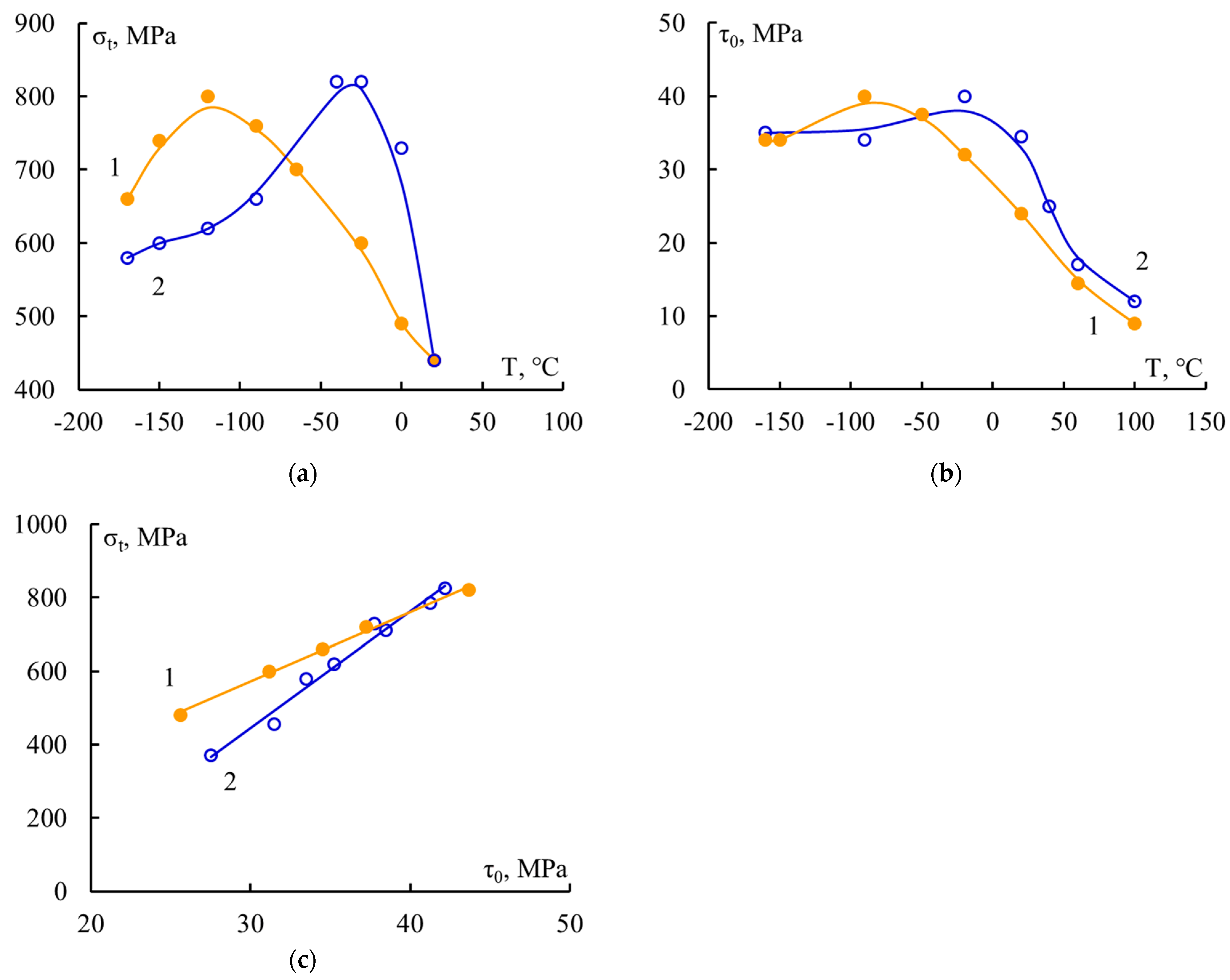
4.3. Influence of Residual Stresses on the Dependences τ–T (Adhesive Strength–Test Temperature)
- (1)
- Above the glass transition region. In this case, the binder was in a highly elastic state. The values of τ were very small and there was little dependence on the size of the junction.
- (2)
- The glass transition region is the region where the binder transitions from a highly elastic to a solid (glassy) state. The values of τ increased monotonically with decreasing temperature and changed slightly as S changed.
- (3)
- Below the glass transition region, the binder was in a solid state. In this temperature range, not only the values of τ, but also the appearance of the τ–T curves, depended on the area of contact between the resin and the fiber.
- Local adhesive strength τloc increases;
- Residual stresses τres increase.
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Bryce, D.; Thomason, J.; Yang, L. Micromechanical and spectroscopic characterisation of the curing perfomance of epoxy resins in the microbond test. In Proceedings of the IOP Confeence Series: Materials Science and Engineering, Roskilde, Denmark, 7–10 September 2020; p. 012019. [Google Scholar]
- Minty, R.F.; Yang, L.; Thomason, J.L. The dependence of interfacial shear strength on temperature and matrix chemistry in glass fibre epoxy composites. Compos. Part A Appl. Sci. Manuf. 2023, 164, 107303. [Google Scholar] [CrossRef]
- Kerber, M.L.; Vinogradov, V.M.; Golovkin, G.S.; Gorbatkina, Y.A.; Krizhanovskiy, V.K.; Kuperman, A.M.; Simonov-Emelyanov, I.D.; Haliulin, V.I.; Bunakov, V.A. Polymer Composite Materials: Structure, Properties, Technology; Profession, 2018. [Google Scholar]
- Mihailin, Y.A. Special Polymer Composite Materials; Science bases and technologies: Saint-Petersburg, Russia, 2009. (In Russian) [Google Scholar]
- Laurikainen, P.; Bhusare, S.; Mohanty, G.; Sarlin, E. Length-scale discrepancy in the properties of epoxy resin specimens. Polymer 2023, 283, 126148. [Google Scholar] [CrossRef]
- Gorbatkina, J.Y.A. Adhesive Strength of Fibre-Polymer Systems; Ellis Horwood: New York, NY, USA; London, UK, 1992. [Google Scholar]
- Liu, S.; Chevali, V.S.; Xu, Z.; Hui, D.; Wang, H. A review of extending perfomance of epoxy resins using carbon nanomaterials. Comp. Part B 2018, 136, 197–214. [Google Scholar] [CrossRef]
- Shukla, M.K.; Sharma, K. Effect of carbon nonefillers on the mechanical and interfacial properties of epoxy based nanocomposites. A review. Polym. Sci. Ser. A 2019, 61, 439–460. [Google Scholar] [CrossRef]
- Matykiewicz, D. Hybrid Epoxy Composites with Both Powder and Fiber Filler: A Review of Mechanical and Thermomechanical Properties. Materials 2020, 13, 1802. [Google Scholar] [CrossRef] [PubMed]
- Mavinkere, S.; Jyotishkumar, R.; Siengchin, P.S.; Tomas, S. Handbook of Epoxy/Fiber Composites; Springer Nature: Berlin/Heidelberg, Germany, 2022. [Google Scholar]
- Ha, Y.; Kim, T.-C.; Baeg, J.-H.; Kim, J.-S.; Shon, M.; Cho, Y.-R. Effect of cooling rate on surface properties of ZnMgAl coating and adhesion to epoxy adhesive. Int. J. Adhes. Adhes. 2022, 117, 103182. [Google Scholar] [CrossRef]
- Bedi, H.S.; Agnihotri, P.K. Interface and Interphase in Carbon Nanotube-Based Polymer Copmosites. A review. In Handbook of Epoxy/Fiber Composites; Mavinkere, S., Jyotishkumar, R., Siengchin, P.S., Tomas, S., Eds.; Springer Nature: Berlin/Heidelberg, Germany, 2022; pp. 147–168. [Google Scholar]
- Chandran, S.; Krishman, P. Microbond fibre bundle pullout technique to evaluate the interfacial adhesion of polyethylene and polypropylene self reinforced composites. Appl. Adhes. Sci. 2019, 7, 5. [Google Scholar] [CrossRef]
- Shahabaz, S.M.; Sharma, S.; Shetty, N.; Shetty, S.D.; Gowrishankar, M.C. Influence of temperature on mechanical properties and machining of fibre reinforced polymer composites: A review. Eng. Sci. 2021, 16, 26–46. [Google Scholar] [CrossRef]
- Zernadji, S.A.; Rokbi, M.; Benhamida, M.; Hammiche, D. Estimation of fiber/polymer bond strength from maximum load values recorded in the micro-bond tests. Mater. Today Proc. 2022, 53, 247–252. [Google Scholar] [CrossRef]
- Qu, C.-B.; Xiao, H.-M.; Huang, G.-W.; Li, N.; Li, M.; Li, F.; Li, Y.-Q.; Liu, Y.; Fu, S.-Y. Effects of cryo-thermal cycling on interlaminar shear strength and thermal expansion coefficient of carbon fiber/graphene oxide-modified epoxy composites. Compos. Commun. 2022, 32, 101180. [Google Scholar] [CrossRef]
- Feng, Y.; Nie, Z.; Deng, P.; Luo, L.; Hu, X.; Su, J.; Li, H.; Fan, X.; Qi, S. An effective approach to improve the thermal conductivity, strength and stress relaxation of carbon nanotubes/epoxy composites based on Vitrimer chemistry. Int. J. Mol. Sci. 2022, 23, 8833. [Google Scholar] [CrossRef]
- Luo, X.; Li, Y.; Li, S.; Liu, X. Enhancement of mechanical properties and bonding properties of flake-zinc-powder-modified epoxy resin composites. Polymers 2022, 14, 5323. [Google Scholar] [CrossRef]
- Sigrüner, M.; Hüsken, G.; Pirskawetz, S.; Herz, J.; Muscat, D.; Strübbe, N. Pull-out behavior of polymer fibers in concrete. J. Polym. Sci. 2023, 61, 2708–2720. [Google Scholar] [CrossRef]
- Babaevsky, P.G.; Salienko, N.V.; Shatalin, A.A. Evaluating the local strength and crack resistance of an glass fiber epoxy composite in the interlayer tension and shear using a finite-element model and experimentally determined parameters of the cohesive zone. Mech. Compos. Mater. 2023, 59, 713–724. [Google Scholar] [CrossRef]
- Lan, Z.; Deng, J.; Xu, Z.; Ye, Z.; Nie, Y. Study of heat treatment effect on mechanical properties of epoxy resin reinforced with fiber glass. Polymers 2023, 15, 2734. [Google Scholar] [CrossRef] [PubMed]
- Yang, G.; Cheng, F.; Zuo, S.; Zhang, J.; Xu, Y.; Hu, X. Crowing carbon nanotubes in situ surrounding carbon fiber surface via chemical vapor deposition to reinfoce flexural strength of carbon fiber composites. Polymers 2023, 15, 2309. [Google Scholar] [CrossRef] [PubMed]
- Yurkov, G.Y.; Pankratov, D.; Koksharov, Y.; Ovtchenkov, Y.; Semenov, A.; Korokhin, R.; Shcherbakova, G.; Gorobinskiy, L.; Burakova, E.; Korolkov, A.; et al. Composite materials based on a ceramic matrix of polycarbosilane and iron-containing nanoparticles. Ceram. Int. 2022, 48, 37410–37422. [Google Scholar] [CrossRef]
- Petrova, T.V.; Tretyakov, I.V.; Kireynov, A.V.; Shapagin, A.V.; Budylin, N.Y.; Alexeeva, O.V.; Beshtoev, B.Z.; Solodilov, V.I.; Yurkov, G.Y.; Berlin, A.A. Structure and properties of epoxy polysulfone systems modified with an active diluent. Polymers 2022, 14, 5320. [Google Scholar] [CrossRef] [PubMed]
- Tarasov, I.V.; Borisov, A.V.O.R.S.; Kireev, V.V.; Sirotin, I.S. Phozphazene-containg epoxy resins based on bisphenol F with enhanced heat resistance and mechanical properties: Sysnthesis anf properties. Polymers 2022, 14, 4547. [Google Scholar] [CrossRef] [PubMed]
- Solidlov, V.I.; Tretyakov, I.V.; Petrova, T.V.; Kireinov, A.V.; Korokhin, R.A.; Yurkov, G.Y. Influence of polyethersulfone on the fracture toughness of epoxy matrices and reinforced plastics on their basis. Mech. Compos. Mater. 2023, 59, 743–756. [Google Scholar] [CrossRef]
- Tretyakov, I.V.; Petrova, T.V.; Kireinov, A.V.; Korokhin, R.A.; Platonova, E.O.; Alexeeva, O.V.; Gorbatkina, Y.A.; Solodilov, V.I.; Yurkov, G.Y.; Berlin, A.A. Fracture of epoxy matrixes modified with thermo-plastic polymers and widning glass fibers reinforced plastics on their base under low-viscosity impact condition. Polymers 2023, 15, 2958. [Google Scholar] [CrossRef] [PubMed]
- McGarry, F.I. Resin-Glass Bond Characterisation. ASTM Bull. 1959, 235, 63–68. [Google Scholar]
- Shiryaeva, G.V.; Andreevskaya, G.D. Adhesion determination method of resins to glass fiber surface. Plast. Massy 1962, 4, 42–43. (In Russian) [Google Scholar]
- Piggott, M.R.; Chua, P.S.; Andison, D. The interface between glass and carbon fibers and thermosetting polymers. Polym. Compos. 1985, 6, 242–248. [Google Scholar] [CrossRef]
- Miller, B.; Muri, P.; Rebenfeld, L. A microbond method for detremination of the shear strength of a fiber/resin interface. Pomsopite Sci. Technol. 1989, 28, 17–32. [Google Scholar]
- Dovgyalo, V.A.; Zhandarov, S.F.; Pisanova, E.V. Determination of adhesive strength in a thermoplastic-resin-thin-fiber system. Mech. Compos. Mater. 1990, 26, 6–9. [Google Scholar] [CrossRef]
- Zhandarov, S.; Pisanova, E.; Mäder, E.; Nairn, J.A. Investigation of load transfer between the fiber and the matrix in pull-out tests with fibers having different diameters. J. Adhes. Sci. Technol. 2001, 15, 205–222. [Google Scholar] [CrossRef]
- Zhandarov, S.F.; Mader, E.; Yurkevich, O.R. Indirect estimation of fiber/polymer bond strength and interfacial friction form maximum load values recorded in microbond and pull-out tests. Part I: Local bond strength. J. Adhes. Sci. Technol. 2002, 16, 1171–1200. [Google Scholar] [CrossRef]
- Turusov, R.A.; Manevich, I. Contact-layer method in adhesion mechanics: Picking fiber from a matrix. Polym. Sci. Ser. D 2010, 3, 75–86. [Google Scholar] [CrossRef]
- Zhandarov, S.; Mader, E. An alternative method of determing the local interfacial shear strength from force—Displacement curves in the pull-out and microbond tests. Int. J. Adhes. Adhes. 2014, 55, 37–40. [Google Scholar] [CrossRef]
- Yamamoto, S.; Kuwahara, R.; Tanaka, K. Effects of Chemistry of Silicon Surfaces on the Curing Process and Adhesive Strength for Epoxy Resin. ACS Appl. Polym. Mater. 2022, 4, 6038–6046. [Google Scholar] [CrossRef]
- Thomason, J. An overview of some sclaing issues un the sample preparation and data interpretation of the microbond test for fibre—Matrix interface characterization. Polym. Test. 2022, 11, 107591. [Google Scholar] [CrossRef]
- Gorbatkina, Y.A.; Ivanova-Mumzhieva, V.G. The Adhesion of Modified Epoxides to Fibers; Cambridge Scholars Publishing: Cambridge, UK, 2022. [Google Scholar]
- Cox, H.L. The elasticity and strength of paper and other fibrous materials. Br. J. Appl. Phys. 1952, 3, 72–79. [Google Scholar] [CrossRef]
- Kelly, A.; Macmillan, N.H. Strong Solids, 3rd ed.; Charendon Press: Oxford, UK, 1986. [Google Scholar]
- Vyatkina, M.A.; Gorbatkina, Y.A.; Gorbunova, I.Y.; Korokhin, R.A.; Pavlukovich, N.G.; Petrova, T.V.; Solodilov, V.I.; Storozhuk, I.P. Effect of Oligomeric Copoluimides on the properties of Epoxy Binders. Mech. Compos. Mater. 2023, 58, 857–866. [Google Scholar] [CrossRef]



| Binder | Binder Type | Curing Conditions | Glass Temperature | Fibers |
|---|---|---|---|---|
| EDT-10 | Amine | 160 °C—8 h | 106 °C | Steel |
| EAnhB | Anhydride | 90 °C—3 h, 120 °C—12 h | 121 °C | Steel |
| MAB | Amine | 160 °C—8 h | 80 °C | Glass |
| PPRS | Polyphenilsiloxane | 200 °C—6 h | 130 °C | Glass |
| Binder | Fibers | Length l, mm | Area S, mm2 | l/d |
|---|---|---|---|---|
| EDT-10 | Steel | 0.3–3.1 | 0.158–1.45 | 2–20.5 |
| EAnhB | Steel | 0.6–2.1 | 0.27–1.01 | 4–14 |
| EDT-10 | Glass | 0.15–0.32 | (5.5–11.5)∙10−3 | 13–28 |
| PPRS | Glass | 0.15–0.35 | (5.5–12.5)∙10−3 | 13–30 |
| Binder | Tg, °C | Tmax, °C | Curing Conditions |
|---|---|---|---|
| BP-4 | 65 | ~30 | 70 °C—2 h, 90 °C—2 h, 110 °C—1.5 h, 130°C—1.5 h, 160 °C—2 h |
| PPSR | 130 | 30 | 200 °C—6 h |
| PN-1 | 30 | ~90 | 100 °C—6 h |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gorbatkina, Y.A.; Ivanova-Mumzhieva, V.G.; Alexeeva, O.V.; Vyatkina, M.A. The Influence of Residual Stresses on the Curve Shape—Describing Interface Behavior in “Polymer–Fiber” Systems. Polymers 2024, 16, 582. https://doi.org/10.3390/polym16050582
Gorbatkina YA, Ivanova-Mumzhieva VG, Alexeeva OV, Vyatkina MA. The Influence of Residual Stresses on the Curve Shape—Describing Interface Behavior in “Polymer–Fiber” Systems. Polymers. 2024; 16(5):582. https://doi.org/10.3390/polym16050582
Chicago/Turabian StyleGorbatkina, Yulia A., Viktoria G. Ivanova-Mumzhieva, Olga V. Alexeeva, and Mariya A. Vyatkina. 2024. "The Influence of Residual Stresses on the Curve Shape—Describing Interface Behavior in “Polymer–Fiber” Systems" Polymers 16, no. 5: 582. https://doi.org/10.3390/polym16050582
APA StyleGorbatkina, Y. A., Ivanova-Mumzhieva, V. G., Alexeeva, O. V., & Vyatkina, M. A. (2024). The Influence of Residual Stresses on the Curve Shape—Describing Interface Behavior in “Polymer–Fiber” Systems. Polymers, 16(5), 582. https://doi.org/10.3390/polym16050582







