Design and Computational Validation of γ-Ray Shielding Effectiveness in Heavy Metal/Rare Earth Oxide–Natural Rubber Composites
Abstract
1. Introduction
2. Design Theory of γ-Ray Shielding Materials
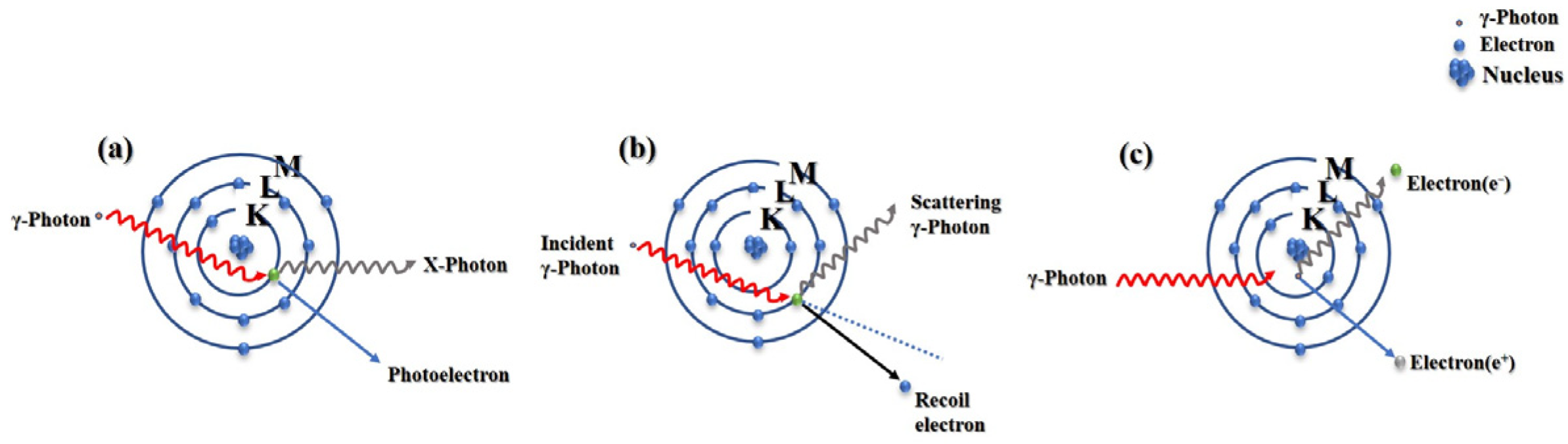
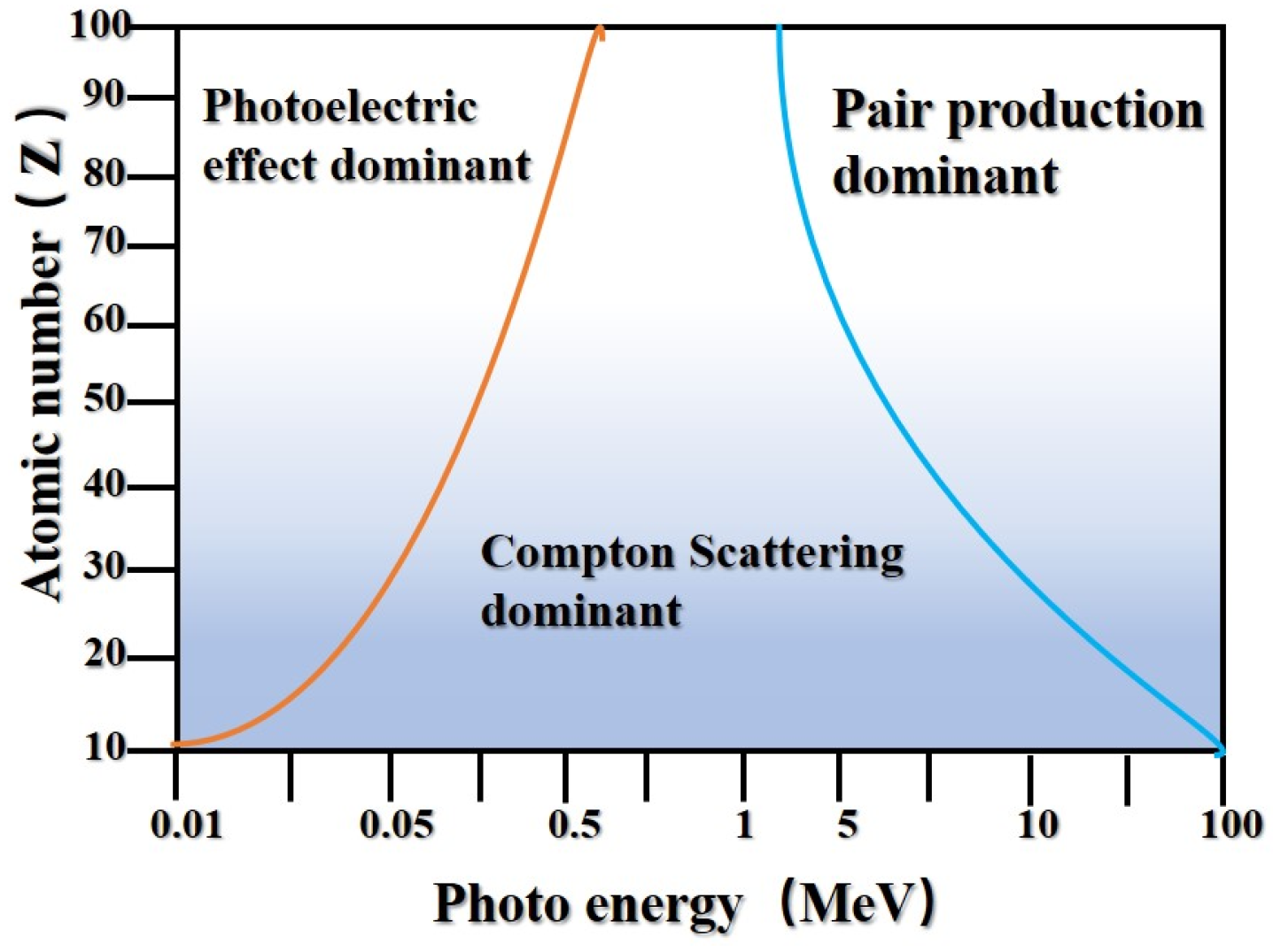
2.1. Principle of γ-Ray Shielding
2.2. Theoretical Calculation of Radiation Shielding
2.3. Analysis of Radiation Simulation Software
3. Preparation of Materials and Methodologies for Simulating and Testing Shielding Performance
3.1. Preparation of Materials
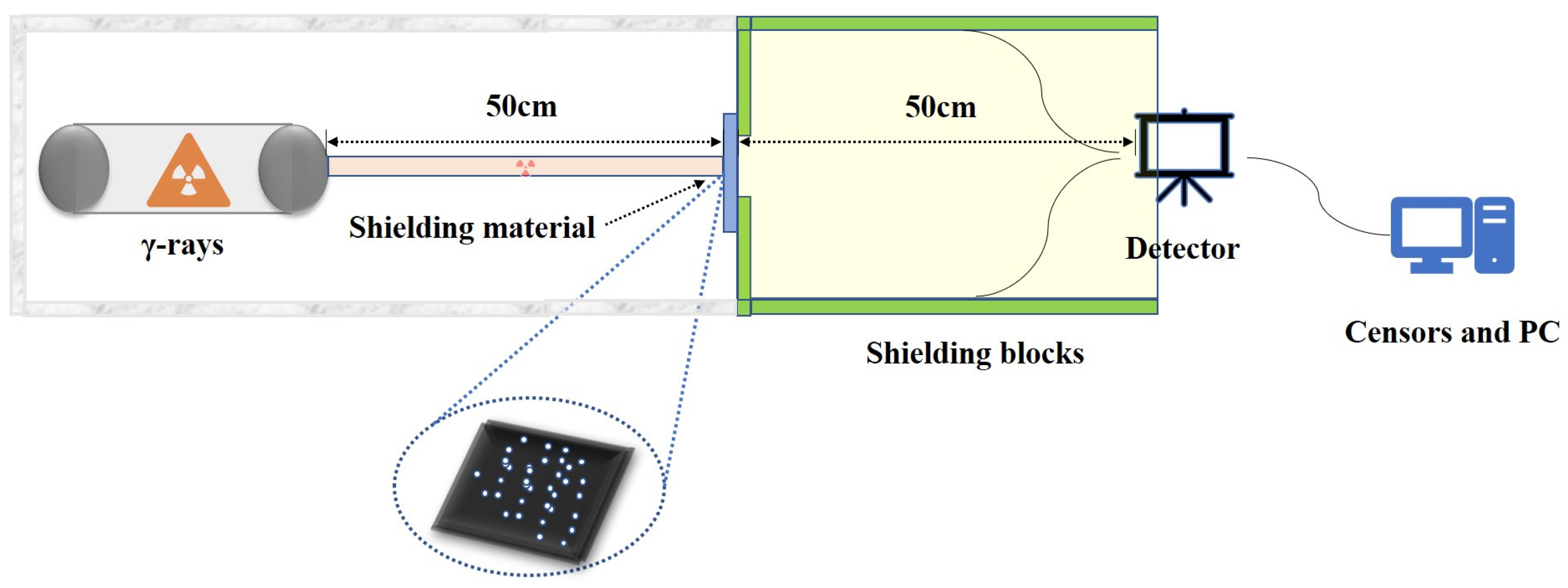
3.2. Design of the Simulation Experiment
4. Results and Discussion
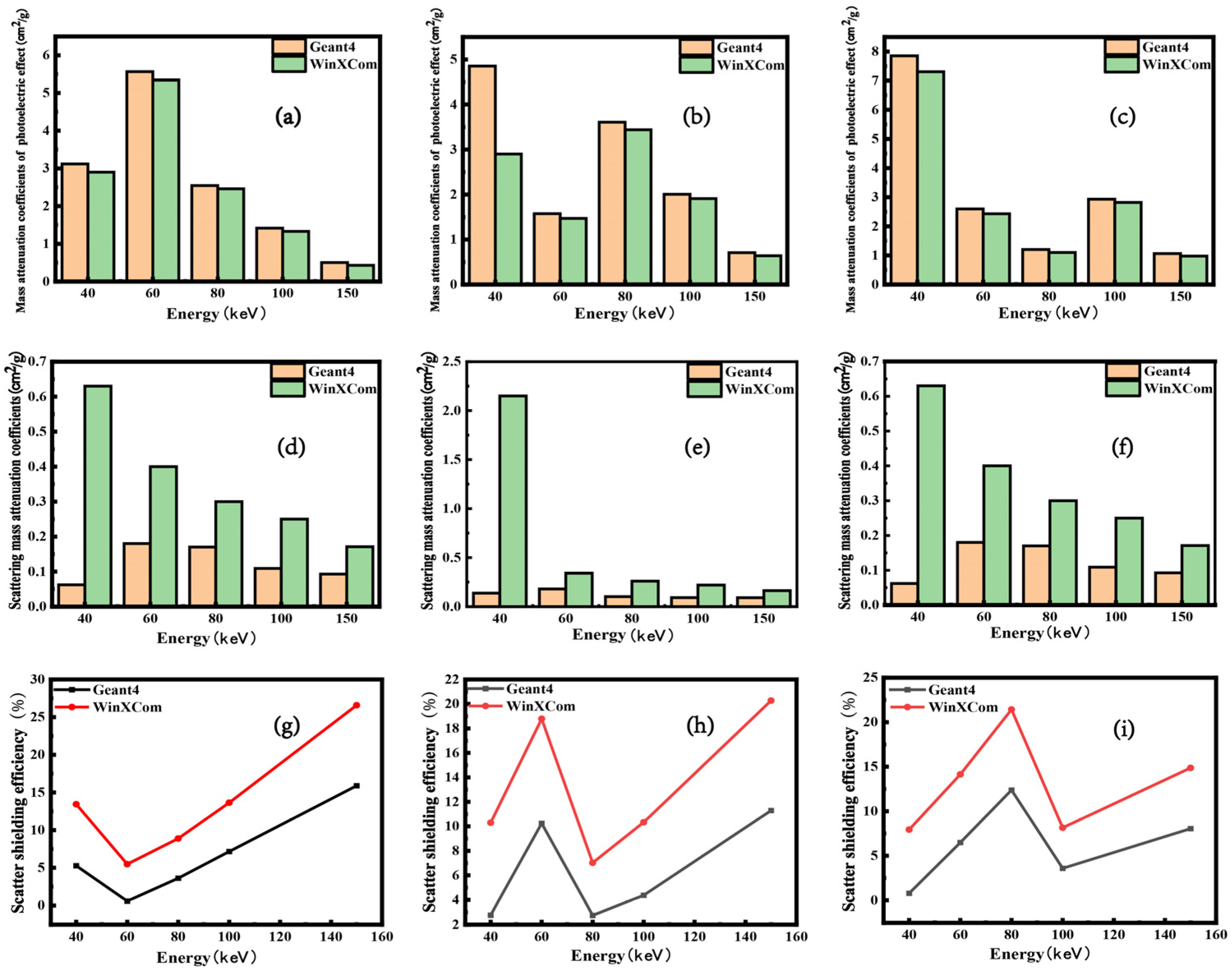
4.1. Comparative Analysis of Shielding Performance of Composite Materials Doped with Single and Multiple Shielding Fillers
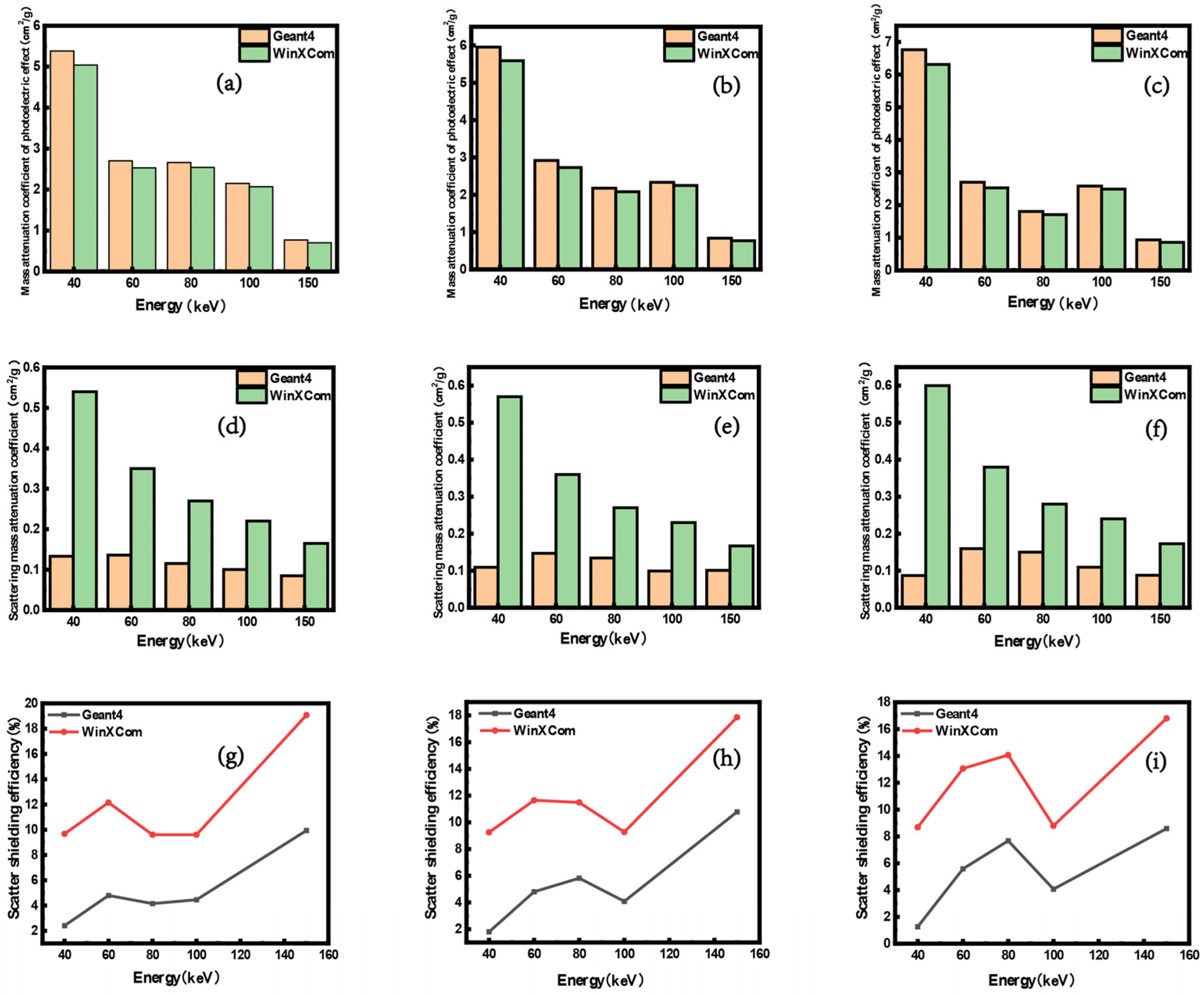
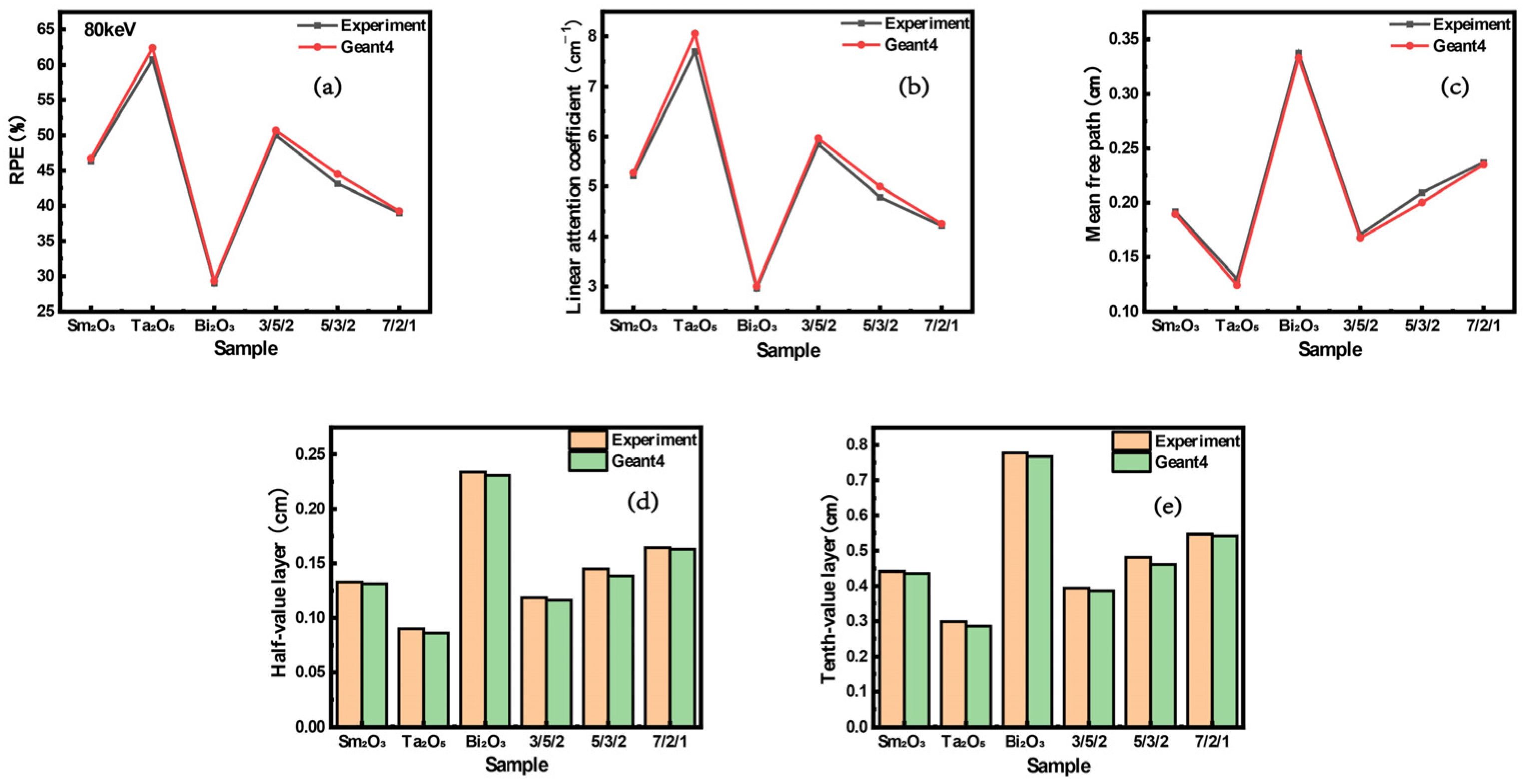
4.2. Simulation and Verification of Radiation Shielding Performance
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Mai, F.-H.; Zhang, Q.-P.; Wang, R.; Meng, L.-C.; Zhang, Y.; Li, J.-L.; Liu, P.-Q.; Li, Y.-T.; Zhou, Y.-L. Polymer Fibers Highly Filled with Styrene Maleic Anhydride-Modified PbWO4 for Improved Wear Comfort of γ-ray-Shielding Articles. ACS Appl. Polym. Mater. 2022, 4, 6394–6402. [Google Scholar] [CrossRef]
- Zegaoui, A.; Derradji, M.; Medjahed, A.; Ghouti, H.A.; Cai, W.-A.; Liu, W.-B.; Dayo, A.Q.; Wang, J.; Liu, Y.-G. Exploring the hybrid effects of short glass/basalt fibers on the mechanical, thermal and gamma-radiation shielding properties of DCBA/BA-a resin composites. Polym.-Plast. Technol. Mater. 2019, 59, 311–322. [Google Scholar] [CrossRef]
- Agrawal, V.; Paulose, R.; Arya, R.; Rajak, G.; Giri, A.; Bijanu, A.; Sanghi, S.K.; Mishra, D.; Prasanth, N.; Khare, A.K.; et al. Green conversion of hazardous red mud into diagnostic X-ray shielding tiles. J. Hazard. Mater. 2022, 424, 127507. [Google Scholar] [CrossRef]
- Tuljittraporn, A.; Yonphan, S.; Chaiphaksa, W.; Kaewkhao, J.; Kothan, S.; Intachai, N.; Kaewjaeng, S.; Johns, J.; Kalkornsurapranee, E. Developing effective gamma and X-ray shielding materials: Thermoplastic natural rubber composites with antimony oxide. Polym. Adv. Technol. 2023, 34, 3818–3825. [Google Scholar] [CrossRef]
- Toyen, D.; Wimolmala, E.; Saenboonruang, K. Multi-Layered Composites of Natural Rubber (NR) and Bismuth Oxide (Bi2O3) with Enhanced X-ray Shielding and Mechanical Properties. Polymers 2023, 15, 2735. [Google Scholar] [CrossRef]
- Bagheri Khatibani, A.; Khoshhal, A.R.; Tochaee, E.B.; Jamnani, S.R.; Moghaddam, H.M. Physical and gamma radiation shielding features of Sm2O3/graphene nanoparticles: A comparison between experimental and simulated gamma shielding capability. Inorg. Chem. Commun. 2024, 167, 112772. [Google Scholar] [CrossRef]
- Muthamma, M.V.; Bubbly, S.G.; Gudennavar, S.B. Attenuation properties of epoxy-Ta2O5 and epoxy-Ta2O5-Bi2O3 composites at γ-ray energies 59.54 and 662 keV. J. Appl. Polym. Sci. 2020, 137, 49366. [Google Scholar] [CrossRef]
- Chaiphaksa, W.; Yonphan, S.; Kalkornsurapranee, E.; Tuljittraporn, A.; Kothan, S.; Kaewjaeng, S.; Kedkaew, C.; Kaewkhao, J. Photon, charged particles, and neutron shielding properties of natural rubber/SnO2 composites. Radiat. Phys. Chem. 2023, 203, 110622. [Google Scholar] [CrossRef]
- Subedi, B.; Lamichhane, T.R. Radiation shielding properties of low-density Ti-based bulk metallic glass composites: A computational study. Phys. Scr. 2023, 98, 035003. [Google Scholar] [CrossRef]
- Gerward, L.; Guilbert, N.; Jensen, K.B.; Levring, H. WinXCom—A program for calculating X-ray attenuation coefficients. Radiat. Phys. Chem. 2004, 71, 653–654. [Google Scholar] [CrossRef]
- Alzahrani, J.S.; Alrowaili, Z.A.; Eke, C.; Mahmoud, Z.M.M.; Mutuwong, C.; Al-Buriahi, M.S. Nuclear shielding properties of Ni-, Fe-, Pb-, and W-based alloys. Radiat. Phys. Chem. 2022, 195, 110090. [Google Scholar] [CrossRef]
- Taqi, A.H.; Ghalib, A.M.; Mohammed, H.N. Shielding properties of Cu-Sn-Pb alloy by Geant4, XCOM and experimental data. Mater. Today Commun. 2021, 26, 101996. [Google Scholar] [CrossRef]
- El-Sharkawy, R.M.; Allam, E.A.; El-Taher, A.; Elsaman, R.; El Sayed Massoud, E.; Mahmoud, M.E. Synergistic effects on gamma-ray shielding by novel light-weight nanocomposite materials of bentonite containing nano Bi2O3 additive. Ceram. Int. 2022, 48, 7291–7303. [Google Scholar] [CrossRef]
- Rezaei-Ochbelagh, D.; Azimkhani, S. Investigation of gamma-ray shielding properties of concrete containing different percentages of lead. Appl. Radiat. Isot. 2012, 70, 2282–2286. [Google Scholar] [CrossRef] [PubMed]
- Li, F.; Liu, Z.; Sun, T. Authentication of vegetable oils by confocal X-ray scattering analysis with coherent/incoherent scattered X-rays. Food Chem. 2016, 210, 435–441. [Google Scholar] [CrossRef]
- Singh, T.B.; Zhang, L.; Huo, X.; Liu, G.; Ni, H.; Zhang, S.; Zhu, W.; Yin, J. The Diagnostic Ability of rs-DWI to Detect Subtle Acute Infarction Lesion in the Different Regions of the Brain and the Comparison between Different b-Values. BioMed Res. Int. 2018, 2018, 7069192. [Google Scholar] [CrossRef]
- Taqi, A.H.; Al Nuaimy, Q.A.M.; Karem, G.A. Study of the properties of soil in Kirkuk, IRAQ. J. Radiat. Res. Appl. Sci. 2016, 9, 259–265. [Google Scholar] [CrossRef]
- Bagheri, R.; Moghaddam, A.K.; Yousefnia, H. Gamma Ray Shielding Study of Barium–Bismuth–Borosilicate Glasses as Transparent Shielding Materials using MCNP-4C Code, XCOM Program, and Available Experimental Data. Nucl. Eng. Technol. 2017, 49, 216–223. [Google Scholar] [CrossRef]
- Gerward, L.; Guilbert, N.; Jensen, K.B.; Levring, H. X-ray absorption in matter. Reengineering XCOM. Radiat. Phys. Chem. 2001, 60, 23–24. [Google Scholar] [CrossRef]
- Alabsy, M.T.; Elzaher, M.A. Radiation shielding performance of metal oxides/EPDM rubber composites using Geant4 simulation and computational study. Sci. Rep. 2023, 13, 7744. [Google Scholar] [CrossRef]
- Margulis, M.; Li, B.; Wu, B.; Sun, G.; Hao, L.; Song, J.; Fischer, U.; Blaise, P. Application of Supermc3.2 To Preliminary Neutronics Analysis for European Hcpb Demo. EPJ Web Conf. 2021, 247, 1–8. [Google Scholar] [CrossRef]
- Böhlen, T.T.; Cerutti, F.; Chin, M.P.W.; Fassò, A.; Ferrari, A.; Ortega, P.G.; Mairani, A.; Sala, P.R.; Smirnov, G.; Vlachoudis, V. The FLUKA Code: Developments and Challenges for High Energy and Medical Applications. Nucl. Data Sheets 2014, 120, 211–214. [Google Scholar] [CrossRef]
- Archambault, J.P.; Mainegra-Hing, E. Comparison between EGSnrc, Geant4, MCNP5 and Penelope for mono-energetic electron beams. Phys. Med. Biol. 2015, 60, 4951–4962. [Google Scholar] [CrossRef] [PubMed]
- Wang, W.; Liu, Y.; Li, S.; Dong, K.; Wang, S.; Cai, P.; Hou, L.; Dou, H.; Liang, D.; Algadi, H.; et al. Lead-free and wearing comfort 3D composite fiber-needled fabric for highly efficient X-ray shielding. Adv. Compos. Hybrid Mater. 2023, 6, 76–87. [Google Scholar] [CrossRef]
- Hoedlmoser, H.; Brönner, J.; Greiter, M.B.; Bandalo, V. ISO 4037:2019 Validation of Radiation Qualities by Means of Half-Value Layer and Hp(10) Dosimetry. Radiat. Prot. Dosim. 2019, 187, 438–450. [Google Scholar] [CrossRef]
- Lim, T.Y.; Wagiran, H.; Hussin, R.; Hashim, S. Mass Attenuation Coefficients and Effective Atomic Numbers of Strontium Borate Glass System in the Energy Range 0.01–1.25 MeV. Adv. Mater. Res. 2014, 895, 315–318. [Google Scholar] [CrossRef]
- Li, Q.; Wang, Y.; Xiao, X.; Zhong, R.; Liao, J.; Guo, J.; Liao, X.; Shi, B. Research on X-ray shielding performance of wearable Bi/Ce-natural leather composite materials. J. Hazard. Mater. 2020, 398, 122943. [Google Scholar] [CrossRef]

| Advantages | Shortcomings | |
|---|---|---|
| Geant4-11.1.1 [20] | Boasts a robust 3D visual interface, facilitating a broad spectrum of applications and the capacity to navigate complex physical processes | Presents a steep learning curve for beginners, necessitating extended learning periods and advanced computer hardware specifications |
| SuperMC-3.3.0 [21] | Demonstrates marked practicability and computational efficiency in specialized nuclear radiation domains | Its applicability in certain domains is constrained, and it lacks sufficient international recognition compared to other software |
| FLUKA-4.1 [22] | Capable of simulating a diverse array of particle interaction processes | Users are required to possess knowledge in physics and computing, with the graphical interface leaving room for improvement |
| EGS-4 [23] | Offers exceptionally high simulation accuracy for electrons and photons | Its simulation capabilities for other particle types are less robust |
| MCNP-5 [22] | This tool is extensively adopted in radiation protection, offering practical neutron simulation capabilities | Encounters limitations when addressing intricate physical phenomena |
| Sample | Filling Composition (200 g) | Density (g/cm3) | Thickness (cm) |
|---|---|---|---|
| 1 | Sm2O3 | 1.997 | 0.1194 |
| 2 | Ta2O5 | 2.172 | 0.1215 |
| 3 | Bi2O3 | 2.182 | 0.1157 |
| 4 | Bi2O3/Ta2O5/Sm2O3(3/5/2) | 2.149 | 0.1186 |
| 5 | Bi2O3/Ta2O5/Sm2O3(5/3/2) | 2.162 | 0.1179 |
| 6 | Bi2O3/Ta2O5/Sm2O3(7/2/1) | 2.175 | 0.1172 |
| γ-Ray Energy (80 keV) Mass Attenuation Coefficients (cm2/g) | |||||
|---|---|---|---|---|---|
| Sample | Experimental Value | Geant4 | Dev (%) | WinXCom | Dev (%) |
| Sm2O3 | 2.584 ± 0.022 | 2.643 | 2.28 ± 0.86 | 2.700 | 4.49 ± 0.88 |
| Ta2O5 | 3.593 ± 0.031 | 3.709 | 3.23 ± 0.86 | 3.700 | 2.98 ± 0.86 |
| Bi2O3 | 1.333 ± 0.017 | 1.376 | 3.23 ± 1.30 | 1.400 | 5.03 ± 1.33 |
| 3/5/2 | 2.673 ± 0.028 | 2.776 | 3.85 ± 1.07 | 2.810 | 5.13 ± 1.09 |
| 5/3/2 | 2.283 ± 0.010 | 2.311 | 1.23 ± 0.44 | 2.350 | 2.94 ± 0.45 |
| 7/2/1 | 1.918 ± 0.013 | 1.955 | 1.93 ± 0.69 | 1.990 | 3.75 ± 0.69 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Li, X.; Yin, Y.; Li, Z.; Yao, H.; Li, Z.; Li, H. Design and Computational Validation of γ-Ray Shielding Effectiveness in Heavy Metal/Rare Earth Oxide–Natural Rubber Composites. Polymers 2024, 16, 2130. https://doi.org/10.3390/polym16152130
Liu Y, Li X, Yin Y, Li Z, Yao H, Li Z, Li H. Design and Computational Validation of γ-Ray Shielding Effectiveness in Heavy Metal/Rare Earth Oxide–Natural Rubber Composites. Polymers. 2024; 16(15):2130. https://doi.org/10.3390/polym16152130
Chicago/Turabian StyleLiu, Yongkang, Xiaopeng Li, Yilin Yin, Zhen Li, Huisheng Yao, Zenghe Li, and Heguo Li. 2024. "Design and Computational Validation of γ-Ray Shielding Effectiveness in Heavy Metal/Rare Earth Oxide–Natural Rubber Composites" Polymers 16, no. 15: 2130. https://doi.org/10.3390/polym16152130
APA StyleLiu, Y., Li, X., Yin, Y., Li, Z., Yao, H., Li, Z., & Li, H. (2024). Design and Computational Validation of γ-Ray Shielding Effectiveness in Heavy Metal/Rare Earth Oxide–Natural Rubber Composites. Polymers, 16(15), 2130. https://doi.org/10.3390/polym16152130






