Experimental and Numerical Analysis of the Damage Mechanism of an Aramid Fabric Panel Engaged in a Medium-Velocity Impact
Abstract
1. Introduction
- It is 43% lighter than glass fiber (density is 1.44 g/cc compared to 2.55 g/cc for glass fiber);
- It is twice as strong as E-Glass and ten times stronger than aluminum;
- It offers the same specific tensile strength as high-strength carbon;
- It has an excellent dimensional stability with a slightly negative coefficient of thermal expansion (−2.4 × 10−6/°C);
- It is resistant to most chemicals except a few strong acids and bases;
- It can withstand extremely low temperatures down to −320 °F (−196 °C) without loss of strength;
- It does not melt but begins to carbonize at about 800 °F (427 °C).
- Providing sufficient endurance to guarantee wearers adequate protection against ballistic threats;
- Being light and compact in nature to avoid hindering the wearer’s body movements and effectiveness;
- Displaying durability, ensuring that its effectiveness does not deteriorate in adverse climatic situations, such as exposure to moisture or UV radiation;
- Possessing flexibility to provide sufficient comfort;
- Featuring excellent kinetic energy of absorption and dissipation.
2. Materials and Methods
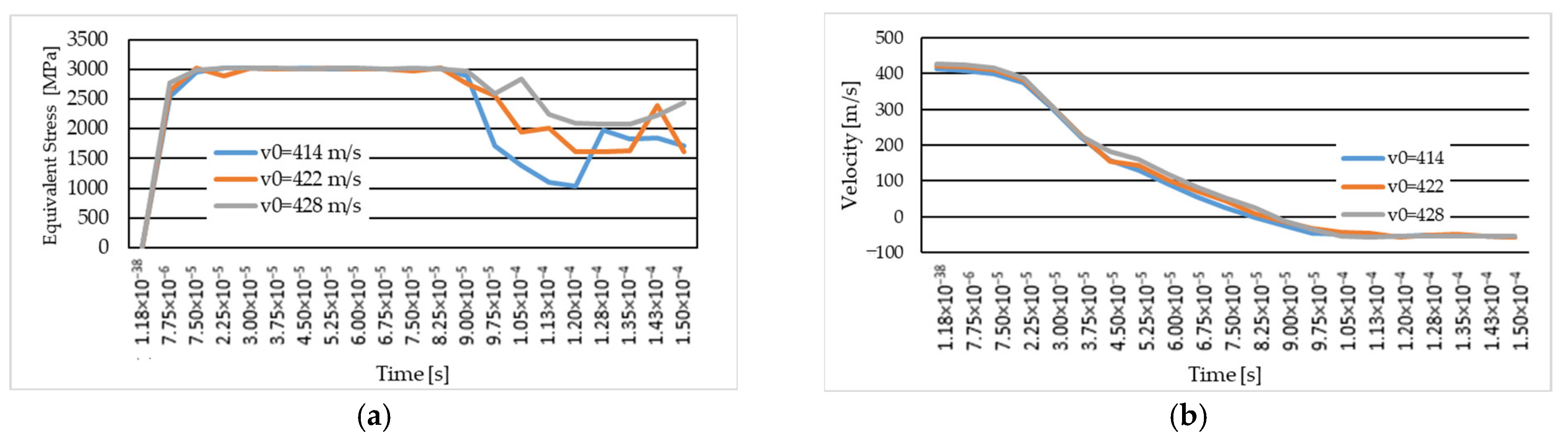
- Variant 1, initial projectile impact velocity, 414 m/s, yield strength, 3000 MPa;
- Variant 2, initial projectile impact velocity, 414 m/s, yield strength, 3600 MPa;
- Variant 3, initial projectile impact velocity, 422 m/s, yield strength, 3000 MPa;
- Variant 4, initial projectile impact velocity, 428 m/s, yield strength, 3000 MPa.
- Three initial impact velocities are studied (v0 = 414 m/s, v0 = 422 m/s, v0 = 428 m/s);
- For the initial projectile velocity of 414 m/s, two values for the yield strength of the yarn (yield strength = 3600 MPa, yield strength = 3000 MPa) are analyzed, and for the initial projectile velocities of 422 m/s and 428 m/s, only one value for the yield strength of the yarn (yield strength = 3000 MPa) is analyzed.
- Boundary conditions: each thread has fixed lateral cross sections.
- Contact conditions (frictional contact): frictional contact between yarns with a friction coefficient value of 0.31 and frictional contact between projectile and yarns with a friction coefficient value of 0.31. The friction coefficient is assumed to be constant.
- The material behavior of the yarn is described using a bilinear model, which suggests a material response characterized by two distinct linear regions.
- The material behavior of the projectile jacket is described using a bilinear model.
- The behavior of the projectile core material is described using the Johnson–Cook model.
- The failure criterion for yarn is Equivalent Plastic Strain (EPS).
- The failure criterion for the projectile jacket is Equivalent Plastic Strain (EPS).
- The failure criterion for the projectile core is Equivalent Plastic Strain (EPS).
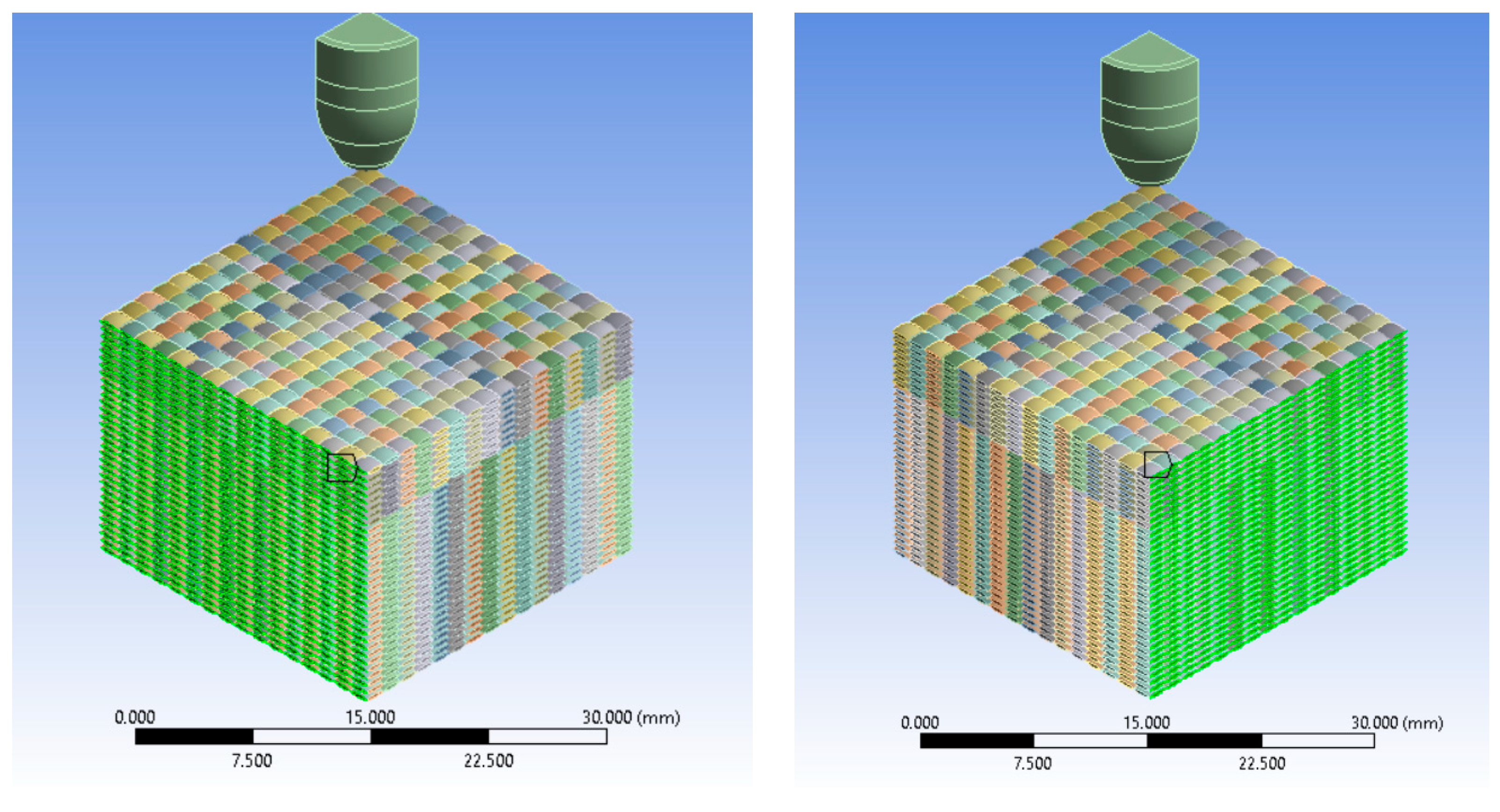
- Two planes of symmetry.
- Mesh = 0.8 mm.
3. Experimental Results
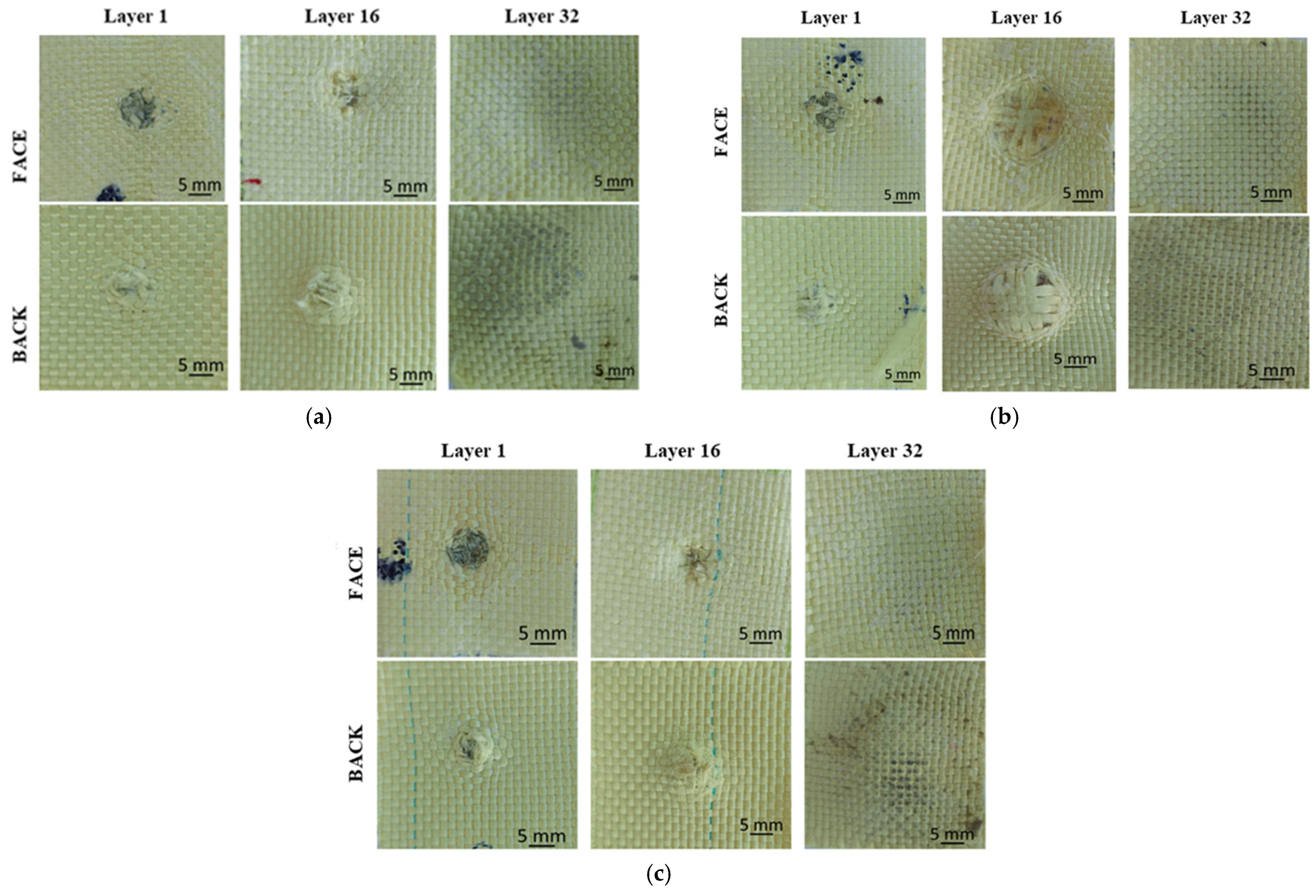
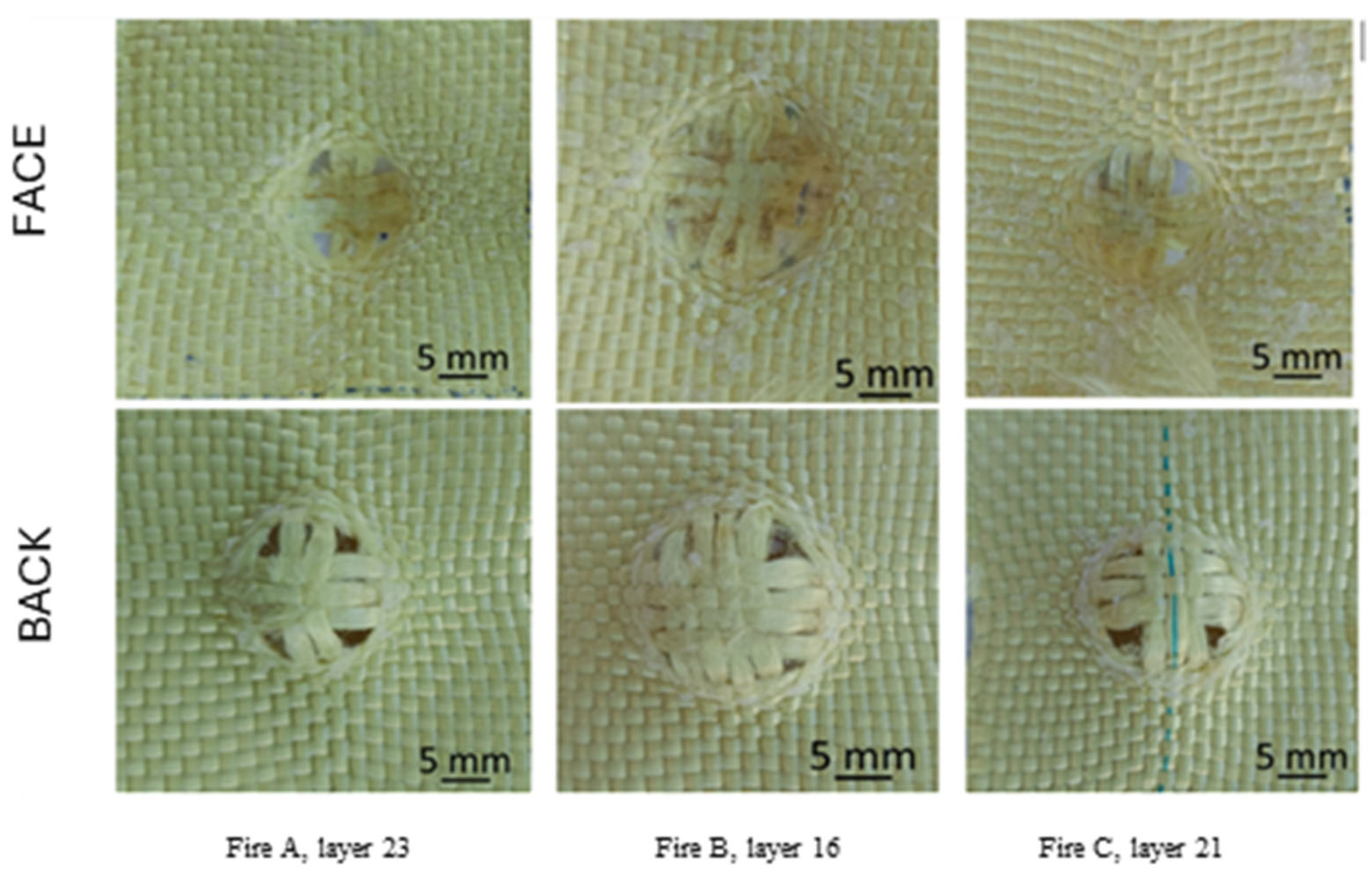
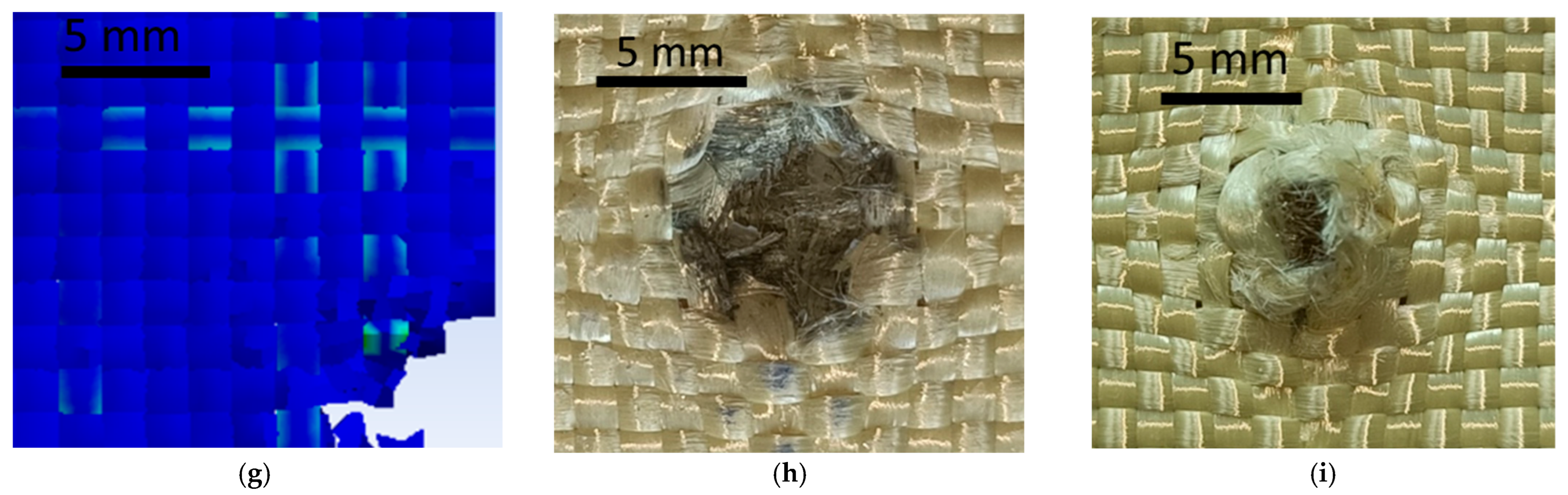
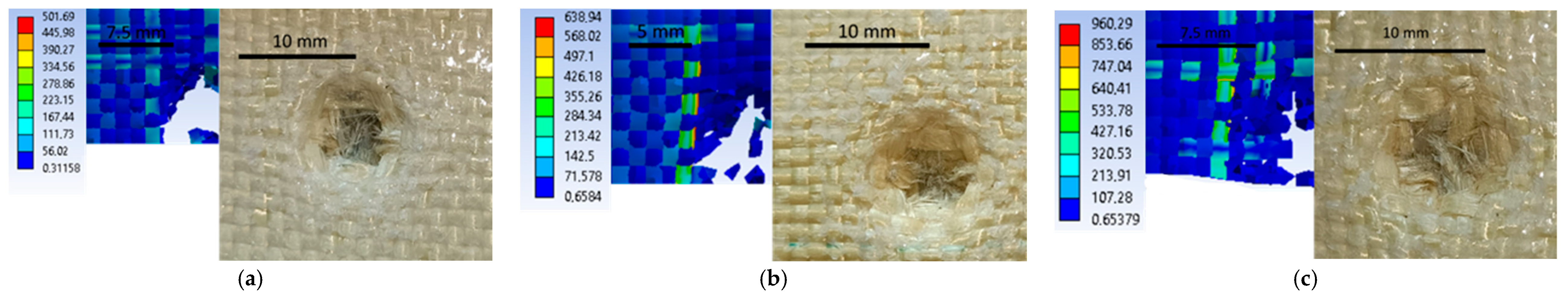
3.1. Analysis of the Damage Mechanism of Ballistic Impact Panel Using Photographs
3.2. Analysis of the Damage Mechanism of Ballistic Impact Panels Using Scanning Electron Microscopy (SEM)
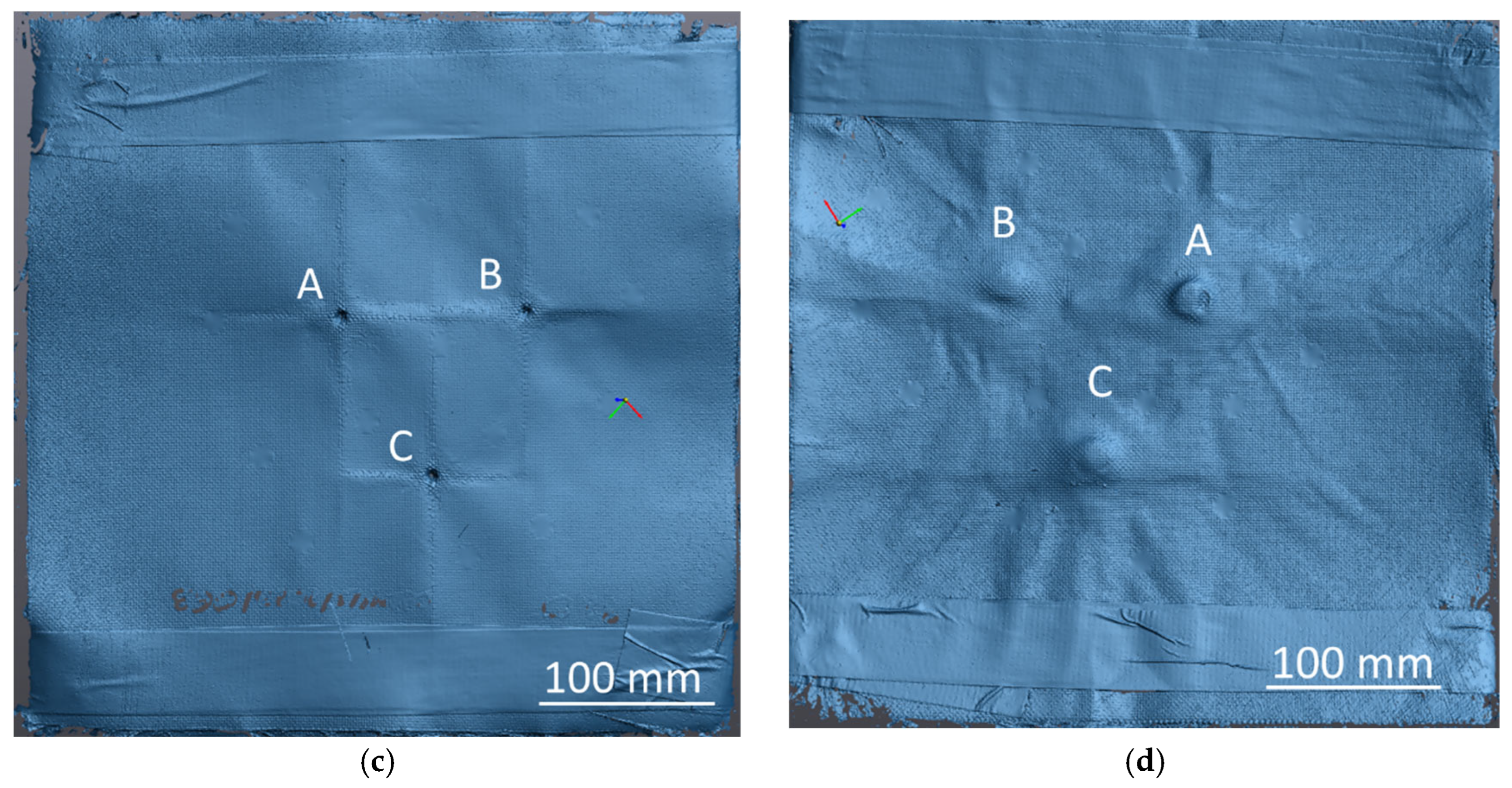
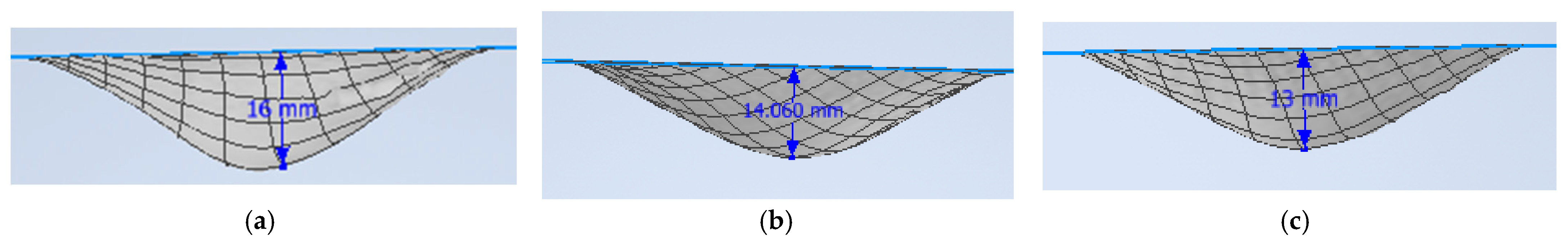
3.3. Analysis of the Indentation Depth in the Support Material
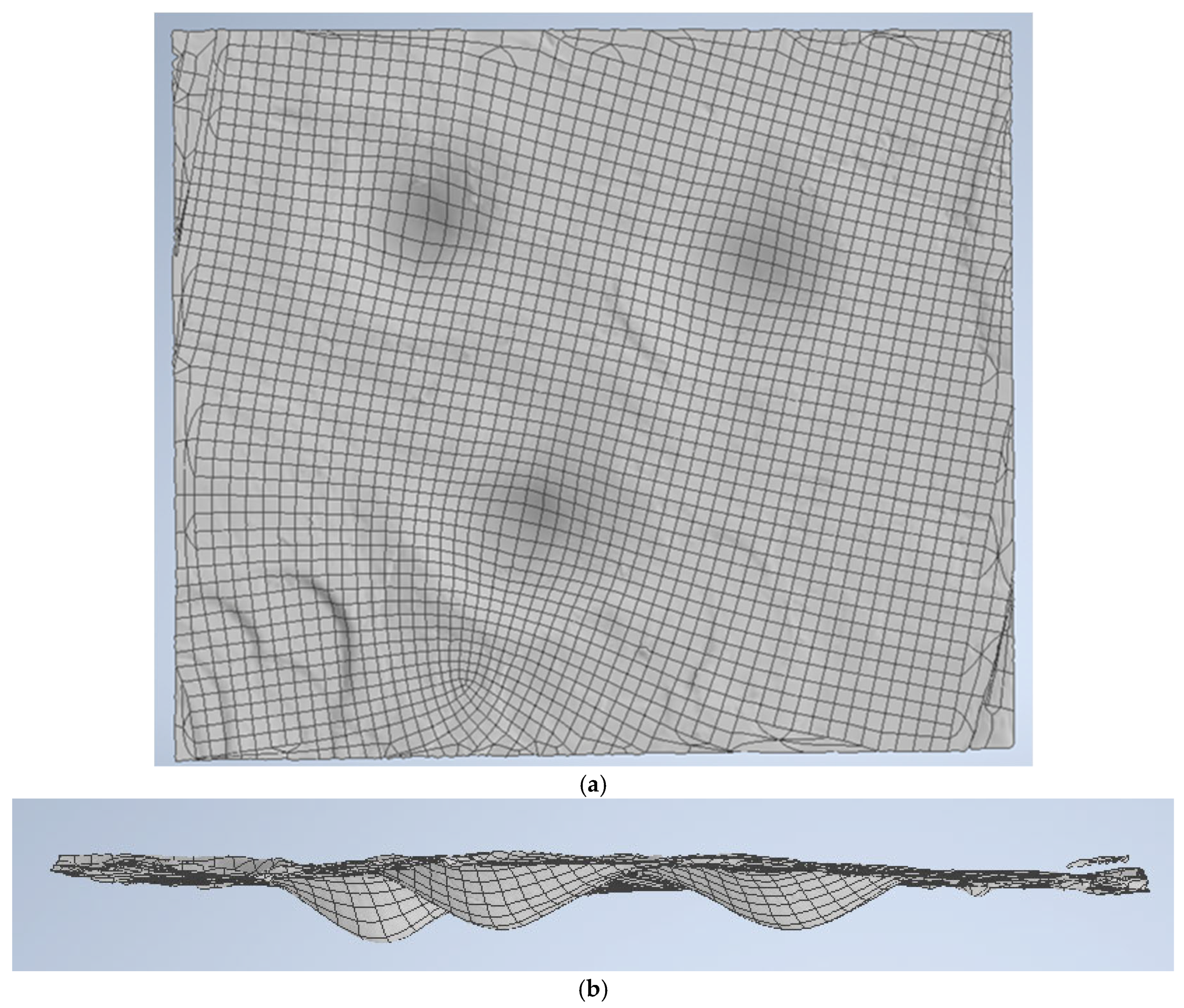
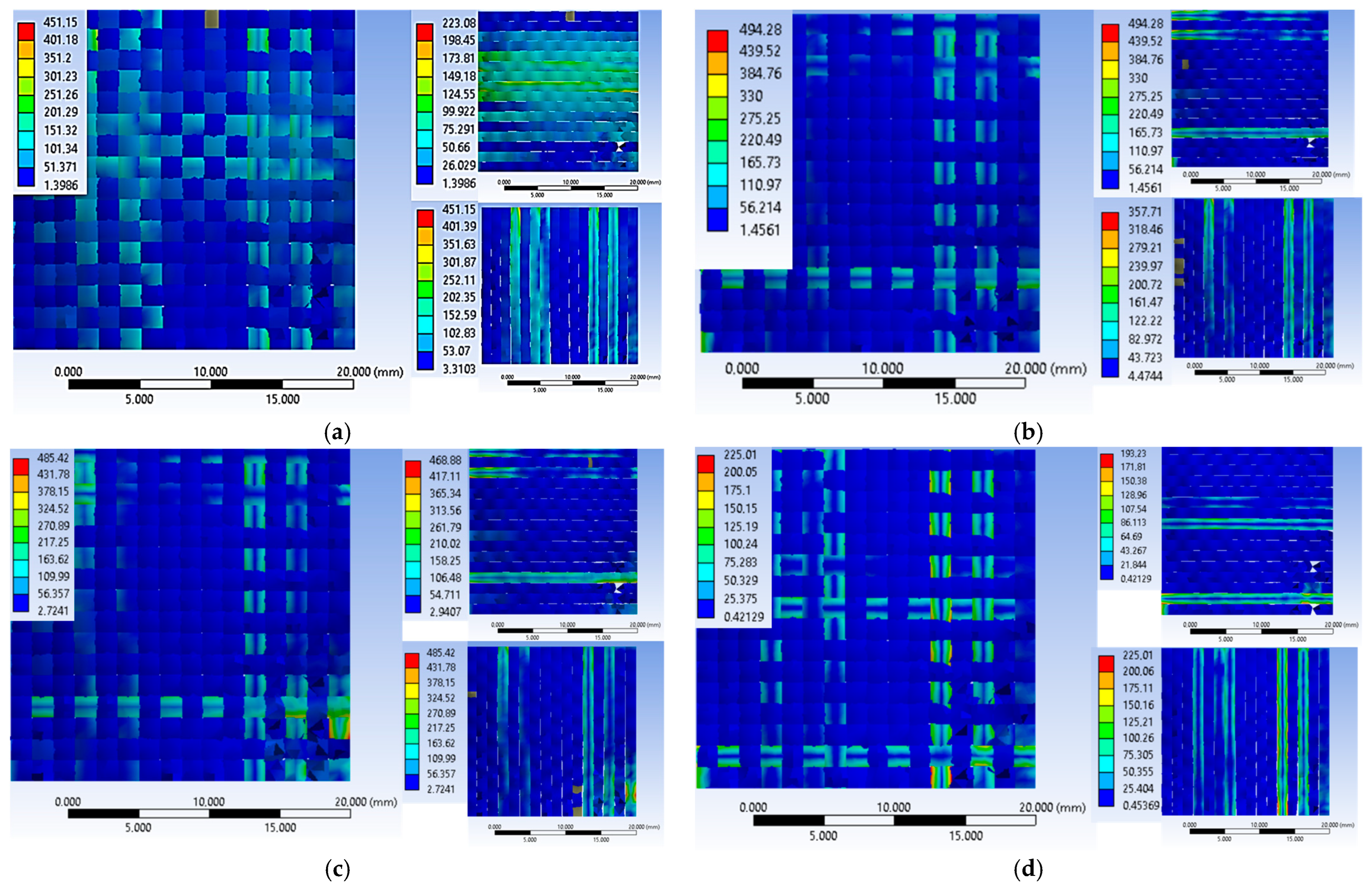
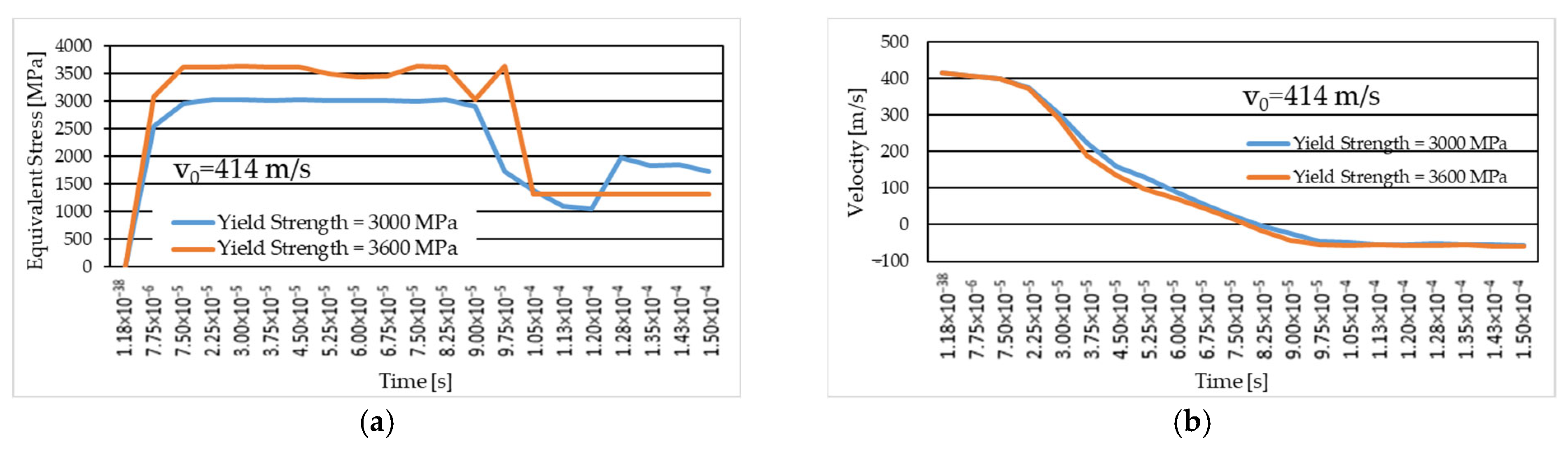
4. Analysis of Results from Numerical Simulations
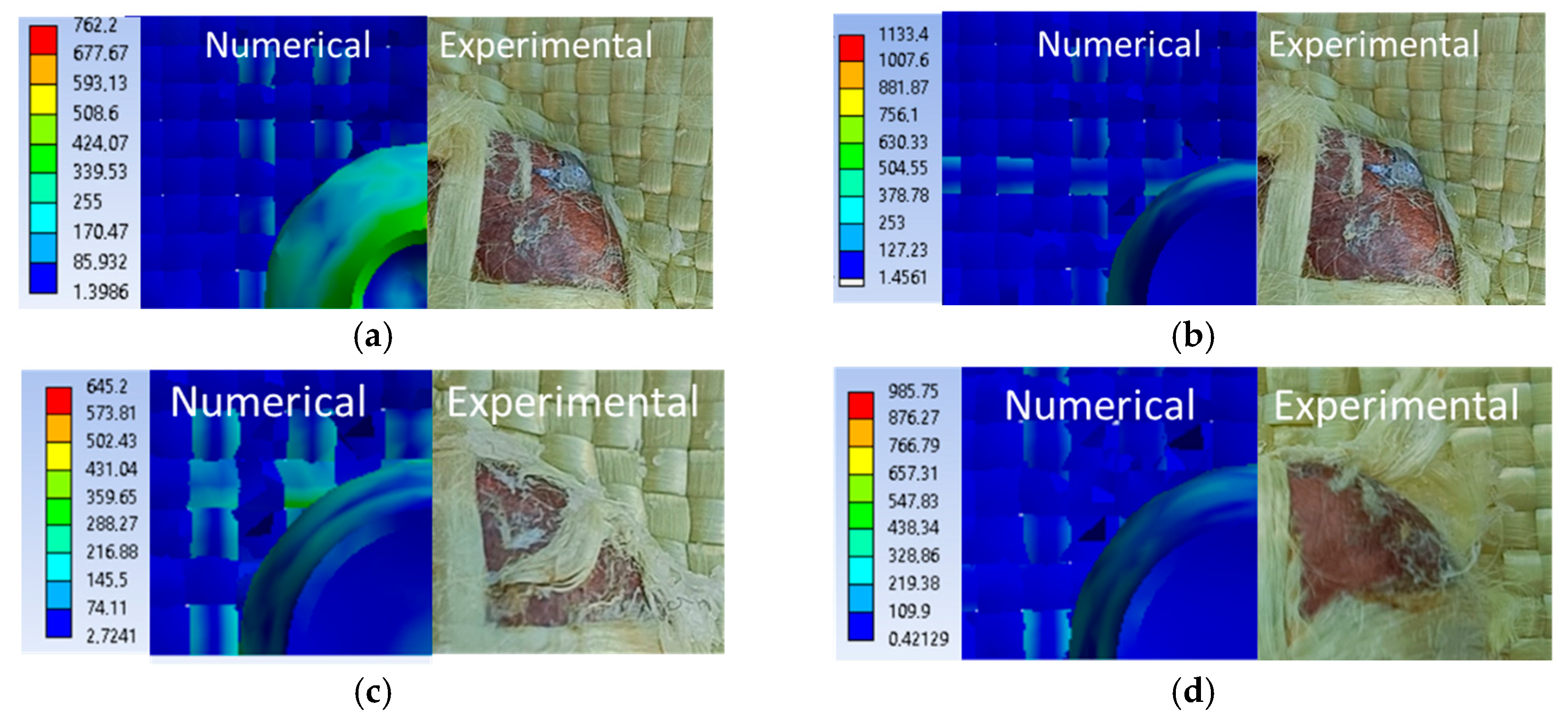
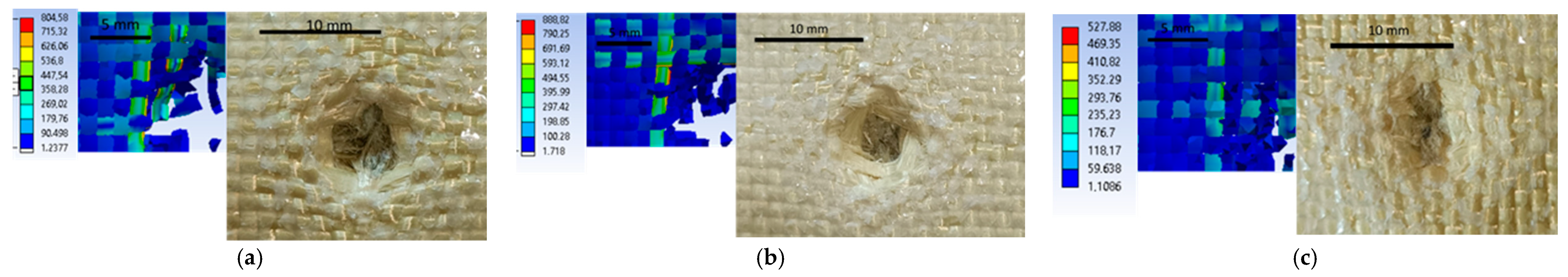
5. Validation of the Numerical Simulations
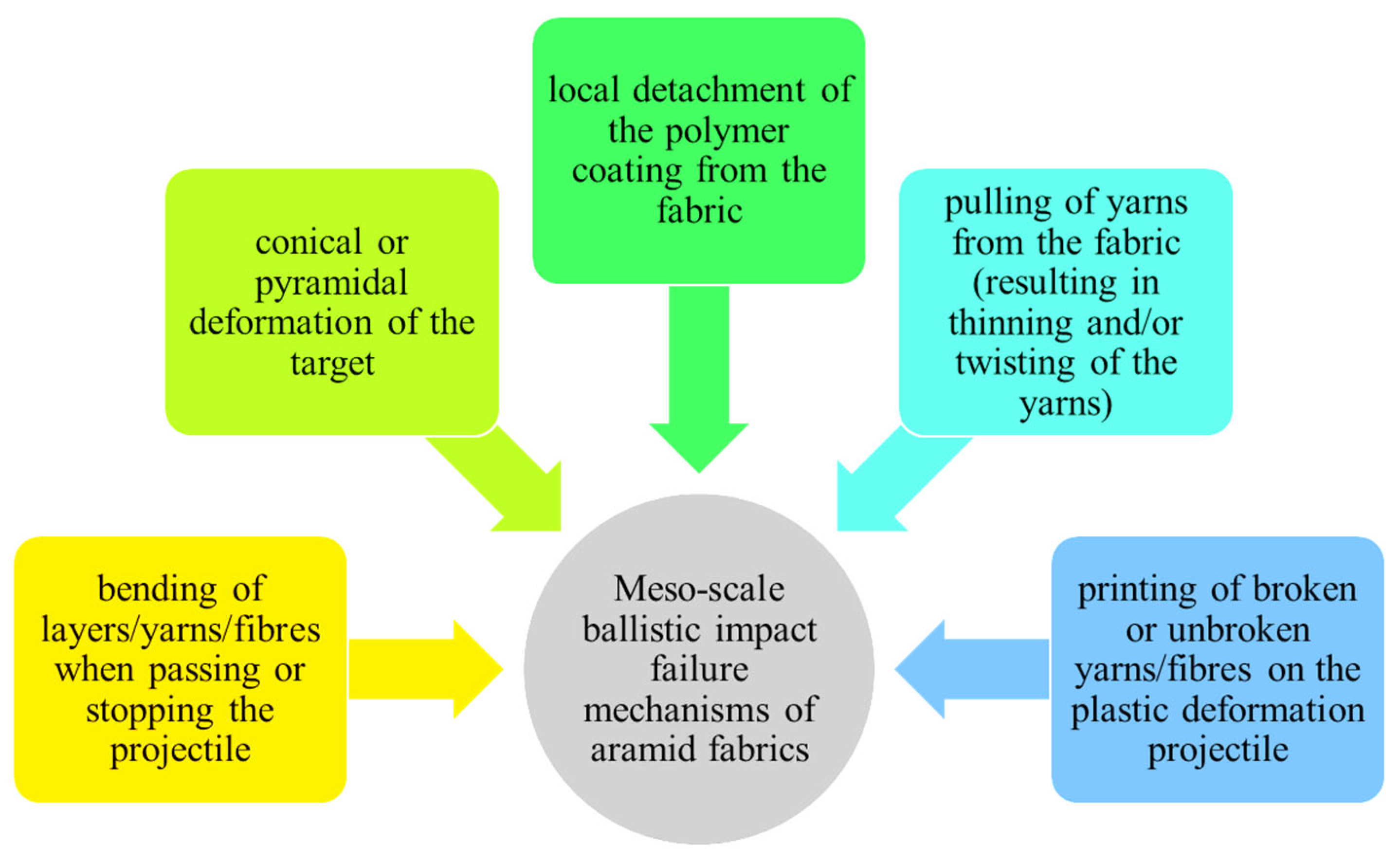
- The number of broken layers (this criterion involves analyzing and recording the number of layers that are broken or damaged during the simulation; this is essential for assessing the effectiveness of the projectile, and its ability to penetrate and damage the target or target structure. The number of broken layers can be an indicator of projectile power and penetration, and is compared with experimental results to validate the model).
- The qualitative aspect (it involves analyzing the simulation results to check that they correspond to the experimental results; this may include assessing how the projectile behaves in terms of deformation, fragmentation, direction of impact, and any other important characteristics; the qualitative aspect is important to ensure that the simulation correctly reproduces the ballistic phenomena and that the results are plausible).
- Zochowski et al. [13] validated laboratory tests (Twaron T750 fabric under impact conditions) both qualitatively and quantitatively. The numerical simulation was also modeled at meso-level, with two planes of symmetry, the projectile is 9 mm FMJ, and initial projectile velocity of 460 m/s.
- Giannaros et al. [67] validated quantitative numerical simulation with laboratory tests of aramid fabrics subjected to ballistic impact. The fabric was modeled at the meso-level. The projectile used is a spherical projectile with a diameter of 12.7 mm and a mass of 8.4 g.
- Clifton et al. [68] validate laboratory tests on the impact influence of polymer composites made of hybrid and non-hybrid fabrics in terms of quality and quantity (impact energy and absorbed energy). Results obtained from numerical simulations and laboratory tests show differences below 14%.
6. Conclusions
- Number of broken layers: This is a quantitative indicator of the strength of the material and its ability to distribute the forces generated by the impact. A lower number of broken layers indicates better performance.
- Qualitative aspect: This provides a visual and structural assessment of the panel after impact, contributing to the understanding of the material behavior and its effectiveness under ballistic stress conditions.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- El Messiry, M. Introduction. In Protective Armor Engineering Design; CRC Press: Boca Raton, FL, USA; Woodhead Publishing: Sawston, UK; pp. 18–22.
- Chen, X.; Zhou, Y.; Wells, G. Numerical and experimental investigations into ballistic performance of hybrid fabric panels. Compos. Part B Eng. 2014, 58, 35–42. [Google Scholar] [CrossRef]
- Hu, Q.R.; Shen, X.Y.; Qian, X.M.; Huang, G.Y.; Yuan, M.Q. The personal protective equipment (PPE) based on individual combat: A systematic review and trend analysis. Def. Technol. 2023, 28, 195–221. [Google Scholar] [CrossRef]
- Crouch, I.G. Body armour–New materials, new systems. Def. Technol. 2019, 15, 241–253. [Google Scholar] [CrossRef]
- McCullough, E.A. Evaluation of protective clothing systems using manikins. In Textiles for Protection; Scott, E.A., Ed.; Woodhead Publishing Series in Textiles; Woodhead Publishing: Sawston, UK, 2005; pp. 217–232. [Google Scholar]
- Sun, D.; Jiang, J.; Zhang, M.; Wang, Z. Ballistic experiments on the mechanism of protective layer against domino effect caused by projectiles. J. Loss Prev. Process Ind. 2016, 40, 17–28. [Google Scholar] [CrossRef]
- Abtew, M.A.; Boussu, F.; Bruniaux, P.; Loghin, C.; Cristian, I. Ballistic impact mechanisms—A review on textiles and fibre-reinforced composites impact responses. Compos. Struct. 2019, 223, 110966. [Google Scholar] [CrossRef]
- Jacobs, M.J.N.; Van Dingenen, J.L.J. Ballistic protection mechanisms in personal armour. J. Mater. Sci. 2001, 36, 3137–3142. [Google Scholar] [CrossRef]
- Bandaru, A.K.; Chavan, V.V.; Ahmad, S.; Alagirusamy, R.; Bhatnagar, N. Ballistic impact response of Kevlar® reinforced thermoplastic composite armors. Int. J. Impact Eng. 2016, 89, 1–13. [Google Scholar] [CrossRef]
- Tam, T.; Bhatnagar, A. High-performance ballistic fibers and tapes. In Lightweight Ballistic Composites, 2nd ed.; Bhatnagar, A., Ed.; Woodhead Publishing Series in Composites Science and Engineering; Woodhead Publishing: Sawston, UK, 2016; pp. 1–39. [Google Scholar]
- Guo, Z.; Chen, W. A merit parameter to determine the stacking order of heterogeneous diphasic soft armor systems. Compos. Struct. 2020, 241, 112086. [Google Scholar] [CrossRef]
- Palta, E.; Fang, H. On a multi-scale finite element model for evaluating ballistic performance of multi-ply woven fabrics. Compos. Struct. 2019, 207, 488–508. [Google Scholar] [CrossRef]
- Zochowski, P.; Bajkowski, M.; Grygoruk, R.; Magier, M.; Burian, W.; Pyka, D.; Bocian, M.; Jamroziak, K. Finite element modeling of ballistic inserts containing aramid fabrics under projectile impact conditions–comparison of methods. Compos. Struct. 2022, 294, 115752. [Google Scholar] [CrossRef]
- Scazzosi, R.; de Souza, S.D.B.; Amico, S.C.; Giglio, M.; Manes, A. Experimental and numerical evaluation of the perforation resistance of multi-layered alumina/aramid fiber ballistic shield impacted by an armor piercing projectile. Compos. Part B Eng. 2022, 230, 109488. [Google Scholar] [CrossRef]
- Lim, J.S. Ballistic behavior of heracron®-based composites: Effect of the number multifilaments on high-speed projectiles. Model. Numer. Simul. Mater. Sci. 2013, 3, 84–89. [Google Scholar] [CrossRef]
- Nikky; Rao, P.S. A review on material & ballistic energy absorption of body armour. Int. J. Res. Sci. Innov. 2018, 5, 93–99. [Google Scholar]
- Ifuku, S.; Maeta, H.; Izawa, H.; Morimoto, M.; Saimoto, H. Facile preparation of aramid nanofibers from Twaron fibers by a downsizing process. RSC Adv. 2014, 4, 40377–40380. [Google Scholar] [CrossRef]
- Risteski, S.; Srebrenkoska, V.; Zhezhova, S. Applying of different fabrics for design of the protective military clothes. Qual. Life 2017, 8, 56–63. [Google Scholar] [CrossRef]
- Yang, H.M. 1.07—Aramid Fibers. In Comprehensive Composite Materials; Kelly, A., Zweben, C., Eds.; Elsevier: Pergamon, Turkey, 2000; pp. 199–229. ISBN 9780080429939. [Google Scholar] [CrossRef]
- He, A.; Xing, T.; Liang, Z.; Luo, Y.; Zhang, Y.; Wang, M.; Huang, Z.; Bai, J.; Wu, L.; Shi, Z.; et al. Advanced Aramid Fibrous Materials: Fundamentals, Advances, and Beyond. Adv. Fiber Mater. 2024, 6, 3–35. [Google Scholar] [CrossRef]
- Ashby, M.F. (Ed.) Chapter 15—Material profiles. In Materials and the Environment, 2nd ed.; Butterworth-Heinemann: Oxford, UK, 2013; pp. 459–595. ISBN 9780123859716. [Google Scholar] [CrossRef]
- Bilisik, K. 18—Aramid fiber reinforced composites. In Fiber Reinforced Composites; Joseph, K., Oksman, K., George, G., Wilson, R., Appukuttan, S., Eds.; Woodhead Publishing Series in Composites Science and Engineering; Woodhead Publishing: Sawston, UK, 2021; pp. 515–559. ISBN 9780128210901. [Google Scholar] [CrossRef]
- Shih, C.-H.; You, J.-L.; Lee, Y.-L.; Cheng, A.-Y.; Chang, C.-P.; Liu, Y.-M.; Ger, M.-D. Design and Ballistic Performance of Hybrid Plates Manufactured from Aramid Composites for Developing Multilayered Armor Systems. Polymers 2022, 14, 5026. [Google Scholar] [CrossRef]
- Liu, J. Aramid Fiber Composites for Sporting Equipment: Exploring Preparation Methods and Mechanical Properties. Sci. Adv. Mater. 2023, 15, 1598–1604. [Google Scholar] [CrossRef]
- Elgohary, D.H. Technological aspects of body armour textiles for ballistic protection application. J. Text. Inst. 2024, 1–15. [Google Scholar] [CrossRef]
- Hearle, J.W.S. (Ed.) 1—Introduction. In High-Performance Fibres; Woodhead Publishing Series in Textiles; Woodhead Publishing: Sawston, UK, 2001; pp. 1–22. [Google Scholar]
- Sabir, T. Fibers used for high-performance apparel. In High-Performance Apparel; Woodhead Publishing: Sawston, UK, 2018; pp. 7–32. [Google Scholar]
- Lewis, E.; Carr, D.J. 7—Personal armor. In Lightweight Ballistic Composites, 2nd ed.; Bhatnagar, A., Ed.; Woodhead Publishing Series in Composites Science and Engineering; Woodhead Publishing: Sawston, UK, 2016; pp. 217–229. [Google Scholar]
- Chen, X.; Zhou, Y. 6—Technical textiles for ballistic protection. In Handbook of Technical Textiles, 2nd ed.; Horrocks, A.R., Anand, S.C., Eds.; Woodhead Publishing: Sawston, UK, 2016; pp. 169–192. ISBN 9781782424659. [Google Scholar] [CrossRef]
- Bilisik, K.; Syduzzaman Md Erdogan, G.; Korkmaz, M. 3—Advances in ballistic protection. In Functional and Technical Textiles; Maity, S., Singha, K., Pandit, P., Eds.; The Textile Institute Book Series; Woodhead Publishing: Sawston, UK, 2023; pp. 71–139. ISBN 9780323915939. [Google Scholar] [CrossRef]
- David, N.V.; Gao, X.; Zheng, J.Q. Ballistic Resistant Body Armor: Contemporary and Prospective Materials and Related Protection Mechanisms. Appl. Mech. Rev. 2009, 62, 050802. [Google Scholar] [CrossRef]
- Grujicic, M.; Bell, W.C.; Pandurangan, B. Design and material selection guidelines and strategies for transparent armor systems. Mater. Des. 2012, 34, 808–819. [Google Scholar] [CrossRef]
- Yadav, R.; Naebe, M.; Wang, X.; Kandasubramanian, B. Body armour materials: From steel to contemporary biomimetic systems. RSC Adv. 2016, 6, 115145–115174. [Google Scholar] [CrossRef]
- Pai, A.; Chandrakant, R.K.; Satish, S.B. Development of materials and structures for shielding applications against Blast and Ballistic impact: A Detailed Review. Thin-Walled Struct. 2022, 179, 109664. [Google Scholar] [CrossRef]
- Nurazzi, N.M.; Asyraf, M.R.M.; Khalina, A.; Abdullah, N.; Aisyah, H.A.; Rafiqah, S.A.; Sabaruddin, F.A.; Kamarudin, S.H.; Norrrahim, M.N.F.; Ilyas, R.A.; et al. A Review on Natural Fiber Reinforced Polymer Composite for Bullet Proof and Ballistic Applications. Polymers 2021, 13, 646. [Google Scholar] [CrossRef] [PubMed]
- Barhoumi, H.; Bhouri, N.; Feki, I.; Baffoun, A.; Hamdaoui, M.; Ben Abdessalem, S. Review of ballistic protection materials: Properties and performances. J. Reinf. Plast. Compos. 2023, 42, 685–699. [Google Scholar] [CrossRef]
- Jadhav, A.B.; Gaikwad, A.; Gori, Y.; Somaiah, A.; Rambabu, G.; Al-Ataby, F.H.; Saxena, K.K. A review of armour’s use of composite materials. Mater. Today Proc. 2023. [Google Scholar] [CrossRef]
- Kumar, S.; Saxena, S.; Sharma, H.; Gangolu, J.; Prabhu, T.A. Development of design guidelines using probabilistic framework for the development of smart thickening fluid based ultra resistant adaptive kinematic soft human armor (SURAKSHA). Reliab. Eng. Syst. Saf. 2023, 236, 109277. [Google Scholar] [CrossRef]
- Thomas, H.L. 9—Non-woven ballistic composites. In Lightweight Ballistic Composites; Bhatnagar, A., Ed.; Woodhead Publishing Series in Composites Science and Engineering; Woodhead Publishing: Sawston, UK, 2006; pp. 240–271. [Google Scholar]
- Zochowski, P.; Cegła, M.; Szczurowski, K.; Mączak, J.; Bajkowski, M.; Bednarczyk, E.; Grygoruk, R.; Magier, M.; Pyka, D.; Bocian, M.; et al. Experimental and numerical study on failure mechanisms of the 7.62 × 25 mm FMJ projectile and hyperelastic target material during ballistic impact. Contin. Mech. Thermodyn. 2023, 35, 1745–1767. [Google Scholar] [CrossRef]
- Bakhtiari, A.; Sheikhi, M.M.; Arab, N.B.M.; Khodarahmi, H. Studying the ballistic performance of a metal target under combined KE projectile impact. Phys. Scr. 2023, 98, 095207. [Google Scholar] [CrossRef]
- NIJ Standard–0101.06; Ballistic Resistance of Personal Body Armor. U.S. Department of Justice Office of Justice Programs National Institute of Justice: Washington, DC, USA, 2008.
- Oliveira, M.S.; Filho, F.d.C.G.; Pereira, A.C.; Nunes, L.F.; da Luz, F.S.; Braga, F.d.O.; Colorado, H.A.; Monteiro, S.N. Ballistic performance and statistical evaluation of multilayered armor with epoxy-fique fabric composites using the Weibull analysis. J. Mater. Res. Technol. 2019, 8, 5899–5908. [Google Scholar] [CrossRef]
- Yang, Y.; Chen, X. Influence of fabric architecture on energy absorption efficiency of soft armour panel under ballistic impact. Compos. Struct. 2019, 224, 111015. [Google Scholar] [CrossRef]
- Jiang, Y.; Qian, K.; Zhang, Y.; Xia, Y.; Xiong, Z.; Zhang, Z.; Yu, K. Experimental characterisation and numerical simulation of ballistic penetration of columnar ceramic/fiber laminate composite armor. Mater. Des. 2022, 224, 111394. [Google Scholar] [CrossRef]
- Saleem, I.A.; Ahmed, P.S.; Abed, M.S. Experimental and numerical investigation of Kevlar and UHMWPE multi-layered armors against ballistic impact. Mater. Today Proc. 2022, 56, 2516–2524. [Google Scholar] [CrossRef]
- Mudzi, P.; Wu, R.; Firouzi, D.; Ching, C.Y.; Farncombe, T.H.; Selvaganapathy, P.R. Use of patterned thermoplastic hot film to create flexible ballistic composite laminates from UHMWPE fabric. Mater. Des. 2022, 214, 110403. [Google Scholar] [CrossRef]
- Yang, Y.; Chen, X. Investigation of failure modes and influence on ballistic performance of Ultra-High Molecular Weight Polyethylene (UHMWPE) uni-directional laminate for hybrid design. Compos. Struct. 2017, 174, 233–243. [Google Scholar] [CrossRef]
- Kumar, V.; Bajya, M.; Majumdar, A.; Dubey, D.K. Design of Cost-Effective Hybrid Soft Armour Panels Using Strategic Replacement of Backing layers with non-Ballistic Materials: A Parametric Study. Int. J. Impact Eng. 2023, 180, 104678. [Google Scholar] [CrossRef]
- Goode, T.; Shoemaker, G.; Schultz, S.; Peters, K.; Pankow, M. Soft body armor time-dependent back face deformation (BFD) with ballistics gel backing. Compos. Struct. 2019, 220, 687–698. [Google Scholar] [CrossRef]
- Ballistic Material Handbook—Teijin Aramid BV. Available online: https://www.intersecexpo.com/uploads/editor_images/file/Rene%20Lohmann-Dubai%20TTS%202014_SHOW-locked.pdf (accessed on 20 March 2023).
- Yang, Y.; Chen, X. Investigation of energy absorption mechanisms in a soft armor panel under ballistic impact. Text. Res. J. 2017, 87, 2475–2486. [Google Scholar] [CrossRef]
- Tan, V.B.C.; Zeng, X.S.; Shim, V.P.W. Characterization and constitutive modeling of aramid fibers at high strain rates. Int. J. Impact Eng. 2008, 35, 1303–1313. [Google Scholar] [CrossRef]
- Tapie, E.; Shim, V.P.W.; Guo, Y.B. Influence of weaving on the mechanical response of aramid yarns subjected to high-speed loading. Int. J. Impact Eng. 2015, 80, 1–12. [Google Scholar] [CrossRef]
- Manes, A.; Serpellini, F.; Pagani, M.; Saponara, M.; Giglio, M. Perforation and penetration of aluminium target plates by armour piercing bullets. Int. J. Impact Eng. 2014, 69, 39–54. [Google Scholar] [CrossRef]
- Peroni, L.; Scapin, M.; Fichera, C.; Manes, A.; Giglio, M. Mechanical properties at high strain-rate of lead core and brass jacket of aNATO 7.62 mm ball bullet in numerical simulations of ballistic impacts. Proc. DYMAT 2012, 26, 01060. [Google Scholar] [CrossRef]
- Forrestal, M.J.; Børvik, T.; Warren, T.L. Perforation of 7075-T651 aluminum armor plates with 7.62 mm APM2 bullets. Exp. Mech. 2010, 50, 1245–1251. [Google Scholar] [CrossRef]
- Johnson, G.R.; Cook, W.H. Fracture characteristics of three metals subjected to various strains, strain rates, temperatures and pressures. Eng. Fract. Mech. 1985, 21, 31–48. [Google Scholar] [CrossRef]
- Børvik, T.; Dey, S.; Clausen, A.H. Perforation resistance of five different high-strength steel plates subjected to small-arms projectiles. Int. J. Impact Eng. 2009, 36, 948–964. [Google Scholar] [CrossRef]
- Wang, Y.; Chen, X.; Young, R.; Kinloch, I.; Wells, G. A numerical study of ply orientation on ballistic impact resistance of multi-ply fabric panels. Compos. Part B Eng. 2015, 68, 259–265. [Google Scholar] [CrossRef]
- Xu, Y.J.; Zhang, H.; Huang, G.Y. Ballistic performance of B4C/STF/Twaron composite fabric. Compos. Struct. 2022, 279, 114754. [Google Scholar] [CrossRef]
- Mawkhlieng, U.; Majumdar, A. Soft body armour. Text. Prog. 2019, 51, 139–224. [Google Scholar] [CrossRef]
- Stopforth, R.; Adali, S. Experimental study of bullet-proofing capabilities of Kevlar, of different weights and number of layers, with 9 mm projectiles. Def. Technol. 2019, 15, 186–192. [Google Scholar] [CrossRef]
- Kumar, N. Bulletproof vest and its improvement—A review. Int. J. Sci. Eng. Dev. Res. 2016, 1, 34–39. [Google Scholar]
- Kośla, K.; Łandwijt, M.; Miklas, M.; Fejdyś, M. Influence of the Accelerated Aging Process on the Fragment-Resistant Properties of Para-Aramid Body Armor. Materials 2022, 15, 6492. [Google Scholar] [CrossRef] [PubMed]
- Viliš, J.; Neumann, V.; Vítek, R.; Zouhar, J.; Pokorný, Z.; Marek, M. Analysis of Ballistic Impact of 7.62 mm FMJ M80 Rifle Projectile into Twaron/UHMWPE Composite Armor. J. Compos. Sci. 2023, 7, 390. [Google Scholar] [CrossRef]
- Giannaros, E.; Kotzakolios, A.; Sotiriadis, G.; Tsantzalis, S.; Kostopoulos, V. On fabric materials response subjected to ballistic impact using meso-scale modeling. Numerical simulation and experimental validation. Compos. Struct. 2018, 204, 745–754. [Google Scholar] [CrossRef]
- Clifton, S.; Shivamurthy, B.; Abdel-Hamid, I.M.; Rajiv, S.; Mahesh, M. Experimental and finite element study on high-velocity impact resistance and energy absorption of hybrid and non-hybrid fabric reinforced polymer composites. J. Mater. Res. Technol. 2022, 18, 5406–5418. [Google Scholar] [CrossRef]




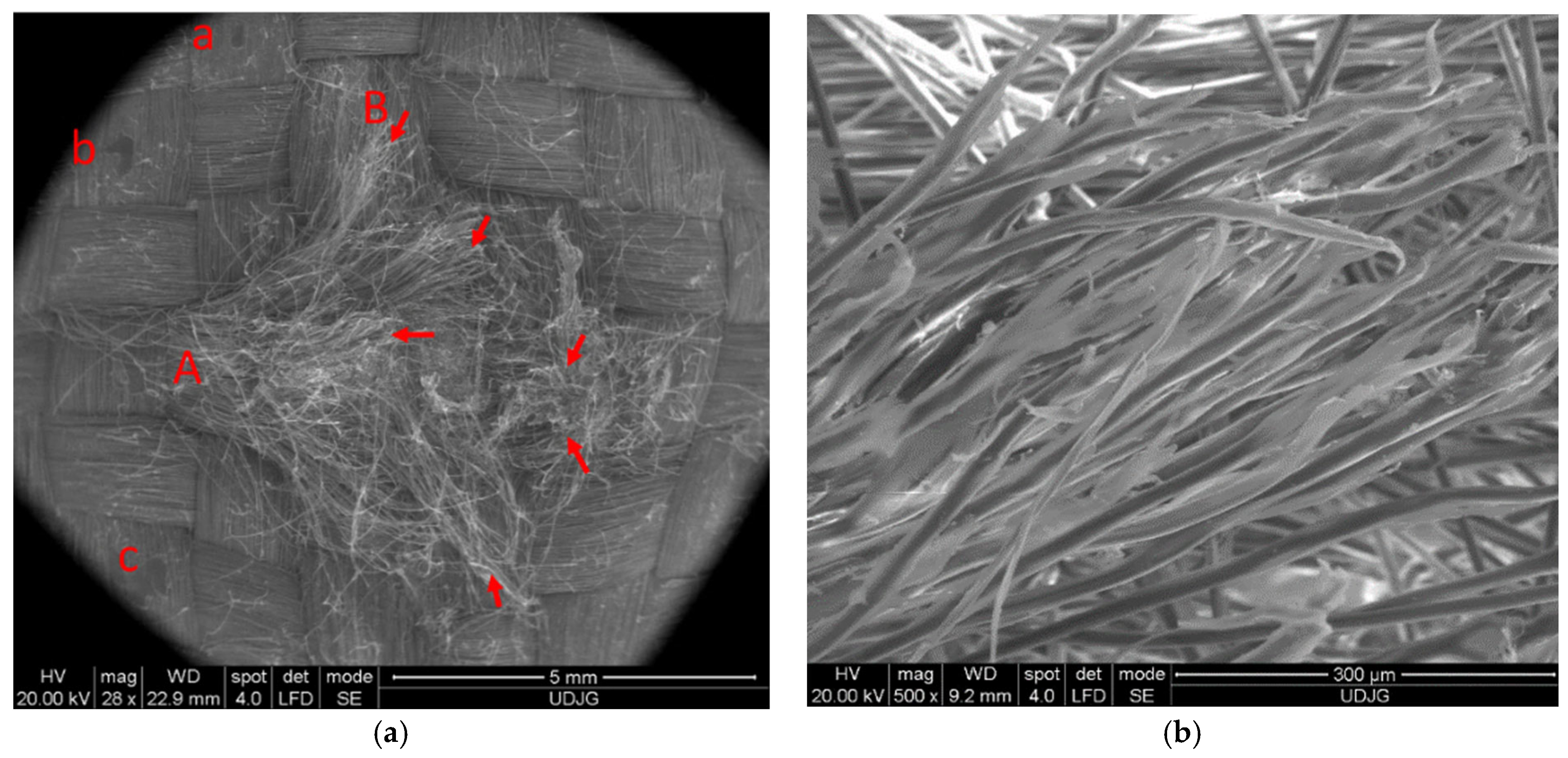

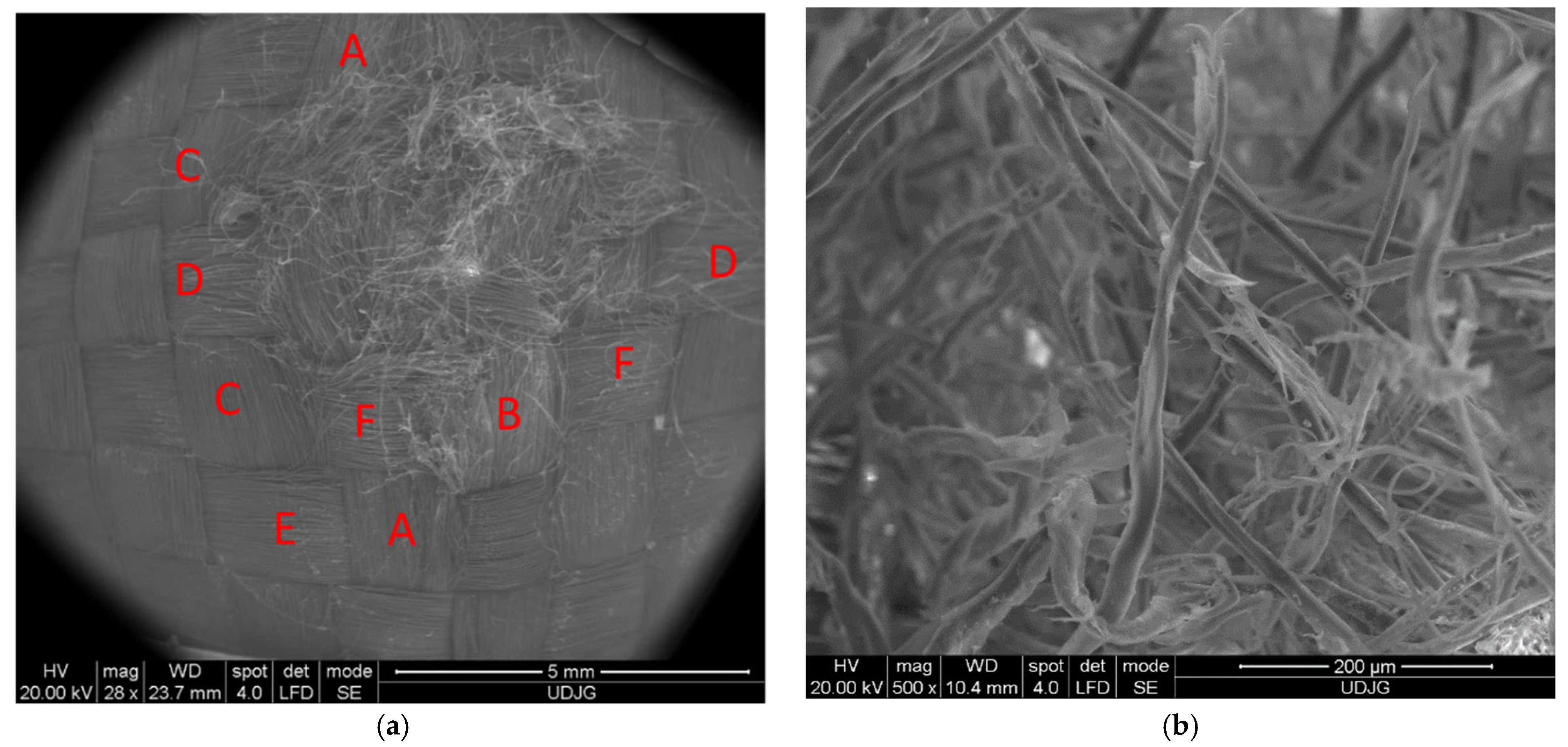
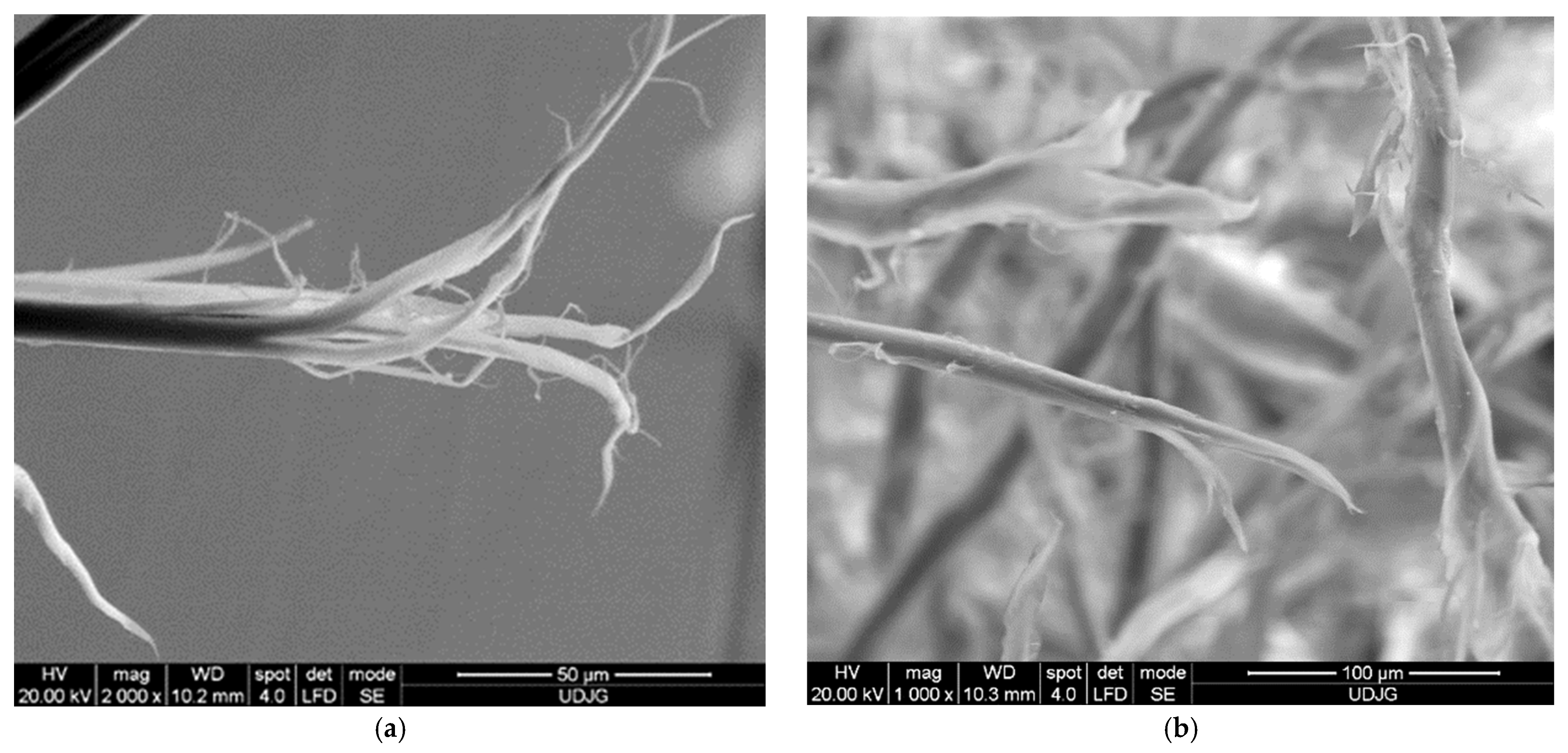







| Linear Density [dtexnom] | Twaron-Type | Set [per 10 cm] | Areal Density [g/m2] | Thickness [mm] | Minimum Breaking Strength [N/5 cm × 1000] | ||
|---|---|---|---|---|---|---|---|
| Warp | Weft | Warp | Weft | ||||
| 1680f1000 | 2000 | 127 | 127 | 410 | 0.62 | 15.5 | 16.60 |
| Panel | Order and Number of Layers of Material | Weight of Material Layers [g] | Total Weight [g] |
|---|---|---|---|
| 32 layers of Twaron CT736 fabric | 16 layers Twaron CT736 | 1202 (±1) | 2421 |
| 16 layers Twaron CT736 | 1219 (±1) |
| Property | Value | Unit |
|---|---|---|
| Density | 1440 | Kg/m3 |
| Isotropic Elasticity | ||
| Young’s Modulus | 91000 | MPa |
| Poisson’s Ratio | 0.35 | - |
| Bulk Modulus | 1.0111 × 1011 | Pa |
| Shear Modulus | 3.3704 × 1010 | Pa |
| Bilinear Isotropic Hardening | ||
| Yield Strength | 3000 | MPa |
| Tangent Modulus | 19,000 | MPa |
| Plastic Strain Failure | ||
| Equivalent Plastic Strain EPS | 0.031 | - |
| Property | Value | Unit |
|---|---|---|
| Density | 8300 | Kg/m3 |
| Isotropic Elasticity | ||
| Young’s Modulus | 1.17 × 105 | MPa |
| Poisson’s Ratio | 0.34 | - |
| Bulk Modulus | 1.2188 × 1011 | Pa |
| Shear Modulus | 4.3657 × 1010 | Pa |
| Bilinear Isotropic Hardening | ||
| Yield Strength | 70 | MPa |
| Tangent Modulus | 1150 | MPa |
| Plastic Strain Failure | ||
| Maximum Equivalent Plastic Strain EPS | 1 | - |
| Property | Value | Unit |
|---|---|---|
| Density | 11,340 | Kg/m3 |
| Isotropic Elasticity | ||
| Young’s Modulus | 16,000 | MPa |
| Poisson’s Ratio | 0.44 | - |
| Bulk Modulus | 4.4444 × 1011 | Pa |
| Shear Modulus | 5.5556 × 109 | Pa |
| Johnson–Cook Strength | ||
| Initial Yield Strength | 24 | MPa |
| Hardening Constant | 300 | MPa |
| Hardening Exponent | 1 | - |
| Strain Rate Constant | 0.1 | - |
| Thermal Softening Exponent | 1 | - |
| Melting Temperature | 760 | K |
| Reference Strain Failure | 1 | (/s) |
| Plastic Strain Failure | ||
| Maximum Equivalent Plastic Strain EPS | 1 | - |
| Sample | Depth of Indentation/Back Face Signature—Measurement with a Screwdriver BFS [mm] | Depth of Identification/Back Face Signature—3D Scanning BFS(3D) [mm] | ΔBFS = BFS-BFS(3D) [mm] | ΔBFS% = (BFS-BFS(3D)) × 100/BFS [%] |
|---|---|---|---|---|
| Fire A | 17 (±0.1) | 16.000 (±0.1) | 1.000 | 5.88 |
| Fire B | 16 (±0.1) | 14.060 (±0.1) | 1.940 | 12.13 |
| Fire C | 14 (±0.1) | 13.000 (±0.1) | 1.000 | 7.14 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chiper Titire, L.; Muntenita, C. Experimental and Numerical Analysis of the Damage Mechanism of an Aramid Fabric Panel Engaged in a Medium-Velocity Impact. Polymers 2024, 16, 1920. https://doi.org/10.3390/polym16131920
Chiper Titire L, Muntenita C. Experimental and Numerical Analysis of the Damage Mechanism of an Aramid Fabric Panel Engaged in a Medium-Velocity Impact. Polymers. 2024; 16(13):1920. https://doi.org/10.3390/polym16131920
Chicago/Turabian StyleChiper Titire, Larisa, and Cristian Muntenita. 2024. "Experimental and Numerical Analysis of the Damage Mechanism of an Aramid Fabric Panel Engaged in a Medium-Velocity Impact" Polymers 16, no. 13: 1920. https://doi.org/10.3390/polym16131920
APA StyleChiper Titire, L., & Muntenita, C. (2024). Experimental and Numerical Analysis of the Damage Mechanism of an Aramid Fabric Panel Engaged in a Medium-Velocity Impact. Polymers, 16(13), 1920. https://doi.org/10.3390/polym16131920






