A Global Approach to Modeling Injection Molding
Abstract
1. Introduction
2. Research Program
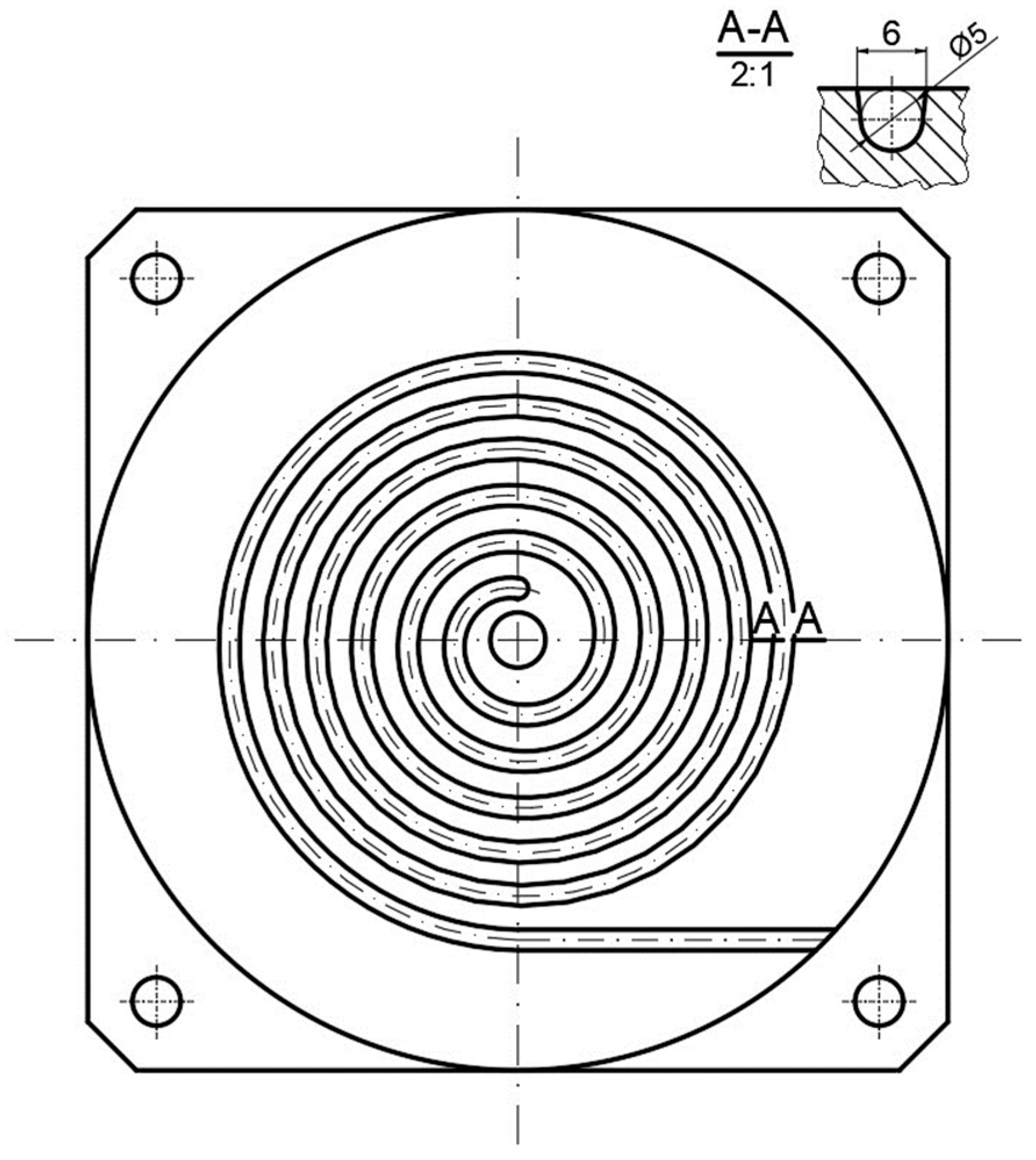
2.1. Experiment
2.2. Process Simulations
2.3. Material and Process Data
3. Results
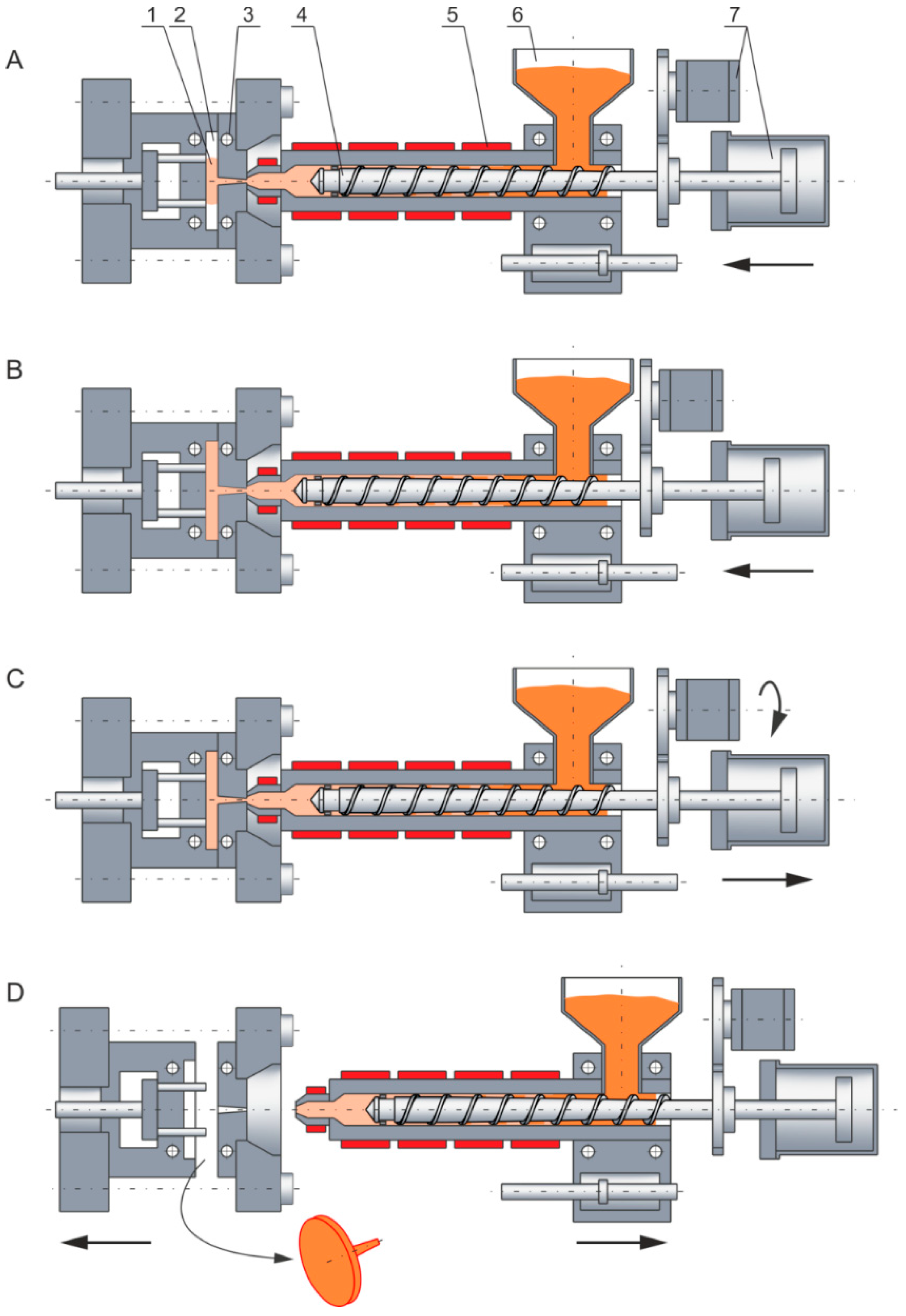
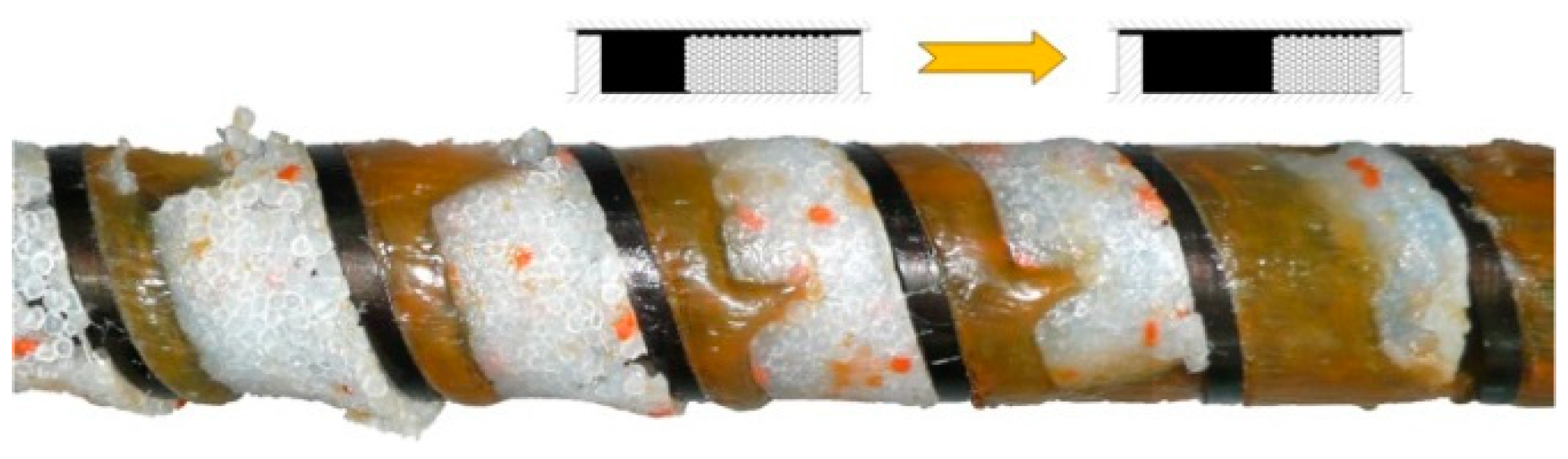
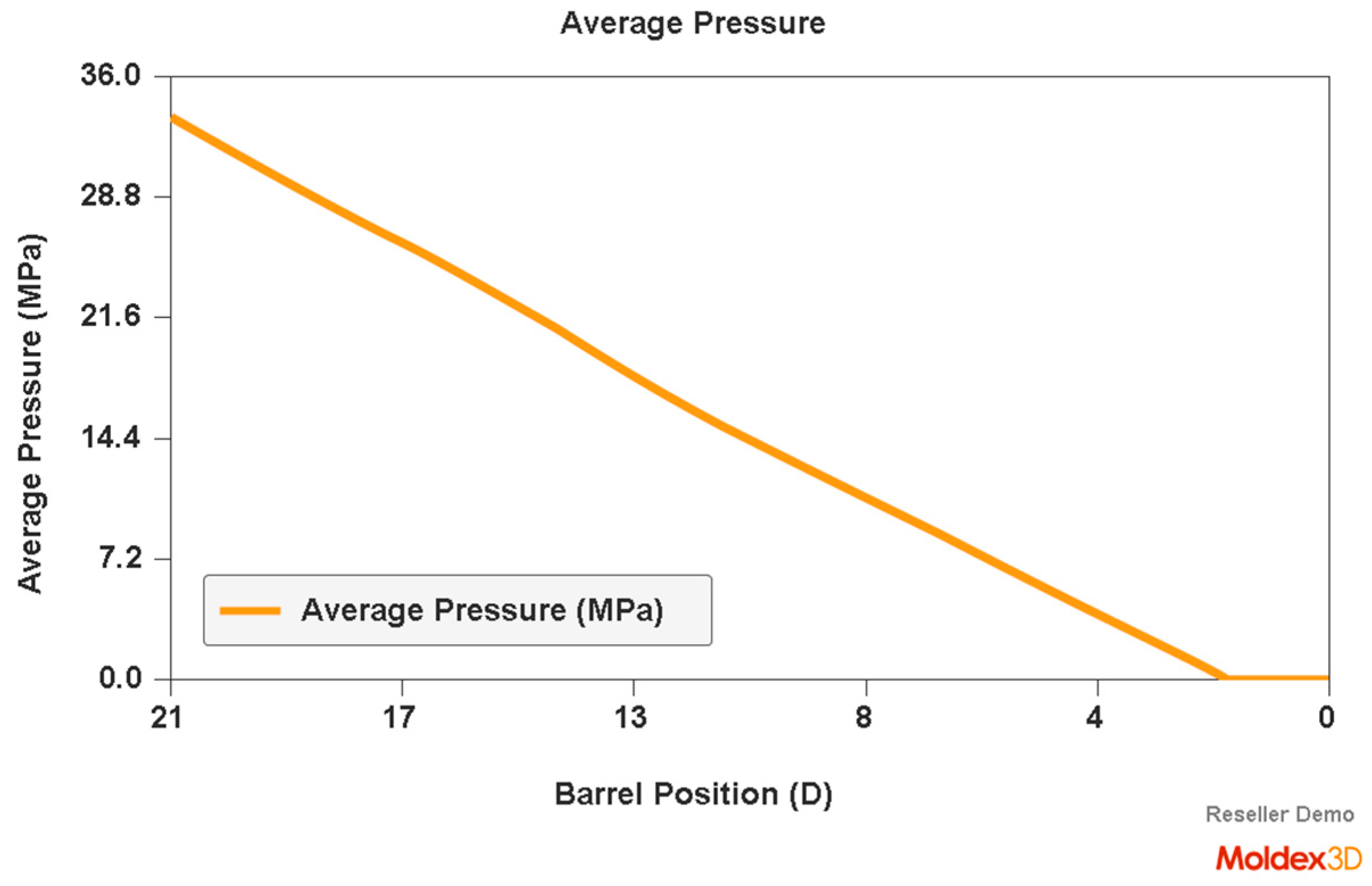
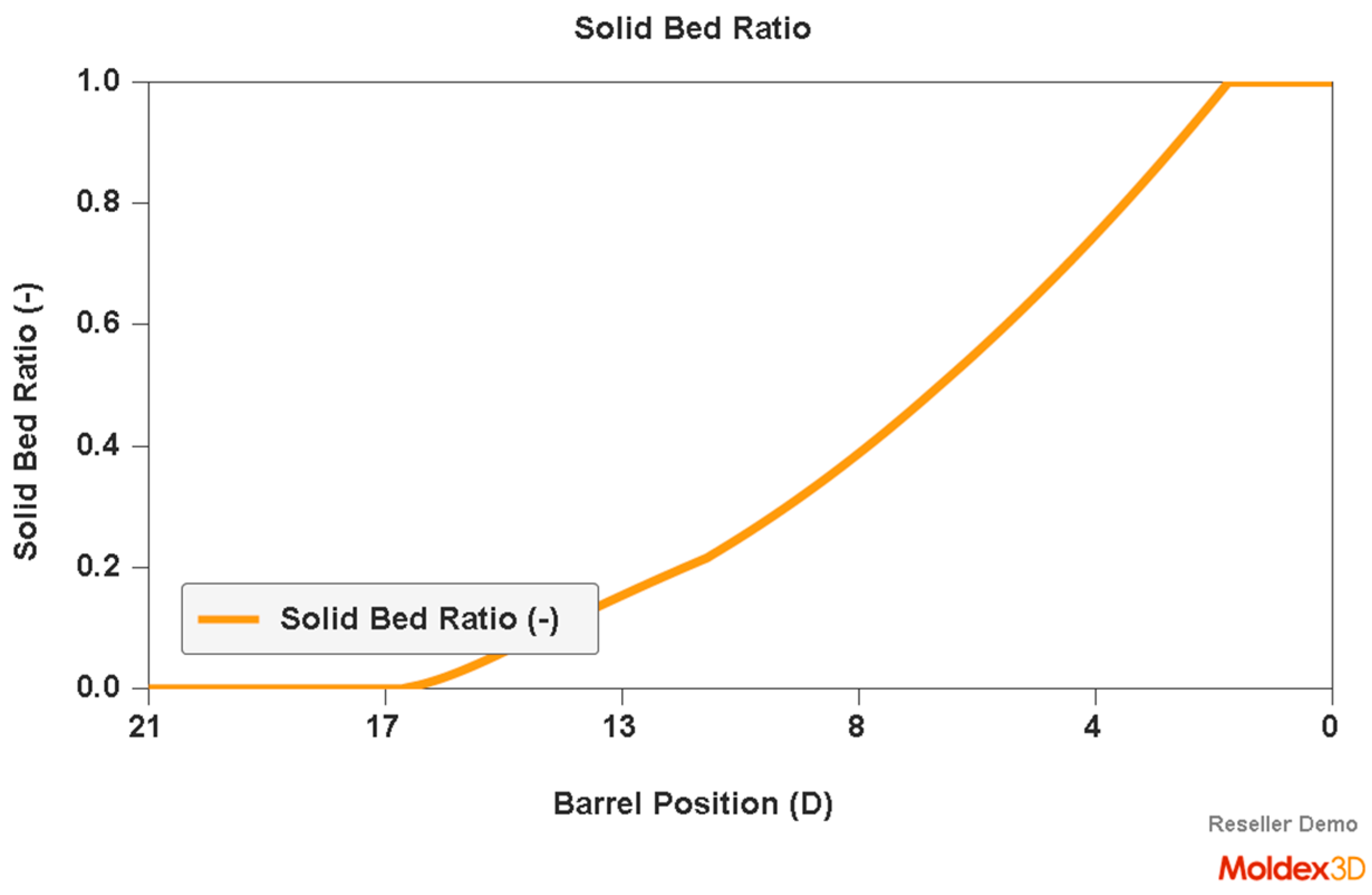
3.1. Plastic Flow in the Plasticizing System
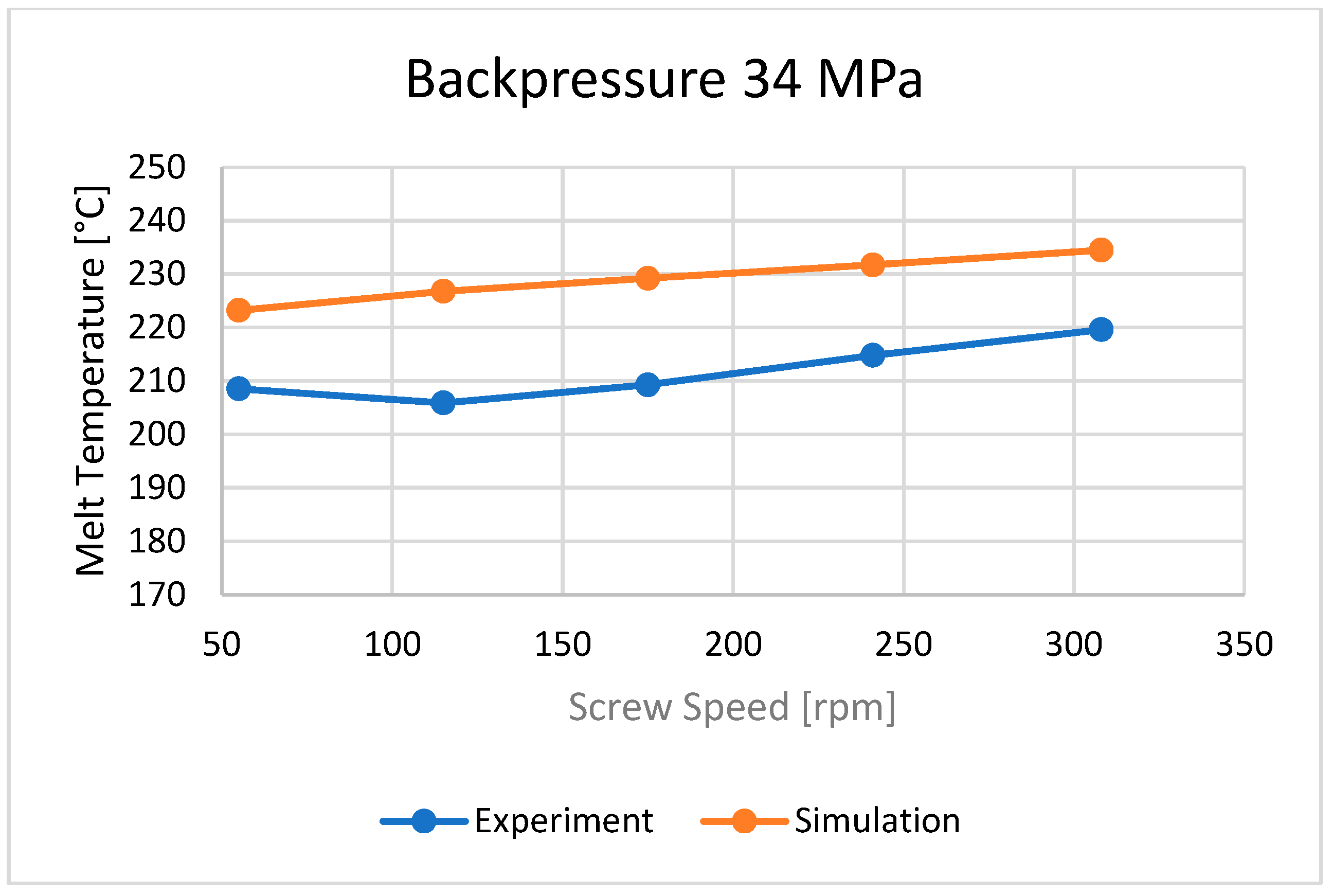
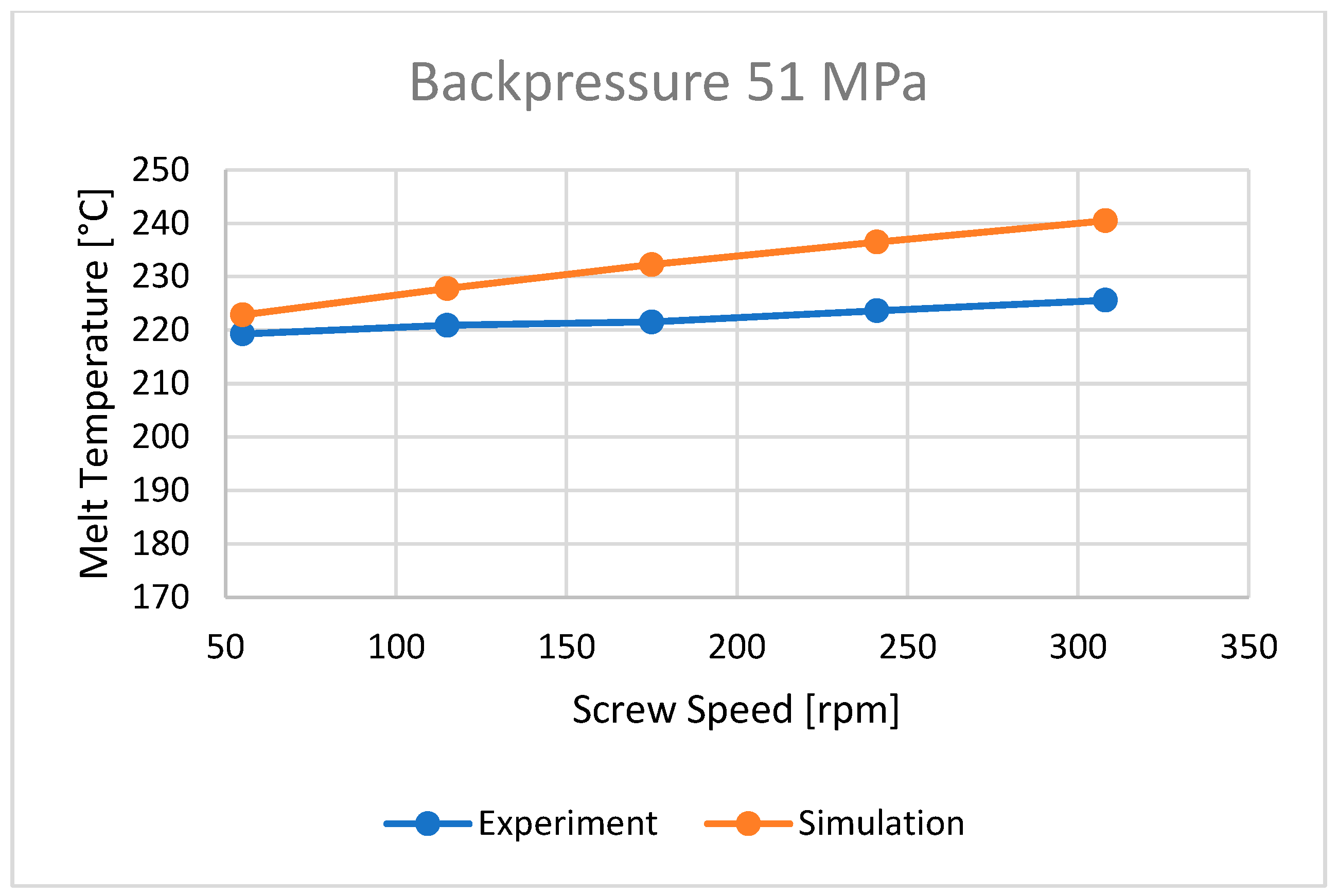
3.2. Plastic Melt Temperature
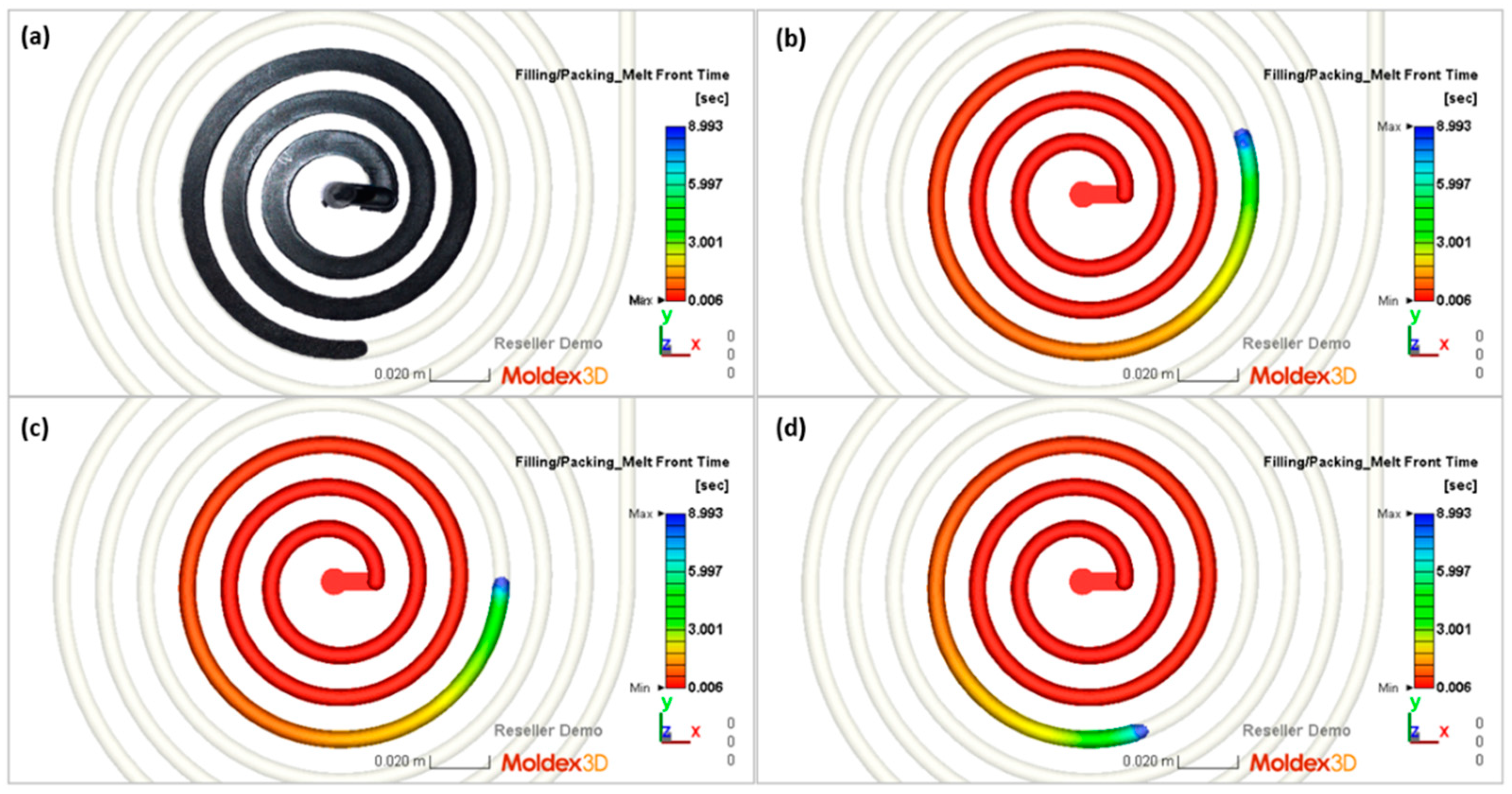
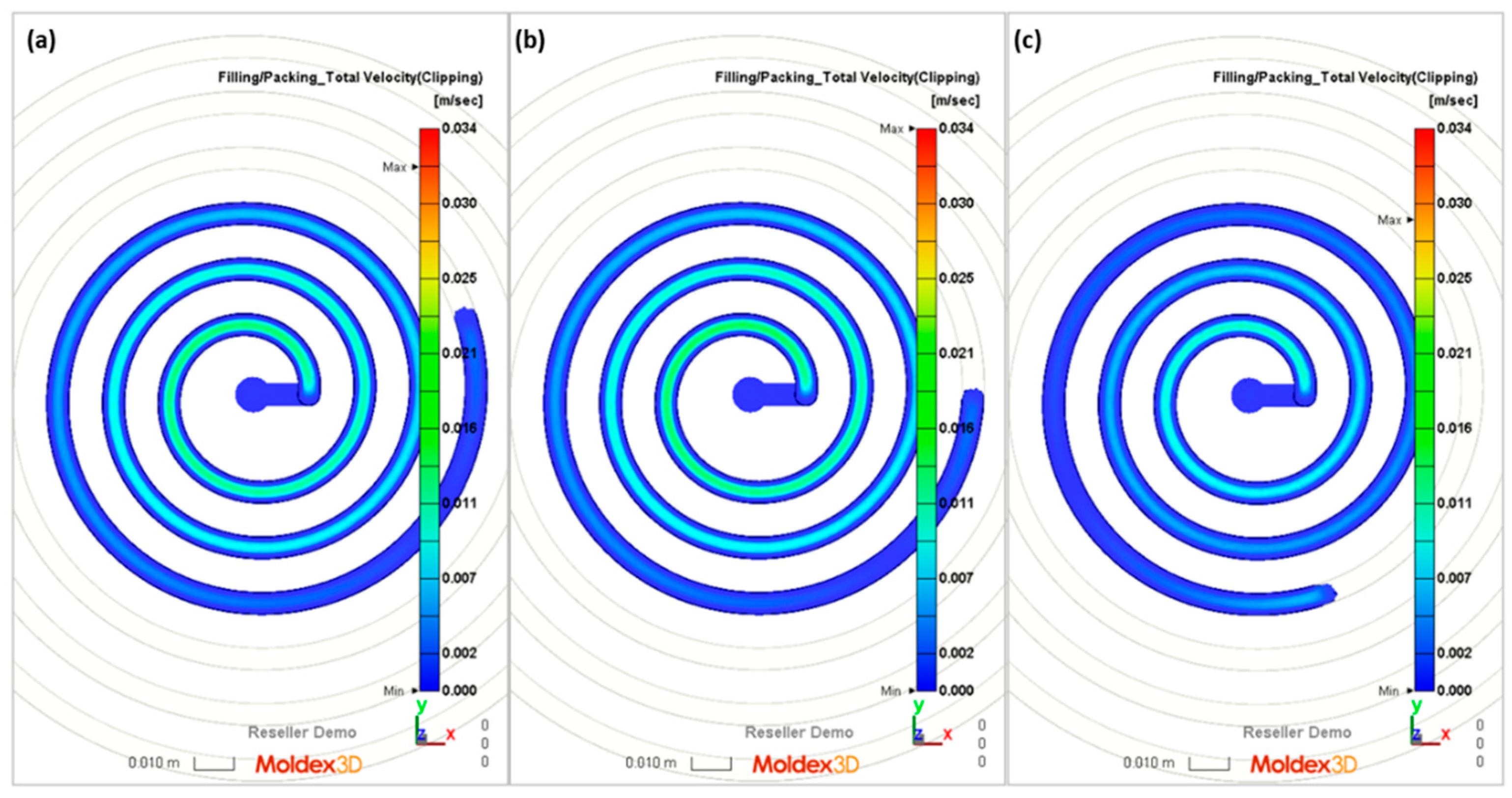
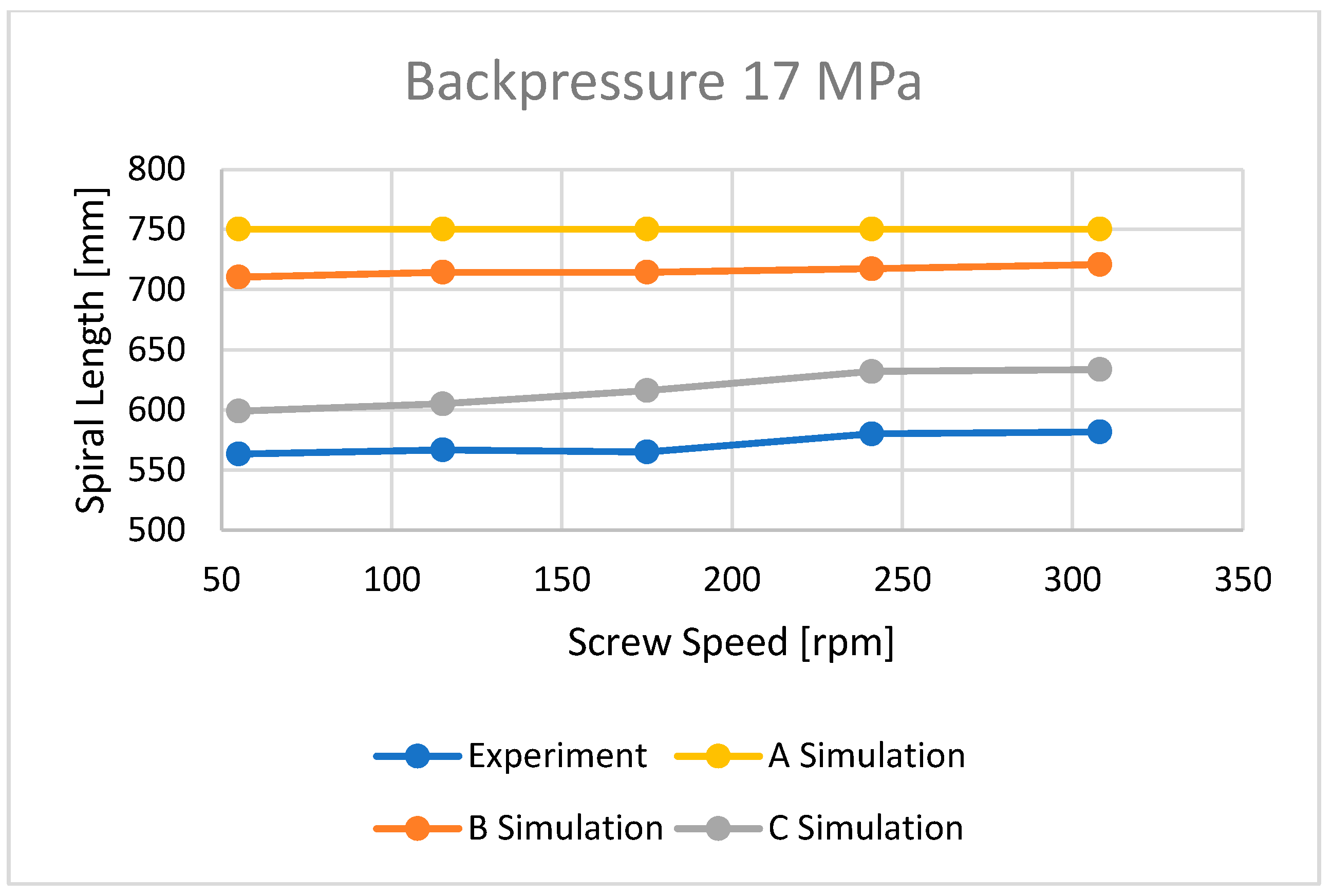
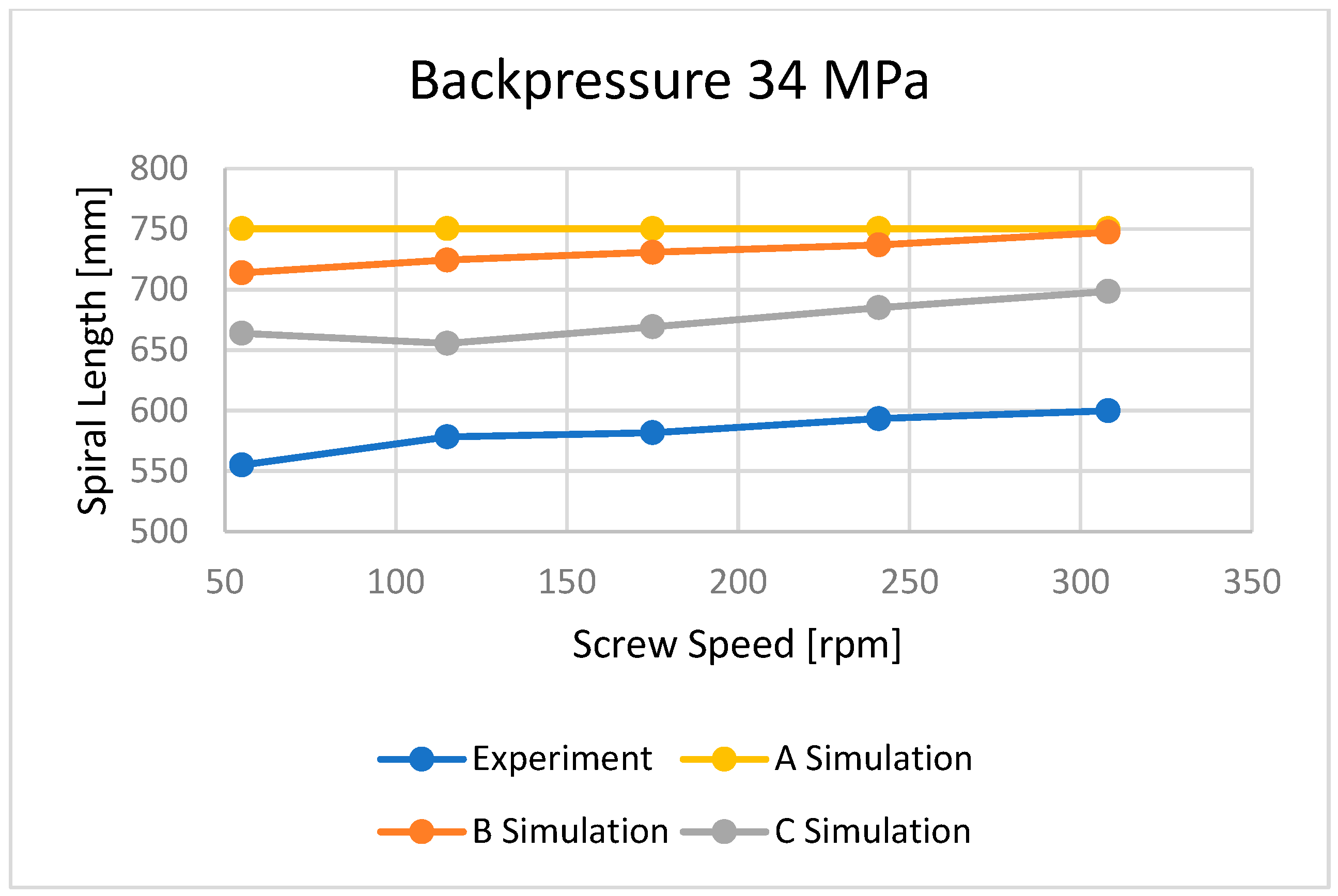
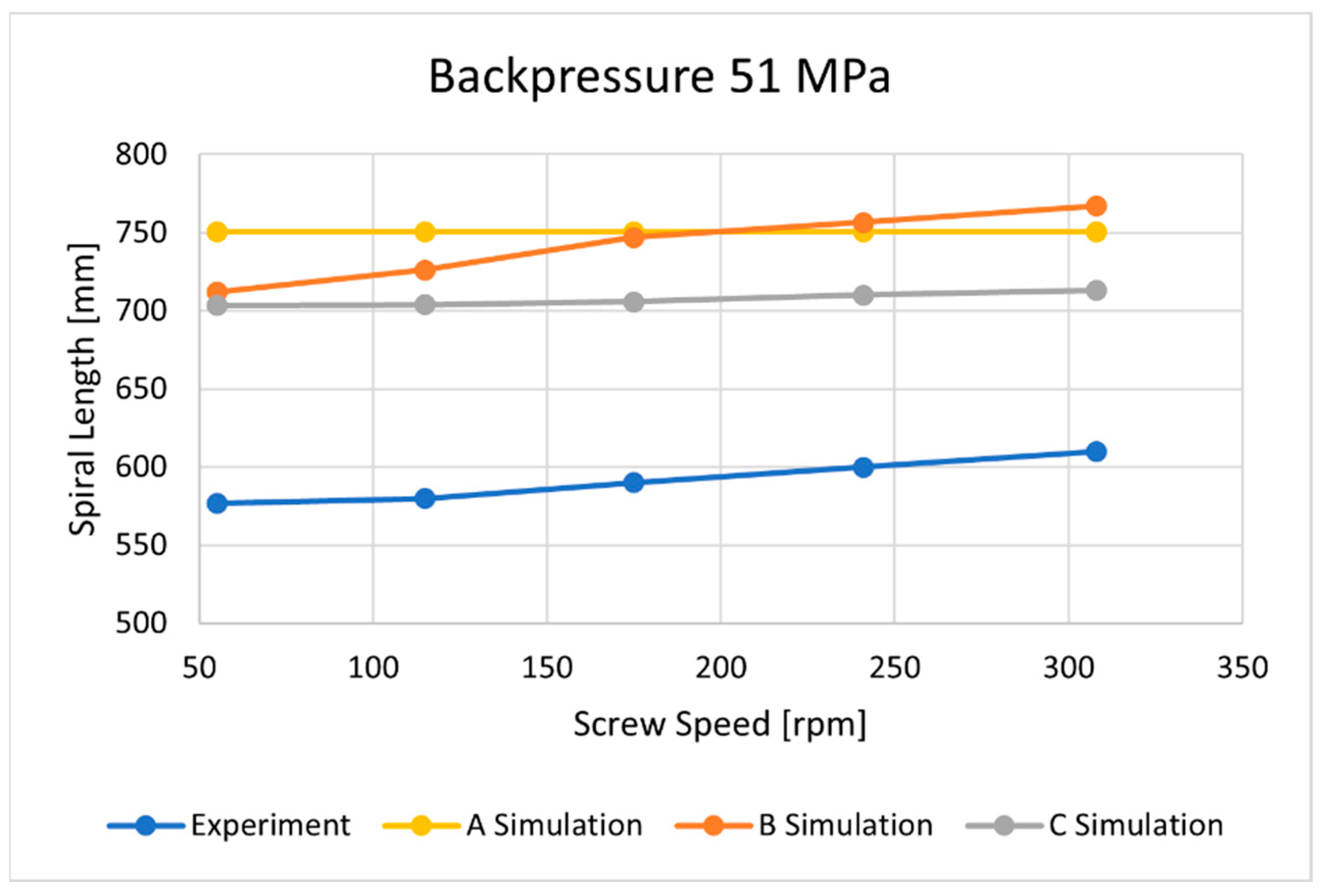
3.3. Flow Length
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wilczyński, K. Rheology in Polymer Processing: Modeling and Simulation; Carl Hanser Verlag: Munich, Germany, 2021; ISBN 978-1-56990-660-6. [Google Scholar]
- Wilczyński, K.; Nastaj, A.; Lewandowski, A.; Wilczyński, K.J.; Buziak, K. Fundamentals of Global Modeling for Polymer Extrusion. Polymers 2019, 11, 2106. [Google Scholar] [CrossRef] [PubMed]
- Carley, J.F.; Mallouk, R.S.; McKelvey, J.M. Simplified Flow Theory for Screw Extruders. Ind. Eng. Chem. 1953, 45, 974–978. [Google Scholar] [CrossRef]
- Carley, J.F.; Strub, R.A. Basic Concepts of Extrusion. Ind. Eng. Chem. 1953, 45, 970–974. [Google Scholar] [CrossRef]
- Darnell, W.H.; Mol, E.A.J. Solids Conveying in Extruders. SPE J. 1956, 20, 20–29. [Google Scholar]
- Maddock, B.H. A Visual Analysis of Flow and Mixing in Extruder Screws. SPE J. 1959, 15, 383. [Google Scholar]
- Street, L.F. Plastifying Extrusion. Intern. Plast. Eng. 1961, 1, 289–296. [Google Scholar]
- Tadmor, Z. Fundamentals of Plasticating Extrusion. I. A Theoretical Model for Melting. Polym. Eng. Sci. 1966, 6, 185–190. [Google Scholar] [CrossRef]
- Marshall, D.I.; Klein, I. Fundamentals of Plasticating Extrusion. II. Experiments. Polym. Eng. Sci. 1966, 6, 191–197. [Google Scholar] [CrossRef]
- Tadmor, Z.; Duvdevani, I.J.; Klein, I. Melting in Plasticating Extruders Theory and Experiments. Polym. Eng. Sci. 1967, 7, 198–217. [Google Scholar] [CrossRef]
- Tadmor, Z.; Klein, I. Computer Programs for Plastic Engineers; Reinhold Book Corporation: New York, NY, USA, 1968. [Google Scholar]
- Wilczyński, K.; Lewandowski, A.; Wilczyński, K.J. Experimental Study for Starve-Fed Single Screw Extrusion of Thermoplastics. Polym. Eng. Sci. 2012, 52, 1258–1270. [Google Scholar] [CrossRef]
- Lopez-Latorre, L.; McKelvey, J.M. Melting and Pressurization in Starve Feed Extrusion. Adv. Polym. Technol. 1984, 3, 355–364. [Google Scholar] [CrossRef]
- Isherwood, D.P.; Pieris, R.N.; Kassatly, J. The Effect of Metered Starve Feeding on the Performance of a Small Extruder. J. Eng. Ind. 1984, 106, 132–136. [Google Scholar] [CrossRef]
- Thompson, M.R.; Donoian, G.; Christiano, J.P. Melting Mechanism of a Starved-Fed Single-Screw Extruder for Calcium Carbonate Filled Polyethylene. Polym. Eng. Sci. 2000, 40, 2014–2026. [Google Scholar] [CrossRef]
- Wilczyński, K.; Nastaj, A.; Wilczyński, K.J. Melting Model for Starve Fed Single Screw Extrusion of Thermoplastics. Int. Polym. Proc. 2013, 28, 34–42. [Google Scholar] [CrossRef]
- Wilczyński, K.J.; Lewandowski, A.; Wilczyński, K. Experimental Study of Melting of Polymer Blends in a Starve Fed Single Screw Extruder. Polym. Eng. Sci. 2016, 56, 1349–1356. [Google Scholar] [CrossRef]
- Wilczyński, K.J.; Nastaj, A.; Lewandowski, A.; Wilczyński, K. A Composite Model for Starve Fed Single Screw Extrusion of Thermoplastics. Polym. Eng. Sci. 2014, 54, 2362–2374. [Google Scholar] [CrossRef]
- Wilczyński, K.J.; Lewandowski, A.; Nastaj, A.; Wilczyński, K. A Global Model for Starve-Fed Nonconventional Single-Screw Extrusion of Thermoplastics. Adv. Polym. Technol. 2017, 36, 23–35. [Google Scholar] [CrossRef]
- Wilczyński, K.J.; Nastaj, A.; Wilczyński, K. A Computer Model for Starve-Fed Single-Screw Extrusion of Polymer Blends. Adv. Polym. Technol. 2018, 37, 2142–2151. [Google Scholar] [CrossRef]
- Lewandowski, A.; Wilczyński, K. Modeling of Twin Screw Extrusion of Polymeric Materials. Polymers 2022, 14, 274. [Google Scholar] [CrossRef]
- Vergnes, B. Melting mechanisms in corotating twin-screw extrusion: A critical review. Int. Polym. Proc. 2023. [Google Scholar] [CrossRef]
- Manzione, L.T. (Ed.) Applications of Computer Aided Engineering in Injection Molding; Carl Hanser Verlag: Munich, Germany, 1992; ISBN 978-0-195-20752-1. [Google Scholar]
- Isayev, A.I.; Liu, S.J.; Kamal, M.R. Injection Molding: Technology and Fundamentals; Carl Hanser Verlag: Munich, Germany, 2009; ISBN 978-3-446-41685-7. [Google Scholar]
- Osswald, T.; Turng, L.S.; Gramman, P. Injection Molding Handbook; Carl Hanser Verlag: Munich, Germany, 2009; ISBN 978-3-446-40781-7. [Google Scholar]
- Kennedy, P.; Zheng, R. Flow Analysis of Injection Molds, 2nd ed.; Carl Hanser Verlag: Munich, Germany, 2013; ISBN 978-1-569-90512-8. [Google Scholar]
- Wang, M.; Chang, R.; Hsu, C. Molding Simulation: Theory and Practice; Carl Hanser Verlag: Munich, Germany, 2018; ISBN 978-1-569-90619-4. [Google Scholar]
- Menges, G.; Michaeli, W.; Mohren, P. How to Make Injection Molds, 3rd ed.; Carl Hanser Verlag: Munich, Germany, 2001; ISBN 978-1-569-90282-0. [Google Scholar]
- Rees, H. Mold Engineering, 2nd ed.; Carl Hanser Verlag: Munich, Germany, 2002; ISBN 978-1-569-90322-3. [Google Scholar]
- Unger, P. Gastrow Injection Molds, 4th ed.; Carl Hanser Verlag: Munich, Germany, 2006; ISBN 978-1-569-90402-2. [Google Scholar]
- Mennig, G.; Stoeckhert, K. Mold-Making Handbook, 3rd ed.; Carl Hanser Verlag: Munich, Germany, 2006; ISBN 978-1-569-90446-6. [Google Scholar]
- Turng, L.; Chen, S. Advanced Injection Molding Technologies; Carl Hanser Verlag: Munich, Germany, 2019; ISBN 978-1-569-90603-3. [Google Scholar]
- Beaumont, J. Runner and Gating Design Handbook, 3rd ed.; Carl Hanser Verlag: Munich, Germany, 2020; ISBN 978-1-569-90590-6. [Google Scholar]
- Catoen, B.; Rees, H. Injection Mold Design Handbook; Carl Hanser Verlag: Munich, Germany, 2021; ISBN 978-1-569-90815-0. [Google Scholar]
- Kazmer, D. Injection Mold Design Engineering, 3rd ed.; Carl Hanser Verlag: Munich, Germany, 2022; ISBN 978-1-569-90570-8. [Google Scholar]
- Johannaber, F. Injection Molding Machines, 4th ed.; Carl Hanser Verlag: Munich, Germany, 2007; ISBN 978-1-569-90418-3. [Google Scholar]
- Fernandes, C.; Pontes, A.J.; Viana, J.C.; Gaspar-Cunha, A. Modeling and Optimization of the Injection-Molding Process: A Review. Adv. Polym. Technol. 2016, 37, 429–449. [Google Scholar] [CrossRef]
- Moldflow. Plastic Injection and Compression Mold Simulation. Available online: www.autodesk.com/products/moldflow (accessed on 17 November 2023).
- Moldex3D. Plastic Injection Molding Simulation Software. Available online: www.moldex3d.com (accessed on 17 November 2023).
- CADMOULD. Plastic Injection Molding Simulation. Available online: www.simcon.com/cadmould (accessed on 17 November 2023).
- Wilczyński, K.; Narowski, P. Simulation Studies on the Effect of Material Characteristics and Runners Layout Geometry on the Filling Imbalance in Geometrically Balanced Injection Molds. Polymers 2019, 11, 639. [Google Scholar] [CrossRef] [PubMed]
- Wilczyński, K.; Narowski, P. A Strategy for Problem Solving of Filling Imbalance in Geometrically Balanced Injection Molds. Polymers 2020, 12, 805. [Google Scholar] [CrossRef] [PubMed]
- COMSOL. Multiphysics Simulation Software. Available online: www.comsol.com/comsol-multiphysics (accessed on 9 May 2022).
- Sullivan, A.; Saigal, A.; Zimmerman, M.A. Practical Simulation and Experimental Measurement of Liquid Crystal Polymer Directionality During Injection Molding. Polym. Eng. Sci. 2019, 59, E414–E424. [Google Scholar] [CrossRef]
- Wilczyński, K.; Wilczyński, K.J.; Buziak, K. Modeling and Experimental Studies on Polymer Melting and Flow in Injection Molding. Polymers 2022, 14, 2106. [Google Scholar] [CrossRef] [PubMed]
- Donovan, R.C.; Thomas, D.E.; Leversen, L.D. An Experimental Study of Plasticating in a Reciprocating-Screw Injection Molding Machine. Polym. Eng. Sci. 1971, 11, 353–360. [Google Scholar] [CrossRef]
- Gao, F.; Jin, Z.; Chen, X. A Visual Barrel System for Study of Reciprocating Screw Injection Molding. Polym. Eng. Sci. 2000, 40, 1334–1343. [Google Scholar] [CrossRef]
- Jin, Z.; Gao, F.; Zhu, F. An Experimental Study of Solid-Bed Break-up in Plasticization of a Reciprocating-Screw Injection Molding. Polym. Eng. Sci. 2004, 44, 1313–1318. [Google Scholar] [CrossRef]
- Pham, T.L.; Balcaen, J.; Charmeau, J.Y.; Bereaux, Y. In-Line Visualisation of Polymer Plastication in an Injection Molding Screw. Key Eng. Mater. 2013, 554, 1683–1691. [Google Scholar] [CrossRef]
- Donovan, R.C. A Theoretical Melting Model for a Reciprocating-Screw Injection Molding Machine. Polym. Eng. Sci. 1971, 11, 361–368. [Google Scholar] [CrossRef]
- Donovan, R.C. The Plasticating Process in Injection Molding. Polym. Eng. Sci. 1974, 14, 101–111. [Google Scholar] [CrossRef]
- Donovan, R.C. A Theoretical Melting Model for Plasticating Extruders. Polym. Eng. Sci. 1971, 11, 247–257. [Google Scholar] [CrossRef]
- Lipshitz, S.D.; Lavie, R.; Tadmor, Z. A Melting Model for Reciprocating Screw Injection-Molding Machines. Polym. Eng. Sci. 1974, 14, 553–559. [Google Scholar] [CrossRef]
- Rauwendaal, C. Melt Conveying in Reciprocating extruders. SPE-ANTEC Technol. Pap. 1991, 36, 433–435. [Google Scholar]
- Rauwendaal, C. Conveying and Melting in Screw Extruders with Axial Movement. Int. Polym. Process. 1992, 7, 26–31. [Google Scholar] [CrossRef]
- Dormeier, S.; Panreck, K. Dynamic behavior of temperature during extrusion. Kunststoffe 1990, 11, 32–33. [Google Scholar]
- Rao, N.S.; Schumacher, G.; Schott, N.R.; Edwards, R. Calculations of Melting Performance of Injection Molding Screws by an Easily Applicable Model. SPE-ANTEC Technol. Pap. 2001, 46, 5. [Google Scholar]
- Potente, H.; Schulte, H.; Effen, N. Simulation of Injection Molding and Comparison with Experimental Values. Int. Polym. Process. 1993, 8, 224–235. [Google Scholar] [CrossRef]
- Potente, H.; Bornemann, M. Non-isothermal Model for the Drive Power Calculation of Single-Screw Plasticating Units. Int. Polym. Process. 2008, 23, 345–350. [Google Scholar] [CrossRef]
- Bereaux, Y.; Charmeau, J.-Y.; Moguedet, M. A simple model of throughput and pressure development for single screw. J. Mater. Process. Technol. 2009, 209, 611–618. [Google Scholar] [CrossRef]
- Yung, K.L.; Yan, X. Analysis of a melting model for an extruder with reciprocation. J. Mater. Process. Technol. 2001, 117, 21–27. [Google Scholar] [CrossRef]
- Yung, K.L.; Yan, X.; Lau, K.H. Simulation of transient process in melting section of reciprocating extruder. Polymer 2002, 3, 983–988. [Google Scholar] [CrossRef]
- Yung, K.L.; Yan, X. Transient melting models for the three stages of reciprocating extrusion. J. Mater. Process. Technol. 2003, 139, 170–177. [Google Scholar] [CrossRef]
- Steller, R.; Iwko, J. Polymer Plastication during Injection Molding. Part 1. Mathematical model. Int. Polym. Process. 2008, 23, 252–262. [Google Scholar] [CrossRef]
- Iwko, J.; Steller, R. Polymer Plastication during Injection Molding. Part 2. Simulations and Experiments. Int. Polym. Process. 2008, 23, 263–269. [Google Scholar] [CrossRef]
- Iwko, J.; Steller, R.; Wróblewski, R. Experimentally Verified Mathematical Model of Polymer Plasticization Process in Injection Molding. Polymers 2018, 10, 968. [Google Scholar] [CrossRef]
- Fernandes, C.; Pontes, A.J.; Viana, J.C.; Nóbrega, J.M.; Gaspar-Cunha, A. Modeling of Plasticating Injection Molding-Experimental Assessment. Int. Polym. Process. 2014, 29, 558–569. [Google Scholar] [CrossRef]
- Gaspar-Cunha, A. Modeling and Optimization of Single Screw Extrusion. Ph.D. Thesis, University of Minho, Braga, Portugal, 2000. [Google Scholar]
- Tadmor, Z.; Klein, I. Engineering Principles of Plasticating Extrusion; Van Nostrand Reinhold Co.: New York, NY, USA, 1970; ISBN 978-0442156350. [Google Scholar]
- Klein, I.; Klein, R.J. The SPR System of CAE Software. In Computer Modeling for Extrusion and Other Continuous Polymer Processes; O’Brien, K.T., Ed.; Hanser Publishers: New York, NY, USA, 1992; Chapter 5; pp. 103–252. ISBN 978-1569900680. [Google Scholar]
- Agur, E.E.; Vlachopoulos, J. Numerical Simulation of a Single-Screw Plasticating Extruder. Polym. Eng. Sci. 1982, 22, 1084–1094. [Google Scholar] [CrossRef]
- Potente, H.; Hanhart, W.; Schöppner, V. Potential Applications for Computer-aided Extruder Design. Int. Polym. Process. 1993, 8, 335–344. [Google Scholar] [CrossRef]
- Potente, H.; Hanhart, W.; Reski, T. Design and Processing Optimization of Extruder Screws. Polym. Eng. Sci. 1994, 34, 937–945. [Google Scholar] [CrossRef]
- Sebastian, D.H.; Rakos, R. Extrusion Process Analysis with PASS. In Computer Modeling for Extrusion and Other Continuous Polymer Processes; O’Brien, K.T., Ed.; Hanser Publishers: New York, NY, USA, 1992; Chapter 7; pp. 331–448. ISBN 978-1569900680. [Google Scholar]
- Wilczyński, K.; Buziak, K.; Wilczyński, K.J.; Lewandowski, A.; Nastaj, A. Computer Modeling for Single-Screw Extrusion of Wood-Plastic Composites. Polymers 2018, 10, 295. [Google Scholar] [CrossRef] [PubMed]
- Fukase, H.; Kunio, T.; Shinya, S.; Nomura, A. A Plasticating Model for Single-Screw Extruders. Polym. Eng. Sci. 1982, 22, 578–586. [Google Scholar] [CrossRef]
- Zawadsky, E.; Karnis, J. Mathematical Model of a Single-Screw Plasticating Extruder. J. Rheol. Acta 1985, 24, 556–565. [Google Scholar] [CrossRef]
- Vincelette, A.R.; Guerrero, C.S.; Carreau, P.J.; Lafleur, P.G. A Model for Single-Screw Plasticating Extruders. Int. Polym. Process. 1989, 4, 232–241. [Google Scholar] [CrossRef]
- Amellal, K.; Lafleur, P.G. Computer Simulation of Conventional and Barrier Screw Extruders. Plast. Rubber Compos. Process. Appl. 1993, 19, 227–239. [Google Scholar]
- PSI—Paderborner Spritzgießsimulation. Available online: https://ktp.uni-paderborn.de/foerderverein/software/psi (accessed on 17 November 2023).
- Chang, R.-Y.; Lin, K.-J. The Hybrid FEM/FDM Computer Model for Analysis of the Metering Section of a Single Screw Extruder. Polym. Eng. Sci. 1995, 35, 1748–1757. [Google Scholar] [CrossRef]
- Altınkaynak, A.; Gupta, M.; Spalding, M.A.; Crabtree, S.L. Melting in a Single Screw Extruder: Experiments and 3D Finite Element Simulations. Int. Polym. Process. 2011, 26, 182–196. [Google Scholar] [CrossRef]






| Process Parameters | |
|---|---|
| Mold temperature, Tmold | 30 °C |
| Melt temperature, Tmelt | 235 °C |
| Injection pressure, pinj | 45 MPa |
| Filling time, tfill | 9 s |
| Material Characteristics | |
| Density - Solid, ρs - Melt, ρm | 1120 kg/m3 1020 kg/m3 |
| MVR (Melt Volume Rate) | 2 cm3/10 min (2.16 kg, 230 °C) |
| Heat capacity - Melt, cpm - Solid, cps | 2320 J/(kg∙K) 1480 J/(kg∙K) |
| Thermal conductivity—melt, km | 0.240 W/(m∙K) |
| Melting temperature, Tm | 120 °C |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Narowski, P.; Wilczyński, K. A Global Approach to Modeling Injection Molding. Polymers 2024, 16, 147. https://doi.org/10.3390/polym16010147
Narowski P, Wilczyński K. A Global Approach to Modeling Injection Molding. Polymers. 2024; 16(1):147. https://doi.org/10.3390/polym16010147
Chicago/Turabian StyleNarowski, Przemysław, and Krzysztof Wilczyński. 2024. "A Global Approach to Modeling Injection Molding" Polymers 16, no. 1: 147. https://doi.org/10.3390/polym16010147
APA StyleNarowski, P., & Wilczyński, K. (2024). A Global Approach to Modeling Injection Molding. Polymers, 16(1), 147. https://doi.org/10.3390/polym16010147








