Compressive Performance of Longitudinal Steel-FRP Composite Bars in Concrete Cylinders Confined by Different Type of FRP Composites
Abstract
:1. Introduction
2. Experimental Program
2.1. Specimen Design
2.2. Material Properties
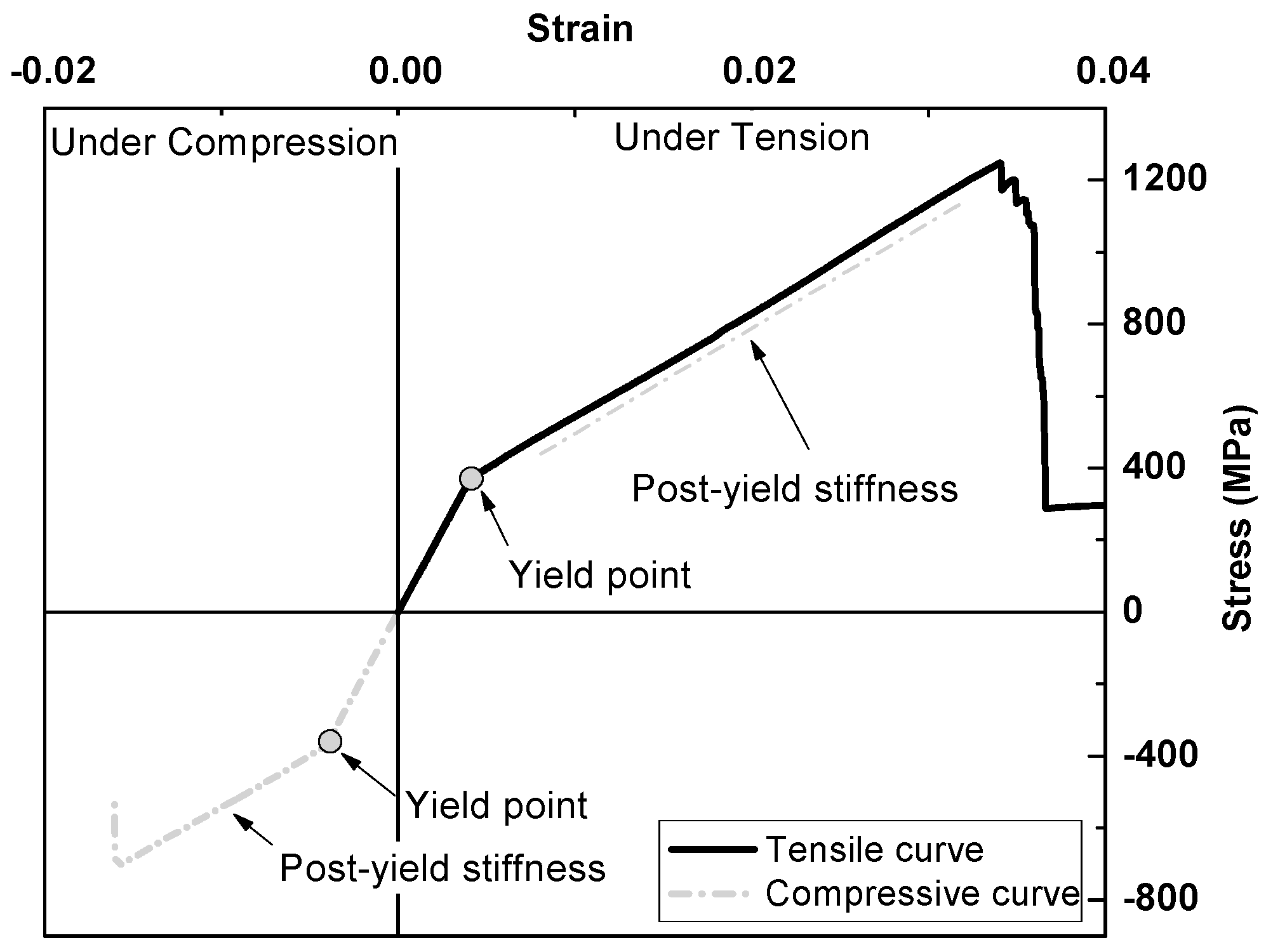
2.2.1. SFCB
2.2.2. CFRP Sheet/CFRP Strip/BFRP Spiral
2.2.3. Concrete
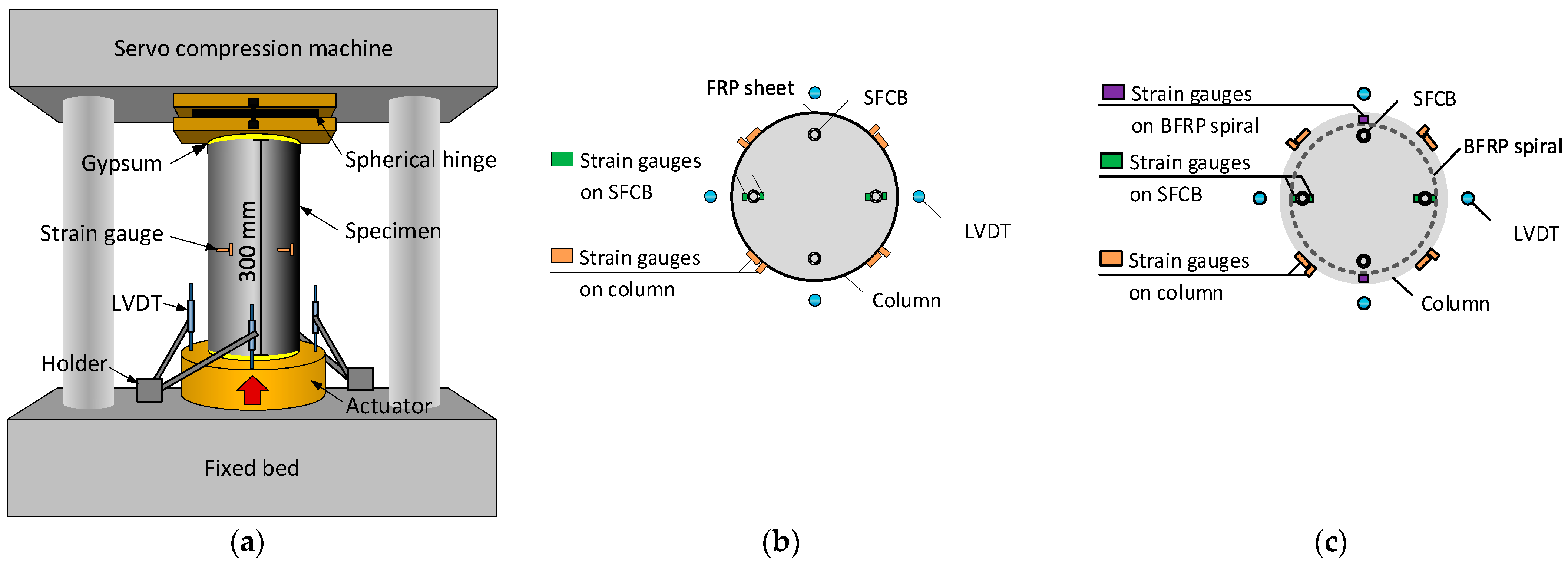
2.3. Test Instruments and Setup
3. Test Results and Discussion
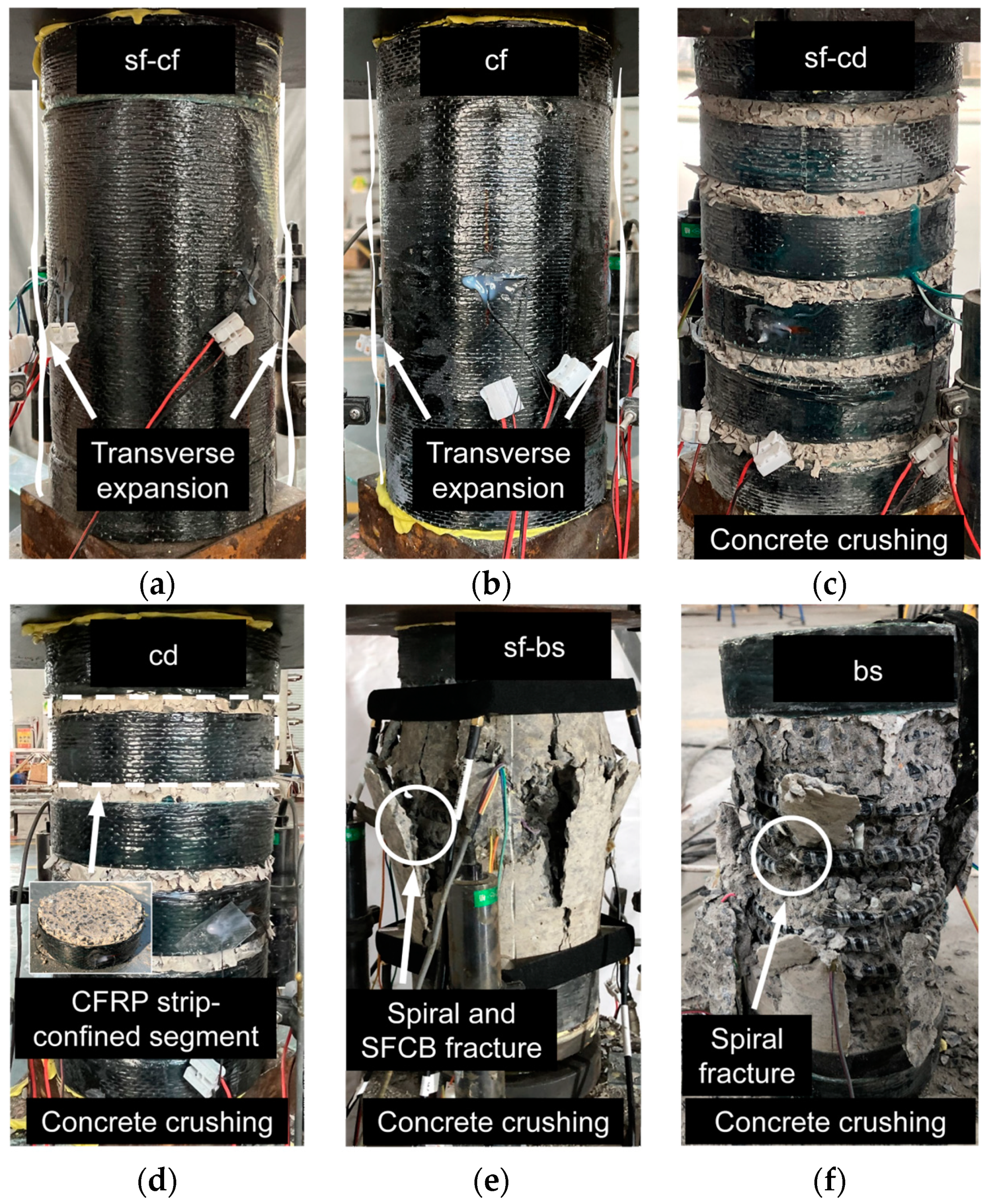
3.1. General Observation and Stress–Strain Curve of Cylinders
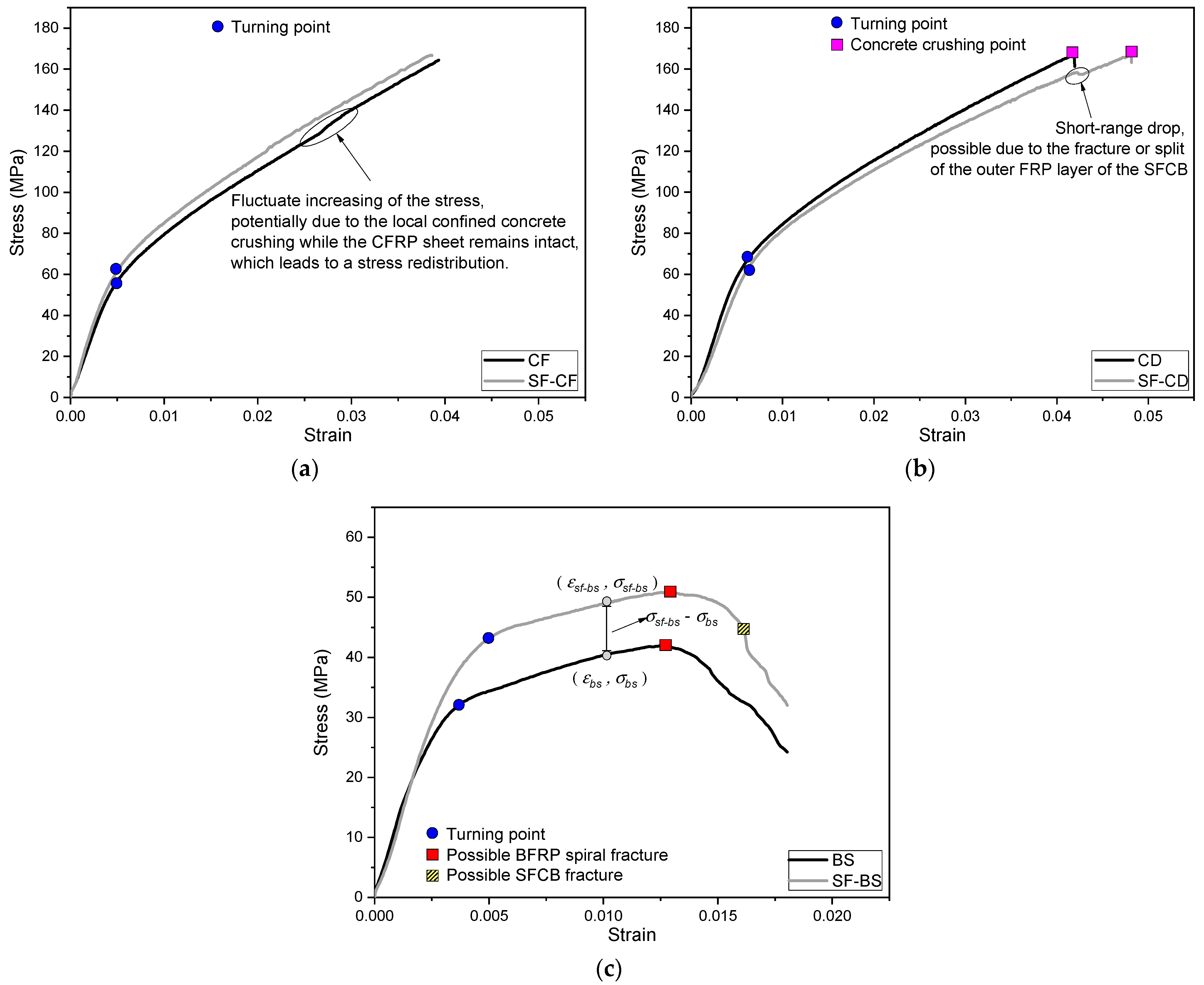
3.1.1. For SF-CF and CF Cylinders
3.1.2. For SF-CD and CD Cylinders
3.1.3. For the SF-BS and BS Cylinders
3.2. Compressive Stress–Strain Curves of SFCBs in Concrete Cylinders Confined with Different FRP Composites
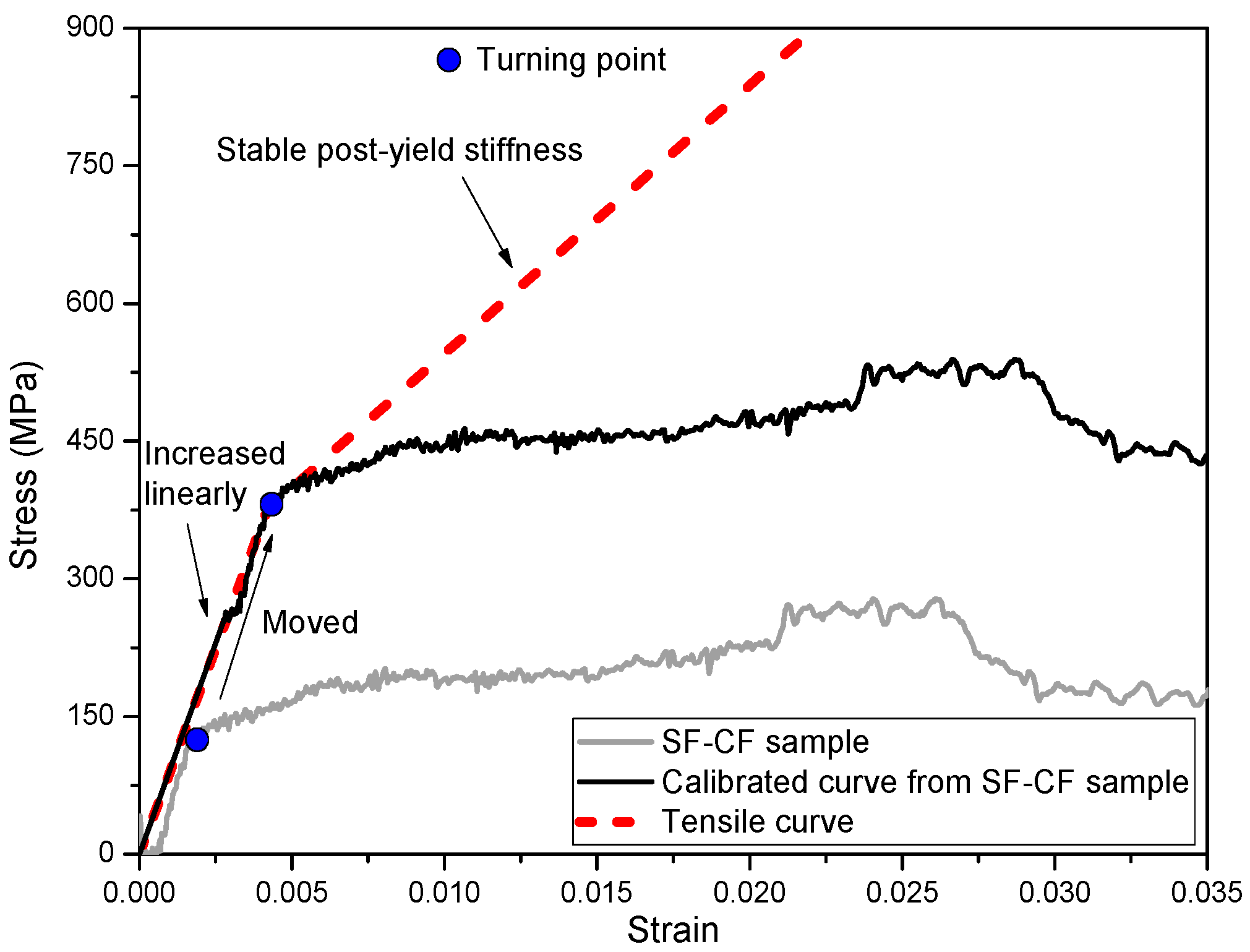
3.2.1. Calculation Method of Compressive Stress–Strain Curve of SFCB
3.2.2. Modification for the Calculated Compressive Stress–Strain Curve of SFCBs
3.2.3. Compressive Mechanism of SFCBs in Different FRP Composites-Confined Concrete Cylinders
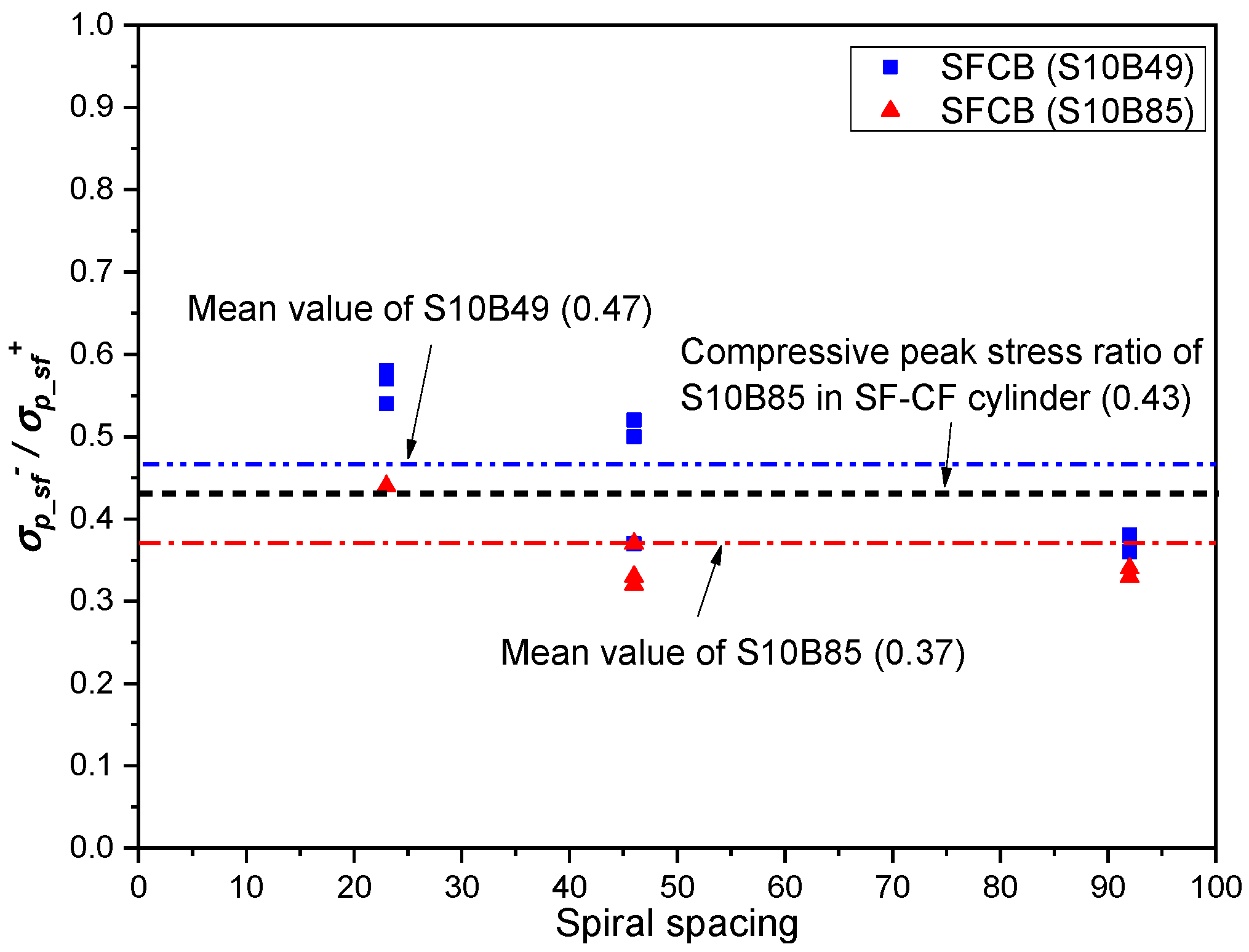
3.3. Compressive Peak Stress of SFCBs Embedded in Concrete Cylinders Confined with Different FRP Composites
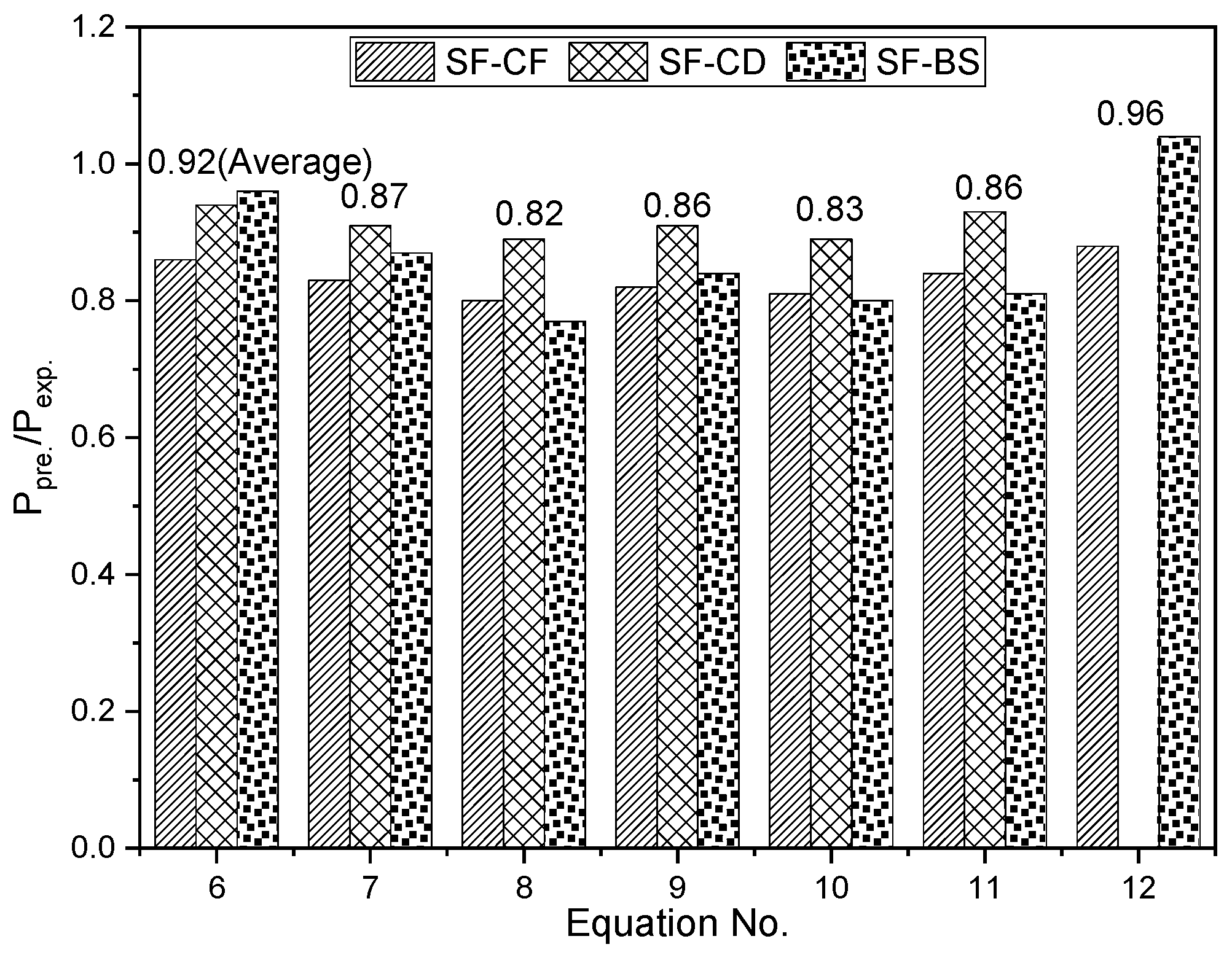
3.4. Evaluation of Design Equations for Load-Carrying Capacity of SFCB-Reinforced Concrete Cylinders
4. Conclusions
- The post-yield stiffness of the confined SFCBs developed to become relatively higher when it was confined with a relatively high elastic modulus CFRP composite than when it was confined with a relatively low elastic modulus of BFRP composite.
- The compressive failure strain of the SFCB in the SF-CF cylinder could have reached 88% of its tensile peak strain, which indicates that a relatively high utilization of the material strength may be achieved for the SFCB restrained with relatively high elastic modulus confinements.
- The design equations that consider the compressive contribution of SFCBs in concrete cylinders with a strength reduction factor of the SFCB (in Method I) or a maximum compressive strain of concrete (in Method II) generally underestimated the load-carrying capacity of SFCB-reinforced concrete cylinders. In addition, this underestimation appears more significant for the cylinder with a relatively strong confinement.
- The approaching method, which applies an actual compressive strength of the SFCB for considering its compressive contribution in concrete cylinders (in Method III), gave the most accurate prediction for the load-carrying capacity of SFCB-reinforced concrete cylinders. However, its applicability and accuracy need to be verified with more experimental data in the future.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- ASCE. Minimum Design Loads for Buildings and Other Structures; American Society of Civil Engineers: Reston, VA, USA, 2010. [Google Scholar]
- LRFDSEIS-2; Guide Specifications for LRFD Seismic Bridge Design. AASHTO: Washington, DC, USA, 2011.
- Calvi, G.; Priestley, M.; Kowalsky, M. Displacement based seismic design of structures. In Proceedings of the New Zealand Conference on Earthquake Engineering, Singapore, 5–7 December 2007; p. 740. [Google Scholar]
- Priestley, M.N.; Seible, F.; Calvi, G.M. Seismic Design and Retrofit of Bridges; John Wiley & Sons: Hoboken, NJ, USA, 1996. [Google Scholar]
- Christopoulos, C.; Pampanin, S.; Nigel Priestley, M.J. Performance-based seismic response of frame structures including residual deformations part I: Single-degree of freedom systems. J. Earthq. Eng. 2003, 7, 97–118. [Google Scholar] [CrossRef]
- Jung, D.; Wilcoski, J.; Andrawes, B. Bidirectional shake table testing of RC columns retrofitted and repaired with shape memory alloy spirals. Eng. Struct. 2018, 160, 171–185. [Google Scholar] [CrossRef]
- Deogekar, P.S.; Andrawes, B. Probabilistic Seismic Demand Models for Shape Memory Alloy Retrofitted RC Bridge Columns. J. Bridge Eng. 2018, 23, 12. [Google Scholar] [CrossRef]
- Sun, Z.Y.; Sun, Y.L.; Zheng, Y.; Iwashita, K.; Zhang, J.; Wu, G. Experimental study on precast concrete columns reinforced with bundled SFCBs under horizontal cyclic loading. J. Build. Eng. 2023, 74, 106882. [Google Scholar] [CrossRef]
- Wei, Y.; Cheng, X.; Wu, G.; Duan, M.; Wang, L. Experimental investigations of concrete-filled steel tubular columns confined with high-strength steel wire. Adv. Struct. Eng. 2019, 22, 2771–2784. [Google Scholar] [CrossRef]
- Sun, Z.Y.; Wu, G.; Wu, Z.S.; Zhang, M.; Hu, X.Q. Experimental Study on the Bond Behavior between Steel Fiber Composite Bar(SFCB) and Concrete. Earthq. Resist. Eng. Retrofit. 2009, 31, 21–27. [Google Scholar]
- Dong, Z.-Q.; Wu, G.; Xu, Y.-Q. Bond and Flexural Behavior of Sea Sand Concrete Members Reinforced with Hybrid Steel-Composite Bars Presubjected to Wet–Dry Cycles. J. Compos. Constr. 2017, 21, 04016095. [Google Scholar] [CrossRef]
- Zhou, Y.; Zheng, Y.; Pan, J.; Sui, L.; Xing, F.; Sun, H.; Li, P. Experimental investigations on corrosion resistance of innovative steel-FRP composite bars using X-ray microcomputed tomography. Compos. Part B Eng. 2019, 161, 272–284. [Google Scholar]
- Solyom, S.; Di Benedetti, M.; Balázs, G.L. Bond of FRP bars in air-entrained concrete: Experimental and statistical study. Constr. Build. Mater. 2021, 300, 124193. [Google Scholar] [CrossRef]
- Liu, J.; Yuan, Y.; Wang, L.; Liu, Z.; Yang, J. Parameter Study of Interfacial Capacities for FRP–Steel Bonded Joints Based on 3D FE Modeling. Materials 2022, 15, 7787. [Google Scholar] [CrossRef] [PubMed]
- Wu, G.; Wu, Z.-S.; Luo, Y.-B.; Sun, Z.-Y.; Hu, X.-Q. Mechanical properties of steel-FRP composite bar under uniaxial and cyclic tensile loads. J. Mater. Civ. Eng. 2010, 22, 1056–1066. [Google Scholar] [CrossRef]
- Sun, Z.-Y.; Wu, G.; Wu, Z.-S.; Zhang, J. Nonlinear Behavior and Simulation of Concrete Columns Reinforced by Steel-FRP Composite Bars. J. Bridge Eng. 2014, 19, 220–234. [Google Scholar] [CrossRef]
- Ibrahim, A.I.; Wu, G.; Sun, Z.-Y. Experimental study of cyclic behavior of concrete bridge columns reinforced by steel basalt-fiber composite bars and hybrid stirrups. J. Compos. Constr. 2016, 21, 04016091. [Google Scholar] [CrossRef]
- Zou, X.; Lin, H.; Feng, P.; Bao, Y.; Wang, J. A review on FRP-concrete hybrid sections for bridge applications. Compos. Struct. 2021, 262, 113336. [Google Scholar] [CrossRef]
- ACI 4401R-15; Guide for the Design and Construction of Structural Concrete Reinforced with Fiber-Reinforced Polymer (FRP) Bars. ACI: Farmington Hills, MI, USA, 2015.
- CSA-S806-R17; Design and Construction of Building Structures with Fibre-Reinforced Polymers. CSA: Mississauga, ON, Canada, 2017.
- Tang, Y.; Sun, Z.; Wu, G.; Wei, Y. Experimental Study on Cyclic Behavior of SFCBs with Different Slenderness Ratios. J. Mater. Civ. Eng. 2021, 33, 04021204. [Google Scholar] [CrossRef]
- Tang, Y.; Sun, Z.; Wu, G. Compressive Behavior of Sustainable Steel-FRP Composite Bars with Different Slenderness Ratios. Sustainability 2019, 11, 1118. [Google Scholar] [CrossRef]
- Tang, Y.; Sun, Z.; Wu, G.; Wei, Y. Compressive Behavior of Steel-FRP Composite Bars Confined with Low Elastic Modulus FRP Spirals in Concrete Columns. J. Compos. Constr. 2022, 26, 04022058. [Google Scholar] [CrossRef]
- Lin, H.; Zeng, H.; Feng, P.; Jiang, C.; Zhang, Y. Bond behavior of FRP-concrete wet-bonding interface under lateral confinement. Eng. Struct. 2023, 292, 116536. [Google Scholar] [CrossRef]
- Zhang, Y.; Wei, Y.; Miao, K.; Li, B. A novel seawater and sea sand concrete-filled FRP-carbon steel composite tube column: Cyclic axial compression behaviour and modelling. Eng. Struct. 2022, 252, 113531. [Google Scholar] [CrossRef]
- Teng, J.; Chen, J.-F.; Smith, S.T.; Lam, L. FRP: Strengthened RC Structures; Wiley-VCH: Weinheim, Germany, 2002; p. 266. ISBN 0-471-48706-6. [Google Scholar]
- ASTM D7205/D7205M-06; Standard Test Method for Tensile Properties of Fiber Reinforced Polymer Matrix Composite Bars. ASTM International: West Conshohocken, PA, USA, 2016.
- TORAY INDUSTRIES, INC. Torayca™ Fabric. Available online: https://www.cf-composites.toray/resources/data_sheets/#anc22023 (accessed on 21 August 2023).
- C469-14; Standard Test Method for Static Modulus of Elasticity and Poisson’s Ratio of Concrete in Compression. ASTM International: West Conshohocken, PA, USA, 2014.
- C39M-21; Standard Test Method for Compressive Strength of Cylindrical Concrete Specimens. ASTM International: West Conshohocken, PA, USA, 2021.
- Tobbi, H.; Farghaly, A.S.; Benmokrane, B. Concrete Columns Reinforced Longitudinally and Transversally with Glass Fiber-Reinforced Polymer Bars. ACI Struct. J. 2012, 109, 551–558. [Google Scholar]
- Afifi, M.Z.; Mohamed, H.M.; Benmokrane, B. Axial Capacity of Circular Concrete Columns Reinforced with GFRP Bars and Spirals. J. Compos. Constr. 2014, 18, 04013017. [Google Scholar] [CrossRef]
- Mohamed, H.M.; Afifi, M.Z.; Benmokrane, B. Performance Evaluation of Concrete Columns Reinforced Longitudinally with FRP Bars and Confined with FRP Hoops and Spirals under Axial Load. J. Bridge Eng. 2014, 19, 04014020. [Google Scholar] [CrossRef]
- Hadi, M.N.S.; Karim, H.; Sheikh, M.N. Experimental Investigations on Circular Concrete Columns Reinforced with GFRP Bars and Helices under Different Loading Conditions. J. Compos. Constr. 2016, 20, 04016009. [Google Scholar] [CrossRef]
- Hadhood, A.; Mohamed, H.M.; Ghrib, F.; Benmokrane, B. Efficiency of glass-fiber reinforced-polymer (GFRP) discrete hoops and bars in concrete columns under combined axial and flexural loads. Compos. Part B Eng. 2017, 114, 223–236. [Google Scholar] [CrossRef]
- Xue, W.; Peng, F.; Fang, Z. Behavior and Design of Slender Rectangular Concrete Columns Longitudinally Reinforced with Fiber-Reinforced Polymer Bars. ACI Struct. J. 2018, 115, 311–322. [Google Scholar] [CrossRef]
- Tang, Y.; Sun, Z.; Wei, Y.; Zou, X. Compressive behavior and design method of BFRP bars constrained with a BFRP spiral with different spacings in concrete members. Eng. Struct. 2022, 268, 114757. [Google Scholar] [CrossRef]

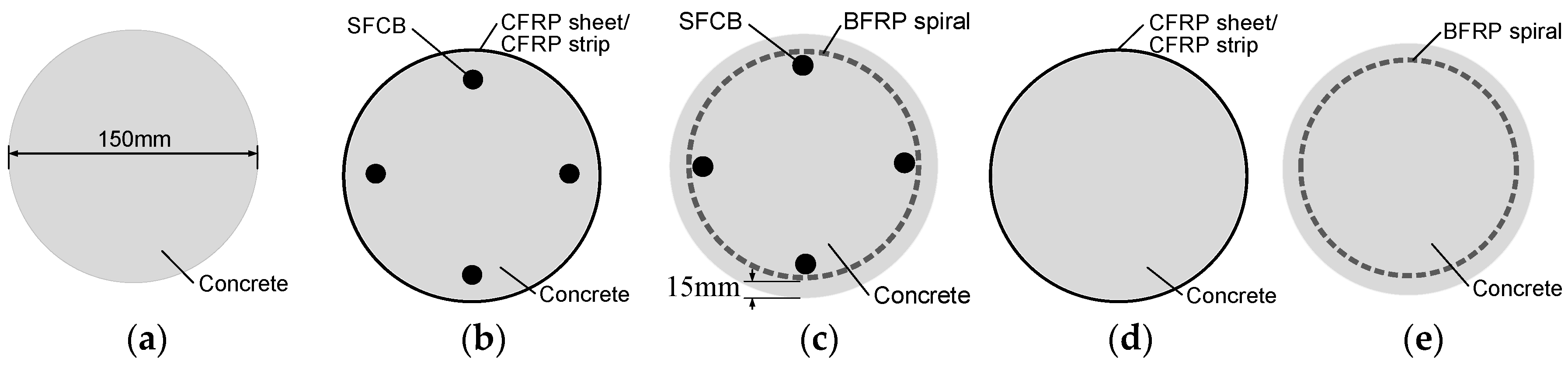


| Cylinder Type | Longi. Reinf. | Trans. Reinf. | Cylinder | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Type | ρl (%) | Type | Layer | bf or df (mm) | sf or s (mm) | El (MPa) | ρf (%) | fl (MPa) | Failure Mode | Peak Stress (MPa) | Peak Strain | |
| C | — | — | — | — | — | — | — | — | — | CC | 43 | 0.004 |
| SF-CF | SFCB | 3.0 | cs | 4 | 300 | — | 2155 | 1.8 | 36.5 | — | 170 | 0.039 |
| SF-CD | SFCB | 3.0 | cd | 6 | 36 | 18 | 2155 | 1.8 | 36.5 | SFF-CC | 167 | 0.048 |
| SF-BS | SFCB | 3.0 | bs | — | 8 | 23 | 1935 | 7.3 | 46.8 | SF-SFF | 54 | 0.015 |
| CF | — | — | cs | 4 | 300 | — | 2155 | 1.8 | 36.5 | — | 170 | 0.039 |
| CD | — | — | cd | 6 | 36 | 18 | 2155 | 1.8 | 36.5 | CC | 167 | 0.042 |
| BS | — | — | bs | — | 8 | 23 | 1935 | 7.3 | 46.8 | SF | 43 | 0.013 |
| Type | D (mm) | Deq (mm) | Elongation (%) | Density (g/cm3) | Yield Strength (MPa) | Tensile Strength (MPa) | Elastic Modulus (GPa) | Post-Yield Modulus (GPa) |
|---|---|---|---|---|---|---|---|---|
| SFCB | 16.8 | 12.9 | 4.5 | — | 376 | 1247 | 92 | 29 |
| Inner steel bar | 10.0 | — | 14.3 | 7.85 | 400 | 528 | 200 | — |
| Basalt fiber | 0.013 | — | 2.5 | 2.63 | — | 2250 | 90 | — |
| Epoxy resin | — | — | 6.1 | 1.06 | — | 95 | 3.6 | — |
| Type | Fiber Weight (g/m2) | Thickness (mm) | Density (g/cm3) | Tensile Strength (MPa) | Elastic Modulus (GPa) |
|---|---|---|---|---|---|
| CFRP sheet | 300 | 0.167 | 1.80 | 4100 | 242 |
| CFRP strip | 300 | 0.167 | 1.80 | 4100 | 242 |
| BFRP spiral | — | — | 2.00 | 1281 | 53 |
| Equation No. | Approaching Method | |||||
|---|---|---|---|---|---|---|
| SF-CF | SF-CD | SF-BS | Average | Deviation | ||
| 6 | I | 0.86 | 0.94 | 0.96 | 0.92 | 0.04 |
| 7 | I | 0.83 | 0.91 | 0.87 | 0.87 | 0.03 |
| 8 | II | 0.80 | 0.89 | 0.77 | 0.82 | 0.05 |
| 9 | II | 0.82 | 0.91 | 0.84 | 0.86 | 0.04 |
| 10 | II | 0.81 | 0.89 | 0.80 | 0.83 | 0.04 |
| 11 | II | 0.84 | 0.93 | 0.81 | 0.86 | 0.05 |
| 12 | III | 0.88 | — | 1.04 | 0.96 | 0.08 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Duan, M.; Tang, Y.; Wang, Y.; Wei, Y.; Wang, J. Compressive Performance of Longitudinal Steel-FRP Composite Bars in Concrete Cylinders Confined by Different Type of FRP Composites. Polymers 2023, 15, 4051. https://doi.org/10.3390/polym15204051
Duan M, Tang Y, Wang Y, Wei Y, Wang J. Compressive Performance of Longitudinal Steel-FRP Composite Bars in Concrete Cylinders Confined by Different Type of FRP Composites. Polymers. 2023; 15(20):4051. https://doi.org/10.3390/polym15204051
Chicago/Turabian StyleDuan, Maojun, Yu Tang, Yusheng Wang, Yang Wei, and Jiaqing Wang. 2023. "Compressive Performance of Longitudinal Steel-FRP Composite Bars in Concrete Cylinders Confined by Different Type of FRP Composites" Polymers 15, no. 20: 4051. https://doi.org/10.3390/polym15204051
APA StyleDuan, M., Tang, Y., Wang, Y., Wei, Y., & Wang, J. (2023). Compressive Performance of Longitudinal Steel-FRP Composite Bars in Concrete Cylinders Confined by Different Type of FRP Composites. Polymers, 15(20), 4051. https://doi.org/10.3390/polym15204051







