Identification of NOL-Ring Composite Materials’ Damage Mechanism Based on the STOA-VMD Algorithm
Abstract
1. Introduction
2. Methods
2.1. Overview of VMD
- The original signal is decomposed into K-independent modes uK(t); the Hilbert transform is then performed to obtain the unilateral spectrum.
- The decomposition sequence is a finite bandwidth modal component with a central frequency.
- The corresponding constrained variational model can be expressed as shown in Equation (1).
- 4.
- The constrained variational problems are transformed into unconstrained variational problems. The augmented function can be introduced, as depicted in Equation (2).
- 5.
- The alternating direction multiplier method (ADMM) is used to find the ‘saddle point’ of the augmented function. The models of uK and ωK after alternating the optimization iterations are as follows:
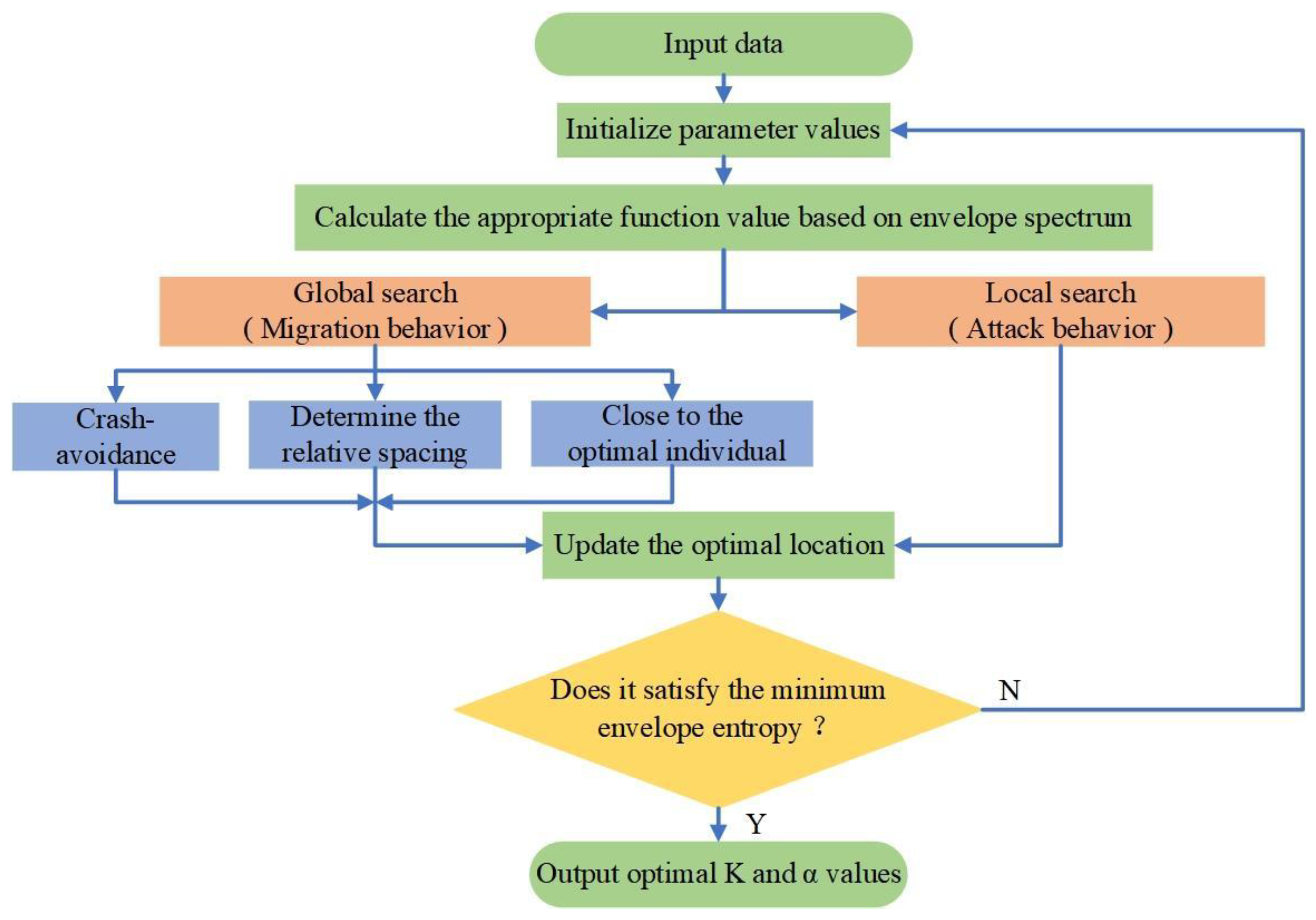
2.2. The VMD Parameters K and Can Be Optimized Based on the STOA
- The individual fitness value and population average fitness were calculated.
- Migration behavior was observed or a global search was performed.a. Crash avoidance:
- 3.
- Attack behavior was observed or a local search performed. The mathematical models for the attack behavior were:
- 4.
- The final position update of the tern was acquired, as follows:
- 5.
- The fitness value was calculated and the global optimal value was retained.
3. Experiments and Results
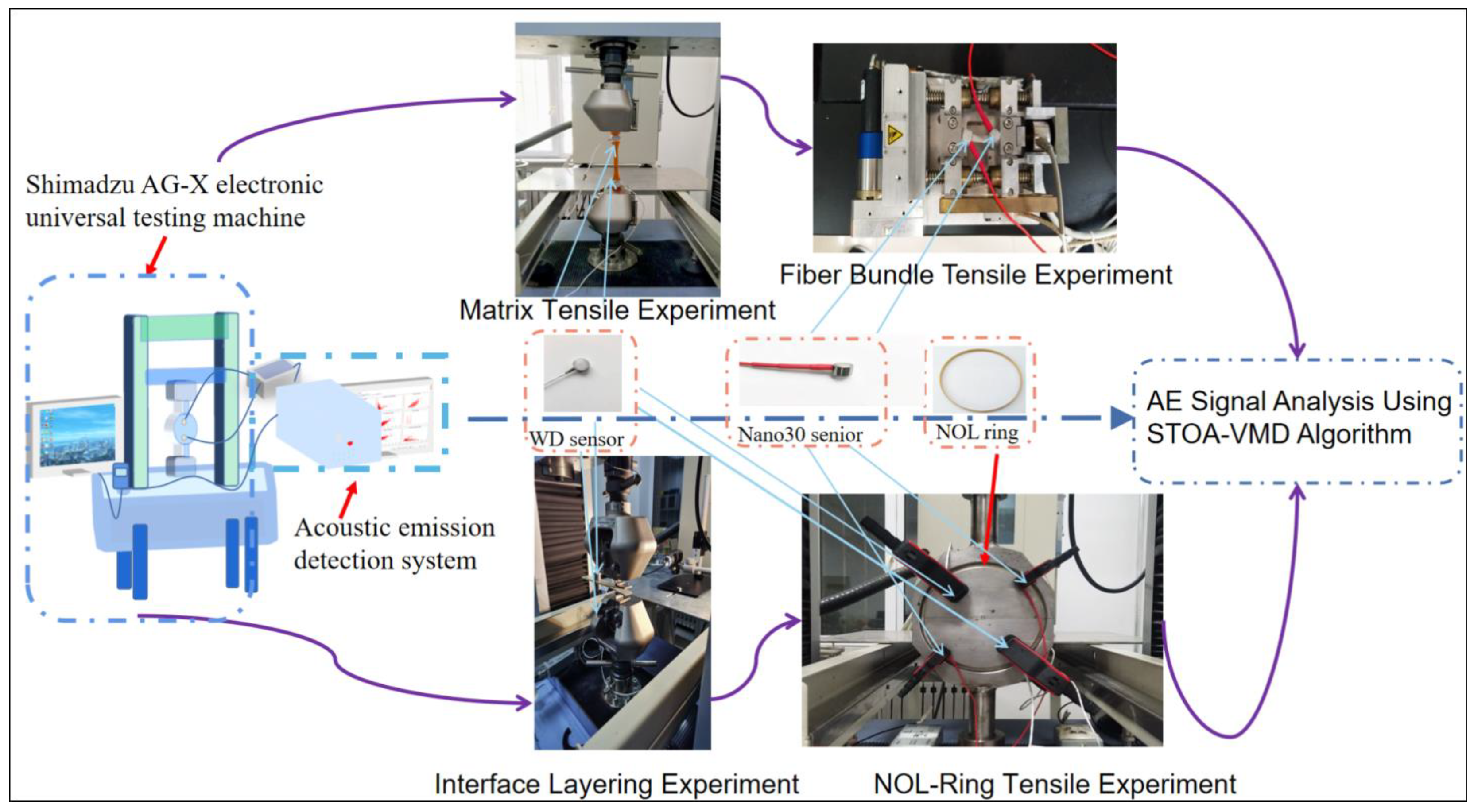
3.1. Experimental Materials and Methods
3.1.1. Composite Laminates
3.1.2. NOL-Ring
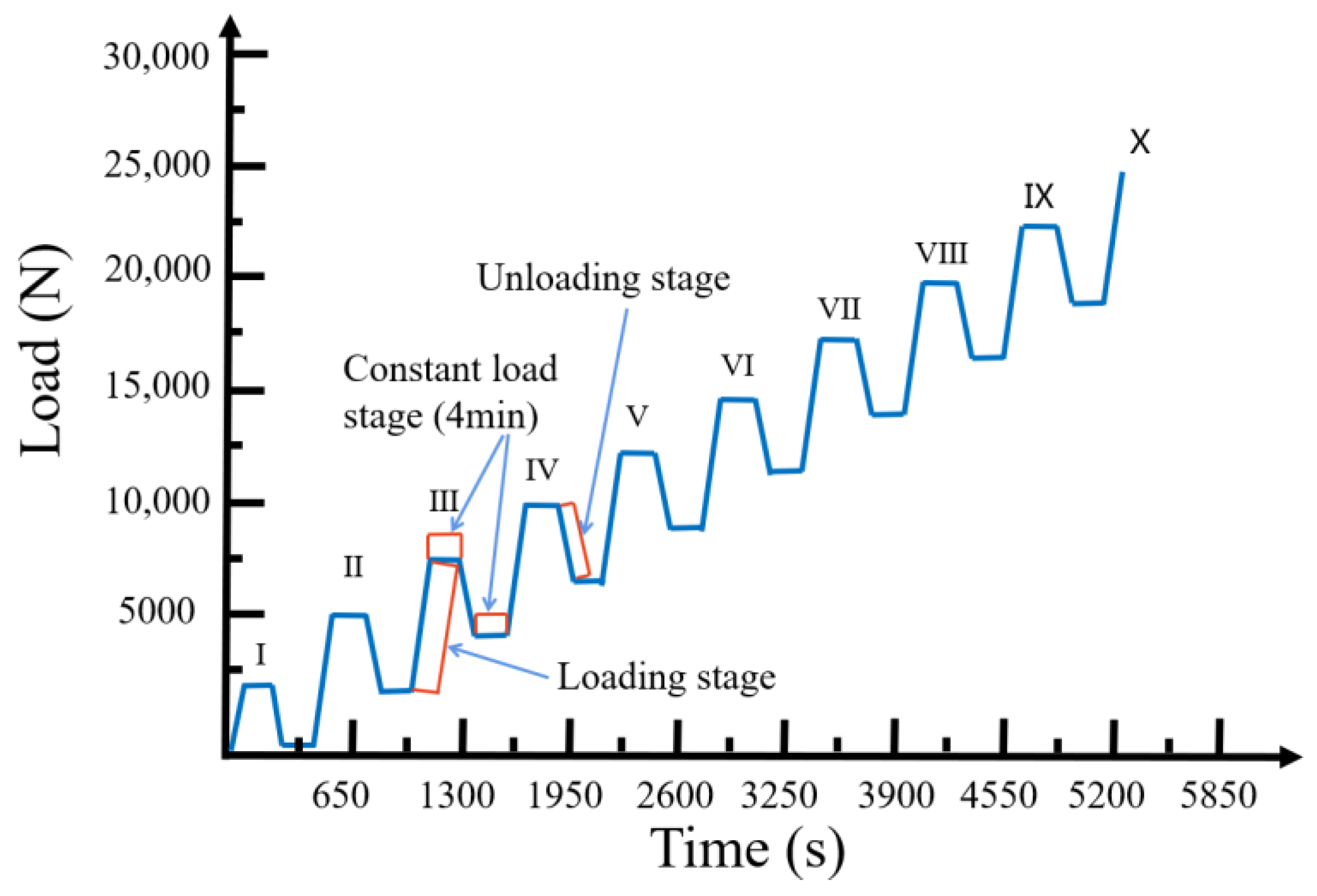
3.1.3. Experimental Equipment Settings
3.2. Results
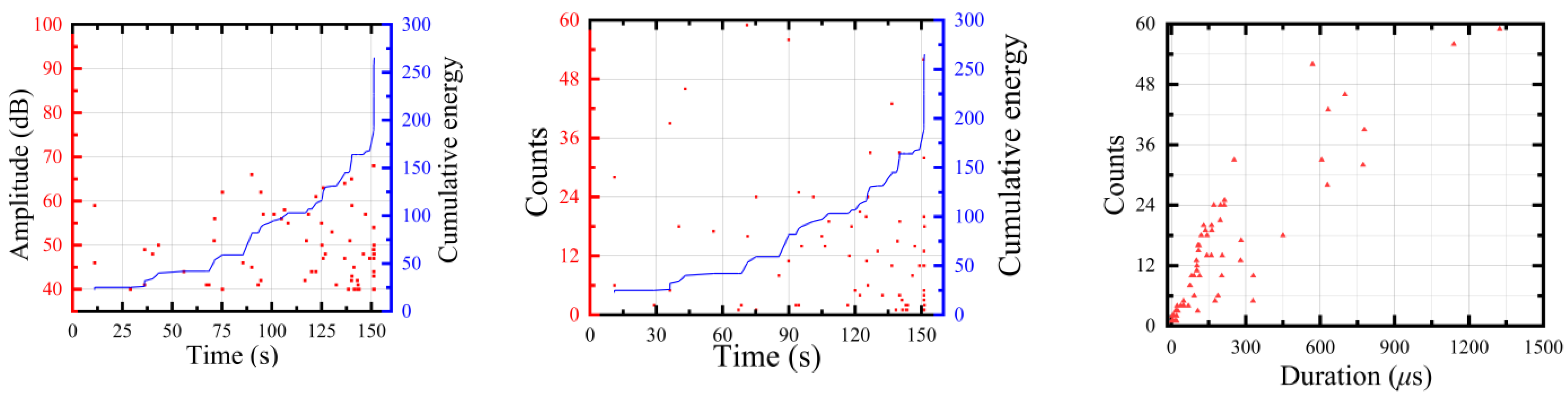
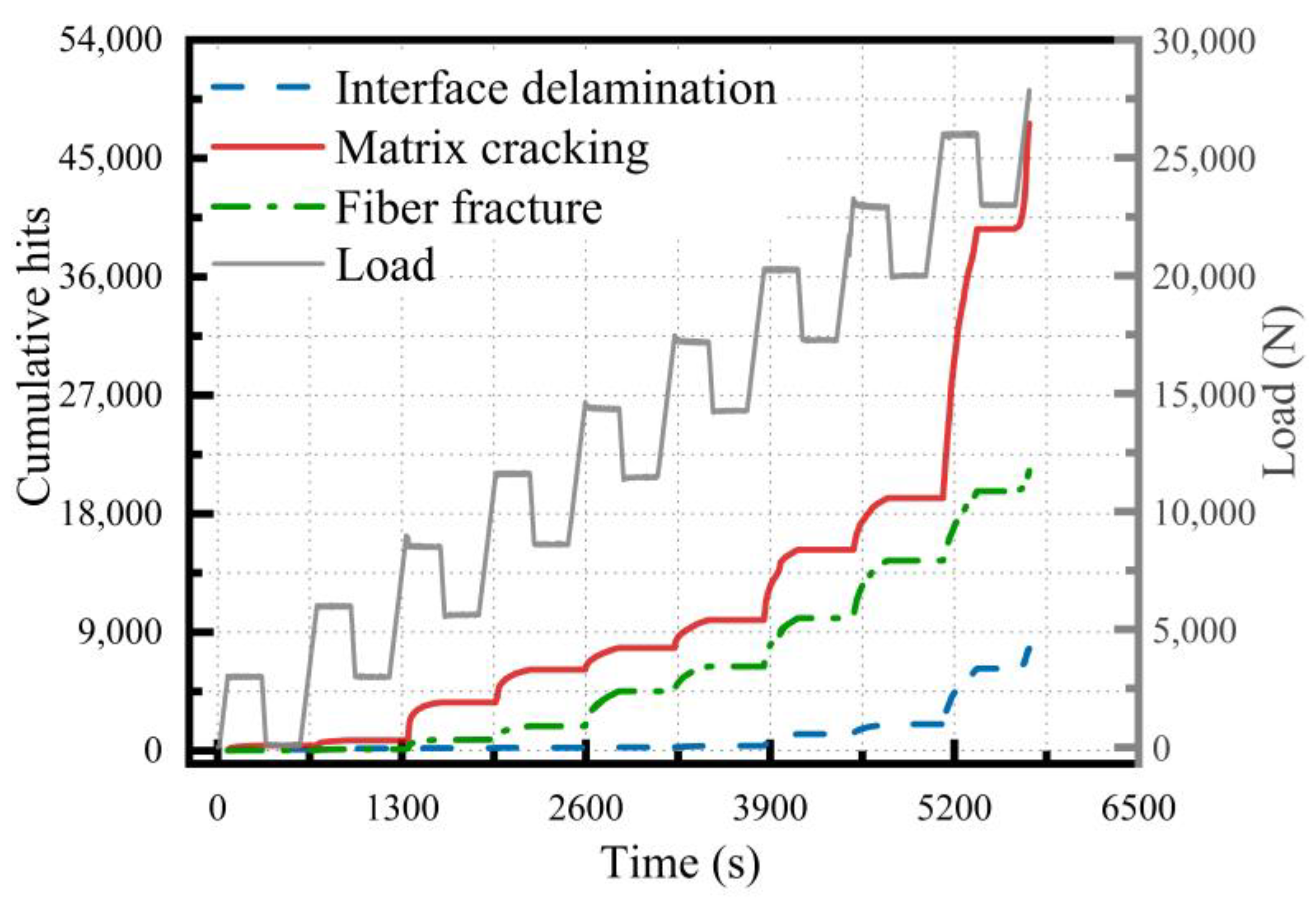
3.2.1. Analysis of the Acoustic Emission Parameter History
3.2.2. Optimization Analysis of the Signal Parameters of the NOL-Ring Step Loading Tensile Test
- Optimizing the VMD parameters K and α
- 2.
- Comparison diagram of time–frequency analysis of matrix cracking signal
- 3.
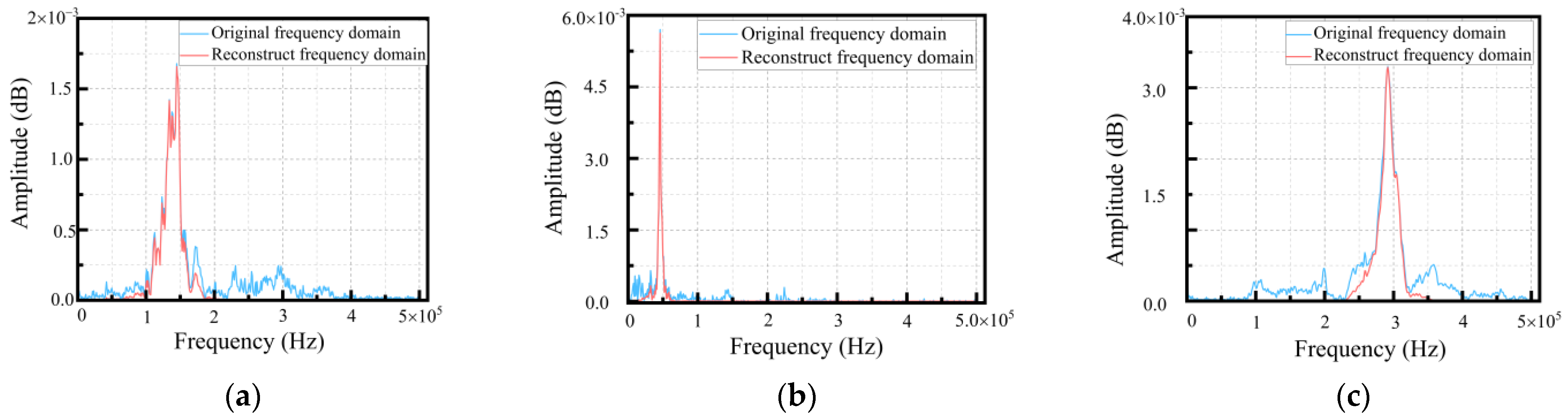
- Comparative analysis of the single damage reconstruction signal
3.2.3. Identification and Verification of the Damage Optimization Algorithm
- Identification and verification of the single damage optimization algorithm
- 2.
- Identification and verification of the NOL-ring damage optimization algorithm
4. Conclusions
- The STOA was used to optimize the VMD parameters, determine the optimal decomposition mode number K and penalty coefficient α, and perform the adaptive decomposition of acoustic emission signals.
- Based on the characteristics of a single damage signal, the damage mechanism recognition algorithm was constructed and the recognition effect was verified. The recognition rates of matrix cracking, fiber fractures, and delamination damage were 94.59%, 94.26%, and 96.45%, respectively.
- By analyzing the AE signal of the glass fiber/epoxy NOL-ring experiment, the algorithm was used to determine the damage mechanism of the NOL-ring experiment, which confirmed that the algorithm could effectively perform damage identification of the glass fiber/epoxy NOL-ring damage complete structure.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zhou, W.; Zhao, W.Z.; Zhang, Y.N.; Ding, Z.J. Cluster analysis of acoustic emission signals and deformation measurement for delaminated glass fiber epoxy composites. Compos. Struct. 2018, 195, 349–358. [Google Scholar] [CrossRef]
- Nikam, D.; Milani, A.S. Effect of fiber orientation on the stresses generated in dental crowns made of glass fiber composites. Compos. Struct. 2022, 279, 114790. [Google Scholar] [CrossRef]
- Liu, H.; Liu, S.; Liu, Z.; Mrad, N.; Milani, A.S. Data-driven approaches for characterization of delamination damage in composite materials. IEEE Trans. Ind. Electron. 2020, 68, 2532–2542. [Google Scholar] [CrossRef]
- Zobeiry, N.; Reiner, J.; Vaziri, R. Theory-guided machine learning for damage characterization of composites. Compos. Struct. 2020, 246, 112407. [Google Scholar] [CrossRef]
- Wei, Q.; Zhu, L.; Zhu, J.; Zhuo, L.; Hao, W.; Xie, W. Characterization of impact fatigue damage in CFRP composites using nonlinear acoustic resonance method. Compos. Struct. 2020, 253, 112804. [Google Scholar] [CrossRef]
- Hu, H.; Wang, B.-T.; Lee, C.-H.; Su, J.-S. Damage detection of surface cracks in composite laminates using modal analysis and strain energy method. Compos. Struct. 2006, 74, 399–405. [Google Scholar] [CrossRef]
- Xu, B.; Wu, J.; Wang, M. Study of modal acoustic emission to monitor the impact damage in a composite plate. J. Vibroeng. 2017, 19, 3335–3348. [Google Scholar] [CrossRef]
- Metaxa, S.; Kalkanis, K.; Psomopoulos, C.S.; Kaminaris, S.D.; Ioannidis, G. A review of structural health monitoring methods for composite materials. Proc. Struct. Integr. 2019, 22, 369–375. [Google Scholar] [CrossRef]
- Kempf, M.; Skrabala, O.; Altstädt, V. Acoustic emission analysis for characterisation of damage mechanisms in fibre reinforced thermosetting polyurethane and epoxy. Compos. Part B Eng. 2014, 56, 477–483. [Google Scholar] [CrossRef]
- Gholizadeh, S.; Leman, Z.; Baharudin, B.; Inayatullah, O. Acoustic emission analysis for characterisation of damage mechanisms in glass fiber reinforced polyester composite. Aust. J. Mech. Eng. 2018, 16, 11–20. [Google Scholar] [CrossRef]
- Oz, F.E.; Ersoy, N.; Lomov, S.V. Do high frequency acoustic emission events always represent fibre failure in CFRP laminates? Compos. Part A Appl. Sci. Manuf. 2017, 103, 230–235. [Google Scholar] [CrossRef]
- Tabrizi, I.E.; Kefal, A.; Zanjani, J.S.M.; Akalin, C.; Yildiz, M. Experimental and numerical investigation on fracture behavior of glass/carbon fiber hybrid composites using acoustic emission method and refined zigzag theory. Compos. Struct. 2019, 223, 110971. [Google Scholar] [CrossRef]
- Li, L.; Lomov, S.V.; Yan, X.; Carvelli, V. Cluster analysis of acoustic emission signals for 2D and 3D woven glass/epoxy composites. Compos. Struct. 2014, 116, 286–299. [Google Scholar] [CrossRef]
- Favre, J.P.; Laizet, J.C. Amplitude and counts per event analysis of the acoustic emission generated by the transverse cracking of cross-ply CFRP. Compos. Sci. Technol. 1989, 36, 27–43. [Google Scholar] [CrossRef]
- Surgeon, M.; Vanswijgenhoven, E.; Wevers, M.; Van der Biest, O. Acoustic emission during tensile testing of SiC-fibre-reinforced BMAS glass-ceramic composites. Compos. Part A Appl. Sci. Manuf. 1997, 28, 473–480. [Google Scholar] [CrossRef]
- Loutas, T.H.; Kostopoulos, V. Health monitoring of carbon/carbon, woven reinforced composites. Damage assessment by using advanced signal processing techniques. Part I: Acoustic emission monitoring and damage mechanisms evolution. Compos. Sci. Technol. 2009, 69, 265–272. [Google Scholar] [CrossRef]
- Jiang, P.; Liu, X.; Li, W.; Guo, F.; Hong, C.; Liu, Y.; Yang, C. Damage characterization of carbon fiber composite pressure vessels based on modal acoustic emission. Materials 2022, 15, 4783. [Google Scholar] [CrossRef]
- Ghaib, M.; Shateri, M.; Thomson, D.; Svecova, D. Study of FRP bars under tension usingacoustic emission detection technique. Civ. Struct. Health Monit. 2018, 8, 285–300. [Google Scholar] [CrossRef]
- Djabali, A.; Toubal, L.; Zitoune, R.; Rechak, S. An experimental investigation of the mechanical behavior and damage of thick laminated carbon/epoxy composite. Compos. Struct. 2018, 184, 178–190. [Google Scholar] [CrossRef]
- Joselin, R.; Chelladurai, T.; Usha, K.M.; Vasudev, E.S. Failure prediction of glass/epoxy specimens using NOL ring test and acoustic emission technique. Int. J. Mater. Prod. Technol. 2015, 50, 180–192. [Google Scholar] [CrossRef]
- Plöckl, M.; Sause, M.G.; Scharringhausen, J.; Horn, S.R. Failure analysis of NOL-ring specimens by acoustic emission. In Proceedings of the 30th European Conference on Acoustic Emission Testing, Granada, Spain, 12–15 September 2012; pp. 1–12. [Google Scholar]
- Dragomiretskiy, K.; Zosso, D. Variational mode decomposition. IEEE Trans. Signal Process. 2014, 62, 531–544. [Google Scholar] [CrossRef]
- Civera, M.; Surace, C. A comparative analysis of signal decomposition techniques for structural health monitoring on an experimental benchmark. Sensors 2021, 21, 1825. [Google Scholar] [CrossRef] [PubMed]
- Yuan, J.; Liu, L.; Yang, Z.; Zhang, Y. Tool wear condition monitoring by combining variational mode decomposition and ensemble learning. Sensors 2020, 20, 6113. [Google Scholar] [CrossRef]
- Bazi, R.; Benkedjouh, T.; Habbouche, H.; Rechak, S.; Zerhouni, N. A hybrid CNN-BiLSTM approach-based variational mode decomposition for tool wear monitoring. Int. J. Adv. Manuf. Technol. 2022, 119, 3803–3817. [Google Scholar] [CrossRef]
- Wan, L.; Zhang, X.; Zhou, Q.; Wen, D.; Ran, X. Acoustic emission identification of wheel wear states in engineering ceramic grinding based on parameter-adaptive VMD. Ceram. Int. 2023, 49, 13618–13630. [Google Scholar] [CrossRef]
- Li, Y.; Tang, B.; Jiang, X.; Yi, Y. Bearing fault feature extraction method based on GA-VMD and center frequency. Math. Probl. Eng. 2022, 2022, 2058258. [Google Scholar] [CrossRef]
- Cao, H.; Jiang, M.; Jia, L.; Ma, M.; Sun, L.; Zhang, L.; Tian, A.; Liang, J. An ultrasonic signal processing method to improve defect depth estimation in composites based on empirical mode decomposition. Meas. Sci. Technol. 2021, 32, 115112. [Google Scholar] [CrossRef]
- Yu, G. A concentrated time–frequency analysis tool for bearing fault diagnosis. IEEE Trans. Instrum. Meas. 2019, 69, 371–381. [Google Scholar] [CrossRef]
- Zhou, Y.; Lu, J.; Hu, Z.; Dong, H.; Yan, W.; Yang, D. Novel feature extraction method of pipeline signals based on multi-scale dispersion entropy partial mean of multi-modal component. Measurement 2022, 205, 112137. [Google Scholar] [CrossRef]
- An, X.; Yang, J. Denoising of hydropower unit vibration signal based on variational mode decomposition and approximate entropy. Trans. Inst. Meas. Contr. 2016, 38, 282–292. [Google Scholar] [CrossRef]
- Liu, T.; Chen, J.; Dong, G.; Xiao, W.; Zhou, X. The fault detection and diagnosis in rolling element bearings using frequency band entropy. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2013, 227, 87–99. [Google Scholar] [CrossRef]
- Ram, R.; Mohanty, M.N. Comparative analysis of EMD and VMD algorithm in speech enhancement. Int. J. Nat. Comput. Res. 2017, 6, 17–35. [Google Scholar] [CrossRef]
- Li, H.; Liu, T.; Wu, X.; Chen, Q. An optimized VMD method and its applications in bearing fault diagnosis. Measurement 2020, 166, 108185. [Google Scholar] [CrossRef]
- Li, H.; Liu, T.; Wu, X.; Chen, Q. Application of optimized variational mode decomposition based on kurtosis and resonance frequency in bearing fault feature extraction. Trans. Inst. Meas. Control 2020, 42, 518–527. [Google Scholar] [CrossRef]
- Yao, T.; Liu, X. Financial time series forecasting: A combinatorial forecasting model based on STOA optimizing VMD. Int. J. Artif. Intell. Tools 2022, 31, 2250042. [Google Scholar] [CrossRef]
- Dhiman, G.; Kaur, A. STOA: A bio-inspired based optimization algorithm for industrial engineering problems. Eng. Appl. Artif. Intell. 2019, 82, 148–174. [Google Scholar] [CrossRef]
- GB/T 7690.3-2013; Reinforcements-Test Method for Yarns—Part 3: Determination of Breaking Force and Breaking Elongation of Glass Fiber. Standardization Administration of China: Beijing, China, 2013.
- GB/T 3362-2017; Test Method for Tensile Properties of Carbon Fiber Multifilament. Standardization Administration of China: Beijing, China, 2017.
- ASTM D5528; Standard Test Method for Mode I Interlaminar Fracture Toughness of Unidirectional Fiber-Reinforced Polymer Matrix Composites. ASTM: West Conshohocken, PA, USA, 2021.

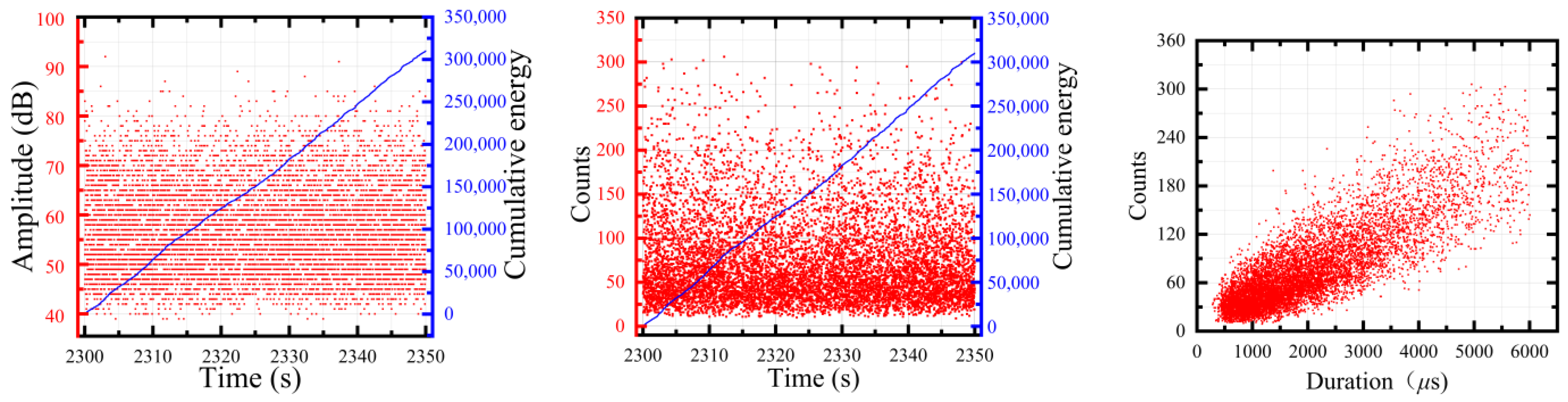
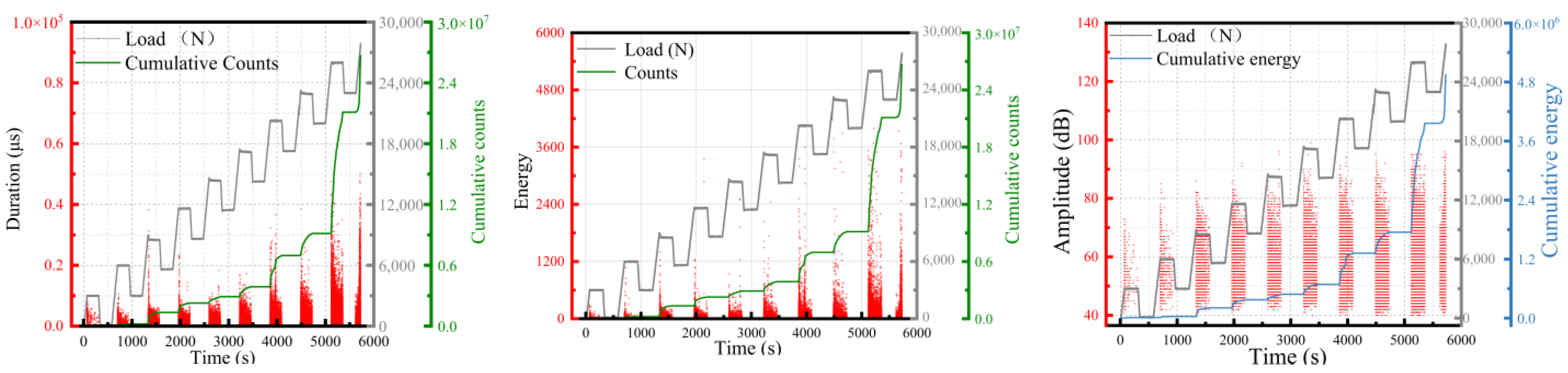
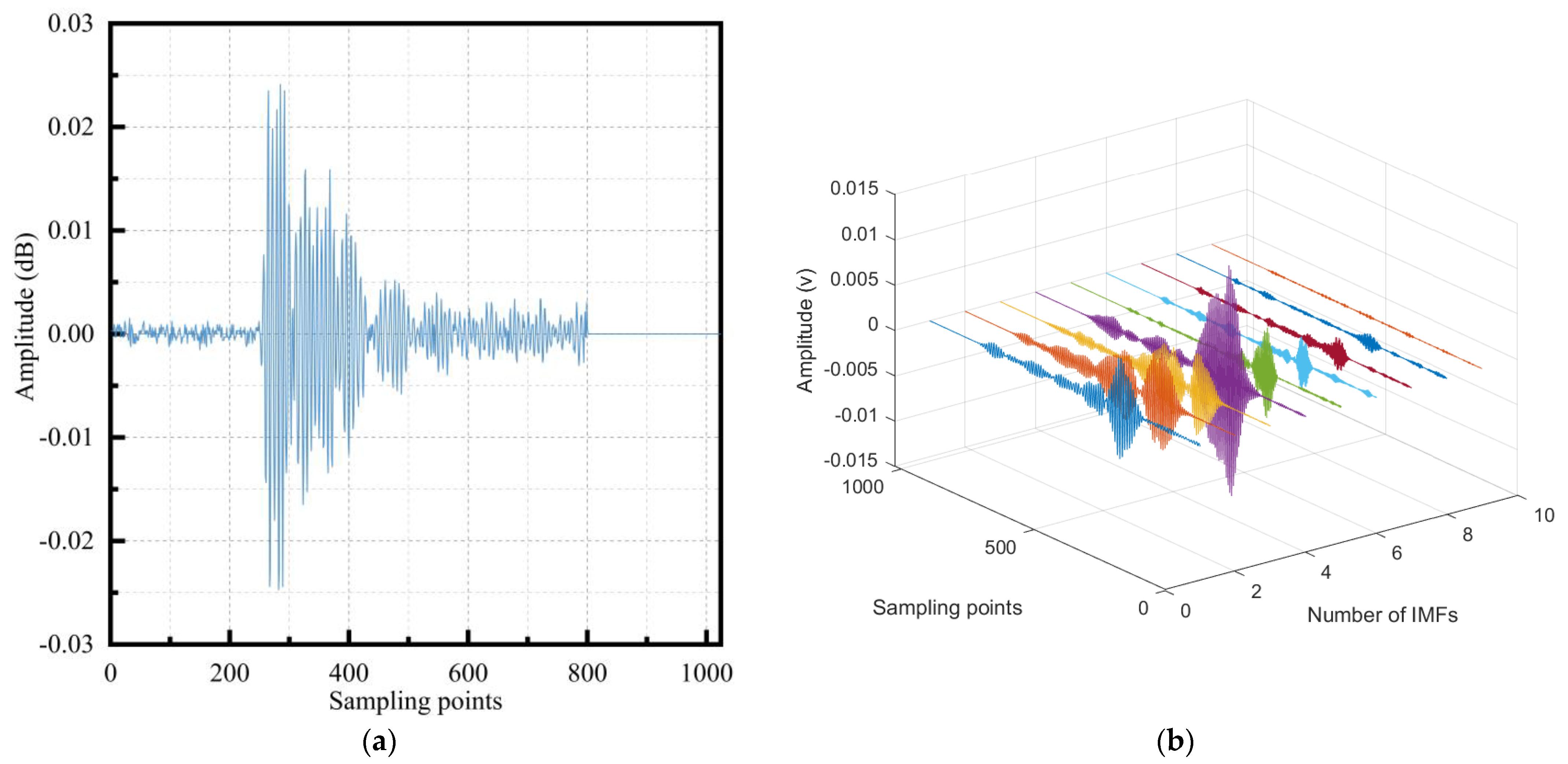
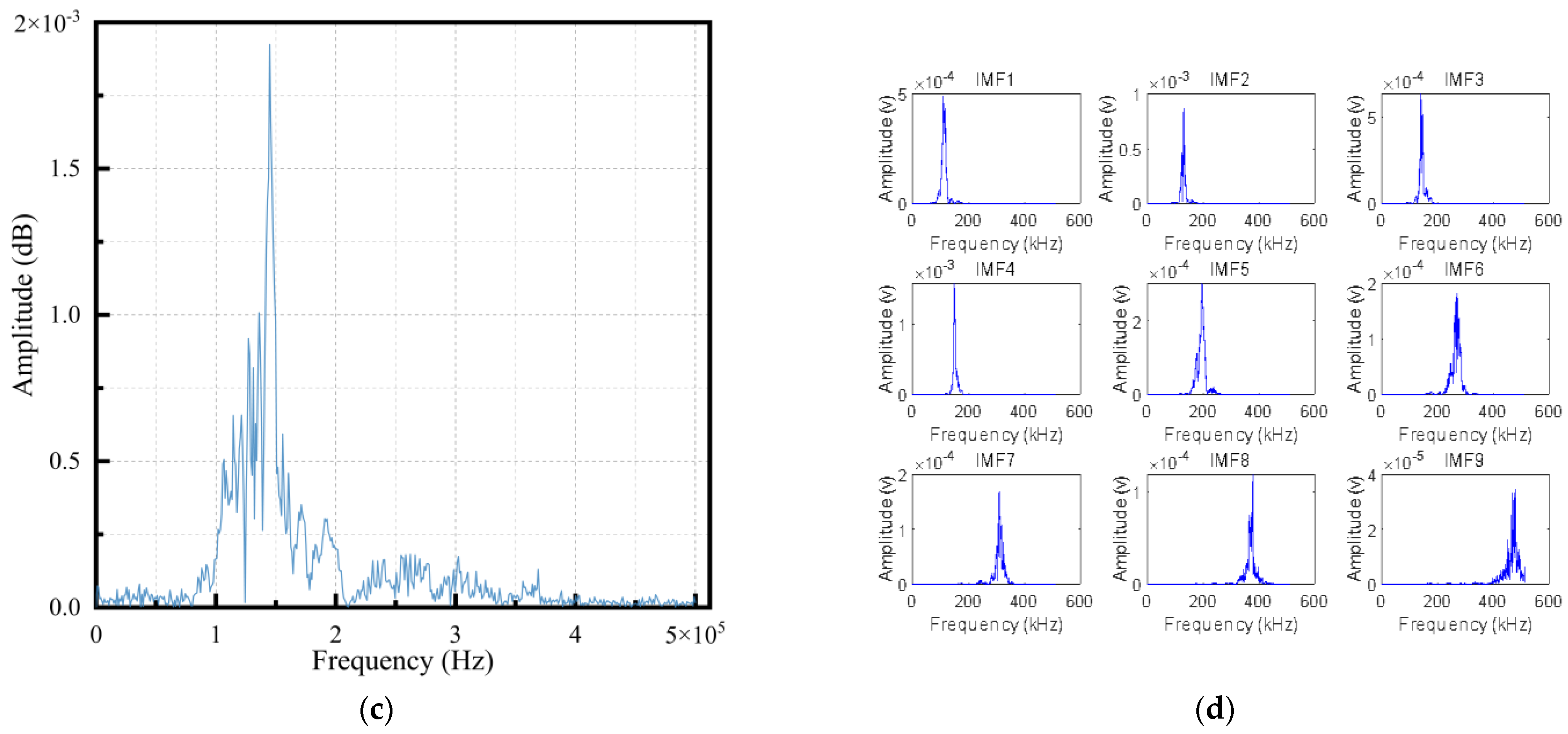


| Type Parameter | Tensile Strength/GPa | Tensile Modulus/GPa | Elongation (%) | Density (kg/m3) | Poisson Ratio |
|---|---|---|---|---|---|
| Matrix | 0.12 | 3 | 0.2 | 980 | 0.38 |
| Glass-fiber bundles | 0.28 | 90 | 3.5 | 1800 | 0.3 |
| Item | Sensor Type | Number Field of Channel (s) | Threshold/dB | Pre-Trigger Time/ | Signal Length |
|---|---|---|---|---|---|
| Matrix tensile | WD | 2 | 35 | 256 | 1024 |
| Glass-fiber bundle tensile | Nona30 | 2 | 35 | 256 | 1024 |
| Interface layering | WD | 2 | 35 | 256 | 1024 |
| NOL-ring tensile | WD | 2 | 35 | 256 | 1024 |
| Nona30 | 2 |
| Type | EMD Decomposition Mode Number | Times | 1 | 2 | 3 | 4 | Average Value |
|---|---|---|---|---|---|---|---|
| Sample 1 | 11 | 9 | 10 | 8 | 9 | 9 | |
| 2856.670 | 2574.752 | 3551.249 | 2786.009 | 2950.084 | |||
| Sample 2 | 10 | 8 | 8 | 9 | 8 | 8.25 | |
| 3446.525 | 1243.647 | 3110.164 | 4000 | 2942.170 |
| Type | Matrix Tensile Test | Fiber Bundle Tensile Test | Interface Layering Test |
|---|---|---|---|
| Cumulative hits | 37 | 1568 | 8964 |
| Matrix cracking/pc | 35 | 79 | 8628 |
| Fiber fracture/pc | 0 | 1478 | 197 |
| Interface delamination/pc | 2 | 2 | 121 |
| Accurate recognition rate/% | 94.59 | 94.26 | 96.45 |
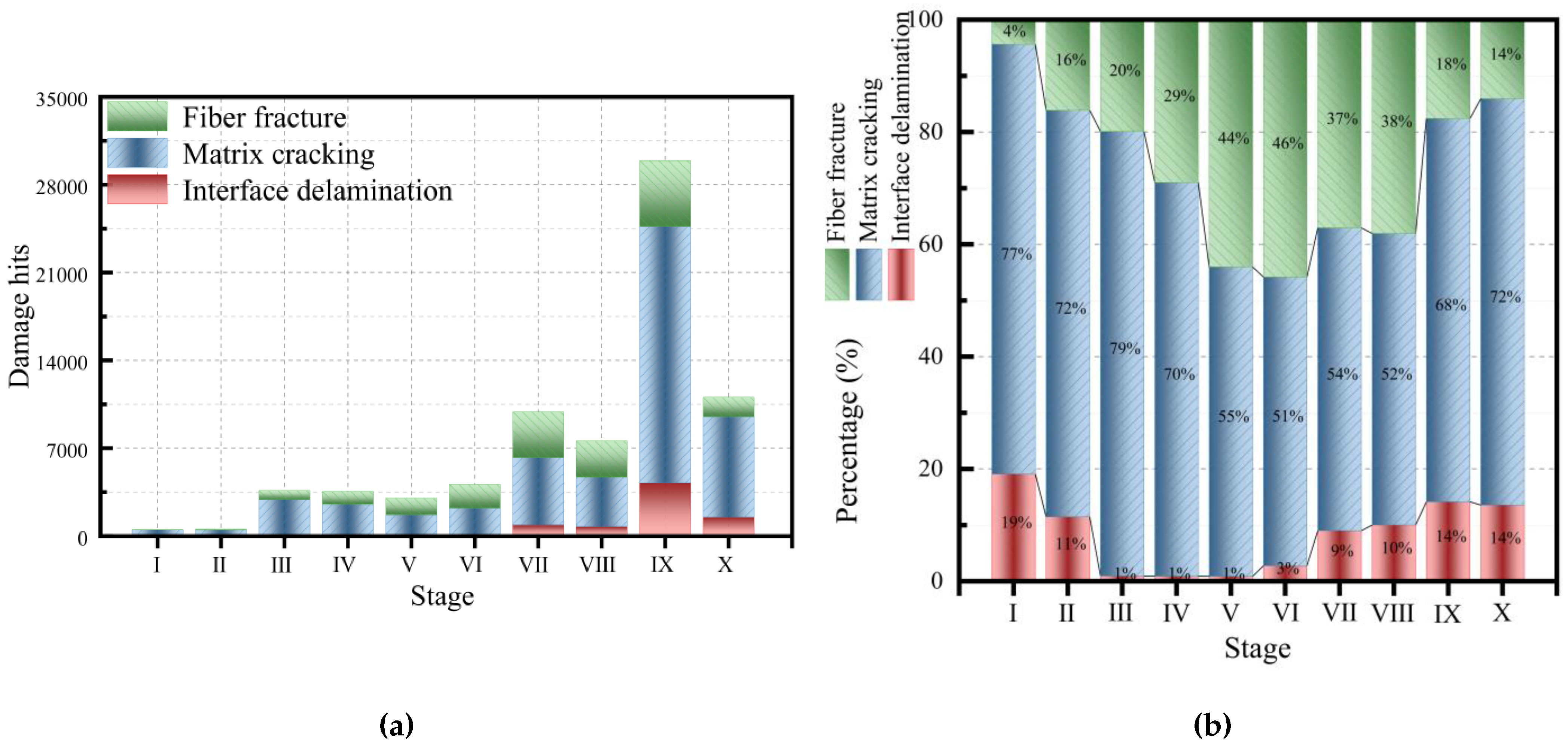
| Type | Stage 1 | Stage 2 | Stage 3 | Stage 4 | Stage 5 | Stage 6 | Stage 7 | Stage 8 | Stage 9 | Stage 10 |
|---|---|---|---|---|---|---|---|---|---|---|
| Damage hits | 503 | 558 | 3627 | 3549 | 3013 | 4109 | 9901 | 7568 | 29,932 | 11,058 |
| Interface delamination | 96 | 64 | 34 | 32 | 26 | 113 | 890 | 758 | 4231 | 1499 |
| Percentage/% | 19.085 | 11.470 | 0.937 | 0.902 | 0.863 | 2.750 | 8.989 | 10.016 | 14.135 | 13.556 |
| Matrix cracking | 385 | 404 | 2871 | 2488 | 1660 | 2113 | 5342 | 3929 | 20,439 | 8005 |
| Percentage/% | 76.541 | 72.401 | 79.156 | 70.104 | 55.095 | 51.424 | 53.954 | 51.916 | 68.285 | 72.391 |
| Fiber fracture | 22 | 90 | 722 | 1029 | 1327 | 1883 | 3669 | 2881 | 5262 | 1554 |
| Percentage/% | 4.374 | 16.129 | 19.906 | 28.994 | 44.042 | 45.826 | 37.057 | 38.068 | 17.580 | 14.053 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, P.; Li, H.; Yan, X.; Zhang, L.; Li, W. Identification of NOL-Ring Composite Materials’ Damage Mechanism Based on the STOA-VMD Algorithm. Polymers 2023, 15, 2647. https://doi.org/10.3390/polym15122647
Jiang P, Li H, Yan X, Zhang L, Li W. Identification of NOL-Ring Composite Materials’ Damage Mechanism Based on the STOA-VMD Algorithm. Polymers. 2023; 15(12):2647. https://doi.org/10.3390/polym15122647
Chicago/Turabian StyleJiang, Peng, Hui Li, Xiaowei Yan, Luying Zhang, and Wei Li. 2023. "Identification of NOL-Ring Composite Materials’ Damage Mechanism Based on the STOA-VMD Algorithm" Polymers 15, no. 12: 2647. https://doi.org/10.3390/polym15122647
APA StyleJiang, P., Li, H., Yan, X., Zhang, L., & Li, W. (2023). Identification of NOL-Ring Composite Materials’ Damage Mechanism Based on the STOA-VMD Algorithm. Polymers, 15(12), 2647. https://doi.org/10.3390/polym15122647





