Compaction of a Polymeric Membrane in Ultra-Low-Pressure Water Filtration
Abstract
:1. Introduction
2. Methodology
2.1. Material
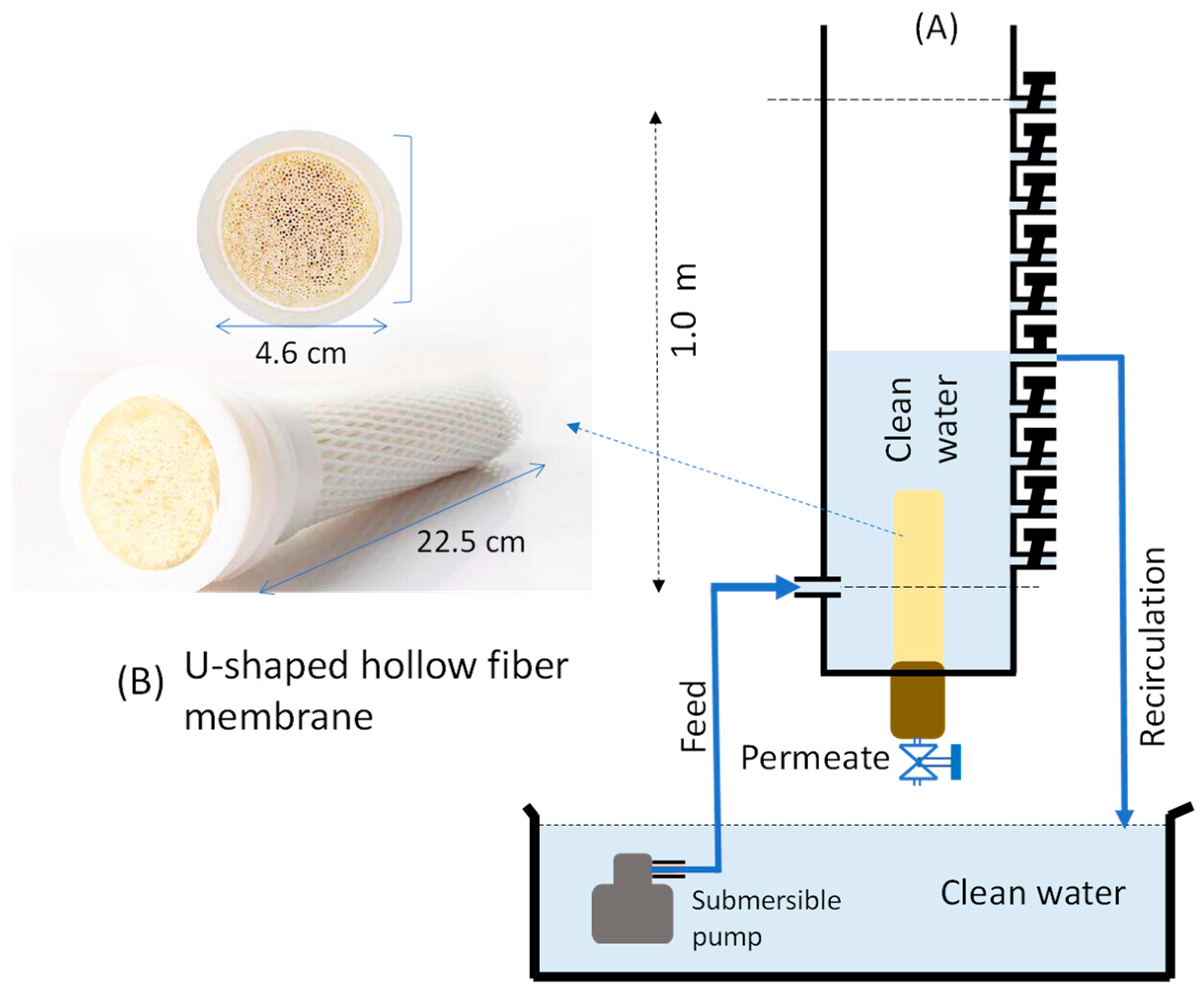
2.2. Filtration Setup
2.3. Filtration Test
2.3.1. Flux and Permeability
2.3.2. Instantaneous and Long-Term Compaction
2.3.3. Pressure Relaxation and Compaction Dynamics
3. Results and Discussion
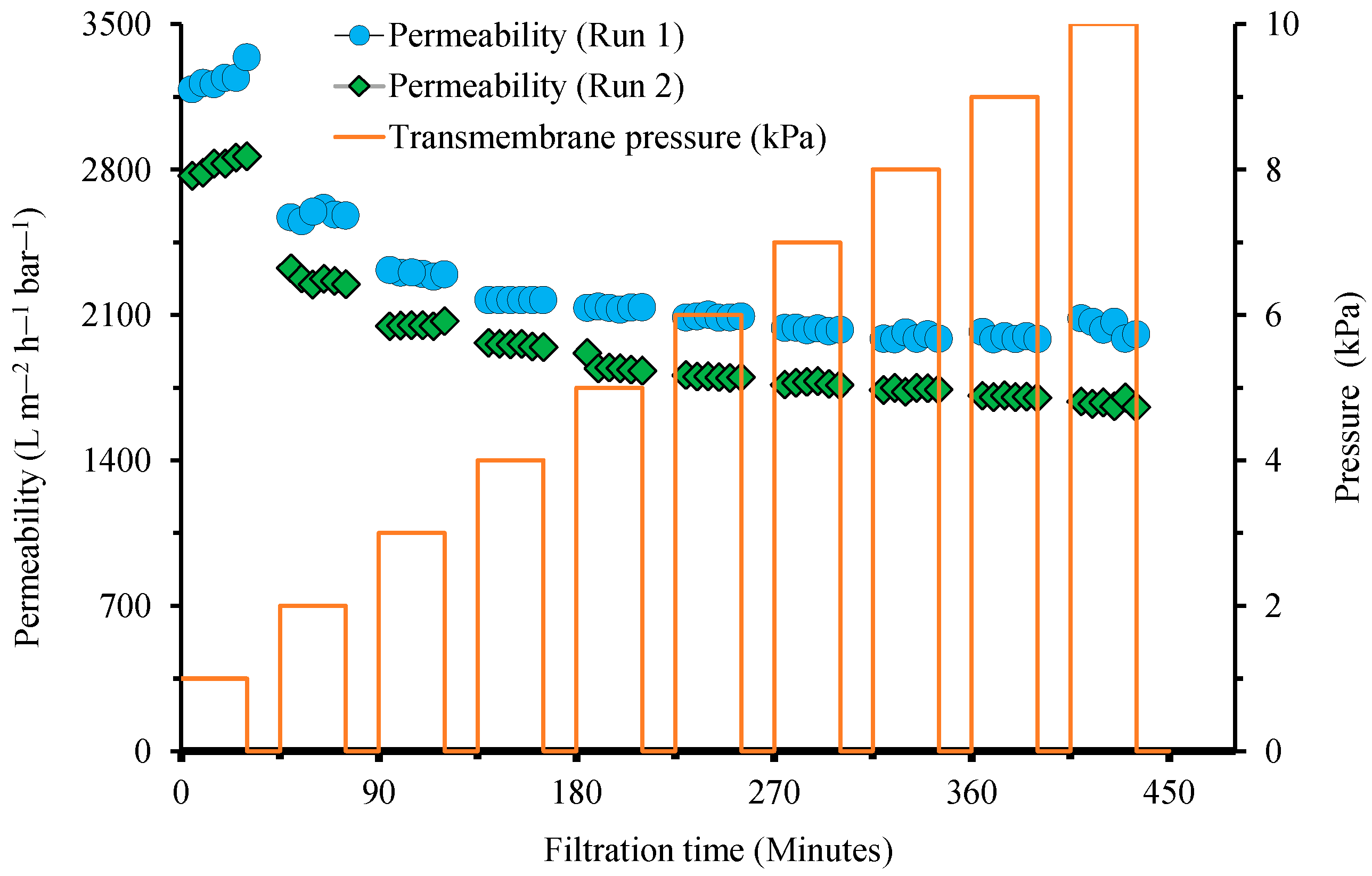
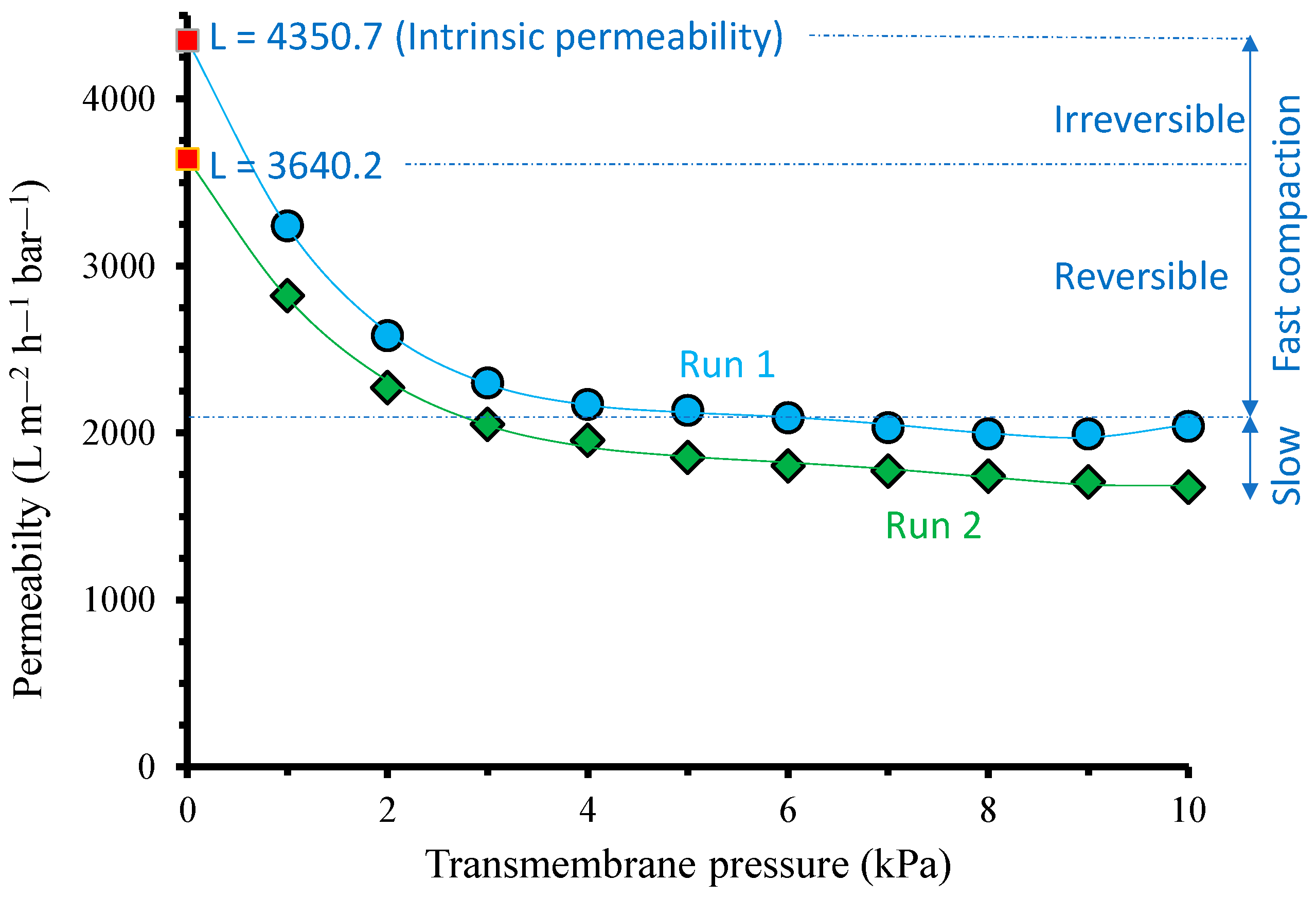
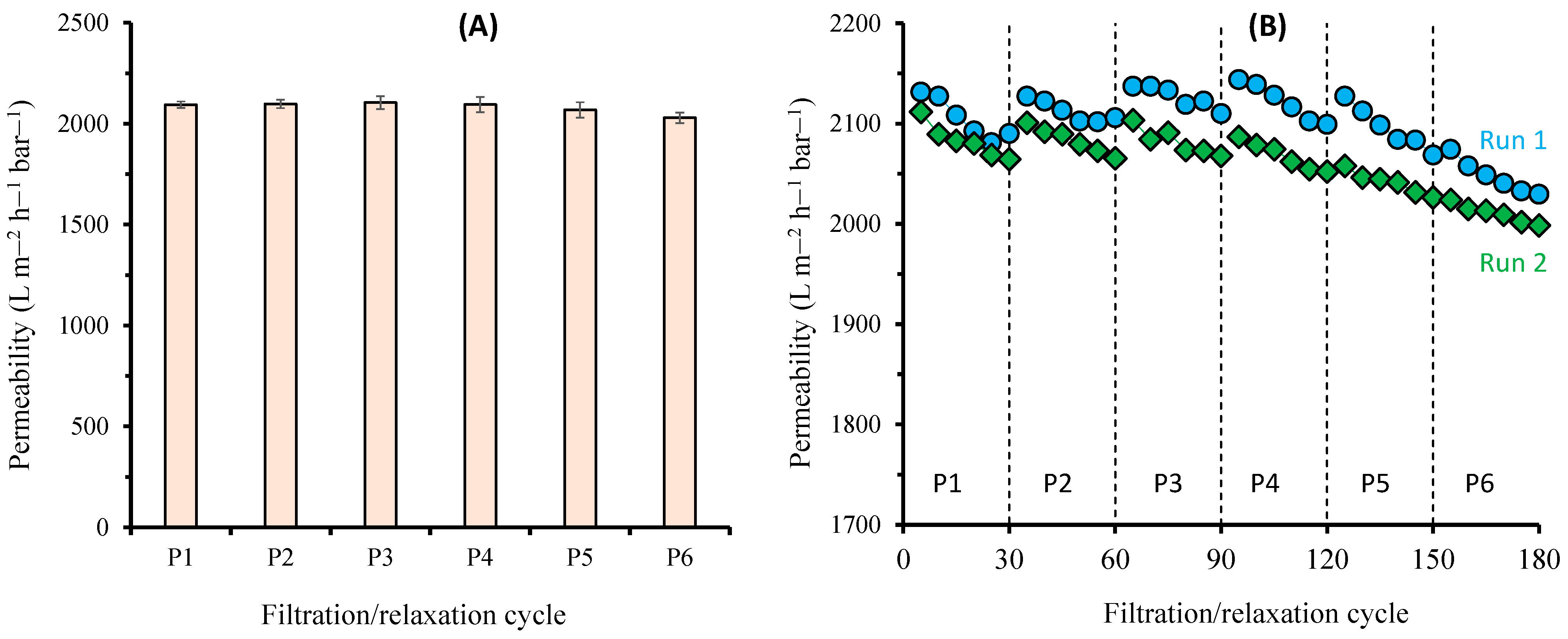
3.1. Instantaneous Compaction
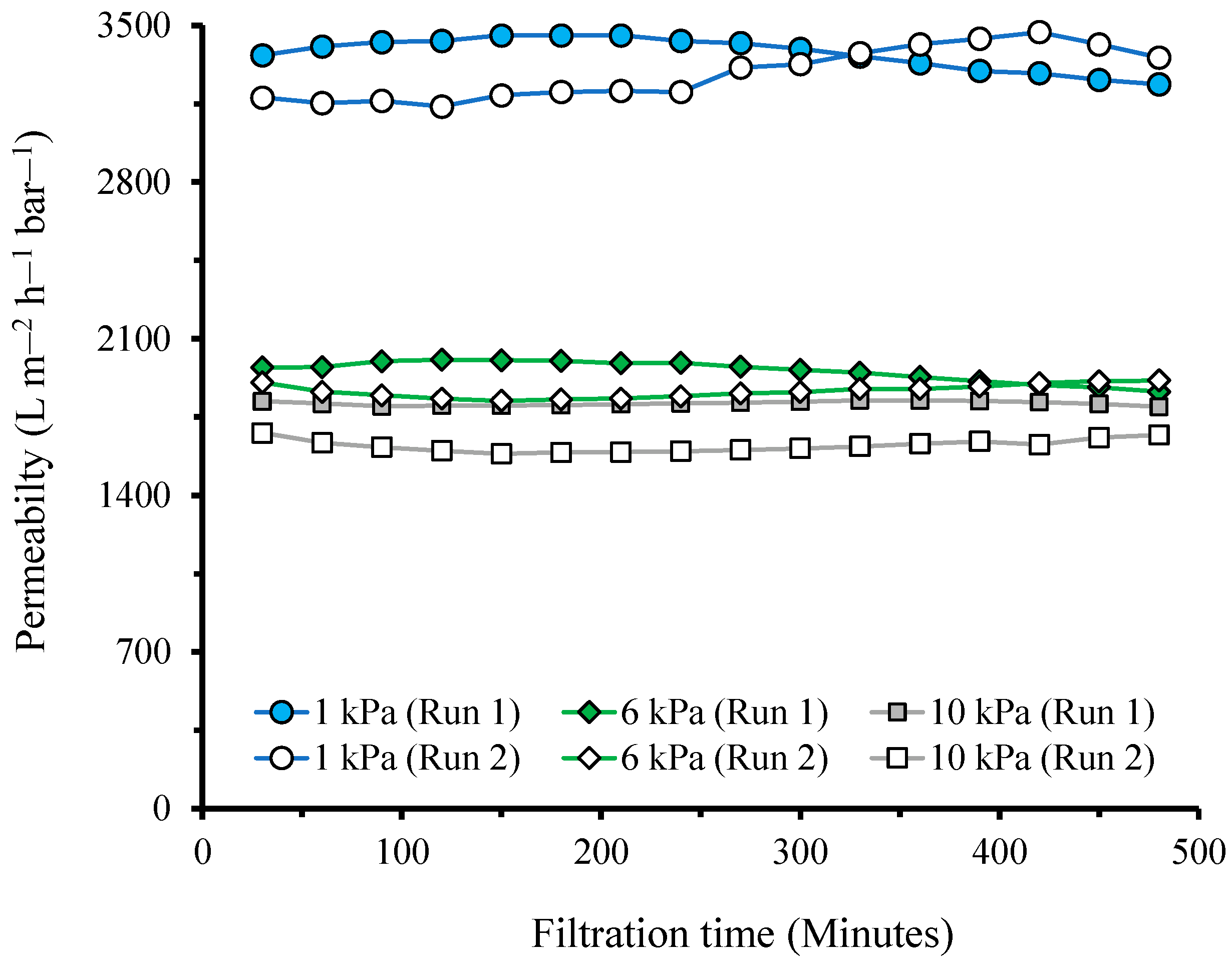
3.2. Slow Compaction
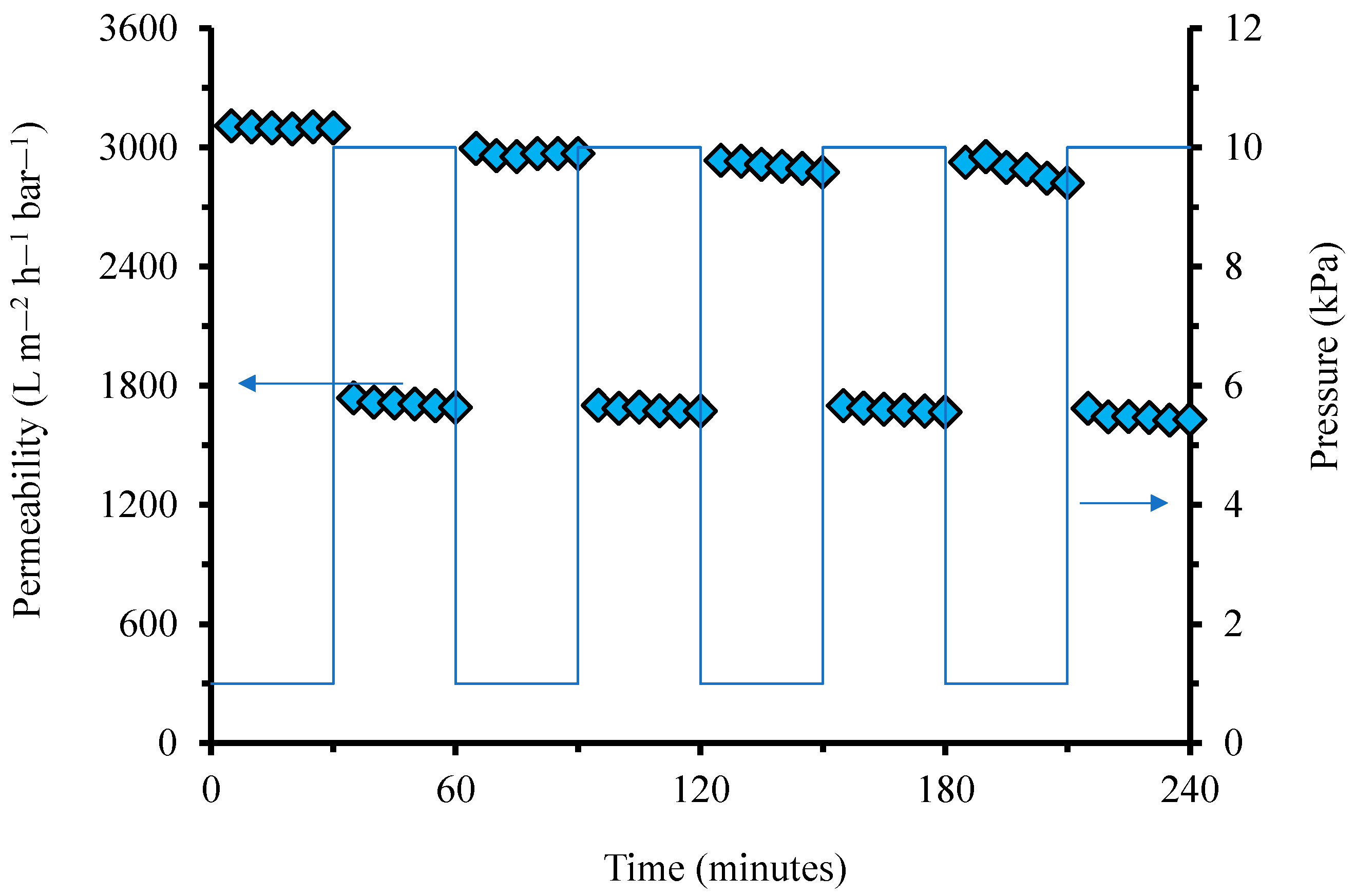
3.3. Compaction Dynamics
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Pronk, W.; Ding, A.; Morgenroth, E.; Derlon, N.; Desmond, P.; Burkhardt, M.; Wu, B.; Fane, A.G. Gravity-Driven Membrane Filtration for Water and Wastewater Treatment: A Review. Water Res. 2019, 149, 553–565. [Google Scholar] [CrossRef]
- Clasen, T.; Naranjo, J.; Frauchiger, D.; Gerba, C. Laboratory Assessment of a Gravity-Fed Ultrafiltration Water Treatment Device Designed for Household Use in Low-Income Settings. Am. J. Trop. Med. Hyg. 2009, 80, 819–823. [Google Scholar] [CrossRef]
- Peter-Varbanets, M.; Zurbrügg, C.; Swartz, C.; Pronk, W. Decentralized Systems for Potable Water and the Potential of Membrane Technology. Water Res. 2009, 43, 245–265. [Google Scholar] [CrossRef]
- Derlon, N.; Koch, N.; Eugster, B.; Posch, T.; Pernthaler, J.; Pronk, W.; Morgenroth, E. Activity of Metazoa Governs Biofilm Structure Formation and Enhances Permeate Flux during Gravity-Driven Membrane (GDM) Filtration. Water Res. 2013, 47, 2085–2095. [Google Scholar] [CrossRef]
- Derlon, N.; Peter-Varbanets, M.; Scheidegger, A.; Pronk, W.; Morgenroth, E. Predation Influences the Structure of Biofilm Developed on Ultrafiltration Membranes. Water Res. 2012, 46, 3323–3333. [Google Scholar] [CrossRef]
- Ding, A.; Song, R.; Cui, H.; Cao, H.; Ngo, H.H.; Chang, H.; Nan, J.; Li, G.; Ma, J. Presence of Powdered Activated Carbon/Zeolite Layer on the Performances of Gravity-Driven Membrane (GDM) System for Drinking Water Treatment: Ammonia Removal and Flux Stabilization. Sci. Total Environ. 2021, 799, 149415. [Google Scholar] [CrossRef] [PubMed]
- Schumann, P.; Andrade, J.A.O.; Jekel, M.; Ruhl, A.S. Packing Granular Activated Carbon into a Submerged Gravity-Driven Flat Sheet Membrane Module for Decentralized Water Treatment. J. Water Process Eng. 2020, 38, 101517. [Google Scholar] [CrossRef]
- Du, P.; Li, X.; Yang, Y.; Zhou, Z.; Fan, X.; Chang, H.; Liang, H. Regulated-Biofilms Enhance the Permeate Flux and Quality of Gravity-Driven Membrane (GDM) by in Situ Coagulation Combined with Activated Alumina Filtration. Water Res. 2022, 209, 117947. [Google Scholar] [CrossRef]
- Jonsson, G. Methods for Determining the Selectivity of Reverse Osmosis Membranes. Desalination 1977, 24, 19–37. [Google Scholar] [CrossRef]
- Persson, K.M.; Gekas, V.; Trägårdh, G. Study of Membrane Compaction and Its Influence on Ultrafiltration Water Permeability. J. Membr. Sci. 1995, 100, 155–162. [Google Scholar] [CrossRef]
- Platzer, N. Polymer Science and Engineering. J. Polym. Sci. B 1972, 10, 74. [Google Scholar] [CrossRef]
- Brinkert, L.; Abidine, N.; Aptel, P. On the Relation between Compaction and Mechanical Properties for Ultrafiltration Hollow Fibers. J. Membr. Sci. 1993, 77, 123–131. [Google Scholar] [CrossRef]
- Müller, N.; Handge, U.A.; Abetz, V. Physical Ageing and Lifetime Prediction of Polymer Membranes for Gas Separation Processes. J. Membr. Sci. 2016, 516, 33–46. [Google Scholar] [CrossRef]
- Murphy, T.M.; Freeman, B.D.; Paul, D.R. Physical Aging of Polystyrene Films Tracked by Gas Permeability. Polymer 2013, 54, 873–880. [Google Scholar] [CrossRef]
- Nielsen, L.E.; Landel, R.F. Mechanical Engineering. In Mechanical Properties of Polymers and Composites, 2nd ed.; CRC Press: Boca Raton, FL, USA, 1994; ISBN 978-0-8247-8964-0. [Google Scholar]
- Tessaro, I.; Jonsson, G.E. Ultrafiltration Membranes: The Effect of Compaction under Pressure and the Solute Dependence. Lat. Am. Appl. Res. 1998, 28, 229–233. [Google Scholar]
- Kallioinen, M.; Pekkarinen, M.; Mänttäri, M.; Nuortila-Jokinen, J.; Nyström, M. Comparison of the Performance of Two Different Regenerated Cellulose Ultrafiltration Membranes at High Filtration Pressure. J. Membr. Sci. 2007, 294, 93–102. [Google Scholar] [CrossRef]
- Zainuddin, N.I.; Bilad, M.R.; Marbelia, L.; Budhijanto, W.; Arahman, N.; Fahrina, A.; Shamsuddin, N.; Zaki, Z.I.; El-Bahy, Z.M.; Nandiyanto, A.B.D.; et al. Sequencing Batch Integrated Fixed-Film Activated Sludge Membrane Process for Treatment of Tapioca Processing Wastewater. Membranes 2021, 11, 875. [Google Scholar] [CrossRef]
- Wan Osman, W.N.A.; Mat Nawi, N.I.; Samsuri, S.; Bilad, M.R.; Khan, A.L.; Hunaepi, H.; Jaafar, J.; Lam, M.K. Ultra Low-Pressure Filtration System for Energy Efficient Microalgae Filtration. Heliyon 2021, 7, e07367. [Google Scholar] [CrossRef]
- Tarnawski, V.R.; Jelen, P. Estimation of Compaction and Fouling Effects during Membrane Processing of Cottage Cheese Whey. J. Food Eng. 1986, 5, 75–90. [Google Scholar] [CrossRef]
- Ebert, K.; Fritsch, D.; Koll, J.; Tjahjawiguna, C. Influence of Inorganic Fillers on the Compaction Behaviour of Porous Polymer Based Membranes. J. Membr. Sci. 2004, 233, 71–78. [Google Scholar] [CrossRef]
- Mosqueda-Jimenez, D.B.; Narbaitz, R.M.; Matsuura, T. Membrane Fouling Test: Apparatus Evaluation. J. Environ. Eng. 2004, 130, 90–99. [Google Scholar] [CrossRef]
- Bowen, W.R.; Gan, Q. Microfiltration of Protein Solutions at Thin Film Composite Membranes. J. Membr. Sci. 1993, 80, 165–173. [Google Scholar] [CrossRef]
- Di Profio, G.; Ji, X.; Curcio, E.; Drioli, E. Submerged Hollow Fiber Ultrafiltration as Seawater Pretreatment in the Logic of Integrated Membrane Desalination Systems. Desalination 2011, 269, 128–135. [Google Scholar] [CrossRef]
- Mehdizadeh, H.; Dickson, J.M.; Eriksson, P.K. Temperature Effects on the Performance of Thin-Film Composite, Aromatic Polyamide Membranes. Ind. Eng. Chem. Res. 1989, 28, 814–824. [Google Scholar] [CrossRef]
- Aghajani, M.; Wang, M.; Cox, L.M.; Killgore, J.P.; Greenberg, A.R.; Ding, Y. Influence of Support-Layer Deformation on the Intrinsic Resistance of Thin Film Composite Membranes. J. Membr. Sci. 2018, 567, 49–57. [Google Scholar] [CrossRef] [PubMed]
- Shi, Y.; Wang, Z.; Du, X.; Gong, B.; Jegatheesan, V.; Haq, I.U. Recent Advances in the Prediction of Fouling in Membrane Bioreactors. Membranes 2021, 11, 381. [Google Scholar] [CrossRef]
- AlSawaftah, N.; Abuwatfa, W.; Darwish, N.; Husseini, G. A Comprehensive Review on Membrane Fouling: Mathematical Modelling, Prediction, Diagnosis, and Mitigation. Water 2021, 13, 1327. [Google Scholar] [CrossRef]
- Judd, S.; Judd, C. The MBR Book: Principles and Applications of Membrane Bioreactors for Water and Wastewater Treatment, 2nd ed.; Butterworth-Heinemann: Oxford, UK, 2011; ISBN 978-0-08-096682-3. [Google Scholar]
- Szabo-Corbacho, M.A.; Pacheco-Ruiz, S.; Míguez, D.; Hooijmans, C.M.; Brdjanovic, D.; García, H.A.; van Lier, J.B. Influence of the Sludge Retention Time on Membrane Fouling in an Anaerobic Membrane Bioreactor (AnMBR) Treating Lipid-Rich Dairy Wastewater. Membranes 2022, 12, 262. [Google Scholar] [CrossRef]
- van der Marel, P.; Zwijnenburg, A.; Kemperman, A.; Wessling, M.; Temmink, H.; van der Meer, W. An Improved Flux-Step Method to Determine the Critical Flux and the Critical Flux for Irreversibility in a Membrane Bioreactor. J. Membr. Sci. 2009, 332, 24–29. [Google Scholar] [CrossRef]
- Fortunato, L.; Ranieri, L.; Naddeo, V.; Leiknes, T. Fouling Control in a Gravity-Driven Membrane (GDM) Bioreactor Treating Primary Wastewater by Using Relaxation and/or Air Scouring. J. Membr. Sci. 2020, 610, 118261. [Google Scholar] [CrossRef]
- Guðjónsdóttir, S.; Ge, L.; Zhao, K.; Lisak, G.; Wu, B. Gravity-Driven Membrane Filtration of Primary Wastewater Effluent for Edible Plant Cultivations: Membrane Performance and Health Risk Assessment. J. Environ. Chem. Eng. 2022, 10, 107046. [Google Scholar] [CrossRef]
- Lee, S.; Sutter, M.; Burkhardt, M.; Wu, B.; Chong, T.H. Biocarriers Facilitated Gravity-Driven Membrane (GDM) Reactor for Wastewater Reclamation: Effect of Intermittent Aeration Cycle. Sci. Total Environ. 2019, 694, 133719. [Google Scholar] [CrossRef]
- Shi, D.; Liu, Y.; Fu, W.; Li, J.; Fang, Z.; Shao, S. A Combination of Membrane Relaxation and Shear Stress Significantly Improve the Flux of Gravity-Driven Membrane System. Water Res. 2020, 175, 115694. [Google Scholar] [CrossRef] [PubMed]
- Lee, S.; Badoux, G.O.; Wu, B.; Chong, T.H. Enhancing Performance of Biocarriers Facilitated Gravity-Driven Membrane (GDM) Reactor for Decentralized Wastewater Treatment: Effect of Internal Recirculation and Membrane Packing Density. Sci. Total Environ. 2021, 762, 144104. [Google Scholar] [CrossRef]
- Derlon, N.; Desmond, P.; Rühs, P.A.; Morgenroth, E. Cross Flow Frequency Determines the Physical Structure and Cohesion of Membrane Biofilms Developed during Gravity-Driven Membrane Ultrafiltration of River Water: Implication for Hydraulic Resistance. J. Membr. Sci. 2022, 643, 120079. [Google Scholar] [CrossRef]
- Wan, H.; Mills, R.; Wang, Y.; Wang, K.; Xu, S.; Bhattacharyya, D.; Xu, Z. Gravity-Driven Electrospun Membranes for Effective Removal of Perfluoro-Organics from Synthetic Groundwater. J. Membr. Sci. 2022, 644, 120180. [Google Scholar] [CrossRef]
- Jiang, H.; Wang, P.; Zhao, Q.; Wang, Z.; Sun, X.; Chen, M.; Han, B.; Ma, J. Enhanced Water Permeance and Antifouling Performance of Gravity-Driven Ultrafiltration Membrane with in-Situ Formed Rigid Pore Structure. J. Membr. Sci. 2022, 644, 120154. [Google Scholar] [CrossRef]
- Jiang, H.; Zhao, Q.; Wang, P.; Ma, J.; Zhai, X. Improved Separation and Antifouling Properties of PVDF Gravity-Driven Membranes by Blending with Amphiphilic Multi-Arms Polymer PPG-Si-PEG. J. Membr. Sci. 2019, 588, 117148. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bilad, M.R.; Junaeda, S.R.; Khery, Y.; Nufida, B.A.; Shamsuddin, N.; Usman, A.; Violet, V. Compaction of a Polymeric Membrane in Ultra-Low-Pressure Water Filtration. Polymers 2022, 14, 3254. https://doi.org/10.3390/polym14163254
Bilad MR, Junaeda SR, Khery Y, Nufida BA, Shamsuddin N, Usman A, Violet V. Compaction of a Polymeric Membrane in Ultra-Low-Pressure Water Filtration. Polymers. 2022; 14(16):3254. https://doi.org/10.3390/polym14163254
Chicago/Turabian StyleBilad, Muhammad Roil, Siti Rahma Junaeda, Yusran Khery, Baiq Asma Nufida, Norazanita Shamsuddin, Anwar Usman, and Violet Violet. 2022. "Compaction of a Polymeric Membrane in Ultra-Low-Pressure Water Filtration" Polymers 14, no. 16: 3254. https://doi.org/10.3390/polym14163254
APA StyleBilad, M. R., Junaeda, S. R., Khery, Y., Nufida, B. A., Shamsuddin, N., Usman, A., & Violet, V. (2022). Compaction of a Polymeric Membrane in Ultra-Low-Pressure Water Filtration. Polymers, 14(16), 3254. https://doi.org/10.3390/polym14163254







