Optimizing the Conditions of Cationic Polyacrylamide Inverse Emulsion Synthesis Reaction to Obtain High–Molecular–Weight Polymers
Abstract
:1. Introduction
2. Materials and Methods
2.1. Chemicals
2.2. Preparation of CPAM (W/O Emulsions)
2.3. Determination of the Conversion and Molecular Weight of CPAM
2.4. Cationic Polyacrylamide Molecular Weight Analysis
2.5. Analysis of the Particle Size Distribution of CPAM
2.6. Structural Analysis
2.7. Statistical Analysis
3. Results and Discussion
3.1. Characterization of the CPAM
3.2. Optimal Parameters Affecting Polymerization by Response Surface Methodology
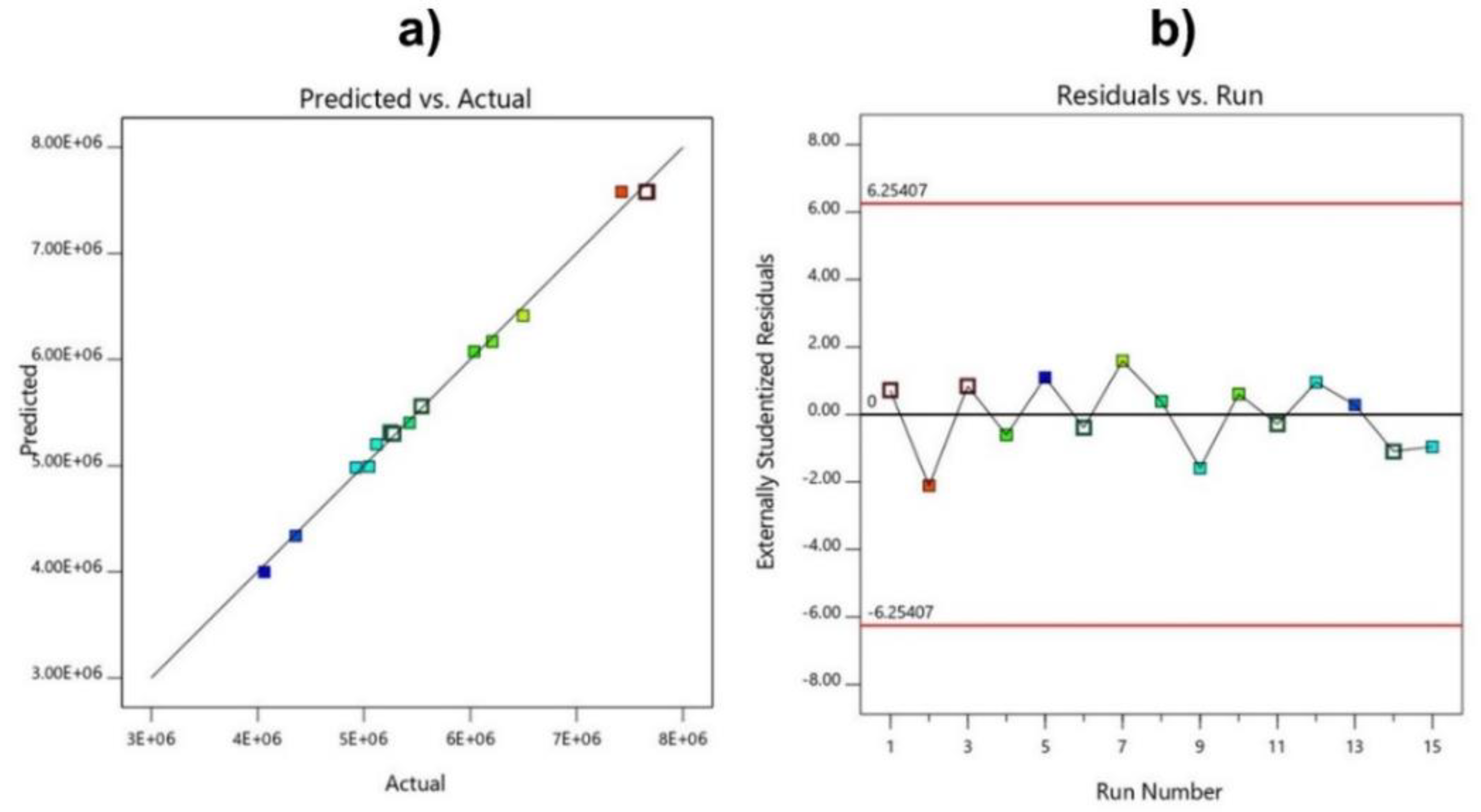
3.3. Verifying the Fit of the Model
3.4. Application of Response Surface Models
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gregory, J.; O’Melia, C.R. Fundamentals of flocculation. Crit. Rev. Environ. Control 1989, 19, 185–230. [Google Scholar] [CrossRef]
- Dao, V.H.; Cameron, N.R.; Saito, K. Synthesis, properties and performance of organic polymers employed in flocculation applications. Polym. Chem. 2016, 7, 11–25. [Google Scholar] [CrossRef] [Green Version]
- Wei, H.; Gao, B.; Ren, J.; Li, A.; Yang, H. Coagulation/flocculation in dewatering of sludge: A review. Water Res. 2018, 143, 608–631. [Google Scholar] [CrossRef]
- Teh, C.Y.; Budiman, P.M.; Shak, K.P.Y.; Wu, T.Y. Recent Advancement of Coagulation–Flocculation and Its Application in Wastewater Treatment. Ind. Eng. Chem. Res. 2016, 55, 4363–4389. [Google Scholar] [CrossRef]
- Lee, C.S.; Robinson, J.; Chong, M.F. A review on application of flocculants in wastewater treatment. Process Saf. Environ. Prot. 2014, 92, 489–508. [Google Scholar] [CrossRef]
- Jiang, Z.; Zhu, J. Cationic Polyacrylamide: Synthesis and Application in Sludge Dewatering Treatment. Asian J. Chem. 2014, 26, 629–633. [Google Scholar] [CrossRef]
- Ma, J.; Fu, K.; Jiang, L.; Ding, L.; Guan, Q.; Zhang, S.; Zhang, H.; Shi, J.; Fu, X. Flocculation performance of cationic polyacrylamide with high cationic degree in humic acid synthetic water treatment and effect of kaolin particles. Sep. Purif. Technol. 2017, 181, 201–212. [Google Scholar] [CrossRef]
- Guan, Q.; Zheng, H.; Zhai, J.; Zhao, C.; Zheng, X.; Tang, X.; Chen, W.; Sun, Y. Effect of template on structure and properties of cationic polyacrylamide: Characterization and mechanism. Ind. Eng. Chem. Res. 2014, 53, 5624–5635. [Google Scholar] [CrossRef]
- Sun, J.; Ma, X.; Li, X.; Fan, J.; Chen, Q.; Liu, X.; Pan, J. Synthesis of a Cationic Polyacrylamide under UV Initiation and Its Flocculation in Estrone Removal. Int. J. Polym. Sci. 2018, 2018, 1–11. [Google Scholar] [CrossRef] [Green Version]
- Zhao, C.; Zheng, H.; Gao, B.; Liu, Y.; Zhai, J.; Zhang, S.; Xu, B. Ultrasound–initiated synthesis of cationic polyacrylamide for oily wastewater treatment: Enhanced interaction between the flocculant and contaminants. Ultrason. Sonochem. 2018, 42, 31–41. [Google Scholar] [CrossRef]
- Zhu, J.; Zheng, H.; Jiang, Z.; Zhang, Z.; Liu, L.; Sun, Y.; Tshukudu, T. Synthesis and characterization of a dewatering reagent: Cationic polyacrylamide (P(AM–DMC–DAC)) for activated sludge dewatering treatment. Desalin. Water Treat. 2013, 51, 2791–2801. [Google Scholar] [CrossRef]
- Cheng, Z.; Dong, Z.; Su, M.; Zhang, Y.; Wang, Z.; He, P. Synthesis of cationic polyacrylamide via inverse emulsion polymerization method for the application in water treatment. J. Macromol. Sci. Part A 2019, 56, 76–85. [Google Scholar] [CrossRef]
- Nguyen, L.N.; Vu, H.P.; Fu, Q.; Abu Hasan Johir, M.; Ibrahim, I.; Mofijur, M.; Labeeuw, L.; Pernice, M.; Ralph, P.J.; Nghiem, L.D. Synthesis and evaluation of cationic polyacrylamide and polyacrylate flocculants for harvesting freshwater and marine microalgae. Chem. Eng. J. 2022, 433, 133623. [Google Scholar] [CrossRef]
- Gao, B.; Lv, Y.; Jiu, H. Synthesis and properties of cationic polyacrylamide containing pyridine quaternary salt. Polym. Int. 2003, 52, 1468–1473. [Google Scholar] [CrossRef]
- Liu, Y.; Zheng, H.; Wang, Y.; Zheng, X.; Wang, M.; Ren, J.; Zhao, C. Synthesis of a cationic polyacrylamide by a photocatalytic surface–initiated method and evaluation of its flocculation and dewatering performance: Nano–TiO2 as a photo initiator. RSC Adv. 2018, 8, 28329–28340. [Google Scholar] [CrossRef] [Green Version]
- Zhou, Y.; Zheng, H.; Wang, Y.; Zhao, R.; Liu, H.; Ding, W.; An, Y. Enhanced municipal sludge dewaterability using an amphiphilic microblocked cationic polyacrylamide synthesized through ultrasonic–initiation: Copolymerization and flocculation mechanisms. Colloids Surfaces A Physicochem. Eng. Asp. 2020, 594, 124645. [Google Scholar] [CrossRef]
- Yang, Z.L.; Gao, B.Y.; Li, C.X.; Yue, Q.Y.; Liu, B. Synthesis and characterization of hydrophobically associating cationic polyacrylamide. Chem. Eng. J. 2010, 161, 27–33. [Google Scholar] [CrossRef]
- Wan, X.; Li, Y.; Wang, X.; Chen, S.; Gu, X. Synthesis of cationic guar gum–graft–polyacrylamide at low temperature and its flocculating properties. Eur. Polym. J. 2007, 43, 3655–3661. [Google Scholar] [CrossRef]
- Sun, W.; Zhang, G.; Cao, H.; Li, H. Synthesis and flocculation properties of star–shaped cationic polyacrylamide. Asian J. Chem. 2013, 25, 7835–7839. [Google Scholar] [CrossRef]
- Qi, X.; Liu, J.; Wang, C.; Li, S.; Li, X.; Liang, Y.; Sarfaraz, K. Synthesis of the hydrophobic cationic polyacrylamide (PADD) initiated by ultrasonic and its flocculation and treatment of coal mine wastewater. Processes 2020, 8, 62. [Google Scholar] [CrossRef] [Green Version]
- Ma, J.; Shi, J.; Ding, H.; Zhu, G.; Fu, K.; Fu, X. Synthesis of cationic polyacrylamide by low–pressure UV initiation for turbidity water flocculation. Chem. Eng. J. 2017, 312, 20–29. [Google Scholar] [CrossRef]
- Liao, Y.; Zheng, H.; Qian, L.; Sun, Y.; Dai, L.; Xue, W. UV–initiated polymerization of hydrophobically associating cationic polyacrylamide modified by a surface–active monomer: A comparative study of synthesis, characterization, and sludge dewatering performance. Ind. Eng. Chem. Res. 2014, 53, 11193–11203. [Google Scholar] [CrossRef]
- Lee, K.E.; Poh, B.T.; Morad, N.; Teng, T.T. Synthesis and characterization of hydrophobically modified cationic polyacrylamide with low concentration of cationic monomer. J. Macromol. Sci. Part A Pure Appl. Chem. 2009, 46, 240–249. [Google Scholar] [CrossRef]
- Zheng, H.L.; Zhu, J.R.; Jiang, Z.Z.; Ji, F.Y.; Tan, M.Z.; Sun, Y.J.; Miao, S.X.; Zheng, X.K. Research on Preparation and Application of Dewatering Agents for Tailings Water Treatment. Adv. Mater. Res. 2011, 414, 172–178. [Google Scholar] [CrossRef]
- Li, W.; Zhao, C.; Zheng, H.; Ding, J.; Hao, S.; Zhou, Y.; Li, X. Review of the Template Copolymerization of Cationic Polyacrylamide. Mini. Rev. Org. Chem. 2018, 15, 141–147. [Google Scholar] [CrossRef]
- Barari, M.; Elahi, M.A.H.D.I.A.; Hemati, M. Synthesis And Characterization Of High Molecular Weight Polyacrylamide Nanoparticles By Inverse-Emulsion Polymerization. Iran. Polym. J. 2011, 20, 65–76. [Google Scholar]
- Mohsin, M.A.; Attia, N.F. Inverse Emulsion Polymerization for the Synthesis of High Molecular Weight Polyacrylamide and Its Application as Sand Stabilizer. Int. J. Polym. Sci. 2015, 2015, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Karimifard, S.; Alavi Moghaddam, M.R. Application of response surface methodology in physicochemical removal of dyes from wastewater: A critical review. Sci. Total Environ. 2018, 640, 772–797. [Google Scholar] [CrossRef]
- Behbahani, M.; Moghaddam, M.R.A.; Arami, M. Techno–economical evaluation of fluoride removal by electrocoagulation process: Optimization through response surface methodology. Desalination 2011, 271, 209–218. [Google Scholar] [CrossRef]
- Berkani, M.; Kadmi, Y.; Bouchareb, M.K.; Bouhelassa, M.; Bouzaza, A. Combinatıon of a Box–Behnken design technique with response surface methodology for optimization of the photocatalytic mineralization of C.I. Basic Red 46 dye from aqueous solution. Arab. J. Chem. 2020, 13, 8338–8346. [Google Scholar] [CrossRef]
- Kim, S.C. Application of response surface method as an experimental design to optimize coagulation–flocculation process for pre–treating paper wastewater. J. Ind. Eng. Chem. 2016, 38, 93–102. [Google Scholar] [CrossRef]
- Liu, Z.; Wei, Y.; Li, B.; He, N. Synthesis of cationic polyacrylamide by aqueous two-phase polymerization in poly(ethylene glycol) chloride solution. J. Appl. Polym. Sci. 2013, 127, 593–598. [Google Scholar] [CrossRef]
- Rattanakawin, C.; Hogg, R. Viscosity behavior of polymeric flocculant solutions. Miner. Eng. 2007, 20, 1033–1038. [Google Scholar] [CrossRef]
- Ma, J.; Fu, K.; Fu, X.; Guan, Q.; Ding, L.; Shi, J.; Zhu, G.; Zhang, X.; Zhang, S.; Jiang, L. Flocculation properties and kinetic investigation of polyacrylamide with different cationic monomer content for high turbid water purification. Sep. Purif. Technol. 2017, 182, 134–143. [Google Scholar] [CrossRef]
- Yang, K.; Chen, J.; Yao, C. Cationic polyacrylamide emulsion with ultra-high concentration as a flocculant for paper mill wastewater treatment. BioResources 2020, 15, 3173–3189. [Google Scholar] [CrossRef]
- Djibrine, B.Z.; Zheng, H.; Wang, M.; Liu, S.; Tang, X.; Khan, S.; Jimenéz, A.N.; Feng, L. An effective flocculation method to the kaolin wastewater treatment by a cationic polyacrylamide (CPAM): Preparation, characterization, and flocculation performance. Int. J. Polym. Sci. 2018, 2018, 1–12. [Google Scholar] [CrossRef]
- Birjandi, N.; Younesi, H.; Bahramifar, N.; Ghafari, S.; Zinatizadeh, A.A.; Sethupathi, S. Optimization of coagulation-flocculation treatment on paper-recycling wastewater: Application of response surface methodology. J. Environ. Sci. Health Part A Toxic/Hazardous Subst. Environ. Eng. 2013, 48, 1573–1582. [Google Scholar] [CrossRef]
- Wang, Y.; Chen, K.; Mo, L.; Li, J.; Xu, J. Optimization of coagulation-flocculation process for papermaking-reconstituted tobacco slice wastewater treatment using response surface methodology. J. Ind. Eng. Chem. 2014, 20, 391–396. [Google Scholar] [CrossRef]
- Vohra, A.; Satyanarayana, T. Statistical optimization of the medium components by response surface methodology to enhance phytase production by Pichia anomala. Process Biochem. 2002, 37, 999–1004. [Google Scholar] [CrossRef]
- Muller, G. Thermal stability of polyacrylamide solutions: Effect of residual impurities in the molecular-weight-degradation process upon heating. Polym. Bull. 1981, 5, 39–45. [Google Scholar] [CrossRef]
- Al-Muntasheri, G.A.; Nasr-El-Din, H.A.; Peters, J.A.; Zitha, P.L.J. Thermal decomposition and hydrolysis of polyacrylamide-co-tert-butyl acrylate. Eur. Polym. J. 2008, 44, 1225–1237. [Google Scholar] [CrossRef]
- Kitahara, Y.; Okuyama, K.; Ozawa, K.; Suga, T.; Takahashi, S.; Fujii, T. Thermal decomposition of acrylamide from polyacrylamide: Time-resolved pyrolysis with ion-attachment mass spectrometry. J. Therm. Anal. Calorim. 2012, 110, 423–429. [Google Scholar] [CrossRef]
- Leung, W.M.; Axelson, D.E.; Van Dyke, J.D. Thermal degradation of polyacrylamide and poly (acrylamide-co-acrylate). J. Polym. Sci. Part A Polym. Chem. 1987, 25, 1825–1846. [Google Scholar] [CrossRef]
- Schmidt, C.U.; Reichert, K.H. Reaction calorimeter a contribution to safe operation of exothermic polymerizations. Chem. Eng. Sci. 1988, 43, 2133–2137. [Google Scholar] [CrossRef]

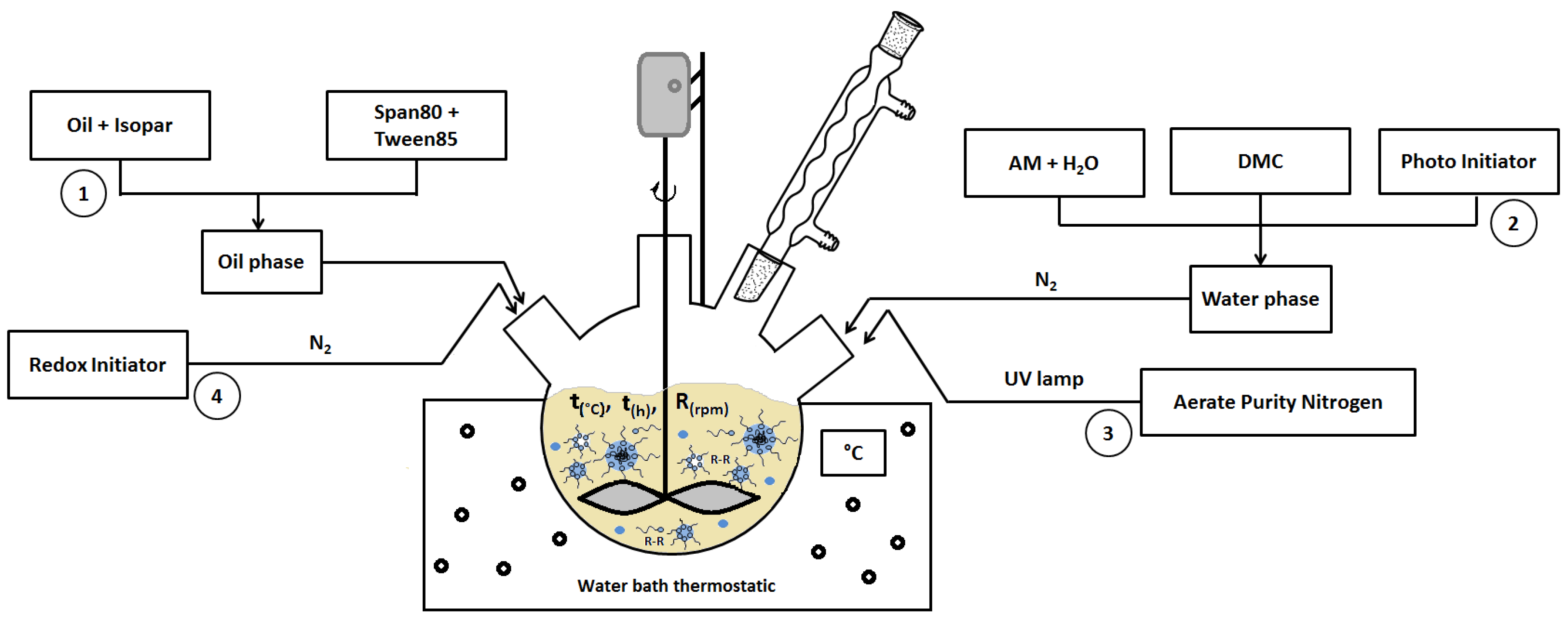
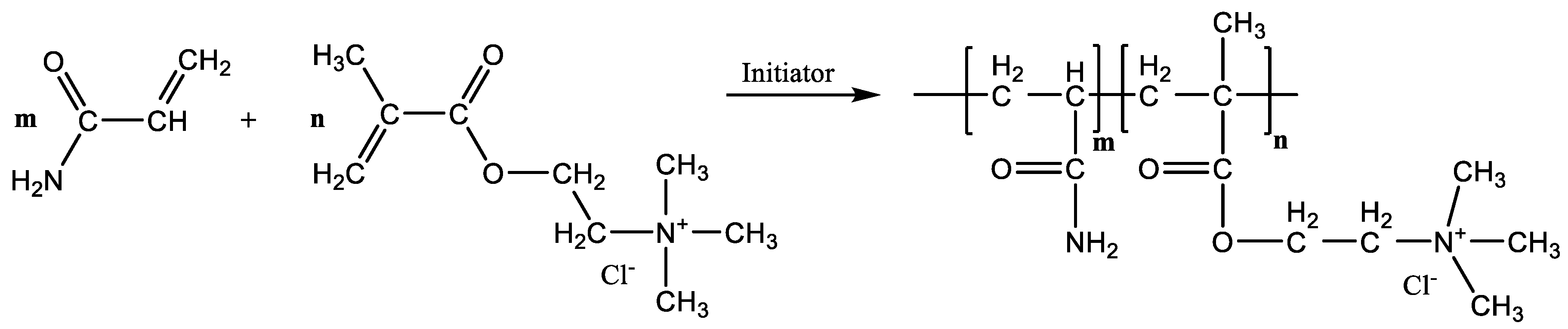
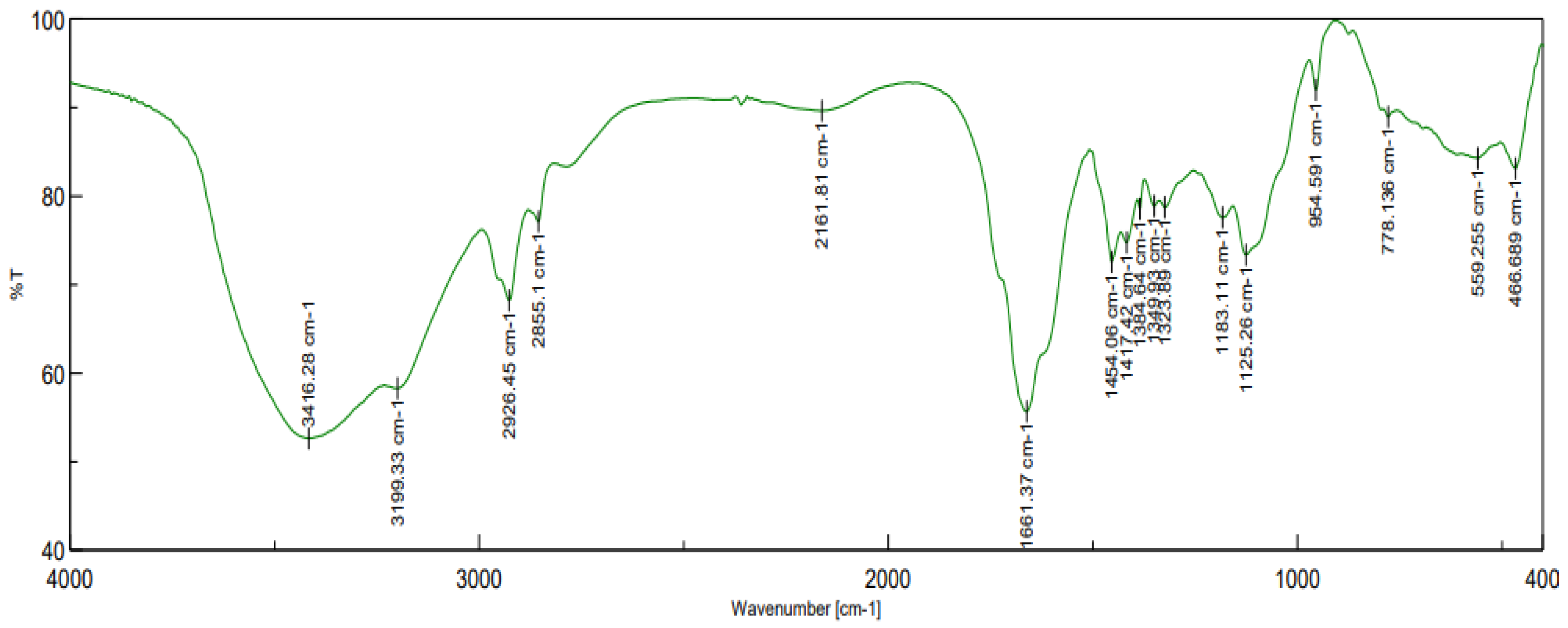

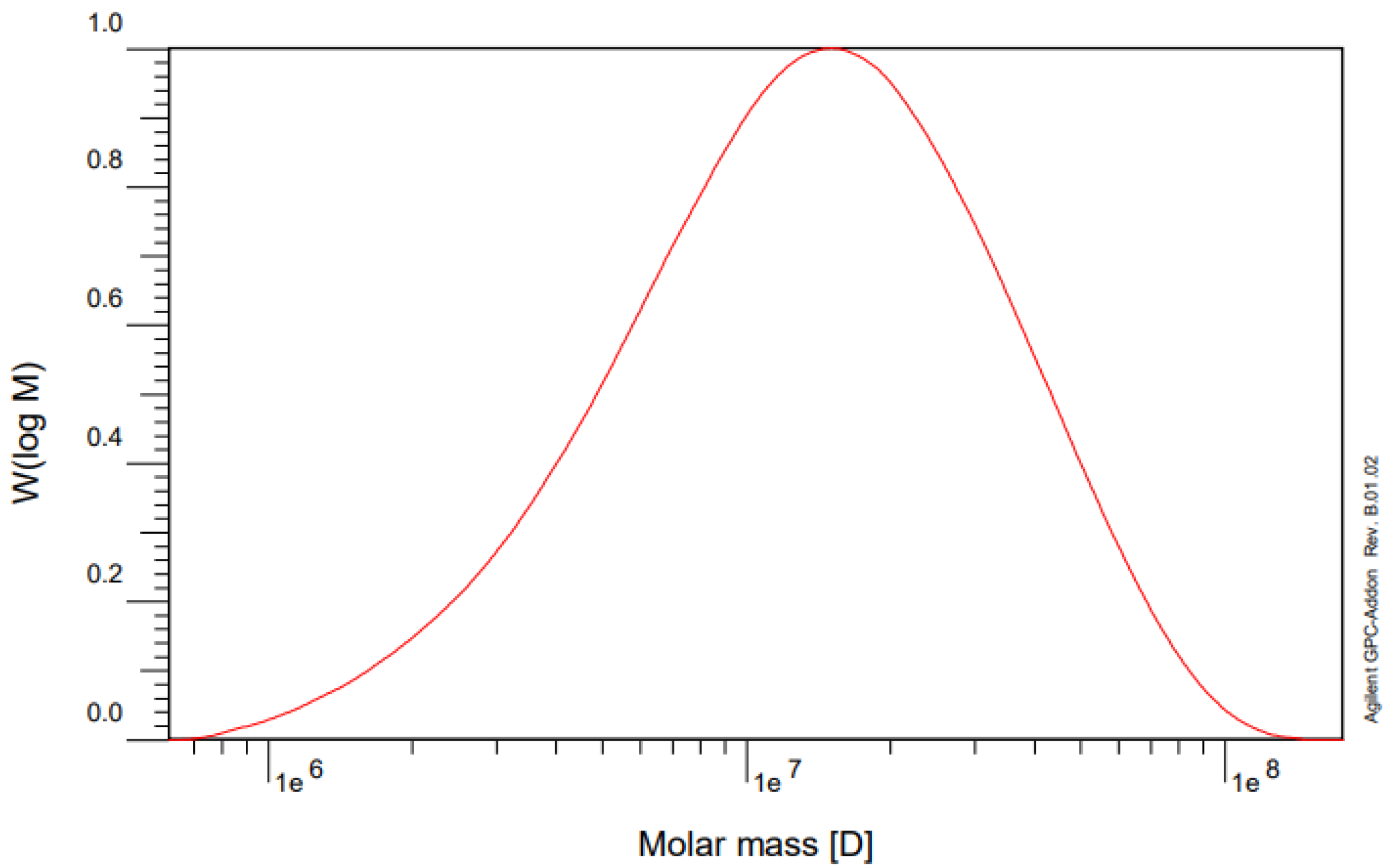




| Reaction No. | Stirring Speed (rpm) | Temperature (°C) | Time (h) | Molecular Weight (Da) | Conversion (%) |
|---|---|---|---|---|---|
| 1 | 2000 | 55 | 7 | 4.06 × 106 | 70.23 |
| 2 | 3000 | 55 | 7 | 5.05 × 106 | 75.43 |
| 3 | 2000 | 65 | 7 | 4.92 × 106 | 83.32 |
| 4 | 3000 | 65 | 7 | 5.25 × 106 | 85.46 |
| 5 | 2000 | 60 | 6 | 4.36 × 106 | 79.32 |
| 6 | 3000 | 60 | 6 | 5.43 × 106 | 86.54 |
| 7 | 2000 | 60 | 8 | 5.28 × 106 | 85.67 |
| 8 | 3000 | 60 | 8 | 5.54 × 106 | 86.90 |
| 9 | 2500 | 55 | 6 | 5.12 × 106 | 63.32 |
| 10 | 2500 | 65 | 6 | 6.21 × 106 | 76.98 |
| 11 | 2500 | 55 | 8 | 6.04 × 106 | 70.34 |
| 12 | 2500 | 65 | 8 | 6.50 × 106 | 78.21 |
| 13 | 2500 | 60 | 7 | 7.67 × 106 | 95.01 |
| 14 | 2500 | 60 | 7 | 7.65 × 106 | 95.34 |
| 15 | 2500 | 60 | 7 | 7.42 × 106 | 94.98 |
| Source | Sum of Squares | Df | Mean Squares | F-Value | p-Value | |
|---|---|---|---|---|---|---|
| Model | 1.79 × 1013 | 9 | 1.98 × 1012 | 137.81 | <0.0001 | Significant |
| A | 8.76 × 1011 | 1 | 8.76 × 1011 | 60.89 | 0.0006 | |
| B | 8.53 × 1011 | 1 | 8.53 × 1011 | 59.29 | 0.0006 | |
| C | 6.27 × 1011 | 1 | 6.27 × 1011 | 43.54 | 0.0012 | |
| AB | 1.09 × 1011 | 1 | 1.09 × 1011 | 7.59 | 0.0401 | |
| AC | 1.67 × 1011 | 1 | 1.67 × 1011 | 11.30 | 0.0201 | |
| BC | 9.90 × 1010 | 1 | 9.90 × 1010 | 6.88 | 0.0469 | |
| A2 | 1.18 × 1010 | 1 | 1.18 × 1013 | 819.00 | <0.0001 | |
| B2 | 3.50 × 1010 | 1 | 3.50 × 1013 | 242.92 | <0.0001 | |
| C2 | 1.53 × 1012 | 1 | 1.53 × 1012 | 106.07 | 0.0001 | |
| Residual | 7.19 × 1010 | 5 | 1.44 × 1010 | |||
| Lack of Fit | 3.40 × 1010 | 3 | 1.13 × 1010 | 0.5968 | 0.6754 | Not significant |
| Pure Error | 3.80 × 1010 | 2 | 1.90 × 1010 | |||
| Cor Total | 1.79 × 1013 | 14 |
| Std. Dev. | 1.20 × 105 | R2 | 0.9960 |
| Mean | 5.77 × 106 | Adjusted R2 | 0.9888 |
| C.V.% | 2.08 | Predicted R2 | 0.9649 |
| Adequate Precision | 36.57 |
| Source | Sum of Squares | Df | Mean Squares | F-Value | p-Value | |
|---|---|---|---|---|---|---|
| Model | 1299.42 | 9 | 144.38 | 853.03 | <0.0001 | Significant |
| A | 31.17 | 1 | 31.17 | 184.13 | <0.0001 | |
| B | 249.20 | 1 | 249.20 | 1472.35 | <0.0001 | |
| C | 27.98 | 1 | 27.98 | 165.28 | <0.0001 | |
| AB | 2.34 | 1 | 2.34 | 13.83 | 0.0137 | |
| AC | 8.97 | 1 | 8.97 | 53.00 | 0.0008 | |
| BC | 8.38 | 1 | 8.38 | 49.52 | 0.0009 | |
| A2 | 15.55 | 1 | 15.55 | 91.90 | 0.0002 | |
| B2 | 770.70 | 1 | 770.70 | 4553.46 | <0.0001 | |
| C2 | 263.64 | 1 | 263.64 | 1557.65 | <0.0001 | |
| Residual | 0.8463 | 5 | 0.1693 | |||
| Lack of Fit | 0.7665 | 3 | 0.2555 | 6.40 | 0.1381 | not significant |
| Pure Error | 0.0798 | 2 | 0.0399 | |||
| Cor Total | 1300.26 | 14 |
| Std. Dev. | 0.4114 | R2 | 0.9993 |
| Mean | 81.80 | Adjusted R2 | 0.9982 |
| C.V. % | 0.5029 | Predicted R2 | 0.9904 |
| Adequate Precision | 94.6566 |
| No. | Stirring Speed (rpm) | Temperature (°C) | Reaction Time (h) | Verified Experiment | Predicted | ||
|---|---|---|---|---|---|---|---|
| Mw (Da) | Conversion (%) | Mw (Da) | Conversion (%) | ||||
| 1 | 2400 | 60 | 7 | 7,325,663 | 94.99 | 7,475,073 | 95.36 |
| 2 | 2400 | 61 | 7 | 7,357,949 | 95.47 | 7,508,111 | 95.94 |
| 3 | 2400 | 62 | 7 | 7,315,272 | 95.16 | 7,463,313 | 95.37 |
| 4 | 2500 | 60 | 7 | 7,480,452 | 95.07 | 7,612,678 | 95.86 |
| 5 | 2500 | 61 | 7 | 7,486,342 | 95.65 | 7,639,107 | 96.42 |
| 6 | 2500 | 62 | 7 | 7,445,982 | 95.05 | 7,587,700 | 95.82 |
| 7 | 2600 | 60 | 7 | 7,465,625 | 95.92 | 7,607,362 | 96.21 |
| 8 | 2600 | 61 | 7 | 7,474,824 | 95.96 | 7,627,182 | 96.73 |
| 9 | 2600 | 62 | 7 | 7,437,792 | 95.33 | 7,569,166 | 96.10 |
| 10 | 2400 | 60 | 6.5 | 7,002,888 | 90.43 | 7,152,600 | 92.17 |
| 11 | 2400 | 61 | 6.5 | 7,052,313 | 91.92 | 7,201,373 | 92.89 |
| 12 | 2400 | 62 | 6.5 | 7,028,117 | 91.57 | 7,172,309 | 92.47 |
| 13 | 2500 | 60 | 6.5 | 7,163,192 | 92.08 | 7,310,367 | 92.82 |
| 14 | 2500 | 61 | 6.5 | 7,207,192 | 92.67 | 7,352,530 | 93.52 |
| 15 | 2500 | 62 | 6.5 | 7,170,218 | 91.78 | 7,316,858 | 93.06 |
| 16 | 2600 | 60 | 6.5 | 7,173,829 | 92.14 | 7,325,213 | 93.32 |
| 17 | 2600 | 61 | 6.5 | 7,217,162 | 93.01 | 7,360,767 | 93.98 |
| 18 | 2600 | 62 | 6.5 | 7,171,252 | 92.75 | 7,318,486 | 93.50 |
| 19 | 2400 | 60 | 7.5 | 7,326,574 | 92.57 | 7,476,096 | 94.32 |
| 20 | 2400 | 61 | 7.5 | 7,343,532 | 93.87 | 7,493,400 | 94.76 |
| 21 | 2400 | 62 | 7.5 | 7,284,210 | 93.29 | 7,432,867 | 94.04 |
| 22 | 2500 | 60 | 7.5 | 7,342,719 | 93.33 | 7,593,539 | 94.68 |
| 23 | 2500 | 61 | 7.5 | 7,358,101 | 92.33 | 7,604,233 | 95.09 |
| 24 | 2500 | 62 | 7.5 | 7,386,350 | 92.99 | 7,537,092 | 94.34 |
| 25 | 2600 | 60 | 7.5 | 7,386,701 | 93.20 | 7,568,062 | 94.87 |
| 26 | 2600 | 61 | 7.5 | 7,390,704 | 94.11 | 7,572,147 | 95.25 |
| 27 | 2600 | 62 | 7.5 | 7,348,429 | 93.82 | 7,498,397 | 94.47 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nguyen, T.H.; Nguyen, N.T.; Nguyen, T.T.P.; Doan, N.T.; Tran, L.A.T.; Nguyen, L.P.D.; Bui, T.T. Optimizing the Conditions of Cationic Polyacrylamide Inverse Emulsion Synthesis Reaction to Obtain High–Molecular–Weight Polymers. Polymers 2022, 14, 2866. https://doi.org/10.3390/polym14142866
Nguyen TH, Nguyen NT, Nguyen TTP, Doan NT, Tran LAT, Nguyen LPD, Bui TT. Optimizing the Conditions of Cationic Polyacrylamide Inverse Emulsion Synthesis Reaction to Obtain High–Molecular–Weight Polymers. Polymers. 2022; 14(14):2866. https://doi.org/10.3390/polym14142866
Chicago/Turabian StyleNguyen, Tung Huy, Nhung Thi Nguyen, Thao Thi Phuong Nguyen, Ngoc Thi Doan, Lam Anh Thi Tran, Linh Pham Duy Nguyen, and Thanh Tien Bui. 2022. "Optimizing the Conditions of Cationic Polyacrylamide Inverse Emulsion Synthesis Reaction to Obtain High–Molecular–Weight Polymers" Polymers 14, no. 14: 2866. https://doi.org/10.3390/polym14142866
APA StyleNguyen, T. H., Nguyen, N. T., Nguyen, T. T. P., Doan, N. T., Tran, L. A. T., Nguyen, L. P. D., & Bui, T. T. (2022). Optimizing the Conditions of Cationic Polyacrylamide Inverse Emulsion Synthesis Reaction to Obtain High–Molecular–Weight Polymers. Polymers, 14(14), 2866. https://doi.org/10.3390/polym14142866






