A Semi-Analytical Model to Predict Infusion Time and Reinforcement Thickness in VARTM and SCRIMP Processes
Abstract
1. Introduction
2. Numerical Model
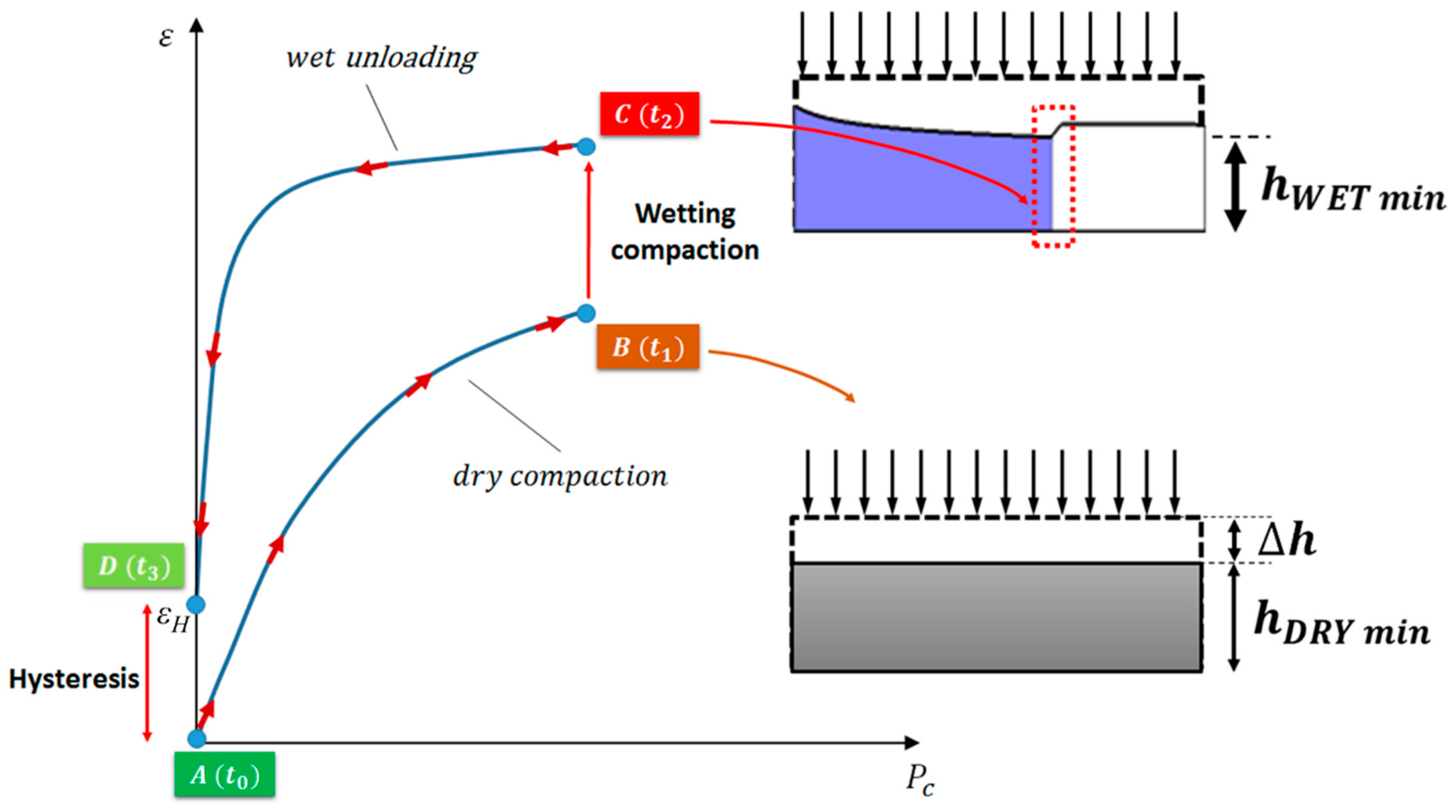
- Test 1: un-deformable preform without distribution network, assuming thickness, fiber volume fraction and permeability corresponding to a completely relaxed preform;
- Test 2: deformable preform without distribution network, considering variable thickness, fiber volume fraction and permeability as a function of the local compacting pressure;
- Test 3: un-deformable preform with distribution network, assuming thickness, fiber volume fraction and permeability corresponding to a completely relaxed preform;
- Test 4: deformable preform with distribution network, considering variable thickness, fiber volume fraction and permeability as a function of the local compacting pressure.
3. Results and Discussion
3.1. VARTM Process
3.2. SCRIMP Process
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Harshe, R. A review on advanced out-of-autoclave composites processing. J. Indian Inst. Sci. 2015, 95, 207–220. [Google Scholar]
- Michaud, V. A Review of Non-saturated Resin Flow in Liquid Composite Moulding processes. Transp. Porous Media 2016, 115, 581–601. [Google Scholar] [CrossRef]
- Summerscales, J.; Searle, T.J. Low-pressure (vacuum infusion) techniques for moulding large composite structures. Proc. Inst. Mech. Eng. Part L J. Mater. Des. Appl. 2005, 219, 45–58. [Google Scholar] [CrossRef]
- Correia, N.C.; Robitaille, F.; Long, A.C.; Rudd, C.D.; Šimáček, P.; Advani, S.G. Analysis of the vacuum infusion moulding process: I. Analytical formulation. Compos. Part A Appl. Sci. Manuf. 2005, 36, 1645–1656. [Google Scholar] [CrossRef]
- Bayldon, J.M.; Daniel, I.M. Flow modeling of the VARTM process including progressive saturation effects. Compos. Part A Appl. Sci. Manuf. 2009, 40, 1044–1052. [Google Scholar] [CrossRef]
- Modi, D.; Correia, N.; Johnson, M.; Long, A.; Rudd, C.; Robitaille, F. Active control of the vacuum infusion process. Compos. Part A Appl. Sci. Manuf. 2007, 38, 1271–1287. [Google Scholar] [CrossRef]
- Acheson, J.A.; Simacek, P.; Advani, S.G. The implications of fiber compaction and saturation on fully coupled VARTM simulation. Compos. Part A Appl. Sci. Manuf. 2004, 35, 159–169. [Google Scholar] [CrossRef]
- Van Wyk, C.M. 20—Note on the compressibility of wool. J. Text. Inst. Trans. 1946, 37, T285–T292. [Google Scholar] [CrossRef]
- Chen, B.; Chou, T.-W. Compaction of woven-fabric preforms in liquid composite molding processes: Single-layer deformation. Compos. Sci. Technol. 1999, 59, 1519–1526. [Google Scholar] [CrossRef]
- Chen, B.; Cheng, A.H.-D.; Chou, T.-W. A nonlinear compaction model for fibrous preforms. Compos. Part A Appl. Sci. Manuf. 2001, 32, 701–707. [Google Scholar] [CrossRef]
- Gutowski, T.G.; Cai, Z.; Kingary, J.; Wineman, S.J. Rainflow/fibre deformation experiments. Composites 1987, 18, 263. [Google Scholar] [CrossRef]
- Sun, X.; Li, S.; Lee, L.J. Mold filling analysis in vacuum-assisted resin transfer molding. Part I: SCRIMP based on a high-permeable medium. Polym. Compos. 1998, 19, 807–817. [Google Scholar] [CrossRef]
- Ni, J.; Li, S.; Sun, X.; Lee, L.J. Mold filling analysis in vacuum-assisted resin transfer molding. Part II: SCRIMP based on grooves. Polym. Compos. 1998, 19, 818–829. [Google Scholar] [CrossRef]
- Han, K.; Jiang, S.; Zhang, C.; Wang, B. Flow modeling and simulation of SCRIMP for composites manufacturing. Compos. Part A Appl. Sci. Manuf. 2000, 31, 79–86. [Google Scholar] [CrossRef]
- Modi, D.; Johnson, M.; Long, A.; Rudd, C. Analysis of pressure profile and flow progression in the vacuum infusion process. Compos. Sci. Technol. 2009, 69, 1458–1464. [Google Scholar] [CrossRef]
- Yenilmez, B.; Senan, M.; Murat Sozer, E. Variation of part thickness and compaction pressure in vacuum infusion process. Compos. Sci. Technol. 2009, 69, 1710–1719. [Google Scholar] [CrossRef]
- Govignon, Q.; Bickerton, S.; Morris, J.; Kelly, P.A. Full field monitoring of the resin flow and laminate properties during the resin infusion process. Compos. Part A Appl. Sci. Manuf. 2008, 39, 1412–1426. [Google Scholar] [CrossRef]
- Song, Y.S.; Youn, J.R. Modeling of resin infusion in vacuum assisted resin transfer molding. Polym. Compos. 2008, 29, 390–395. [Google Scholar] [CrossRef]
- Lopatnikov, S.; Simacek, P.; GillespieJr, J.; Advani, S.G. A closed form solution to describe infusion of resin under vacuum in deformable fibrous porous media. Model. Simul. Mater. Sci. Eng. 2004, 12, S191–S204. [Google Scholar] [CrossRef]
- Park, C.H.; Saouab, A. Analytical modeling of composite molding by resin infusion with flexible tooling: VARI and RFI processes. J. Compos. Mater. 2009, 43, 1877–1900. [Google Scholar] [CrossRef]
- Hoa, S.V. Principles of the Manufacturing of Composite Materials, 1st ed.; Destech Publications, Inc.: Lancaster, PA, USA, 2009; ISBN 1932078266. [Google Scholar]
- Terzaghi, K. Theoretical Soil Mechanics, 1st ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 1943; ISBN 9780470172766. [Google Scholar]
- Kang, M.K.; Lee, W.I.; Hahn, H.T. Analysis of vacuum bag resin transfer molding process. Compos. Part A Appl. Sci. Manuf. 2001, 32, 1553–1560. [Google Scholar] [CrossRef]
- Li, J.; Zhang, C.; Liang, R.; Wang, B.; Walsh, S. Modeling and analysis of thickness gradient and variations in vacuum-assisted resin transfer molding process. Polym. Compos. 2008, 29, 473–482. [Google Scholar] [CrossRef]
- Robitaille, F.; Gauvin, R. Compaction of textile reinforcements for composites manufacturing. I: Review of experimental results. Polym. Compos. 1998, 19, 198–216. [Google Scholar] [CrossRef]
- Robitaille, F.; Gauvin, R. Compaction of textile reinforcements for composites manufacturing. II: Compaction and relaxation of dry and H2O-saturated woven reinforcements. Polym. Compos. 1998, 19, 543–557. [Google Scholar] [CrossRef]
- Robitaille, F.; Gauvin, R. Compaction of textile reinforcements for composites manufacturing. III: Reorganization of the fiber network. Polym. Compos. 1999, 20, 48–61. [Google Scholar] [CrossRef]
- Toll, S. Packing mechanics of fiber reinforcements. Polym. Eng. Sci. 1998, 38, 1337–1350. [Google Scholar] [CrossRef]
- Lundström, T.S.; Toll, S.; Håkanson, J.M. Measurement of the permeability tensor of compressed fibre beds. Transp. Porous Media 2002, 47, 363–380. [Google Scholar] [CrossRef]
- Kozeny, J. Ueber kapillare Leitung des Wassers im Boden. Sitzungsber Akad. Wiss. Wien 1927, 136, 271–306. [Google Scholar]
- Carman, P.C. Fluid flow through granular beds. Chem. Eng. Res. Des. 1997, 75, S32–S48. [Google Scholar] [CrossRef]
- Gebart, B.R. Permeability of unidirectional reinforcements for RTM. J. Compos. Mater. 1992, 26, 1100–1133. [Google Scholar] [CrossRef]
- Kim, Y.R.; McCarthy, S.P.; Fanucci, J.P. Compressibility and relaxation of fiber reinforcements during composite processing. Polym. Compos. 1991, 12, 13–19. [Google Scholar] [CrossRef]
- Grimsley, B.W. Characterization of the Vacuum Assisted Resin Transfer Molding Process for Fabrication of Aerospace Composites. Ph.D. Thesis, Virginia State University, Petersburg, VA, USA, 2005. [Google Scholar]
- Lundström, T.S.; Gebart, B.R.; Sandlund, E. In-plane permeability measurements on fiber reinforcements by the multi-cavity parallel flow technique. Polym. Compos. 1999, 20, 146–154. [Google Scholar] [CrossRef]
- Simacek, P.; Eksik, Ö.; Heider, D.; Gillespie, J.W.; Advani, S. Experimental validation of post-filling flow in vacuum assisted resin transfer molding processes. Compos. Part A Appl. Sci. Manuf. 2012, 43, 370–380. [Google Scholar] [CrossRef]
- Fink, B.K.; Hsiao, K.-T.; Mathur, R.; Gillespie, J.W.J.; Advani, S.G. An Analytical Vacuum-Assisted Resin Transfer Molding (VARTM) Flow Model; U.S. Army Research Laboratory: Adelphi, MD, USA, 2000; pp. 1–65. [Google Scholar]
- Hsiao, K.-T.; Mathur, R.; Advani, S.G.; Gillespie, J.W.; Fink, B.K. A closed form solution for flow during the vacuum assisted resin transfer molding process. J. Manuf. Sci. Eng. 2000, 122, 463. [Google Scholar] [CrossRef]





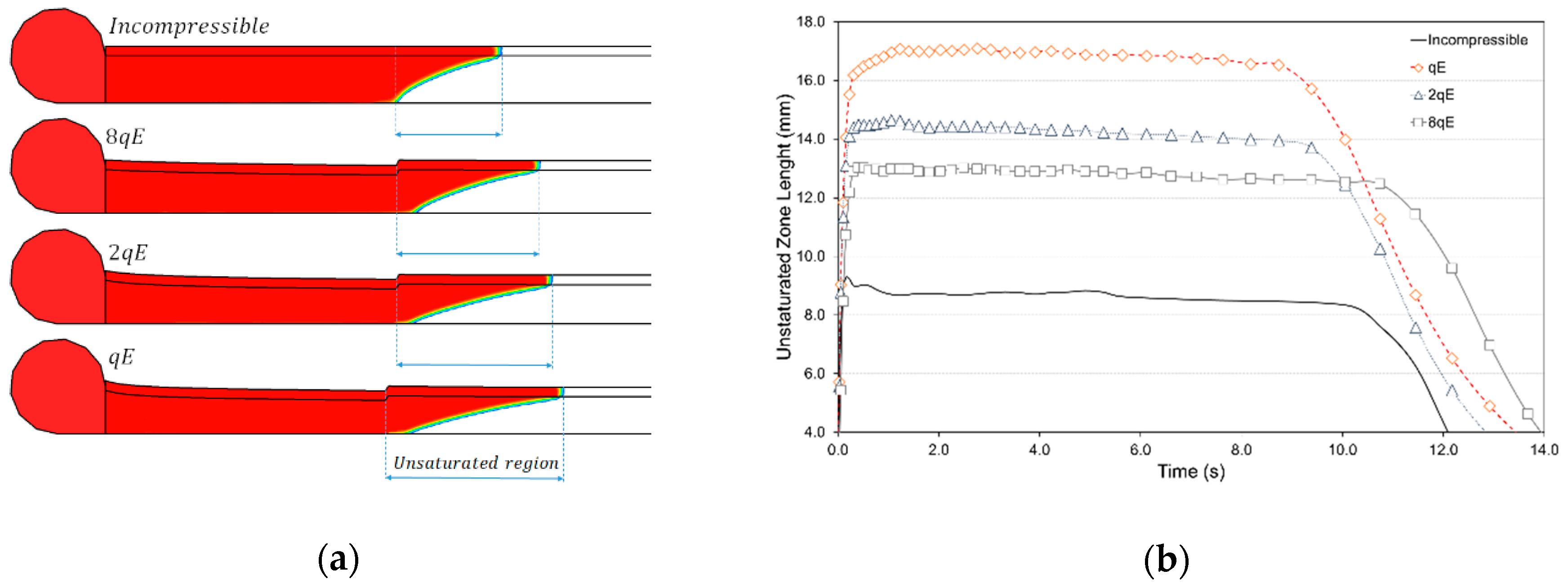
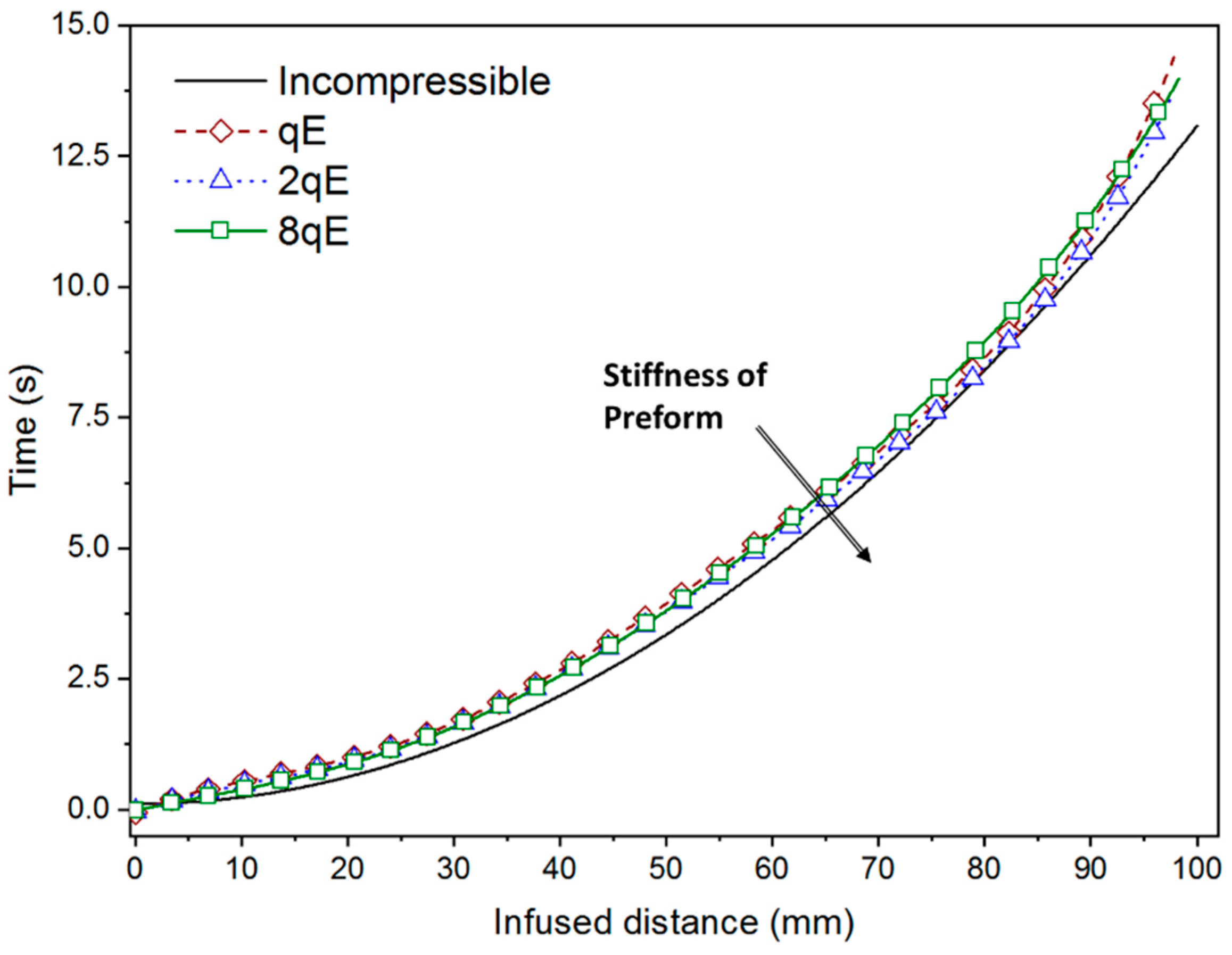
| Parameter | Unit | Test 1 | Test 2 | Test 3 | Test 4 |
|---|---|---|---|---|---|
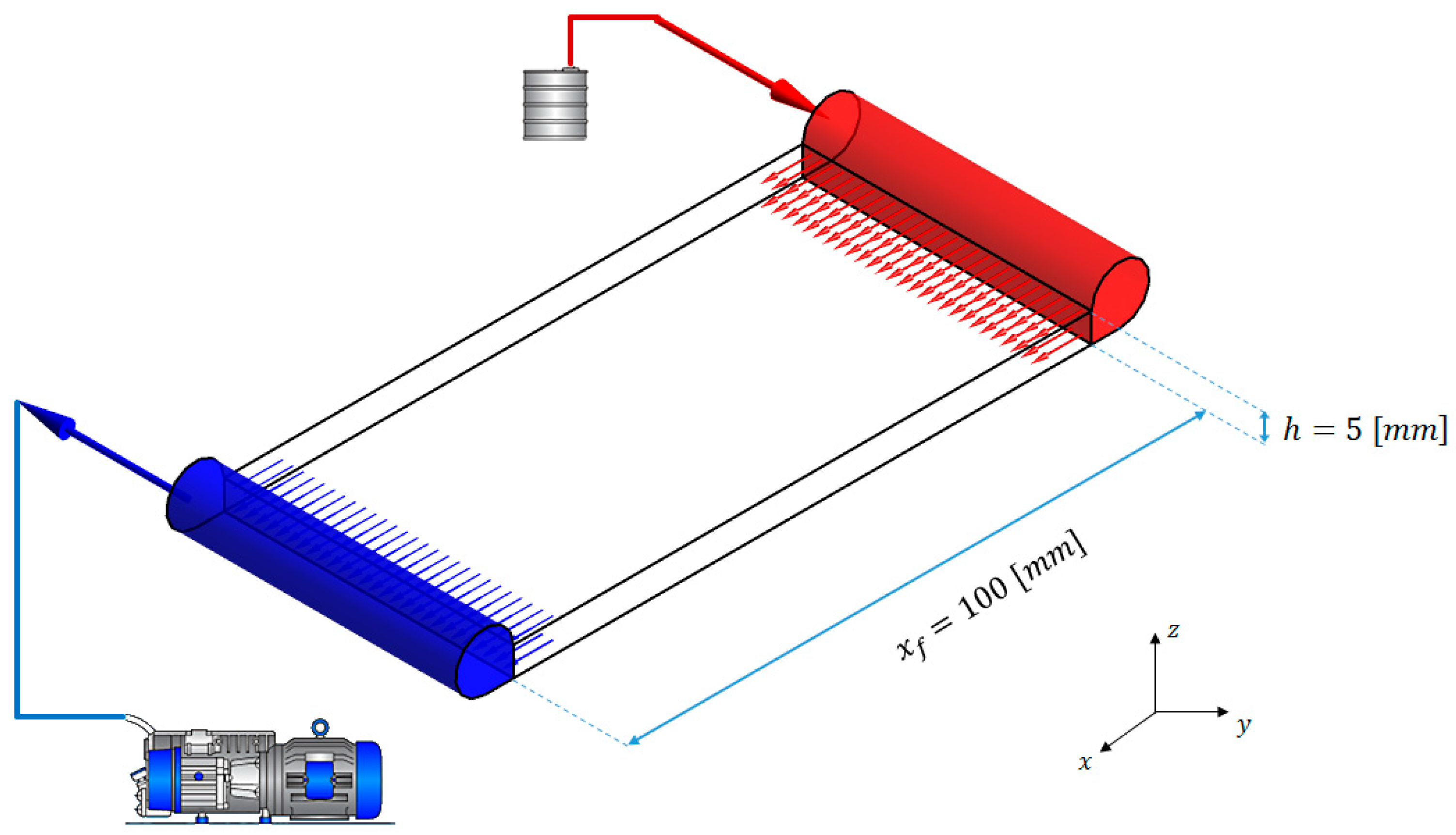
| xf | (mm) | 100 | |||
| H0 | (mm) | 5 | |||
| hDM | (mm) | - | 0.5 | ||
| Vf0 | - | 0.40 | |||
| Vf0H | - | 0.46 | 0.46 | ||
| VfDM | - | - | 0.01 | ||
| c1 | (m2) | 7.41e−11 | |||
| c2 | (m2) | 9.06e−10 | |||
| KDM | (m2) | - | 2.5e−9 | ||
| Pa | (Pa) | 101325 | |||
| Pv | (Pa) | 5000 | |||
| µ | (Pa s) | 0.1 | |||
| qE | (MPa) | ∞ | 289 | ∞ | 289 |
| ndry | - | - | 11.85 | - | 11.85 |
| nwet | - | - | 15.45 | - | 15.45 |
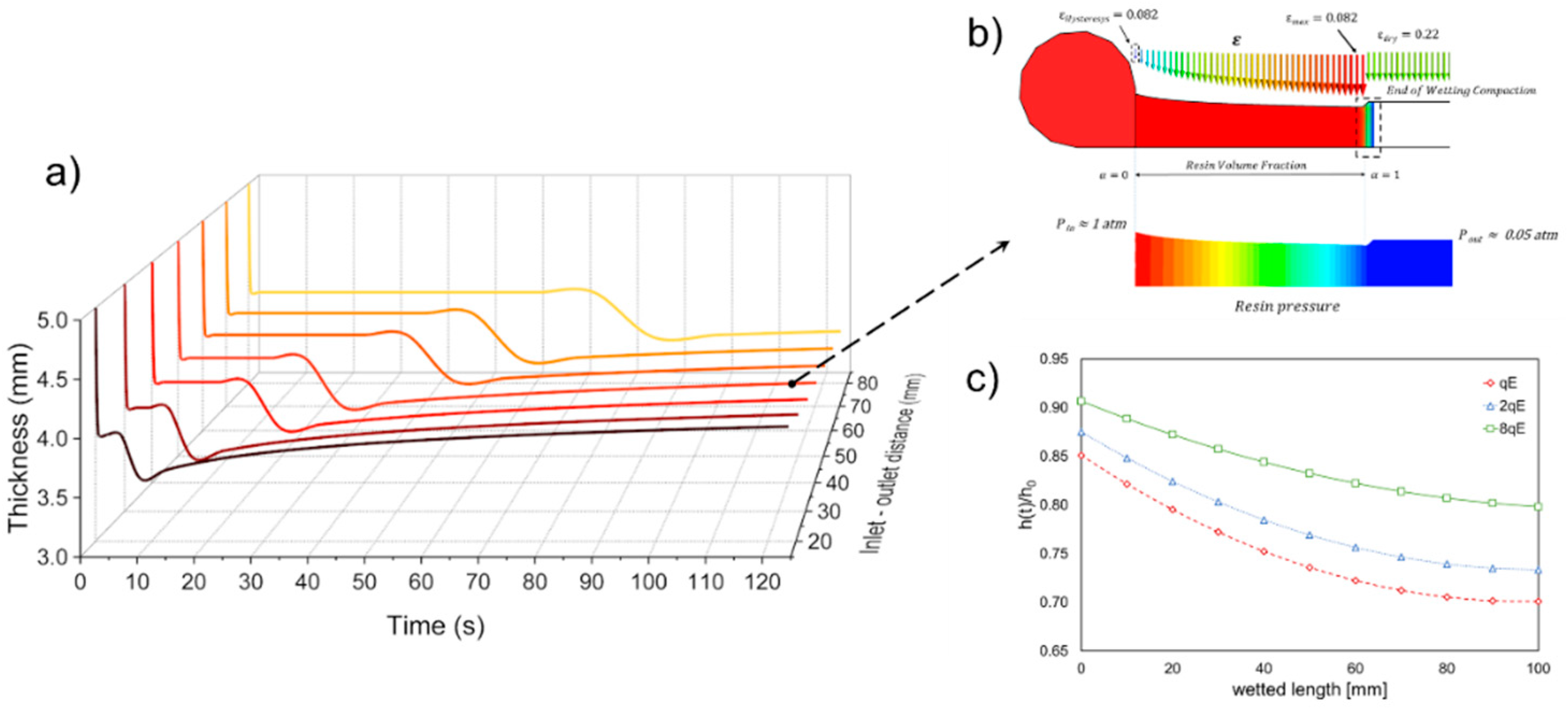
| Thickness/Deformation Values | qE | 2qE | 8qE |
|---|---|---|---|
| Initial thickness (mm) | 5 | 5 | 5 |
| Dry compaction thickness (mm) | 3.91 | 4.13 | 4.54 |
| εdry (-) | 0.21 | 0.18 | 0.09 |
| Wetting compaction (mm) | 3.4 | 3.6 | 3.95 |
| εwet (-) | 0.32 | 0.28 | 0.21 |
| Final thickness (mm) 1 | 4.25 | 4.37 | 4.53 |
| εhysteresis (-) | 0.15 | 0.13 | 0.094 |
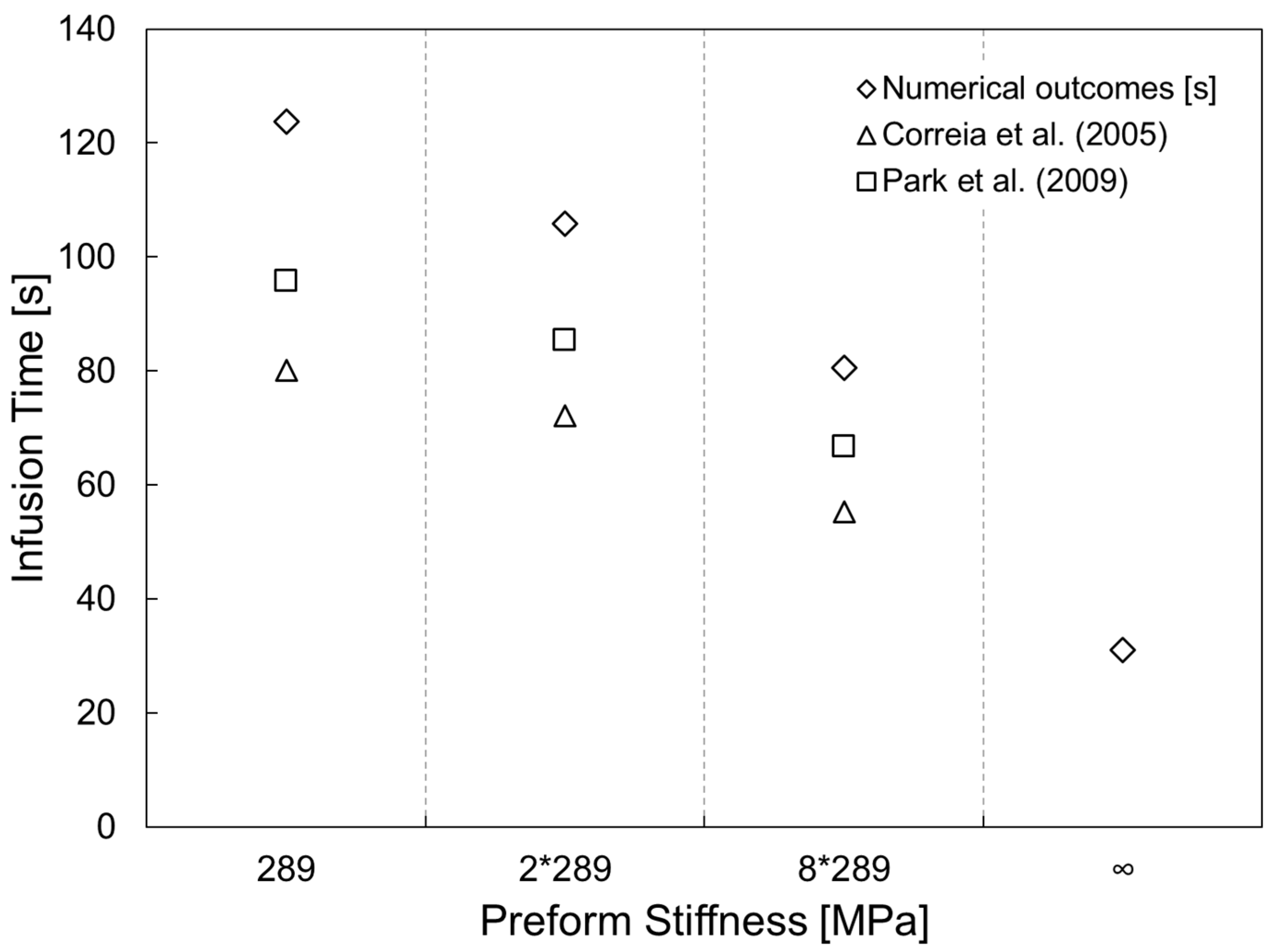
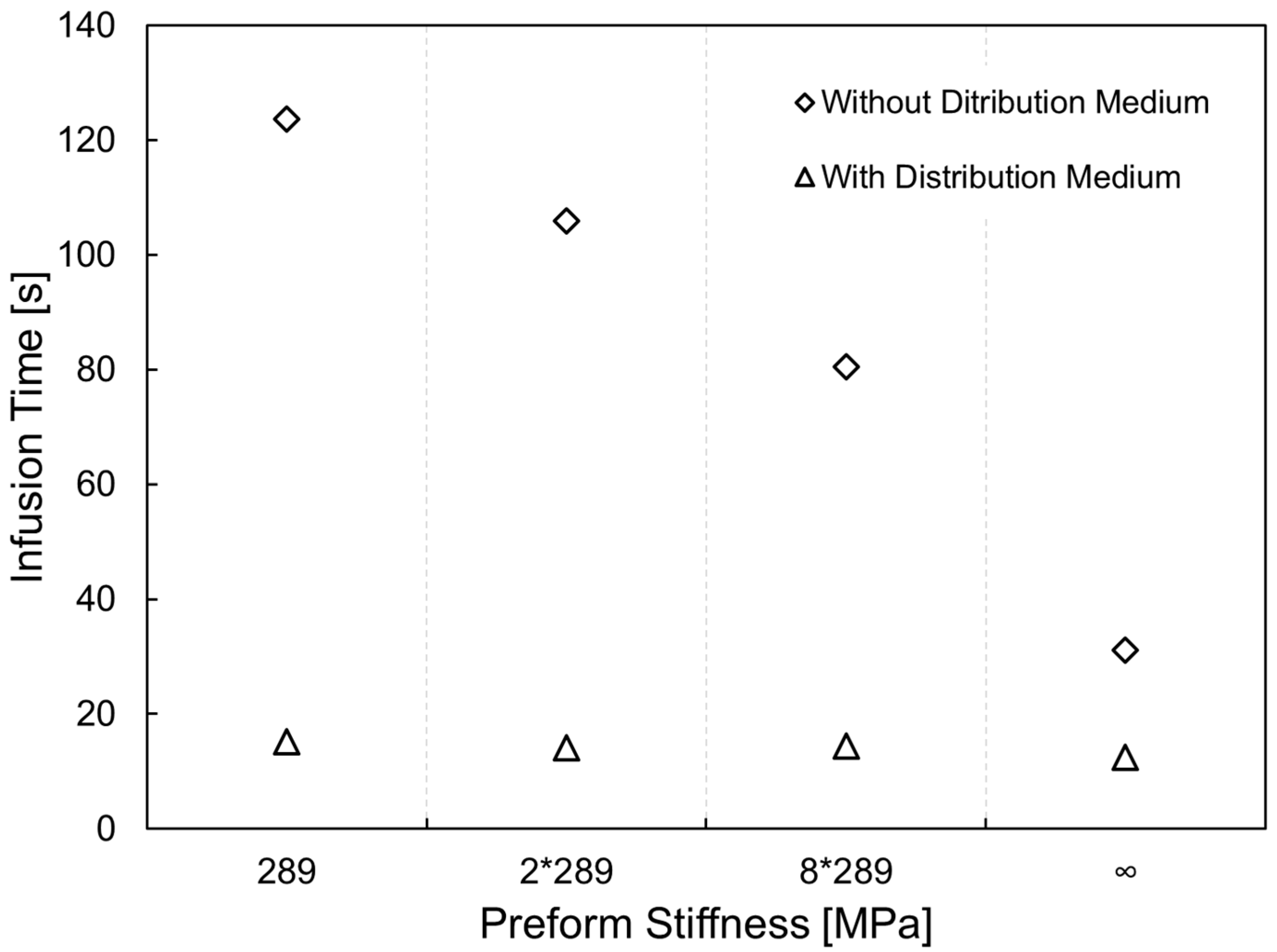
| Numerical Infusion Time (s) | qE (MPa) | Δt Compared to Incompressible Preform (%) | Δt between without DM and with DM | |
|---|---|---|---|---|
| (s) | (%) | |||
| 15.1 | 289 | 21.7 | 108.6 | 87.8 |
| 14.1 | 2 × 289 | 13.7 | 91.8 | 86.7 |
| 14.4 | 8 × 289 | 16.1 | 66.1 | 82.1 |
| 12.4 | ∞ | - | 18.7 | 60.1 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rubino, F.; Carlone, P. A Semi-Analytical Model to Predict Infusion Time and Reinforcement Thickness in VARTM and SCRIMP Processes. Polymers 2019, 11, 20. https://doi.org/10.3390/polym11010020
Rubino F, Carlone P. A Semi-Analytical Model to Predict Infusion Time and Reinforcement Thickness in VARTM and SCRIMP Processes. Polymers. 2019; 11(1):20. https://doi.org/10.3390/polym11010020
Chicago/Turabian StyleRubino, Felice, and Pierpaolo Carlone. 2019. "A Semi-Analytical Model to Predict Infusion Time and Reinforcement Thickness in VARTM and SCRIMP Processes" Polymers 11, no. 1: 20. https://doi.org/10.3390/polym11010020
APA StyleRubino, F., & Carlone, P. (2019). A Semi-Analytical Model to Predict Infusion Time and Reinforcement Thickness in VARTM and SCRIMP Processes. Polymers, 11(1), 20. https://doi.org/10.3390/polym11010020







