Statistical Approach to Diffraction of Periodic and Non-Periodic Crystals—Review
Abstract
:1. Introduction
2. The Average Unit Cell (AUC) Concept and the Statistical Method
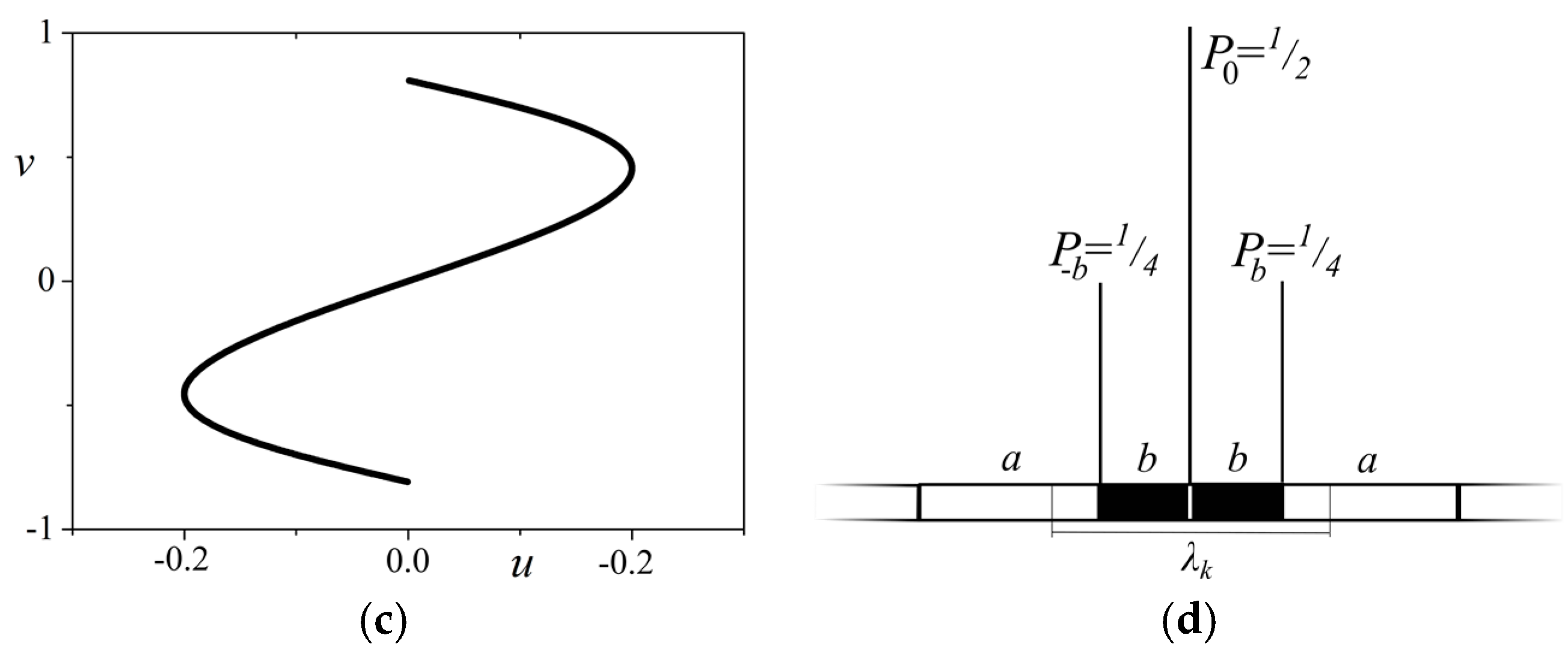
2.1. AUC for Periodic Structures
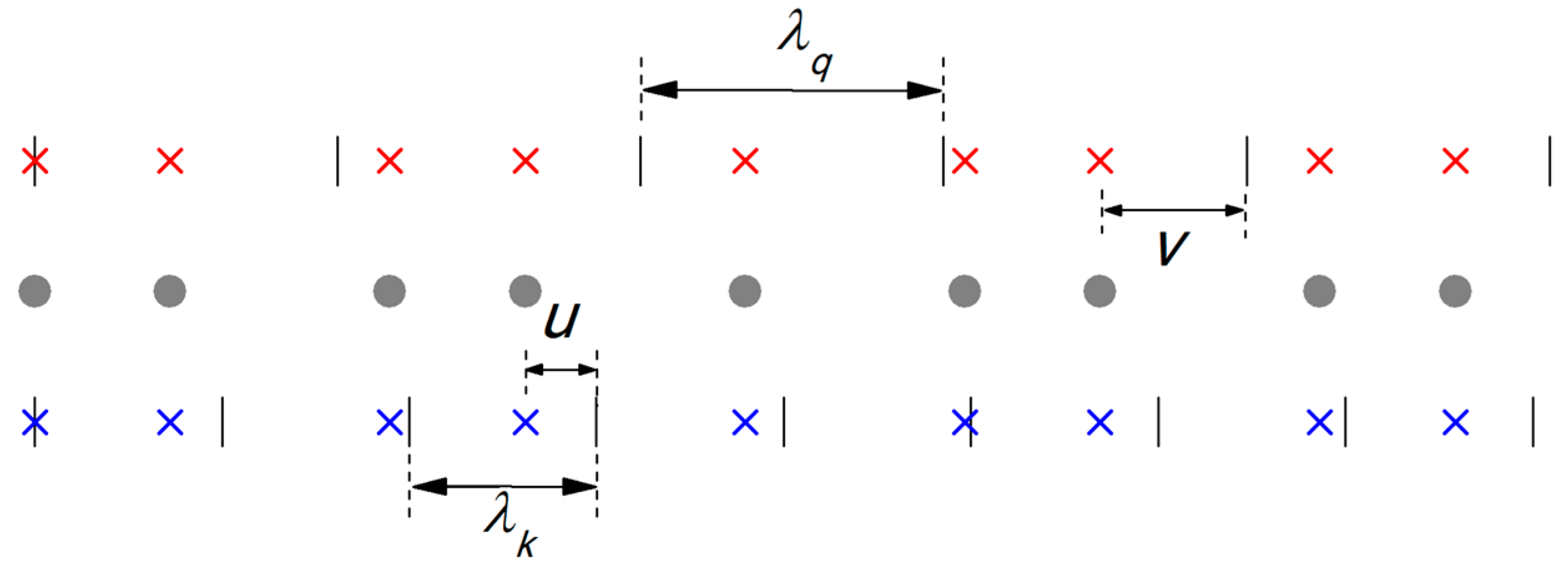
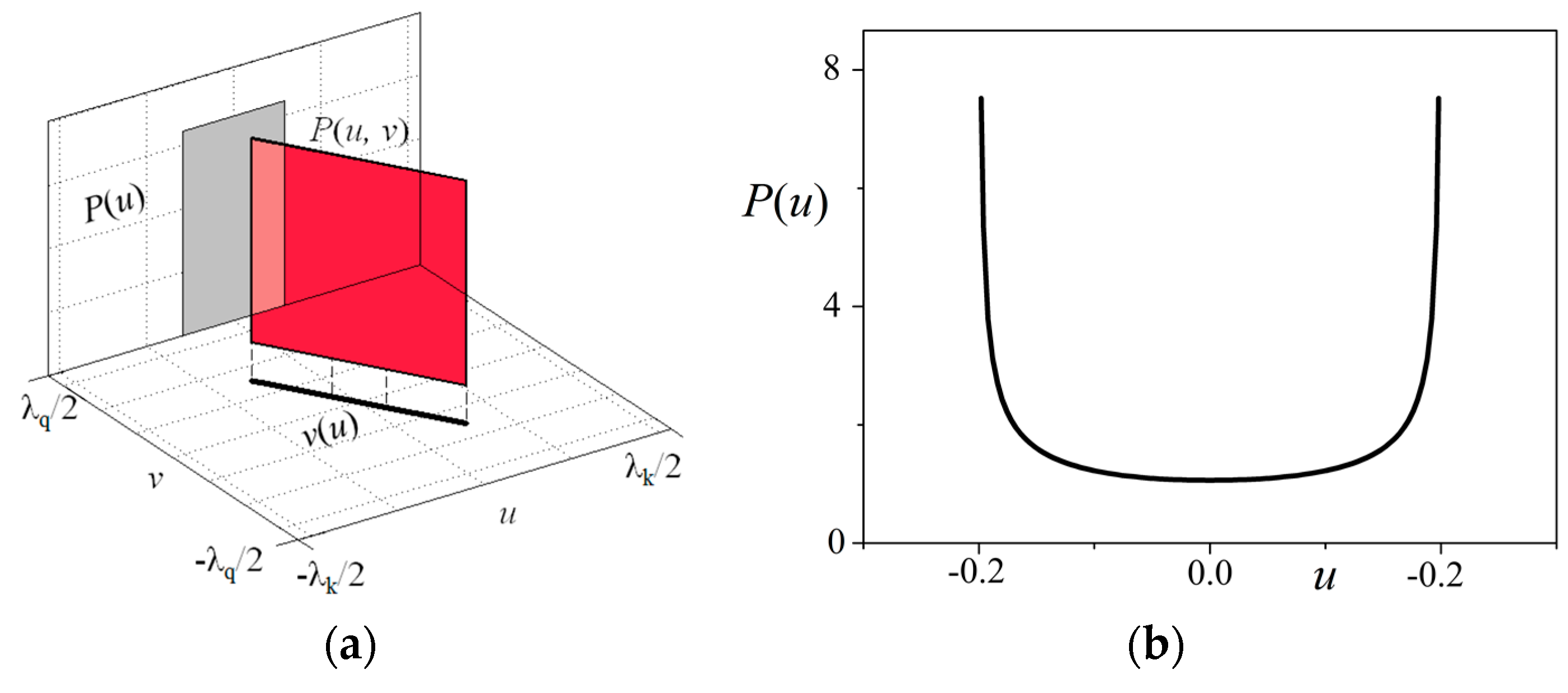
2.2. AUC for Quasicrystals
2.3. AUC for Harmonically Modulated Structure
2.4. AUC for Thue-Morse Sequence
3. Structure Factor Derivation
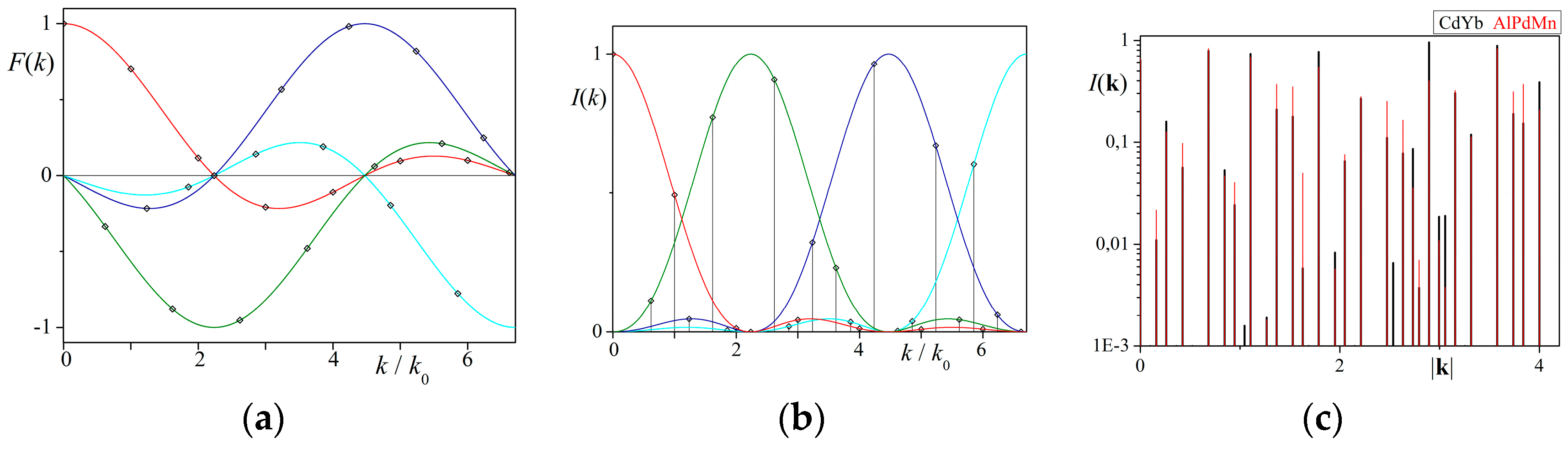
3.1. Structure Factor for Quasicrystals
3.2. Structure Factor for Modulated Structures
3.3. Structure Factor for Thue-Morse Sequence
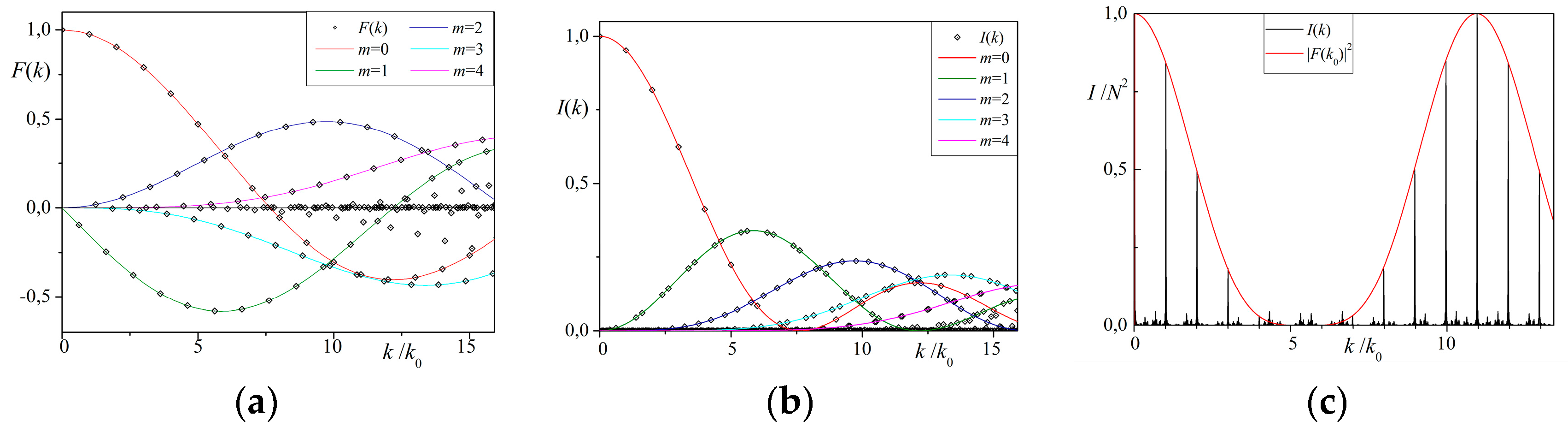
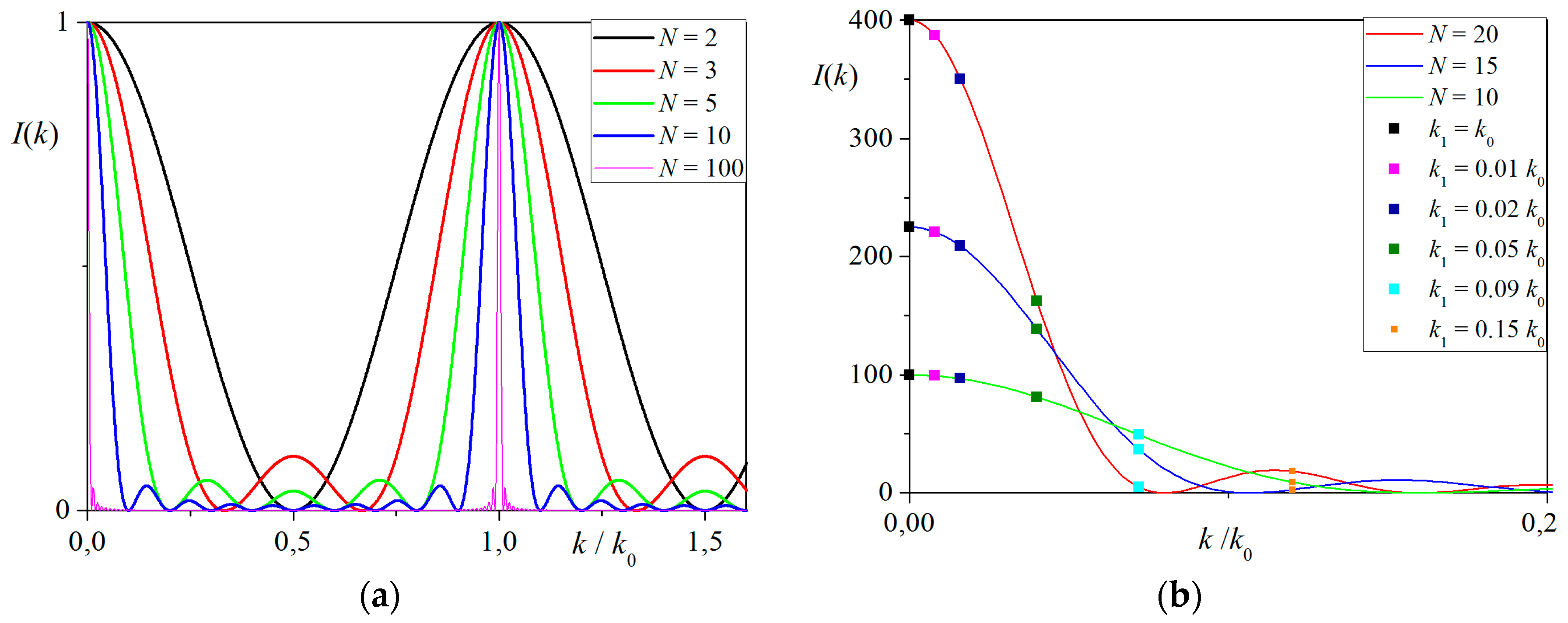
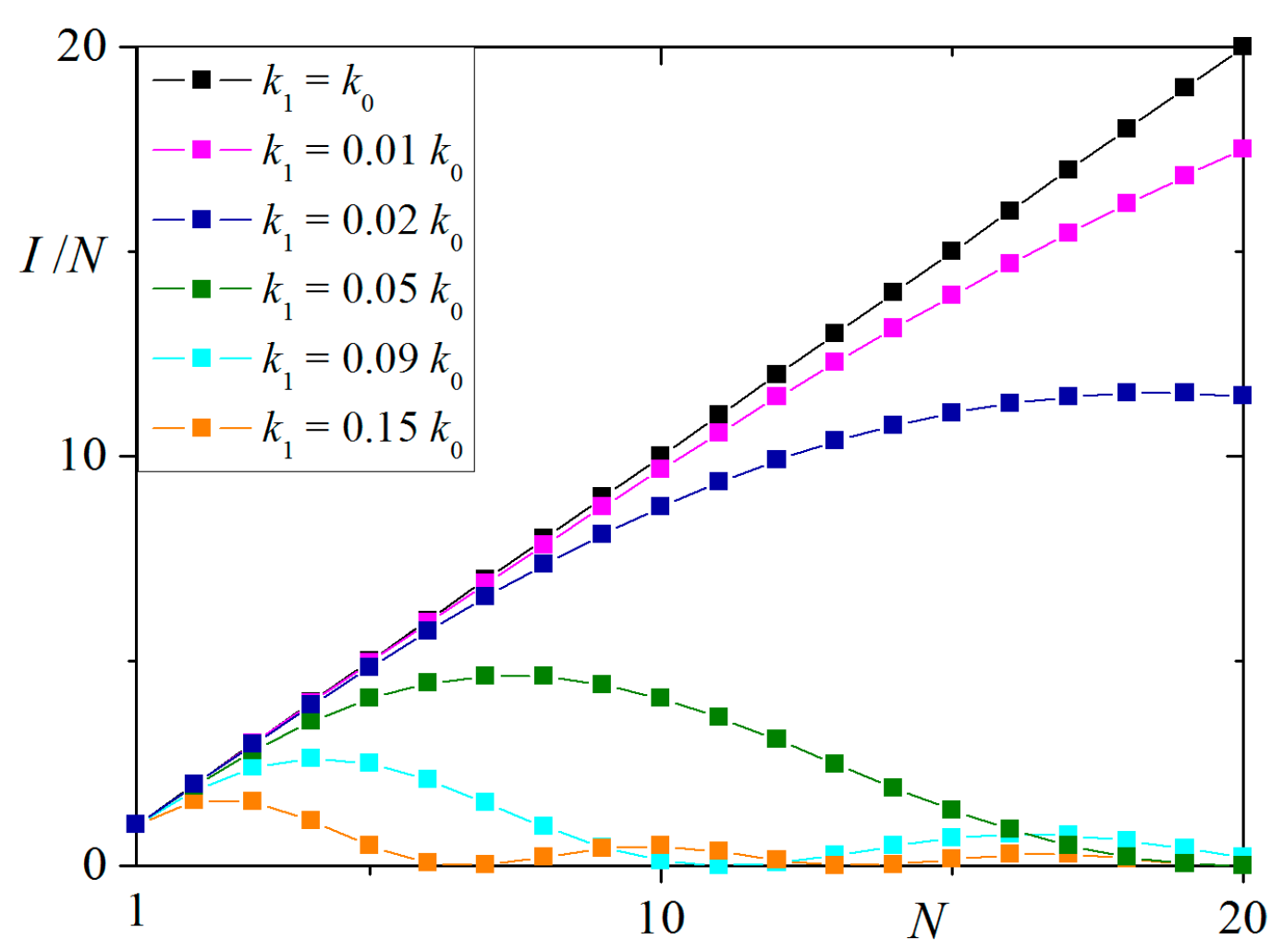
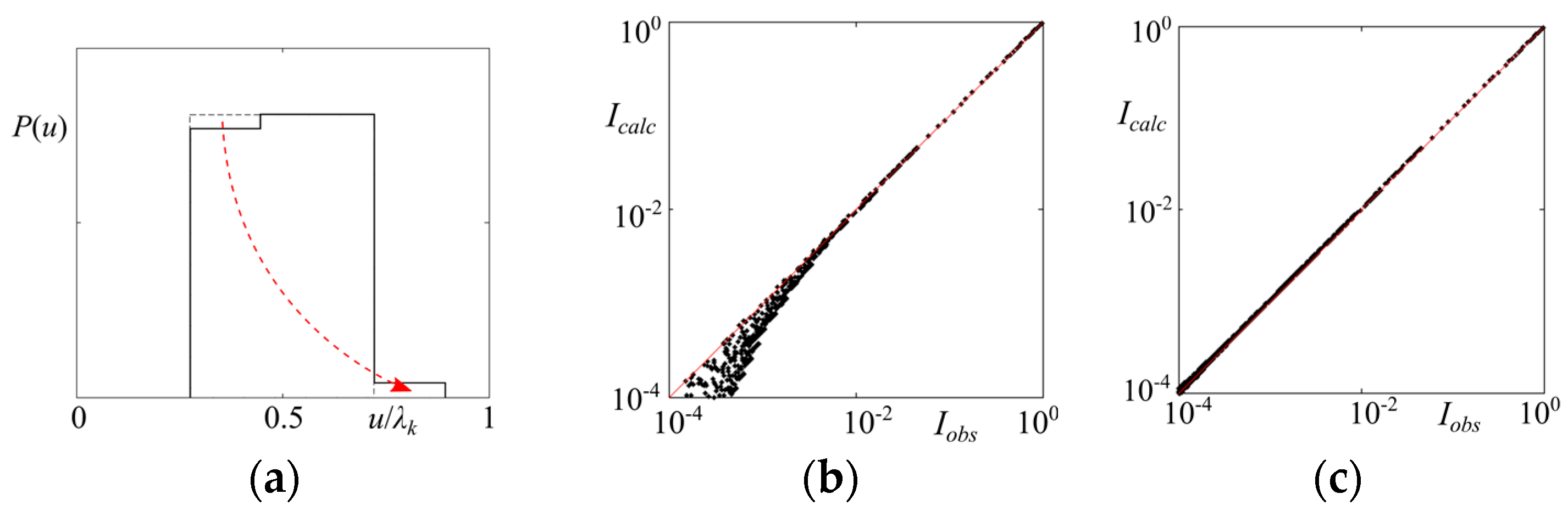
4. AUC-Based Analysis of a Peak Profile
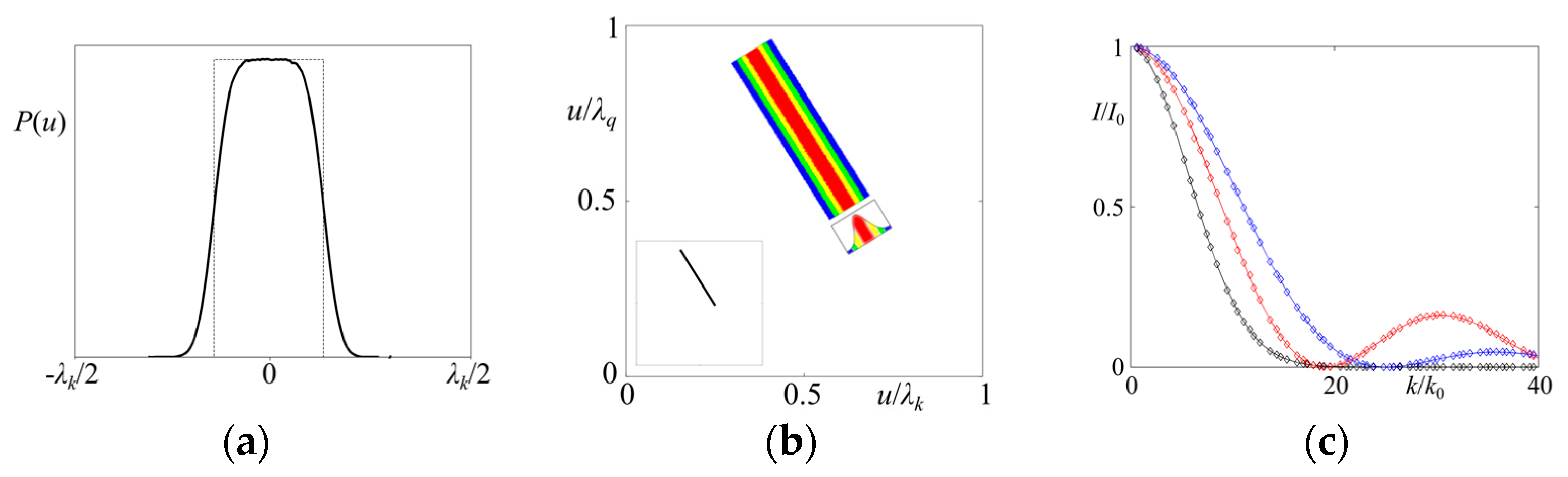
5. Structure Disorder in Aperiodic Crystals
5.1. Phonons
5.2. Phasons
6. Statistical Approach and Superspace Method
7. Summary
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Shechtman, D.S.; Blech, I.; Gratias, D.; Cahn, J.W. Metallic Phase with Long-Range Orientational Order and No Translational Symmetry. Phys Rev. Lett. 1984, 53, 1951–1954. [Google Scholar] [CrossRef]
- Levine, D.; Steinhardt, P.J. Quasicrystals: A New Class of Ordered Structures. Phys. Rev. Lett. 1984, 53, 2477–2480. [Google Scholar] [CrossRef]
- Baake, M.; Grimm, U. Mathematical diffraction of aperiodic structures. Chem. Soc. Rev. 2012, 41, 6821–6843. [Google Scholar] [CrossRef] [PubMed]
- Baake, M.; Grimm, U. Aperiodic Order; Cambridge University Press: Cambridge, UK, 2013. [Google Scholar]
- Kalugin, P.A.; Kitaev, A.Y.; Levitov, L.S. 6-dimensional properties of Al(86)Mn(14) alloy. J. Phys. Lett. 1985, 46, L601–L607. [Google Scholar] [CrossRef]
- Duneau, M.; Katz, A. Quasiperiodic patterns. Phys. Rev. Lett. 1985, 54, 2688–2691. [Google Scholar] [CrossRef] [PubMed]
- Elser, V. The diffraction pattern of projected structures. Acta Cryst. A 1986, 42, 36–43. [Google Scholar] [CrossRef]
- Yamamoto, A. Crystallography of Quasiperiodic Crystals. Acta Cryst. A 1996, 52, 509–560. [Google Scholar] [CrossRef]
- Janssen, T.; Chapuis, G.; de Boissieu, M. Aperiodic Crystals: From Modulated Phases to Quasicrystals; Oxford University Press: Oxford, UK, 2007. [Google Scholar]
- Steurer, W.; Deloudi, S. Crystallography of Quasicrystals; Springer: Berlin, Germany, 2009. [Google Scholar]
- Takakura, H.; Yamamoto, A.; Tsai, A.-P. The structure of a decagonal Al72Ni20Co8 quasicrystal. Acta Cryst. A 2001, 57, 576–585. [Google Scholar] [CrossRef]
- Cervellino, A.; Haibach, T.; Steurer, W. Structure solution of the basic decagonal Al-Co-Ni phase by the atomic surfaces modelling method. Acta Cryst. B 2002, 58, 8–33. [Google Scholar] [CrossRef]
- Takakura, H.; Gomez, C.P.; Yamamoto, A.; de Boissieu, M. Atomic structure of the binary icosahedral Yb-Cd quasicrystal. Nat. Mater. 2007, 6, 58–63. [Google Scholar] [CrossRef]
- Wolny, J. The reference lattice concept and its application to the analysis of diffraction patterns. Phil. Mag. A 1998, 77, 395–412. [Google Scholar] [CrossRef]
- Wolny, J.; Kozakowski, B.; Kuczera, P.; Strzalka, R.; Wnek, A. Real Space Structure Factor for Different Quasicrystals. Isr. J. Chem. 2011, 51, 1275–1291. [Google Scholar] [CrossRef]
- Wolny, J. Average Unit Cell of the Fibonacci Chain. Acta Cryst. A 1998, 54, 1014–1018. [Google Scholar] [CrossRef]
- Senechal, M. Quasicrystals and Geometry; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Wolny, J.; Kozakowski, B.; Kuczera, P.; Pytlik, L.; Strzalka, R. Real Space Structure Factor and Scaling for Quasicrystals. In Aperiodic Crystals; Schmied, S., Lifshitz, R., Withers, R.L., Eds.; Springer: Berlin, Germany, 2013; pp. 211–218. [Google Scholar]
- Wolny, J.; Kozakowski, B.; Kuczera, P.; Pytlik, L.; Strzalka, R. What periodicities can be found in diffraction patterns of quasicrystals? Acta Cryst. A 2014, 70, 181–185. [Google Scholar] [CrossRef] [PubMed]
- Wolny, J.; Kozakowski, B.; Repetowicz, P. Construction of average unit cell for Penrose tiling. J. Alloy. Comp. 2002, 342, 198–202. [Google Scholar] [CrossRef]
- Kozakowski, B.; Wolny, J. Structure factor for decorated Penrose tiling. Acta Cryst. A 2010, 66, 489–498. [Google Scholar] [CrossRef] [PubMed]
- Chodyn, M.; Kuczera, P.; Wolny, J. Generalized Penrose tiling as a quasilattice for decagonal quasicrystal structure analysis. Acta Cryst. A 2015, 71, 161–168. [Google Scholar] [CrossRef] [PubMed]
- Strzalka, R.; Buganski, I.; Wolny, J. Structure factor for an icosahedral quasicrystal within a statistical approach. Acta Cryst. A 2015, 71, 279–290. [Google Scholar] [CrossRef] [PubMed]
- Strzalka, R.; Wolny, J.; Kuczera, P. The Choice of Vector Basis for Ammann Tiling in a Context of the Average Unit Cell. In Aperiodic Crystals; Schmied, S., Lifshitz, R., Withers, R.L., Eds.; Springer: Berlin, Germany, 2013; pp. 203–210. [Google Scholar]
- Wolny, J.; Kuczera, P.; Strzalka, R. Periodically distributed objects with quasicrystalline diffraction pattern. Appl. Phys. Lett. 2015, 106, 131905. [Google Scholar] [CrossRef]
- Urban, G.; Wolny, J. Diffraction analysis of sinusoidal modulated structures in average unit cell approach. Ferroelectrics 2001, 250, 131–134. [Google Scholar] [CrossRef]
- Wolny, J.; Buganski, I.; Strzalka, R. Diffraction pattern of modulated structures described by Bessel functions. Phil. Mag. 2016, 96, 1344–1359. [Google Scholar] [CrossRef]
- Miekisz, J. Quasicrystals: Microscopic Models on Nonperiodic Structures; Leuven University Press: Leuven, Belgium, 1993. [Google Scholar]
- Baake, M.; Grimm, U.; Nilsson, J. Scaling of the Thue-Morse diffraction measure. Acta Phys. Pol. A 2014, 126, 431–434. [Google Scholar] [CrossRef]
- Wolny, J.; Wnek, A.; Verger-Gaugry, J.L. Fractal behaviour of diffraction pattern of Thue-Morse sequence. J. Comput. Phys. 2000, 163, 313–327. [Google Scholar] [CrossRef]
- Buczek, P.; Sadun, L.; Wolny, J. Periodic diffraction patterns for 1D quasicrystals. Acta Phys. Pol. B 2005, 36, 919–933. [Google Scholar]
- Kozakowski, B.; Wolny, J. Average Unit Cell in Fourier Space and Its Application to Decagonal Quasicrystals. In Aperiodic Crystals; Schmied, S., Lifshitz, R., Withers, R.L., Eds.; Springer: Berlin, Germany, 2013; pp. 125–132. [Google Scholar]
- Kuczera, P.; Kozakowski, B.; Wolny, J.; Steurer, W. Real space structure refinement of the basic Ni-rich decagonal Al-Ni-Co phase. J. Phys. Conf. Ser. 2010, 226, 012001. [Google Scholar] [CrossRef]
- Kuczera, P.; Wolny, J.; Fleischer, F.; Steurer, W. Structure refinement of decagonal Al-Ni-Co, superstructure type I. Philos. Mag. 2011, 91, 2500–2509. [Google Scholar] [CrossRef]
- Kuczera, P.; Wolny, J.; Steurer, W. Comparative structural study of decagonal quasicrystals in the systems Al–Cu–Me (Me = Co, Rh, Ir). Acta Cryst. B 2012, 68, 578–589. [Google Scholar] [CrossRef] [PubMed]
- Strzalka, R.; Buganski, I.; Wolny, J. Simple decoration model of icosahedral quasicrystals in statistical approach. Acta Phys. Pol A 2016, 130. (In Print) [Google Scholar]
- Levine, D.; Steinhardt, P.J. Quasicrystals. I. Definition and structure. Phys Rev. B 1986, 34, 596–616. [Google Scholar] [CrossRef]
- Urban, G.; Wolny, J. From harmonically modulated structures to quasicrystals. Phil. Mag. 2006, 86, 629–635. [Google Scholar] [CrossRef]
- Van Aalst, W.; den Hollander, J.; Peterse, W.J.A.M.; de Wolff, P.M. The modulated structure of γ -Na2CO3 in a harmonic approximation. Acta Cryst. B 1976, 32, 47–58. [Google Scholar] [CrossRef]
- Petricek, V.; Coppens, P.; Becker, P. Structure analysis of displacively modulated molecular crystals. Acta Cryst. A 1985, 41, 478–483. [Google Scholar] [CrossRef]
- Paciorek, W.A.; Kucharczyk, D. Structure-factor calculations in refinement of a modulated crystal structure. Acta Cryst. A 1985, 41, 462–466. [Google Scholar] [CrossRef]
- Monshi, A.; Foroughi, M.R.; Monshi, M.R. Modified Scherrer equation to estimate more accurately nano-crystallite size using XRD. WJNSE 2012, 2, 154–160. [Google Scholar] [CrossRef]
- Kubena, J. The effect of X-ray coherence on crystalline diffraction. Czech. J. Phys. 1968, 18, 777–783. [Google Scholar] [CrossRef]
- Kubena, J. X-ray diffraction on a three-dimensional lattice with account taken of the coherence of X-rays. Czech. J. Phys. 1968, 18, 1233–1243. [Google Scholar] [CrossRef]
- Scardi, P.; Leoni, M. Whole powder pattern modelling. Acta Cryst. A 2002, 58, 190–200. [Google Scholar] [CrossRef]
- Wolny, J. Use of the Debye-Waller approximation in diffraction-pattern calculation. Acta Cryst. A 1992, 48, 918–921. [Google Scholar] [CrossRef]
- Wolny, J. Static fluctuation distributions and their influence on diffraction patterns. J. Phys. Condens. Matter 1993, 5, 6663–6672. [Google Scholar] [CrossRef]
- Buganski, I.; Strzalka, R.; Wolny, J. The estimation of phason flips in 1D quasicrystal from the diffraction pattern. Phys. Status Solidi B 2016, 253, 450–457. [Google Scholar] [CrossRef]
- Wolny, J.; Buganski, I.; Kuczera, P.; Strzalka, R. Pushing the limits of crystallography. J. Appl Crystallogr. 2016. submitted. [Google Scholar]
- De Boissieu, M. Phonons and Phasons in Icosahedral Quasicrystal. Isr. J. Chem. 2011, 51, 1292–1303. [Google Scholar] [CrossRef]
- De Boissieu, M. Phonons, phasons and atomic dynamics in quasicrystals. Chem. Soc. Rev. 2012, 41, 6778–6786. [Google Scholar] [CrossRef] [PubMed]
- Kuczera, P.; Wolny, J.; Steurer, W. High-temperature structural study of decagonal Al-Cu-Rh. Acta Cryst. B 2014, 70, 306–315. [Google Scholar] [CrossRef] [PubMed]
- Ors, T.; Takakura, H.; Abe, E.; Steurer, W. The quasiperiodic average structure of highly disordered decagonal Zn-Mg-Dy and its temperature dependence. Acta Cryst. B 2014, 70, 315–330. [Google Scholar] [CrossRef] [PubMed]
- Yamada, T.; Takakura, H.; Euchner, H.; Gomez, C.P.; Bosak, A.; Fertey, P.; de Boissieu, M. Atomic structure and phason modes of the Sc-Zn icosahedral quasicrystal. IUCrJ 2016, 3, 247–258. [Google Scholar] [CrossRef] [PubMed]
- Bancel, P.A. Dynamical Phasons in a Perfect Quasicrystal. Phys. Rev. Lett. 1989, 63, 2741. [Google Scholar] [CrossRef] [PubMed]
- Socolar, J.E.S.; Lubensky, T.C.; Steinhardt, P.J. Phonons, phasons, and dislocations in quasicrystals. Phys. Rev. B 1986, 34, 3345–3360. [Google Scholar] [CrossRef]
- De Boissieu, M. Stability of quasicrystals: Energy, entropy and phason modes. Phil. Mag. 2006, 86, 1115–1122. [Google Scholar] [CrossRef]
- Lubensky, T.C.; Ramaswamy, S.; Toner, J. Hydrodynamics of icosahedral quasicrystals. Phys. Rev. B 1985, 32, 7444–7452. [Google Scholar] [CrossRef]
- Lubensky, T.C.; Socolar, E.S.; Steinhardt, P.J.; Bancel, P.A.; Hainey, P.A. Distortion and Peak Broadening in Quasicrystal Diffraction Patterns. Phys. Rev. Lett. 1986, 57, 1440–1443. [Google Scholar] [CrossRef] [PubMed]
- De Wolff, P.M.; Janssen, T.; Janner, A. The superspace groups for incommensurate crystal structures with a one-dimensional modulation. Acta Cryst. A 1981, 37, 625–636. [Google Scholar] [CrossRef]
- Orzechowski, D.; Wolny, J. Influence of short-range order on diffraction patterns. Phil. Mag. 2007, 87, 3049–3054. [Google Scholar] [CrossRef]

© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Strzalka, R.; Buganski, I.; Wolny, J. Statistical Approach to Diffraction of Periodic and Non-Periodic Crystals—Review. Crystals 2016, 6, 104. https://doi.org/10.3390/cryst6090104
Strzalka R, Buganski I, Wolny J. Statistical Approach to Diffraction of Periodic and Non-Periodic Crystals—Review. Crystals. 2016; 6(9):104. https://doi.org/10.3390/cryst6090104
Chicago/Turabian StyleStrzalka, Radoslaw, Ireneusz Buganski, and Janusz Wolny. 2016. "Statistical Approach to Diffraction of Periodic and Non-Periodic Crystals—Review" Crystals 6, no. 9: 104. https://doi.org/10.3390/cryst6090104
APA StyleStrzalka, R., Buganski, I., & Wolny, J. (2016). Statistical Approach to Diffraction of Periodic and Non-Periodic Crystals—Review. Crystals, 6(9), 104. https://doi.org/10.3390/cryst6090104




